Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bierkens, Joris
Grazzi, Sebastiano
van der Meulen, Frank
and
Schauer, Moritz
2021.
A piecewise deterministic Monte Carlo method for diffusion bridges.
Statistics and Computing,
Vol. 31,
Issue. 3,
Mider, Marcin
Schauer, Moritz
and
van der Meulen, Frank
2021.
Continuous-discrete smoothing of diffusions.
Electronic Journal of Statistics,
Vol. 15,
Issue. 2,
Beskos, Alexandros
Crisan, Dan
Jasra, Ajay
Kantas, Nikolas
and
Ruzayqat, Hamza
2021.
Score-Based Parameter Estimation for a Class of Continuous-Time State Space Models.
SIAM Journal on Scientific Computing,
Vol. 43,
Issue. 4,
p.
A2555.
Clairon, Quentin
and
Samson, Adeline
2022.
Optimal control for parameter estimation in partially observed hypoelliptic stochastic differential equations.
Computational Statistics,
Vol. 37,
Issue. 5,
p.
2471.
Chada, Neil K.
Jasra, Ajay
and
Yu, Fangyuan
2022.
Unbiased estimation of the Hessian for partially observed diffusions.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 478,
Issue. 2262,
Arnaudon, Alexis
van der Meulen, Frank
Schauer, Moritz
and
Sommer, Stefan
2022.
Diffusion Bridges for Stochastic Hamiltonian Systems and Shape Evolutions.
SIAM Journal on Imaging Sciences,
Vol. 15,
Issue. 1,
p.
293.
Delyon, Bernard
and
Marchand, Jean-Louis
2023.
Conditioning diffusions with respect to incomplete observations.
Statistical Inference for Stochastic Processes,
Vol. 26,
Issue. 3,
p.
499.
Corstanje, Marc
van der Meulen, Frank
and
Schauer, Moritz
2023.
Conditioning continuous-time Markov processes by guiding.
Stochastics,
Vol. 95,
Issue. 6,
p.
963.
Pieper-Sethmacher, Thorben
van der Meulen, Frank
and
van der Vaart, Aad
2025.
On a class of exponential changes of measure for stochastic PDEs.
Stochastic Processes and their Applications,
Vol. 185,
Issue. ,
p.
104630.
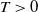 $T>0$
only linear combinations of its components are observed. That is, one only observes the vector
$T>0$
only linear combinations of its components are observed. That is, one only observes the vector 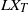 $L X_T$
for a given matrix L. In this paper we show how samples from the conditioned process can be generated. The main contribution of this paper is to prove that guided proposals, introduced in [35], can be used in a unified way for both uniformly elliptic and hypo-elliptic diffusions, even when L is not the identity matrix. This is illustrated by excellent performance in two challenging cases: a partially observed twice-integrated diffusion with multiple wells and the partially observed FitzHugh–Nagumo model.
$L X_T$
for a given matrix L. In this paper we show how samples from the conditioned process can be generated. The main contribution of this paper is to prove that guided proposals, introduced in [35], can be used in a unified way for both uniformly elliptic and hypo-elliptic diffusions, even when L is not the identity matrix. This is illustrated by excellent performance in two challenging cases: a partially observed twice-integrated diffusion with multiple wells and the partially observed FitzHugh–Nagumo model.