Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-29T09:01:46.522Z Has data issue: false hasContentIssue false
  • English
  • Français

The infinitely-many-neutral-alleles diffusion model

Published online by Cambridge University Press:  01 July 2016

S. N. Ethier  and
Thomas G. Kurtz
S. N. Ethier*
Affiliation:
Michigan State University
Thomas G. Kurtz*
Affiliation:
University of Wisconsin-Madison
*
∗Postal address: Department of Statistics and Probability, Michigan State University, East Lansing, MI 48824, U.S.A.
∗∗Postal address: Department of Mathematics, University of Wisconsin-Madison, Madison, WI 53706, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Abstract

A diffusion process X(·) in the infinite-dimensional ordered simplex is characterized in terms of the generator 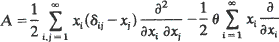defined on an appropriate domain. It is shown that X(·) is the limit in distribution of several sequences of discrete stochastic models of the infinitely-many-neutral-alleles type. It is further shown that X(·) has a unique stationary distribution and is reversible and ergodic. Kingman's limit theorem for the descending order statistics of the symmetric Dirichlet distribution is obtained as a corollary.

Keywords

INFINITE-DIMENSIONAL DIFFUSION PROCESSWEAK CONVERGENCESTATIONARY DISTRIBUTIONREVERSIBILITYERGODICITYPOISSON–DIRICHLET DISTRIBUTIONEWENS' SAMPLING FORMULA
Type
Research Article
Information
Advances in Applied Probability , Volume 13 , Issue 3 , September 1981 , pp. 429 - 452
Copyright
Copyright © Applied Probability Trust 1981 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Research supported in part by the National Science Foundation.

References

Blumenthal, R. M. and Getoor, R. K. (1968) Markov Processes and Potential Theory. Academic Press, New York.Google Scholar
Dynkin, E. B. (1965) Markov Processes, Vol. I. Academic Press, New York.Google Scholar
Ewens, W. J. (1972) The sampling theory of selectively neutral alleles. Theoret. Popn Biol. 3, 87–112.CrossRefGoogle ScholarPubMed
Ewens, W. J. (1979) Mathematical Population Genetics. Springer-Verlag, Berlin.Google Scholar
Ewens, W. J. and Kirby, K. (1975) The eigenvalues of the neutral alleles process. Theoret. Popn Biol. 7, 212–220.Google Scholar
Griffiths, R. C. (1979a) On the distribution of allele frequencies in a diffusion model. Theoret. Popn Biol. 15, 140–158.Google Scholar
Griffiths, R. C. (1979b) A transition density expansion for a multi-allele diffusion model. Adv. Appl. Prob. 11, 310–325.Google Scholar
Griffiths, R. C. (1979c) Exact sampling distributions from the infinite neutral alleles model. Adv. Appl. Prob. 11, 326–354.Google Scholar
Karlin, S. and McGregor, J. (1967) The number of mutant forms maintained in a population. Proc. 5th Berkeley Symposium on Math. Statist. Prob. 4, 415–438.Google Scholar
Kimura, M. (1979) The neutral theory of molecular evolution. Scientific Amer. 241 (November), 98–126.CrossRefGoogle ScholarPubMed
Kimura, M. and Crow, J. F. (1964) The number of alleles that can be maintained in a finite population. Genetics 49, 725–738.Google Scholar
Kingman, J. F. C. (1975) Random discrete distributions. J. R. Statist. Soc. B 37, 1–22.Google Scholar
Kingman, J. F. C. (1977) The population structure associated with the Ewens sampling formula. Theoret. Popn Biol. 11, 274–283.CrossRefGoogle ScholarPubMed
Kingman, J. F. C. (1978) Random partitions in population genetics. Proc. R. Soc. London A 361, 1–20.Google Scholar
Kurtz, T. G. (1975) Semigroups of conditioned shifts and approximation of Markov processes. Ann. Prob. 3, 618–642.Google Scholar
Norman, M. F. (1977) Ergodicity of diffusion and temporal uniformity of diffusion approximation. J. Appl. Prob. 14, 399–404.Google Scholar
Sato, K. (1978) Diffusion operators in population genetics and convergence of Markov chains. In Measure Theory Applications to Stochastic Analysis. Lecture Notes in Mathematics 695, 127–143. Springer-Verlag, Berlin.Google Scholar
Shiga, T. (1981) Diffusion processes in population genetics. J. Math. Kyoto Univ. Google Scholar
Watterson, G. A. (1974) The sampling theory of selectively neutral alleles. Adv. Appl. Prob. 6, 463–488.Google Scholar
Watterson, G. A. (1976a) Reversibility and the age of an allele. I. Moran's infinitely many neutral alleles model. Theoret. Popn Biol. 10, 239–253.Google Scholar
Watterson, G. A. (1976b) The stationary distribution of the infinitely-many neutral alleles diffusion model. J. Appl. Prob. 13, 639–651.Google Scholar
Watterson, G. A. and Guess, H. A. (1977) Is the most frequent allele the oldest? Theoret. Popn Biol. 11, 141–160.Google Scholar
Wright, S. (1949) Adaptation and selection. In Genetics, Paleontology, and Evolution. ed. Jepson, G. L., Mayr, E., Simpson, G. G., 365–389. Princeton University Press.Google Scholar