Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Davis, Richard
and
Resnick, Sidney
1988.
Extremes of moving averages of random variables from the domain of attraction of the double exponential distribution.
Stochastic Processes and their Applications,
Vol. 30,
Issue. 1,
p.
41.
Cline, Daren B.H.
and
Resnick, Sidney I.
1992.
Multivariate subexponential distributions.
Stochastic Processes and their Applications,
Vol. 42,
Issue. 1,
p.
49.
Willekens, E.
and
Teugels, J. L.
1992.
Asymptotic expansions for waiting time probabilities in an M/G/1 queue with long-tailed service time.
Queueing Systems,
Vol. 10,
Issue. 4,
p.
295.
Cline, D.B.H.
and
Samorodnitsky, G.
1994.
Subexponentiality of the product of independent random variables.
Stochastic Processes and their Applications,
Vol. 49,
Issue. 1,
p.
75.
Asmussen, Søren
and
Klüppelberg, Claudia
1996.
Large deviations results for subexponential tails, with applications to insurance risk.
Stochastic Processes and their Applications,
Vol. 64,
Issue. 1,
p.
103.
Asmussen, Søren
1996.
Stochastic Networks.
Vol. 117,
Issue. ,
p.
197.
Perline, Richard
1998.
Mixed Poisson distributions tail equivalent to their mixing distributions.
Statistics & Probability Letters,
Vol. 38,
Issue. 3,
p.
229.
Asmussen, Søren
1998.
Subexponential asymptotics for stochastic processes: extremal behavior, stationary distributions and first passage probabilities.
The Annals of Applied Probability,
Vol. 8,
Issue. 2,
Liebner, Simone
1998.
Advances in Stochastic Models for Reliability, Quality and Safety.
p.
67.
Schmidli, Hanspeter
1999.
On the Distribution of the Surplus Prior and at Ruin.
ASTIN Bulletin,
Vol. 29,
Issue. 2,
p.
227.
Asmussen, S.
and
Kalashnikov, V.
1999.
Failure rates of regenerative systems with heavy tails.
Journal of Mathematical Sciences,
Vol. 93,
Issue. 4,
p.
486.
Boots, N.K.
and
Shahabuddin, P.
2000.
Simulating GI/GI/1 queues and insurance risk processes with subexponential distributions.
Vol. 1,
Issue. ,
p.
656.
Nam Kyoo Boots
and
Shahabuddin, P.
2001.
Simulating ruin probabilities in insurance risk processes with subexponential claims.
p.
468.
FOCARDI, SERGIO M.
and
FABOZZI, FRANK J.
2003.
Fat Tails, Scaling, and Stable Laws:A Critical Look at Modeling Extremal Events in Financial Phenomena.
The Journal of Risk Finance,
Vol. 5,
Issue. 1,
p.
5.
Klüppelberg, Claudia
2004.
Encyclopedia of Actuarial Science.
Schmidli, Hanspeter
2005.
On optimal investment and subexponential claims.
Insurance: Mathematics and Economics,
Vol. 36,
Issue. 1,
p.
25.
Ganesh, Ayalvadi
and
Torrisi, Giovanni Luca
2006.
A class of risk processes with delayed claims: ruin probability estimates under heavy tail conditions.
Journal of Applied Probability,
Vol. 43,
Issue. 04,
p.
916.
Ganesh, Ayalvadi
and
Torrisi, Giovanni Luca
2006.
A class of risk processes with delayed claims: ruin probability estimates under heavy tail conditions.
Journal of Applied Probability,
Vol. 43,
Issue. 4,
p.
916.
Ganesh, Ayalvadi J.
and
Torrisi, Giovanni Luca
2008.
Large Deviations of the Interference in a Wireless Communication Model.
IEEE Transactions on Information Theory,
Vol. 54,
Issue. 8,
p.
3505.
Fasen, Vicky
and
Klüppelberg, Claudia
2008.
Encyclopedia of Quantitative Risk Analysis and Assessment.


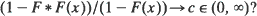 Sufficient conditions and examples are given.
Sufficient conditions and examples are given.