Article contents
Convexity of a set of stochastically ordered random variables
Published online by Cambridge University Press: 01 July 2016
Abstract
It is shown that a set of random variables with increasing and convex (concave) survival functions are stochastically increasing and convex (concave) in the sample path sense. This stochastic convexity (concavity) result is then used to establish convexity (concavity) results for (i) a single-server queueing system with a time-out control policy, (ii) residual life, (iii) stationary renewal excess life and (iv) M/G/1 queues. These results are new and could not be derived without the direct or indirect aid of the above stochastic convexity (concavity) result. Furthermore, we illustrate that the above stochastic convexity (concavity) result can be applied to obtain new bounds for queueing systems. Specifically, let 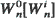 be the waiting time of the nth customer in a GI/G/1 queue with inter-arrival time survival function
be the waiting time of the nth customer in a GI/G/1 queue with inter-arrival time survival function 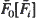 and service time survival function
and service time survival function 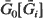 . Using the above convexity result it is shown that if
. Using the above convexity result it is shown that if 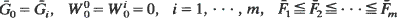 and for some
and for some 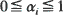 such that
such that 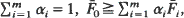 then
then 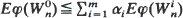 for all increasing convex functions φ, whenever the expectations exist. A similar result for
for all increasing convex functions φ, whenever the expectations exist. A similar result for 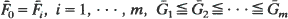 and
and  is also obtained. Other examples are also included.
is also obtained. Other examples are also included.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Applied Probability Trust 1990
Footnotes
Supported by the Air Force Office of Scientific Research, USAF under Grant AFOSR-84–0205. Reproduction in whole or in part is permitted for any purpose by the United States Government.
References
- 18
- Cited by


