Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-26T18:52:23.545Z Has data issue: false hasContentIssue false
  • English
  • Français

Error Analysis for a Non-Monotone FEM for a Singularly Perturbed Problem with Two Small Parameters

Published online by Cambridge University Press:  10 March 2015

Yanping Chen ,
Haitao Leng  and
Li-Bin Liu
Yanping Chen*
Affiliation:
School of Mathematical Sciences, South China Normal University, Guangzhou 510631, China
Haitao Leng
Affiliation:
School of Mathematical Sciences, South China Normal University, Guangzhou 510631, China
Li-Bin Liu
Affiliation:
School of Mathematical Sciences, South China Normal University, Guangzhou 510631, China Department of Mathematics and Computer Science, Chizhou College, Anhui 247000, China
*
*Corresponding author. Email: [email protected] (Y. P. Chen)
Get access

Abstract

In this paper, we consider a singularly perturbed convection-diffusion problem. The problem involves two small parameters that gives rise to two boundary layers at two endpoints of the domain. For this problem, a non-monotone finite element methods is used. A priori error bound in the maximum norm is obtained. Based on the a priori error bound, we show that there exists Bakhvalov-type mesh that gives optimal error bound of 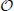(N−2) which is robust with respect to the two perturbation parameters. Numerical results are given that confirm the theoretical result.

Keywords

65N30Non-monotonesingularly perturbedconvection-diffusionBakhvalov-typetwo small parameters
Type
Research Article
Information
Advances in Applied Mathematics and Mechanics , Volume 7 , Issue 2 , April 2015 , pp. 196 - 206
Copyright
Copyright © Global-Science Press 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Linss, T., Roos, H.-G. and Vulanovic, R., Uniform pointwise convergence on Shishkin-type meshes for quasi-linwar convection-diffusion problems, Siam J. Numer. Anal., 38 (2000), pp. 897–912.CrossRefGoogle Scholar
[2]Liu, W. B. and Tang, T., Error analysis for a Galerkin-spectral method with coordinate transformation for solving singularly perturbed problems, Appl. Numer. Math., 38 (2001), pp. 315–345.CrossRefGoogle Scholar
[3]Tang, T. and Trummer, M. R., Boundary layer resolving pseudospectral methods for singular perturbation problems, Siam J. Sci. Comput., 17 (1996), pp. 430–438.CrossRefGoogle Scholar
[4]Vulanovic, R., A priori meshes for singularly perturbed quasilinear two-point boundary value problems, Ima J. Numer. Anal., 21 (2001), pp. 349–366.CrossRefGoogle Scholar
[5]Cai, X., Cai, D. L., and Wu, R. Q.et al., High accuracy non-equidistant method for singularly perturbation reaction-diffusion problem, Appl. Math. Mech., 30 (2009), pp. 175–182.CrossRefGoogle Scholar
[6]Linss, T., Maximum norm error analysis of a non-monotone FEM for a singularly perturbed reaction-diffusion problem, Bit., 47 (2007), pp. 379–391.CrossRefGoogle Scholar
[7]Linss, T. and Roos, H.-G., Analysis of a finite-difference scheme for a singularly perturbed problem with two small parameters, J. Math. Anal. Appl., 289 (2004), pp. 355–366.CrossRefGoogle Scholar
[8]Gracica, J. L., O’Riordan, E. and Pickett, M. L., A parameter robust second order numerical method for a singularly perturbed two-parameter problem, Appl. Numer. Math., 56 (2006), pp. 962–980.CrossRefGoogle Scholar
[9]Linss, T., A posteriori error estimation for a singularly perturbed problem with two small parameters, Int. J. Numer. Anal. Mod., 7(3) (2010), pp. 491–506.Google Scholar
[10]Roos, H.-G. and Uzelac, Z., The SDFEM for a convection-diffusion problem with two small parameters, Comput. Meth. Appl. Math., 3(3) (2003), pp. 443–458.CrossRefGoogle Scholar
[11]Wu, Y., Zhang, N. and Yuan, J., A robust adaptive method for singularly perturbed convection-diffusion problem with two small parameters, Comput. Math. Appl., 66 (2013), pp. 996–1009.CrossRefGoogle Scholar
[12]De Boor, C., Good approximation by splines with variable knots, In: Meri, A. and Sharma, A. (eds.) Spline Functions Approx. Theory, Proc. Sympos. Univ. Alberta, Edmonton 1972, pp. 57–72.Google Scholar