Skip to main content Accessibility help

We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.

Close cookie message
×
Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-23T14:56:22.485Z Has data issue: false hasContentIssue false

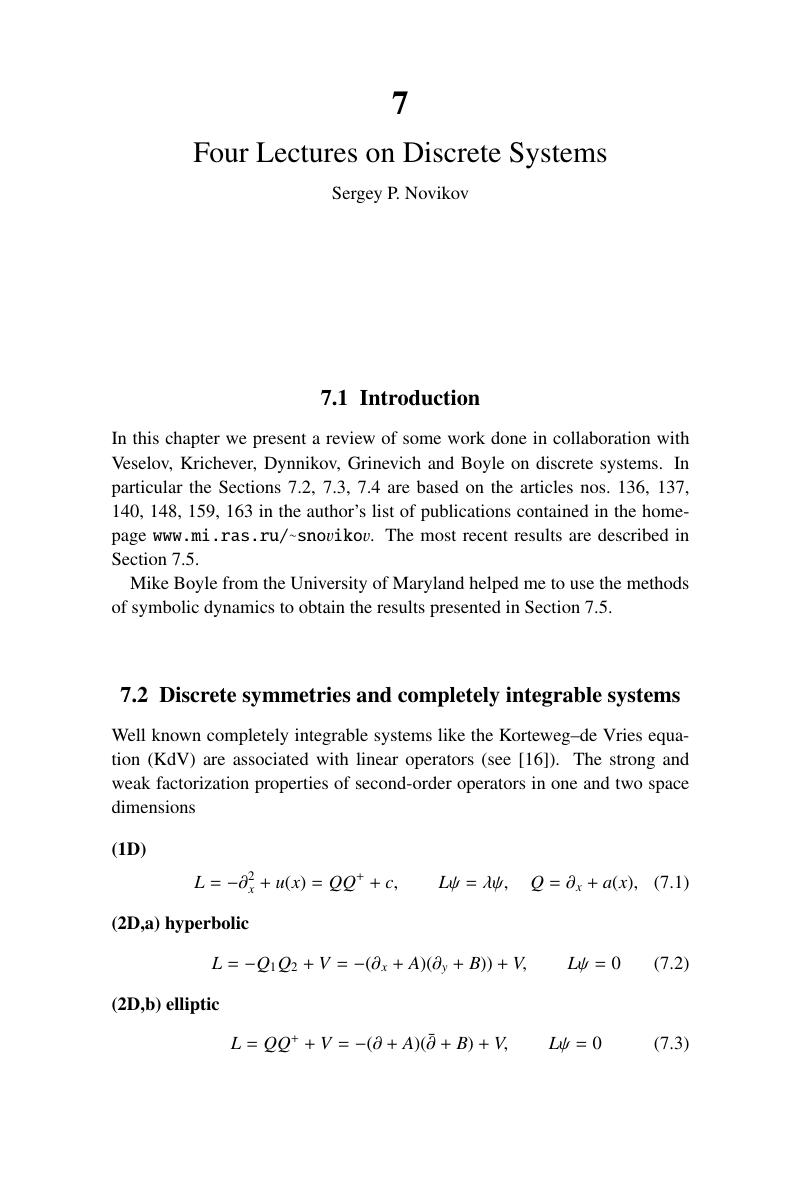
7 - Four Lectures on Discrete Systems

Published online by Cambridge University Press:  05 July 2011

By
Sergey P. Novikov
Edited by
Decio Levi ,
Peter Olver ,
Zora Thomova  and
Pavel Winternitz
Sergey P. Novikov
Affiliation:
University of Maryland
Decio Levi
Affiliation:
Università degli Studi Roma Tre
Peter Olver
Affiliation:
University of Minnesota
Zora Thomova
Affiliation:
SUNY Institute of Technology
Pavel Winternitz
Affiliation:
Université de Montréal
Get access

Summary

A summary is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
Image of the first page of this content. For PDF version, please use the ‘Save PDF’ preceeding this image.'
Type
Chapter
Information
Symmetries and Integrability of Difference Equations , pp. 191 - 206
Publisher: Cambridge University Press
Print publication year: 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Bobenko, A. I., Mercat, C., and Suris, Yu. B. 2005. Linear and nonlinear theories of discrete analytic functions. Integrable structure and isomonodromic Green's function. J. Reine Angew. Math., 583, 117–161.CrossRefGoogle Scholar
[2] Chekhov, L. O., and Puzyrnikova, N. V. 2000. Integrable systems on graphs. Uspekhi Mat. Nauk, 55(5(335)), 181–182. English transl., Russian Math. Surveys55(5), 992–994.Google Scholar
[3] Dubrovin, B. A., and Krichever, I. M. 1976. The Schrödinger equation in a periodic field and Riemann surfaces. Dokl. Akad. Nauk SSSR, 229(1), 15–18. Russian.Google Scholar
[4] Dynnikov, I. A., and Novikov, S. P. 2003. Geometry of the triangle equation on two-manifolds. Mosc. Math. J., 3(2), 419–438.Google Scholar
[5] Ferrand, J. 1944. Fonctions préharmoniques et fonctions préholomorphes. Bull. Sci. Math. (2), 68, 152–180.Google Scholar
[6] Fokas, A. S., and Santini, P. M. 1988. Bi-Hamiltonian formulation of the Kadomtsev–Petviashvili and Benjamin–Ono equations. J. Math. Phys., 29(3), 604–617.CrossRefGoogle Scholar
[7] Gervais, J. L., and Neveu, A. 1979. Local harmonicity of the Wilson loop integral in classical Yang–Mills theory. Nuclear Phys. B, 153, 445–454.CrossRefGoogle Scholar
[8] Grinevich, P. G., and Manakov, S. V. 1986. Inverse problem of scattering theory for the two-dimensional Schrödinger operator, the ∂-method and nonlinear equations. Funktsional. Anal. i Prilozhen., 20(2), 14–24. English transl., Functional Anal. Appl.20(2), 94–103.CrossRefGoogle Scholar
[9] Grinevich, P. G., and Novikov, R. G. 1986. Analogues of multisoliton potentials for the two-dimensional Schrödinger operator, and a nonlocal Riemann problem. Dokl. Akad. Nauk SSSR, 286(1), 19–22. English transl., Soviet Math. Dokl.33(1), 9–12.Google Scholar
[10] Grinevich, P. G., and Novikov, R. G. 2007. The Cauchy kernel for the Novikov–Dynnikov DN-discrete complex analysis on a triangular lattice. Uspekhi Mat. Nauk, 62(4), 155–156. English transl., Russian Math. Surveys62(4), 799–801.Google Scholar
[11] Grinevich, P. G., and Novikov, S. P. 1988. A two-dimensional “inverse scattering problem” for negative energies, and generalized-analytic functions. I. Energies lower than the ground state. Funktsional. Anal. i Prilozhen., 22(1), 23–33. English transl., Funct. Anal. Appl.22(1), 19–27.CrossRefGoogle Scholar
[12] Krichever, I. M., and Novikov, S. P. 1999. Trivalent graphs and solitons. Uspekhi Mat. Nauk, 54(6), 149–150. English transl., Russian Math. Surveys54(6), 1248–1249.Google Scholar
[13] Manakov, S. V. 1976. The method of the inverse scattering problem, and two-dimensional evolution equations. Uspehi Mat. Nauk, 31(5), 245–246. English transl., Russian Math. Surveys31(5), 245–246.Google Scholar
[14] Novikov, S. P. 1997. Algebraic properties of two-dimensional difference operators. Uspekhi Mat. Nauk, 52(1), 225–226. English transl., Russian Math. Surveys52(1), 226–227.Google Scholar
[15] Novikov, S. P. 2004. Discrete connections and linear difference equations. Tr. Mat. Inst. Steklova, 247(Geom. Topol. i Teor. Mnozh.), 186–201. English transl., Proc. Steklov Inst. Math.2004(4), 168–183.Google Scholar
[16] Novikov, S. P., and Dynnikov, I. A. 1997. Discrete spectral symmetries of small-dimensional differential operators and difference operators on regular lattices and two-dimensional manifolds. Uspekhi Mat. Nauk, 52(5), 175–234. English transl., Russian Math. Surveys52(5), 1057–1116.Google Scholar
[17] Novikov, S. P., and Veselov, A. P. 1986. Two-dimensional Schrödinger operator: inverse scattering transform and evolutional equations. Phys. D, 18(1-3), 267–273.CrossRefGoogle Scholar
[18] Seifert, H., and Threlfall, W. 1980. Seifert and Threlfall: a textbook of topology. Pure Appl. Math., vol. 89. New York: Academic Press.Google Scholar
[19] Veselov, A. P., and Novikov, S. P. 1984a. Finite-gap two-dimensional potential Schrödinger operators. Explicit formulas and evolution equations. Dokl. Akad. Nauk SSSR, 279(1), 20–24. English transl., Soviet Math. Dokl.30(3), 588–591.Google Scholar
[20] Veselov, A. P., and Novikov, S. P. 1984b. Finite-gap two-dimensional Schrödinger operators. Potential operators. Dokl. Akad. Nauk SSSR, 279(4), 784–788.Google Scholar
[21] ,Wikipedia. 2009. Simplicial complex. http://en.wikipedia.org/wiki/Simplicial_complex.
[22] Wilson, K. G. 1974. Confinement of quarks. Phys. Rev. D, 10(8), 2445–2459.CrossRefGoogle Scholar

Save book to Kindle

To save this book to your Kindle, first ensure [email protected] is added to your Approved Personal Document E-mail List under your Personal Document Settings on the Manage Your Content and Devices page of your Amazon account. Then enter the ‘name’ part of your Kindle email address below. Find out more about saving to your Kindle.

Note you can select to save to either the @free.kindle.com or @kindle.com variations. ‘@free.kindle.com’ emails are free but can only be saved to your device when it is connected to wi-fi. ‘@kindle.com’ emails can be delivered even when you are not connected to wi-fi, but note that service fees apply.

Find out more about the Kindle Personal Document Service.

Available formats
×

Save book to Dropbox

To save content items to your account, please confirm that you agree to abide by our usage policies. If this is the first time you use this feature, you will be asked to authorise Cambridge Core to connect with your account. Find out more about saving content to Dropbox.

Available formats
×

Save book to Google Drive

To save content items to your account, please confirm that you agree to abide by our usage policies. If this is the first time you use this feature, you will be asked to authorise Cambridge Core to connect with your account. Find out more about saving content to Google Drive.

Available formats
×