Book contents
- Frontmatter
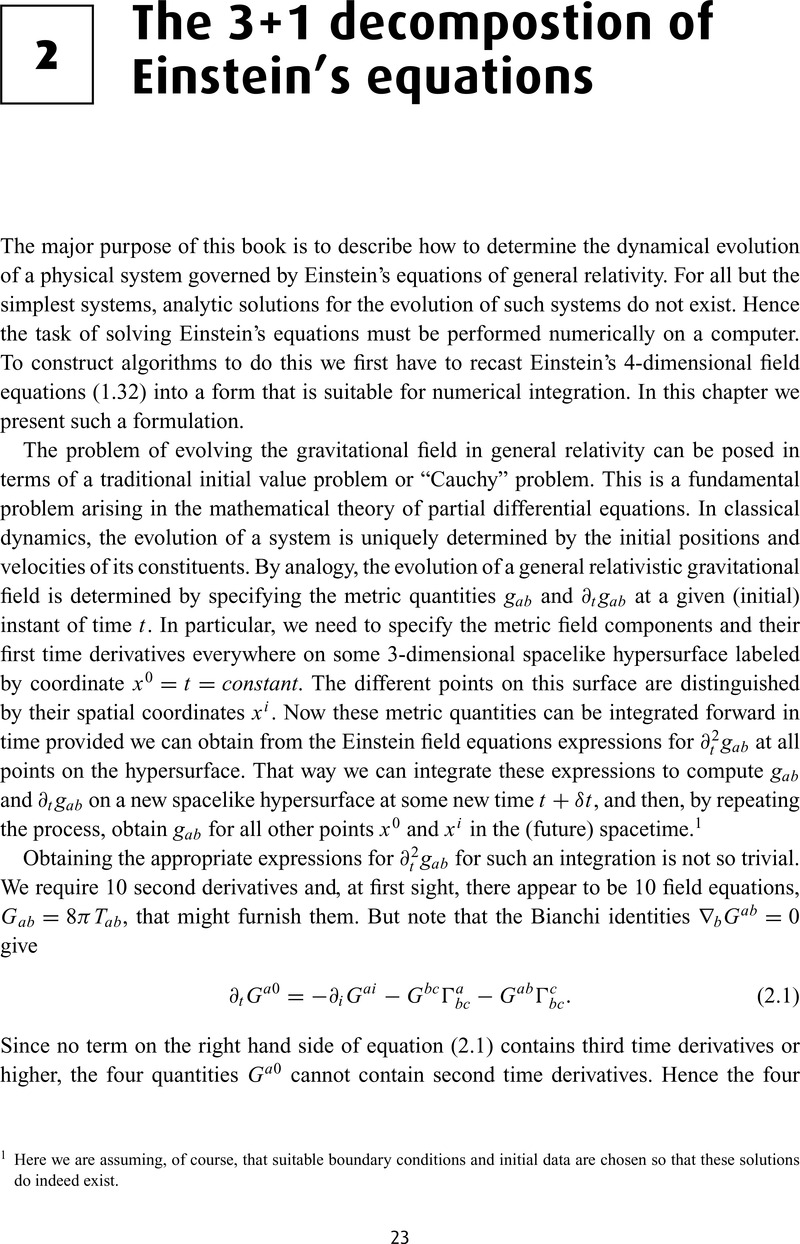
- Contents
- Preface
- Suggestions for using this book
- 1 General relativity preliminaries
- 2 The 3 + 1 decompostion of Einstein's equations
- 3 Constructing initial data
- 4 Choosing coordinates: the lapse and shift
- 5 Matter sources
- 6 Numerical methods
- 7 Locating black hole horizons
- 8 Spherically symmetric spacetimes
- 9 Gravitational waves
- 10 Collapse of collisionless clusters in axisymmetry
- 11 Recasting the evolution equations
- 12 Binary black hole initial data
- 13 Binary black hole evolution
- 14 Rotating stars
- 15 Binary neutron star initial data
- 16 Binary neutron star evolution
- 17 Binary black hole–neutron stars: initial data and evolution
- 18 Epilogue
- A Lie derivatives, Killing vectors, and tensor densities
- B Solving the vector Laplacian
- C The surface element on the apparent horizon
- D Scalar, vector and tensor spherical harmonics
- E Post-Newtonian results
- F Collisionless matter evolution in axisymmetry: basic equations
- G Rotating equilibria: gravitational field equations
- H Moving puncture representions of Schwarzschild: analytical results
- I Binary black hole puncture simulations as test problems
- References
- Index
2 - The 3 + 1 decompostion of Einstein's equations
Published online by Cambridge University Press: 05 March 2013
Book contents
- Frontmatter
- Contents
- Preface
- Suggestions for using this book
- 1 General relativity preliminaries
- 2 The 3 + 1 decompostion of Einstein's equations
- 3 Constructing initial data
- 4 Choosing coordinates: the lapse and shift
- 5 Matter sources
- 6 Numerical methods
- 7 Locating black hole horizons
- 8 Spherically symmetric spacetimes
- 9 Gravitational waves
- 10 Collapse of collisionless clusters in axisymmetry
- 11 Recasting the evolution equations
- 12 Binary black hole initial data
- 13 Binary black hole evolution
- 14 Rotating stars
- 15 Binary neutron star initial data
- 16 Binary neutron star evolution
- 17 Binary black hole–neutron stars: initial data and evolution
- 18 Epilogue
- A Lie derivatives, Killing vectors, and tensor densities
- B Solving the vector Laplacian
- C The surface element on the apparent horizon
- D Scalar, vector and tensor spherical harmonics
- E Post-Newtonian results
- F Collisionless matter evolution in axisymmetry: basic equations
- G Rotating equilibria: gravitational field equations
- H Moving puncture representions of Schwarzschild: analytical results
- I Binary black hole puncture simulations as test problems
- References
- Index
Summary
A summary is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
- Type
- Chapter
- Information
- Numerical RelativitySolving Einstein's Equations on the Computer, pp. 23 - 53Publisher: Cambridge University PressPrint publication year: 2010