Relying only on the classical Bahadur–Rao approximation for large deviations (LDs) of univariate sample means, we derive strong LD approximations for probabilities involving two sets of sample means. The main result concerns the exact asymptotics (as n→∞) of
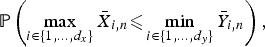 $$ {\open P}\left({\max_{i\in\{1,\ldots,d_x\}}\bar X_{i,n} \les \min_{i\in\{1,\ldots,d_y\}}\bar Y_{i,n}}\right),$$
$$ {\open P}\left({\max_{i\in\{1,\ldots,d_x\}}\bar X_{i,n} \les \min_{i\in\{1,\ldots,d_y\}}\bar Y_{i,n}}\right),$$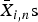 ${\bar X}_{i,n}{\rm s}$ (
${\bar X}_{i,n}{\rm s}$ ( ${\bar Y}_{i,n}{\rm s}$, respectively) denoting dx (dy) independent copies of sample means associated with the random variable X (Y). Assuming
${\bar Y}_{i,n}{\rm s}$, respectively) denoting dx (dy) independent copies of sample means associated with the random variable X (Y). Assuming 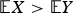 ${\open E}X \gt {\open E}Y$ , this is a rare event probability that vanishes essentially exponentially, but with an additional polynomial term. We also point out how the probability of interest can be estimated using importance sampling in a logarithmically efficient way. To demonstrate the usefulness of the result, we show how it can be applied to compare the order statistics of the sample means of the two populations. This has various applications, for instance in queuing or packing problems.
${\open E}X \gt {\open E}Y$ , this is a rare event probability that vanishes essentially exponentially, but with an additional polynomial term. We also point out how the probability of interest can be estimated using importance sampling in a logarithmically efficient way. To demonstrate the usefulness of the result, we show how it can be applied to compare the order statistics of the sample means of the two populations. This has various applications, for instance in queuing or packing problems.