Let S0 := 0 and Sk := ξ1 + ··· + ξk for k ∈ ℕ := {1, 2, …}, where {ξk : k ∈ ℕ} are independent copies of a random variable ξ with values in ℕ and distribution pk := P{ξ = k}, k ∈ ℕ. We interpret the random walk {Sk : k = 0, 1, 2, …} as a particle jumping to the right through integer positions. Fix n ∈ ℕ and modify the process by requiring that the particle is bumped back to its current state each time a jump would bring the particle to a state larger than or equal to n. This constraint defines an increasing Markov chain {Rk(n) : k = 0, 1, 2, …} which never reaches the state n. We call this process a random walk with barrier n. Let Mn denote the number of jumps of the random walk with barrier n. This paper focuses on the asymptotics of Mn as n tends to ∞. A key observation is that, under p1 > 0, {Mn : n ∈ ℕ} satisfies the distributional recursion M1 = 0 and 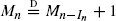 for n = 2, 3, …, where In is independent of M2, …, Mn−1 with distribution P{In = k} = pk / (p1 + ··· + pn−1), k ∈ {1, …, n − 1}. Depending on the tail behavior of the distribution of ξ, several scalings for Mn and corresponding limiting distributions come into play, including stable distributions and distributions of exponential integrals of subordinators. The methods used in this paper are mainly probabilistic. The key tool is to compare (couple) the number of jumps, Mn, with the first time, Nn, when the unrestricted random walk {Sk : k = 0, 1, …} reaches a state larger than or equal to n. The results are applied to derive the asymptotics of the number of collision events (that take place until there is just a single block) for β(a, b)-coalescent processes with parameters 0 < a < 2 and b = 1.
for n = 2, 3, …, where In is independent of M2, …, Mn−1 with distribution P{In = k} = pk / (p1 + ··· + pn−1), k ∈ {1, …, n − 1}. Depending on the tail behavior of the distribution of ξ, several scalings for Mn and corresponding limiting distributions come into play, including stable distributions and distributions of exponential integrals of subordinators. The methods used in this paper are mainly probabilistic. The key tool is to compare (couple) the number of jumps, Mn, with the first time, Nn, when the unrestricted random walk {Sk : k = 0, 1, …} reaches a state larger than or equal to n. The results are applied to derive the asymptotics of the number of collision events (that take place until there is just a single block) for β(a, b)-coalescent processes with parameters 0 < a < 2 and b = 1.

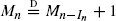 for
for 