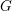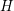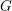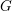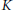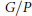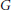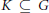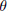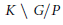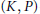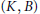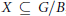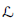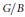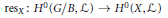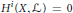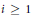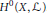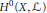Let $G$ be a simple algebraic group. A closed subgroup $H$ of $G$ is said to be spherical if it has a dense orbit on the flag variety $G/B$ of $G$. Reductive spherical subgroups of simple Lie groups were classified by Krämer in 1979. In 1997, Brundan showed that each example from Krämer’s list also gives rise to a spherical subgroup in the corresponding simple algebraic group in any positive characteristic. Nevertheless, up to now there has been no classification of all such instances in positive characteristic. The goal of this paper is to complete this classification. It turns out that there is only one additional instance (up to isogeny) in characteristic 2 which has no counterpart in Krämer’s classification. As one of our key tools, we prove a general deformation result for subgroup schemes that allows us to deduce the sphericality of subgroups in positive characteristic from the same property for subgroups in characteristic zero.

We study 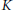$K$-orbits in 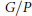$G/P$ where 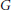$G$ is a complex connected reductive group, $P\,\subseteq \,G$ is a parabolic subgroup, and 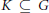$K\,\subseteq \,G$ is the fixed point subgroup of an involutive automorphism 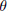$\theta$. Generalizing work of Springer, we parametrize the (finite) orbit set 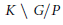$K\,\backslash \,G/P$ and we determine the isotropy groups. As a consequence, we describe the closed (resp. affine) orbits in terms of 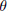$\theta$-stable (resp. 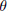$\theta$-split) parabolic subgroups. We also describe the decomposition of any 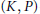$(K,\,P)$-double coset in 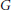$G$ into 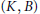$(K,\,B)$-double cosets, where $B\,\subseteq \,P$ is a Borel subgroup. Finally, for certain 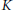$K$-orbit closures 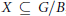$X\,\subseteq \,G/B$, and for any homogeneous line bundle 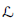$\mathcal{L}$ on 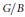$G/B$ having nonzero global sections, we show that the restriction map 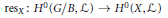$\text{re}{{\text{s}}_{X}}\,:\,{{H}^{0}}\,\left( G\,/\,B,\,\mathcal{L} \right)\,\to \,{{H}^{0}}\,\left( X,\,\mathcal{L} \right)$ is surjective and that 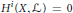${{H}^{i}}\,\left( X,\mathcal{L} \right)\,=\,0$ for 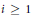$i\,\ge \,1$. Moreover, we describe the 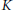$K$-module 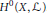${{H}^{0}}\left( X,L \right)$. This gives information on the restriction to 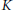$K$ of the simple 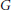$G$-module 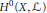${{H}^{0}}\,\left( G\,/\,B,\mathcal{L} \right)$. Our construction is a geometric analogue of Vogan and Sepanski’s approach to extremal 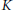$K$-types.