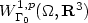Skip to main content Accessibility help

We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.

Close cookie message
×
  1. Home
  2. Search

Refine search

Refine search

Access:
Content type:
Publication date:
Apply   From and To filters
Subject: Show more
Journals Show more
Publishers: Show more
Societies Show more
Series: Show more
Collections: Show more

Actions for selected content:

Select all | Deselect all
  • View selected items
  • Export citations
  • Download PDF (zip)
  • Save to Kindle
  • Save to Dropbox
  • Save to Google Drive

Save Search

You can save your searches here and later view and run them again in "My saved searches".

Please provide a title, maximum of 40 characters.
Cancel
×

1 results

  • The nonlinear membrane model: a Young measureand varifold formulation

  • Med Lamine Leghmizi, Christian Licht, Gérard Michaille
  • Journal:
    ESAIM: Control, Optimisation and Calculus of Variations / Volume 11 / Issue 3 / July 2005
    Published online by Cambridge University Press:
    15 July 2005, pp. 449-472
    Print publication:
    July 2005

  • We establish two new formulations of the membrane problem by working in the space of $W^{1,p}_{\Gamma_0}(\Omega,\mathbf R^3)$ 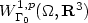-Young measures and $W^{1,p}_{\Gamma_0}(\Omega,\mathbf R^3)$ 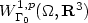-varifolds. The energyfunctional related to these formulations is obtained as a limit of the 3d formulation of the behavior of a thin layer for a suitable variational convergence associated with the narrow convergence of Young measures and with some weak convergence of varifolds. Theinterest of the first formulation is to encode the oscillation informations on the gradients minimizing sequences related to the classicalformulation. The second formulation moreover accounts for concentration effects.