We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We prove that if A is the infinitesimal generator of a bounded analytic semigroup in a sector {z ∊ C : |arg z| ≦ (απ)/2} of bounded linear operators on a Banach space, then the following inequalities hold:
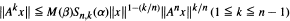
for any x ∊ D(An) and for any 0 < β < α. This result helps us to answer in affirmative a question raised by M. W. Certain and T. G. Kurtz [3]. Similar inequalities are proved for cosine operator funtions.
