Let F be the fraction field of the ring of Witt vectors over a perfect field of characteristic p (for example $F=\mathbb{Q}_p$), and let GF be the absolute Galois group of F. The main result of this article is the following: a p-adic representation of GF, which is a limit of subquotients of crystalline representations with Hodge–Tate weights in an interval [a; b], is itself crystalline with Hodge–Tate weights in [a; b]. In order to show this, we study the $(\phi,\Gamma)$-modules attached to crystalline representations, which allows us to improve some results of Fontaine, Wach and Colmez.

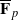 -representations of
-representations of 