We provide an alternative approach to the Faddeev–Reshetikhin–Takhtajan presentation of the quantum group $\uqg$, with $L$-operators as generators and relations ruled by an $R$-matrix. We look at $\uqg$ as being generated by the quantum Borel subalgebras $U_q(\mathfrak{b}_+)$ and $U_q(\mathfrak{b}_-)$, and use the standard presentation of the latter as quantum function algebras. When $\mathfrak{g}=\mathfrak{gl}_n$, these Borel quantum function algebras are generated by the entries of a triangular $q$-matrix. Thus, eventually, $U_q(\mathfrak{gl}_n)$ is generated by the entries of an upper triangular and a lower triangular $q$-matrix, which share the same diagonal. The same elements generate over $\Bbbk[q,q^{-1}]$ the unrestricted $\Bbbk [q,q^{-1}]$-integral form of $U_q(\mathfrak{gl}_n)$ of De Concini and Procesi, which we present explicitly, together with a neat description of the associated quantum Frobenius morphisms at roots of 1. All this holds, mutatis mutandis, for $\mathfrak{g}=\mathfrak{sl}_n$ too.

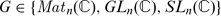 , let
, let  be the quantum function algebra – over
be the quantum function algebra – over 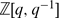 – associated to
– associated to 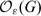 be the specialisation of the latter at a root of unity ϵ, whose order ℓ is odd. There is a quantum Frobenius morphism that embeds
be the specialisation of the latter at a root of unity ϵ, whose order ℓ is odd. There is a quantum Frobenius morphism that embeds  the function algebra of
the function algebra of 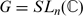 , it is known by [3], [4] that (the complexification of) such a module is free, with rank ℓ
, it is known by [3], [4] that (the complexification of) such a module is free, with rank ℓ and
and  are free Frobenius extensions over
are free Frobenius extensions over 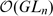 and
and 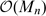 , thus extending some results of [5].
, thus extending some results of [5].