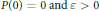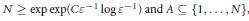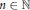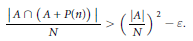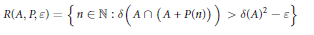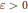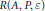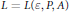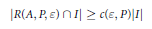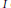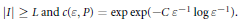The electoral success of extreme right parties (ERPs) has attracted a disproportionate number of studies. By contrast, research into the mainstream parties’ reactions to ERPs has engendered little interest. With few exceptions, the effects of the centre-right parties’ strategic options in electoral competitions with ERPs remain unexplored. To overcome this shortcoming, this investigation examines the strategies employed by the French centre-right party – Union pour un Movement Populaire (UMP) against the Front National in the 2007 and the 2012 presidential elections by focusing on the topics of immigration and integration. This study suggests that the adoption of accommodating approaches in both elections was followed by distinct levels of success in 2007 and 2012. Drawing on a qualitative comparative analysis, this article explores three hypotheses in order to enhance understanding of the divergent effectiveness of the UMP’s accommodative approaches in the elections studied.

Let $P\in \mathbb{Z}\left[ n \right]$ with 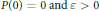$P(0)\,=\,0\,\text{and}\,\varepsilon \,>\,0$. We show, using Fourier analytic techniques, that if 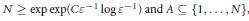$N\ge \exp \exp \left( C{{\varepsilon }^{-1}}\log {{\varepsilon }^{-1}} \right)\,\text{and}\,A\,\subseteq \,\left\{ 1,\,.\,.\,.\,,\,N \right\}$ then there must exist 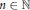$n\in \mathbb{N}$ such that

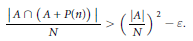$$\frac{\left| A\cap \left( A+P\left( n \right) \right) \right|}{N}>{{\left( \frac{\left| A \right|}{N} \right)}^{2}}-\,\varepsilon $$

In addition to this we show, using the same Fourier analytic methods, that if $A\subseteq \mathbb{N}$, then the set of $\varepsilon $-optimal return times

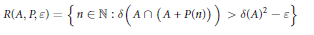$$R\left( A,P,\varepsilon \right)=\left\{ n\in \mathbb{N}:\delta \left( A\cap \left. \left( A+P\left( n \right) \right) \right)> \right.\delta {{\left( A \right)}^{2}}-\varepsilon \right\}$$

is syndetic for every 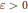$\varepsilon >0$. Moreover, we show that 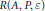$R\left( A,\,P,\,\varepsilon \right)$ is dense in every sufficiently long interval, in particular we show that there exists an 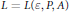$L=L\left( \varepsilon ,P,A \right)$ such that

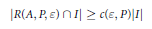$$\left| R\left( A,P,\varepsilon \right)\cap I \right|\ge c\left( \varepsilon ,P \right)\left| I \right|$$

for all intervals 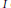$I$ of natural numbers with 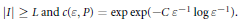$\left| I \right|\,\ge \,L\,\text{and}\,c\left( \varepsilon ,\,P \right)\,=\,\exp \exp \,\left( -C\,{{\varepsilon }^{-1}}\,\log {{\varepsilon }^{-1}} \right).$

One of the most remarkable results of the elections for the European Parliament on 4–7 June 2009 is that the Swedish Piratpartiet won one seat, to which another will be added if the Treaty of Lisbon enters into force. One of the aims of this party is a reform of copyright law to restore the, in its eyes, lost balance between the potentially conflicting interests of creating and spreading culture. The party strives for, among other things, the decriminalisation of all non-commercial copying and use of copyrighted material on the internet.

A glimpse of EU government

When president Sarkozy said, before the European Parliament on 16 December 2008, that the French presidency had allowed the European institutions to function at full capacity and successfully, he corrected a widely held image. The image, proffered in the media and in even better informed circles, was that he, Nicolas Sarkozy, happening into a repetition of crises which plunged him into his element, had personally gone around to solve one crisis after the other. According to Sarkozy himself, that was not the case. It was the European states, the Brussels institutions and the Parliament that had for once taken their responsibility and had acted.