Let [An,k]n,k⩾0 be an infinite lower triangular array satisfying the recurrence

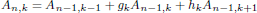
for n ⩾ 1 and k ⩾ 0, where A0,0 = 1, A0,k = Ak,–1 = 0 for k > 0. We present some criteria for the log-concavity of rows and strong q-log-convexity of generating functions of rows. Our results can be applied to many well-known triangular arrays, such as the Pascal triangle, the Stirling triangle of the second kind, the Bell triangle, the large Schröder triangle, the Motzkin triangle, and the Catalan triangles of Aigner and Shapiro, in a unified approach. In addition, we prove that the binomial transformation not only preserves the strong q-log-convexity property, but also preserves the strong q-log-concavity property. Finally, we demonstrate that the strong q-log-convexity property is preserved by the Stirling transformation and Whitney transformation of the second kind, which extends some known results for the strong q-log-convexity property.

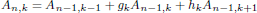
The Boros–Moll polynomials Pm (a) arise in the evaluation of a quartic integral. It has been conjectured by Boros and Moll that these polynomials are infinitely log-concave. In this paper, we show that Pm (a) is 2-log-concave for any m ≥ 2. Let di (m) be the coefficient of ai in Pm (a). We also show that the sequence is log-concave.