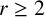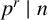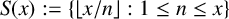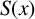1 Introduction and results
Let
$\lfloor t \rfloor $
be the integral part of
$t\in \mathbb {R}$
and let r be a fixed integer
$\geq 2$
. A positive integer n is called r-free if in its canonical prime representation, each exponent is
$<r$
; a 2-free integer is also called square-free. Let
$\mu _r$
be the characteristic function of the r-free integers. There is considerable research on the distribution of r-free integers over certain special sets, such as the set of integer parts, the Beatty sequence
$\lfloor \alpha n+\beta \rfloor $
, [Reference Abercrombie, Banks and Shparlinski1, Reference Goryashin8, Reference Gűloğlu and Nevans9, Reference Veasna, Srichan and Mavecha19] and the Piatetski-Shapiro sequence
$\lfloor n^c \rfloor $
, [Reference Cao and Zhai5–Reference Deshouillers7, Reference Rieger13–Reference Srichan15, Reference Stux17, Reference Tangsupphathawat, Srichan and Laohakosol18, Reference Zhang and Jinjiang21]. In 2019, Bordellés et al. [Reference Bordellés, Dai, Heyman, Pan and Shparlinski4] established an asymptotic formula for a sum of the form
$$ \begin{align*} \sum_{n\leq x}f\bigg( \bigg\lfloor\frac{x}{n}\bigg\rfloor \bigg), \end{align*} $$
where f is an arithmetic function subject to some growth condition, and applied it in particular to Euler’s totient function. It is thus natural to consider such a sum for various other functions. For example, in [Reference Bordellés3], Bordellés proved that
$$ \begin{align} S_{\mu_2}(x)=x\sum_{n=1}^\infty \frac{\mu_2(n)}{n(n+1)}+O(x^{1919/4268+\epsilon}), \end{align} $$
where
$\mu _2(n)$
is the characteristic function of the set of square-free numbers. Later, Liu et al. [Reference Liu, Wu and Yang12] improved the O-term in (1.1) to
$O(x^{2/5+\epsilon })$
. In 2022, Stucky [Reference Stucky16] generalised the sum in (1.1) to the case of r-free integers and showed that for
$r\geq 3,$
$$ \begin{align*} S_{\mu_r}(x)=\sum_{n=1}^\infty \frac{\mu_r(n)}{n(n+1)}x+O(x^{\theta_r}),\quad \theta_r:=\frac{r+1}{3r+1}, \end{align*} $$
where
$\mu _r(n)$
is the characteristic function of the set of r-free numbers. Very recently, Heyman [Reference Heyman10] considered the floor function set
$$ \begin{align} S(x):=\bigg\{\bigg\lfloor \frac{x}{n} \bigg\rfloor :1\leq n\leq x\bigg\} \end{align} $$
and studied the number of primes in
$S(x)$
. Our objective here is to investigate how the r-free integers are distributed over the set
$S(x)$
, that is, to ask for an asymptotic estimate for the function
$$ \begin{align} T_{\mu_r}(x):=\sum_{m\in S(x)}\mu_r(m). \end{align} $$
There first arises the question whether the sum (1.3) is identical or related to the sum
$$ \begin{align} S_{\mu_r}(x):=\sum_{n\leq x}\mu_r\bigg( \bigg\lfloor\frac{x}{n}\bigg\rfloor \bigg). \end{align} $$
To answer this question, we rewrite the sum (1.3) as
$$ \begin{align*} T_{\mu_r}(x)=\sum_{\substack{m\leq x\\ \exists n\in \mathbb{N} \text{ such that }\lfloor x/n \rfloor=m}}\mu_r(m). \end{align*} $$
Observe that each argument appears once in the sum (1.3), but usually appears several times in the sum (1.4), as seen in the following example. Taking
$x=20$
and
$r=2$
, for the sum (1.4), we get
$$ \begin{align*} &S_{\mu_2}(20)=\sum_{n\leq 20}\mu_2\bigg( \bigg\lfloor\frac{20}{n}\bigg\rfloor \bigg)\\ &=\mu_2(20)+\mu_2(10)+\mu_2(6)+\mu_2(5)+\mu_2(4)+\mu_2(3)+\mu_2(2)+\mu_2(2)+\mu_2(2)+\mu_2(2)\\ &\quad +\mu_2(1)+\mu_2(1)+\mu_2(1)+\mu_2(1)+\mu_2(1)+\mu_2(1)+\mu_2(1)+\mu_2(1)+\mu_2(1)+\mu_2(1)\\ &=\mu_2(20)+\mu_2(10)+\mu_2(6)+\mu_2(5)+\mu_2(4)+\mu_2(3)+4\mu_2(2)+10\mu_2(1)=18, \end{align*} $$
while for the sum (1.3), we get
$S(20)=\{\lfloor {20}/{n} \rfloor :1\leq n\leq 20\}=\{1, 2, 3, 4, 5, 6, 10, 20\}$
and
$$ \begin{align*} T_{\mu_2}(20)&=\sum_{m\in S(20)}\mu_2(m) \\ &=\mu_2(20)+\mu_2(10)+\mu_2(6)+\mu_2(5)+\mu_2(4)+\mu_2(3)+\mu_2(2)+\mu_2(1) =6. \end{align*} $$
Our first main result is proved using the following estimate of Yu and Wu [Reference Yu and Wu20].
Lemma 1.1 [Reference Yu and Wu20].
Let
$x\in \mathbb {R},\ x>0$
, let
$q, a\in \mathbb {Z}$
such that
$0\leq a<q\leq x^{1/4}\log ^{-3/2} x$
and let
$S(x)$
be as defined in (1.2). Then,
$$ \begin{align*} \sum_{\substack{n\in S(x)\\ n \, \equiv\, a\ ({\mathrm{mod }}\, q)}}1=\frac{2x^{1/2}}{q }+ O \bigg(\frac{x^{1/3}}{q^{1/3}}\log x\bigg). \end{align*} $$
Remark 1.2. For real large x, let
$S(x)$
be as defined in (1.2). We shall need the estimate
$\lvert {S(x)}\rvert =O(x^{1/2})$
in our proofs. To verify this, note that for
$n\in \{1,2,\ldots \lfloor x\rfloor \}$
:
-
• if
$\lfloor x/n \rfloor =1$
, then
$n\leq x$
; -
• if
$\lfloor x/n \rfloor =2$
, then
$n\leq x/2$
;⋮
-
• if
$\lfloor x/n \rfloor =\lfloor x^{1/2}\rfloor $
, then
$n< x^{1/2}$
.
It follows that for
$n<x^{1/2}$
, the function
$\lfloor x/n\rfloor $
takes at most
$\lfloor x^{1/2}\rfloor $
distinct integral values. However, if
$n\geq x^{1/2}$
, the function
$\lfloor x/n\rfloor \leq x^{1/2}$
can then take at most
$\lfloor x^{1/2}\rfloor $
integral values. Thus,
$\lvert {S(x)}\rvert \leq 2\lfloor x^{1/2}\rfloor =O(x^{1/2})$
.
Our first theorem reads as follows.
Theorem 1.3. Let
$S(x)$
and
$T_{\mu _r}$
be as defined in (1.2) and (1.3). Then,
$$ \begin{align*} T_{\mu_r}(x)=\frac{2x^{1/2}}{\zeta(r)}+\begin{cases} O(x^{3/8}\log^{3/4} x) & \text{for } r=2, \\ O(x^{1/3}\log x) & \text{for } r\geq3.\\ \end{cases} \end{align*} $$
Regarding our second main result, very recently, Zhang [Reference Zhang22] improved the results of Bordellés (1.1), Stuctky [Reference Stucky16] and Liu et al. [Reference Liu, Wu and Yang12] by proving using the exponent pair method, that
$$ \begin{align*} S_{\mu_r}(x)=x\sum_{n=1}^\infty \frac{\mu_r(n)}{n(n+1)}+\begin{cases} O(x^{11/29}\log^{2} x) & \text{for } r=2, \\ O(x^{1/3}\log^2 x) & \text{for } r=3,\\ O(x^{1/3}\log x), & \text{for } r\geq 3. \end{cases} \end{align*} $$
We use the same idea as in [Reference Zhang22] to give another formula, which, in the case
$r=2$
, is slightly weaker than that in Theorem 1.3.
Theorem 1.4. For an exponent pair
$(\kappa ,\lambda )$
such that
${1}/{2}<{\lambda }/{(1+\kappa )}$
, we have
$$ \begin{align*} T_{\mu_r}(x)=\frac{2x^{1/2}}{\zeta(r)}+\begin{cases} O( x^{1/4+\lambda/4(1+\kappa)}(\log x)^{3/2-3\lambda/2(1+\kappa)}) & \text{for } r=2, \\ O(x^{1/3}\log x) & \text{for } r\geq3. \end{cases} \end{align*} $$
We note in passing that a result better than that in Theorem 1.4 for the case
$r=2$
can be derived, assuming the Riemann hypothesis, by taking the exponent pair
$(\kappa , \lambda )=(2/7, 4/7)$
to get
$$ \begin{align*} T_{\mu_2}(x)=\frac{2x^{1/2}}{\zeta(2)}+ O( x^{13/36}(\log x)^{1/6} ). \end{align*} $$
Under the Riemann hypothesis, we can omit the restriction on the exponent pair
$(\kappa ,\lambda )$
such that
${1}/{2}<{\lambda }/{(1+\kappa )}$
and obtain the following result.
Theorem 1.5. Assume the Riemann hypothesis. For an exponent pair
$(\kappa ,\lambda )$
such that
${1}/{4}<{\lambda }/{(1+\kappa )}$
, we have
$$ \begin{align*} T_{\mu_2}(x)=\frac{2x^{1/2}}{\zeta(2)}+ O( x^{1/4+\lambda/4(1+\kappa)}(\log x)^{3/2-3\lambda/2(1+\kappa)}). \end{align*} $$
2 Proofs
Proof of Theorem 1.3.
Since
$\mu _r(n)$
is the characteristic function of the set of r-free numbers, by the well-known identity
$\mu _r(n)=\sum _{d^r\mid n}\mu (d)$
, we have
$$ \begin{align*} T_{\mu_r}(x)&=\sum_{n\in S(x)}\mu_r(n)=\sum_{n\in S(x)}\sum_{d^r\mid n}\mu(d) =\sum_{d\leq x^{1/r}}\mu(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d^r\textrm{)}} }}1\\ &=\sum_{d\leq x^{1/4r}\log^{-3/2r} x}\mu(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d^r\textrm{)}} }}1+\sum_{ x^{1/4r}\log^{-3/2r} x< d\leq x^{1/r}}\mu(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d^r\textrm{)}} }}1. \end{align*} $$
Using Lemma 1.1 to compute the first sum, we have
$$ \begin{align*} \sum_{d\leq x^{1/4r}\log^{-3/2r} x}\mu(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d^r\textrm{)}} }}1 & =\sum_{d\leq x^{1/4r}\log^{-3/2r} x}\mu(d)\bigg(\frac{2x^{1/2}}{d^r }+ O \bigg(\frac{x^{1/3}}{d^{r/3}}\log x\bigg)\bigg)\\ &=\frac{2x^{1/2}}{\zeta(r)}+\begin{cases} O(x^{3/8}\log^{3/4} x) & \text{for } r=2, \\ O(x^{1/3}\log x) & \text{for } r\geq3. \end{cases} \end{align*} $$
By the remark preceding the statement of Theorem 1.3, we have
$\lvert {S(x)}\rvert =O(x^{1/2})$
and so the second sum is bounded by
$$ \begin{align*} \sum_{ x^{1/4r}\log^{-3/2r} x< d\leq x^{1/r}}\mu(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d^2\textrm{)}} }}1&=O\bigg(x^{1/2}\sum_{ x^{1/4r}\log^{-3/2r} x< d\leq x^{1/r}}\frac{1}{ d^2}\bigg)\\ &=\begin{cases} O(x^{3/8}\log^{3/4} x) & \text{for } r=2, \\ O(x^{1/4}\log^{1/2} x) & \text{for } r\geq3, \end{cases} \end{align*} $$
which completes the proof of Theorem 1.3.
Proof of Theorem 1.4.
In view of [Reference Ivić11, (14.23)],
$$ \begin{align*}\mu_r(n)=\sum_{d\mid n}g(d),\quad\text{where } g(d)=\begin{cases} \mu(\ell) & \text{if } d=\ell^r,\ \text{for some } \ell\in \mathbb{N}, \\ 0 & \text{otherwise.} \end{cases}\end{align*} $$
From the definition of
$g(d)$
,
$$ \begin{align} \sum_{d\leq x}\lvert{g(d)}\rvert=\begin{cases} O(x^{1/2}) & \text{if } r=2, \\ O(x^{1/3}) & \text{if } r\geq 3. \end{cases} \end{align} $$
Let
$(\kappa ,\lambda )$
be an exponent pair such that
${1}/{2}<{\lambda }/{(1+\kappa )}\ (< 1)$
. Trivially, from (2.1),
$$ \begin{align} \sum_{d\leq x}\lvert{g(d)}\rvert=O(x^{{\lambda}/{(1+\kappa)}}). \end{align} $$
For
$x>1$
,
$$ \begin{align*} \sum_{n\in S(x)}\mu_r(n)&=\sum_{n\in S(x)}\sum_{d\mid n}g(d)=\sum_{d\leq x}g(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d\textrm{)}}} }1\\ &=\sum_{d\leq x^{1/4}\log^{-3/2} x}g(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d\textrm{)}}} }1+\sum_{ x^{1/4}\log^{-3/2} x< d\leq x}g(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d\textrm{)}} }}1. \end{align*} $$
We use Lemma 1.1 to compute the first sum. We have
$$ \begin{align*} \sum_{d\leq x^{1/4}\log^{-3/2} x} g(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d\textrm{)}} }}1&=\sum_{d\leq x^{1/4}\log^{-3/2} x}g(d)\bigg(\frac{2x^{1/2}}{d }+ O \bigg(\frac{x^{1/3}}{d^{1/3}}\log x\bigg)\bigg)\\ &=2x^{1/2}\sum_{d\leq x^{1/4}\log^{-3/2} x}\frac{g(d)}{d }+O\bigg(x^{1/3}\log x\sum_{d\leq x^{1/4}\log^{-3/2} x} \frac{\lvert{g(d)}\rvert}{d^{1/3} }\bigg). \end{align*} $$
Denote the first and the second sums on the right-hand side by
$\Sigma _1$
and
$\Sigma _2$
, respectively. Using partial summation (or Abel’s identity [Reference Apostol2, Theorem 4.2]) and (2.2),
$$ \begin{align*} \sum_{d\leq x^{1/4}\log^{-3/2} x}\frac{g(d)}{d }&=\sum_{d=1}^\infty \frac{g(d)}{d }-\sum_{d> x^{1/4}\log^{-3/2} x}\frac{g(d)}{d } =\sum_{d=1}^\infty \frac{g(d)}{d }+O \bigg(\sum_{d> x^{1/4}\log^{-3/2} x}\frac{\lvert{g(d)}\rvert}{d }\bigg) \\ &=\sum_{d=1}^\infty \frac{g(d)}{d }+O( x^{-1/4+\lambda/4(1+\kappa)}(\log x)^{3/2-3\lambda/2(1+\kappa)} ) \end{align*} $$
and so
$$ \begin{align*} \Sigma_1=2x^{1/2}\sum_{d=1}^\infty \frac{g(d)}{d }+O( x^{1/4+\lambda/4(1+\kappa)}(\log x)^{3/2-3\lambda/2(1+\kappa)} ). \end{align*} $$
Again from (2.2), by Abel’s identity,
$$ \begin{align*} \sum_{d\leq x^{1/4}\log^{-3/2} x}\frac{\lvert{g(d)}\rvert}{d^{1/3} }\ll x^{-1/12+\lambda/4(1+\kappa)}(\log x)^{1/2-3\lambda/2(1+\kappa)} \end{align*} $$
and so
$$ \begin{align*} \Sigma_2=O( x^{1/4+\lambda/4(1+\kappa)}(\log x)^{3/2-3\lambda/2(1+\kappa)} ). \end{align*} $$
Then,
$$ \begin{align*} \sum_{d\leq x^{1/4}\log^{-3/2} x}g(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d\textrm{)}}} }1=2x^{1/2}\sum_{d=1}^\infty \frac{g(d)}{d }+O( x^{1/4+\lambda/4(1+\kappa)}(\log x)^{3/2-3\lambda/2(1+\kappa)} ). \end{align*} $$
Next, we bound the second sum. Using
$\lvert {S(x)}\rvert =O(x^{1/2})$
,
$$ \begin{align*} \sum_{ x^{1/4}\log^{-3/2} x< d\leq x}g(d)\sum_{\substack{n\in S(x)\\ n \, \equiv\, {0\, \textrm{(mod} \; d\textrm{)}}} }1=O\bigg(x^{1/2}\sum_{ x^{1/4}\log^{-3/2} x< d\leq x}\frac{\lvert{g(d)}\rvert}{d}\bigg). \end{align*} $$
By Abel’s identity and (2.2), we arrive at
$$ \begin{align*} x^{1/2}\sum_{ x^{1/4}\log^{-3/2} x< d\leq x}\frac{\lvert{g(d)}\rvert}{d}\ll x^{1/4+\lambda/4(1+\kappa)}(\log x)^{3/2-3\lambda/2(1+\kappa)}, \end{align*} $$
and Theorem 1.4 follows.
Proof of Theorem 1.5.
The proof is the same as the proof of Theorem 1.4. We assume the Riemann hypothesis. Thus, we can replace (2.1) by
$$ \begin{align} \sum_{d\leq x}\lvert{g(d)}\rvert=O(x^{{1}/{4}+\epsilon}). \end{align} $$
Using (2.3), we choose the exponent pairs
$(\kappa ,\lambda )$
such that
${1}/{4}<{\lambda }/{(1+\kappa )}$
. The result follows.
