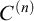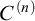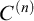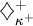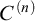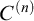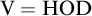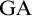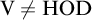1 Introduction
The present paper is a contribution to the long-standing program in set theory of studying the robustness of strong large-cardinal notions under forcing extensions. Specifically, we are interested in the section of the large-cardinal hierarchy ranging between extendible cardinals and Vopěnka’s Principle (
![]() $\mathrm {VP}$
).
$\mathrm {VP}$
).
In a groundbreaking work, Laver [Reference Laver19] proved that supercompactness, one of the most prominent large-cardinal properties, can be made indestructible under a wide range of forcing notions. Indeed, given a supercompact cardinal
![]() $\kappa $
, Laver showed that there is a forcing notion (the Laver preparation) that preserves the supercompactness of
$\kappa $
, Laver showed that there is a forcing notion (the Laver preparation) that preserves the supercompactness of
![]() $\kappa $
and makes it indestructible under further
$\kappa $
and makes it indestructible under further
![]() ${<}\kappa $
-directed closed forcing.
${<}\kappa $
-directed closed forcing.
Inspired by the work of Laver, several authors subsequently obtained similar results for other classical large-cardinal notions. For instance, Gitik and Shelah [Reference Gitik and Shelah13] show that a strong cardinal
![]() $\kappa $
can be made indestructible under so-called
$\kappa $
can be made indestructible under so-called
![]() $\kappa ^+$
-weakly closed forcing satisfying the Prikry condition; Hamkins [Reference Hamkins15] uses the lottery preparation forcing to make various types of large cardinals indestructible under appropriate forcing notions. More recently, Brooke-Taylor [Reference Brooke-Taylor8] shows that
$\kappa ^+$
-weakly closed forcing satisfying the Prikry condition; Hamkins [Reference Hamkins15] uses the lottery preparation forcing to make various types of large cardinals indestructible under appropriate forcing notions. More recently, Brooke-Taylor [Reference Brooke-Taylor8] shows that
![]() $\mathrm {VP}$
is indestructible under reverse Easton forcing iterations of increasingly directed-closed forcing notions, without the need for any preparatory forcing. In the present paper we are concerned with the preservation by forcing of
$\mathrm {VP}$
is indestructible under reverse Easton forcing iterations of increasingly directed-closed forcing notions, without the need for any preparatory forcing. In the present paper we are concerned with the preservation by forcing of
![]() $C^{(n)}$
-extendible cardinals. This family of large cardinals was introduced in [Reference Bagaria, Casacuberta, Mathias and Rosickỳ2] (see also [Reference Bagaria1]) as a strengthening of the classical notion of extendibility and was shown to provide natural milestones on the road from supercompact cardinals up to
$C^{(n)}$
-extendible cardinals. This family of large cardinals was introduced in [Reference Bagaria, Casacuberta, Mathias and Rosickỳ2] (see also [Reference Bagaria1]) as a strengthening of the classical notion of extendibility and was shown to provide natural milestones on the road from supercompact cardinals up to
![]() $\mathrm {VP}$
. Extendible cardinals have experienced a renewed interest after Woodin’s proof of the HOD-Dichotomy [Reference Woodin29]. Also,
$\mathrm {VP}$
. Extendible cardinals have experienced a renewed interest after Woodin’s proof of the HOD-Dichotomy [Reference Woodin29]. Also,
![]() $C^{(n)}$
-extendible cardinals have had remarkable applications in category theory and algebraic topology (see [Reference Bagaria, Casacuberta, Mathias and Rosickỳ2]). Thus, the investigation of the preservation of such cardinals under forcing is a worthwhile project, which may lead to further applications.
$C^{(n)}$
-extendible cardinals have had remarkable applications in category theory and algebraic topology (see [Reference Bagaria, Casacuberta, Mathias and Rosickỳ2]). Thus, the investigation of the preservation of such cardinals under forcing is a worthwhile project, which may lead to further applications.
Recall (see [Reference Bagaria1]) that, for each
![]() $n<\omega $
, the class
$n<\omega $
, the class
![]() $C^{(n)}$
is the
$C^{(n)}$
is the
![]() $\Pi _n$
-definable closed unbounded proper class of all ordinals
$\Pi _n$
-definable closed unbounded proper class of all ordinals
![]() $\alpha $
that are
$\alpha $
that are
![]() $\Sigma _n$
-correct, i.e., such that
$\Sigma _n$
-correct, i.e., such that
![]() $V_\alpha $
is a
$V_\alpha $
is a
![]() $\Sigma _n$
-elementary substructure of
$\Sigma _n$
-elementary substructure of
![]() $\mathrm {V}$
. Also, recall that a cardinal
$\mathrm {V}$
. Also, recall that a cardinal
![]() $\kappa $
is
$\kappa $
is
![]() $C^{(n)}$
-extendible if for every
$C^{(n)}$
-extendible if for every
![]() $\lambda>\kappa $
there is an ordinal
$\lambda>\kappa $
there is an ordinal
![]() $\mu $
and an elementary embedding
$\mu $
and an elementary embedding
![]() $j:V_\lambda \to V_\mu $
such that
$j:V_\lambda \to V_\mu $
such that
![]() $\mathrm {crit}(j)=\kappa $
,
$\mathrm {crit}(j)=\kappa $
,
![]() $j(\kappa )>\lambda $
, and
$j(\kappa )>\lambda $
, and
![]() $j(\kappa )\in C^{(n)}$
.
$j(\kappa )\in C^{(n)}$
.
It turns out that
![]() $\mathrm {VP}(\Pi _{n+1})$
, namely
$\mathrm {VP}(\Pi _{n+1})$
, namely
![]() $\mathrm {VP}$
restricted to classes of structures that are
$\mathrm {VP}$
restricted to classes of structures that are
![]() $\Pi _{n+1}$
-definable, is equivalent to the existence of a
$\Pi _{n+1}$
-definable, is equivalent to the existence of a
![]() $C^{(n)}$
-extendible cardinal. Hence
$C^{(n)}$
-extendible cardinal. Hence
![]() $\mathrm {VP}$
is equivalent to the existence of a
$\mathrm {VP}$
is equivalent to the existence of a
![]() $C^{(n)}$
-extendible cardinal for each
$C^{(n)}$
-extendible cardinal for each
![]() $n\geq 1$
(see [Reference Bagaria1] for details). It is in this sense that
$n\geq 1$
(see [Reference Bagaria1] for details). It is in this sense that
![]() $C^{(n)}$
-extendible cardinals are canonical representatives of the large-cardinal hierarchy in the region between the first supercompact cardinal and
$C^{(n)}$
-extendible cardinals are canonical representatives of the large-cardinal hierarchy in the region between the first supercompact cardinal and
![]() $\mathrm {VP}$
.
$\mathrm {VP}$
.
In general, the preservation of very large cardinals by forcing is a delicate issue since it imposes strong forms of agreement between the ground model and the generic forcing extension. For example, suppose
![]() $\kappa \in C^{(n)}$
is inaccessible and
$\kappa \in C^{(n)}$
is inaccessible and
![]() $\mathbb {P}$
is a
$\mathbb {P}$
is a
![]() ${<}\kappa $
-distributive forcing notion. If
${<}\kappa $
-distributive forcing notion. If
![]() $\Vdash _{\mathbb {P}}\text {"}\kappa \in \dot {C}^{(n)}\text {"}$
then
$\Vdash _{\mathbb {P}}\text {"}\kappa \in \dot {C}^{(n)}\text {"}$
then
![]() $V_\kappa \prec _{\Sigma _n} V^{\mathbb {P}}$
. This underlines the fact that the more correct a large cardinal is, the harder it is to preserve its correctness under forcing, and therefore the more fragile it becomes. Indeed, one runs into trouble when seeking a result akin to Laver’s indestructibility for supercompact cardinals for stronger large cardinals such as extendible. This phenomenon was first pointed out by Tsaprounis in his Ph.D. thesis [Reference Tsaprounis26] and it was afterwards extensively studied in [Reference Bagaria, Hamkins, Tsaprounis and Usuba4], where the following theorem illustrates the fragility we just described.
$V_\kappa \prec _{\Sigma _n} V^{\mathbb {P}}$
. This underlines the fact that the more correct a large cardinal is, the harder it is to preserve its correctness under forcing, and therefore the more fragile it becomes. Indeed, one runs into trouble when seeking a result akin to Laver’s indestructibility for supercompact cardinals for stronger large cardinals such as extendible. This phenomenon was first pointed out by Tsaprounis in his Ph.D. thesis [Reference Tsaprounis26] and it was afterwards extensively studied in [Reference Bagaria, Hamkins, Tsaprounis and Usuba4], where the following theorem illustrates the fragility we just described.
Theorem 1.1 [Reference Bagaria, Hamkins, Tsaprounis and Usuba4].
Suppose that
![]() $V_\kappa \prec _{\Sigma _2} V_\lambda $
and
$V_\kappa \prec _{\Sigma _2} V_\lambda $
and
![]() $G\subseteq \mathbb {P}$
is a
$G\subseteq \mathbb {P}$
is a
![]() $\mathrm {V}$
-generic filter for nontrivial strategically
$\mathrm {V}$
-generic filter for nontrivial strategically
![]() ${<}\kappa $
-closed forcing
${<}\kappa $
-closed forcing
![]() $\mathbb {P}\in V_\eta $
, where
$\mathbb {P}\in V_\eta $
, where
![]() $\eta \leq \lambda $
. Then for every
$\eta \leq \lambda $
. Then for every
![]() $\theta \geq \eta $
,
$\theta \geq \eta $
,
In particular, if
![]() $\kappa $
is an extendible cardinal and
$\kappa $
is an extendible cardinal and
![]() $\mathbb {P}$
is any nontrivial strategically
$\mathbb {P}$
is any nontrivial strategically
![]() ${<}\kappa $
-closed set forcing notion, then forcing with
${<}\kappa $
-closed set forcing notion, then forcing with
![]() $\mathbb {P}$
destroys the extendibility of
$\mathbb {P}$
destroys the extendibility of
![]() $\kappa $
. Moreover, the theorem implies that there is no hope to obtain indestructibility results for
$\kappa $
. Moreover, the theorem implies that there is no hope to obtain indestructibility results for
![]() $\Sigma _3$
-correct large cardinals. Thus, if one aims for a general theory of preservation of
$\Sigma _3$
-correct large cardinals. Thus, if one aims for a general theory of preservation of
![]() $C^{(n)}$
-extendible cardinals one should concentrate on class forcing notions.
$C^{(n)}$
-extendible cardinals one should concentrate on class forcing notions.
The structure of the paper is as follows: In Section 2 we prove that
![]() $C^{(n)}$
-extendible cardinals are uniformly characterizable in a Magidor-like way, i.e., similar to Magidor’s characterization of supercompact cardinals. This reinforces the fact that
$C^{(n)}$
-extendible cardinals are uniformly characterizable in a Magidor-like way, i.e., similar to Magidor’s characterization of supercompact cardinals. This reinforces the fact that
![]() $C^{(n)}$
-extendible cardinals are a natural model-theoretic strengthening of supercompactness, first shown in [Reference Bagaria, Casacuberta, Mathias and Rosickỳ2] (see also [Reference Bagaria1]). This characterization of
$C^{(n)}$
-extendible cardinals are a natural model-theoretic strengthening of supercompactness, first shown in [Reference Bagaria, Casacuberta, Mathias and Rosickỳ2] (see also [Reference Bagaria1]). This characterization of
![]() $C^{(n)}$
-extendibility is used in later sections for carrying out preservation arguments under class forcing. The same characterization has been independently given by Boney in [Reference Boney6], and also in [Reference Bagaria, Gitman and Schindler3] for the virtual forms of higher-level analogs of supercompact cardinals (i.e., n-remarkable cardinals) and virtual
$C^{(n)}$
-extendibility is used in later sections for carrying out preservation arguments under class forcing. The same characterization has been independently given by Boney in [Reference Boney6], and also in [Reference Bagaria, Gitman and Schindler3] for the virtual forms of higher-level analogs of supercompact cardinals (i.e., n-remarkable cardinals) and virtual
![]() $C^{(n)}$
-extendible cardinals.
$C^{(n)}$
-extendible cardinals.
Section 3 is devoted to the analysis of some reflection properties of class forcing iterations that will be useful in later sections for the study of the preservation of
![]() $C^{(n)}$
-extendible cardinals. Two key notions are that of adequate iteration and
$C^{(n)}$
-extendible cardinals. Two key notions are that of adequate iteration and
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _k$
-reflecting cardinal. For the same purpose, in Section 4 we introduce the notion of
$\Sigma _k$
-reflecting cardinal. For the same purpose, in Section 4 we introduce the notion of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _k$
-supercompact cardinal and show how it relates to
$\Sigma _k$
-supercompact cardinal and show how it relates to
![]() $C^{(n)}$
-extendible cardinals.
$C^{(n)}$
-extendible cardinals.
In Section 5 we define the notion of suitable iteration and prove a general result about the preservation of
![]() $C^{(n)}$
-extendible cardinals under a wide class of
$C^{(n)}$
-extendible cardinals under a wide class of
![]() $\mathrm {ORD}$
-length forcing iterations (cf. Theorem 5.4). The prize we pay for considering such general iterations is that we just prove that any
$\mathrm {ORD}$
-length forcing iterations (cf. Theorem 5.4). The prize we pay for considering such general iterations is that we just prove that any
![]() $C^{(n+1)}$
-extendible cardinal retains its
$C^{(n+1)}$
-extendible cardinal retains its
![]() $C^{(n)}$
-extendibility (cf. Corollary 5.14).
$C^{(n)}$
-extendibility (cf. Corollary 5.14).
Section 6 is focussed on applications of Theorem 5.4. We give a new proof of Brooke-Taylor’s theorem on the preservation of
![]() $\mathrm {VP}$
[Reference Brooke-Taylor8]. The main advantage with respect to the original proof is that our technique allows for a finer control over the amount of Vopěnka’s Principle that is preserved.
$\mathrm {VP}$
[Reference Brooke-Taylor8]. The main advantage with respect to the original proof is that our technique allows for a finer control over the amount of Vopěnka’s Principle that is preserved.
In Section 7 we concentrate on a more concrete class of forcing iterations we call fitting and improve the results obtained in Section 5. Our main result here is Theorem 7.5. In Section 8 we give several applications of this theorem. First, we show that
![]() $C^{(n)}$
-extendible cardinals are preserved by standard Easton class-forcing iterations for any
$C^{(n)}$
-extendible cardinals are preserved by standard Easton class-forcing iterations for any
![]() $\Delta _2$
-definable possible behaviour of the power-set function on regular cardinals. This extends the main result of [Reference Tsaprounis28]. Second, with an eye on Woodin’s HOD Conjecture, we explore briefly the connections between
$\Delta _2$
-definable possible behaviour of the power-set function on regular cardinals. This extends the main result of [Reference Tsaprounis28]. Second, with an eye on Woodin’s HOD Conjecture, we explore briefly the connections between
![]() $C^{(n)}$
-extendible cardinals (and thus also
$C^{(n)}$
-extendible cardinals (and thus also
![]() $\mathrm {VP}$
) with the principle
$\mathrm {VP}$
) with the principle
![]() $\mathrm {V}=\mathrm {HOD}$
. In particular, we prove that it is possible to force a complete disagreement, and in many possible forms, between
$\mathrm {V}=\mathrm {HOD}$
. In particular, we prove that it is possible to force a complete disagreement, and in many possible forms, between
![]() $\mathrm {V}$
and
$\mathrm {V}$
and
![]() $\mathrm {HOD}$
with respect to the calculation of successors of regular cardinals, while
$\mathrm {HOD}$
with respect to the calculation of successors of regular cardinals, while
![]() $C^{(n)}$
-extendible cardinals are preserved. Third, we show that, assuming the GCH, the class forcing iteration of Cummings–Foreman–Magidor that forces
$C^{(n)}$
-extendible cardinals are preserved. Third, we show that, assuming the GCH, the class forcing iteration of Cummings–Foreman–Magidor that forces
![]() $\diamondsuit _{\kappa ^+}^+$
at every
$\diamondsuit _{\kappa ^+}^+$
at every
![]() $\kappa $
([Reference Cummings, Foreman and Magidor10]) preserves
$\kappa $
([Reference Cummings, Foreman and Magidor10]) preserves
![]() $C^{(n)}$
-extendible cardinals. Finally, we prove that
$C^{(n)}$
-extendible cardinals. Finally, we prove that
![]() $C^{(n)}$
-extendible cardinals are consistent with
$C^{(n)}$
-extendible cardinals are consistent with
![]() $\square _{\lambda ,\mathrm {cof}(\lambda )}$
for a proper class of singular cardinals
$\square _{\lambda ,\mathrm {cof}(\lambda )}$
for a proper class of singular cardinals
![]() $\lambda $
. This result is optimal in the sense explained in Section 8.4.
$\lambda $
. This result is optimal in the sense explained in Section 8.4.
In Section 9, we address the question of the preservation of
![]() $C^{(n)}$
-extendible cardinals under general (non-weakly homogeneous, non-definable) suitable iterations. As applications of our analysis we prove the consistency of
$C^{(n)}$
-extendible cardinals under general (non-weakly homogeneous, non-definable) suitable iterations. As applications of our analysis we prove the consistency of
![]() $C^{(n)}$
-extendible cardinals with
$C^{(n)}$
-extendible cardinals with
![]() $\mathrm {V}=\mathrm {HOD}$
, and also with
$\mathrm {V}=\mathrm {HOD}$
, and also with
![]() $\mathrm {GA}+\mathrm {V}\neq \mathrm {HOD}$
. This latter is a strengthening of a result of Hamkins, Reitz, and Woodin [Reference Hamkins, Reitz and Woodin14].
$\mathrm {GA}+\mathrm {V}\neq \mathrm {HOD}$
. This latter is a strengthening of a result of Hamkins, Reitz, and Woodin [Reference Hamkins, Reitz and Woodin14].
2 A Magidor-like characterization of
 $C^{(n)}$
-extendibility
$C^{(n)}$
-extendibility
We shall prove that
![]() $C^{(n)}$
-extendible cardinals (defined in page 6 of the Introduction) can be characterized in a way analogous to the following characterization of supercompact cardinals due to Magidor.Footnote
1
$C^{(n)}$
-extendible cardinals (defined in page 6 of the Introduction) can be characterized in a way analogous to the following characterization of supercompact cardinals due to Magidor.Footnote
1
Theorem 2.1 [Reference Magidor21].
For a cardinal
![]() $\delta $
, the following statements are equivalent
$\delta $
, the following statements are equivalent
![]() $:$
$:$
-
(1)
 $\delta $
is a supercompact cardinal.
$\delta $
is a supercompact cardinal. -
(2) For every
 $\lambda>\delta $
in
$\lambda>\delta $
in
 $C^{(1)}$
and for every
$C^{(1)}$
and for every
 $a\in V_\lambda $
, there exist ordinals
$a\in V_\lambda $
, there exist ordinals
 $\bar {\delta }<\bar {\lambda }<\delta $
and there exist some
$\bar {\delta }<\bar {\lambda }<\delta $
and there exist some
 $\bar {a}\in V_{\bar {\lambda }}$
and an elementary embedding
$\bar {a}\in V_{\bar {\lambda }}$
and an elementary embedding
 $j:V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that
$j:V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that
 $:$
$:$
-
•
 $\mathrm {crit}(j)=\bar {\delta }$
and
$\mathrm {crit}(j)=\bar {\delta }$
and
 $j(\bar {\delta })=\delta $
.
$j(\bar {\delta })=\delta $
. -
•
 $j(\bar {a})=a$
.
$j(\bar {a})=a$
. -
•
 ${\bar {\lambda }}\in C^{(1)}$
.
${\bar {\lambda }}\in C^{(1)}$
.
-
The existence of a supercompact cardinal is thus characterized by a form of reflection for
![]() $\Sigma _1$
-correct strata of the universe, for it implies that any
$\Sigma _1$
-correct strata of the universe, for it implies that any
![]() $\Sigma _1$
-truth (i.e., any
$\Sigma _1$
-truth (i.e., any
![]() $\Sigma _1$
sentence, with parameters, true in
$\Sigma _1$
sentence, with parameters, true in
![]() $\mathrm {V}$
) is captured (up to some change of parameters) by some level below the supercompact cardinal. The following notion generalizes this reflection property to higher levels of complexity.
$\mathrm {V}$
) is captured (up to some change of parameters) by some level below the supercompact cardinal. The following notion generalizes this reflection property to higher levels of complexity.
Definition 2.2 (
 $\Sigma _n$
-supercompact cardinal).
$\Sigma _n$
-supercompact cardinal).
Let
![]() $n\geq 1$
. A cardinal
$n\geq 1$
. A cardinal
![]() $\delta $
is said to be
$\delta $
is said to be
![]() $\Sigma _n$
-supercompact if for every
$\Sigma _n$
-supercompact if for every
![]() $\lambda>\delta $
in
$\lambda>\delta $
in
![]() $C^{(n)}$
and
$C^{(n)}$
and
![]() $a\in V_\lambda $
, there exist
$a\in V_\lambda $
, there exist
![]() $\bar {\delta }<\bar {\lambda }<\delta $
and
$\bar {\delta }<\bar {\lambda }<\delta $
and
![]() $\bar {a}\in V_{\bar {\lambda }}$
, and there exists an elementary embedding
$\bar {a}\in V_{\bar {\lambda }}$
, and there exists an elementary embedding
![]() $j:V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that:
$j:V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that:
-
•
 $\mathrm {crit}(j)=\bar {\delta }$
and
$\mathrm {crit}(j)=\bar {\delta }$
and
 $j(\bar {\delta })=\delta $
.
$j(\bar {\delta })=\delta $
. -
•
 $j(\bar {a})=a$
.
$j(\bar {a})=a$
. -
•
 ${\bar {\lambda }}\in C^{(n)}$
.
${\bar {\lambda }}\in C^{(n)}$
.
Lemma 2.3. For
![]() $n\geq 1$
, every
$n\geq 1$
, every
![]() $\Sigma _n$
-supercompact cardinal belongs to the class
$\Sigma _n$
-supercompact cardinal belongs to the class
![]() $C^{(n+1)}$
.
$C^{(n+1)}$
.
Proof We prove the lemma by induction on
![]() $n\geq 1$
. The base case
$n\geq 1$
. The base case
![]() $n=1$
follows by combining Magidor’s theorem (cf. Theorem 2.1) with the well-known fact that supercompact cardinals are
$n=1$
follows by combining Magidor’s theorem (cf. Theorem 2.1) with the well-known fact that supercompact cardinals are
![]() $\Sigma _2$
-correct [Reference Kanamori18, Proposition 22.3]. Thus, we shall assume by induction that the lemma holds for n and prove it for
$\Sigma _2$
-correct [Reference Kanamori18, Proposition 22.3]. Thus, we shall assume by induction that the lemma holds for n and prove it for
![]() $n+1$
.
$n+1$
.
Let
![]() $\delta $
be a
$\delta $
be a
![]() $\Sigma _{n+1}$
-supercompact cardinal, and let
$\Sigma _{n+1}$
-supercompact cardinal, and let
![]() $\varphi (x,y)$
be a
$\varphi (x,y)$
be a
![]() $\Pi _{n+1}$
formula with
$\Pi _{n+1}$
formula with
![]() $a\in V_\delta $
. Suppose first that
$a\in V_\delta $
. Suppose first that
![]() $V_\delta $
satisfies the sentence
$V_\delta $
satisfies the sentence
![]() $\exists x\varphi (x,a)$
and let
$\exists x\varphi (x,a)$
and let
![]() $b\in V_\delta $
be a witness for it. Since
$b\in V_\delta $
be a witness for it. Since
![]() $\delta $
is
$\delta $
is
![]() $\Sigma _n$
-supercompact, the induction hypothesis guarantees that
$\Sigma _n$
-supercompact, the induction hypothesis guarantees that
![]() $\delta \in C^{(n+1)}$
, so that
$\delta \in C^{(n+1)}$
, so that
![]() $\varphi (b,a)$
is true.
$\varphi (b,a)$
is true.
Conversely, suppose that
![]() $\exists x\varphi (x,a)$
is true. Let
$\exists x\varphi (x,a)$
is true. Let
![]() $\lambda <\delta $
be such that
$\lambda <\delta $
be such that
![]() $a\in V_\lambda $
. Let b be a witness for this formula and let
$a\in V_\lambda $
. Let b be a witness for this formula and let
![]() $\mu \in C^{(n+2)}\setminus \delta ^+$
be such that
$\mu \in C^{(n+2)}\setminus \delta ^+$
be such that
![]() $b\in V_\mu $
. Then,
$b\in V_\mu $
. Then,
![]() $V_\mu \models \exists x\varphi (x,a)$
. By the
$V_\mu \models \exists x\varphi (x,a)$
. By the
![]() $\Sigma _{n+1}$
-supercompactness of
$\Sigma _{n+1}$
-supercompactness of
![]() $\delta $
we may find
$\delta $
we may find
![]() $\bar {\lambda },\bar {\delta }<\bar {\mu }<\delta $
,
$\bar {\lambda },\bar {\delta }<\bar {\mu }<\delta $
,
![]() $\bar {\mu }\in C^{(n+2)}$
,
$\bar {\mu }\in C^{(n+2)}$
,
![]() $\bar {a}\in V_{\overline {\lambda }}$
, and
$\bar {a}\in V_{\overline {\lambda }}$
, and
![]() $j:V_{\bar {\mu }}\rightarrow V_\mu $
such that
$j:V_{\bar {\mu }}\rightarrow V_\mu $
such that
![]() $\mathrm {crit}(j)=\bar {\delta }$
,
$\mathrm {crit}(j)=\bar {\delta }$
,
![]() $j(\bar {\delta })=\delta $
,
$j(\bar {\delta })=\delta $
,
![]() $j(\bar {\lambda })=\lambda $
, and
$j(\bar {\lambda })=\lambda $
, and
![]() $j(\bar {a})=a$
. In particular,
$j(\bar {a})=a$
. In particular,
![]() $V_{\bar {\mu }}\models \exists x\varphi (x,\bar {a})$
. Notice that, by elementarity,
$V_{\bar {\mu }}\models \exists x\varphi (x,\bar {a})$
. Notice that, by elementarity,
![]() $\bar {\lambda }<\bar {\delta }$
, hence
$\bar {\lambda }<\bar {\delta }$
, hence
![]() $a=j(\bar {a})=\bar {a}$
, and thus
$a=j(\bar {a})=\bar {a}$
, and thus
![]() $V_{\bar {\mu }}\models \exists x\varphi (x,a)$
. Finally, since
$V_{\bar {\mu }}\models \exists x\varphi (x,a)$
. Finally, since
![]() $\bar {\mu }<\delta $
and
$\bar {\mu }<\delta $
and
![]() $\bar {\mu }\in C^{(n+2)}$
, our induction hypothesis yields
$\bar {\mu }\in C^{(n+2)}$
, our induction hypothesis yields
![]() $V_{\bar {\mu }}\prec _{\Sigma _{n+1}}V_\delta $
. From this latter assertion it is clear that
$V_{\bar {\mu }}\prec _{\Sigma _{n+1}}V_\delta $
. From this latter assertion it is clear that
![]() $V_\delta \models \exists x\varphi (x,a)$
, as wanted.
$V_\delta \models \exists x\varphi (x,a)$
, as wanted.
Theorem 2.4. For
![]() $n\geq 1$
,
$n\geq 1$
,
![]() $\delta $
is a
$\delta $
is a
![]() $C^{(n)}$
-extendible cardinal if and only if
$C^{(n)}$
-extendible cardinal if and only if
![]() $\delta $
is
$\delta $
is
![]() $\Sigma _{n+1}$
-supercompact.
$\Sigma _{n+1}$
-supercompact.
Proof Suppose that
![]() $\delta $
is
$\delta $
is
![]() $C^{(n)}$
-extendible. Fix any
$C^{(n)}$
-extendible. Fix any
![]() $\lambda>\delta $
in
$\lambda>\delta $
in
![]() $C^{(n+1)}$
and
$C^{(n+1)}$
and
![]() $a\in V_\lambda $
. By
$a\in V_\lambda $
. By
![]() $C^{(n)}$
-extendibility, let
$C^{(n)}$
-extendibility, let
![]() $\mu>\lambda $
in
$\mu>\lambda $
in
![]() $C^{(n+1)}$
, and let
$C^{(n+1)}$
, and let
![]() $j: V_\mu \longrightarrow V_\theta $
be such that
$j: V_\mu \longrightarrow V_\theta $
be such that
![]() $\mathrm {crit}(j)=\delta $
,
$\mathrm {crit}(j)=\delta $
,
![]() $j(\delta )>\mu $
and
$j(\delta )>\mu $
and
![]() $j(\delta )\in C^{(n)}$
, for some ordinal
$j(\delta )\in C^{(n)}$
, for some ordinal
![]() $\theta $
. Notice that
$\theta $
. Notice that
![]() $j\upharpoonright V_{\lambda }\in V_\theta $
.
$j\upharpoonright V_{\lambda }\in V_\theta $
.
Claim 2.4.1.
![]() $V_\theta $
satisfies the following sentence
$V_\theta $
satisfies the following sentence
![]() $:$
$:$
 $$ \begin{align*} \exists \bar{\lambda}<j(\delta)\;\exists \bar{\delta}<\bar{\lambda}\;\exists \bar{a}\in V_{\bar{\lambda}}\;\exists j^*: V_{\bar{\lambda}}\longrightarrow V_{j(\lambda)}\nonumber\\ (j^*(\bar{a})=j(a)\;\wedge\; j^*(\bar{\delta})=j(\delta)\;\wedge\; V_{\bar{\lambda}}\prec_{\Sigma_{n+1}} V_{j(\lambda)}).\nonumber \end{align*} $$
$$ \begin{align*} \exists \bar{\lambda}<j(\delta)\;\exists \bar{\delta}<\bar{\lambda}\;\exists \bar{a}\in V_{\bar{\lambda}}\;\exists j^*: V_{\bar{\lambda}}\longrightarrow V_{j(\lambda)}\nonumber\\ (j^*(\bar{a})=j(a)\;\wedge\; j^*(\bar{\delta})=j(\delta)\;\wedge\; V_{\bar{\lambda}}\prec_{\Sigma_{n+1}} V_{j(\lambda)}).\nonumber \end{align*} $$
Proof of claim It is sufficient to show that
![]() $V_\lambda \prec _{\Sigma _{n+1}} V_{j(\lambda )}$
, for then the claim follows as witnessed by
$V_\lambda \prec _{\Sigma _{n+1}} V_{j(\lambda )}$
, for then the claim follows as witnessed by
![]() $\lambda $
,
$\lambda $
,
![]() $\delta $
, a, and
$\delta $
, a, and
![]() $j\upharpoonright V_\lambda $
.
$j\upharpoonright V_\lambda $
.
On the one hand, notice that
![]() $V_\delta \prec _{\Sigma _{n+1}} V_\mu $
, because
$V_\delta \prec _{\Sigma _{n+1}} V_\mu $
, because
![]() $C^{(n)}$
-extendible cardinals are
$C^{(n)}$
-extendible cardinals are
![]() $\Sigma _{n+2}$
-correct. By elementarity, this implies
$\Sigma _{n+2}$
-correct. By elementarity, this implies
![]() $V_{j(\delta )}\prec _{\Sigma _{n+1}} V_{\theta }$
. On the other hand, since
$V_{j(\delta )}\prec _{\Sigma _{n+1}} V_{\theta }$
. On the other hand, since
![]() $j(\delta )>\mu $
and
$j(\delta )>\mu $
and
![]() $j(\delta )\in C^{(n)}$
, it is true that
$j(\delta )\in C^{(n)}$
, it is true that
![]() $V_\mu \prec _{\Sigma _{n+1}} V_{j(\delta )}$
and thus
$V_\mu \prec _{\Sigma _{n+1}} V_{j(\delta )}$
and thus
![]() $V_\mu \prec _{\Sigma _{n+1}} V_{\theta }$
. In addition, since
$V_\mu \prec _{\Sigma _{n+1}} V_{\theta }$
. In addition, since
![]() $\mu $
and
$\mu $
and
![]() $\lambda $
were both
$\lambda $
were both
![]() $\Sigma _{n+1}$
-correct, it is the case that
$\Sigma _{n+1}$
-correct, it is the case that
![]() $V_\lambda \prec _{\Sigma _{n+1}} V_\mu $
. Hence,
$V_\lambda \prec _{\Sigma _{n+1}} V_\mu $
. Hence,
![]() $V_\lambda \prec _{\Sigma _{n+1}}V_\theta $
. Also, by elementarity,
$V_\lambda \prec _{\Sigma _{n+1}}V_\theta $
. Also, by elementarity,
![]() $V_{j(\lambda )}\prec _{\Sigma _{n+1}} V_{\theta }$
. Combining these two facts, we have that
$V_{j(\lambda )}\prec _{\Sigma _{n+1}} V_{\theta }$
. Combining these two facts, we have that
![]() $V_\lambda \prec _{\Sigma _{n+1}} V_{j(\lambda )}$
.
$V_\lambda \prec _{\Sigma _{n+1}} V_{j(\lambda )}$
.
By elementarity,
![]() $V_\mu $
satisfies the above sentence with the parameters
$V_\mu $
satisfies the above sentence with the parameters
![]() $j(\delta )$
and
$j(\delta )$
and
![]() $j(\lambda )$
replaced by
$j(\lambda )$
replaced by
![]() $\delta $
and
$\delta $
and
![]() $\lambda $
, respectively. Hence, since
$\lambda $
, respectively. Hence, since
![]() $\mu \in C^{(n+1)}$
, the sentence is true in the universe. Since
$\mu \in C^{(n+1)}$
, the sentence is true in the universe. Since
![]() $\lambda $
was arbitrarily chosen in
$\lambda $
was arbitrarily chosen in
![]() $C^{(n+1)}$
, this implies that
$C^{(n+1)}$
, this implies that
![]() $\delta $
is a
$\delta $
is a
![]() $\Sigma _{n+1}$
-supercompact cardinal.
$\Sigma _{n+1}$
-supercompact cardinal.
For the converse implication, let
![]() $\lambda $
be greater than
$\lambda $
be greater than
![]() $\delta $
and let us show that there exists an elementary embedding
$\delta $
and let us show that there exists an elementary embedding
![]() $j: V_\lambda \longrightarrow V_\theta $
, for some ordinal
$j: V_\lambda \longrightarrow V_\theta $
, for some ordinal
![]() $\theta $
, such that
$\theta $
, such that
![]() $\mathrm {crit}(j)=\delta $
,
$\mathrm {crit}(j)=\delta $
,
![]() $j(\delta )>\lambda $
, and
$j(\delta )>\lambda $
, and
![]() $j(\delta )\in C^{(n)}$
. Take
$j(\delta )\in C^{(n)}$
. Take
![]() $\mu>\lambda $
in
$\mu>\lambda $
in
![]() $C^{(n+1)}$
and let
$C^{(n+1)}$
and let
![]() $\bar {\delta },\bar {\lambda }<\bar {\mu }$
and
$\bar {\delta },\bar {\lambda }<\bar {\mu }$
and
![]() $j:V_{\bar {\mu }}\longrightarrow V_\mu $
be such that
$j:V_{\bar {\mu }}\longrightarrow V_\mu $
be such that
![]() $\mathrm {crit}(j)=\bar {\delta }$
,
$\mathrm {crit}(j)=\bar {\delta }$
,
![]() $j(\bar {\delta })=\delta $
,
$j(\bar {\delta })=\delta $
,
![]() $j(\bar {\lambda })=\lambda $
, and
$j(\bar {\lambda })=\lambda $
, and
![]() ${\bar {\mu }}\in C^{(n+1)}$
. Now notice that the sentence
${\bar {\mu }}\in C^{(n+1)}$
. Now notice that the sentence
is
![]() $\Sigma _{n+1}$
-expressible. Moreover, it is true in
$\Sigma _{n+1}$
-expressible. Moreover, it is true in
![]() $\mathrm {V}$
as witnessed by
$\mathrm {V}$
as witnessed by
![]() $\lambda $
and j because
$\lambda $
and j because
![]() $j(\bar {\delta })=\delta>\bar {\lambda }$
and
$j(\bar {\delta })=\delta>\bar {\lambda }$
and
![]() $\delta \in C^{(n)}$
(cf. Lemma 2.3). Thus, since
$\delta \in C^{(n)}$
(cf. Lemma 2.3). Thus, since
![]() $V_{\bar {\mu }}$
is
$V_{\bar {\mu }}$
is
![]() $\Sigma _{n+1}$
-correct and contains
$\Sigma _{n+1}$
-correct and contains
![]() $\bar {\delta }$
and
$\bar {\delta }$
and
![]() $\bar {\lambda }$
, it is also true in
$\bar {\lambda }$
, it is also true in
![]() $V_{\bar {\mu }}$
. By elementarity,
$V_{\bar {\mu }}$
. By elementarity,
![]() $V_\mu $
thinks that the sentence
$V_\mu $
thinks that the sentence
is true. Since
![]() $\mu \in C^{(n+1)}$
, the above displayed sentence is true in
$\mu \in C^{(n+1)}$
, the above displayed sentence is true in
![]() $\mathrm {V}$
and so
$\mathrm {V}$
and so
![]() $\delta $
is
$\delta $
is
![]() $\lambda $
-
$\lambda $
-
![]() $C^{(n)}$
-extendible. As
$C^{(n)}$
-extendible. As
![]() $\lambda $
was arbitrarily chosen,
$\lambda $
was arbitrarily chosen,
![]() $\delta $
is a
$\delta $
is a
![]() $C^{(n)}$
-extendible cardinal.
$C^{(n)}$
-extendible cardinal.
Remark 2.5. For
![]() $\lambda \in C^{(n)}$
, a cardinal
$\lambda \in C^{(n)}$
, a cardinal
![]() $\delta $
is called
$\delta $
is called
![]() $\lambda $
-
$\lambda $
-
![]() $C^{(n)+}$
-extendible if it is
$C^{(n)+}$
-extendible if it is
![]() $\lambda $
-
$\lambda $
-
![]() $C^{(n)}$
-extendible, witnessed by some elementary embedding
$C^{(n)}$
-extendible, witnessed by some elementary embedding
![]() $j\colon V_\lambda \rightarrow V_\theta $
with
$j\colon V_\lambda \rightarrow V_\theta $
with
![]() $\theta \in C^{(n)}$
. Likewise,
$\theta \in C^{(n)}$
. Likewise,
![]() $\delta $
is called
$\delta $
is called
![]() $C^{(n)+}$
-extendible if it is
$C^{(n)+}$
-extendible if it is
![]() $\lambda $
-
$\lambda $
-
![]() $C^{(n)+}$
-extendible for every
$C^{(n)+}$
-extendible for every
![]() $\lambda>\delta $
in
$\lambda>\delta $
in
![]() $C^{(n)}$
(see [Reference Bagaria1, Section 4]). A close inspection of the preceding argument reveals that
$C^{(n)}$
(see [Reference Bagaria1, Section 4]). A close inspection of the preceding argument reveals that
![]() $\Sigma _{n+1}$
-supercompactness is actually equivalent to
$\Sigma _{n+1}$
-supercompactness is actually equivalent to
![]() $C^{(n)+}$
-extendibility. This gives an alternative proof of [Reference Tsaprounis28, Corollary 3.5].
$C^{(n)+}$
-extendibility. This gives an alternative proof of [Reference Tsaprounis28, Corollary 3.5].
Corollary 2.6. A cardinal is extendible if and only if it is
![]() $\Sigma _2$
-supercompact.
$\Sigma _2$
-supercompact.
A close inspection of the proof of Theorem 2.4 shows that the following holds:
Corollary 2.7. For
![]() $n\geq 1$
, a cardinal
$n\geq 1$
, a cardinal
![]() $\delta $
is
$\delta $
is
![]() $C^{(n)}$
-extendible if and only if for a proper class of
$C^{(n)}$
-extendible if and only if for a proper class of
![]() $\lambda $
in
$\lambda $
in
![]() $C^{(n+1)}$
, for every
$C^{(n+1)}$
, for every
![]() $\alpha < \lambda $
there exist
$\alpha < \lambda $
there exist
![]() $\bar {\delta }, \bar {\alpha }<{\bar {\lambda }}$
and an elementary embedding
$\bar {\delta }, \bar {\alpha }<{\bar {\lambda }}$
and an elementary embedding
![]() $j:V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that
$j:V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that
![]() $:$
$:$
-
•
 $\mathrm {crit}(j)=\bar {\delta }$
and
$\mathrm {crit}(j)=\bar {\delta }$
and
 $j(\bar {\delta })=\delta $
.
$j(\bar {\delta })=\delta $
. -
•
 $j(\bar {\alpha })=\alpha $
.
$j(\bar {\alpha })=\alpha $
. -
•
 ${\bar {\lambda }}\in C^{(n+1)}$
.
${\bar {\lambda }}\in C^{(n+1)}$
.
In the light of the above results it is natural to define the class of
![]() $C^{(0)}$
-extendible cardinals as the class of supercompact cardinals. Note that since every
$C^{(0)}$
-extendible cardinals as the class of supercompact cardinals. Note that since every
![]() $C^{(n+1)}$
-extendible cardinal is a limit of
$C^{(n+1)}$
-extendible cardinal is a limit of
![]() $C^{(n)}$
-extendible cardinals (see [Reference Bagaria1]), every
$C^{(n)}$
-extendible cardinals (see [Reference Bagaria1]), every
![]() $\Sigma _{n+1}$
-supercompact cardinal is a limit of
$\Sigma _{n+1}$
-supercompact cardinal is a limit of
![]() $\Sigma _n$
-supercompact cardinals. It will become apparent in the following sections that the notion of
$\Sigma _n$
-supercompact cardinals. It will become apparent in the following sections that the notion of
![]() $\Sigma _{n+1}$
-supercompactness is a useful reformulation of
$\Sigma _{n+1}$
-supercompactness is a useful reformulation of
![]() $C^{(n)}$
-extendibility in the context of class forcing.
$C^{(n)}$
-extendibility in the context of class forcing.
3 Some reflection properties for class forcing iterations
In the sequel we will only work with ORD-length forcing iterations, since extendible cardinals are generally destroyed by set-size ones (see Theorem 1.1 and the related discussion).
If
![]() $\mathbb {P}$
is a forcing iteration, and G is
$\mathbb {P}$
is a forcing iteration, and G is
![]() $\mathbb {P}$
-generic over V, then for every ordinal
$\mathbb {P}$
-generic over V, then for every ordinal
![]() $\lambda $
, we denote as customary by
$\lambda $
, we denote as customary by
![]() $G_\lambda $
the
$G_\lambda $
the
![]() $\mathbb {P}_\lambda $
-generic filter induced by G, i.e.,
$\mathbb {P}_\lambda $
-generic filter induced by G, i.e.,
![]() $G_\lambda :=G\cap \mathbb {P}_\lambda $
. Also, as usual we denote by
$G_\lambda :=G\cap \mathbb {P}_\lambda $
. Also, as usual we denote by
![]() $i_{G_\lambda }(\tau )$
the interpretation of the
$i_{G_\lambda }(\tau )$
the interpretation of the
![]() $\mathbb {P}_\lambda $
-name
$\mathbb {P}_\lambda $
-name
![]() $\tau $
by the filter
$\tau $
by the filter
![]() $G_\lambda $
.
$G_\lambda $
.
For the main preservation results given in the following sections we will need to ensure that there are many cardinals
![]() $\lambda $
that satisfy
$\lambda $
that satisfy
![]() $V_\lambda [G_\lambda ]=V[G]_\lambda $
.
$V_\lambda [G_\lambda ]=V[G]_\lambda $
.
Definition 3.1. Let
![]() $\mathbb {P}$
be a forcing iteration. A cardinal
$\mathbb {P}$
be a forcing iteration. A cardinal
![]() $\lambda $
is
$\lambda $
is
![]() $\mathbb {P}$
-reflecting if
$\mathbb {P}$
-reflecting if
![]() $\mathbb {P}$
forces that
$\mathbb {P}$
forces that
![]() $V[\dot {G}]_\lambda = V_\lambda [\dot {G}_\lambda ]$
.
$V[\dot {G}]_\lambda = V_\lambda [\dot {G}_\lambda ]$
.
Remark 3.2. Note that since the rank of
![]() $i_{G_\lambda }(\tau )$
in
$i_{G_\lambda }(\tau )$
in
![]() $V[G_\lambda ]$
is never bigger than the rank of
$V[G_\lambda ]$
is never bigger than the rank of
![]() $\tau $
in
$\tau $
in
![]() $\mathrm {V}$
, for any
$\mathrm {V}$
, for any
![]() $\tau \in V^{\mathbb {P}_\lambda }$
, we clearly have
$\tau \in V^{\mathbb {P}_\lambda }$
, we clearly have
Thus,
![]() $\mathbb {P}$
always forces “
$\mathbb {P}$
always forces “
![]() $V_\lambda [\dot {G}_\lambda ]\subseteq V[\dot {G}]_\lambda $
.” Hence a cardinal
$V_\lambda [\dot {G}_\lambda ]\subseteq V[\dot {G}]_\lambda $
.” Hence a cardinal
![]() $\lambda $
is
$\lambda $
is
![]() $\mathbb {P}$
-reflecting if and only if
$\mathbb {P}$
-reflecting if and only if
![]() $\mathbb {P}$
forces “
$\mathbb {P}$
forces “
![]() $V[\dot {G}]_\lambda \subseteq V_\lambda [\dot {G}_\lambda ]$
.”
$V[\dot {G}]_\lambda \subseteq V_\lambda [\dot {G}_\lambda ]$
.”
Proposition 3.3.
![]() $K:=\{\lambda \mid \lambda \text { is } \mathbb {P}\text {-reflecting}\}$
is closed.
$K:=\{\lambda \mid \lambda \text { is } \mathbb {P}\text {-reflecting}\}$
is closed.
Proof Let
![]() $\kappa $
be an accumulation point of K. Let
$\kappa $
be an accumulation point of K. Let
![]() $p\in \mathbb {P}$
and
$p\in \mathbb {P}$
and
![]() $\tau \in V^{\mathbb {P}}$
be such that
$\tau \in V^{\mathbb {P}}$
be such that
![]() $p\Vdash _{\mathbb {P}}\tau \in V[\dot {G}]_\kappa $
. Since
$p\Vdash _{\mathbb {P}}\tau \in V[\dot {G}]_\kappa $
. Since
![]() $\kappa $
is a limit cardinal there is
$\kappa $
is a limit cardinal there is
![]() $q\leq _{\mathbb {P}} p$
and
$q\leq _{\mathbb {P}} p$
and
![]() $\lambda <\kappa $
such that
$\lambda <\kappa $
such that
![]() $q\Vdash _{\mathbb {P}} \tau \in V[\dot {G}]_\lambda $
. Extending q if necessary, we may find
$q\Vdash _{\mathbb {P}} \tau \in V[\dot {G}]_\lambda $
. Extending q if necessary, we may find
![]() $\theta \in K\cap \kappa $
above
$\theta \in K\cap \kappa $
above
![]() $\lambda $
such that
$\lambda $
such that
![]() $q\Vdash _{\mathbb {P}}\tau \in V[\dot {G}]_\lambda \subseteq V_\theta [\dot {G}_\theta ]$
. Thus,
$q\Vdash _{\mathbb {P}}\tau \in V[\dot {G}]_\lambda \subseteq V_\theta [\dot {G}_\theta ]$
. Thus,
![]() $\kappa \in K$
, as wanted.
$\kappa \in K$
, as wanted.
The following proposition gives some sufficient conditions for a cardinal to be
![]() $\mathbb {P}$
-reflecting.
$\mathbb {P}$
-reflecting.
Proposition 3.4. Suppose
![]() $\lambda $
is an inaccessible cardinal and
$\lambda $
is an inaccessible cardinal and
![]() $\mathbb {P}$
is a forcing iteration such that :
$\mathbb {P}$
is a forcing iteration such that :
![]() $\mathbb {P}_\lambda $
is a
$\mathbb {P}_\lambda $
is a
![]() $\lambda $
-cc forcing which preserves the inaccessibility of
$\lambda $
-cc forcing which preserves the inaccessibility of
![]() $\lambda $
,
$\lambda $
,
![]() $\mathbb {P}_\lambda \subseteq V_\lambda $
, and
$\mathbb {P}_\lambda \subseteq V_\lambda $
, and
![]() $\Vdash _{\mathbb {P}_{\lambda }}\text {"}\,\dot {\mathbb {Q}}$
is
$\Vdash _{\mathbb {P}_{\lambda }}\text {"}\,\dot {\mathbb {Q}}$
is
![]() $\lambda $
-distributive,” where
$\lambda $
-distributive,” where
![]() $\mathbb {P}\cong \mathbb {P}_{\lambda }\ast \mathbb {Q}$
. Then
$\mathbb {P}\cong \mathbb {P}_{\lambda }\ast \mathbb {Q}$
. Then
![]() $\lambda $
is
$\lambda $
is
![]() $\mathbb {P}$
-reflecting.
$\mathbb {P}$
-reflecting.
Proof On the one hand, by induction on the rank and using the fact that
![]() $\mathbb {P}_{\lambda }$
is
$\mathbb {P}_{\lambda }$
is
![]() $\lambda $
-cc and preserves the inaccessibility of
$\lambda $
-cc and preserves the inaccessibility of
![]() $\lambda $
, one can easily show that
$\lambda $
, one can easily show that
![]() $V[G_\lambda ]_\lambda \subseteq V_{\lambda } [G_\lambda ]$
.
$V[G_\lambda ]_\lambda \subseteq V_{\lambda } [G_\lambda ]$
.
On the other hand, as
![]() $\mathbb {P}_\lambda $
preserves the inaccessibility of
$\mathbb {P}_\lambda $
preserves the inaccessibility of
![]() $\lambda $
,
$\lambda $
,
![]() $|V[G_\lambda ]_\lambda |=\lambda $
. Hence, since
$|V[G_\lambda ]_\lambda |=\lambda $
. Hence, since
![]() $\Vdash _{\mathbb {P}_{\lambda }}\text {"}\,\dot {\mathbb {Q}} \text { is } \lambda \text {-distributive},\text {"}$
and so
$\Vdash _{\mathbb {P}_{\lambda }}\text {"}\,\dot {\mathbb {Q}} \text { is } \lambda \text {-distributive},\text {"}$
and so
![]() $i_{G_\lambda }(\dot {\mathbb {Q}})$
does not add any new subsets of
$i_{G_\lambda }(\dot {\mathbb {Q}})$
does not add any new subsets of
![]() $V[G_\lambda ]_\lambda $
, we have
$V[G_\lambda ]_\lambda $
, we have
Hence,
![]() $V[G]_\lambda \subseteq V_\lambda [G_\lambda ]$
.
$V[G]_\lambda \subseteq V_\lambda [G_\lambda ]$
.
Let
![]() $\mathcal {L}$
denote the language of set theory augmented with an additional unary predicate
$\mathcal {L}$
denote the language of set theory augmented with an additional unary predicate
![]() $\mathbb {P}$
. We will denote by
$\mathbb {P}$
. We will denote by
![]() $\Sigma _k^{\mathcal {L}}$
(resp.
$\Sigma _k^{\mathcal {L}}$
(resp.
![]() $\Pi _k^{\mathcal {L}}$
) the class of
$\Pi _k^{\mathcal {L}}$
) the class of
![]() $\Sigma _k$
(resp.
$\Sigma _k$
(resp.
![]() $\Pi _k$
) formulae of
$\Pi _k$
) formulae of
![]() $\mathcal {L}$
. This choice of language will be useful when working with expressions involving a given iteration
$\mathcal {L}$
. This choice of language will be useful when working with expressions involving a given iteration
![]() $\mathbb {P}$
. For instance, we shall need to compute the complexity of the notion
$\mathbb {P}$
. For instance, we shall need to compute the complexity of the notion
as a property of
![]() $\alpha $
, when
$\alpha $
, when
![]() $\mathbb {P}$
is a definable ORD-length forcing iteration.
$\mathbb {P}$
is a definable ORD-length forcing iteration.
Remark 3.5. For such
![]() $\mathbb {P}$
and
$\mathbb {P}$
and
![]() $\alpha $
, since we are dealing with iterations it would perhaps seem more natural to consider the predicate
$\alpha $
, since we are dealing with iterations it would perhaps seem more natural to consider the predicate
![]() $\mathbb {P}_\alpha $
instead of
$\mathbb {P}_\alpha $
instead of
![]() $\mathbb {P}\cap V_\alpha $
. However, if
$\mathbb {P}\cap V_\alpha $
. However, if
![]() $\mathbb {P}_\alpha \subseteq V_\alpha $
(and so
$\mathbb {P}_\alpha \subseteq V_\alpha $
(and so
![]() $\alpha $
is a limit ordinal and the direct limit is taken at stage
$\alpha $
is a limit ordinal and the direct limit is taken at stage
![]() $\alpha $
of the iteration), then we have
$\alpha $
of the iteration), then we have
![]() $ \mathbb {P}\cap V_\alpha =\mathbb {P}_\alpha $
.
$ \mathbb {P}\cap V_\alpha =\mathbb {P}_\alpha $
.
It is a well-known fact (see, e.g., [Reference Jech16, Section 13]) that the truth predicate (in the language of set theory) for
![]() $\Sigma _0$
formulae is
$\Sigma _0$
formulae is
![]() $\Delta _1$
definable (i.e., both
$\Delta _1$
definable (i.e., both
![]() $\Sigma _1$
and
$\Sigma _1$
and
![]() $\Pi _1$
-definable); and in general, the truth predicate for
$\Pi _1$
-definable); and in general, the truth predicate for
![]() $\Sigma _k$
(resp.
$\Sigma _k$
(resp.
![]() $\Pi _k$
) formulae, for
$\Pi _k$
) formulae, for
![]() $k\geq 1$
, is
$k\geq 1$
, is
![]() $\Sigma _k$
-definable (resp.
$\Sigma _k$
-definable (resp.
![]() $\Pi _k$
). However, if
$\Pi _k$
). However, if
![]() $\mathbb {P}$
is a definable predicate, then the complexity (in the language of set theory) of the truth predicate for formulae in the language
$\mathbb {P}$
is a definable predicate, then the complexity (in the language of set theory) of the truth predicate for formulae in the language
![]() $\mathcal {L}$
depends naturally on the complexity of the definition of
$\mathcal {L}$
depends naturally on the complexity of the definition of
![]() $\mathbb {P}$
.
$\mathbb {P}$
.
Proposition 3.6. If
![]() $\mathbb {P}$
is either
$\mathbb {P}$
is either
![]() $\Sigma _m$
or
$\Sigma _m$
or
![]() $\Pi _m$
-definable, then the truth predicate
$\Pi _m$
-definable, then the truth predicate
![]() $\vDash _{\Sigma _0^{\mathcal {L}}}$
for
$\vDash _{\Sigma _0^{\mathcal {L}}}$
for
![]() $\Sigma _0^{\mathcal {L}}$
formulae is
$\Sigma _0^{\mathcal {L}}$
formulae is
![]() $\Delta _{m+1} \ ($
i.e., both
$\Delta _{m+1} \ ($
i.e., both
![]() $\Sigma _{m+1}$
and
$\Sigma _{m+1}$
and
![]() $\Pi _{m+1})$
. In general, for
$\Pi _{m+1})$
. In general, for
![]() $k\geq 1$
, the truth predicate
$k\geq 1$
, the truth predicate
![]() $\vDash _{\Sigma _k^{ \mathcal {L}}}$
for
$\vDash _{\Sigma _k^{ \mathcal {L}}}$
for
![]() $\Sigma _k^{\mathcal {L}}$
formulae
$\Sigma _k^{\mathcal {L}}$
formulae
![]() $($
resp.
$($
resp.
![]() $\vDash _{\Pi _k^{ \mathcal {L}}}$
for
$\vDash _{\Pi _k^{ \mathcal {L}}}$
for
![]() $\Pi _{k}^{\mathcal {L}}$
formulae) is
$\Pi _{k}^{\mathcal {L}}$
formulae) is
![]() $\Sigma _{m +k} \ ($
resp.
$\Sigma _{m +k} \ ($
resp.
![]() $\Pi _{m+k})$
. If
$\Pi _{m+k})$
. If
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _m$
-definable
$\Delta _m$
-definable
![]() $($
with
$($
with
![]() $m\geq 1)$
, then
$m\geq 1)$
, then
![]() $\vDash _{\Sigma _0^{\mathcal {L}}}$
is
$\vDash _{\Sigma _0^{\mathcal {L}}}$
is
![]() $\Delta _m$
, and
$\Delta _m$
, and
![]() $\vDash _{\Sigma _k^{ \mathcal {L}}} \ ($
resp.
$\vDash _{\Sigma _k^{ \mathcal {L}}} \ ($
resp.
![]() $\vDash _{\Pi _k^{ \mathcal {L}}})$
is
$\vDash _{\Pi _k^{ \mathcal {L}}})$
is
![]() $\Sigma _{m +k-1} \ ($
resp.
$\Sigma _{m +k-1} \ ($
resp.
![]() $\Pi _{m+k-1})$
.
$\Pi _{m+k-1})$
.
Proof Note first that the only atomic formulas in the language
![]() $\mathcal {L}$
are of the form “
$\mathcal {L}$
are of the form “
![]() $x\in y$
,” “
$x\in y$
,” “
![]() $x=y$
,” or “
$x=y$
,” or “
![]() $x\in \mathbb {P}\,,$
” where x and y are variable symbols. Hence, if
$x\in \mathbb {P}\,,$
” where x and y are variable symbols. Hence, if
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Sigma _m$
(resp.
$\Sigma _m$
(resp.
![]() $\Pi _m$
) definable, then the formula
$\Pi _m$
) definable, then the formula
![]() $\text {"}x \in \mathbb {P}\,\text {"}$
is equivalent to a
$\text {"}x \in \mathbb {P}\,\text {"}$
is equivalent to a
![]() $\Sigma _m$
(resp.
$\Sigma _m$
(resp.
![]() $\Pi _m$
) formula of the language of set theory. It follows that a Boolean combination of atomic formulas of the language
$\Pi _m$
) formula of the language of set theory. It follows that a Boolean combination of atomic formulas of the language
![]() $\mathcal {L}$
is equivalent to a Boolean combination of
$\mathcal {L}$
is equivalent to a Boolean combination of
![]() $\Sigma _m$
and
$\Sigma _m$
and
![]() $\Pi _m$
formulas in the language of set theory. Hence, the truth predicate for Boolean combinations of atomic formulas in the language
$\Pi _m$
formulas in the language of set theory. Hence, the truth predicate for Boolean combinations of atomic formulas in the language
![]() $\mathcal {L}$
is
$\mathcal {L}$
is
![]() $\Delta _{m+1}$
definable. The same applies to formulas with bounded quantifiers. By induction on k one can now show (as in [Reference Jech16, Section 13]) that
$\Delta _{m+1}$
definable. The same applies to formulas with bounded quantifiers. By induction on k one can now show (as in [Reference Jech16, Section 13]) that
![]() $\vDash _{\Sigma _k^{ \mathcal {L}}}$
(resp.
$\vDash _{\Sigma _k^{ \mathcal {L}}}$
(resp.
![]() $\vDash _{\Pi _k^{ \mathcal {L}}}$
) is
$\vDash _{\Pi _k^{ \mathcal {L}}}$
) is
![]() $\Sigma _{m +k}$
(resp.
$\Sigma _{m +k}$
(resp.
![]() $\Pi _{m+k}$
) definable.
$\Pi _{m+k}$
) definable.
If
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _m$
-definable, with
$\Delta _m$
-definable, with
![]() $m\geq 1$
, then “
$m\geq 1$
, then “
![]() $x\in \mathbb {P}$
” is both
$x\in \mathbb {P}$
” is both
![]() $\Sigma _m$
and
$\Sigma _m$
and
![]() $\Pi _m$
-expressible. It easily follows that
$\Pi _m$
-expressible. It easily follows that
![]() $\vDash _{\Sigma _0^{\mathcal {L}}}$
is
$\vDash _{\Sigma _0^{\mathcal {L}}}$
is
![]() $\Delta _m$
-definable, and by induction on k one readily shows that
$\Delta _m$
-definable, and by induction on k one readily shows that
![]() $\vDash _{\Sigma _k^{ \mathcal {L}}}$
(resp.
$\vDash _{\Sigma _k^{ \mathcal {L}}}$
(resp.
![]() $\vDash _{\Pi _k^{ \mathcal {L}}}$
) is
$\vDash _{\Pi _k^{ \mathcal {L}}}$
) is
![]() $\Sigma _{m +k-1}$
(resp.
$\Sigma _{m +k-1}$
(resp.
![]() $\Pi _{m+k-1}$
) definable.
$\Pi _{m+k-1}$
) definable.
Definition 3.7. For
![]() $k\geq 0$
and a predicateFootnote
2
$k\geq 0$
and a predicateFootnote
2
![]() $\mathbb {P}$
, we shall denote by
$\mathbb {P}$
, we shall denote by
![]() $C^{(k)}_{\mathbb {P}}$
the class of all ordinals
$C^{(k)}_{\mathbb {P}}$
the class of all ordinals
![]() $\alpha $
such that
$\alpha $
such that
It is easily seen that
![]() $C^{(k)}_{\mathbb {P}}$
is a closed unbounded proper class, and
$C^{(k)}_{\mathbb {P}}$
is a closed unbounded proper class, and
![]() $C^{(k+1)}_{\mathbb {P}}\subseteq C^{(k)}_{\mathbb {P}}$
, for each
$C^{(k+1)}_{\mathbb {P}}\subseteq C^{(k)}_{\mathbb {P}}$
, for each
![]() $k\geq 0$
. Notice that
$k\geq 0$
. Notice that
![]() $C^{(0)}_{\mathbb {P}}$
is the class of all ordinals. Let us compute next the complexity of
$C^{(0)}_{\mathbb {P}}$
is the class of all ordinals. Let us compute next the complexity of
![]() $C^{(k)}_{\mathbb {P}}$
, in the language of set theory, when
$C^{(k)}_{\mathbb {P}}$
, in the language of set theory, when
![]() $\mathbb {P}$
is a definable predicate.
$\mathbb {P}$
is a definable predicate.
Notation 3.8. For
![]() $m<\omega $
, we will denote by
$m<\omega $
, we will denote by
![]() $\Gamma _m$
(resp.
$\Gamma _m$
(resp.
![]() $\mathbf {\Gamma }_m$
) the collection of all the formulas in the language of set theory that are either
$\mathbf {\Gamma }_m$
) the collection of all the formulas in the language of set theory that are either
![]() $\Sigma _m$
or
$\Sigma _m$
or
![]() $\Pi _m$
(resp. with parameters). In the sequel, expressions such as “X is
$\Pi _m$
(resp. with parameters). In the sequel, expressions such as “X is
![]() $\Gamma _m$
” should be read as “X is definable by a formula in
$\Gamma _m$
” should be read as “X is definable by a formula in
![]() $\Gamma _m$
.”
$\Gamma _m$
.”
Proposition 3.9. The class
![]() $C^{(k)}_{\mathbb {P}}$
is
$C^{(k)}_{\mathbb {P}}$
is
Proof The class
![]() $C^{(0)}_{\mathbb {P}}$
is
$C^{(0)}_{\mathbb {P}}$
is
![]() $\Pi _0$
, being the class of all ordinals. If
$\Pi _0$
, being the class of all ordinals. If
![]() $k\geq 1$
, then we have that
$k\geq 1$
, then we have that
![]() $\alpha \in C^{(k)}_{\mathbb {P}}$
if and only if
$\alpha \in C^{(k)}_{\mathbb {P}}$
if and only if
![]() $\alpha \in C^{(k-1)}_{\mathbb {P}}$
and
$\alpha \in C^{(k-1)}_{\mathbb {P}}$
and
Now using induction on k and Proposition 3.6 the complexity of the class
![]() $C^{(k)}_{\mathbb {P}}$
is easily seen to be as desired.
$C^{(k)}_{\mathbb {P}}$
is easily seen to be as desired.
It is well-known that if
![]() $\mathbb {P}$
is a set-forcing notion, then for every condition
$\mathbb {P}$
is a set-forcing notion, then for every condition
![]() $p\in \mathbb {P}$
, and
$p\in \mathbb {P}$
, and
![]() $\mathbb {P}$
-names
$\mathbb {P}$
-names
![]() $\tau _1,\ldots ,\tau _n$
, if
$\tau _1,\ldots ,\tau _n$
, if
![]() $\varphi (x_1,\ldots ,x_n)$
is a
$\varphi (x_1,\ldots ,x_n)$
is a
![]() $\Sigma _k$
(resp.
$\Sigma _k$
(resp.
![]() $\Pi _k$
) formula of the language of set theory, with
$\Pi _k$
) formula of the language of set theory, with
![]() $k\geq 1$
, then the sentence “
$k\geq 1$
, then the sentence “
![]() $p\Vdash _{\mathbb {P}} \varphi (\tau _1,\ldots ,\tau _n)$
” is also
$p\Vdash _{\mathbb {P}} \varphi (\tau _1,\ldots ,\tau _n)$
” is also
![]() $\Sigma _k$
(resp.
$\Sigma _k$
(resp.
![]() $\Pi _k$
), in the parameters
$\Pi _k$
), in the parameters
![]() $\mathbb {P}$
and
$\mathbb {P}$
and
![]() $\tau _1,\ldots ,\tau _n$
. We shall see next that for definable
$\tau _1,\ldots ,\tau _n$
. We shall see next that for definable
![]() ${\mathrm {ORD}}$
-length forcing iterations that satisfy some mild conditions the complexity of the forcing relation for
${\mathrm {ORD}}$
-length forcing iterations that satisfy some mild conditions the complexity of the forcing relation for
![]() $\mathbb {P}$
depends only on the complexity of the definition of
$\mathbb {P}$
depends only on the complexity of the definition of
![]() $\mathbb {P}$
. Thus, if, e.g.,
$\mathbb {P}$
. Thus, if, e.g.,
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Gamma _m$
-definable, then the forcing relation for
$\Gamma _m$
-definable, then the forcing relation for
![]() $\Sigma _k$
formulae is
$\Sigma _k$
formulae is
![]() $\Sigma _{m+k}$
-definable (Proposition 3.13).
$\Sigma _{m+k}$
-definable (Proposition 3.13).
Definition 3.10. Let
![]() $\mathbb {P}$
be a definable
$\mathbb {P}$
be a definable
![]() ${\mathrm {ORD}}$
-length forcing iteration. Then
${\mathrm {ORD}}$
-length forcing iteration. Then
![]() $\mathbb {P}$
is adequate if there is a sufficiently rich finite fragment
$\mathbb {P}$
is adequate if there is a sufficiently rich finite fragment
![]() $ZFC^*$
of ZFC which allows to define the forcing relation
$ZFC^*$
of ZFC which allows to define the forcing relation
![]() $\Vdash _{\mathbb {P}}$
for
$\Vdash _{\mathbb {P}}$
for
![]() $\Delta _0$
-formulae of the language of set theory and proving the Forcing Theorem for such formulae, and there is a proper class of ordinals
$\Delta _0$
-formulae of the language of set theory and proving the Forcing Theorem for such formulae, and there is a proper class of ordinals
![]() $\kappa $
such that
$\kappa $
such that
![]() $V_\kappa \models ZFC^*$
and
$V_\kappa \models ZFC^*$
and
![]() $\mathbb {P}^{V_\kappa } =\mathbb {P}_\kappa $
(hence also
$\mathbb {P}^{V_\kappa } =\mathbb {P}_\kappa $
(hence also
![]() $\mathbb {P}_\kappa =\mathbb {P}\cap V_\kappa $
).Footnote
3
$\mathbb {P}_\kappa =\mathbb {P}\cap V_\kappa $
).Footnote
3
Although there are examples of definable class forcing notions
![]() $\mathbb {P}$
for which the forcing relation
$\mathbb {P}$
for which the forcing relation
![]() $\Vdash _{\mathbb {P}}$
is not definable (see [Reference Lücke, Njegomir, Holy, Krapf and Schlicht20, Section 7]), if
$\Vdash _{\mathbb {P}}$
is not definable (see [Reference Lücke, Njegomir, Holy, Krapf and Schlicht20, Section 7]), if
![]() $\mathbb {P}$
satisfies certain conditions, e.g., is either pretame (see [Reference Friedman12]), progressively-closed [Reference Reitz23], or suitable (Definition 5.1), then the forcing relation
$\mathbb {P}$
satisfies certain conditions, e.g., is either pretame (see [Reference Friedman12]), progressively-closed [Reference Reitz23], or suitable (Definition 5.1), then the forcing relation
![]() $\Vdash _{\mathbb {P}}$
is definable and the Forcing Theorem holds for
$\Vdash _{\mathbb {P}}$
is definable and the Forcing Theorem holds for
![]() $\mathbb {P}$
.
$\mathbb {P}$
.
Proposition 3.11. Let
![]() $\mathbb {P}$
be adequate and let
$\mathbb {P}$
be adequate and let
![]() $ZFC^*$
be a finite fragment of ZFC which is sufficient for defining the forcing relation
$ZFC^*$
be a finite fragment of ZFC which is sufficient for defining the forcing relation
![]() $\Vdash _{\mathbb {P}}$
for
$\Vdash _{\mathbb {P}}$
for
![]() $\Delta _0$
-formulae of the language of set theory and proving the Forcing Theorem for such formulae. Suppose M is a transitive set and
$\Delta _0$
-formulae of the language of set theory and proving the Forcing Theorem for such formulae. Suppose M is a transitive set and
![]() $\kappa \in M$
is such that
$\kappa \in M$
is such that
![]() $M_\kappa \models ZFC^\ast $
and
$M_\kappa \models ZFC^\ast $
and
![]() $\mathbb {P}^{ M_\kappa } =\mathbb {P}_\kappa $
. Then, for every
$\mathbb {P}^{ M_\kappa } =\mathbb {P}_\kappa $
. Then, for every
![]() $\Delta _0$
-formula
$\Delta _0$
-formula
![]() $\varphi $
of the language of set theory, every
$\varphi $
of the language of set theory, every
![]() $p\in \mathbb {P}$
, and every
$p\in \mathbb {P}$
, and every
![]() $\mathbb {P}$
-name
$\mathbb {P}$
-name
![]() $\tau $
such that
$\tau $
such that
![]() $p,\tau \in M_\kappa $
,
$p,\tau \in M_\kappa $
,
Proof Assume
![]() $p\Vdash _{\mathbb {P}} \varphi (\tau )$
. Suppose, for a contradiction, that
$p\Vdash _{\mathbb {P}} \varphi (\tau )$
. Suppose, for a contradiction, that
![]() $q\in \mathbb {P}_\kappa $
is such that
$q\in \mathbb {P}_\kappa $
is such that
![]() $q\leq _{\mathbb {P}_\kappa } p$
and
$q\leq _{\mathbb {P}_\kappa } p$
and
![]() $M_\kappa \models \text {"}q\Vdash _{\mathbb {P}_\kappa } \neg \varphi (\tau ).\text {"}$
Suppose G is
$M_\kappa \models \text {"}q\Vdash _{\mathbb {P}_\kappa } \neg \varphi (\tau ).\text {"}$
Suppose G is
![]() $\mathbb {P}$
-generic over V, with
$\mathbb {P}$
-generic over V, with
![]() $q\in G$
. Then
$q\in G$
. Then
Note that, since
![]() $\mathbb {P}^{M_\kappa } =\mathbb {P}_\kappa $
, a direct limit is taken at
$\mathbb {P}^{M_\kappa } =\mathbb {P}_\kappa $
, a direct limit is taken at
![]() $\kappa $
(for otherwise some conditions in
$\kappa $
(for otherwise some conditions in
![]() $\mathbb {P}_\kappa $
would be proper classes in
$\mathbb {P}_\kappa $
would be proper classes in
![]() $M_\kappa $
), and therefore being a dense subset of
$M_\kappa $
), and therefore being a dense subset of
![]() $\mathbb {P}_\kappa $
is absolute between M and V. Thus,
$\mathbb {P}_\kappa $
is absolute between M and V. Thus,
![]() $G_\kappa :=G\cap \mathbb {P}_\kappa $
is
$G_\kappa :=G\cap \mathbb {P}_\kappa $
is
![]() $\mathbb {P}_\kappa $
-generic over
$\mathbb {P}_\kappa $
-generic over
![]() $M_\kappa $
. Also, since
$M_\kappa $
. Also, since
![]() $\tau \in M_\kappa $
, hence
$\tau \in M_\kappa $
, hence
![]() $\tau \in M_\kappa ^{\mathbb {P}_\kappa }$
,
$\tau \in M_\kappa ^{\mathbb {P}_\kappa }$
,
![]() $i_{G_\kappa }(\tau )\in M_\kappa [G_\kappa ]$
and
$i_{G_\kappa }(\tau )\in M_\kappa [G_\kappa ]$
and
![]() $i_G(\tau )=i_{G_\kappa }(\tau )$
. Since
$i_G(\tau )=i_{G_\kappa }(\tau )$
. Since
![]() $\varphi $
is
$\varphi $
is
![]() $\Delta _0$
, hence absolute for transitive classes,
$\Delta _0$
, hence absolute for transitive classes,
![]() $M_\kappa [G_\kappa ] \models \varphi (i_{G_\kappa }(\tau )).$
But as
$M_\kappa [G_\kappa ] \models \varphi (i_{G_\kappa }(\tau )).$
But as
![]() $q\in G_\kappa $
, this contradicts
$q\in G_\kappa $
, this contradicts
![]() $M_\kappa \models \text {"}q\Vdash _{\mathbb {P}_\kappa }\neg \varphi (\tau ).\text {"}$
$M_\kappa \models \text {"}q\Vdash _{\mathbb {P}_\kappa }\neg \varphi (\tau ).\text {"}$
A similar argument proves the converse.
Corollary 3.12. Let
![]() $\mathbb {P}$
be adequate. Then, for every
$\mathbb {P}$
be adequate. Then, for every
![]() $\Delta _0$
-formula
$\Delta _0$
-formula
![]() $\varphi $
, every
$\varphi $
, every
![]() $p\in \mathbb {P}$
, and every
$p\in \mathbb {P}$
, and every
![]() $\mathbb {P}$
-name
$\mathbb {P}$
-name
![]() $\tau $
, the sentence “
$\tau $
, the sentence “
![]() $p\Vdash _{\mathbb {P}} \varphi (\tau )$
” is
$p\Vdash _{\mathbb {P}} \varphi (\tau )$
” is
![]() $:$
$:$
-
(1)
 $\Delta _{1}^{\mathcal {L}},$
$\Delta _{1}^{\mathcal {L}},$
-
(2)
 $\Delta _{m+1}$
, if
$\Delta _{m+1}$
, if
 $\mathbb {P}$
is
$\mathbb {P}$
is
 $\Gamma _{m}$
-definable
$\Gamma _{m}$
-definable
 $($
some
$($
some
 $m\geq 1)$
,
$m\geq 1)$
, -
(3)
 $\Delta _{m}$
, if
$\Delta _{m}$
, if
 $\mathbb {P}$
is
$\mathbb {P}$
is
 $\Delta _{m}$
-definable
$\Delta _{m}$
-definable
 $($
some
$($
some
 $m\geq 1)$
,
$m\geq 1)$
,
with p and
![]() $\tau $
as parameters.
$\tau $
as parameters.
Proof The proof of Proposition 3.11 shows that “
![]() $p\Vdash _{\mathbb {P}} \varphi (\tau )$
” holds if and only if
$p\Vdash _{\mathbb {P}} \varphi (\tau )$
” holds if and only if
and also if and only if
Now the two displayed sentences above are easily seen to be
![]() $\Pi _1^{\mathcal {L}}$
and
$\Pi _1^{\mathcal {L}}$
and
![]() $\Sigma _1^{\mathcal {L}}$
, respectively, with p and
$\Sigma _1^{\mathcal {L}}$
, respectively, with p and
![]() $\tau $
as parameters.
$\tau $
as parameters.
Items (2) and (3) now follow easily from (1) and Proposition 3.6.
Proposition 3.13. Let
![]() $\mathbb {P}$
be adequate. Let
$\mathbb {P}$
be adequate. Let
![]() $k\geq 1$
. Then, for every
$k\geq 1$
. Then, for every
![]() $\Sigma _k \ ($
resp.
$\Sigma _k \ ($
resp.
![]() $\Pi _k)$
formula
$\Pi _k)$
formula
![]() $\varphi (t)$
of the language of set theory, every
$\varphi (t)$
of the language of set theory, every
![]() $p\in \mathbb {P}$
, and every
$p\in \mathbb {P}$
, and every
![]() $\mathbb {P}$
-name
$\mathbb {P}$
-name
![]() $\tau $
, the sentence
$\tau $
, the sentence
![]() $\text {"}p\Vdash _{\mathbb {P}} \varphi (\tau )\text {"}$
is
$\text {"}p\Vdash _{\mathbb {P}} \varphi (\tau )\text {"}$
is
![]() $:$
$:$
-
(1)
 $\Sigma _{k}^{\mathcal {L}} \ ($
resp.
$\Sigma _{k}^{\mathcal {L}} \ ($
resp.
 $\Pi _{k}^{\mathcal {L}}).$
$\Pi _{k}^{\mathcal {L}}).$
-
(2)
 $\Sigma _{ m+k} \ ($
resp.
$\Sigma _{ m+k} \ ($
resp.
 $\Pi _{m+k})$
, if
$\Pi _{m+k})$
, if
 $\mathbb {P}$
is
$\mathbb {P}$
is
 $\Gamma _{m}$
-definable
$\Gamma _{m}$
-definable
 $($
some
$($
some
 $m\geq 1).$
$m\geq 1).$
-
(3)
 $\Sigma _{ m+k-1} \ ($
resp.
$\Sigma _{ m+k-1} \ ($
resp.
 $\Pi _{m+k-1})$
, if
$\Pi _{m+k-1})$
, if
 $\mathbb {P}$
is
$\mathbb {P}$
is
 $\Delta _{m}$
-definable
$\Delta _{m}$
-definable
 $($
some
$($
some
 $m\geq 1)$
.
$m\geq 1)$
.
Proof First, note that the class
![]() $V^{\mathbb {P}}$
of
$V^{\mathbb {P}}$
of
![]() $\mathbb {P}$
-names is
$\mathbb {P}$
-names is
![]() $\Delta _{1}^{\mathcal {L}}$
. Now given a
$\Delta _{1}^{\mathcal {L}}$
. Now given a
![]() $\Sigma _k$
formula
$\Sigma _k$
formula
![]() $\exists x \forall y \exists z \ldots \psi (x,y,z,\ldots ,t)$
of the language of set theory, and given a condition
$\exists x \forall y \exists z \ldots \psi (x,y,z,\ldots ,t)$
of the language of set theory, and given a condition
![]() $p\in \mathbb {P}$
and
$p\in \mathbb {P}$
and
![]() $\tau \in V^{\mathbb {P}}$
,
$\tau \in V^{\mathbb {P}}$
,
if and only if
Since
![]() $p\Vdash _{\mathbb {P}} \psi (\sigma ,\sigma ', \sigma ", \tau )$
is
$p\Vdash _{\mathbb {P}} \psi (\sigma ,\sigma ', \sigma ", \tau )$
is
![]() $\Delta _1^{\mathcal {L}}$
(Corollary 3.12), the last displayed sentence is
$\Delta _1^{\mathcal {L}}$
(Corollary 3.12), the last displayed sentence is
![]() $\Sigma _{k}^{\mathcal {L}}$
, as wanted.
$\Sigma _{k}^{\mathcal {L}}$
, as wanted.
Items (2) and (3) follow easily from (1) and Proposition 3.6.
Let us compute next the complexity of the notion of
![]() $\mathbb {P}$
-reflecting cardinal, for adequate
$\mathbb {P}$
-reflecting cardinal, for adequate
![]() $\mathbb {P}$
.
$\mathbb {P}$
.
Proposition 3.14. Let
![]() $\mathbb {P}$
be adequate. Then the sentence “
$\mathbb {P}$
be adequate. Then the sentence “
![]() $\kappa $
is a
$\kappa $
is a
![]() $\mathbb {P}$
-reflecting cardinal” is
$\mathbb {P}$
-reflecting cardinal” is
![]() $:$
$:$
-
(1)
 $\Pi _{2}^{\mathcal {L}}$
, with
$\Pi _{2}^{\mathcal {L}}$
, with
 $\kappa $
as a parameter.
$\kappa $
as a parameter. -
(2)
 $\Pi _{m+2}$
, if
$\Pi _{m+2}$
, if
 $\mathbb {P}$
is
$\mathbb {P}$
is
 $\Gamma _m$
-definable, with
$\Gamma _m$
-definable, with
 $m\geq 1$
.
$m\geq 1$
. -
(3)
 $\Pi _{m+1 }$
, if
$\Pi _{m+1 }$
, if
 $\mathbb {P}$
is
$\mathbb {P}$
is
 $\Delta _m$
-definable, with
$\Delta _m$
-definable, with
 $m\geq 1$
.
$m\geq 1$
.
Proof Note that
![]() $\mathbb {P}$
forces
$\mathbb {P}$
forces
![]() $V[\dot {G}]_\kappa \subseteq V_\kappa [\dot {G}_\kappa ]$
if and only if the
$V[\dot {G}]_\kappa \subseteq V_\kappa [\dot {G}_\kappa ]$
if and only if the
![]() $\mathcal {L}$
-sentence
$\mathcal {L}$
-sentence
holds in
![]() $\langle V,\in ,\mathbb {P}\rangle $
. Thus, since
$\langle V,\in ,\mathbb {P}\rangle $
. Thus, since
![]() $V^{\mathbb {P}}$
is a
$V^{\mathbb {P}}$
is a
![]() $\Delta _1^{\mathcal {L}}$
-definable class, and the expressions “
$\Delta _1^{\mathcal {L}}$
-definable class, and the expressions “
![]() $p\Vdash _{\mathbb {P}} rk(\tau )<\kappa $
” and “
$p\Vdash _{\mathbb {P}} rk(\tau )<\kappa $
” and “
![]() $q\Vdash _{\mathbb {P}}\sigma =\tau $
” are also
$q\Vdash _{\mathbb {P}}\sigma =\tau $
” are also
![]() $\Delta _1^{\mathcal {L}}$
(Corollary 3.12), it is easily seen that the sentence “
$\Delta _1^{\mathcal {L}}$
(Corollary 3.12), it is easily seen that the sentence “
![]() $\kappa $
is a
$\kappa $
is a
![]() $\mathbb {P}$
-reflecting cardinal” is
$\mathbb {P}$
-reflecting cardinal” is
![]() $\Pi _{2}^{\mathcal {L}}$
, with
$\Pi _{2}^{\mathcal {L}}$
, with
![]() $\kappa $
as an additional parameter.
$\kappa $
as an additional parameter.
Items (2) and (3) follow easily from (1) using Proposition 3.6.
Let us consider, for the sake of conciseness, the following strengthening of the notion of
![]() $\mathbb {P}$
-reflecting cardinal (cf. Definition 3.1).
$\mathbb {P}$
-reflecting cardinal (cf. Definition 3.1).
Definition 3.15. If
![]() $k\geq 1$
and
$k\geq 1$
and
![]() $\mathbb {P}$
is an
$\mathbb {P}$
is an
![]() ${\mathrm {ORD}}$
-length forcing iteration, then a cardinal
${\mathrm {ORD}}$
-length forcing iteration, then a cardinal
![]() $\kappa $
is
$\kappa $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _k$
-reflecting if it is
$\Sigma _k$
-reflecting if it is
![]() $\mathbb {P}$
-reflecting, belongs to
$\mathbb {P}$
-reflecting, belongs to
![]() $C^{(k)}_{\mathbb {P}}$
, and
$C^{(k)}_{\mathbb {P}}$
, and
![]() $\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
.
$\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
.
The next lemma will be crucial in future arguments.
Lemma 3.16. Suppose
![]() $\mathbb {P}$
is adequate. Then for every
$\mathbb {P}$
is adequate. Then for every
![]() $k\geq 0$
, if
$k\geq 0$
, if
![]() $\kappa $
is
$\kappa $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _k$
-reflecting and
$\Sigma _k$
-reflecting and
![]() $V_\kappa \models ZFC^*$
, then
$V_\kappa \models ZFC^*$
, then
![]() $\mathbb {P}$
forces
$\mathbb {P}$
forces
![]() $V[\dot {G}]_\kappa \prec _{\Sigma _k} V[\dot {G}]$
.
$V[\dot {G}]_\kappa \prec _{\Sigma _k} V[\dot {G}]$
.
Proof The claim is clear for
![]() $k=0$
. So, assume inductively that
$k=0$
. So, assume inductively that
![]() $\mathbb {P}$
forces
$\mathbb {P}$
forces
![]() $V[\dot {G}]_\kappa \prec _{\Sigma _{k-1}} V[\dot {G}]$
. Notice that, as
$V[\dot {G}]_\kappa \prec _{\Sigma _{k-1}} V[\dot {G}]$
. Notice that, as
![]() $\kappa $
is
$\kappa $
is
![]() $\mathbb {P}$
-reflecting, any member of
$\mathbb {P}$
-reflecting, any member of
![]() $V[\dot {G}]_\kappa $
is given by a
$V[\dot {G}]_\kappa $
is given by a
![]() $\mathbb {P}$
-name in
$\mathbb {P}$
-name in
![]() $V_\kappa $
. Let
$V_\kappa $
. Let
![]() $\varphi ({x})$
be a
$\varphi ({x})$
be a
![]() $\Sigma _k$
formula in the language of set theory and let
$\Sigma _k$
formula in the language of set theory and let
![]() $\tau \in V_\kappa $
be a
$\tau \in V_\kappa $
be a
![]() $\mathbb {P}_\kappa $
-name such that
$\mathbb {P}_\kappa $
-name such that
![]() $p\Vdash _{\mathbb {P}}\varphi (\tau )$
, for some
$p\Vdash _{\mathbb {P}}\varphi (\tau )$
, for some
![]() $p\in \mathbb {P}$
. We only need to show that
$p\in \mathbb {P}$
. We only need to show that
![]() $p\Vdash _{\mathbb {P}} \text {"}V[\dot {G}]_\kappa \models \varphi (\tau ).\text {"}$
$p\Vdash _{\mathbb {P}} \text {"}V[\dot {G}]_\kappa \models \varphi (\tau ).\text {"}$
Claim 3.16.1. The set of conditions
![]() $q\in \mathbb {P}_\kappa $
such that
$q\in \mathbb {P}_\kappa $
such that
![]() $q\Vdash _{\mathbb {P}}\varphi (\tau )$
is dense below
$q\Vdash _{\mathbb {P}}\varphi (\tau )$
is dense below
![]() $p\restriction \kappa $
.
$p\restriction \kappa $
.
Proof of claim Suppose, aiming for a contradiction, that
![]() $p' \leq _{\mathbb {P}_\kappa } p\restriction \kappa $
is such that
$p' \leq _{\mathbb {P}_\kappa } p\restriction \kappa $
is such that
![]() $q\not \Vdash _{\mathbb {P}}\varphi (\tau )$
, for all
$q\not \Vdash _{\mathbb {P}}\varphi (\tau )$
, for all
![]() $q\leq _{\mathbb {P}_\kappa } p'$
. Since
$q\leq _{\mathbb {P}_\kappa } p'$
. Since
![]() $\langle V_\kappa ,\in ,\mathbb {P}_\kappa \rangle \prec _{\Sigma _k}\langle V,\in ,\mathbb {P}\rangle $
, and for every
$\langle V_\kappa ,\in ,\mathbb {P}_\kappa \rangle \prec _{\Sigma _k}\langle V,\in ,\mathbb {P}\rangle $
, and for every
![]() $q\in \mathbb {P}_\kappa $
the sentence “
$q\in \mathbb {P}_\kappa $
the sentence “
![]() $q \not \Vdash _{\mathbb {P}} \varphi (\tau )$
” is
$q \not \Vdash _{\mathbb {P}} \varphi (\tau )$
” is
![]() $\Pi _{k}^{\mathcal {L}}$
(Proposition 3.13), we have that “
$\Pi _{k}^{\mathcal {L}}$
(Proposition 3.13), we have that “
![]() $q\not \Vdash _{\mathbb {P}_\kappa } \varphi (\tau )$
” holds in
$q\not \Vdash _{\mathbb {P}_\kappa } \varphi (\tau )$
” holds in
![]() $\langle V_\kappa ,\in , \mathbb {P}_\kappa \rangle $
. Therefore,
$\langle V_\kappa ,\in , \mathbb {P}_\kappa \rangle $
. Therefore,
Again, since
![]() $\langle V_\kappa ,\in ,\mathbb {P}_\kappa \rangle \prec _{\Sigma _{k}}\langle V,\in ,\mathbb {P}\rangle $
, and the quoted displayed sentence is
$\langle V_\kappa ,\in ,\mathbb {P}_\kappa \rangle \prec _{\Sigma _{k}}\langle V,\in ,\mathbb {P}\rangle $
, and the quoted displayed sentence is
![]() $\Pi _{k}^{\mathcal {L}}$
,
$\Pi _{k}^{\mathcal {L}}$
,
which yields the desired contradiction to the fact that
![]() $p\Vdash _{\mathbb {P}}\varphi (\tau )$
.
$p\Vdash _{\mathbb {P}}\varphi (\tau )$
.
By the claim,
![]() $p\restriction \kappa \Vdash _{\mathbb {P}}\varphi (\tau )$
, and since
$p\restriction \kappa \Vdash _{\mathbb {P}}\varphi (\tau )$
, and since
![]() $\langle V_\kappa ,\in ,\mathbb {P}_\kappa \rangle \prec _{\Sigma _{k}}\langle V,\in ,\mathbb {P}\rangle $
and the above sentence is
$\langle V_\kappa ,\in ,\mathbb {P}_\kappa \rangle \prec _{\Sigma _{k}}\langle V,\in ,\mathbb {P}\rangle $
and the above sentence is
![]() $\Sigma ^{\mathcal {L}}_{k}$
we have
$\Sigma ^{\mathcal {L}}_{k}$
we have
Thus, if G is
![]() $\mathbb {P}$
-generic over V, with
$\mathbb {P}$
-generic over V, with
![]() $p\in G$
, then
$p\in G$
, then
![]() $V_\kappa [G_\kappa ]\models \varphi (i_{G_\kappa }(\tau ))$
. Hence, since
$V_\kappa [G_\kappa ]\models \varphi (i_{G_\kappa }(\tau ))$
. Hence, since
![]() $i_{G_\kappa }(\tau )=i_G(\tau )$
, and
$i_{G_\kappa }(\tau )=i_G(\tau )$
, and
![]() $\kappa $
is
$\kappa $
is
![]() $\mathbb {P}$
-reflecting,
$\mathbb {P}$
-reflecting,
![]() $V[G]_\kappa \models \varphi (i_G(\tau ))$
, as wanted.
$V[G]_\kappa \models \varphi (i_G(\tau ))$
, as wanted.
For future use, let us calculate the complexity of the predicate “
![]() $\kappa $
is a
$\kappa $
is a
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _k$
-reflecting cardinal” for definable forcing iterations.
$\Sigma _k$
-reflecting cardinal” for definable forcing iterations.
Proposition 3.17. Let
![]() $\mathbb {P}$
be adequate. Then the predicate “
$\mathbb {P}$
be adequate. Then the predicate “
![]() $\kappa $
is
$\kappa $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _k$
-reflecting” is
$\Sigma _k$
-reflecting” is
![]() $:$
$:$
 $$ \begin{align*} \begin{array}{ll} \Pi_{m+2}, & \text{ if } k=1 \text{ and } \mathbb{P} \text{ is } \Gamma_m.\\ \Pi_{m+k}, & \text{ if } k>1 \text{ and } \mathbb{P} \text{ is } \Gamma_m.\\ \Pi_{m+1}, & \text{ if } k=1 \text{ and } \mathbb{P} \text{ is } \Delta_m.\\ \Pi_{m+k-1},& \text{ if } k>1 \text{ and } \mathbb{P} \text{ is } \Delta_m. \end{array} \end{align*} $$
$$ \begin{align*} \begin{array}{ll} \Pi_{m+2}, & \text{ if } k=1 \text{ and } \mathbb{P} \text{ is } \Gamma_m.\\ \Pi_{m+k}, & \text{ if } k>1 \text{ and } \mathbb{P} \text{ is } \Gamma_m.\\ \Pi_{m+1}, & \text{ if } k=1 \text{ and } \mathbb{P} \text{ is } \Delta_m.\\ \Pi_{m+k-1},& \text{ if } k>1 \text{ and } \mathbb{P} \text{ is } \Delta_m. \end{array} \end{align*} $$
Proof First, the assertion “
![]() $\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
” is
$\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
” is
![]() $\Pi _{m+1}$
(in the parameter
$\Pi _{m+1}$
(in the parameter
![]() $\kappa $
) if
$\kappa $
) if
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Pi _m$
-definable, and
$\Pi _m$
-definable, and
![]() $\Pi _m$
if
$\Pi _m$
if
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Sigma _m$
-definable. For the quoted sentence holds if and only if:
$\Sigma _m$
-definable. For the quoted sentence holds if and only if:
Second, by Proposition 3.14, the sentence “
![]() $\kappa $
is a
$\kappa $
is a
![]() $\mathbb {P}$
-reflecting cardinal” is
$\mathbb {P}$
-reflecting cardinal” is
![]() $\Pi _{m+2}$
if
$\Pi _{m+2}$
if
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Gamma _m$
-definable; and it is
$\Gamma _m$
-definable; and it is
![]() $\Pi _{m+1}$
if
$\Pi _{m+1}$
if
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _m$
-definable. Finally, as shown in Proposition 3.9, for
$\Delta _m$
-definable. Finally, as shown in Proposition 3.9, for
![]() $k\geq 0$
the class
$k\geq 0$
the class
![]() $C^{(k)}_{\mathbb {P}}$
is
$C^{(k)}_{\mathbb {P}}$
is
![]() $\Pi _{m+k}$
if
$\Pi _{m+k}$
if
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Pi _m$
or
$\Pi _m$
or
![]() $\Sigma _m$
-definable; and it is
$\Sigma _m$
-definable; and it is
![]() $\Pi _{m+k-1}$
if
$\Pi _{m+k-1}$
if
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _m$
-definable.
$\Delta _m$
-definable.
An easy calculation now shows that the predicate “
![]() $\kappa $
is a
$\kappa $
is a
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _k$
-reflecting cardinal” has the claimed complexity.
$\Sigma _k$
-reflecting cardinal” has the claimed complexity.
4
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _n$
-supercompactness
$\Sigma _n$
-supercompactness
The following definition gives a refinement of the notion of
![]() $\Sigma _n$
-supercompact cardinal, relative to definable iterations.
$\Sigma _n$
-supercompact cardinal, relative to definable iterations.
Definition 4.1 (
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _n$
-supercompactness).
$\Sigma _n$
-supercompactness).
If
![]() $n\geq 1$
and
$n\geq 1$
and
![]() $\mathbb {P}$
is an
$\mathbb {P}$
is an
![]() ${\mathrm {ORD}}$
-length forcing iteration, then a cardinal
${\mathrm {ORD}}$
-length forcing iteration, then a cardinal
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompact if there exists a proper class of
$\Sigma _n$
-supercompact if there exists a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting cardinals, and for every such cardinal
$\Sigma _n$
-reflecting cardinals, and for every such cardinal
![]() $\lambda>\delta $
and every
$\lambda>\delta $
and every
![]() $a\in V_\lambda $
there exist
$a\in V_\lambda $
there exist
![]() $\bar {\delta }<\bar {\lambda }<\delta $
and
$\bar {\delta }<\bar {\lambda }<\delta $
and
![]() $\bar {a}\in V_{\bar {\lambda }}$
, together with an elementary embedding
$\bar {a}\in V_{\bar {\lambda }}$
, together with an elementary embedding
![]() $j: V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that:
$j: V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that:
-
(i)
 $\mathrm {crit}(j)=\bar {\delta }$
and
$\mathrm {crit}(j)=\bar {\delta }$
and
 $j(\bar {\delta })=\delta $
.
$j(\bar {\delta })=\delta $
. -
(ii)
 $j(\bar {a})=a$
.
$j(\bar {a})=a$
. -
(iii)
 $\bar {\lambda }$
is
$\bar {\lambda }$
is
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _n$
-reflecting.
$\Sigma _n$
-reflecting.
The next proposition and corollary unveil the connection between the notions of
![]() $\Sigma _n$
-supercompact and
$\Sigma _n$
-supercompact and
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompact cardinals. For conciseness, let us denote by
$\Sigma _n$
-supercompact cardinals. For conciseness, let us denote by
![]() $\mathcal {S}^{\Sigma _n}$
,
$\mathcal {S}^{\Sigma _n}$
,
![]() $\mathcal {S}^{\Sigma _n}_{\mathbb {P}}$
, and
$\mathcal {S}^{\Sigma _n}_{\mathbb {P}}$
, and
![]() $\mathcal {E}^{(n)}$
the classes of
$\mathcal {E}^{(n)}$
the classes of
![]() $\Sigma _n$
-supercompact,
$\Sigma _n$
-supercompact,
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompact, and
$\Sigma _n$
-supercompact, and
![]() $C^{(n)}$
-extendible cardinals, respectively.
$C^{(n)}$
-extendible cardinals, respectively.
Proposition 4.2. Let
![]() $\mathbb {P}$
be an
$\mathbb {P}$
be an
![]() ${\mathrm {ORD}}$
-length forcing iteration, and suppose there is a proper class of
${\mathrm {ORD}}$
-length forcing iteration, and suppose there is a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting cardinals. Then,
$\Sigma _n$
-reflecting cardinals. Then,
-
(1)
 $\mathcal {S}^{\Sigma _{n}}_{\mathbb {P}}\subseteq \mathcal {S}^{\Sigma _{n}}$
.
$\mathcal {S}^{\Sigma _{n}}_{\mathbb {P}}\subseteq \mathcal {S}^{\Sigma _{n}}$
. -
(2) If
 $\mathbb {P}$
is adequate and
$\mathbb {P}$
is adequate and
 $\Delta _2$
-definable, then
$\Delta _2$
-definable, then
 $\mathcal {S}^{\Sigma _{3}}\subseteq \mathcal {S}^{\Sigma _1}_{\mathbb {P}}$
.
$\mathcal {S}^{\Sigma _{3}}\subseteq \mathcal {S}^{\Sigma _1}_{\mathbb {P}}$
. -
(3) If
 $\mathbb {P}$
is adequate and
$\mathbb {P}$
is adequate and
 $\Delta _{m+1}$
-definable, some
$\Delta _{m+1}$
-definable, some
 $m\geq 1$
, and either m or n are greater than
$m\geq 1$
, and either m or n are greater than
 $1$
, then
$1$
, then
 $\mathcal {S}^{\Sigma _{m+n}}\subseteq \mathcal {S}^{\Sigma _n}_{\mathbb {P}}$
.
$\mathcal {S}^{\Sigma _{m+n}}\subseteq \mathcal {S}^{\Sigma _n}_{\mathbb {P}}$
.
In particular, if
![]() $\mathbb {P}$
is adequate and
$\mathbb {P}$
is adequate and
![]() $\Delta _2$
, then every
$\Delta _2$
, then every
![]() $\Sigma _2$
-supercompact cardinal is
$\Sigma _2$
-supercompact cardinal is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _1$
-supercompact
$\Sigma _1$
-supercompact
![]() $;$
and for every
$;$
and for every
![]() $n>1$
, a cardinal is
$n>1$
, a cardinal is
![]() $\Sigma _{n}$
-supercompact if and only if it is
$\Sigma _{n}$
-supercompact if and only if it is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n}$
-supercompact.
$\Sigma _{n}$
-supercompact.
Proof (1): Assume
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n}$
-supercompact. Let
$\Sigma _{n}$
-supercompact. Let
![]() $\lambda>\delta $
be a
$\lambda>\delta $
be a
![]() $\Sigma _{n}$
-correct cardinal and let
$\Sigma _{n}$
-correct cardinal and let
![]() $a\in V_\lambda $
. Let
$a\in V_\lambda $
. Let
![]() $\kappa>\lambda $
be a
$\kappa>\lambda $
be a
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting cardinal. Notice that
$\Sigma _n$
-reflecting cardinal. Notice that
![]() $V_\kappa \vDash \text {"}\lambda \in C^{(n)}.\text {"}$
By
$V_\kappa \vDash \text {"}\lambda \in C^{(n)}.\text {"}$
By
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompactness, there are
$\Sigma _n$
-supercompactness, there are
![]() $\bar {\delta } < \bar {\kappa }<\delta $
, with
$\bar {\delta } < \bar {\kappa }<\delta $
, with
![]() $\bar {\kappa }$
being
$\bar {\kappa }$
being
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting, and there are
$\Sigma _n$
-reflecting, and there are
![]() $\bar {a}\in V_{\bar {\kappa }}$
and
$\bar {a}\in V_{\bar {\kappa }}$
and
![]() $\bar {\lambda } <\bar {\kappa }$
, together with an elementary embedding
$\bar {\lambda } <\bar {\kappa }$
, together with an elementary embedding
![]() $j:V_{\bar {\kappa }}\longrightarrow V_\kappa $
such that
$j:V_{\bar {\kappa }}\longrightarrow V_\kappa $
such that
![]() $\mathrm {crit}(j)=\bar {\delta } \ j(\bar {\delta })=\delta $
,
$\mathrm {crit}(j)=\bar {\delta } \ j(\bar {\delta })=\delta $
,
![]() $j(\bar {a})=a$
, and
$j(\bar {a})=a$
, and
![]() $j(\bar {\lambda })=\lambda $
. By elementarity,
$j(\bar {\lambda })=\lambda $
. By elementarity,
![]() $V_{\bar {\kappa }}$
thinks that
$V_{\bar {\kappa }}$
thinks that
![]() $\bar {\lambda }$
is a
$\bar {\lambda }$
is a
![]() $\Sigma _{n}$
-correct cardinal. Since
$\Sigma _{n}$
-correct cardinal. Since
![]() $\bar {\kappa }\in C^{(n)}$
, it follows that
$\bar {\kappa }\in C^{(n)}$
, it follows that
![]() $\bar {\lambda }\in C^{(n)}$
.
$\bar {\lambda }\in C^{(n)}$
.
(2) and (3): Assume
![]() $\delta $
is
$\delta $
is
![]() $\Sigma _3$
-supercompact (in case (2), i.e.,
$\Sigma _3$
-supercompact (in case (2), i.e.,
![]() $m=n=1$
) or
$m=n=1$
) or
![]() $\Sigma _{m+n}$
-supercompact, in case (3). Let
$\Sigma _{m+n}$
-supercompact, in case (3). Let
![]() $\lambda>\delta $
be
$\lambda>\delta $
be
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n}$
-reflecting, and let
$\Sigma _{n}$
-reflecting, and let
![]() $\kappa>\lambda $
be a
$\kappa>\lambda $
be a
![]() $\Sigma _{m+n}$
-correct cardinal. Since being a
$\Sigma _{m+n}$
-correct cardinal. Since being a
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n}$
-reflecting cardinal is a
$\Sigma _{n}$
-reflecting cardinal is a
![]() $\Pi _{3}$
property (case (2)), and a
$\Pi _{3}$
property (case (2)), and a
![]() $\Pi _{m+n}$
property otherwise (case (3)),
$\Pi _{m+n}$
property otherwise (case (3)),
![]() $V_\kappa $
thinks that
$V_\kappa $
thinks that
![]() $\lambda $
is
$\lambda $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n}$
-reflecting (cf. Proposition 3.17). By our assumption, there exist
$\Sigma _{n}$
-reflecting (cf. Proposition 3.17). By our assumption, there exist
![]() $\bar {\delta }<\bar {\lambda }<\bar {\kappa }<\delta $
with
$\bar {\delta }<\bar {\lambda }<\bar {\kappa }<\delta $
with
![]() $\bar {\kappa }\in C^{(3)}$
(case (2)), or
$\bar {\kappa }\in C^{(3)}$
(case (2)), or
![]() $\bar {\kappa }\in C^{(m+n)}$
, otherwise, and there exists an elementary embedding
$\bar {\kappa }\in C^{(m+n)}$
, otherwise, and there exists an elementary embedding
![]() $j:V_{\bar {\kappa }}\longrightarrow V_\kappa $
such that
$j:V_{\bar {\kappa }}\longrightarrow V_\kappa $
such that
![]() $\mathrm {crit}(j)=\bar {\delta }$
,
$\mathrm {crit}(j)=\bar {\delta }$
,
![]() $j(\bar {\delta })=\delta $
, and
$j(\bar {\delta })=\delta $
, and
![]() $j(\bar {\lambda })=\lambda $
. By elementarity,
$j(\bar {\lambda })=\lambda $
. By elementarity,
![]() $V_{\bar {\kappa }}$
thinks that
$V_{\bar {\kappa }}$
thinks that
![]() $\bar {\lambda }$
is
$\bar {\lambda }$
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n}$
-reflecting. Since
$\Sigma _{n}$
-reflecting. Since
![]() $V_{\bar {\kappa }}\prec _{\Sigma _{3}}V$
(case (2)), or
$V_{\bar {\kappa }}\prec _{\Sigma _{3}}V$
(case (2)), or
![]() $V_{\bar {\kappa }}\prec _{\Sigma _{m+n}}V$
, otherwise,
$V_{\bar {\kappa }}\prec _{\Sigma _{m+n}}V$
, otherwise,
![]() $\bar {\lambda }$
is
$\bar {\lambda }$
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n}$
-reflecting in
$\Sigma _{n}$
-reflecting in
![]() $\mathrm {V}$
. Thus, the restriction
$\mathrm {V}$
. Thus, the restriction
![]() $j\upharpoonright V_{\bar {\lambda }}$
witnesses the
$j\upharpoonright V_{\bar {\lambda }}$
witnesses the
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompactness of
$\Sigma _n$
-supercompactness of
![]() $\delta $
.
$\delta $
.
The proposition above together with Theorem 2.4 yields the following:
Corollary 4.3. Suppose
![]() $\mathbb {P}$
is an
$\mathbb {P}$
is an
![]() ${\mathrm {ORD}}$
-length forcing iteration and there is a proper class of
${\mathrm {ORD}}$
-length forcing iteration and there is a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals. Then,
$\Sigma _{n+1}$
-reflecting cardinals. Then,
-
(1)
 $\mathcal {S}^{\Sigma _{n+1}}_{\mathbb {P}}\subseteq \mathcal {E}^{(n)}$
.
$\mathcal {S}^{\Sigma _{n+1}}_{\mathbb {P}}\subseteq \mathcal {E}^{(n)}$
. -
(2) If
 $\mathbb {P}$
is adequate and
$\mathbb {P}$
is adequate and
 $\Delta _{m+1}$
-definable, some
$\Delta _{m+1}$
-definable, some
 $m\geq 1$
, then
$m\geq 1$
, then
 $\mathcal {E}^{(m+n)}\subseteq \mathcal {S}^{\Sigma _{n+1}}_{\mathbb {P}}$
.
$\mathcal {E}^{(m+n)}\subseteq \mathcal {S}^{\Sigma _{n+1}}_{\mathbb {P}}$
.
In particular, if
![]() $\mathbb {P}$
is adequate and
$\mathbb {P}$
is adequate and
![]() $\Delta _{2}$
-definable, then for every
$\Delta _{2}$
-definable, then for every
![]() $n\geq 1$
, every
$n\geq 1$
, every
![]() $C^{(n+1)}$
-extendible is
$C^{(n+1)}$
-extendible is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-supercompact.
$\Sigma _{n+1}$
-supercompact.
5 Suitable iterations
The following is a property enjoyed by many well-known ORD-length forcing iterations, such as Jensen’s canonical class forcing for obtaining the global GCH [Reference Jensen17], or the McAloon class-forcing iteration for forcing
![]() $\mathrm {V=HOD}$
[Reference McAloon22]. The property will be needed to prove a general result (Theorem 5.4) about the preservation of
$\mathrm {V=HOD}$
[Reference McAloon22]. The property will be needed to prove a general result (Theorem 5.4) about the preservation of
![]() $C^{(n)}$
-extendibility.
$C^{(n)}$
-extendibility.
Definition 5.1 (Suitable iterations).
An
![]() ${\mathrm {ORD}}$
-length forcing iteration
${\mathrm {ORD}}$
-length forcing iteration
![]() $\mathbb {P}$
is suitable if it is the direct limit of an Easton support iterationFootnote
4
$\mathbb {P}$
is suitable if it is the direct limit of an Easton support iterationFootnote
4
![]() $\langle \langle \mathbb {P}_\alpha :\,\alpha \in {\mathrm {ORD}}\rangle ,\,\langle \dot {\mathbb {Q}}_\alpha :\,\alpha \in {\mathrm {ORD}}\rangle \rangle $
with the property that for each
$\langle \langle \mathbb {P}_\alpha :\,\alpha \in {\mathrm {ORD}}\rangle ,\,\langle \dot {\mathbb {Q}}_\alpha :\,\alpha \in {\mathrm {ORD}}\rangle \rangle $
with the property that for each
![]() $\lambda \in {\mathrm {ORD}}$
there is some
$\lambda \in {\mathrm {ORD}}$
there is some
![]() $\theta \in {\mathrm {ORD}}$
greater than
$\theta \in {\mathrm {ORD}}$
greater than
![]() $\lambda $
such that
$\lambda $
such that
for all
![]() $\nu \geq \theta $
.
$\nu \geq \theta $
.
It is well-known that for suitable
![]() $\mathrm {ORD}$
-length forcing iterations
$\mathrm {ORD}$
-length forcing iterations
![]() $\mathbb {P}$
, the forcing relation
$\mathbb {P}$
, the forcing relation
![]() $\Vdash _{\mathbb {P}}$
is definable, the forcing theorem holds, and forcing with
$\Vdash _{\mathbb {P}}$
is definable, the forcing theorem holds, and forcing with
![]() $\mathbb {P}$
preserves ZFC (see [Reference Friedman12] or [Reference Reitz23]). The condition of eventual
$\mathbb {P}$
preserves ZFC (see [Reference Friedman12] or [Reference Reitz23]). The condition of eventual
![]() $\lambda $
-directed closedness in the definition above can be strengthened on a club proper class. Namely,
$\lambda $
-directed closedness in the definition above can be strengthened on a club proper class. Namely,
Proposition 5.2. Let
![]() $\mathbb {P}$
be a suitable iteration. The class
$\mathbb {P}$
be a suitable iteration. The class
is a club class.
Proof Closedness is obvious. As for unboundedness, fix any
![]() $\lambda $
and build inductively a sequence
$\lambda $
and build inductively a sequence
![]() $\{\theta _n\}_{n\in \omega }$
of ordinals greater than
$\{\theta _n\}_{n\in \omega }$
of ordinals greater than
![]() $\lambda $
such that for all
$\lambda $
such that for all
![]() $\eta \geq \theta _{n+1}$
,
$\eta \geq \theta _{n+1}$
,
Notice now that
![]() $\theta ^*:=\sup _n\theta _n$
is an element of C.
$\theta ^*:=\sup _n\theta _n$
is an element of C.
The next theorem establishes some sufficient conditions for the preservation of
![]() $C^{(n)}$
-extendible cardinals under definable iterations. Recall that a partial ordering
$C^{(n)}$
-extendible cardinals under definable iterations. Recall that a partial ordering
![]() $\mathbb {P}$
is weakly homogeneous if for any
$\mathbb {P}$
is weakly homogeneous if for any
![]() $p,q\in \mathbb {P}$
there is an automorphism
$p,q\in \mathbb {P}$
there is an automorphism
![]() $\pi $
of
$\pi $
of
![]() $\mathbb {P}$
such that
$\mathbb {P}$
such that
![]() $\pi (p)$
and q are compatible.
$\pi (p)$
and q are compatible.
In the case of definable ORD-length forcing iterations we define weak homogeneity as follows:
Definition 5.3. A
![]() $\Gamma _m$
-definable ORD-length forcing iteration
$\Gamma _m$
-definable ORD-length forcing iteration
![]() $\mathbb {P}$
is weakly homogeneous if there exists a
$\mathbb {P}$
is weakly homogeneous if there exists a
![]() $\Gamma _m$
formula
$\Gamma _m$
formula
![]() $\varphi (x,y, z_1, z_2, z_3)$
such that for every
$\varphi (x,y, z_1, z_2, z_3)$
such that for every
![]() $\alpha $
,
$\alpha $
,
![]() $\mathbb {P}_\alpha $
forces that for every
$\mathbb {P}_\alpha $
forces that for every
![]() $\dot {p},\dot {q}\in \dot {\mathbb {Q}}_{\alpha }$
,
$\dot {p},\dot {q}\in \dot {\mathbb {Q}}_{\alpha }$
,
![]() $\varphi (x,y, \dot {p},\dot {q},\alpha )$
defines an automorphism
$\varphi (x,y, \dot {p},\dot {q},\alpha )$
defines an automorphism
![]() $\pi $
of
$\pi $
of
![]() $\dot {\mathbb {Q}}_{\alpha }$
such that
$\dot {\mathbb {Q}}_{\alpha }$
such that
![]() $\pi (\dot {p})$
and
$\pi (\dot {p})$
and
![]() $\dot {q}$
are compatible.
$\dot {q}$
are compatible.
Let us note that if
![]() $\mathbb {P}$
is a weakly homogeneous
$\mathbb {P}$
is a weakly homogeneous
![]() $\Gamma _m$
-definable iteration, and
$\Gamma _m$
-definable iteration, and
![]() $\lambda $
is
$\lambda $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Gamma _m$
-reflecting, then
$\Gamma _m$
-reflecting, then
![]() $\mathbb {P}_\lambda $
is also a weakly homogeneous iteration in
$\mathbb {P}_\lambda $
is also a weakly homogeneous iteration in
![]() $V_\lambda $
, with the same formula witnessing it. Also notice that, for every
$V_\lambda $
, with the same formula witnessing it. Also notice that, for every
![]() $\alpha $
,
$\alpha $
,
![]() $\mathbb {P}_\alpha $
forces that the remaining part of the iteration is weakly homogeneous. Indeed, in
$\mathbb {P}_\alpha $
forces that the remaining part of the iteration is weakly homogeneous. Indeed, in
![]() $V^{\mathbb {P}_\alpha }$
, for every
$V^{\mathbb {P}_\alpha }$
, for every
![]() $p,q\in \mathbb {P}_{[\alpha , {\mathrm {ORD}})}$
, the map
$p,q\in \mathbb {P}_{[\alpha , {\mathrm {ORD}})}$
, the map
![]() $\pi $
given by:
$\pi $
given by:
![]() $\pi (x)=y$
iff for all
$\pi (x)=y$
iff for all
![]() $\beta>0$
,
$\beta>0$
,
is a definable automorphism of
![]() $\dot {\mathbb {P}}_{[\alpha , {\mathrm {ORD}})}$
(with parameters
$\dot {\mathbb {P}}_{[\alpha , {\mathrm {ORD}})}$
(with parameters
![]() $\dot {p}$
and
$\dot {p}$
and
![]() $\dot {q}$
) such that
$\dot {q}$
) such that
![]() forces
forces
![]() $\pi (\dot {p})$
and
$\pi (\dot {p})$
and
![]() $\dot {q}$
to be compatible.
$\dot {q}$
to be compatible.
Theorem 5.4. Let
![]() $m,n\geq 1$
and
$m,n\geq 1$
and
![]() $m\leq n$
. Let
$m\leq n$
. Let
![]() $\mathbb {P}$
be an adequate
$\mathbb {P}$
be an adequate
![]() $\Delta _{m+1}$
-definable and weakly homogeneous suitable iteration. Suppose there is a proper class of
$\Delta _{m+1}$
-definable and weakly homogeneous suitable iteration. Suppose there is a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals. If
$\Sigma _{n+1}$
-reflecting cardinals. If
![]() $\delta $
is a
$\delta $
is a
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-supercompact cardinal, then
$\Sigma _{n+1}$
-supercompact cardinal, then
Proof Suppose G is
![]() $\mathbb {P}$
-generic over
$\mathbb {P}$
-generic over
![]() $\mathrm {V}$
. By Corollary 2.7 and Lemma 3.16, it is sufficient to take an arbitrary
$\mathrm {V}$
. By Corollary 2.7 and Lemma 3.16, it is sufficient to take an arbitrary
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinal
$\Sigma _{n+1}$
-reflecting cardinal
![]() $\lambda>\delta $
and any
$\lambda>\delta $
and any
![]() $\alpha <\lambda $
, and find a
$\alpha <\lambda $
, and find a
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinal
$\Sigma _{n+1}$
-reflecting cardinal
![]() $\bar {\lambda }$
, ordinals
$\bar {\lambda }$
, ordinals
![]() $\bar {\delta }, \bar {\alpha }<\bar {\lambda }$
, and an elementary embedding
$\bar {\delta }, \bar {\alpha }<\bar {\lambda }$
, and an elementary embedding
![]() $j: V[G]_{\bar {\lambda }}\longrightarrow V[G]_\lambda $
such that
$j: V[G]_{\bar {\lambda }}\longrightarrow V[G]_\lambda $
such that
![]() $\mathrm {crit}(j)=\bar {\delta }$
,
$\mathrm {crit}(j)=\bar {\delta }$
,
![]() $j(\bar {\delta })=\delta $
, and
$j(\bar {\delta })=\delta $
, and
![]() $j(\bar {\alpha })=\alpha $
.
$j(\bar {\alpha })=\alpha $
.
So pick a
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinal
$\Sigma _{n+1}$
-reflecting cardinal
![]() $\lambda>\delta $
and any
$\lambda>\delta $
and any
![]() $\alpha <\lambda $
. Since
$\alpha <\lambda $
. Since
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-supercompact there exist
$\Sigma _{n+1}$
-supercompact there exist
![]() $\bar {\delta }<\bar {\lambda }< \delta $
and
$\bar {\delta }<\bar {\lambda }< \delta $
and
![]() $\bar {\alpha }<\bar {\lambda }$
, together with an elementary embedding
$\bar {\alpha }<\bar {\lambda }$
, together with an elementary embedding
![]() $j: V_{\bar {\lambda }}\longrightarrow V_{\lambda }$
such that
$j: V_{\bar {\lambda }}\longrightarrow V_{\lambda }$
such that
![]() $:$
$:$
-
(i)
 $\mathrm {crit}(j)=\bar {\delta }$
and
$\mathrm {crit}(j)=\bar {\delta }$
and
 $j(\bar {\delta })=\delta $
.
$j(\bar {\delta })=\delta $
. -
(ii)
 $j(\bar {\alpha })=\alpha $
.
$j(\bar {\alpha })=\alpha $
. -
(iii)
 $\bar {\lambda }$
is
$\bar {\lambda }$
is
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _{n+1}$
-reflecting.
$\Sigma _{n+1}$
-reflecting.
It will then suffice to show that j can be lifted to an elementary embedding
![]() $j:V_{\bar {\lambda }}[G_{\bar {\lambda }}]\longrightarrow V_\lambda [G_\lambda ]$
, for then, since both
$j:V_{\bar {\lambda }}[G_{\bar {\lambda }}]\longrightarrow V_\lambda [G_\lambda ]$
, for then, since both
![]() $\lambda $
and
$\lambda $
and
![]() $\bar {\lambda }$
are
$\bar {\lambda }$
are
![]() $\mathbb {P}$
-reflecting, we have that
$\mathbb {P}$
-reflecting, we have that
![]() $V_\lambda [G_\lambda ]=V[G]_\lambda $
and
$V_\lambda [G_\lambda ]=V[G]_\lambda $
and
![]() $V_{\bar {\lambda }}[G_{\bar {\lambda }}]=V[G]_{\bar {\lambda }}$
.
$V_{\bar {\lambda }}[G_{\bar {\lambda }}]=V[G]_{\bar {\lambda }}$
.
The iterations
![]() $\mathbb {P}_{\bar {\lambda }}$
and
$\mathbb {P}_{\bar {\lambda }}$
and
![]() $\mathbb {P}_{{\lambda }}$
factorize as follows:
$\mathbb {P}_{{\lambda }}$
factorize as follows:
-
(i)
 $\mathbb {P}_{\bar {\lambda }}\cong \mathbb {P}_{\bar {\delta }}\ast \mathbb {Q}$
with
$\mathbb {P}_{\bar {\lambda }}\cong \mathbb {P}_{\bar {\delta }}\ast \mathbb {Q}$
with
 $|\mathbb {Q}|\leq \bar {\lambda }$
.
$|\mathbb {Q}|\leq \bar {\lambda }$
. -
(ii)
 $\mathbb {P}_{{\lambda }}\cong \mathbb {P}_{{\delta }}\ast \mathbb {Q}^*$
with
$\mathbb {P}_{{\lambda }}\cong \mathbb {P}_{{\delta }}\ast \mathbb {Q}^*$
with  $$ \begin{align*} \Vdash_{\mathbb{P}_{\delta}} \text{"}\mathbb{Q}^* \text{ is weakly homogeneous and } \delta\text{-directed closed."} \end{align*} $$
$$ \begin{align*} \Vdash_{\mathbb{P}_{\delta}} \text{"}\mathbb{Q}^* \text{ is weakly homogeneous and } \delta\text{-directed closed."} \end{align*} $$
Indeed, (i) is clear since
![]() $\bar {\lambda }$
is a
$\bar {\lambda }$
is a
![]() $\beth $
-fixed point. For (ii), since
$\beth $
-fixed point. For (ii), since
![]() $\mathbb {P}$
is weakly homogeneous and
$\mathbb {P}$
is weakly homogeneous and
![]() $\lambda $
is
$\lambda $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting,
$\Sigma _{n+1}$
-reflecting,
![]() $\mathbb {P}_\lambda $
is weakly homogeneous in
$\mathbb {P}_\lambda $
is weakly homogeneous in
![]() $V_\lambda $
, and therefore
$V_\lambda $
, and therefore
![]() $\mathbb {P}_\delta $
forces that
$\mathbb {P}_\delta $
forces that
![]() $\mathbb {Q}^\ast $
is weakly homogeneous. Thus, we only need to see that
$\mathbb {Q}^\ast $
is weakly homogeneous. Thus, we only need to see that
![]() $\Vdash _{\mathbb {P}_{\delta }}$
“
$\Vdash _{\mathbb {P}_{\delta }}$
“
![]() $\mathbb {Q}^*$
is
$\mathbb {Q}^*$
is
![]() $\delta $
-directed closed.”
$\delta $
-directed closed.”
Recall from Proposition 5.2 that the class
is a club class. Thus, it will be sufficient to show that
![]() $\delta $
is a limit point of
$\delta $
is a limit point of
![]() $C(\mathbb {P})$
, and therefore it belongs to
$C(\mathbb {P})$
, and therefore it belongs to
![]() $C(\mathbb {P})$
. So, let
$C(\mathbb {P})$
. So, let
![]() $\mu <\delta $
and notice that since
$\mu <\delta $
and notice that since
![]() $\mathbb {P}$
is a suitable iteration, the sentence
$\mathbb {P}$
is a suitable iteration, the sentence
![]() $\varphi (\mu )$
asserting:
$\varphi (\mu )$
asserting:
holds in
![]() $\mathrm {V}$
. Since
$\mathrm {V}$
. Since
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _{m+1}$
-definable,
$\Delta _{m+1}$
-definable,
![]() $\varphi (\mu )$
is easily seen to be equivalent to the
$\varphi (\mu )$
is easily seen to be equivalent to the
![]() $\Sigma _{m+2}$
sentence
$\Sigma _{m+2}$
sentence
Since
![]() $\delta $
is a
$\delta $
is a
![]() $\Sigma _{n+2}$
-correct cardinal (by Lemma 2.3 and Proposition 4.2), and
$\Sigma _{n+2}$
-correct cardinal (by Lemma 2.3 and Proposition 4.2), and
![]() $m\leq n$
, there must be a witness for
$m\leq n$
, there must be a witness for
![]() $\varphi (\mu )$
below
$\varphi (\mu )$
below
![]() $\delta $
. Arguing inductively, we define an
$\delta $
. Arguing inductively, we define an
![]() $\omega $
-sequence of ordinals above
$\omega $
-sequence of ordinals above
![]() $\mu $
with limit in
$\mu $
with limit in
![]() $C(\mathbb {P})$
. This shows that
$C(\mathbb {P})$
. This shows that
![]() $C(\mathbb {P})$
is unbounded in
$C(\mathbb {P})$
is unbounded in
![]() $\delta $
, as wanted.
$\delta $
, as wanted.
Since
![]() $\delta \in C^{(n+2)}$
, and j is elementary with
$\delta \in C^{(n+2)}$
, and j is elementary with
![]() $j(\bar {\delta })=\delta $
, we have that
$j(\bar {\delta })=\delta $
, we have that
![]() $j(\mathbb {P}_{\bar {\delta }})=\mathbb {P}_\delta $
. Also, since
$j(\mathbb {P}_{\bar {\delta }})=\mathbb {P}_\delta $
. Also, since
![]() $\bar {\delta }$
is the critical point of j, we have that
$\bar {\delta }$
is the critical point of j, we have that
![]() $j\text {"}G_{\bar {\delta }}=G_{\bar {\delta }} \subseteq G_\delta $
, and so
$j\text {"}G_{\bar {\delta }}=G_{\bar {\delta }} \subseteq G_\delta $
, and so
![]() $j\restriction V_{\bar {\lambda }}$
can be lifted to an elementary embedding
$j\restriction V_{\bar {\lambda }}$
can be lifted to an elementary embedding
Let us denote by
![]() $G_{[\bar {\delta },\bar {\lambda })}$
and
$G_{[\bar {\delta },\bar {\lambda })}$
and
![]() $G_{[{\delta },{\lambda })}$
the filters
$G_{[{\delta },{\lambda })}$
the filters
![]() $G\cap \mathbb {Q}$
and
$G\cap \mathbb {Q}$
and
![]() $G\cap \mathbb {Q}^*$
, respectively. Notice that these filters are generic for
$G\cap \mathbb {Q}^*$
, respectively. Notice that these filters are generic for
![]() $\mathbb {Q}$
and
$\mathbb {Q}$
and
![]() $\mathbb {Q}^*$
over
$\mathbb {Q}^*$
over
![]() $V_{\bar {\lambda }}[G_{\bar {\delta }}]$
and
$V_{\bar {\lambda }}[G_{\bar {\delta }}]$
and
![]() $V_{\lambda }[G_\delta ]$
, respectively. In order to lift the embedding j to the further generic extension
$V_{\lambda }[G_\delta ]$
, respectively. In order to lift the embedding j to the further generic extension
![]() $V_{\bar {\lambda }}[G_{\bar {\lambda }}]=V_{\bar {\lambda }}[G_{\bar {\delta }}][G_{[\bar {\delta },\bar {\lambda })}]$
, notice first that
$V_{\bar {\lambda }}[G_{\bar {\lambda }}]=V_{\bar {\lambda }}[G_{\bar {\delta }}][G_{[\bar {\delta },\bar {\lambda })}]$
, notice first that
![]() $j"G_{[\bar {\delta },\bar {\lambda })}$
is a directed subset of
$j"G_{[\bar {\delta },\bar {\lambda })}$
is a directed subset of
![]() $\mathbb {Q}^\ast $
of cardinality
$\mathbb {Q}^\ast $
of cardinality
![]() $\leq \bar {\lambda }$
. Also, since
$\leq \bar {\lambda }$
. Also, since
![]() $j\upharpoonright V_{\bar {\lambda }}\in V_\lambda [G_\delta ]$
, and
$j\upharpoonright V_{\bar {\lambda }}\in V_\lambda [G_\delta ]$
, and
![]() $j"G_{[\bar {\delta },\bar {\lambda })}$
can be computed from
$j"G_{[\bar {\delta },\bar {\lambda })}$
can be computed from
![]() $j\upharpoonright V_{\bar {\lambda }}$
and
$j\upharpoonright V_{\bar {\lambda }}$
and
![]() $G_{[\bar {\delta },\bar {\lambda })}$
, we have that
$G_{[\bar {\delta },\bar {\lambda })}$
, we have that
![]() $j"G_{[\bar {\delta },\bar {\lambda })}\in V_\lambda [G_\delta ]$
. Therefore, since
$j"G_{[\bar {\delta },\bar {\lambda })}\in V_\lambda [G_\delta ]$
. Therefore, since
![]() $\mathbb {Q}^*$
is a
$\mathbb {Q}^*$
is a
![]() $\delta $
-directed closed forcing notion in
$\delta $
-directed closed forcing notion in
![]() $V_{\lambda }[G_\delta ]$
, there is some condition
$V_{\lambda }[G_\delta ]$
, there is some condition
![]() $p\in \mathbb {Q}^*$
such that
$p\in \mathbb {Q}^*$
such that
![]() $p\leq q$
, for every
$p\leq q$
, for every
![]() $q\in j"G_{[\bar {\delta },\bar {\lambda })}$
. Thus, p is a master condition in
$q\in j"G_{[\bar {\delta },\bar {\lambda })}$
. Thus, p is a master condition in
![]() $\mathbb {Q}^*$
for the embedding j and the generic filter
$\mathbb {Q}^*$
for the embedding j and the generic filter
![]() $G_{[\bar {\delta },\bar {\lambda })}$
. So, if
$G_{[\bar {\delta },\bar {\lambda })}$
. So, if
![]() $H\subseteq \mathbb {Q}^*$
is a generic filter over
$H\subseteq \mathbb {Q}^*$
is a generic filter over
![]() $V_{\lambda }[G_\delta ]$
containing p, then j can be lifted to an elementary embedding
$V_{\lambda }[G_\delta ]$
containing p, then j can be lifted to an elementary embedding
Claim 5.4.1. In
![]() $V[G]$
there exists some generic filter
$V[G]$
there exists some generic filter
![]() $H\subseteq \mathbb {Q}^*$
over
$H\subseteq \mathbb {Q}^*$
over
![]() $V_{\lambda }[G_\delta ]$
containing p such that
$V_{\lambda }[G_\delta ]$
containing p such that
![]() $V_{\lambda }[G_\delta \ast H]=V_{\lambda }[G_\lambda ]$
.
$V_{\lambda }[G_\delta \ast H]=V_{\lambda }[G_\lambda ]$
.
Proof of claim By (ii) above,
![]() $\mathbb {Q}^*$
is a weakly homogeneous class forcing in
$\mathbb {Q}^*$
is a weakly homogeneous class forcing in
![]() $V_{\lambda }[G_\delta ]$
. Thus, the set of conditions
$V_{\lambda }[G_\delta ]$
. Thus, the set of conditions
![]() $r\in \mathbb {Q}^*$
for which there is an automorphism
$r\in \mathbb {Q}^*$
for which there is an automorphism
![]() $\pi $
of
$\pi $
of
![]() $\mathbb {Q}^*$
that is definable in
$\mathbb {Q}^*$
that is definable in
![]() $V_\lambda [G_\delta ]$
and such that
$V_\lambda [G_\delta ]$
and such that
![]() $\pi (r)\leq p$
is dense. Pick such an r in
$\pi (r)\leq p$
is dense. Pick such an r in
![]() $G_{[\delta ,\lambda )}$
and such an automorphism
$G_{[\delta ,\lambda )}$
and such an automorphism
![]() $\pi $
. Now, notice that the filter H generated by the set
$\pi $
. Now, notice that the filter H generated by the set
![]() $\pi "G_{[\delta ,\lambda )}$
contains
$\pi "G_{[\delta ,\lambda )}$
contains
![]() $\pi (r)$
and therefore it contains p. Since H is definable by means of
$\pi (r)$
and therefore it contains p. Since H is definable by means of
![]() $\pi $
and
$\pi $
and
![]() $G_{[\delta ,\lambda )}$
, and also
$G_{[\delta ,\lambda )}$
, and also
![]() $\pi $
is definable in
$\pi $
is definable in
![]() $V_\lambda [G_\delta ]$
, we conclude that
$V_\lambda [G_\delta ]$
, we conclude that
![]() $V_{\lambda }[G_\delta \ast H]=V_{\lambda }[G_\lambda ]$
.
$V_{\lambda }[G_\delta \ast H]=V_{\lambda }[G_\lambda ]$
.
By taking
![]() $H\subseteq \mathbb {Q}^*$
as in the claim above, we thus obtain a lifting
$H\subseteq \mathbb {Q}^*$
as in the claim above, we thus obtain a lifting
as wanted.
Remark 5.5. Note that the above proof shows more than what is stated in Theorem 5.4. Specifically, what is proved is the following local result: every elementary embedding (in V) witnessing some partial degree of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-supercompactness lifts to an elementary embedding (in
$\Sigma _{n+1}$
-supercompactness lifts to an elementary embedding (in
![]() $V^{\mathbb {P}}$
) witnessing the same partial degree of
$V^{\mathbb {P}}$
) witnessing the same partial degree of
![]() $C^{(n)}$
-extendibility.
$C^{(n)}$
-extendibility.
Corollary 5.6. Suppose
![]() $n\geq 1$
. Let
$n\geq 1$
. Let
![]() $\mathbb {P}$
be an adequate
$\mathbb {P}$
be an adequate
![]() $\Delta _2$
-definable and weakly homogeneous suitable iteration. Suppose there is a proper class of
$\Delta _2$
-definable and weakly homogeneous suitable iteration. Suppose there is a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals. If
$\Sigma _{n+1}$
-reflecting cardinals. If
![]() $\delta $
is a
$\delta $
is a
![]() $C^{(n+1)}$
-extendible cardinal, then
$C^{(n+1)}$
-extendible cardinal, then
Proof Since
![]() $\mathbb {P}$
is adequate,
$\mathbb {P}$
is adequate,
![]() $\Delta _2$
-definable, and there exists a proper class of
$\Delta _2$
-definable, and there exists a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals, Corollary 4.3 implies that
$\Sigma _{n+1}$
-reflecting cardinals, Corollary 4.3 implies that
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-supercompact. Now, Theorem 5.4 applies to get the desired result.
$\Sigma _{n+1}$
-supercompact. Now, Theorem 5.4 applies to get the desired result.
Let
![]() $\mathbb {P}$
be an adequate
$\mathbb {P}$
be an adequate
![]() $\Delta _{m+1}$
-definable suitable iteration. Let us look next into the conditions under which, for some
$\Delta _{m+1}$
-definable suitable iteration. Let us look next into the conditions under which, for some
![]() $n\geq 1$
, there exists a proper class of
$n\geq 1$
, there exists a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals (this was one of the assumptions of Theorem 5.4).
$\Sigma _{n+1}$
-reflecting cardinals (this was one of the assumptions of Theorem 5.4).
First, the class
![]() $C^{(n)}_{\mathbb {P}}$
is closed, unbounded, and
$C^{(n)}_{\mathbb {P}}$
is closed, unbounded, and
![]() $\Pi _{m+n}$
-definable (Proposition 3.9). Also, the class D of ordinals
$\Pi _{m+n}$
-definable (Proposition 3.9). Also, the class D of ordinals
![]() $\alpha $
such that
$\alpha $
such that
![]() $\mathbb {P}_\alpha =\mathbb {P}\cap V_\alpha $
is
$\mathbb {P}_\alpha =\mathbb {P}\cap V_\alpha $
is
![]() $\Pi _{m+1}$
-definable (cf. the proof of Proposition 3.17). Observe that D is a proper class by assumption, hence
$\Pi _{m+1}$
-definable (cf. the proof of Proposition 3.17). Observe that D is a proper class by assumption, hence
![]() $\overline {D}$
(the closure of D) is a club proper class. Further, the following holds:
$\overline {D}$
(the closure of D) is a club proper class. Further, the following holds:
Lemma 5.7. The class K of
![]() $\mathbb {P}$
-reflecting cardinals is unbounded.
$\mathbb {P}$
-reflecting cardinals is unbounded.
Proof We begin with the following auxiliary claim:
Claim 5.7.1. For each
![]() $\alpha \in \operatorname {\mathrm {ORD}}$
there is a
$\alpha \in \operatorname {\mathrm {ORD}}$
there is a
![]() $\mathbb {P}$
-name
$\mathbb {P}$
-name
![]() $\tau _\alpha $
such that
$\tau _\alpha $
such that
Proof By induction on
![]() $\alpha $
. If
$\alpha $
. If
![]() $\alpha =0$
, then the result is clear. So, assume that
$\alpha =0$
, then the result is clear. So, assume that
![]() $\langle \tau _\beta \mid \beta <\alpha \rangle $
has already been found, and let us find
$\langle \tau _\beta \mid \beta <\alpha \rangle $
has already been found, and let us find
![]() $\tau _\alpha $
. If
$\tau _\alpha $
. If
![]() $\alpha $
is a limit ordinal, then let
$\alpha $
is a limit ordinal, then let
![]() $\tau _\alpha :=\bigcup _{\beta <\alpha }\tau _\beta $
. Clearly,
$\tau _\alpha :=\bigcup _{\beta <\alpha }\tau _\beta $
. Clearly,
![]() $\tau _\alpha $
is as desired.
$\tau _\alpha $
is as desired.
Now suppose that
![]() $\alpha $
is a successor ordinal; say,
$\alpha $
is a successor ordinal; say,
![]() $\alpha =\bar {\alpha }+1$
. Let
$\alpha =\bar {\alpha }+1$
. Let
![]() $\varphi \colon \delta \rightarrow \tau _{\bar {\alpha }}$
be a bijection, some cardinal
$\varphi \colon \delta \rightarrow \tau _{\bar {\alpha }}$
be a bijection, some cardinal
![]() $\delta $
. Thus,
$\delta $
. Thus,
![]() $\Vdash _{\mathbb {P}}\text {"}\check {\varphi }:\check {\delta }\to \tau _{\bar {\alpha }} \text { is onto.}\text {"}$
Since by the induction hypothesis,
$\Vdash _{\mathbb {P}}\text {"}\check {\varphi }:\check {\delta }\to \tau _{\bar {\alpha }} \text { is onto.}\text {"}$
Since by the induction hypothesis,
![]() $\Vdash _{\mathbb {P}}\text {"}\tau _{\bar {\alpha }}=V[\dot {G}]_{\bar {\alpha }},\text {"}$
we have that
$\Vdash _{\mathbb {P}}\text {"}\tau _{\bar {\alpha }}=V[\dot {G}]_{\bar {\alpha }},\text {"}$
we have that
![]() $\Vdash _{\mathbb {P}}\text {"}\check {\varphi }:\check {\delta }\to V[\dot {G}]_{\bar {\alpha }} \text { is onto.}\text {"}$
As
$\Vdash _{\mathbb {P}}\text {"}\check {\varphi }:\check {\delta }\to V[\dot {G}]_{\bar {\alpha }} \text { is onto.}\text {"}$
As
![]() $\mathbb {P}$
is suitable we can find a cardinal
$\mathbb {P}$
is suitable we can find a cardinal
![]() $\theta $
such that
$\theta $
such that
![]() $\tau _{\bar {\alpha }}$
is a
$\tau _{\bar {\alpha }}$
is a
![]() $\mathbb {P}_\theta $
-name and, moreover,
$\mathbb {P}_\theta $
-name and, moreover,
As the remaining part
![]() $\mathbb {P}_{[\theta ,\operatorname {\mathrm {ORD}})}$
of the iteration does not add any new subsets of
$\mathbb {P}_{[\theta ,\operatorname {\mathrm {ORD}})}$
of the iteration does not add any new subsets of
![]() $\delta $
, hence no new elements of
$\delta $
, hence no new elements of
![]() $V[\dot {G}]_\alpha $
, we have that
$V[\dot {G}]_\alpha $
, we have that
So we can just take
![]() $\tau _\alpha $
to be a
$\tau _\alpha $
to be a
![]() $\mathbb {P}_\theta $
-name for
$\mathbb {P}_\theta $
-name for
![]() $V[\dot {G}_\theta ]_\alpha $
, where
$V[\dot {G}_\theta ]_\alpha $
, where
![]() $\dot {G}_\theta $
is the standard
$\dot {G}_\theta $
is the standard
![]() $\mathbb {P}_\theta $
-name for the generic.
$\mathbb {P}_\theta $
-name for the generic.
We are now in conditions to show that the class K of
![]() $\mathbb {P}$
-reflecting cardinals is unbounded. To this aim fix any ordinal
$\mathbb {P}$
-reflecting cardinals is unbounded. To this aim fix any ordinal
![]() $\alpha $
and define inductively a sequence of ordinals
$\alpha $
and define inductively a sequence of ordinals
![]() $\langle \alpha _n :n<\omega \rangle $
in D, with
$\langle \alpha _n :n<\omega \rangle $
in D, with
![]() $\alpha _0=\alpha $
and
$\alpha _0=\alpha $
and
![]() $\Vdash _{\mathbb {P}}\text {"}V[\dot {G}]_{\alpha _n}\subseteq V_{\alpha _{n+1}}[\dot {G}_{\alpha _{n+1}}],\text {"}$
as follows: Suppose that
$\Vdash _{\mathbb {P}}\text {"}V[\dot {G}]_{\alpha _n}\subseteq V_{\alpha _{n+1}}[\dot {G}_{\alpha _{n+1}}],\text {"}$
as follows: Suppose that
![]() $\alpha _n$
has already been defined. By the above claim there is a
$\alpha _n$
has already been defined. By the above claim there is a
![]() $\mathbb {P}$
-name
$\mathbb {P}$
-name
![]() $\tau $
such that
$\tau $
such that
![]() $\mathbb {P}$
forces “
$\mathbb {P}$
forces “
![]() $\tau =V[\dot {G}]_{\alpha _n}$
.” Let
$\tau =V[\dot {G}]_{\alpha _n}$
.” Let
![]() $\beta $
be an ordinal such that
$\beta $
be an ordinal such that
![]() $p\in \mathbb {P}_\beta $
and
$p\in \mathbb {P}_\beta $
and
![]() $\mathbb {P}_\beta =\mathbb {P}\cap V_\beta $
. Then,
$\mathbb {P}_\beta =\mathbb {P}\cap V_\beta $
. Then,
![]() $\Vdash _{\mathbb {P}}\text {"}\tau \in V_\beta [\dot {G}_\beta ]\text {"}$
and thus
$\Vdash _{\mathbb {P}}\text {"}\tau \in V_\beta [\dot {G}_\beta ]\text {"}$
and thus
![]() $\Vdash _{\mathbb {P}} \text {"}V[\dot {G}]_{\alpha _n}\subseteq V_{\alpha _{n+1}}[\dot {G}_{\alpha _{n+1}}],\text {"}$
where
$\Vdash _{\mathbb {P}} \text {"}V[\dot {G}]_{\alpha _n}\subseteq V_{\alpha _{n+1}}[\dot {G}_{\alpha _{n+1}}],\text {"}$
where
![]() $\beta :=\alpha _{n+1}$
. Finally, let
$\beta :=\alpha _{n+1}$
. Finally, let
![]() $\lambda $
be the supremum of the
$\lambda $
be the supremum of the
![]() $\alpha _n$
’s. In this case we have that
$\alpha _n$
’s. In this case we have that
![]() $\Vdash _{\mathbb {P}} \text {"}V[\dot {G}]_\lambda \subseteq V_\lambda [\dot {G}_\lambda ],\text {"}$
and so
$\Vdash _{\mathbb {P}} \text {"}V[\dot {G}]_\lambda \subseteq V_\lambda [\dot {G}_\lambda ],\text {"}$
and so
![]() $\lambda $
is
$\lambda $
is
![]() $\mathbb {P}$
-reflecting.
$\mathbb {P}$
-reflecting.
Thus, K is a
![]() $\Pi _{m+2}$
-definable club proper class (see Propositions 3.3 and 3.14). For each
$\Pi _{m+2}$
-definable club proper class (see Propositions 3.3 and 3.14). For each
![]() $n\geq 1$
, set
$n\geq 1$
, set
![]() $\mathcal {K}_{n}:=C^{(n)}_{\mathbb {P}} \cap {K}\cap \overline {D}$
.
$\mathcal {K}_{n}:=C^{(n)}_{\mathbb {P}} \cap {K}\cap \overline {D}$
.
Proposition 5.8. Let
![]() $\mathbb {P}$
be an adequate
$\mathbb {P}$
be an adequate
![]() $\Delta _{m+1}$
-definable suitable iteration. Then
$\Delta _{m+1}$
-definable suitable iteration. Then
![]() $\mathcal {K}_{n+1} \ ($
resp.
$\mathcal {K}_{n+1} \ ($
resp.
![]() $\mathcal {K}_1)$
is a
$\mathcal {K}_1)$
is a
![]() $\Pi _{m+n+1}$
-definable
$\Pi _{m+n+1}$
-definable
![]() $($
resp.
$($
resp.
![]() $\Pi _{m+2})$
club class such that
$\Pi _{m+2})$
club class such that
![]() $\mathcal {K}_{n+1}\cap \mathrm {Reg} \ ($
resp.
$\mathcal {K}_{n+1}\cap \mathrm {Reg} \ ($
resp.
![]() $\mathcal {K}_1\cap \mathrm {Reg})$
is contained in the class of
$\mathcal {K}_1\cap \mathrm {Reg})$
is contained in the class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals
$\Sigma _{n+1}$
-reflecting cardinals
![]() $($
resp.
$($
resp.
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{1}$
-reflecting cardinals).
$\Sigma _{1}$
-reflecting cardinals).
Proof Clearly,
![]() $\mathcal {K}_{n+1}$
is a club class. Also, by the above discussion,
$\mathcal {K}_{n+1}$
is a club class. Also, by the above discussion,
![]() $\mathcal {K}_{n+1}$
is
$\mathcal {K}_{n+1}$
is
![]() $\Pi _{m+n+1}$
, and
$\Pi _{m+n+1}$
, and
![]() $\mathcal {K}_1$
is
$\mathcal {K}_1$
is
![]() $\Pi _{m+2}$
. Let
$\Pi _{m+2}$
. Let
![]() $\kappa \in \mathcal {K}_{n+1}\cap \mathrm {Reg}$
. To show that
$\kappa \in \mathcal {K}_{n+1}\cap \mathrm {Reg}$
. To show that
![]() $\kappa $
is
$\kappa $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting it is enough to verify that
$\Sigma _{n+1}$
-reflecting it is enough to verify that
![]() $\kappa \in D$
.
$\kappa \in D$
.
Since
![]() $\kappa $
is an accumulation point of D,
$\kappa $
is an accumulation point of D,
![]() $\mathbb {P}\cap V_\kappa \subseteq \mathbb {P}_\kappa $
. Conversely,
$\mathbb {P}\cap V_\kappa \subseteq \mathbb {P}_\kappa $
. Conversely,
![]() $\kappa $
is inaccessible, hence
$\kappa $
is inaccessible, hence
![]() $\mathbb {P}_\kappa $
is the direct limit of the previous stages, and so
$\mathbb {P}_\kappa $
is the direct limit of the previous stages, and so
![]() $\mathbb {P}_\kappa \subseteq \mathbb {P}\cap V_\kappa $
. Hence
$\mathbb {P}_\kappa \subseteq \mathbb {P}\cap V_\kappa $
. Hence
![]() $\kappa \in D$
. The proof for
$\kappa \in D$
. The proof for
![]() $\mathcal {K}_1$
is the same.
$\mathcal {K}_1$
is the same.
Recall that if
![]() $\kappa $
is a cardinal and
$\kappa $
is a cardinal and
![]() $\alpha $
an ordinal,
$\alpha $
an ordinal,
-
•
 $\kappa $
is
$\kappa $
is
 $0$
-Mahlo if
$0$
-Mahlo if
 $\kappa $
is inaccessible;
$\kappa $
is inaccessible; -
•
 $\kappa $
is
$\kappa $
is
 $(\alpha +1)$
-Mahlo iff
$(\alpha +1)$
-Mahlo iff
 $\{\lambda <\kappa \mid \lambda \text { is } \alpha \text {-Mahlo}\}$
is stationary;
$\{\lambda <\kappa \mid \lambda \text { is } \alpha \text {-Mahlo}\}$
is stationary; -
• In case
 $\alpha>0$
is a limit ordinal,
$\alpha>0$
is a limit ordinal,
 $\kappa $
is
$\kappa $
is
 $\alpha $
-Mahlo iff
$\alpha $
-Mahlo iff
 $\kappa $
is
$\kappa $
is
 $\beta $
-Mahlo for every
$\beta $
-Mahlo for every
 $\beta <\alpha $
.
$\beta <\alpha $
.
Definition 5.9. For
![]() $n<\omega $
and
$n<\omega $
and
![]() $\alpha \in \mathrm {ORD}$
, the class
$\alpha \in \mathrm {ORD}$
, the class
![]() ${\mathrm {ORD}}$
is
${\mathrm {ORD}}$
is
![]() $\mathbf {\Gamma _n}$
-
$\mathbf {\Gamma _n}$
-
![]() $(\alpha +1)$
-Mahlo if every
$(\alpha +1)$
-Mahlo if every
![]() $\mathbf {\Gamma _n}$
-definableFootnote
5
club proper class of ordinals contains an
$\mathbf {\Gamma _n}$
-definableFootnote
5
club proper class of ordinals contains an
![]() $\alpha $
-Mahlo cardinal.
$\alpha $
-Mahlo cardinal.
Note that a cardinal is Mahlo if and only if it is
![]() $1$
-Mahlo, hence
$1$
-Mahlo, hence
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Gamma _n}$
-Mahlo if and only if
$\mathbf {\Gamma _n}$
-Mahlo if and only if
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Gamma _n}$
-1-Mahlo.
$\mathbf {\Gamma _n}$
-1-Mahlo.
Definition 5.10. A proper class S of ordinals is
![]() $\boldsymbol {\Gamma }_{\mathbf {n}}$
-stationary if S intersects every
$\boldsymbol {\Gamma }_{\mathbf {n}}$
-stationary if S intersects every
![]() $\boldsymbol {\Gamma }_{\mathbf {n}}$
-definable club proper class of ordinals.
$\boldsymbol {\Gamma }_{\mathbf {n}}$
-definable club proper class of ordinals.
Proposition 5.11. Let
![]() $\mathbb {P}$
be an adequate
$\mathbb {P}$
be an adequate
![]() $\Delta _{m+1}$
-definable suitable iteration. If
$\Delta _{m+1}$
-definable suitable iteration. If
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Pi _{m+n+1}}$
-Mahlo, then the class of
$\mathbf {\Pi _{m+n+1}}$
-Mahlo, then the class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals is
$\Sigma _{n+1}$
-reflecting cardinals is
![]() $\mathbf {\Pi _{m+n+1}}$
-stationary, so it is a proper class.
$\mathbf {\Pi _{m+n+1}}$
-stationary, so it is a proper class.
Proof Let
![]() $\mathcal {C}$
be a
$\mathcal {C}$
be a
![]() $\mathbf {\Pi _{m+n+1}}$
club proper class of ordinals. By Proposition 5.8,
$\mathbf {\Pi _{m+n+1}}$
club proper class of ordinals. By Proposition 5.8,
![]() $\mathcal {C}\cap \mathcal {K}_{n+1}$
is a
$\mathcal {C}\cap \mathcal {K}_{n+1}$
is a
![]() $\mathbf {\Pi _{m+n+1}}$
club proper class such that
$\mathbf {\Pi _{m+n+1}}$
club proper class such that
![]() $\mathcal {C}\cap \mathcal {K}_{n+1}\cap \mathrm {Reg}$
is contained in the class of
$\mathcal {C}\cap \mathcal {K}_{n+1}\cap \mathrm {Reg}$
is contained in the class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals. Thus, the class of
$\Sigma _{n+1}$
-reflecting cardinals. Thus, the class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals is
$\Sigma _{n+1}$
-reflecting cardinals is
![]() $\mathbf {\Pi _{m+n+1}}$
-stationary. To show the class is unbounded, given any cardinal
$\mathbf {\Pi _{m+n+1}}$
-stationary. To show the class is unbounded, given any cardinal
![]() $\kappa $
, let
$\kappa $
, let
![]() $\mathcal {C}:=C^{(n+m)}\setminus \kappa ^+$
. Then
$\mathcal {C}:=C^{(n+m)}\setminus \kappa ^+$
. Then
![]() $\mathcal {C}$
is a
$\mathcal {C}$
is a
![]() $\mathbf {\Pi _{m+n+1}}$
-definable club proper class which, as before, contains a
$\mathbf {\Pi _{m+n+1}}$
-definable club proper class which, as before, contains a
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinal.
$\Sigma _{n+1}$
-reflecting cardinal.
Lemma 5.12. Let
![]() $\mathbb {P}$
be a
$\mathbb {P}$
be a
![]() $\Delta _{m+1}$
-definable suitable iteration. If
$\Delta _{m+1}$
-definable suitable iteration. If
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Pi _{m+1}}$
-Mahlo, then
$\mathbf {\Pi _{m+1}}$
-Mahlo, then
![]() $\mathbb {P}$
is adequate.
$\mathbb {P}$
is adequate.
Proof Since
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _{m+1}$
-definable,
$\Delta _{m+1}$
-definable,
![]() $\mathbb {P}^{V_\kappa }=\mathbb {P}\cap V_\kappa $
for every
$\mathbb {P}^{V_\kappa }=\mathbb {P}\cap V_\kappa $
for every
![]() $\kappa \in C^{(m+1)}$
. Since
$\kappa \in C^{(m+1)}$
. Since
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Pi _{m+1}}$
-Mahlo, and direct limits are taken at inaccessible stages of the iteration, the class of inaccessible cardinals
$\mathbf {\Pi _{m+1}}$
-Mahlo, and direct limits are taken at inaccessible stages of the iteration, the class of inaccessible cardinals
![]() $\kappa $
such that
$\kappa $
such that
![]() $\mathbb {P}^{V_\kappa }=\mathbb {P}_\kappa $
is a proper class. Thus,
$\mathbb {P}^{V_\kappa }=\mathbb {P}_\kappa $
is a proper class. Thus,
![]() $\mathbb {P}$
is adequate.
$\mathbb {P}$
is adequate.
The following corollaries now follow immediately from Theorem 5.4, Corollary 5.6, Proposition 5.11, and Lemma 5.12:
Corollary 5.13. Let
![]() $m,n\geq 1$
and
$m,n\geq 1$
and
![]() $m\leq n$
. Let
$m\leq n$
. Let
![]() $\mathbb {P}$
be
$\mathbb {P}$
be
![]() $\Delta _{m+1}$
-definable weakly homogeneous suitable iteration. Suppose
$\Delta _{m+1}$
-definable weakly homogeneous suitable iteration. Suppose
![]() ${\mathrm {ORD}}$
is
${\mathrm {ORD}}$
is
![]() $\mathbf {\Pi _{m+n+1}}$
-Mahlo. If
$\mathbf {\Pi _{m+n+1}}$
-Mahlo. If
![]() $\delta $
is a
$\delta $
is a
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-supercompact cardinal, then
$\Sigma _{n+1}$
-supercompact cardinal, then
Corollary 5.14. Let
![]() $n\geq 1$
and let
$n\geq 1$
and let
![]() $\mathbb {P}$
be
$\mathbb {P}$
be
![]() $\Delta _2$
-definable weakly homogeneous suitable iteration. Suppose
$\Delta _2$
-definable weakly homogeneous suitable iteration. Suppose
![]() ${\mathrm {ORD}}$
is
${\mathrm {ORD}}$
is
![]() $\mathbf {\Pi _{n+2}}$
-Mahlo. If
$\mathbf {\Pi _{n+2}}$
-Mahlo. If
![]() $\delta $
is a
$\delta $
is a
![]() $C^{(n+1)}$
-extendible cardinal, then
$C^{(n+1)}$
-extendible cardinal, then
6 Vopěnka’s principle and suitable iterations
Let us recall the following characterizations of Vopěnka’s Principle, as well as of its restriction to definable classes of structures of a given complexity, in terms of
![]() $C^{(n)}$
-extendible cardinals.
$C^{(n)}$
-extendible cardinals.
Theorem 6.1 [Reference Bagaria, Casacuberta, Mathias and Rosickỳ2].
The following are equivalent
![]() $:$
$:$
-
(1)
 $\mathrm {VP}$
.
$\mathrm {VP}$
. -
(2) For every
 $n\geq 1$
there exists a
$n\geq 1$
there exists a
 $C^{(n)}$
-extendible cardinal.
$C^{(n)}$
-extendible cardinal.
Theorem 6.2 [Reference Bagaria1].
Let
![]() $n\geq 1$
. The following are equivalent
$n\geq 1$
. The following are equivalent
![]() $:$
$:$
-
(1)
 $\mathrm {VP}(\mathbf {\Pi _{n+1}})$
, i.e.,
$\mathrm {VP}(\mathbf {\Pi _{n+1}})$
, i.e.,
 $\mathrm {VP}$
restricted to proper classes of structures that are
$\mathrm {VP}$
restricted to proper classes of structures that are
 ${\Pi _{n+1}}$
-definable with parameters.
${\Pi _{n+1}}$
-definable with parameters. -
(2) There exists a proper class of
 $C^{(n)}$
-extendible cardinals.
$C^{(n)}$
-extendible cardinals.
For
![]() $n=0$
, the equivalence is between
$n=0$
, the equivalence is between
![]() $\mathrm {VP} (\mathbf {\Pi _1})$
and the existence of a proper class of supercompact cardinal. The lightface version also holds. Namely,
$\mathrm {VP} (\mathbf {\Pi _1})$
and the existence of a proper class of supercompact cardinal. The lightface version also holds. Namely,
![]() $\mathrm {VP}(\Pi _{n+1})$
is equivalent to the existence of a
$\mathrm {VP}(\Pi _{n+1})$
is equivalent to the existence of a
![]() $C^{(n)}$
-extendible cardinal (see [Reference Bagaria1]).
$C^{(n)}$
-extendible cardinal (see [Reference Bagaria1]).
Vopěnka’s Principle can be also characterized in terms of the existence of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompact cardinals (cf. Theorems 6.4 and 6.5). The following lemma will be useful for this purpose.
$\Sigma _n$
-supercompact cardinals (cf. Theorems 6.4 and 6.5). The following lemma will be useful for this purpose.
Lemma 6.3. Let
![]() $n\geq 1$
. Then
$n\geq 1$
. Then
![]() $\mathrm {VP}(\mathbf {\Pi _n})$
implies that
$\mathrm {VP}(\mathbf {\Pi _n})$
implies that
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Sigma _{n+1}}$
-
$\mathbf {\Sigma _{n+1}}$
-
![]() $(\kappa +1)$
-Mahlo, for every cardinal
$(\kappa +1)$
-Mahlo, for every cardinal
![]() $\kappa $
.Footnote
6
$\kappa $
.Footnote
6
Proof Let us prove the lemma for
![]() $n>1$
. The case
$n>1$
. The case
![]() $n=1$
is similar, using the fact that
$n=1$
is similar, using the fact that
![]() $\mathrm {VP}(\mathbf {\Pi _1})$
is equivalent to the existence of a proper class of supercompact cardinal, and that every supercompact cardinal belongs to
$\mathrm {VP}(\mathbf {\Pi _1})$
is equivalent to the existence of a proper class of supercompact cardinal, and that every supercompact cardinal belongs to
![]() $C^{(2)}$
. So, let
$C^{(2)}$
. So, let
![]() $n>1$
and assume that
$n>1$
and assume that
![]() $\mathrm {VP}(\mathbf {\Pi _{n}})$
holds.
$\mathrm {VP}(\mathbf {\Pi _{n}})$
holds.
Let
![]() $\mathcal {C}$
be a
$\mathcal {C}$
be a
![]() $\mathbf {\Sigma _{n+1}}$
-definable club proper class of ordinals and let
$\mathbf {\Sigma _{n+1}}$
-definable club proper class of ordinals and let
![]() $\varphi (x,\vec {a})$
be some
$\varphi (x,\vec {a})$
be some
![]() $\Sigma _{n+1}$
-formula defining it. Let
$\Sigma _{n+1}$
-formula defining it. Let
![]() $\kappa $
be a cardinal and
$\kappa $
be a cardinal and
![]() $\lambda $
be a
$\lambda $
be a
![]() $C^{(n-1)}$
-extendible cardinal with
$C^{(n-1)}$
-extendible cardinal with
![]() $\vec {a}\in V_\lambda $
and
$\vec {a}\in V_\lambda $
and
![]() $\kappa <\lambda $
. Note that this
$\kappa <\lambda $
. Note that this
![]() $\lambda $
exists by virtue of Theorem 6.2. We claim that
$\lambda $
exists by virtue of Theorem 6.2. We claim that
![]() $\mathcal {C}\cap \lambda $
is unbounded in
$\mathcal {C}\cap \lambda $
is unbounded in
![]() $\lambda $
. For if
$\lambda $
. For if
![]() $\alpha <\lambda $
, then the sentence “
$\alpha <\lambda $
, then the sentence “
![]() $\exists \beta>\alpha (\beta \in \mathcal {C})$
” is
$\exists \beta>\alpha (\beta \in \mathcal {C})$
” is
![]() $\Sigma _{n+1}$
(with
$\Sigma _{n+1}$
(with
![]() $\vec {a}$
as parameters), hence it is true in
$\vec {a}$
as parameters), hence it is true in
![]() $V_\lambda $
because
$V_\lambda $
because
![]() $\lambda $
is
$\lambda $
is
![]() $C^{(n-1)}$
-extendible and so it belongs to
$C^{(n-1)}$
-extendible and so it belongs to
![]() $C^{(n+1)}$
[Reference Bagaria1, Proposition 3.4]. Since
$C^{(n+1)}$
[Reference Bagaria1, Proposition 3.4]. Since
![]() $\mathcal {C}$
is closed,
$\mathcal {C}$
is closed,
![]() $\lambda \in \mathcal {C}$
. Since
$\lambda \in \mathcal {C}$
. Since
![]() $\lambda $
is
$\lambda $
is
![]() $\lambda $
-Mahlo, hence also
$\lambda $
-Mahlo, hence also
![]() $\kappa $
-Mahlo, the result follows.
$\kappa $
-Mahlo, the result follows.
Theorem 6.4. The following are equivalent
![]() $:$
$:$
-
(1)
 $\mathrm {VP}$
holds.
$\mathrm {VP}$
holds. -
(2) For every
 $n\geq 1$
and every definable weakly homogeneous suitable iteration
$n\geq 1$
and every definable weakly homogeneous suitable iteration
 $\mathbb {P}$
, there is a proper class of
$\mathbb {P}$
, there is a proper class of
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _{n}$
-reflecting cardinals and there exists a proper class of
$\Sigma _{n}$
-reflecting cardinals and there exists a proper class of
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _n$
-supercompact cardinal.
$\Sigma _n$
-supercompact cardinal.
Proof
![]() $(1) \Rightarrow (2)$
: Let
$(1) \Rightarrow (2)$
: Let
![]() $n\geq 1$
and let
$n\geq 1$
and let
![]() $\mathbb {P}$
be
$\mathbb {P}$
be
![]() $\Delta _{m+1}$
-definable weakly homogeneous suitable iteration, some
$\Delta _{m+1}$
-definable weakly homogeneous suitable iteration, some
![]() $m\geq 1$
. By Lemma 5.12,
$m\geq 1$
. By Lemma 5.12,
![]() $\mathbb {P}$
is adequate. By Lemma 6.3,
$\mathbb {P}$
is adequate. By Lemma 6.3,
![]() ${\mathrm {ORD}}$
is
${\mathrm {ORD}}$
is
![]() $\mathbf {\Pi _{m+n+1}}$
-Mahlo and so Proposition 5.11 yields a proper class of
$\mathbf {\Pi _{m+n+1}}$
-Mahlo and so Proposition 5.11 yields a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals. Also, by Corollary 4.3 and Theorem 6.2 we infer that there is a proper class of
$\Sigma _{n+1}$
-reflecting cardinals. Also, by Corollary 4.3 and Theorem 6.2 we infer that there is a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompact cardinal.
$\Sigma _n$
-supercompact cardinal.
![]() $(2)\Rightarrow (1)$
: Since (2) holds, by Corollary 4.3 there exists a proper class of
$(2)\Rightarrow (1)$
: Since (2) holds, by Corollary 4.3 there exists a proper class of
![]() $C^{(n)}$
-extendible cardinals, for every
$C^{(n)}$
-extendible cardinals, for every
![]() $n\geq 1$
, hence by Theorem 6.2,
$n\geq 1$
, hence by Theorem 6.2,
![]() $\mathrm {VP}$
holds.
$\mathrm {VP}$
holds.
The following gives more precise information about the relationship between
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompact cardinals and fragments of
$\Sigma _n$
-supercompact cardinals and fragments of
![]() $\mathrm {VP}$
.
$\mathrm {VP}$
.
Theorem 6.5. Let
![]() $m,n\geq 1$
and let
$m,n\geq 1$
and let
![]() $\mathbb {P}$
be an
$\mathbb {P}$
be an
![]() ${\mathrm {ORD}}$
-length forcing iteration. Then,
${\mathrm {ORD}}$
-length forcing iteration. Then,
-
(1) Assume there is a proper class of
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _{n+1}$
-reflecting cardinals. If there is a
$\Sigma _{n+1}$
-reflecting cardinals. If there is a
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _{n+1}$
-supercompact cardinal, then
$\Sigma _{n+1}$
-supercompact cardinal, then
 $\mathrm {VP}(\Pi _{n+1})$
holds
$\mathrm {VP}(\Pi _{n+1})$
holds
 $;$
and if there is a proper class of
$;$
and if there is a proper class of
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _{n+1}$
-supercompact cardinals, then
$\Sigma _{n+1}$
-supercompact cardinals, then
 $\mathrm {VP}(\mathbf {\Pi _{n+1}})$
holds.
$\mathrm {VP}(\mathbf {\Pi _{n+1}})$
holds. -
(2) If
 $\mathbb {P}$
is
$\mathbb {P}$
is
 $\Delta _{m+1}$
-definable, some
$\Delta _{m+1}$
-definable, some
 $m\geq 1$
, suitable, and weakly homogeneous, then
$m\geq 1$
, suitable, and weakly homogeneous, then
 $\mathrm {VP}(\mathbf {\Pi _{m+n+1}})$
implies the existence of a proper class of
$\mathrm {VP}(\mathbf {\Pi _{m+n+1}})$
implies the existence of a proper class of
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _{n+1}$
-reflecting cardinals and a proper class of
$\Sigma _{n+1}$
-reflecting cardinals and a proper class of
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _{n+1}$
-supercompact cardinals.
$\Sigma _{n+1}$
-supercompact cardinals.
Proof Item (1) is a direct consequence of Corollary 4.3 and our remarks following Theorem 6.2. As for (2), Theorem 6.2 shows that
![]() $\mathrm {VP}(\mathbf {\Pi _{m+n+1}})$
is equivalent to the existence of a proper class of
$\mathrm {VP}(\mathbf {\Pi _{m+n+1}})$
is equivalent to the existence of a proper class of
![]() $C^{(m+n)}$
-extendible cardinals. Also, Lemma 6.3 shows that
$C^{(m+n)}$
-extendible cardinals. Also, Lemma 6.3 shows that
![]() $\mathrm {VP}(\mathbf {\Pi _{m+n+1}})$
implies that ORD is
$\mathrm {VP}(\mathbf {\Pi _{m+n+1}})$
implies that ORD is
![]() $\mathbf {\Sigma _{m+n+2}}$
-Mahlo, hence Lemma 5.12 implies that
$\mathbf {\Sigma _{m+n+2}}$
-Mahlo, hence Lemma 5.12 implies that
![]() $\mathbb {P}$
is adequate. Thus, by Proposition 5.11, there exists a proper class of
$\mathbb {P}$
is adequate. Thus, by Proposition 5.11, there exists a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals. By Corollary 4.3 there exists also a proper class of
$\Sigma _{n+1}$
-reflecting cardinals. By Corollary 4.3 there exists also a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-supercompact cardinals.
$\Sigma _{n+1}$
-supercompact cardinals.
We end this section by proving a level-by-level version of Brooke-Taylor’s result on the preservation of Vopěnka’s Principle under definable suitable iterations.
Theorem 6.6. Let
![]() $m,n\geq 1$
and
$m,n\geq 1$
and
![]() $m\leq n$
. Let
$m\leq n$
. Let
![]() $\mathbb {P}$
be a
$\mathbb {P}$
be a
![]() $\Delta _{m+1}$
-definable weakly homogeneous suitable iteration. If
$\Delta _{m+1}$
-definable weakly homogeneous suitable iteration. If
![]() $\mathrm {VP}(\mathbf {\Pi _{m+n+1}})$
holds, then
$\mathrm {VP}(\mathbf {\Pi _{m+n+1}})$
holds, then
Proof By Theorem 6.5(2), there is a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals, as well as a proper class of
$\Sigma _{n+1}$
-reflecting cardinals, as well as a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-supercompact cardinals. Also, by Lemma 5.12,
$\Sigma _{n+1}$
-supercompact cardinals. Also, by Lemma 5.12,
![]() $\mathbb {P}$
is adequate. Now the result follows combining Theorem 5.4 and the remarks just after Theorem 6.2.
$\mathbb {P}$
is adequate. Now the result follows combining Theorem 5.4 and the remarks just after Theorem 6.2.
Corollary 6.7 [Reference Brooke-Taylor8].
Let
![]() $\mathbb {P}$
be a definable weakly homogeneous suitable iteration. If
$\mathbb {P}$
be a definable weakly homogeneous suitable iteration. If
![]() $\mathrm {VP}$
holds in
$\mathrm {VP}$
holds in
![]() $\mathrm {V}$
, then
$\mathrm {V}$
, then
![]() $\mathrm {VP}$
holds in
$\mathrm {VP}$
holds in
![]() $V^{\mathbb {P}}$
.
$V^{\mathbb {P}}$
.
Our version of Brooke-Taylor’s result differs from the original one in that we require the weak homogeneity of
![]() $\mathbb {P}$
. However, our proof shows more than Brooke-Taylor’s, for it shows that every relevant elementary embedding from the ground model lifts to an elementary embedding in the forcing extension (recall Remark 5.5). Even though weak homogeneity holds for a wide family of forcing notions, it puts some restrictions on the sort of statements that can be forced. One example is “
$\mathbb {P}$
. However, our proof shows more than Brooke-Taylor’s, for it shows that every relevant elementary embedding from the ground model lifts to an elementary embedding in the forcing extension (recall Remark 5.5). Even though weak homogeneity holds for a wide family of forcing notions, it puts some restrictions on the sort of statements that can be forced. One example is “
![]() $\mathrm {V}=\mathrm {HOD}.$
” In Section 9.1 we will address this problem and will prove Theorem 6.6 without the weak homogeneity assumption (Theorem 9.6). We are very grateful to Brooke-Taylor for his valuable comments on this matter.
$\mathrm {V}=\mathrm {HOD}.$
” In Section 9.1 we will address this problem and will prove Theorem 6.6 without the weak homogeneity assumption (Theorem 9.6). We are very grateful to Brooke-Taylor for his valuable comments on this matter.
7
 $C^{(n)}$
-extendible cardinals and fitting iterations
$C^{(n)}$
-extendible cardinals and fitting iterations
While our main corollary from Section 5 (cf. Corollary 5.14) applies to a wide class of forcing iterations, it requires that the cardinal we begin with is
![]() $C^{(n+1)}$
-extendible. In this section we argue that this extra assumption can be avoided by restricting to a certain subclass of forcing iterations, which nevertheless are still general enough.
$C^{(n+1)}$
-extendible. In this section we argue that this extra assumption can be avoided by restricting to a certain subclass of forcing iterations, which nevertheless are still general enough.
Definition 7.1. An
![]() $\mathrm {ORD}$
-length forcing iteration
$\mathrm {ORD}$
-length forcing iteration
![]() $\mathbb {P}$
is fitting if it is
$\mathbb {P}$
is fitting if it is
![]() $\Delta _2$
-definable, suitable, and weakly homogeneous, and there is a
$\Delta _2$
-definable, suitable, and weakly homogeneous, and there is a
![]() $\Delta _2$
-definable
$\Delta _2$
-definable
![]() $\mathbf {\Sigma _{2}}$
-stationary class
$\mathbf {\Sigma _{2}}$
-stationary class
![]() $K_{\mathbb {P}}$
of regular
$K_{\mathbb {P}}$
of regular
![]() $\mathbb {P}$
-reflecting cardinals
$\mathbb {P}$
-reflecting cardinals
![]() $\kappa $
such that
$\kappa $
such that
![]() $\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
.
$\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
.
Remark 7.2. Note that every fitting iteration is adequate. Also notice that
![]() $K_{\mathbb {P}}\cap C^{(1)}$
is a
$K_{\mathbb {P}}\cap C^{(1)}$
is a
![]() $\Delta _2$
-definable proper class of inaccessible cardinals.
$\Delta _2$
-definable proper class of inaccessible cardinals.
Theorem 7.3. Suppose that
![]() $\mathbb {P}$
is a fitting iteration. If
$\mathbb {P}$
is a fitting iteration. If
![]() $\delta $
is a supercompact cardinal in
$\delta $
is a supercompact cardinal in
![]() $C(\mathbb {P})$
, then
$C(\mathbb {P})$
, then
![]() $\Vdash _{\mathbb {P}}\text {"}\delta \text { is supercompact."}$
Footnote
7
$\Vdash _{\mathbb {P}}\text {"}\delta \text { is supercompact."}$
Footnote
7
Proof Let
![]() $\delta $
be a supercompact cardinal in
$\delta $
be a supercompact cardinal in
![]() $C(\mathbb {P})$
and suppose G is a
$C(\mathbb {P})$
and suppose G is a
![]() $\mathbb {P}$
-generic filter over
$\mathbb {P}$
-generic filter over
![]() $\mathrm {V}$
. It will be sufficient to verify that for each
$\mathrm {V}$
. It will be sufficient to verify that for each
![]() $\lambda \in K_{\mathbb {P}}\cap C^{(1)}$
there are
$\lambda \in K_{\mathbb {P}}\cap C^{(1)}$
there are
![]() $\bar {\lambda }\in K_{\mathbb {P}}\cap C^{(1)}$
and
$\bar {\lambda }\in K_{\mathbb {P}}\cap C^{(1)}$
and
![]() $\bar {\delta }$
with
$\bar {\delta }$
with
![]() $\bar {\delta }< \bar {\lambda }<\delta ,$
and an elementary embedding
$\bar {\delta }< \bar {\lambda }<\delta ,$
and an elementary embedding
with
![]() $\mathrm {crit}(j)=\bar {\delta }$
and
$\mathrm {crit}(j)=\bar {\delta }$
and
![]() $j(\bar {\delta })=\delta $
. For since
$j(\bar {\delta })=\delta $
. For since
![]() $\lambda $
and
$\lambda $
and
![]() $\bar {\lambda }$
are
$\bar {\lambda }$
are
![]() $\mathbb {P}$
-reflecting we then have
$\mathbb {P}$
-reflecting we then have
![]() $V_{\bar {\lambda }}[G_{\bar {\lambda }}]=V[G]_{\bar {\lambda }}$
and
$V_{\bar {\lambda }}[G_{\bar {\lambda }}]=V[G]_{\bar {\lambda }}$
and
![]() $V_{\lambda }[G_{\lambda }]=V[G]_{\lambda }$
.
$V_{\lambda }[G_{\lambda }]=V[G]_{\lambda }$
.
So let
![]() $\lambda $
be as above. Since
$\lambda $
be as above. Since
![]() $\delta $
is
$\delta $
is
![]() $\Sigma _1$
-supercompact, given
$\Sigma _1$
-supercompact, given
![]() $\mu \in C^{(1)}$
with
$\mu \in C^{(1)}$
with
![]() $\mu>\lambda $
, there is
$\mu>\lambda $
, there is
![]() $\bar {\mu }\in C^{(1)}$
and
$\bar {\mu }\in C^{(1)}$
and
![]() $\bar {\delta }<\bar {\lambda }<\bar {\mu }$
, and an elementary embedding
$\bar {\delta }<\bar {\lambda }<\bar {\mu }$
, and an elementary embedding
![]() $j:V_{\bar {\mu }}\rightarrow V_\mu $
with
$j:V_{\bar {\mu }}\rightarrow V_\mu $
with
![]() $\mathrm {crit}(j)=\bar {\delta }$
,
$\mathrm {crit}(j)=\bar {\delta }$
,
![]() $j(\bar {\delta })=\delta $
, and
$j(\bar {\delta })=\delta $
, and
![]() $j(\bar {\lambda })=\lambda $
.
$j(\bar {\lambda })=\lambda $
.
Since
![]() $K_{\mathbb {P}}$
is
$K_{\mathbb {P}}$
is
![]() $\Delta _2$
-definable,
$\Delta _2$
-definable,
![]() $V_\mu \models \lambda \in K_{\mathbb {P}}\cap C^{(1)}$
. Hence, by elementarity and
$V_\mu \models \lambda \in K_{\mathbb {P}}\cap C^{(1)}$
. Hence, by elementarity and
![]() $\Sigma _1$
-correctness of
$\Sigma _1$
-correctness of
![]() $\bar {\mu }$
,
$\bar {\mu }$
,
![]() $\bar {\lambda }\in K_{\mathbb {P}}\cap C^{(1)}$
.
$\bar {\lambda }\in K_{\mathbb {P}}\cap C^{(1)}$
.
We only need to show how to lift
![]() $j\restriction V_{\bar {\lambda }}:V_{\bar {\lambda }}\rightarrow V_\lambda $
to an elementary embedding
$j\restriction V_{\bar {\lambda }}:V_{\bar {\lambda }}\rightarrow V_\lambda $
to an elementary embedding
![]() $V_{\bar {\lambda }}[G_{\bar {\lambda }}]\rightarrow V_\lambda [G_\lambda ].$
Since
$V_{\bar {\lambda }}[G_{\bar {\lambda }}]\rightarrow V_\lambda [G_\lambda ].$
Since
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _2$
-definable,
$\Delta _2$
-definable,
![]() $\mathbb {P}^{V_\lambda }=\mathbb {P}\cap V_\lambda =\mathbb {P}_\lambda $
, where the rightmost equality follows from
$\mathbb {P}^{V_\lambda }=\mathbb {P}\cap V_\lambda =\mathbb {P}_\lambda $
, where the rightmost equality follows from
![]() $\lambda \in K_{\mathbb {P}}$
. Analogously, the same holds for
$\lambda \in K_{\mathbb {P}}$
. Analogously, the same holds for
![]() $\mathbb {P}^{V_{\bar {\lambda }}}$
. Thus, we have:
$\mathbb {P}^{V_{\bar {\lambda }}}$
. Thus, we have:
-
(i)
 $\mathbb {P}_{\bar {\lambda }}\cong \mathbb {P}_{\bar {\delta }}\ast \dot {\mathbb {Q}}$
, with
$\mathbb {P}_{\bar {\lambda }}\cong \mathbb {P}_{\bar {\delta }}\ast \dot {\mathbb {Q}}$
, with
 $|\dot {\mathbb {Q}}|=\bar {\lambda }$
.
$|\dot {\mathbb {Q}}|=\bar {\lambda }$
. -
(ii)
 $\mathbb {P}_\lambda \cong \mathbb {P}_{\delta }\ast \dot {\mathbb {Q}}^*$
and
$\mathbb {P}_\lambda \cong \mathbb {P}_{\delta }\ast \dot {\mathbb {Q}}^*$
and
 $\Vdash _{\mathbb {P}_{\delta }}\text {"}\dot {\mathbb {Q}}^* \text { is weakly homogeneous."}$
$\Vdash _{\mathbb {P}_{\delta }}\text {"}\dot {\mathbb {Q}}^* \text { is weakly homogeneous."}$
Since
![]() $\delta \in C(\mathbb {P})$
,
$\delta \in C(\mathbb {P})$
,
![]() $\Vdash _{\mathbb {P}_\delta }\text {"}\dot {\mathbb {Q}}^* \text { is } \delta \text {-directed closed."}$
We may now proceed as in the proof of Theorem 5.4 to get the desired elementary embedding
$\Vdash _{\mathbb {P}_\delta }\text {"}\dot {\mathbb {Q}}^* \text { is } \delta \text {-directed closed."}$
We may now proceed as in the proof of Theorem 5.4 to get the desired elementary embedding
![]() $j:V_{\bar {\lambda }}[G_{\bar {\lambda }}]\rightarrow V_\lambda [G_\lambda ].$
$j:V_{\bar {\lambda }}[G_{\bar {\lambda }}]\rightarrow V_\lambda [G_\lambda ].$
Lemma 7.4. Suppose that
![]() $\mathbb {P}$
is a fitting iteration and
$\mathbb {P}$
is a fitting iteration and
![]() $\delta $
is an extendible cardinal. Then,
$\delta $
is an extendible cardinal. Then,
![]() $\Vdash _{\mathbb {P}}\text {"}\delta \text { is extendible."}$
$\Vdash _{\mathbb {P}}\text {"}\delta \text { is extendible."}$
Proof Suppose G is a
![]() $\mathbb {P}$
-generic filter over
$\mathbb {P}$
-generic filter over
![]() $\mathrm {V}$
. It suffices to verify that for each
$\mathrm {V}$
. It suffices to verify that for each
![]() $\lambda \in K_{\mathbb {P}}$
greater than
$\lambda \in K_{\mathbb {P}}$
greater than
![]() $\delta $
, there is
$\delta $
, there is
![]() $\theta \in K_{\mathbb {P}}$
and an elementary embedding
$\theta \in K_{\mathbb {P}}$
and an elementary embedding
with
![]() $\mathrm {crit}(j)=\delta $
and
$\mathrm {crit}(j)=\delta $
and
![]() $j(\delta )>\lambda $
. For since
$j(\delta )>\lambda $
. For since
![]() $\lambda $
and
$\lambda $
and
![]() $\theta $
are
$\theta $
are
![]() $\mathbb {P}$
-reflecting we then have
$\mathbb {P}$
-reflecting we then have
![]() $V_{\lambda }[G_{\lambda }]=V[G]_{\lambda }$
and
$V_{\lambda }[G_{\lambda }]=V[G]_{\lambda }$
and
![]() $V_{\theta }[G_{\theta }]=V[G]_{\theta }$
.
$V_{\theta }[G_{\theta }]=V[G]_{\theta }$
.
So let
![]() $\lambda $
be as above. Since
$\lambda $
be as above. Since
![]() $\delta $
is extendible, hence
$\delta $
is extendible, hence
![]() $C^{(1)+}$
-extendible, given
$C^{(1)+}$
-extendible, given
![]() $\mu \in C^{(1)}$
,
$\mu \in C^{(1)}$
,
![]() $\mu>\lambda $
, there is
$\mu>\lambda $
, there is
![]() $\eta \in C^{(1)}$
and an elementary embedding
$\eta \in C^{(1)}$
and an elementary embedding
![]() $j:V_\mu \rightarrow V_\eta $
with
$j:V_\mu \rightarrow V_\eta $
with
![]() $\mathrm {crit}(j)=\delta $
and
$\mathrm {crit}(j)=\delta $
and
![]() $j(\delta )>\mu $
. Since
$j(\delta )>\mu $
. Since
![]() $K_{\mathbb {P}}$
is
$K_{\mathbb {P}}$
is
![]() $\Delta _2$
-definable,
$\Delta _2$
-definable,
![]() $V_\mu \models \lambda \in K_{\mathbb {P}}$
, hence by elementarity and since
$V_\mu \models \lambda \in K_{\mathbb {P}}$
, hence by elementarity and since
![]() $\eta \in C^{(1)}$
,
$\eta \in C^{(1)}$
,
![]() $j(\lambda )\in K_{\mathbb {P}}$
.
$j(\lambda )\in K_{\mathbb {P}}$
.
Let
![]() $\theta :=j(\lambda )$
. We will show how to lift
$\theta :=j(\lambda )$
. We will show how to lift
![]() $j\restriction V_\lambda :V_\lambda \rightarrow V_\theta $
to an elementary embedding
$j\restriction V_\lambda :V_\lambda \rightarrow V_\theta $
to an elementary embedding
![]() $V_\lambda [G_\lambda ]\rightarrow V_\theta [G_\theta ]$
. Since
$V_\lambda [G_\lambda ]\rightarrow V_\theta [G_\theta ]$
. Since
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _2$
-definable,
$\Delta _2$
-definable,
![]() $\mathbb {P}^{V_\lambda }=\mathbb {P}\cap V_\lambda =\mathbb {P}_\lambda $
, where the rightmost equality follows from
$\mathbb {P}^{V_\lambda }=\mathbb {P}\cap V_\lambda =\mathbb {P}_\lambda $
, where the rightmost equality follows from
![]() $\lambda \in K_{\mathbb {P}}$
. Analogously, the same holds for
$\lambda \in K_{\mathbb {P}}$
. Analogously, the same holds for
![]() $\mathbb {P}^{V_\theta }$
. Thus, we have:
$\mathbb {P}^{V_\theta }$
. Thus, we have:
-
(i)
 $\mathbb {P}_\lambda \cong \mathbb {P}_\delta \ast \dot {\mathbb {Q}}$
, with
$\mathbb {P}_\lambda \cong \mathbb {P}_\delta \ast \dot {\mathbb {Q}}$
, with
 $|\dot {\mathbb {Q}}|=\lambda $
.
$|\dot {\mathbb {Q}}|=\lambda $
. -
(ii)
 $\mathbb {P}_\theta \cong \mathbb {P}_{i(\delta )}\ast \dot {\mathbb {Q}}^*$
and
$\mathbb {P}_\theta \cong \mathbb {P}_{i(\delta )}\ast \dot {\mathbb {Q}}^*$
and
 $\Vdash _{\mathbb {P}_{i(\delta )}}\text {"}\dot {\mathbb {Q}}^* \text { is weakly homogeneous."}$
$\Vdash _{\mathbb {P}_{i(\delta )}}\text {"}\dot {\mathbb {Q}}^* \text { is weakly homogeneous."}$
Claim 7.4.1.
![]() $\Vdash _{\mathbb {P}_{i(\delta )}}\text {"}\dot {\mathbb {Q}}^* \text { is } i(\delta )\text {-directed closed."}$
$\Vdash _{\mathbb {P}_{i(\delta )}}\text {"}\dot {\mathbb {Q}}^* \text { is } i(\delta )\text {-directed closed."}$
Proof of claim We first show that
![]() $\delta $
is an accumulation point of
$\delta $
is an accumulation point of
![]() $C(\mathbb {P})$
, hence it belongs to
$C(\mathbb {P})$
, hence it belongs to
![]() $C(\mathbb {P})$
. So, fix an ordinal
$C(\mathbb {P})$
. So, fix an ordinal
![]() $\sigma _0<\delta $
. Since
$\sigma _0<\delta $
. Since
![]() $\mathbb {P}$
is suitable the
$\mathbb {P}$
is suitable the
![]() $\Sigma _3$
-formula
$\Sigma _3$
-formula
![]() $\varphi (\sigma _0)$
$\varphi (\sigma _0)$
holds. Since
![]() $\delta \in C^{(3)}$
and
$\delta \in C^{(3)}$
and
![]() $\sigma _0<\delta $
, there is a witness
$\sigma _0<\delta $
, there is a witness
![]() $\sigma _0<\sigma _1<\delta $
for
$\sigma _0<\sigma _1<\delta $
for
![]() $\varphi (\sigma _0)$
. Arguing inductively we define a sequence
$\varphi (\sigma _0)$
. Arguing inductively we define a sequence
![]() $\langle \sigma _n\mid n<\omega \rangle $
of ordinals
$\langle \sigma _n\mid n<\omega \rangle $
of ordinals
![]() ${<}\delta $
such that
${<}\delta $
such that
![]() $\sigma _{n+1}$
is a witness for
$\sigma _{n+1}$
is a witness for
![]() $\varphi (\sigma _n)$
. Setting
$\varphi (\sigma _n)$
. Setting
![]() $\sigma _\omega :=\sup _{n<\omega }\sigma _n$
one can easily see that
$\sigma _\omega :=\sup _{n<\omega }\sigma _n$
one can easily see that
![]() $\sigma _\omega \in C(\mathbb {P})\cap \delta $
.
$\sigma _\omega \in C(\mathbb {P})\cap \delta $
.
Since
![]() $\delta \in C(\mathbb {P})$
, for each
$\delta \in C(\mathbb {P})$
, for each
![]() $\sigma \in [\delta ,\lambda )$
,
$\sigma \in [\delta ,\lambda )$
,
![]() $\Vdash _{\mathbb {P}_\delta }\text {"}\dot {\mathbb {P}}_{[\delta ,\sigma ]} \text { is }\delta \text {-directed closed."}$
Footnote
8
Since
$\Vdash _{\mathbb {P}_\delta }\text {"}\dot {\mathbb {P}}_{[\delta ,\sigma ]} \text { is }\delta \text {-directed closed."}$
Footnote
8
Since
![]() $\lambda $
is inaccessible,
$\lambda $
is inaccessible,
![]() $^{<\delta }{V_\lambda }\subseteq V_\lambda $
, and therefore the same is true in
$^{<\delta }{V_\lambda }\subseteq V_\lambda $
, and therefore the same is true in
![]() $V_\lambda $
, namely
$V_\lambda $
, namely
![]() $V_\lambda \models \text {"}\Vdash _{\mathbb {P}_\delta }\,\dot {\mathbb {Q}} \text { is } \delta \text {-directed closed."}$
By elementarity,
$V_\lambda \models \text {"}\Vdash _{\mathbb {P}_\delta }\,\dot {\mathbb {Q}} \text { is } \delta \text {-directed closed."}$
By elementarity,
Since
![]() $\theta $
is inaccessible,
$\theta $
is inaccessible,
![]() $^{<i(\delta )}{V_\theta }\subseteq V_\theta $
, and therefore the quoted sentence displayed above also holds in V, as desired.
$^{<i(\delta )}{V_\theta }\subseteq V_\theta $
, and therefore the quoted sentence displayed above also holds in V, as desired.
From this point on the argument for the lifting of
![]() $j\restriction V_\lambda $
to an elementary embedding
$j\restriction V_\lambda $
to an elementary embedding
![]() $V_\lambda [G_\lambda ]\rightarrow V_\theta [G_\theta ]$
is essentially the same as in Theorem 5.4.
$V_\lambda [G_\lambda ]\rightarrow V_\theta [G_\theta ]$
is essentially the same as in Theorem 5.4.
Theorem 7.5. Suppose that
![]() $\mathbb {P}$
is a fitting iteration such that
$\mathbb {P}$
is a fitting iteration such that
![]() $C(\mathbb {P})$
contains all supercompact cardinals, in case there are any. Then forcing with
$C(\mathbb {P})$
contains all supercompact cardinals, in case there are any. Then forcing with
![]() $\mathbb {P}$
preserves
$\mathbb {P}$
preserves
![]() $C^{(n)}$
-extendible cardinals, for all
$C^{(n)}$
-extendible cardinals, for all
![]() $n<\omega $
.Footnote
9
$n<\omega $
.Footnote
9
Proof We prove the theorem by induction over n. The case
![]() $n=1$
is covered by Lemma 7.4. So, suppose that
$n=1$
is covered by Lemma 7.4. So, suppose that
![]() $n\geq 2$
and for each
$n\geq 2$
and for each
![]() $0\leq k< n$
forcing with
$0\leq k< n$
forcing with
![]() $\mathbb {P}$
preserves
$\mathbb {P}$
preserves
![]() $C^{(k)}$
-extendible cardinals.
$C^{(k)}$
-extendible cardinals.
We argue similarly as in the proof of Lemma 7.4. So, let
![]() $\delta $
be a
$\delta $
be a
![]() $C^{(n)}$
-extendible cardinal and suppose G is a
$C^{(n)}$
-extendible cardinal and suppose G is a
![]() $\mathbb {P}$
-generic filter over
$\mathbb {P}$
-generic filter over
![]() $\mathrm {V}$
. Let
$\mathrm {V}$
. Let
![]() $\lambda \in K^{(1)}_{\mathbb {P}}$
be greater than
$\lambda \in K^{(1)}_{\mathbb {P}}$
be greater than
![]() $\delta $
, and let
$\delta $
, and let
![]() $\mu \in C^{(n)}$
be greater than
$\mu \in C^{(n)}$
be greater than
![]() $\lambda $
. Then, there is
$\lambda $
. Then, there is
![]() $\eta \in C^{(n)}$
and an elementary embedding
$\eta \in C^{(n)}$
and an elementary embedding
with
![]() $\mathrm {crit}(j)=\delta $
,
$\mathrm {crit}(j)=\delta $
,
![]() $j(\delta )>\mu $
, and
$j(\delta )>\mu $
, and
![]() $j(\delta )\in C^{(n)}$
. Actually,
$j(\delta )\in C^{(n)}$
. Actually,
![]() $j(\delta )$
is
$j(\delta )$
is
![]() $C^{(n-2)}$
-extendible. Since
$C^{(n-2)}$
-extendible. Since
![]() $n\geq 2$
and
$n\geq 2$
and
![]() $K_{\mathbb {P}}$
is
$K_{\mathbb {P}}$
is
![]() $\Delta _{2}$
-definable,
$\Delta _{2}$
-definable,
![]() $V_\mu \models \lambda \in K_{\mathbb {P}}$
. Hence, by elementarity and since
$V_\mu \models \lambda \in K_{\mathbb {P}}$
. Hence, by elementarity and since
![]() $\eta \in C^{(n)}$
,
$\eta \in C^{(n)}$
,
![]() $j(\lambda )\in K_{\mathbb {P}}$
.
$j(\lambda )\in K_{\mathbb {P}}$
.
Arguing exactly as in the proof of Theorem 7.4, we can lift
![]() $j\upharpoonright V_\lambda $
to an elementary embedding
$j\upharpoonright V_\lambda $
to an elementary embedding
![]() $V[G]_\lambda \rightarrow V[G]_\theta $
, where
$V[G]_\lambda \rightarrow V[G]_\theta $
, where
![]() $\theta :=j(\lambda )$
.
$\theta :=j(\lambda )$
.
It only remains to show that
![]() $j(\delta )\in C^{(n)}$
. Suppose first
$j(\delta )\in C^{(n)}$
. Suppose first
![]() $n=2$
. Since
$n=2$
. Since
![]() $j(\delta )$
is supercompact and every supercompact belongs to
$j(\delta )$
is supercompact and every supercompact belongs to
![]() $C^{(2)}$
, it is enough to show that
$C^{(2)}$
, it is enough to show that
![]() $j(\delta )$
is supercompact in
$j(\delta )$
is supercompact in
![]() $V[G]$
. By our assumption,
$V[G]$
. By our assumption,
![]() $j(\delta )\in C(\mathbb {P})$
, hence
$j(\delta )\in C(\mathbb {P})$
, hence
![]() $j(\delta )$
is supercompact in
$j(\delta )$
is supercompact in
![]() $V[G]$
(cf. Theorem 7.3).
$V[G]$
(cf. Theorem 7.3).
For
![]() $1\leq n-2$
and
$1\leq n-2$
and
![]() $j(\delta )$
being
$j(\delta )$
being
![]() $C^{(n-2)}$
-extendible, our induction hypothesis implies that
$C^{(n-2)}$
-extendible, our induction hypothesis implies that
![]() $i(\delta )$
is
$i(\delta )$
is
![]() $C^{(n-2)}$
-extendible in
$C^{(n-2)}$
-extendible in
![]() $V[G]$
. In particular,
$V[G]$
. In particular,
![]() $V[G]_{j(\delta )}\prec _{\Sigma _n} V[G]$
. Thus,
$V[G]_{j(\delta )}\prec _{\Sigma _n} V[G]$
. Thus,
![]() $\delta $
is
$\delta $
is
![]() $C^{(n)}$
-extendible in
$C^{(n)}$
-extendible in
![]() $V[G]$
.
$V[G]$
.
In the next section we provide several applications of this theorem.
8 Some applications
8.1 Forcing the GCH and related combinatorial principles
Let
![]() $\mathbb {P}=\langle \mathbb {P}_\alpha; \dot {\mathbb {Q}}_\alpha :\alpha \in \mathrm {ORD}\rangle $
be the standard Jensen’s proper class iteration for forcing the global GCH. Namely,
$\mathbb {P}=\langle \mathbb {P}_\alpha; \dot {\mathbb {Q}}_\alpha :\alpha \in \mathrm {ORD}\rangle $
be the standard Jensen’s proper class iteration for forcing the global GCH. Namely,
![]() $\mathbb {P}$
is the direct limit of the iteration with Easton support where
$\mathbb {P}$
is the direct limit of the iteration with Easton support where
![]() $\mathbb {P}_0$
is the trivial forcing and for each ordinal
$\mathbb {P}_0$
is the trivial forcing and for each ordinal
![]() $\alpha $
, if
$\alpha $
, if
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is an uncountable cardinal,"}$
then
$\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is an uncountable cardinal,"}$
then
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_{\alpha } = \mathrm {Add},\text {"}$
and
$\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_{\alpha } = \mathrm {Add},\text {"}$
and
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha \text { is trivial"}$
otherwise.
$\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha \text { is trivial"}$
otherwise.
Lemma 8.1. Assume
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
![]() $2$
-Mahlo. Then
$2$
-Mahlo. Then
![]() $\mathbb {P}$
is fitting and
$\mathbb {P}$
is fitting and
![]() $C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
$C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
Proof Clearly,
![]() $\mathbb {P}$
is a suitable and weakly homogeneous iteration. Also,
$\mathbb {P}$
is a suitable and weakly homogeneous iteration. Also,
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _2$
-definable, as “
$\Delta _2$
-definable, as “
![]() $p\in \mathbb {P}$
” if and only if
$p\in \mathbb {P}$
” if and only if
![]() $V_\alpha \models \text {"}p\in \mathbb {P},\text {"}$
for (some) every
$V_\alpha \models \text {"}p\in \mathbb {P},\text {"}$
for (some) every
![]() $\alpha \in C^{(1)}$
such that
$\alpha \in C^{(1)}$
such that
![]() $p\in V_\alpha $
.
$p\in V_\alpha $
.
Let
![]() $K_{\mathbb {P}}$
denote the class of all Mahlo cardinals. Clearly,
$K_{\mathbb {P}}$
denote the class of all Mahlo cardinals. Clearly,
![]() $K_{\mathbb {P}}$
is
$K_{\mathbb {P}}$
is
![]() $\Delta _2$
-definable. Also, since
$\Delta _2$
-definable. Also, since
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Sigma }_2$
-
$\mathbf {\Sigma }_2$
-
![]() $2$
-Mahlo,
$2$
-Mahlo,
![]() $K_{\mathbb {P}}$
is a
$K_{\mathbb {P}}$
is a
![]() $\mathbf {\Sigma _2}$
-stationary proper class. Let
$\mathbf {\Sigma _2}$
-stationary proper class. Let
![]() $\kappa \in K_{\mathbb {P}}$
. Thus,
$\kappa \in K_{\mathbb {P}}$
. Thus,
![]() $\kappa $
is regular and, clearly,
$\kappa $
is regular and, clearly,
![]() $\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
.
$\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
.
Claim 8.1.1.
![]() $\kappa $
is
$\kappa $
is
![]() $\mathbb {P}$
-reflecting.
$\mathbb {P}$
-reflecting.
Proof of claim Clearly,
![]() $\kappa $
is inaccessible and
$\kappa $
is inaccessible and
![]() $\Vdash _{\mathbb {P}_\kappa } \text {"}\dot {\mathbb {Q}} \text { is } \kappa \text {-distributive,"}$
where
$\Vdash _{\mathbb {P}_\kappa } \text {"}\dot {\mathbb {Q}} \text { is } \kappa \text {-distributive,"}$
where
![]() $\mathbb {P}\cong \mathbb {P}_\kappa \ast \dot {\mathbb {Q}}$
. Also,
$\mathbb {P}\cong \mathbb {P}_\kappa \ast \dot {\mathbb {Q}}$
. Also,
![]() $\mathbb {P}_\kappa \subseteq V_\kappa $
. Let
$\mathbb {P}_\kappa \subseteq V_\kappa $
. Let
![]() $\lambda <\kappa $
be forced by
$\lambda <\kappa $
be forced by
![]() $\mathbb {P}_\kappa $
to be a cardinal. For every inaccessible cardinal
$\mathbb {P}_\kappa $
to be a cardinal. For every inaccessible cardinal
![]() $\theta <\kappa $
,
$\theta <\kappa $
,
![]() $\theta \in C(\mathbb {P})$
, hence
$\theta \in C(\mathbb {P})$
, hence
and
![]() $\mathbb {P}_\theta =\mathbb {P}\cap V_\theta $
. Since
$\mathbb {P}_\theta =\mathbb {P}\cap V_\theta $
. Since
![]() $\kappa $
is Mahlo and
$\kappa $
is Mahlo and
![]() $\{\theta <\kappa \mid \mathbb {P}_\theta =\mathbb {P}\cap V_\theta \}$
is unbounded,
$\{\theta <\kappa \mid \mathbb {P}_\theta =\mathbb {P}\cap V_\theta \}$
is unbounded,
![]() $\mathbb {P}_\kappa $
is
$\mathbb {P}_\kappa $
is
![]() $\kappa $
-cc. Also, standard arguments show that
$\kappa $
-cc. Also, standard arguments show that
![]() $\mathbb {P}_\kappa $
forces “
$\mathbb {P}_\kappa $
forces “
![]() $|\dot {\mathcal {P}}(\lambda )|<\check {\kappa }$
.” Altogether,
$|\dot {\mathcal {P}}(\lambda )|<\check {\kappa }$
.” Altogether,
![]() $\mathbb {P}$
forces
$\mathbb {P}$
forces
![]() $\kappa $
to be inaccessible. Finally, we appeal to Proposition 3.4 to get that
$\kappa $
to be inaccessible. Finally, we appeal to Proposition 3.4 to get that
![]() $\kappa $
is
$\kappa $
is
![]() $\mathbb {P}$
-reflecting, as wanted.
$\mathbb {P}$
-reflecting, as wanted.
The claim about
![]() $C(\mathbb {P})$
containing all supercompacts is obvious.
$C(\mathbb {P})$
containing all supercompacts is obvious.
In [Reference Tsaprounis28, Section 5], Tsaprounis shows that
![]() $\mathbb {P}$
preserves
$\mathbb {P}$
preserves
![]() $C^{(n)}$
-extendible cardinals. The following theorem gives an improvement of his result:
$C^{(n)}$
-extendible cardinals. The following theorem gives an improvement of his result:
Theorem 8.2.
-
(1) If
 $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
 $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
 $2$
-Mahlo, and
$2$
-Mahlo, and
 $\delta $
is supercompact then
$\delta $
is supercompact then  $$ \begin{align*} \Vdash _{\mathbb{P}}\text{"}\delta \text{ is supercompact."} \end{align*} $$
$$ \begin{align*} \Vdash _{\mathbb{P}}\text{"}\delta \text{ is supercompact."} \end{align*} $$
-
(2) If for some
 $n\geq 1$
,
$n\geq 1$
,
 $\delta $
is
$\delta $
is
 $C^{(n)}$
-extendible then
$C^{(n)}$
-extendible then  $$ \begin{align*} \Vdash _{\mathbb{P}}\text{"}\delta \text{ is } C^{(n)}\text{-extendible."} \end{align*} $$
$$ \begin{align*} \Vdash _{\mathbb{P}}\text{"}\delta \text{ is } C^{(n)}\text{-extendible."} \end{align*} $$
InFootnote
10
particular, if there is a proper class of supercompact cardinals
![]() $($
equivalently, if
$($
equivalently, if
![]() $\mathrm {VP}(\mathbf {\Pi _1})$
holds), then forcing with
$\mathrm {VP}(\mathbf {\Pi _1})$
holds), then forcing with
![]() $\mathbb {P}$
preserves
$\mathbb {P}$
preserves
![]() $C^{(n)}$
-extendible cardinals, for all
$C^{(n)}$
-extendible cardinals, for all
![]() $n<\omega $
.
$n<\omega $
.
Proof This follows from Theorem 7.5 and Lemma 8.1. The particular case can be proved using Lemma 6.3.
Recall that a class function E from the class
![]() $\mathrm {Reg}$
of infinite regular cardinals to the class of cardinals is an Easton function if it satisfies König’s theorem (i.e.,
$\mathrm {Reg}$
of infinite regular cardinals to the class of cardinals is an Easton function if it satisfies König’s theorem (i.e.,
![]() $\mathrm {cf}(E(\kappa ))>\kappa $
, for all
$\mathrm {cf}(E(\kappa ))>\kappa $
, for all
![]() $\kappa \in \mathrm {Reg}$
) and is increasingly monotone. Let
$\kappa \in \mathrm {Reg}$
) and is increasingly monotone. Let
![]() $\mathbb {P}_E$
be the direct limit of the iteration
$\mathbb {P}_E$
be the direct limit of the iteration
![]() $\langle \mathbb {P}_\alpha , \dot {\mathbb {Q}}_\alpha :\,\alpha \in \mathrm {ORD}\rangle $
with Easton support where
$\langle \mathbb {P}_\alpha , \dot {\mathbb {Q}}_\alpha :\,\alpha \in \mathrm {ORD}\rangle $
with Easton support where
![]() $\mathbb {P}_0$
is the trivial forcing and for each ordinal
$\mathbb {P}_0$
is the trivial forcing and for each ordinal
![]() $\alpha $
, if
$\alpha $
, if
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is a regular cardinal,"}$
then
$\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is a regular cardinal,"}$
then
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha =\mathrm {Add}(\alpha ,E(\alpha )),\text {"}$
and
$\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha =\mathrm {Add}(\alpha ,E(\alpha )),\text {"}$
and
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha \text { is trivial"}$
otherwise. Standard arguments [Reference Jech16, Section 15] show that if the GCH holds in the ground model, then
$\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha \text { is trivial"}$
otherwise. Standard arguments [Reference Jech16, Section 15] show that if the GCH holds in the ground model, then
![]() $\mathbb {P}_E$
preserves all cardinals and cofinalities and forces that
$\mathbb {P}_E$
preserves all cardinals and cofinalities and forces that
![]() $2^\kappa =E(\kappa )$
, for each
$2^\kappa =E(\kappa )$
, for each
![]() $\kappa \in \mathrm {Reg}$
.
$\kappa \in \mathrm {Reg}$
.
Lemma 8.3. Let E be a
![]() $\Delta _2$
-definable Easton function and assume that
$\Delta _2$
-definable Easton function and assume that
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
![]() $2$
-Mahlo. Then
$2$
-Mahlo. Then
![]() $\mathbb {P}_E$
is fitting and
$\mathbb {P}_E$
is fitting and
![]() $C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
$C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
Proof Arguing as in Lemma 8.1,
![]() $\mathbb {P}_E$
is a
$\mathbb {P}_E$
is a
![]() $\Delta _2$
-definable, weakly homogeneous suitable iteration. Set
$\Delta _2$
-definable, weakly homogeneous suitable iteration. Set
![]() $\mathbb {P}:=\mathbb {P}_E$
and let
$\mathbb {P}:=\mathbb {P}_E$
and let
Clearly, every
![]() $\kappa \in K_{\mathbb {P}}$
is regular and witnesses
$\kappa \in K_{\mathbb {P}}$
is regular and witnesses
![]() $\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
. The argument for the verification that each
$\mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
. The argument for the verification that each
![]() $\kappa \in K_{\mathbb {P}}$
is
$\kappa \in K_{\mathbb {P}}$
is
![]() $\mathbb {P}$
-reflecting is analogous to the one given in Lemma 8.1. The claim about
$\mathbb {P}$
-reflecting is analogous to the one given in Lemma 8.1. The claim about
![]() $C(\mathbb {P})$
is obvious. Also, since E is
$C(\mathbb {P})$
is obvious. Also, since E is
![]() $\Delta _2$
-definable, so is
$\Delta _2$
-definable, so is
![]() $K_{\mathbb {P}}$
. Moreover,
$K_{\mathbb {P}}$
. Moreover,
![]() $K_{\mathbb {P}}$
is
$K_{\mathbb {P}}$
is
![]() $\mathbf {\Sigma _2}$
-stationary, for if
$\mathbf {\Sigma _2}$
-stationary, for if
![]() $\mathcal {C}$
is a
$\mathcal {C}$
is a
![]() $\mathbf {\Sigma _2}$
-definable club class of ordinals, then
$\mathbf {\Sigma _2}$
-definable club class of ordinals, then
![]() $\mathcal {C}_E:=\{\alpha \in \mathcal {C}\mid E[\alpha ]\subseteq \alpha \}$
is a
$\mathcal {C}_E:=\{\alpha \in \mathcal {C}\mid E[\alpha ]\subseteq \alpha \}$
is a
![]() $\mathbf {\Sigma _2}$
-definable proper club class, and since
$\mathbf {\Sigma _2}$
-definable proper club class, and since
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
![]() $2$
-Mahlo,
$2$
-Mahlo,
![]() $K_{\mathbb {P}}\cap \mathcal {C}\neq \varnothing $
.
$K_{\mathbb {P}}\cap \mathcal {C}\neq \varnothing $
.
Similarly as in Theorem 8.2, we now obtain the following:
Theorem 8.4. Assume the
![]() $\mathrm {GCH}$
holds and let E be a
$\mathrm {GCH}$
holds and let E be a
![]() $\Delta _2$
-definable Easton function.Footnote
11
Then the following hold
$\Delta _2$
-definable Easton function.Footnote
11
Then the following hold
![]() $:$
$:$
-
(1) If
 $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
 $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
 $2$
-Mahlo and
$2$
-Mahlo and
 $\delta $
is supercompact then
$\delta $
is supercompact then  $$ \begin{align*} \Vdash _{\mathbb{P}_E}\text{"}\delta \text{is supercompact."} \end{align*} $$
$$ \begin{align*} \Vdash _{\mathbb{P}_E}\text{"}\delta \text{is supercompact."} \end{align*} $$
-
(2) If
 $n\geq 1$
and
$n\geq 1$
and
 $\delta $
is
$\delta $
is
 $C^{(n)}$
-extendible then
$C^{(n)}$
-extendible then  $$ \begin{align*} \Vdash _{\mathbb{P}_E}\text{"}\delta \text{ is } C^{(n)}\text{-extendible."} \end{align*} $$
$$ \begin{align*} \Vdash _{\mathbb{P}_E}\text{"}\delta \text{ is } C^{(n)}\text{-extendible."} \end{align*} $$
In particular, if the
![]() $\mathrm {GCH}$
holds and there is a proper class of supercompact cardinals
$\mathrm {GCH}$
holds and there is a proper class of supercompact cardinals
![]() $($
equivalently,
$($
equivalently,
![]() $\mathrm {VP}(\mathbf {\Pi _1})$
holds), then forcing with
$\mathrm {VP}(\mathbf {\Pi _1})$
holds), then forcing with
![]() $\mathbb {P}_E$
preserves
$\mathbb {P}_E$
preserves
![]() $C^{(n)}$
-extendible cardinals, for all
$C^{(n)}$
-extendible cardinals, for all
![]() $n<\omega $
.
$n<\omega $
.
Corollary 8.5. Let E be a
![]() $\Delta _2$
-definable Easton function and assume the
$\Delta _2$
-definable Easton function and assume the
![]() $\mathrm {GCH}$
holds. Then the following are true
$\mathrm {GCH}$
holds. Then the following are true
![]() $:$
$:$
-
(1) If
 $\mathrm {VP}(\mathbf {\Pi _{1}})$
holds, then
$\mathrm {VP}(\mathbf {\Pi _{1}})$
holds, then
 $\Vdash _{\mathbb {P}_E}\text {"}\mathrm {VP}(\mathbf {\Pi _{1}}).\text {"}$
$\Vdash _{\mathbb {P}_E}\text {"}\mathrm {VP}(\mathbf {\Pi _{1}}).\text {"}$
-
(2) If
 $\mathrm {VP}({\Pi _{n+1}})$
holds, then
$\mathrm {VP}({\Pi _{n+1}})$
holds, then
 $\Vdash _{\mathbb {P}_E}\text {"}\mathrm {VP}({\Pi _{n+1}}).\text {"}$
Also, if
$\Vdash _{\mathbb {P}_E}\text {"}\mathrm {VP}({\Pi _{n+1}}).\text {"}$
Also, if
 $\mathrm {VP}(\mathbf {\Pi _{n+1}})$
holds then
$\mathrm {VP}(\mathbf {\Pi _{n+1}})$
holds then
 $\Vdash _{\mathbb {P}_E}\text {"}\mathrm {VP}(\mathbf {\Pi _{n+1}}).\text {"}$
$\Vdash _{\mathbb {P}_E}\text {"}\mathrm {VP}(\mathbf {\Pi _{n+1}}).\text {"}$
The next corollary shows that
![]() $\mathrm {VP}$
is also consistent with any possible behaviour of the power-set function given by an arbitrary definable Easton function.
$\mathrm {VP}$
is also consistent with any possible behaviour of the power-set function given by an arbitrary definable Easton function.
Corollary 8.6. If
![]() $\mathrm {VP}$
holds, then in some class forcing extension, for every definable Easton function E there is a further class forcing extension that preserves
$\mathrm {VP}$
holds, then in some class forcing extension, for every definable Easton function E there is a further class forcing extension that preserves
![]() $\mathrm {VP}$
and where
$\mathrm {VP}$
and where
![]() $2^\kappa =E(\kappa )$
, for every
$2^\kappa =E(\kappa )$
, for every
![]() $\kappa \in \mathrm {Reg}$
.
$\kappa \in \mathrm {Reg}$
.
Proof First force with the standard Jensen’s iteration for forcing the GCH and call the resulting generic extension V. By Corollary 6.7,
![]() $V\models \mathrm {VP}$
. Then, given a V-definable Easton function E, force over V with
$V\models \mathrm {VP}$
. Then, given a V-definable Easton function E, force over V with
![]() $\mathbb {P}_E$
. Once again by Corollary 6.7,
$\mathbb {P}_E$
. Once again by Corollary 6.7,
![]() $V^{\mathbb {P}_E}\models \mathrm {VP}$
. Finally, since
$V^{\mathbb {P}_E}\models \mathrm {VP}$
. Finally, since
![]() $V\models \mathrm {GCH}$
, it follows that
$V\models \mathrm {GCH}$
, it follows that
![]() $\mathbb {P}_E$
forces
$\mathbb {P}_E$
forces
![]() $2^\kappa =E(\kappa )$
, for every
$2^\kappa =E(\kappa )$
, for every
![]() $\kappa \in \mathrm {Reg}$
.
$\kappa \in \mathrm {Reg}$
.
8.2 A remark on Woodin’s HOD Conjecture
The HOD Dichotomy theorem of Woodin states that if there exists an extendible cardinal, then either
![]() $\mathrm {V}$
is close to HOD or is far from it. Specifically, if
$\mathrm {V}$
is close to HOD or is far from it. Specifically, if
![]() $\kappa $
is an extendible cardinal, then either (1): for every singular cardinal
$\kappa $
is an extendible cardinal, then either (1): for every singular cardinal
![]() $\lambda>\delta $
,
$\lambda>\delta $
,
![]() $\lambda $
is singular in HOD and
$\lambda $
is singular in HOD and
![]() $(\lambda ^+)^{\mathrm {HOD}}=\lambda ^+$
, or (2): every regular cardinal
$(\lambda ^+)^{\mathrm {HOD}}=\lambda ^+$
, or (2): every regular cardinal
![]() $\lambda>\kappa $
is
$\lambda>\kappa $
is
![]() $\omega $
-strongly measurable in HOD (see [Reference Woodin29]). Woodin’s HOD Hypothesis asserts that there is a proper class of regular cardinals that are not
$\omega $
-strongly measurable in HOD (see [Reference Woodin29]). Woodin’s HOD Hypothesis asserts that there is a proper class of regular cardinals that are not
![]() $\omega $
-strongly measurable in HOD, and therefore that the first option of the HOD Dichotomy is the true one. Woodin’s HOD Conjecture asserts that the HOD Hypothesis is provable in the theory ZFC + “There exists an extendible cardinal.” Our arguments may be used to show that if the HOD Conjecture holds, and therefore it is provable in ZFC + “There exists an extendible cardinal” that above the first extendible cardinal every singular cardinal
$\omega $
-strongly measurable in HOD, and therefore that the first option of the HOD Dichotomy is the true one. Woodin’s HOD Conjecture asserts that the HOD Hypothesis is provable in the theory ZFC + “There exists an extendible cardinal.” Our arguments may be used to show that if the HOD Conjecture holds, and therefore it is provable in ZFC + “There exists an extendible cardinal” that above the first extendible cardinal every singular cardinal
![]() $\lambda $
is singular in HOD and
$\lambda $
is singular in HOD and
![]() $(\lambda ^+)^{\mathrm {HOD}}=\lambda ^+$
, there may still be no agreement at all between V and HOD about successors of regular cardinals. Moreover, many singular cardinals in
$(\lambda ^+)^{\mathrm {HOD}}=\lambda ^+$
, there may still be no agreement at all between V and HOD about successors of regular cardinals. Moreover, many singular cardinals in
![]() $\mathrm {HOD}$
need not be cardinals in
$\mathrm {HOD}$
need not be cardinals in
![]() $\mathrm {V}$
. Let us give some examples.
$\mathrm {V}$
. Let us give some examples.
Let
![]() $\mathbb {P}$
be the direct limit of the iteration
$\mathbb {P}$
be the direct limit of the iteration
![]() $\langle \mathbb {P}_\alpha; \dot {\mathbb {Q}_\alpha }:\,\alpha \in \mathrm {ORD}\rangle $
with Easton support, where
$\langle \mathbb {P}_\alpha; \dot {\mathbb {Q}_\alpha }:\,\alpha \in \mathrm {ORD}\rangle $
with Easton support, where
![]() $\mathbb {P}_0$
is the trivial forcing and for each ordinal
$\mathbb {P}_0$
is the trivial forcing and for each ordinal
![]() $\alpha $
, if
$\alpha $
, if
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is regular"}$
then
$\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is regular"}$
then
![]() $\Vdash _{\mathbb {P}_{\alpha }}\text {"}\boldsymbol {\dot {\mathbb {Q}}}_{\alpha } = \dot {\mathrm {Coll}}(\alpha ,\alpha ^+),\text {"}$
and
$\Vdash _{\mathbb {P}_{\alpha }}\text {"}\boldsymbol {\dot {\mathbb {Q}}}_{\alpha } = \dot {\mathrm {Coll}}(\alpha ,\alpha ^+),\text {"}$
and
![]() $\Vdash _{\mathbb {P}_{\alpha }}\text {"}\dot {\mathbb {Q}_{\alpha }} \text { is trivial"}$
otherwise. Arguing essentially as in Lemma 8.1 we obtain the following:
$\Vdash _{\mathbb {P}_{\alpha }}\text {"}\dot {\mathbb {Q}_{\alpha }} \text { is trivial"}$
otherwise. Arguing essentially as in Lemma 8.1 we obtain the following:
Lemma 8.7. Assume
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Sigma }_2$
-
$\mathbf {\Sigma }_2$
-
![]() $2$
-Mahlo. Then,
$2$
-Mahlo. Then,
![]() $\mathbb {P}$
is fitting and
$\mathbb {P}$
is fitting and
![]() $C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
$C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
Theorem 8.8.
-
(1) If
 $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
 $\mathbf {\Sigma }_2$
-
$\mathbf {\Sigma }_2$
-
 $2$
-Mahlo, and
$2$
-Mahlo, and
 $\delta $
is supercompact then
$\delta $
is supercompact then  $$ \begin{align*}\Vdash _{\mathbb{P}} \text{"}\delta \text{ is supercompact."}\end{align*} $$
$$ \begin{align*}\Vdash _{\mathbb{P}} \text{"}\delta \text{ is supercompact."}\end{align*} $$
-
(2) If
 $n\geq 1$
and
$n\geq 1$
and
 $\delta $
is
$\delta $
is
 $C^{(n)}$
-extendible, then
$C^{(n)}$
-extendible, then  $$ \begin{align*} \Vdash_{\mathbb{P}} \text{"}\delta \text{ is } C^{(n)}\text{-extendible."} \end{align*} $$
$$ \begin{align*} \Vdash_{\mathbb{P}} \text{"}\delta \text{ is } C^{(n)}\text{-extendible."} \end{align*} $$
Moreover,
![]() $\mathbb {P}$
forces
$\mathbb {P}$
forces
![]() $"\forall \lambda \in \mathrm {Reg}\,((\lambda ^+)^{\mathrm {HOD}}<\lambda ^+)$
.”
$"\forall \lambda \in \mathrm {Reg}\,((\lambda ^+)^{\mathrm {HOD}}<\lambda ^+)$
.”
Proof For the preservation of
![]() $C^{(n)}$
-extendible cardinals we combine Lemma 8.7 and Theorem 7.5. To prove the claim about successors of regular cardinals, note that if
$C^{(n)}$
-extendible cardinals we combine Lemma 8.7 and Theorem 7.5. To prove the claim about successors of regular cardinals, note that if
![]() $\lambda $
is a regular cardinal in
$\lambda $
is a regular cardinal in
![]() $V^{\mathbb {P}}$
, then it was also a regular cardinal at stage
$V^{\mathbb {P}}$
, then it was also a regular cardinal at stage
![]() $\lambda $
of the iteration, hence its successor was collapsed at stage
$\lambda $
of the iteration, hence its successor was collapsed at stage
![]() $\lambda +1$
. Thus, on the one hand,
$\lambda +1$
. Thus, on the one hand,
![]() $(\lambda ^+)^V<(\lambda ^+)^{V^{\mathbb {P}}}.$
On the other hand,
$(\lambda ^+)^V<(\lambda ^+)^{V^{\mathbb {P}}}.$
On the other hand,
![]() $\mathbb {P}$
is weakly homogeneous and ordinal definable, hence
$\mathbb {P}$
is weakly homogeneous and ordinal definable, hence
![]() $\mathrm {HOD}^{{V}^{\mathbb {P}}}\subseteq HOD^V$
(see, e.g., [Reference Jech16] for details). Hence, in
$\mathrm {HOD}^{{V}^{\mathbb {P}}}\subseteq HOD^V$
(see, e.g., [Reference Jech16] for details). Hence, in
![]() $V^{\mathbb {P}}$
,
$V^{\mathbb {P}}$
,
![]() $(\lambda ^+)^{\mathrm {HOD}}<\lambda ^+$
, as wanted.
$(\lambda ^+)^{\mathrm {HOD}}<\lambda ^+$
, as wanted.
Corollary 8.9. Forcing with
![]() $\mathbb {P}$
preserves
$\mathbb {P}$
preserves
![]() $\mathrm {VP}$
and forces
$\mathrm {VP}$
and forces
![]() $(\lambda ^+)^{\mathrm {HOD}}<\lambda ^+$
for every regular cardinal
$(\lambda ^+)^{\mathrm {HOD}}<\lambda ^+$
for every regular cardinal
![]() $\lambda $
.
$\lambda $
.
Theorem 8.8 yields the parallel of the main theorem from [Reference Dobrinen and Friedman11], at the level of
![]() $C^{(n)}$
-extendible cardinals.
$C^{(n)}$
-extendible cardinals.
Suppose now that K is a function on the class of infinite cardinals such that
![]() $K(\lambda )> \lambda $
, and K is increasingly monotone, for every
$K(\lambda )> \lambda $
, and K is increasingly monotone, for every
![]() $\lambda $
. Let
$\lambda $
. Let
![]() $\mathbb {P}_K$
be the direct limit of an iteration
$\mathbb {P}_K$
be the direct limit of an iteration
![]() $\langle \mathbb {P}_\alpha; \dot {\mathbb {Q}}_\alpha :\,\alpha \in \mathrm {ORD}\rangle $
with Easton support, where
$\langle \mathbb {P}_\alpha; \dot {\mathbb {Q}}_\alpha :\,\alpha \in \mathrm {ORD}\rangle $
with Easton support, where
![]() $\mathbb {P}_0$
is the trivial forcing and for each ordinal
$\mathbb {P}_0$
is the trivial forcing and for each ordinal
![]() $\alpha $
, if
$\alpha $
, if
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is regular"}$
then
$\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is regular"}$
then
![]() $\Vdash _{\mathbb {P}_\alpha }{"}\dot {\mathbb {Q}}_\alpha =\dot {\mathrm {Coll}}(\alpha ,K(\alpha )),\text {"}$
and
$\Vdash _{\mathbb {P}_\alpha }{"}\dot {\mathbb {Q}}_\alpha =\dot {\mathrm {Coll}}(\alpha ,K(\alpha )),\text {"}$
and
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha \text { is trivial"}$
otherwise. Notice that for each
$\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha \text { is trivial"}$
otherwise. Notice that for each
![]() $\alpha $
such that
$\alpha $
such that
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is regular,"}$
the remaining part of the iteration after stage
$\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \text { is regular,"}$
the remaining part of the iteration after stage
![]() $\alpha $
is
$\alpha $
is
![]() $\alpha $
-closed, hence it preserves
$\alpha $
-closed, hence it preserves
![]() $\alpha $
. Also note that if K is
$\alpha $
. Also note that if K is
![]() $\Delta _m$
-definable (
$\Delta _m$
-definable (
![]() $m\geq 1$
), then
$m\geq 1$
), then
![]() $\mathbb {P}_K$
is also
$\mathbb {P}_K$
is also
![]() $\Delta _m$
-definable. Clearly,
$\Delta _m$
-definable. Clearly,
![]() $\mathbb {P}_K$
is suitable and weakly homogeneous.
$\mathbb {P}_K$
is suitable and weakly homogeneous.
Lemma 8.10. Assume
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Sigma }_2$
-
$\mathbf {\Sigma }_2$
-
![]() $2$
-Mahlo. Let K be a
$2$
-Mahlo. Let K be a
![]() $\Delta _2$
-definable class function as above. Then,
$\Delta _2$
-definable class function as above. Then,
![]() $\mathbb {P}_K$
is a fitting iteration and
$\mathbb {P}_K$
is a fitting iteration and
![]() $C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
$C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
Proof Let
![]() $K_{\mathbb {P}}:=\{\kappa \mid \kappa \text { is Mahlo and } K[\kappa ]\subseteq \kappa \}$
. The proof that
$K_{\mathbb {P}}:=\{\kappa \mid \kappa \text { is Mahlo and } K[\kappa ]\subseteq \kappa \}$
. The proof that
![]() $K_{\mathbb {P}}$
is a witness for the fittingness of
$K_{\mathbb {P}}$
is a witness for the fittingness of
![]() $\mathbb {P}$
is similar to those given in Lemmas 8.3 and 8.7. The claim about
$\mathbb {P}$
is similar to those given in Lemmas 8.3 and 8.7. The claim about
![]() $C(\mathbb {P})$
is obvious.
$C(\mathbb {P})$
is obvious.
Theorem 8.11. Let K be a
![]() $\Delta _2$
-definable class function as above.
$\Delta _2$
-definable class function as above.
-
(1) If
 $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
 $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
 $2$
-Mahlo, and
$2$
-Mahlo, and
 $\delta $
is supercompact then
$\delta $
is supercompact then  $$ \begin{align*} \Vdash _{\mathbb{P}_K} \text{"}\delta \text{ is supercompact."} \end{align*} $$
$$ \begin{align*} \Vdash _{\mathbb{P}_K} \text{"}\delta \text{ is supercompact."} \end{align*} $$
-
(2) If
 $\delta $
is
$\delta $
is
 $C^{(n)}$
-extendible, for some
$C^{(n)}$
-extendible, for some
 $n\geq 1$
, then
$n\geq 1$
, then  $$ \begin{align*} \Vdash _{\mathbb{P}_K} \text{"}\delta \text{ is } C^{(n)}\text{-extendible."} \end{align*} $$
$$ \begin{align*} \Vdash _{\mathbb{P}_K} \text{"}\delta \text{ is } C^{(n)}\text{-extendible."} \end{align*} $$
Moreover,
![]() $\mathbb {P}_K$
forces
$\mathbb {P}_K$
forces
for all infinite regular cardinals
![]() $\lambda $
.
$\lambda $
.
Proof The preservation of
![]() $C^{(n)}$
-extendible cardinals,
$C^{(n)}$
-extendible cardinals,
![]() $n<\omega $
, follows from Lemma 8.10 and Theorem 7.5.
$n<\omega $
, follows from Lemma 8.10 and Theorem 7.5.
If G is
![]() $\mathbb {P}_K$
-generic over
$\mathbb {P}_K$
-generic over
![]() $\mathrm {V}$
and
$\mathrm {V}$
and
![]() $\lambda $
is regular in
$\lambda $
is regular in
![]() $V[G]$
, then it is also regular at the
$V[G]$
, then it is also regular at the
![]() $\lambda $
-stage of the iteration. Hence,
$\lambda $
-stage of the iteration. Hence,
![]() $\mathbb {Q}_\lambda =\mathrm {Coll}(\lambda ,K(\lambda ))$
, and therefore
$\mathbb {Q}_\lambda =\mathrm {Coll}(\lambda ,K(\lambda ))$
, and therefore
![]() $K(\lambda )<\lambda ^+$
holds in
$K(\lambda )<\lambda ^+$
holds in
![]() $V[G]$
. The other inequality (i.e.,
$V[G]$
. The other inequality (i.e.,
![]() $(\lambda ^+)^{\mathrm {HOD}}\leq K(\lambda )$
) follows from the fact that
$(\lambda ^+)^{\mathrm {HOD}}\leq K(\lambda )$
) follows from the fact that
![]() $\mathbb {P}_K$
is weakly homogeneous and ordinal definable, and thus that
$\mathbb {P}_K$
is weakly homogeneous and ordinal definable, and thus that
![]() $\mathrm {HOD}^{V[G]}\subseteq \mathrm {HOD}^V$
.
$\mathrm {HOD}^{V[G]}\subseteq \mathrm {HOD}^V$
.
The theorem above implies that many kinds of disagreement between successors of regulars in
![]() $\mathrm {HOD}$
and in
$\mathrm {HOD}$
and in
![]() $\mathrm {V}$
may be forced while preserving
$\mathrm {V}$
may be forced while preserving
![]() $C^{(n)}$
-extendible cardinals. It also implies that one can destroy many singular cardinals in
$C^{(n)}$
-extendible cardinals. It also implies that one can destroy many singular cardinals in
![]() $\mathrm {HOD}$
while preserving
$\mathrm {HOD}$
while preserving
![]() $C^{(n)}$
-extendible cardinals. For example, let K be such that
$C^{(n)}$
-extendible cardinals. For example, let K be such that
![]() $K(\lambda )$
is the least singular cardinal in
$K(\lambda )$
is the least singular cardinal in
![]() $\mathrm {HOD}$
greater than
$\mathrm {HOD}$
greater than
![]() $\lambda $
, i.e.,
$\lambda $
, i.e.,
![]() $K(\lambda )=(\lambda ^{+\omega })^{\mathrm {HOD}}$
. It is easily seen that K, and therefore also
$K(\lambda )=(\lambda ^{+\omega })^{\mathrm {HOD}}$
. It is easily seen that K, and therefore also
![]() $\mathbb {P}_K$
as defined above, are
$\mathbb {P}_K$
as defined above, are
![]() $\Delta _2$
-definable. Then we have the following.
$\Delta _2$
-definable. Then we have the following.
Corollary 8.12. For each
![]() $n\geq 1$
,
$n\geq 1$
,
![]() $\mathbb {P}_K$
preserves
$\mathbb {P}_K$
preserves
![]() $C^{(n)}$
-extendible cardinals and forces
$C^{(n)}$
-extendible cardinals and forces
![]() $\text {"}\forall \lambda \in \mathrm {Reg}\,((\lambda ^{+\omega })^{\mathrm {HOD}}<\lambda ^+).\text {"}$
$\text {"}\forall \lambda \in \mathrm {Reg}\,((\lambda ^{+\omega })^{\mathrm {HOD}}<\lambda ^+).\text {"}$
8.3 On diamonds
Other combinatorial statements that we can force while preserving
![]() $C^{(n)}$
-extendible cardinals are the diamond principles
$C^{(n)}$
-extendible cardinals are the diamond principles
![]() $\diamondsuit _S$
. Namely, given a stationary set
$\diamondsuit _S$
. Namely, given a stationary set
![]() $S\subseteq \kappa $
, a sequence
$S\subseteq \kappa $
, a sequence
![]() $\langle A_\alpha :\,\alpha \in S\rangle $
is a
$\langle A_\alpha :\,\alpha \in S\rangle $
is a
![]() $\diamondsuit _S$
-sequence if
$\diamondsuit _S$
-sequence if
![]() $A_\alpha \subseteq \alpha $
and for every
$A_\alpha \subseteq \alpha $
and for every
![]() $A\subseteq \kappa $
the set
$A\subseteq \kappa $
the set
![]() $\{\alpha \in S:\, A\cap \alpha =A_\alpha \}$
is stationary. We say that
$\{\alpha \in S:\, A\cap \alpha =A_\alpha \}$
is stationary. We say that
![]() $\diamondsuit _S$
holds if there is a
$\diamondsuit _S$
holds if there is a
![]() $\diamondsuit _S$
-sequence.
$\diamondsuit _S$
-sequence.
It is well-known that
![]() $\mathrm {Add}(\kappa ^+,1)$
automatically forces
$\mathrm {Add}(\kappa ^+,1)$
automatically forces
![]() $\diamondsuit _{S}$
, for every stationary
$\diamondsuit _{S}$
, for every stationary
![]() $S\subseteq \kappa ^+$
in
$S\subseteq \kappa ^+$
in
![]() $V^{\mathrm {Add}(\kappa ^+,1)}$
. Thus, from Theorem 8.2, we obtain:
$V^{\mathrm {Add}(\kappa ^+,1)}$
. Thus, from Theorem 8.2, we obtain:
Corollary 8.13.
-
(1) If
 $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
 $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
 $2$
-Mahlo and
$2$
-Mahlo and
 $\delta $
is a supercompact cardinal, then there is a generic extension where
$\delta $
is a supercompact cardinal, then there is a generic extension where
 $\delta $
is still supercompact and
$\delta $
is still supercompact and
 $\diamondsuit _{S}$
holds for every cardinal
$\diamondsuit _{S}$
holds for every cardinal
 $\kappa $
and every stationary
$\kappa $
and every stationary
 $S\subseteq \kappa ^+$
.
$S\subseteq \kappa ^+$
. -
(2) If
 $n\geq 1$
and
$n\geq 1$
and
 $\delta $
is a
$\delta $
is a
 $C^{(n)}$
-extendible cardinal, there is a generic extension where
$C^{(n)}$
-extendible cardinal, there is a generic extension where
 $\delta $
is
$\delta $
is
 $C^{(n)}$
-extendible and
$C^{(n)}$
-extendible and
 $\diamondsuit _{S}$
holds for every cardinal
$\diamondsuit _{S}$
holds for every cardinal
 $\kappa $
and every stationary
$\kappa $
and every stationary
 $S\subseteq \kappa ^+$
.
$S\subseteq \kappa ^+$
.
Hence, if
![]() $\mathrm {VP}$
holds in
$\mathrm {VP}$
holds in
![]() $\mathrm {V}$
, there is a generic extension where
$\mathrm {V}$
, there is a generic extension where
![]() $\mathrm {VP}$
holds together with
$\mathrm {VP}$
holds together with
![]() $\diamondsuit _{S}$
, for every
$\diamondsuit _{S}$
, for every
![]() $\kappa $
and every stationary
$\kappa $
and every stationary
![]() $S\subseteq \kappa ^+$
.
$S\subseteq \kappa ^+$
.
Another relevant diamond principle is the so-called
![]() $\diamondsuit ^+_{\kappa ^+}$
-principle.
$\diamondsuit ^+_{\kappa ^+}$
-principle.
A sequence
![]() $\langle \mathcal {A}_\alpha :\,\alpha \in \kappa ^+\rangle $
is a
$\langle \mathcal {A}_\alpha :\,\alpha \in \kappa ^+\rangle $
is a
![]() $\diamondsuit ^+_{\kappa ^+}$
-sequence if
$\diamondsuit ^+_{\kappa ^+}$
-sequence if
![]() $\mathcal {A}_\alpha \in [\mathcal {P}(\alpha )]^{\leq \kappa }$
and for every
$\mathcal {A}_\alpha \in [\mathcal {P}(\alpha )]^{\leq \kappa }$
and for every
![]() $A\subseteq \kappa ^+$
there is a club
$A\subseteq \kappa ^+$
there is a club
![]() $C\subseteq \kappa ^+$
such that
$C\subseteq \kappa ^+$
such that
We say that
![]() $\diamondsuit ^+_{\kappa ^+}$
holds if there is a
$\diamondsuit ^+_{\kappa ^+}$
holds if there is a
![]() $\diamondsuit ^+_{\kappa ^+}$
-sequence.
$\diamondsuit ^+_{\kappa ^+}$
-sequence.
In [Reference Cummings, Foreman and Magidor10, Theorem 12.2] it is shown that, assuming
![]() $2^\kappa =\kappa ^+$
and
$2^\kappa =\kappa ^+$
and
![]() $2^{\kappa ^+}=\kappa ^{++}$
, there is a
$2^{\kappa ^+}=\kappa ^{++}$
, there is a
![]() $\kappa ^+$
-closed and
$\kappa ^+$
-closed and
![]() $\kappa ^{++}$
-cc forcing notion that forces
$\kappa ^{++}$
-cc forcing notion that forces
![]() $\diamondsuit ^+_{\kappa ^+}$
. The forcing is an iteration
$\diamondsuit ^+_{\kappa ^+}$
. The forcing is an iteration
![]() $\mathbb {D}^+_{\kappa ^{++}}=\langle \mathbb {P}_\alpha ,\dot {\mathbb {Q}}_\beta : \beta <\alpha \leq \kappa ^{++}\rangle $
with supports of size
$\mathbb {D}^+_{\kappa ^{++}}=\langle \mathbb {P}_\alpha ,\dot {\mathbb {Q}}_\beta : \beta <\alpha \leq \kappa ^{++}\rangle $
with supports of size
![]() $\leq \kappa $
, where
$\leq \kappa $
, where
![]() $\mathbb {P}_0$
is the natural forcing notion that introduces a sequence
$\mathbb {P}_0$
is the natural forcing notion that introduces a sequence
![]() $\vec {\mathcal {A}}$
of the right form whereas the rest of the iterates forces the club sets
$\vec {\mathcal {A}}$
of the right form whereas the rest of the iterates forces the club sets
![]() $C\subseteq \kappa ^+$
witnessing that
$C\subseteq \kappa ^+$
witnessing that
![]() $\vec {\mathcal {A}}$
is a
$\vec {\mathcal {A}}$
is a
![]() $\diamondsuit ^+_{\kappa ^+}$
-sequence.
$\diamondsuit ^+_{\kappa ^+}$
-sequence.
Arguing as in Lemma 8.1 one has the following:
Lemma 8.14. Assume the
![]() $\mathrm {GCH}$
holds and that
$\mathrm {GCH}$
holds and that
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
![]() $2$
-Mahlo. Let
$2$
-Mahlo. Let
![]() $\mathbb {D}$
be the standard Easton support iteration of the forcings
$\mathbb {D}$
be the standard Easton support iteration of the forcings
![]() $\mathbb {D}^{+}_{\kappa ^{++}}$
, for
$\mathbb {D}^{+}_{\kappa ^{++}}$
, for
![]() $\kappa $
a cardinal. Then
$\kappa $
a cardinal. Then
![]() $\mathbb {D}$
is fitting and
$\mathbb {D}$
is fitting and
![]() $C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
$C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
Theorem 8.15. Assume the
![]() $\mathrm {GCH}$
holds.
$\mathrm {GCH}$
holds.
-
(1) If
 $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
 $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
 $2$
-Mahlo and
$2$
-Mahlo and
 $\delta $
is a supercompact cardinal, then forcing with
$\delta $
is a supercompact cardinal, then forcing with
 $\mathbb {D}$
preserves the supercompactness of
$\mathbb {D}$
preserves the supercompactness of
 $\delta $
and yields a generic extension where
$\delta $
and yields a generic extension where
 $\diamondsuit ^+_{\kappa ^+}$
holds, for every cardinal
$\diamondsuit ^+_{\kappa ^+}$
holds, for every cardinal
 $\kappa $
.
$\kappa $
. -
(2) If
 $n\geq 1$
and
$n\geq 1$
and
 $\delta $
is a
$\delta $
is a
 $C^{(n)}$
-extendible cardinal, then forcing with
$C^{(n)}$
-extendible cardinal, then forcing with
 $\mathbb {D}$
preserves the
$\mathbb {D}$
preserves the
 $C^{(n)}$
-extendibility of
$C^{(n)}$
-extendibility of
 $\delta $
and yields a generic extension where
$\delta $
and yields a generic extension where
 $\diamondsuit ^+_{\kappa ^+}$
holds, for every cardinal
$\diamondsuit ^+_{\kappa ^+}$
holds, for every cardinal
 $\kappa $
.
$\kappa $
.
Hence, if
![]() $\mathrm {VP}$
and the
$\mathrm {VP}$
and the
![]() $\mathrm {GCH}$
hold in
$\mathrm {GCH}$
hold in
![]() $\mathrm {V}$
, then
$\mathrm {V}$
, then
![]() $\mathrm {VP}$
also holds in
$\mathrm {VP}$
also holds in
![]() $V^{\mathbb {D}}$
, together with
$V^{\mathbb {D}}$
, together with
![]() $\diamondsuit _{\kappa ^+}^+$
, for every cardinal
$\diamondsuit _{\kappa ^+}^+$
, for every cardinal
![]() $\kappa $
.
$\kappa $
.
The claim of the theorem above referring to
![]() $\mathrm {VP}$
was first proved by Brooke-Taylor in [Reference Brooke-Taylor8, Corollary 26]
$\mathrm {VP}$
was first proved by Brooke-Taylor in [Reference Brooke-Taylor8, Corollary 26]
8.4 On weak square sequences
A classical result due to Solovay is that Jensen’s square principle
![]() $\square _\lambda $
must fail for every cardinal
$\square _\lambda $
must fail for every cardinal
![]() $\lambda $
greater than or equal to the first strongly compact cardinal [Reference Solovay25]. This result was subsequently refined by Jensen, who proved that
$\lambda $
greater than or equal to the first strongly compact cardinal [Reference Solovay25]. This result was subsequently refined by Jensen, who proved that
![]() $\square _\lambda $
fails for any subcompact cardinal
$\square _\lambda $
fails for any subcompact cardinal
![]() $\lambda $
, a much weaker notion than supercompactness. Further sharper results are due to Brooke-Taylor and Friedman [Reference Brooke-Taylor and Friedman9] and to Bagaria and Magidor [Reference Bagaria and Magidor5]. In our context, namely with the existence of extendible cardinals, and therefore with the failure of
$\lambda $
, a much weaker notion than supercompactness. Further sharper results are due to Brooke-Taylor and Friedman [Reference Brooke-Taylor and Friedman9] and to Bagaria and Magidor [Reference Bagaria and Magidor5]. In our context, namely with the existence of extendible cardinals, and therefore with the failure of
![]() $\square _\lambda $
for a tail of
$\square _\lambda $
for a tail of
![]() $\lambda $
’s, we shall consider
$\lambda $
’s, we shall consider
![]() $\square _{\lambda ,\mu }$
-principles, a weak form of the square principle introduced by Schimmerling in [Reference Schimmerling24].
$\square _{\lambda ,\mu }$
-principles, a weak form of the square principle introduced by Schimmerling in [Reference Schimmerling24].
Following up on Solovay’s work, Shelah proved that if
![]() $\kappa $
is supercompact and
$\kappa $
is supercompact and
![]() $\mathrm {cof}(\lambda )<\kappa <\lambda $
, then
$\mathrm {cof}(\lambda )<\kappa <\lambda $
, then
![]() $\square _{\lambda ,\lambda }$
(also known as
$\square _{\lambda ,\lambda }$
(also known as
![]() $\square ^*_\lambda $
) fails [Reference Cummings, Foreman and Magidor10, Section 2]. Also, Burke and Kanamori showed that if
$\square ^*_\lambda $
) fails [Reference Cummings, Foreman and Magidor10, Section 2]. Also, Burke and Kanamori showed that if
![]() $\kappa $
is
$\kappa $
is
![]() $\lambda ^+$
-strongly compact, then
$\lambda ^+$
-strongly compact, then
![]() $\square _{\lambda ,<\mathrm {cof}(\lambda )}$
fails [Reference Cummings, Foreman and Magidor10].Footnote
12
The remaining cases, namely
$\square _{\lambda ,<\mathrm {cof}(\lambda )}$
fails [Reference Cummings, Foreman and Magidor10].Footnote
12
The remaining cases, namely
![]() $\square _{\lambda ,\mu }$
with
$\square _{\lambda ,\mu }$
with
![]() $\kappa \leq \mathrm {cof}(\lambda )\leq \mu \leq \lambda $
turned out to be consistent with the existence of a supercompact cardinal
$\kappa \leq \mathrm {cof}(\lambda )\leq \mu \leq \lambda $
turned out to be consistent with the existence of a supercompact cardinal
![]() $\kappa $
[Reference Cummings, Foreman and Magidor10, Section 9]. Specifically, in [Reference Cummings, Foreman and Magidor10, Theorem 9.2], the authors define, for each
$\kappa $
[Reference Cummings, Foreman and Magidor10, Section 9]. Specifically, in [Reference Cummings, Foreman and Magidor10, Theorem 9.2], the authors define, for each
![]() $\kappa \leq \mathrm {cof}(\lambda )<\lambda $
, a forcing notion
$\kappa \leq \mathrm {cof}(\lambda )<\lambda $
, a forcing notion
![]() $\mathbb {S}_\lambda $
which forces
$\mathbb {S}_\lambda $
which forces
![]() $\square _{\lambda , \mathrm {cof}(\lambda )}$
and is
$\square _{\lambda , \mathrm {cof}(\lambda )}$
and is
![]() $\mathrm {cof}(\lambda )$
-directed closed and
$\mathrm {cof}(\lambda )$
-directed closed and
![]() $\lambda $
-strategically closed. Thus, if
$\lambda $
-strategically closed. Thus, if
![]() $\kappa $
is Laver-indestructible, then forcing with
$\kappa $
is Laver-indestructible, then forcing with
![]() $\mathbb {S}_\lambda $
preserves the supercompactness of
$\mathbb {S}_\lambda $
preserves the supercompactness of
![]() $\kappa $
. In this section we shall extend this result to
$\kappa $
. In this section we shall extend this result to
![]() $C^{(n)}$
-extendible cardinals.
$C^{(n)}$
-extendible cardinals.
Let K be the class function with
![]() $\text {dom}\,(K)=\mathrm {Card}\setminus \aleph _1$
, such that
$\text {dom}\,(K)=\mathrm {Card}\setminus \aleph _1$
, such that
![]() $K(\lambda ):= \text {"The first singular cardinal of cofinality } \lambda ^+.\text {"}$
Observe that K is
$K(\lambda ):= \text {"The first singular cardinal of cofinality } \lambda ^+.\text {"}$
Observe that K is
![]() $\Delta _2$
-definable. Now let
$\Delta _2$
-definable. Now let
![]() $\mathbb {P}_K$
be denote the direct limit of
$\mathbb {P}_K$
be denote the direct limit of
![]() $\langle \mathbb {P}_\alpha ;\dot {\mathbb {Q}}_\alpha :\,\alpha \in \mathrm {ORD}\rangle $
, the iteration with Easton support where
$\langle \mathbb {P}_\alpha ;\dot {\mathbb {Q}}_\alpha :\,\alpha \in \mathrm {ORD}\rangle $
, the iteration with Easton support where
![]() $\mathbb {P}_0$
is the trivial forcing and for every
$\mathbb {P}_0$
is the trivial forcing and for every
![]() $\alpha $
, if
$\alpha $
, if
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \in \dot {\mathrm {Card}}\setminus \dot {\aleph }_1,\text {"}$
then
$\Vdash _{\mathbb {P}_\alpha }\text {"}\alpha \in \dot {\mathrm {Card}}\setminus \dot {\aleph }_1,\text {"}$
then
![]() $\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha =\dot {\mathbb {S}}_{K(\alpha )},\text {"}$
and
$\Vdash _{\mathbb {P}_\alpha }\text {"}\dot {\mathbb {Q}}_\alpha =\dot {\mathbb {S}}_{K(\alpha )},\text {"}$
and
![]() otherwise.
otherwise.
Remark 8.16. For every
![]() $\alpha $
, if
$\alpha $
, if
![]() $\Vdash _{\mathbb {P}_\alpha } \text {"}\alpha \in \dot {\mathrm {Card}}\setminus \dot {\aleph }_1,\text {"}$
then
$\Vdash _{\mathbb {P}_\alpha } \text {"}\alpha \in \dot {\mathrm {Card}}\setminus \dot {\aleph }_1,\text {"}$
then
![]() $\mathbb {P}_{[\alpha ,\mathrm {ORD})}$
is
$\mathbb {P}_{[\alpha ,\mathrm {ORD})}$
is
![]() $\alpha ^+$
-directed closed and
$\alpha ^+$
-directed closed and
![]() $K(\alpha )$
-strategically closed.
$K(\alpha )$
-strategically closed.
We now prove that
![]() $\mathbb {P}_K$
forces class many instances of
$\mathbb {P}_K$
forces class many instances of
![]() $\square _{K(\lambda ),\lambda ^+}$
.
$\square _{K(\lambda ),\lambda ^+}$
.
Lemma 8.17.
![]() $\Vdash _{\mathbb {P}}\text {"}\forall \lambda \geq \dot {\aleph }_1\,(\square _{K(\lambda ),\lambda ^+} \text { holds}).\text {"}$
$\Vdash _{\mathbb {P}}\text {"}\forall \lambda \geq \dot {\aleph }_1\,(\square _{K(\lambda ),\lambda ^+} \text { holds}).\text {"}$
Proof Suppose
![]() $\lambda $
is an uncountable cardinal in
$\lambda $
is an uncountable cardinal in
![]() $V^{\mathbb {P}}$
. Then, by the above remark,
$V^{\mathbb {P}}$
. Then, by the above remark,
![]() $\lambda $
is also an uncountable cardinal in
$\lambda $
is also an uncountable cardinal in
![]() $V^{\mathbb {P}_\lambda }$
, hence
$V^{\mathbb {P}_\lambda }$
, hence
![]() $\Vdash _{\mathbb {P}_\lambda }\text {"}\dot {\mathbb {Q}}_\lambda =\dot {\mathbb {S}}_{K(\lambda )}.\text {"}$
Thus,
$\Vdash _{\mathbb {P}_\lambda }\text {"}\dot {\mathbb {Q}}_\lambda =\dot {\mathbb {S}}_{K(\lambda )}.\text {"}$
Thus,
![]() $\square _{K(\lambda ),\lambda ^+}$
holds in
$\square _{K(\lambda ),\lambda ^+}$
holds in
![]() $V^{\mathbb {P}_{\lambda +1}}$
. Again, by the above remark,
$V^{\mathbb {P}_{\lambda +1}}$
. Again, by the above remark,
![]() $\mathbb {P}_{[\lambda +1,\mathrm {ORD})}$
is distributive enough to preserve
$\mathbb {P}_{[\lambda +1,\mathrm {ORD})}$
is distributive enough to preserve
![]() $\square _{K(\lambda ),\lambda ^+}$
.
$\square _{K(\lambda ),\lambda ^+}$
.
Arguing as in Lemma 8.3 we can show that
![]() $\mathbb {P}_K$
is a fitting iteration. Precisely, we have the following:
$\mathbb {P}_K$
is a fitting iteration. Precisely, we have the following:
Lemma 8.18. Assume
![]() $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
![]() $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
![]() $2$
-Mahlo. Then
$2$
-Mahlo. Then
![]() $\mathbb {P}_K$
is fitting and
$\mathbb {P}_K$
is fitting and
![]() $C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
$C(\mathbb {P})$
contains all supercompact cardinals, in case there are any.
Theorem 8.19.
-
(1) If
 $\mathrm {ORD}$
is
$\mathrm {ORD}$
is
 $\mathbf {\Sigma _2}$
-
$\mathbf {\Sigma _2}$
-
 $2$
-Mahlo, then
$2$
-Mahlo, then
 $\mathbb {P}_K$
preserves supercompact cardinals.
$\mathbb {P}_K$
preserves supercompact cardinals. -
(2) For each
 $n\geq 1$
, forcing with
$n\geq 1$
, forcing with
 $\mathbb {P}_K$
preserves
$\mathbb {P}_K$
preserves
 $C^{(n)}$
-extendible cardinals.
$C^{(n)}$
-extendible cardinals.
Moreover, in any generic extension by
![]() $\mathbb {P}_K$
the following holds
$\mathbb {P}_K$
the following holds
![]() $:$
for every uncountable cardinal
$:$
for every uncountable cardinal
![]() $\lambda $
, if
$\lambda $
, if
![]() $K(\lambda )$
is the first singular cardinal of cofinality
$K(\lambda )$
is the first singular cardinal of cofinality
![]() $\lambda ^+$
, then
$\lambda ^+$
, then
![]() $\square _{K(\lambda ),\lambda ^+}$
holds.
$\square _{K(\lambda ),\lambda ^+}$
holds.
Corollary 8.20. If
![]() $\mathrm {VP}$
holds, then there is a class forcing iteration that preserves
$\mathrm {VP}$
holds, then there is a class forcing iteration that preserves
![]() $\mathrm {VP}$
and forces
$\mathrm {VP}$
and forces
![]() $\square _{\lambda ,\mathrm {cof}(\lambda )}$
, for a proper class of singular cardinals
$\square _{\lambda ,\mathrm {cof}(\lambda )}$
, for a proper class of singular cardinals
![]() $\lambda $
.
$\lambda $
.
9 General class forcing iterations
In this section we follow up the discussion at the end of Section 6 about nonweakly homogeneous suitable iterations. One prominent example is the iteration
![]() $\mathbb {P}$
that forces
$\mathbb {P}$
that forces
![]() $\mathrm {V}=\mathrm {HOD}$
by coding the universe into the power-set function pattern. This iteration is suitable but not weakly homogenous. One may also want to consider class forcing iterations
$\mathrm {V}=\mathrm {HOD}$
by coding the universe into the power-set function pattern. This iteration is suitable but not weakly homogenous. One may also want to consider class forcing iterations
![]() $\mathbb {P}$
over some model M such that
$\mathbb {P}$
over some model M such that
![]() $\mathbb {P}$
is not definable in M. To deal with such general class forcing notions we shall work within the theory
$\mathbb {P}$
is not definable in M. To deal with such general class forcing notions we shall work within the theory
![]() $\mathrm {ZFC}_{\mathbb {P}}$
, namely ZFC with the axiom schemata of Separation and Replacement allowing for formulas in the language of set theory with the additional predicate symbol
$\mathrm {ZFC}_{\mathbb {P}}$
, namely ZFC with the axiom schemata of Separation and Replacement allowing for formulas in the language of set theory with the additional predicate symbol
![]() $\mathbb {P}$
.
$\mathbb {P}$
.
Definition 9.1 (
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $C^{(n)}$
-extendible cardinal).
$C^{(n)}$
-extendible cardinal).
For
![]() $n\geq 1$
, we say that a cardinal
$n\geq 1$
, we say that a cardinal
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible if for every cardinal
$C^{(n)}$
-extendible if for every cardinal
![]() $\lambda \in C^{(n)}_{\mathbb {P}}$
,
$\lambda \in C^{(n)}_{\mathbb {P}}$
,
![]() $\lambda>\delta $
, there is an ordinal
$\lambda>\delta $
, there is an ordinal
![]() $\theta $
and an elementary embedding
$\theta $
and an elementary embedding
with
![]() $\mathrm {crit}(j)=\delta $
,
$\mathrm {crit}(j)=\delta $
,
![]() $j(\delta )>\lambda $
, and
$j(\delta )>\lambda $
, and
![]() $j(\delta )\in C^{(n)}$
. If, moreover, we can pick
$j(\delta )\in C^{(n)}$
. If, moreover, we can pick
![]() $\theta \in C^{(n)}_{\mathbb {P}}$
, then we say that
$\theta \in C^{(n)}_{\mathbb {P}}$
, then we say that
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)+}$
-extendible.
$C^{(n)+}$
-extendible.
Similarly, we may also consider the notion of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompactness, for a general class
$\Sigma _n$
-supercompactness, for a general class
![]() $\mathbb {P}$
which is not necessarily definable.
$\mathbb {P}$
which is not necessarily definable.
Definition 9.2 (
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _n$
-supercompactness).
$\Sigma _n$
-supercompactness).
If
![]() $n\geq 1$
, then a cardinal
$n\geq 1$
, then a cardinal
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-supercompact if for every
$\Sigma _n$
-supercompact if for every
![]() $\lambda \in C^{(n)}_{\mathbb {P}}$
greater than
$\lambda \in C^{(n)}_{\mathbb {P}}$
greater than
![]() $\delta $
, and every
$\delta $
, and every
![]() $a\in V_\lambda $
there exist
$a\in V_\lambda $
there exist
![]() $\bar {\delta }<\bar {\lambda }<\delta $
and
$\bar {\delta }<\bar {\lambda }<\delta $
and
![]() $\bar {a}\in V_{\bar {\lambda }}$
, and there exists an elementary embedding
$\bar {a}\in V_{\bar {\lambda }}$
, and there exists an elementary embedding
![]() $j: V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that:
$j: V_{\bar {\lambda }}\longrightarrow V_\lambda $
such that:
-
•
 $\mathrm {crit}(j)=\bar {\delta }$
and
$\mathrm {crit}(j)=\bar {\delta }$
and
 $j(\bar {\delta })=\delta $
.
$j(\bar {\delta })=\delta $
. -
•
 $j(\bar {a})=a$
.
$j(\bar {a})=a$
. -
•
 $\bar {\lambda }\in C^{(n)}_{\mathbb {P}}$
.
$\bar {\lambda }\in C^{(n)}_{\mathbb {P}}$
.
The same arguments as in the proof of Theorem 2.4 yield the following equivalence.
Theorem 9.3. For every
![]() $n\geq 1$
, every class
$n\geq 1$
, every class
![]() $\mathbb {P}$
, and every cardinal
$\mathbb {P}$
, and every cardinal
![]() $\kappa $
, the following are equivalent
$\kappa $
, the following are equivalent
![]() $:$
$:$
-
(1)
 $\kappa $
is
$\kappa $
is
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $C^{(n)}$
-extendible.
$C^{(n)}$
-extendible. -
(2)
 $\kappa $
is
$\kappa $
is
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $\Sigma _{n+1}$
-supercompact.
$\Sigma _{n+1}$
-supercompact. -
(3)
 $\kappa $
is
$\kappa $
is
 $\mathbb {P}$
-
$\mathbb {P}$
-
 $C^{(n)+}$
-extendible.Footnote
13
$C^{(n)+}$
-extendible.Footnote
13
We will say that a cardinal
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible with
$C^{(n)}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target if in Definition 9.1 we may moreover require that
$\Sigma _n$
-reflecting target if in Definition 9.1 we may moreover require that
![]() $j(\delta )$
is
$j(\delta )$
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting (cf. Definition 3.15). Likewise, one defines the notion of
$\Sigma _n$
-reflecting (cf. Definition 3.15). Likewise, one defines the notion of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)+}$
-extendible with
$C^{(n)+}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target. Arguing as usual one can check that both notions are equivalent.
$\Sigma _n$
-reflecting target. Arguing as usual one can check that both notions are equivalent.
Clearly, any
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)+}$
-extendible with
$C^{(n)+}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target is
$\Sigma _n$
-reflecting target is
![]() $C^{(n)}$
-extendible. Moreover, if the predicate
$C^{(n)}$
-extendible. Moreover, if the predicate
![]() $\mathbb {P}$
is definable with low complexity and satisfies some minor requirements, then, as the next proposition shows, a cardinal is
$\mathbb {P}$
is definable with low complexity and satisfies some minor requirements, then, as the next proposition shows, a cardinal is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)+}$
-extendible with
$C^{(n)+}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target if and only if is
$\Sigma _n$
-reflecting target if and only if is
![]() $C^{(n)}$
-extendible.
$C^{(n)}$
-extendible.
Proposition 9.4. Let
![]() $n,m\geq 1$
with
$n,m\geq 1$
with
![]() $m\leq n$
. Let
$m\leq n$
. Let
![]() $\mathbb {P}$
be a
$\mathbb {P}$
be a
![]() $\Delta _{m+1}$
-definable
$\Delta _{m+1}$
-definable
![]() $\operatorname {\mathrm {ORD}}$
-length forcing iteration and assume that
$\operatorname {\mathrm {ORD}}$
-length forcing iteration and assume that
is a proper class containing all
![]() $C^{(n-2)}$
-extendible cardinals.Footnote
14
Then, every
$C^{(n-2)}$
-extendible cardinals.Footnote
14
Then, every
![]() $C^{(n)}$
-extendible cardinal belonging to
$C^{(n)}$
-extendible cardinal belonging to
![]() $C^{(m+n+1)}$
is
$C^{(m+n+1)}$
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)+}$
-extendible with
$C^{(n)+}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target.
$\Sigma _n$
-reflecting target.
In particular, if
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _2$
-definable, a cardinal is
$\Delta _2$
-definable, a cardinal is
![]() $C^{(n)}$
-extendible if and only if it is
$C^{(n)}$
-extendible if and only if it is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)+}$
-extendible with
$C^{(n)+}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target.
$\Sigma _n$
-reflecting target.
Proof Let
![]() $\delta $
be a
$\delta $
be a
![]() $C^{(n)}$
-extendible cardinal in
$C^{(n)}$
-extendible cardinal in
![]() $C^{(m+n+1)}$
.
$C^{(m+n+1)}$
.
Claim 9.4.1.
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible.
$C^{(n)}$
-extendible.
Proof of claim Let
![]() $\mu>\lambda >\delta $
be with
$\mu>\lambda >\delta $
be with
![]() $\lambda \in C^{(n)}_{\mathbb {P}}$
and
$\lambda \in C^{(n)}_{\mathbb {P}}$
and
![]() $\mu \in C^{(n)}$
. Since
$\mu \in C^{(n)}$
. Since
![]() $\delta $
is actually
$\delta $
is actually
![]() $C^{(n)+}$
-extendible (cf. Remark 2.5) we may pick
$C^{(n)+}$
-extendible (cf. Remark 2.5) we may pick
![]() $\eta \in C^{(n)}$
together with an elementary embedding
$\eta \in C^{(n)}$
together with an elementary embedding
![]() $j\colon V_\mu \rightarrow V_{\eta }$
with
$j\colon V_\mu \rightarrow V_{\eta }$
with
![]() $\mathrm {crit}(j)=\delta $
,
$\mathrm {crit}(j)=\delta $
,
![]() $j(\delta )>\mu $
, and
$j(\delta )>\mu $
, and
![]() $j(\delta )\in C^{(n)}$
. Observe that
$j(\delta )\in C^{(n)}$
. Observe that
![]() $V_{j(\lambda )}\prec _{\Sigma _n} V_\eta \prec _{\Sigma _n} V$
, hence
$V_{j(\lambda )}\prec _{\Sigma _n} V_\eta \prec _{\Sigma _n} V$
, hence
where the right-most equality follows from
![]() $\Delta _{m+1}$
-definability of
$\Delta _{m+1}$
-definability of
![]() $\mathbb {P}$
and
$\mathbb {P}$
and
![]() $m\leq n$
. Altogether,
$m\leq n$
. Altogether,
![]() $j\upharpoonright \langle V_\lambda ,\in ,\mathbb {P}\cap V_\lambda \rangle $
yields the desired embedding.
$j\upharpoonright \langle V_\lambda ,\in ,\mathbb {P}\cap V_\lambda \rangle $
yields the desired embedding.
By Theorem 9.3,
![]() $\delta $
is actually
$\delta $
is actually
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)+}$
-extendible, so we concentrate on proving the other assertion. Let
$C^{(n)+}$
-extendible, so we concentrate on proving the other assertion. Let
![]() $\lambda \in C^{(n)}_{\mathbb {P}}$
with
$\lambda \in C^{(n)}_{\mathbb {P}}$
with
![]() $\lambda>\delta $
, together with an elementary embedding
$\lambda>\delta $
, together with an elementary embedding
with
![]() $\mathrm {crit}(j)=\delta $
,
$\mathrm {crit}(j)=\delta $
,
![]() $\theta \in C^{(n)}_{\mathbb {P}}$
,
$\theta \in C^{(n)}_{\mathbb {P}}$
,
![]() $j(\delta )>\lambda $
, and
$j(\delta )>\lambda $
, and
![]() $j(\delta )\in C^{(n)}$
. Note that
$j(\delta )\in C^{(n)}$
. Note that
![]() $j(\delta )$
is
$j(\delta )$
is
![]() $C^{(n-2)}$
-extendible hence
$C^{(n-2)}$
-extendible hence
![]() $\mathbb {P}$
-reflecting and a witness for
$\mathbb {P}$
-reflecting and a witness for
![]() $\mathbb {P}\cap V_{j(\delta )}=\mathbb {P}_{j(\delta )}$
.
$\mathbb {P}\cap V_{j(\delta )}=\mathbb {P}_{j(\delta )}$
.
Claim 9.4.2.
![]() $j(\delta )\in C^{(n)}_{\mathbb {P}}$
.
$j(\delta )\in C^{(n)}_{\mathbb {P}}$
.
Proof of Claim Since
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _{m+1}$
-definable,
$\Delta _{m+1}$
-definable,
![]() $C^{(n)}_{\mathbb {P}}$
is a
$C^{(n)}_{\mathbb {P}}$
is a
![]() $\Pi _{n+m}$
-definable club class (cf. Proposition 3.9). In particular, as
$\Pi _{n+m}$
-definable club class (cf. Proposition 3.9). In particular, as
![]() $\delta \in C^{(n+m+1)}$
,
$\delta \in C^{(n+m+1)}$
,
![]() $\delta $
is an accumulation point of
$\delta $
is an accumulation point of
![]() $C^{(n)}_{\mathbb {P}}$
and thus a member of
$C^{(n)}_{\mathbb {P}}$
and thus a member of
![]() $C^{(n)}_{\mathbb {P}}$
.
$C^{(n)}_{\mathbb {P}}$
.
Since
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _{m+1}$
,
$\Delta _{m+1}$
,
![]() $m\leq n$
and
$m\leq n$
and
![]() $\delta ,\lambda \in C^{(n)}_{\mathbb {P}}$
,
$\delta ,\lambda \in C^{(n)}_{\mathbb {P}}$
,
![]() $\langle V_\delta ,\in , \mathbb {P}^{V_\delta }\rangle \prec _{\Sigma _n}\langle V_\lambda ,\in ,\mathbb {P}^{V_\lambda }\rangle $
. By elementarity,
$\langle V_\delta ,\in , \mathbb {P}^{V_\delta }\rangle \prec _{\Sigma _n}\langle V_\lambda ,\in ,\mathbb {P}^{V_\lambda }\rangle $
. By elementarity,
![]() $\langle V_{j(\delta )},\in , \mathbb {P}^{V_{j(\delta )}}\rangle \prec _{\Sigma _n}\langle V_\theta ,\in ,\mathbb {P}^{V_\theta }\rangle $
and so, since
$\langle V_{j(\delta )},\in , \mathbb {P}^{V_{j(\delta )}}\rangle \prec _{\Sigma _n}\langle V_\theta ,\in ,\mathbb {P}^{V_\theta }\rangle $
and so, since
![]() $\theta \in C^{(n)}_{\mathbb {P}}$
,
$\theta \in C^{(n)}_{\mathbb {P}}$
,
![]() $\langle V_{j(\delta )},\in , \mathbb {P}^{V_{j(\delta )}}\rangle \prec _{\Sigma _n}\langle V,\in ,\mathbb {P}\rangle $
. Once again, since
$\langle V_{j(\delta )},\in , \mathbb {P}^{V_{j(\delta )}}\rangle \prec _{\Sigma _n}\langle V,\in ,\mathbb {P}\rangle $
. Once again, since
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _{m+1}$
-definable,
$\Delta _{m+1}$
-definable,
![]() $m\leq n$
and
$m\leq n$
and
![]() $j(\delta )\in C^{(n)}$
,
$j(\delta )\in C^{(n)}$
,
![]() $\mathbb {P}^{V_{j(\delta )}}=\mathbb {P}\cap V_{j(\delta )}$
, hence
$\mathbb {P}^{V_{j(\delta )}}=\mathbb {P}\cap V_{j(\delta )}$
, hence
![]() $j(\delta )\in C^{(n)}_{\mathbb {P}}$
.
$j(\delta )\in C^{(n)}_{\mathbb {P}}$
.
The above claims combined give the proof of the proposition.
The main result of the section is the following:
Theorem 9.5. Let
![]() $\mathbb {P}$
be a
$\mathbb {P}$
be a
![]() $($
not necessarily definable) suitable iteration. Assume that there is a proper class of
$($
not necessarily definable) suitable iteration. Assume that there is a proper class of
![]() $\mathbb {P}$
-reflecting cardinals
$\mathbb {P}$
-reflecting cardinals
![]() $\lambda $
such that
$\lambda $
such that
![]() $\mathbb {P}_\lambda =\mathbb {P}\cap V_\lambda $
.Footnote
15
For each
$\mathbb {P}_\lambda =\mathbb {P}\cap V_\lambda $
.Footnote
15
For each
![]() $n\geq 1$
, if
$n\geq 1$
, if
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible with
$C^{(n)}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target then
$\Sigma _n$
-reflecting target then
Actually for
![]() $n=1$
the above is true by just assuming that
$n=1$
the above is true by just assuming that
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(1)}$
-extendible.
$C^{(1)}$
-extendible.
Proof Let
![]() $\lambda>\delta $
be
$\lambda>\delta $
be
![]() $\mathbb {P}$
-reflecting and such that
$\mathbb {P}$
-reflecting and such that
![]() $\mathbb {P}_\lambda =\mathbb {P}\cap V_\lambda $
. It will be sufficient to prove that if
$\mathbb {P}_\lambda =\mathbb {P}\cap V_\lambda $
. It will be sufficient to prove that if
![]() $G_\lambda $
is
$G_\lambda $
is
![]() $\mathbb {P}_\lambda $
-generic over
$\mathbb {P}_\lambda $
-generic over
![]() $\mathrm {V}$
, then in the generic extension
$\mathrm {V}$
, then in the generic extension
![]() $V[G_\lambda ]$
, the set D of conditions
$V[G_\lambda ]$
, the set D of conditions
![]() $r\in \mathbb {P}_{[\lambda ,Ord)}$
that force the existence of an elementary embedding
$r\in \mathbb {P}_{[\lambda ,Ord)}$
that force the existence of an elementary embedding
some
![]() $\theta $
, with
$\theta $
, with
![]() $\mathrm {crit}(j)=\delta $
,
$\mathrm {crit}(j)=\delta $
,
![]() $j(\delta )>\lambda $
, and
$j(\delta )>\lambda $
, and
![]() $j(\delta )\in C^{(n)}$
, is dense in
$j(\delta )\in C^{(n)}$
, is dense in
![]() $\mathbb {P}_{[\lambda ,Ord)}$
.
$\mathbb {P}_{[\lambda ,Ord)}$
.
In
![]() $V[G_\lambda ]$
, let r be a condition in
$V[G_\lambda ]$
, let r be a condition in
![]() $\mathbb {P}_{[\lambda ,Ord)}$
. Back in
$\mathbb {P}_{[\lambda ,Ord)}$
. Back in
![]() $\mathrm {V}$
, let
$\mathrm {V}$
, let
![]() $\mu \in C^{(n)}_{\mathbb {P}}$
be greater than
$\mu \in C^{(n)}_{\mathbb {P}}$
be greater than
![]() $\lambda $
and such that
$\lambda $
and such that
Since
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)+}$
-extendible with
$C^{(n)+}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target, in the ground model
$\Sigma _n$
-reflecting target, in the ground model
![]() $\mathrm {V}$
there exists an elementary embedding
$\mathrm {V}$
there exists an elementary embedding
with
![]() $\mathrm {crit}(j)=\delta $
such that
$\mathrm {crit}(j)=\delta $
such that
![]() $j(\delta )>\mu $
,
$j(\delta )>\mu $
,
![]() $\theta \in C^{(n)}_{\mathbb {P}}$
, and
$\theta \in C^{(n)}_{\mathbb {P}}$
, and
![]() $ j(\delta )$
being
$ j(\delta )$
being
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting.
$\Sigma _n$
-reflecting.
For each
![]() $q\in \mathbb {P}_\lambda $
there is an ordinal
$q\in \mathbb {P}_\lambda $
there is an ordinal
![]() $\alpha <\delta $
such that
$\alpha <\delta $
such that
![]() $\text {supp}\,(q)\cap \delta \subseteq \alpha $
. Hence,
$\text {supp}\,(q)\cap \delta \subseteq \alpha $
. Hence,
![]() $\text {supp}\,(j(q))\cap j(\delta )\subseteq \alpha $
, and so
$\text {supp}\,(j(q))\cap j(\delta )\subseteq \alpha $
, and so
![]() $j(q)$
is a
$j(q)$
is a
![]() $\mathbb {P}_{j(\lambda )}$
-condition such that
$\mathbb {P}_{j(\lambda )}$
-condition such that

Since
![]() $\mu <j(\delta )$
we have that
$\mu <j(\delta )$
we have that
![]() $\text {supp}\,(j(q))\cap [\lambda ,\mu ) =\varnothing $
. So, by our choice of the ordinal
$\text {supp}\,(j(q))\cap [\lambda ,\mu ) =\varnothing $
. So, by our choice of the ordinal
![]() $\mu $
, in
$\mu $
, in
![]() $V[G_\lambda ]$
we can take
$V[G_\lambda ]$
we can take
![]() $r^*\in \mathbb {P}_{[\mu , Ord)}$
such that
$r^*\in \mathbb {P}_{[\mu , Ord)}$
such that
for all
![]() $q\in G_\lambda $
. Then, the condition
$q\in G_\lambda $
. Then, the condition
![]() $r\wedge r^\ast $
such that
$r\wedge r^\ast $
such that
 $$ \begin{align*}(r\wedge r^*)(\beta):=\left\{\begin{array}{ccc} r(\beta)& \text{if}& \beta\in [\lambda,\mu),\\ r^*(\beta)& \text{if}& \beta\in[\mu, j(\lambda)) \end{array}\right.\end{align*} $$
$$ \begin{align*}(r\wedge r^*)(\beta):=\left\{\begin{array}{ccc} r(\beta)& \text{if}& \beta\in [\lambda,\mu),\\ r^*(\beta)& \text{if}& \beta\in[\mu, j(\lambda)) \end{array}\right.\end{align*} $$
is well-defined and works as a master condition for j and the forcing
![]() $\mathbb {P}_{j(\lambda )}/G_\lambda $
, because
$\mathbb {P}_{j(\lambda )}/G_\lambda $
, because
Thus, for any
![]() $\mathbb {P}_{j(\lambda )}$
-generic filter
$\mathbb {P}_{j(\lambda )}$
-generic filter
![]() $G_{j(\lambda )}$
over
$G_{j(\lambda )}$
over
![]() $\mathrm {V}$
extending
$\mathrm {V}$
extending
![]() $G_\lambda $
and containing
$G_\lambda $
and containing
![]() $r\wedge r^\ast $
, the elementary embedding
$r\wedge r^\ast $
, the elementary embedding
lifts to an elementary embedding
Now, since
![]() $\lambda $
is
$\lambda $
is
![]() $\mathbb {P}$
-reflecting,
$\mathbb {P}$
-reflecting,
![]() $\mathbb {P}$
forces that
$\mathbb {P}$
forces that
![]() $V_\lambda [\dot {G}_\lambda ]=V[\dot {G}]_\lambda $
. Hence, by the choice of
$V_\lambda [\dot {G}_\lambda ]=V[\dot {G}]_\lambda $
. Hence, by the choice of
![]() $\mu $
, the same is forced by
$\mu $
, the same is forced by
![]() $\mathbb {P}_\mu $
. By the elementarity of j, the structure
$\mathbb {P}_\mu $
. By the elementarity of j, the structure
![]() $\langle V_\theta , \in ,\mathbb {P}\cap V_\theta \rangle $
thinks that the forcing
$\langle V_\theta , \in ,\mathbb {P}\cap V_\theta \rangle $
thinks that the forcing
![]() $\mathbb {P}\cap V_\theta $
forces
$\mathbb {P}\cap V_\theta $
forces
![]() $V_{j(\lambda )}[\dot {G}_{j(\lambda )}]=V[\dot {G}]_{j(\lambda )}$
. So, since
$V_{j(\lambda )}[\dot {G}_{j(\lambda )}]=V[\dot {G}]_{j(\lambda )}$
. So, since
![]() $\theta \in C^{(n)}_{\mathbb {P}}$
,
$\theta \in C^{(n)}_{\mathbb {P}}$
,
![]() $\mathbb {P}$
forces the same. Also, since
$\mathbb {P}$
forces the same. Also, since
![]() $j(\delta )$
is
$j(\delta )$
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting in
$\Sigma _n$
-reflecting in
![]() $\mathrm {V}$
and
$\mathrm {V}$
and
![]() $V_{j(\delta )}\models \mathrm {ZFC}$
, Lemma 3.16 yields
$V_{j(\delta )}\models \mathrm {ZFC}$
, Lemma 3.16 yields
We have thus found a condition below r, namely
![]() $r\wedge r^\ast $
, forcing the existence of an elementary embedding
$r\wedge r^\ast $
, forcing the existence of an elementary embedding
with
![]() $\mathrm {crit}(j^\ast )=\delta $
,
$\mathrm {crit}(j^\ast )=\delta $
,
![]() $j^\ast (\delta )>\lambda $
, and
$j^\ast (\delta )>\lambda $
, and
![]() $j^\ast (\delta )\in C^{(n)}$
, as wanted.
$j^\ast (\delta )\in C^{(n)}$
, as wanted.
9.1
 $\mathrm {VP}$
and non-homogeneous suitable iterations
$\mathrm {VP}$
and non-homogeneous suitable iterations
We now use Theorem 9.5 to prove Theorem 6.6 without the homogeneity assumption on
![]() $\mathbb {P}$
, hence yielding the desired refinement of Brooke-Taylor’s main theorem of [Reference Brooke-Taylor8].
$\mathbb {P}$
, hence yielding the desired refinement of Brooke-Taylor’s main theorem of [Reference Brooke-Taylor8].
Theorem 9.6. Let
![]() $n,m\geq 1$
with
$n,m\geq 1$
with
![]() $m\leq n$
. Let
$m\leq n$
. Let
![]() $\mathbb {P}$
be a
$\mathbb {P}$
be a
![]() $\Delta _{m+1}$
-definable suitable iteration. If
$\Delta _{m+1}$
-definable suitable iteration. If
![]() $\mathrm {VP}(\mathbf {\Pi _{m+n+1})}$
holds then
$\mathrm {VP}(\mathbf {\Pi _{m+n+1})}$
holds then
![]() $V^{\mathbb {P}}\models \mathrm {VP}(\mathbf {\Pi _{n+1}})$
.
$V^{\mathbb {P}}\models \mathrm {VP}(\mathbf {\Pi _{n+1}})$
.
In particular, if
![]() $\mathrm {VP}$
holds and
$\mathrm {VP}$
holds and
![]() $\mathbb {P}$
is a definable suitable iteration, then
$\mathbb {P}$
is a definable suitable iteration, then
Proof By Theorem 6.2,
![]() $\mathrm {VP}(\mathbf {\Pi _{m+n+1})}$
yields the existence of a proper class of
$\mathrm {VP}(\mathbf {\Pi _{m+n+1})}$
yields the existence of a proper class of
![]() $C^{(m+n)}$
-extendible cardinals. Also, by Theorem 6.5, it entails the existence of a proper class of
$C^{(m+n)}$
-extendible cardinals. Also, by Theorem 6.5, it entails the existence of a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _{n+1}$
-reflecting cardinals, hence a proper class of
$\Sigma _{n+1}$
-reflecting cardinals, hence a proper class of
![]() $\mathbb {P}$
-reflecting cardinals
$\mathbb {P}$
-reflecting cardinals
![]() $\lambda $
such that
$\lambda $
such that
![]() $\mathbb {P}_\lambda =\mathbb {P}\cap V_\lambda $
. In particular, the assumptions of Theorem 9.6 are met.
$\mathbb {P}_\lambda =\mathbb {P}\cap V_\lambda $
. In particular, the assumptions of Theorem 9.6 are met.
Claim 9.6.1. For each
![]() $n\geq 2$
, every
$n\geq 2$
, every
![]() $C^{(m+n)}$
-extendible is
$C^{(m+n)}$
-extendible is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible with
$C^{(n)}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target.
$\Sigma _n$
-reflecting target.
Proof of claim If
![]() $\delta $
is
$\delta $
is
![]() $C^{(m+n)}$
-extendible then it is
$C^{(m+n)}$
-extendible then it is
![]() $C^{(n)}$
-extendible and
$C^{(n)}$
-extendible and
![]() $\Sigma _{m+n+2}$
-correct. Thus, Claim 9.4.2 implies that
$\Sigma _{m+n+2}$
-correct. Thus, Claim 9.4.2 implies that
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible.
$C^{(n)}$
-extendible.
Let
![]() $\lambda \in C^{(n)}_{\mathbb {P}}$
be with
$\lambda \in C^{(n)}_{\mathbb {P}}$
be with
![]() $\lambda>\theta $
. By
$\lambda>\theta $
. By
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)+}$
-extendibility of
$C^{(n)+}$
-extendibility of
![]() $\delta $
, there is
$\delta $
, there is
![]() $\theta \in C^{(n)}_{\mathbb {P}}$
and an elementary embedding
$\theta \in C^{(n)}_{\mathbb {P}}$
and an elementary embedding
with
![]() $\mathrm {crit}(j)=\delta $
,
$\mathrm {crit}(j)=\delta $
,
![]() $j(\delta )>\lambda $
, and
$j(\delta )>\lambda $
, and
![]() $j(\delta )\in C^{(n)}$
.
$j(\delta )\in C^{(n)}$
.
Subclaim 9.6.1.1.
![]() $j(\delta )$
is
$j(\delta )$
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting.
$\Sigma _n$
-reflecting.
Proof of subclaim Since
![]() $\delta $
is
$\delta $
is
![]() $C^{(n+m)}$
-extendible, hence
$C^{(n+m)}$
-extendible, hence
![]() $C^{(n)}$
-extendible and
$C^{(n)}$
-extendible and
![]() $\delta \in C^{(n+m+1)}$
, Claim 9.4.2 yields
$\delta \in C^{(n+m+1)}$
, Claim 9.4.2 yields
![]() $j(\delta )\in C^{(n)}_{\mathbb {P}}$
. Let us now check that
$j(\delta )\in C^{(n)}_{\mathbb {P}}$
. Let us now check that
![]() $\mathbb {P}_{j(\delta )}=\mathbb {P}\cap V_{j(\delta )}$
and that
$\mathbb {P}_{j(\delta )}=\mathbb {P}\cap V_{j(\delta )}$
and that
![]() $j(\delta )$
is
$j(\delta )$
is
![]() $\mathbb {P}$
-reflecting.
$\mathbb {P}$
-reflecting.
For each
![]() $\alpha <j(\delta )$
the formula
$\alpha <j(\delta )$
the formula
is
![]() $\Sigma _{m+1}$
, with parameter
$\Sigma _{m+1}$
, with parameter
![]() $\alpha $
. Since
$\alpha $
. Since
![]() $j(\delta )\in C^{(n+m)}$
, for each
$j(\delta )\in C^{(n+m)}$
, for each
![]() $\alpha <j(\delta )$
there is
$\alpha <j(\delta )$
there is
![]() $\alpha <\beta <j(\delta )$
witnessing the above. Combining this with the
$\alpha <\beta <j(\delta )$
witnessing the above. Combining this with the
![]() $\Delta _{m+1}$
-definability of
$\Delta _{m+1}$
-definability of
![]() $\mathbb {P}$
and with
$\mathbb {P}$
and with
![]() $1\leq m\leq n$
it follows that
$1\leq m\leq n$
it follows that
Let us now prove that
![]() $j(\delta )$
is
$j(\delta )$
is
![]() $\mathbb {P}$
-reflecting by showing that all the assumptions of Proposition 3.4 are met. First,
$\mathbb {P}$
-reflecting by showing that all the assumptions of Proposition 3.4 are met. First,
![]() $j(\delta )$
is inaccessible (actually, Mahlo) and by the above displayed expression
$j(\delta )$
is inaccessible (actually, Mahlo) and by the above displayed expression
![]() $\mathbb {P}$
is a forcing iteration such that
$\mathbb {P}$
is a forcing iteration such that
![]() $\mathbb {P}_{j(\delta )}\subseteq V_{j(\delta )}$
. Second, thanks to the Mahloness of
$\mathbb {P}_{j(\delta )}\subseteq V_{j(\delta )}$
. Second, thanks to the Mahloness of
![]() $j(\delta )$
, the iteration
$j(\delta )$
, the iteration
![]() $\mathbb {P}_{j(\delta )}$
is
$\mathbb {P}_{j(\delta )}$
is
![]() $j(\delta )$
-cc and so it is not hard to show that
$j(\delta )$
-cc and so it is not hard to show that
![]() $\mathbb {P}_{j(\delta )}$
preserves the inaccessibility of
$\mathbb {P}_{j(\delta )}$
preserves the inaccessibility of
![]() $j(\delta )$
. Finally, we claim that
$j(\delta )$
. Finally, we claim that
![]() $j(\delta )\in C(\mathbb {P})$
, and so that
$j(\delta )\in C(\mathbb {P})$
, and so that
![]() $\Vdash _{\mathbb {P}_{j(\delta )}}\text {"}\mathbb {P}_{[j(\delta ),\operatorname {\mathrm {ORD}})} \text { is } j(\delta )\text {-distributive."}$
$\Vdash _{\mathbb {P}_{j(\delta )}}\text {"}\mathbb {P}_{[j(\delta ),\operatorname {\mathrm {ORD}})} \text { is } j(\delta )\text {-distributive."}$
Indeed, arguing as in the proof of Theorem 5.4 and using that the cardinal
![]() $j(\delta )$
is
$j(\delta )$
is
![]() $\Sigma _{m+n}$
-correct, hence
$\Sigma _{m+n}$
-correct, hence
![]() $\Sigma _{m+2}$
-correct, one infers that
$\Sigma _{m+2}$
-correct, one infers that
![]() $j(\delta )$
is an accumulation point of
$j(\delta )$
is an accumulation point of
![]() $C(\mathbb {P})$
, hence
$C(\mathbb {P})$
, hence
![]() $j(\delta )\in C(\mathbb {P})$
.Footnote
16
$j(\delta )\in C(\mathbb {P})$
.Footnote
16
This shows that
![]() $\delta $
is
$\delta $
is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible with
$C^{(n)}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target.
$\Sigma _n$
-reflecting target.
For simplicity let us assume that
![]() $n\geq 2$
, as the argument for
$n\geq 2$
, as the argument for
![]() $n=1$
is the same. The above claim alongside the previous comments implies the existence of a proper class of
$n=1$
is the same. The above claim alongside the previous comments implies the existence of a proper class of
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible cardinals with
$C^{(n)}$
-extendible cardinals with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target, hence Theorem 9.5 yields the existence of a proper class of
$\Sigma _n$
-reflecting target, hence Theorem 9.5 yields the existence of a proper class of
![]() $C^{(n)}$
-extendible cardinals in
$C^{(n)}$
-extendible cardinals in
![]() $V^{\mathbb {P}}$
and so
$V^{\mathbb {P}}$
and so
![]() $V^{\mathbb {P}}\models \mathrm {VP}(\mathbf {\Pi _{n+1}})$
.
$V^{\mathbb {P}}\models \mathrm {VP}(\mathbf {\Pi _{n+1}})$
.
9.2 On
 $\mathrm {V=HOD}$
and the Ground Axiom
$\mathrm {V=HOD}$
and the Ground Axiom
The first forcing iteration producing a generic extension where
![]() $\mathrm {V}=\mathrm {HOD}$
holds was defined by McAloon [Reference McAloon22]. The idea is to code the universe into the power-set function pattern so that all sets become definable using ordinals as parameters. For more sophisticated codings see [Reference Brooke-Taylor7].
$\mathrm {V}=\mathrm {HOD}$
holds was defined by McAloon [Reference McAloon22]. The idea is to code the universe into the power-set function pattern so that all sets become definable using ordinals as parameters. For more sophisticated codings see [Reference Brooke-Taylor7].
For the purposes of the current section we may also assume that the GCH holds, for otherwise we can force it while preserving
![]() $C^{(n)}$
-extendible cardinals (cf. Theorem 8.4).
$C^{(n)}$
-extendible cardinals (cf. Theorem 8.4).
Let
![]() $\mathbb {P}$
be the class forcing notion from [Reference Reitz23, Theorem 3.3]. It is easy to see that
$\mathbb {P}$
be the class forcing notion from [Reference Reitz23, Theorem 3.3]. It is easy to see that
![]() $\mathbb {P}$
is
$\mathbb {P}$
is
![]() $\Delta _2$
-definable and that the class of
$\Delta _2$
-definable and that the class of
![]() $\mathbb {P}$
-reflecting cardinals
$\mathbb {P}$
-reflecting cardinals
![]() $\kappa $
such that
$\kappa $
such that
![]() $ \mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
contains all Mahlo cardinals, if there are any. Moreover, if there is a
$ \mathbb {P}\cap V_\kappa =\mathbb {P}_\kappa $
contains all Mahlo cardinals, if there are any. Moreover, if there is a
![]() $C^{(n)}$
-extendible cardinal, there are class many Mahlo cardinals, hence the above class is proper, and every
$C^{(n)}$
-extendible cardinal, there are class many Mahlo cardinals, hence the above class is proper, and every
![]() $C^{(n)}$
-extendible cardinal is also
$C^{(n)}$
-extendible cardinal is also
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible with
$C^{(n)}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target (see Proposition 9.4). Thus
$\Sigma _n$
-reflecting target (see Proposition 9.4). Thus
![]() $\mathbb {P}$
fulfils the assumptions of Theorem 9.5. As the GCH holds,
$\mathbb {P}$
fulfils the assumptions of Theorem 9.5. As the GCH holds,
![]() $\mathbb {P}$
yields a cardinal-preserving generic extension in which the Continuum Coding Axiom (CCA) holds, hence where
$\mathbb {P}$
yields a cardinal-preserving generic extension in which the Continuum Coding Axiom (CCA) holds, hence where
![]() $\mathrm {V}=\mathrm {HOD}$
holds [Reference Reitz23, Theorem 3.3]. Altogether, we obtain the following, which extends [Reference Reitz23, Theorem 3.9].
$\mathrm {V}=\mathrm {HOD}$
holds [Reference Reitz23, Theorem 3.3]. Altogether, we obtain the following, which extends [Reference Reitz23, Theorem 3.9].
Corollary 9.7. Forcing with
![]() $\mathbb {P}$
produces a generic extension of
$\mathbb {P}$
produces a generic extension of
![]() $\mathrm {CCA}+\neg \mathrm {GCH}$
and preserves
$\mathrm {CCA}+\neg \mathrm {GCH}$
and preserves
![]() $C^{(n)}$
-extendible cardinals, for
$C^{(n)}$
-extendible cardinals, for
![]() $n\geq 1$
. In particular,
$n\geq 1$
. In particular,
![]() $C^{(n)}$
-extendible cardinals are consistent with
$C^{(n)}$
-extendible cardinals are consistent with
![]() $\mathrm {V=HOD}$
.
$\mathrm {V=HOD}$
.
Likewise, the following extends [Reference Hamkins, Reitz and Woodin14, Corollary 4]:
Corollary 9.8. There is a class iteration forcing “
![]() $\text {V}\neq \text {HOD}+\text {GA}$
” and preserving
$\text {V}\neq \text {HOD}+\text {GA}$
” and preserving
![]() $C^{(n)}$
-extendible cardinals, for
$C^{(n)}$
-extendible cardinals, for
![]() $n\geq 1$
.
$n\geq 1$
.
Proof Let
![]() $\mathbb {P}$
be the forcing iteration of Corollary 9.7 and let
$\mathbb {P}$
be the forcing iteration of Corollary 9.7 and let
![]() $\dot {\mathbb {Q}}$
be a
$\dot {\mathbb {Q}}$
be a
![]() $\mathbb {P}$
-name for the iteration with Easton support that forces with
$\mathbb {P}$
-name for the iteration with Easton support that forces with
![]() $\mathrm {Add}(\kappa ,1)$
at each regular cardinal
$\mathrm {Add}(\kappa ,1)$
at each regular cardinal
![]() $\kappa $
such that
$\kappa $
such that
![]() $2^{<\kappa }=\kappa $
. Set
$2^{<\kappa }=\kappa $
. Set
![]() $\mathbb {R}:=\mathbb {P}\ast \dot {\mathbb {Q}}$
. By the argument in [Reference Hamkins, Reitz and Woodin14, Theorem 3],
$\mathbb {R}:=\mathbb {P}\ast \dot {\mathbb {Q}}$
. By the argument in [Reference Hamkins, Reitz and Woodin14, Theorem 3],
![]() $V^{\mathbb {R}}\models \text {"V}\neq \text {HOD}+\text {GA."}$
Since
$V^{\mathbb {R}}\models \text {"V}\neq \text {HOD}+\text {GA."}$
Since
![]() $\mathbb {R}$
is
$\mathbb {R}$
is
![]() $\Delta _2$
-definable and the class of
$\Delta _2$
-definable and the class of
![]() $\mathbb {R}$
-reflecting cardinals
$\mathbb {R}$
-reflecting cardinals
![]() $\kappa $
such that
$\kappa $
such that
![]() $ \mathbb {R}\cap V_\kappa =\mathbb {R}_\kappa $
contains all Mahlo cardinals, every
$ \mathbb {R}\cap V_\kappa =\mathbb {R}_\kappa $
contains all Mahlo cardinals, every
![]() $C^{(n)}$
-extendible cardinal is
$C^{(n)}$
-extendible cardinal is
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $C^{(n)}$
-extendible with
$C^{(n)}$
-extendible with
![]() $\mathbb {P}$
-
$\mathbb {P}$
-
![]() $\Sigma _n$
-reflecting target. As
$\Sigma _n$
-reflecting target. As
![]() $\mathbb {R}$
satisfies the hypotheses of Theorem 9.5, the result follows.
$\mathbb {R}$
satisfies the hypotheses of Theorem 9.5, the result follows.
Acknowledgments
The authors are grateful to the anonymous referee for his valuable suggestions on previous versions of the manuscript. This gratitude is extended to Hiroshi Sakai for his very efficient task as editor. The research work of both authors was supported by the Spanish Government under grant MTM2017-86777-P, and by the Generalitat de Catalunya (Catalan Government) under grant SGR 270-2017. The research of the second author has also been supported by MECD Grant FPU15/00026.