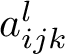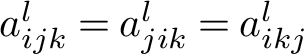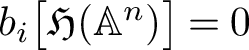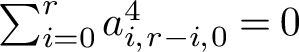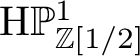1 Introduction
Quadratic forms, topology and
 $\mathbb A^1$
-homotopy
$\mathbb A^1$
-homotopy
The K-theoretic study of quadratic forms has its origin in Bass’s 1965–1966 lectures at the Tata institute (see [Reference Bass and Roy8]), building on Wall’s invariant of a quadratic form; the 0th and first groups of what was later called Hermitian K-theory appeared there. It was quickly linked with the so-called surgery problem by Wall in [Reference Wall40], where the L-group variant was introduced. The term Hermitian K-theory, for Bass’s initial groups and their higher version, was introduced by Karoubi and Villamayor in their CRAS paper [Reference Karoubi and Villamayor18] dated from 1971. One year earlier, in his famous paper [Reference Milnor23], Milnor provided the first links between K-theory defined via symbols (i.e., Milnor K-theory), Galois cohomology and Witt groups, sowing the seed of what has become motivic homotopy theory. The theory of Morel and Voevodsky – introduced in 2000 and originally called
![]() $\mathbb A^1$
-homotopy theory – soon revealed its connections with quadratic forms under the leadership of Morel. Indeed, the analysis of Voevodsky’s proof of Milnor conjecture led Morel to the main computation of
$\mathbb A^1$
-homotopy theory – soon revealed its connections with quadratic forms under the leadership of Morel. Indeed, the analysis of Voevodsky’s proof of Milnor conjecture led Morel to the main computation of
![]() $\mathbb A^1$
-homotopy theory to date: the identification of the
$\mathbb A^1$
-homotopy theory to date: the identification of the
![]() $0$
th stable homotopy group of the sphere spectrum with the Grothendieck-Witt ring of the base field k and, further, of the
$0$
th stable homotopy group of the sphere spectrum with the Grothendieck-Witt ring of the base field k and, further, of the
![]() $\mathbb Z$
-graded
$\mathbb Z$
-graded
![]() $0$
th stable homotopy groupFootnote 1 with the so-called Milnor-Witt K-theory of k (see [Reference Morel25, Cor. 1.25]).
$0$
th stable homotopy groupFootnote 1 with the so-called Milnor-Witt K-theory of k (see [Reference Morel25, Cor. 1.25]).
Before going further into the quadratic part of motivic homotopy theory, let us go back in history for a moment to one of the sources of Beilinson’s program, the Chern character. From the initial point of view, say, over a smooth k-variety X (more generally, a regular scheme X), this is a rational ring isomorphism between the Grothendieck group of vector bundles over X and the Chow ring of X, an incarnation of Serre’s intersection Tor formula. Grothendieck’s initial breakthrough motivated a thorough line of research in algebraic topology, which tries to classify spectra (i.e., representable cohomology theories) in terms of their characteristic classes. After Quillen and Adams, to any (complex) oriented spectra is associated a formal group law
![]() $F(x,y)$
(or FGL for short) that expresses the behaviour of its associated first Chern classes with respect to tensor products: For line bundles L and
$F(x,y)$
(or FGL for short) that expresses the behaviour of its associated first Chern classes with respect to tensor products: For line bundles L and
![]() $L'$
, we have
$L'$
, we have
This gives the following classical results:
-
• singular cohomology is the universal such theory with additive FGL;
-
• complex K-theory is the universal one with multiplicative FGL;
-
• cobordism is the universal one with the universal FGL.
The topological Chern character is then interpreted as the unique rational morphism of oriented spectra from the multiplicative one to the additive one.Footnote 2 Further, rationally, all cohomologies are ‘ordinary’: a direct sum of copies of singular cohomologies, in particular oriented.
The notion of oriented cohomology theory was naturally extended to
![]() $\mathbb A^1$
-homotopy theory, giving the following table of analogiesFootnote 3:
$\mathbb A^1$
-homotopy theory, giving the following table of analogiesFootnote 3:

In the motivic context, the Chern character was constructed by Riou (cf. [Reference Riou30]), extending the initial work of Gillet and Soulé (cf. [Reference Soulé35]). Despite this appealing analogy, in rational stable
![]() $\mathbb A^1$
-homotopy not all cohomologies reduce to motivic cohomology. For example, Chow-Witt groups are not oriented in general. Back to our starting point, Hermitian K-theory, though representable (over regular bases), is also nonorientable, even with rational coefficients.
$\mathbb A^1$
-homotopy not all cohomologies reduce to motivic cohomology. For example, Chow-Witt groups are not oriented in general. Back to our starting point, Hermitian K-theory, though representable (over regular bases), is also nonorientable, even with rational coefficients.
Panin-Walter weak orientations
Motivated by these examples, Panin and Walter introduced in a series of fundamental papers a weaker notion of orientation ([Reference Panin and Walter28, Reference Panin and Walter26]). Recall that an orientation on a ring spectrum
![]() $\mathbb E$
in the stable homotopy category
$\mathbb E$
in the stable homotopy category
![]() $\operatorname {\mathrm {SH}}(S)$
can be expressed as the data for each vector bundle
$\operatorname {\mathrm {SH}}(S)$
can be expressed as the data for each vector bundle
![]() $V/X$
over a smooth S-scheme X of rank n of an isomorphism, called the Thom isomorphism:
$V/X$
over a smooth S-scheme X of rank n of an isomorphism, called the Thom isomorphism:
where
![]() $\mathbb E^{**}$
is the associated (bigraded) cohomology and
$\mathbb E^{**}$
is the associated (bigraded) cohomology and
![]() $\operatorname {\mathrm {Th}}(V)=V/V^\times $
is the Thom space of V. The idea of Panin and Walter is to ask for the existence of Thom isomorphisms only for a restricted class of vector bundles; namely, those corresponding to G-torsors for linear algebraic groups such as
$\operatorname {\mathrm {Th}}(V)=V/V^\times $
is the Thom space of V. The idea of Panin and Walter is to ask for the existence of Thom isomorphisms only for a restricted class of vector bundles; namely, those corresponding to G-torsors for linear algebraic groups such as
![]() $\mathrm {SL}_n$
or
$\mathrm {SL}_n$
or
![]() $\mathrm {Sp}_{2r}$
(in which case,
$\mathrm {Sp}_{2r}$
(in which case,
![]() $n=2r$
is even). These give rise to the notions of
$n=2r$
is even). These give rise to the notions of
![]() $\mathrm {SL}$
-orientation and
$\mathrm {SL}$
-orientation and
![]() $\mathrm {Sp}$
-orientation (the later is weaker; see Definition 2.1.3 for details). The extraordinary cohomology theories mentioned above fulfil this new axiomatisation. Moreover, they are organised in mirror of the classically oriented cohomologies, as described in the following table:
$\mathrm {Sp}$
-orientation (the later is weaker; see Definition 2.1.3 for details). The extraordinary cohomology theories mentioned above fulfil this new axiomatisation. Moreover, they are organised in mirror of the classically oriented cohomologies, as described in the following table:

MW-motivic cohomology (see [Reference Bachmann, Calmès, Déglise, Fasel and Østvær7]) is to Chow-Witt groups what motivic cohomology groups are to usual Chow groups. We will denote by
![]() $\mathbf {H}_{\mathrm {MW}} R_S$
the ring spectrum that represents R-linear MW-motivic cohomology over a scheme S and refer the reader to our conventions at the end of this introduction for more precision. Note also that higher GW-theory is a shortcut for higher Grothendieck-Witt groups. This terminology is used in [Reference Schlichting and Tripathi34]. Note that higher GW-theory agrees with K-theory of symplectic (respectively symmetric) bundles in bidegree
$\mathbf {H}_{\mathrm {MW}} R_S$
the ring spectrum that represents R-linear MW-motivic cohomology over a scheme S and refer the reader to our conventions at the end of this introduction for more precision. Note also that higher GW-theory is a shortcut for higher Grothendieck-Witt groups. This terminology is used in [Reference Schlichting and Tripathi34]. Note that higher GW-theory agrees with K-theory of symplectic (respectively symmetric) bundles in bidegree
![]() $(8n+4,4n+2)$
(respectively
$(8n+4,4n+2)$
(respectively
![]() $(8n,4n)$
).Footnote 4
$(8n,4n)$
).Footnote 4
Let us focus on the notion of
![]() $\mathrm {Sp}$
-orientation in this introduction. Recall that an
$\mathrm {Sp}$
-orientation in this introduction. Recall that an
![]() $\mathrm {Sp}$
-torsor corresponds to a vector bundle V equipped with a nondegenerate symplectic form
$\mathrm {Sp}$
-torsor corresponds to a vector bundle V equipped with a nondegenerate symplectic form
![]() $\psi $
, which we call symplectic bundles. Panin and Walter have associated to any symplectic bundle a quaternionic projective bundle
$\psi $
, which we call symplectic bundles. Panin and Walter have associated to any symplectic bundle a quaternionic projective bundle
![]() $\mathrm {H}\mathbb {P}(V,\psi )$
, equipped with a canonical rank 2 symplectic bundle (see Paragraph 2.2.2 for details); below,
$\mathrm {H}\mathbb {P}(V,\psi )$
, equipped with a canonical rank 2 symplectic bundle (see Paragraph 2.2.2 for details); below,
![]() $\mathrm {H}\mathbb {P}^\infty $
denotes the infinite-dimensional quaternionic projective space. Given these considerations, the beauty of
$\mathrm {H}\mathbb {P}^\infty $
denotes the infinite-dimensional quaternionic projective space. Given these considerations, the beauty of
![]() $\mathrm {Sp}$
-orientations, say, on a ring spectrum
$\mathrm {Sp}$
-orientations, say, on a ring spectrum
![]() $\mathbb E$
, is to be perfectly analogous to classical orientations, as summarised in the following table:
$\mathbb E$
, is to be perfectly analogous to classical orientations, as summarised in the following table:

We refer the reader to Section 2 for details on the notions and definitions appearing on the right-hand side. Given this perfect table, one is naturally led to wonder what is the analogue of formal group laws in in the symplectically oriented case. The problem with the formula (FGL) in the symplectic case is that a tensor product of two symplectic bundles is not a symplectic bundle: indeed, the tensor product of two symplectic forms is not symplectic but symmetric. However, a triple product of symplectic forms is again symplectic.
Walter’s ternary laws
This leads to the notion of ternary laws that we introduce in this article following an unpublished work of Walter. The idea is formally identical to that of formal group laws, except that we work with
![]() $P=\mathrm {H}\mathbb {P}^\infty _S$
over some base S and triple products. Let us give the formula for the comfort of the reader (see Definition 2.3.2). On
$P=\mathrm {H}\mathbb {P}^\infty _S$
over some base S and triple products. Let us give the formula for the comfort of the reader (see Definition 2.3.2). On
![]() $P^3$
, we get three tautological symplectic bundles
$P^3$
, we get three tautological symplectic bundles
![]() $\mathfrak U_1$
,
$\mathfrak U_1$
,
![]() $\mathfrak U_2$
,
$\mathfrak U_2$
,
![]() $\mathfrak U_3$
. Then
$\mathfrak U_3$
. Then
![]() $\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3$
has rank
$\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3$
has rank
![]() $8$
and admits four nontrivial Borel classes. In particular, one associates to an
$8$
and admits four nontrivial Borel classes. In particular, one associates to an
![]() $\mathrm {Sp}$
-oriented ring spectrum
$\mathrm {Sp}$
-oriented ring spectrum
![]() $\mathbb E$
over S four power series in three variables
$\mathbb E$
over S four power series in three variables
![]() $x,y,z$
, say:
$x,y,z$
, say:
 $$ \begin{align*}F_l(x,y,z)=\sum_{i,j,k \geq 0} a^l_{ijk}.x^iy^jz^k, l=1,2,3,4 \end{align*} $$
$$ \begin{align*}F_l(x,y,z)=\sum_{i,j,k \geq 0} a^l_{ijk}.x^iy^jz^k, l=1,2,3,4 \end{align*} $$
defined by the formula in
![]() $\mathbb E^{**}(S)[[b_1(\mathfrak U_1),b_1(\mathfrak U_2),b_1(\mathfrak U_3)]]$
:
$\mathbb E^{**}(S)[[b_1(\mathfrak U_1),b_1(\mathfrak U_2),b_1(\mathfrak U_3)]]$
:
 $$ \begin{align*} F_l\big(b_1(\mathfrak U_1),b_1(\mathfrak U_2),b_1(\mathfrak U_3)\big):=b_l(\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3). \end{align*} $$
$$ \begin{align*} F_l\big(b_1(\mathfrak U_1),b_1(\mathfrak U_2),b_1(\mathfrak U_3)\big):=b_l(\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3). \end{align*} $$
The situation is thus notably more complicated than the
![]() $\mathrm {GL}$
-oriented case. Nevertheless, one can derive some relations amongst the coefficients
$\mathrm {GL}$
-oriented case. Nevertheless, one can derive some relations amongst the coefficients
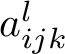 $a_{ijk}^l$
that we summarise here:
$a_{ijk}^l$
that we summarise here:
-
• Degree:
 $a_{ijk}^l$
is a cohomology class in
$a_{ijk}^l$
is a cohomology class in
 $\mathbb E^{4d,2d}(S)$
,
$\mathbb E^{4d,2d}(S)$
,
 $d=l-i-j-k$
.
$d=l-i-j-k$
. -
• Symmetry (Paragraph 2.3.3): for all integers
 $i,j,k$
,
$i,j,k$
,
 $a_{ijk}^l=a_{jik}^l=a_{ikj}^l$
.
$a_{ijk}^l=a_{jik}^l=a_{ikj}^l$
. -
• Neutral identities (Proposition 2.3.4): when
 $\mathbb E$
is
$\mathbb E$
is
 $\mathrm {SL}$
-oriented, one gets where h and
$\mathrm {SL}$
-oriented, one gets where h and $$ \begin{align*} & i \neq l \Rightarrow a^l_{i00}=0, \\ & a^1_{100}=a^3_{300}=2h, \ a^2_{200}=2(h-\epsilon), \ a^4_{400}=1, \\ & {\sum}_{i=0}^r a^4_{i,r-i,0}=0, \end{align*} $$
$$ \begin{align*} & i \neq l \Rightarrow a^l_{i00}=0, \\ & a^1_{100}=a^3_{300}=2h, \ a^2_{200}=2(h-\epsilon), \ a^4_{400}=1, \\ & {\sum}_{i=0}^r a^4_{i,r-i,0}=0, \end{align*} $$
 $\epsilon $
are the image of natural endomorphisms of the sphere spectrum over S via the ring map
$\epsilon $
are the image of natural endomorphisms of the sphere spectrum over S via the ring map
 $\operatorname {\mathrm {End}}(\mathbb S_S) \rightarrow \mathbb E^{00}(S)$
.Footnote 5
$\operatorname {\mathrm {End}}(\mathbb S_S) \rightarrow \mathbb E^{00}(S)$
.Footnote 5
Our first contribution is to determine the ternary laws associated with MW-motivic cohomology.
Theorem A See Theorem 3.3.2 and Corollary 3.3.5
Let R be a ring of coefficients and S a scheme such that one of the following conditions hold:
-
(a)
 $R=\mathbb Z$
, S is a scheme over a perfect field k of characteristic
$R=\mathbb Z$
, S is a scheme over a perfect field k of characteristic
 $\neq 2,3$
.
$\neq 2,3$
. -
(b)
 $R=\mathbb Q$
, S is an arbitrary scheme.
$R=\mathbb Q$
, S is an arbitrary scheme.
Then the
![]() $\mathrm {SL}$
-oriented ring spectrum
$\mathrm {SL}$
-oriented ring spectrum
![]() $\mathbf {H}_{\mathrm {MW}} R_S$
has the following ternary laws:
$\mathbf {H}_{\mathrm {MW}} R_S$
has the following ternary laws:
 $$ \begin{align*}F_l(x,y,z)= \begin{cases} 2h.\sigma(x), & l=1, \\ 2(h-\epsilon).\sigma(x^2)+2h.\sigma(xy), & l=2, \\ 2h.\sigma(x^3)+2h.\sigma(x^2y)+8(2h-\epsilon).xyz, & l=3, \\ \sigma(x^4)-2h.\sigma(x^3y)+2(h-\epsilon).\sigma(x^2y^2)+2h.\sigma(x^2yz), & l=4 \end{cases} \end{align*} $$
$$ \begin{align*}F_l(x,y,z)= \begin{cases} 2h.\sigma(x), & l=1, \\ 2(h-\epsilon).\sigma(x^2)+2h.\sigma(xy), & l=2, \\ 2h.\sigma(x^3)+2h.\sigma(x^2y)+8(2h-\epsilon).xyz, & l=3, \\ \sigma(x^4)-2h.\sigma(x^3y)+2(h-\epsilon).\sigma(x^2y^2)+2h.\sigma(x^2yz), & l=4 \end{cases} \end{align*} $$
where for a monomial
![]() $P=x^ay^bz^c$
,
$P=x^ay^bz^c$
,
![]() $\sigma (x^ay^bz^c)$
means the symmetric polynomial obtained as the sum of the elements in the orbit of P under the action of the symmetric group permuting the variables
$\sigma (x^ay^bz^c)$
means the symmetric polynomial obtained as the sum of the elements in the orbit of P under the action of the symmetric group permuting the variables
![]() $x,y,z$
.
$x,y,z$
.
Given the preceding tables of analogy, these ternary laws are the analogue of the additive formal group law and we call them the additive ternary laws. The theorem ultimately reduces to case (a) and to the ‘Witt part’ of MW-motivic cohomology, which represents unramified Witt cohomology. In this latter case, we are able to compute the relevant Borel classes of triple products of symplectic rank
![]() $2$
bundles, based on previous computations of Levine (see [Reference Levine20]) and on a geometric determination of the associated symplectic form (this is where we have to assume that
$2$
bundles, based on previous computations of Levine (see [Reference Levine20]) and on a geometric determination of the associated symplectic form (this is where we have to assume that
![]() $6 \in k^\times $
; see Appendix A).
$6 \in k^\times $
; see Appendix A).
The Borel character
The main contribution of our article is the construction of the symplectic analogue of the Chern character, which we call the Borel character. Recall that the former was introduced by Grothendieck as a bridge between
![]() $K_0$
-theory and Chow groups, later extended to higher K-theory and higher Chow groups. In the interpretation of Riou [Reference Riou30], it appears as an isomorphism between the rational (homotopy invariant) K-theory spectrum and the periodised rational motivic cohomology spectrum (to reflect Bott periodicity of K-theory). It can also be interpreted as the unique isomorphism between the universal rational ring spectra respectively with multiplicative and additive formal group laws, reflecting now the exponential map between these formal group laws.
$K_0$
-theory and Chow groups, later extended to higher K-theory and higher Chow groups. In the interpretation of Riou [Reference Riou30], it appears as an isomorphism between the rational (homotopy invariant) K-theory spectrum and the periodised rational motivic cohomology spectrum (to reflect Bott periodicity of K-theory). It can also be interpreted as the unique isomorphism between the universal rational ring spectra respectively with multiplicative and additive formal group laws, reflecting now the exponential map between these formal group laws.
The method of Riou for building the Chern character uses the classical toolkit for studying cohomological operations in stable homotopy, as pioneered by Adams. In this setting, there is an important conceptual distinction between the unstable and stable operations. In his fundamental work, Riou not only computed all unstable operations in algebraic K-theory but also gave criteria so that these operations can be lifted to stable ones.
In our article, we extend Riou’s method to the symplectic case, replacing K-theory by Hermitian K-theory. Moreover, we slightly extend the domain of applicability of Riou’s result by determining all possible natural transformations for presheaves over the category of smooth S-schemes
![]() $\mathscr Sm_S$
between the Hermitian K-theory
$\mathscr Sm_S$
between the Hermitian K-theory
![]() $\mathbf {KSp}_0$
and some cohomology group with coefficients in an arbitrary
$\mathbf {KSp}_0$
and some cohomology group with coefficients in an arbitrary
![]() $\mathrm {Sp}$
-oriented cohomology. More precisely:
$\mathrm {Sp}$
-oriented cohomology. More precisely:
Theorem B see Theorems 4.1.4 and 4.4.6
Let
![]() $\mathbb E$
be an
$\mathbb E$
be an
![]() $\mathrm {Sp}$
-oriented ring spectrum over a regular
$\mathrm {Sp}$
-oriented ring spectrum over a regular
![]() $\mathbb Z[{1}/{2}]$
-scheme S, with Borel classes
$\mathbb Z[{1}/{2}]$
-scheme S, with Borel classes
![]() $b_i$
and ring of coefficients
$b_i$
and ring of coefficients
![]() $\mathbb E^{**}=\mathbb E^{**}(S)$
. Let
$\mathbb E^{**}=\mathbb E^{**}(S)$
. Let
![]() $(n,i) \in \mathbb Z^2$
be a pair of integers.
$(n,i) \in \mathbb Z^2$
be a pair of integers.
-
(1) The following application is a bijection:
where $$ \begin{align*} \big(E^{**}[[t_r,r \geq 1]]^{(n,i)}\big)^{\mathbb Z} & \rightarrow \operatorname{\mathrm{Hom}}_{\mathrm{Sets}}(KSp_0,\mathbb E^{n,i}) \\ (F_r)_{r \in \mathbb Z} & \mapsto \{(\mathfrak V,r) \mapsto F_r\big(b_1(\mathfrak V),\ldots,b_r(\mathfrak V),0,\ldots\big)\}, \end{align*} $$
$$ \begin{align*} \big(E^{**}[[t_r,r \geq 1]]^{(n,i)}\big)^{\mathbb Z} & \rightarrow \operatorname{\mathrm{Hom}}_{\mathrm{Sets}}(KSp_0,\mathbb E^{n,i}) \\ (F_r)_{r \in \mathbb Z} & \mapsto \{(\mathfrak V,r) \mapsto F_r\big(b_1(\mathfrak V),\ldots,b_r(\mathfrak V),0,\ldots\big)\}, \end{align*} $$
 $E^{**}[[t_r,r \geq 1]]^{(n,i)}$
denotes the formal power series with coefficients in the graded ring
$E^{**}[[t_r,r \geq 1]]^{(n,i)}$
denotes the formal power series with coefficients in the graded ring
 $\mathbb E^{**}$
, in the indeterminate
$\mathbb E^{**}$
, in the indeterminate
 $t_r$
, which are homogeneous of degree
$t_r$
, which are homogeneous of degree
 $(n,i)$
, each
$(n,i)$
, each
 $t_r$
being given degree
$t_r$
being given degree
 $(4r,2r)$
;
$(4r,2r)$
;
 $\mathfrak V$
is a symplectic bundle over some X in
$\mathfrak V$
is a symplectic bundle over some X in
 $\mathscr Sm_S$
, and r is its rank.
$\mathscr Sm_S$
, and r is its rank.
-
(2) The following application is an isomorphism of bigraded abelian groups:
where b is an indeterminate whose bidegree is $$ \begin{align*}E^{**}[[b]] \rightarrow \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}(KSp_0,\mathbb E^{*,*}), b^n \mapsto \tilde{\chi}_{2n}:KSp_0 \rightarrow \mathbb E^{4n,2n}, \end{align*} $$
$$ \begin{align*}E^{**}[[b]] \rightarrow \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}(KSp_0,\mathbb E^{*,*}), b^n \mapsto \tilde{\chi}_{2n}:KSp_0 \rightarrow \mathbb E^{4n,2n}, \end{align*} $$
 $(4,2)$
;
$(4,2)$
;
 $\tilde {\chi }_n$
is the natural transformation defined on a symplectic bundle
$\tilde {\chi }_n$
is the natural transformation defined on a symplectic bundle
 $\mathfrak V$
over some X in
$\mathfrak V$
over some X in
 $\mathscr Sm_S$
by the formula; see (4.1.7.a):
$\mathscr Sm_S$
by the formula; see (4.1.7.a): 
As a matter of terminology, we call the morphisms in (1) (respectively (2)) the set (respectively group) of unstable (respectively additive) symplectic operations (or simply
![]() $\mathrm {Sp}$
-operation) on
$\mathrm {Sp}$
-operation) on
![]() $\mathbb E$
of bidegree
$\mathbb E$
of bidegree
![]() $(n,i)$
. The proof follows the strategy designed by Riou, adapted to the
$(n,i)$
. The proof follows the strategy designed by Riou, adapted to the
![]() $\mathrm {Sp}$
-oriented case. We note that some form of the first assertion already appears in the foundational work of Panin and Walter [Reference Panin and Walter26, Th. 11.4].
$\mathrm {Sp}$
-oriented case. We note that some form of the first assertion already appears in the foundational work of Panin and Walter [Reference Panin and Walter26, Th. 11.4].
The next step is to determine stable operations; that is, natural transformations of representable cohomology theories compatible with the stability isomorphism. In contrast with the classical situation, it is more convenient to consider the (pointed) sphere
in the case of
![]() $\mathrm {Sp}$
-oriented ring spectra— this is justified by the
$\mathrm {Sp}$
-oriented ring spectra— this is justified by the
![]() $(8,4)$
-periodicity of the symplectic K-theory spectrum
$(8,4)$
-periodicity of the symplectic K-theory spectrum
![]() $\mathbf {KSp}$
. In this setting and along classical lines, the stability class associated with any ring spectra
$\mathbf {KSp}$
. In this setting and along classical lines, the stability class associated with any ring spectra
![]() $\mathbb E$
over a base scheme S is the structural class
$\mathbb E$
over a base scheme S is the structural class
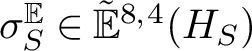 $\sigma ^{\mathbb E}_S \in \tilde {\mathbb E}^{8,4}(H_S)$
in reduced cohomology such that for any smooth S-scheme X, the following exterior product map is an isomorphism:
$\sigma ^{\mathbb E}_S \in \tilde {\mathbb E}^{8,4}(H_S)$
in reduced cohomology such that for any smooth S-scheme X, the following exterior product map is an isomorphism:
 $$ \begin{align*}\tilde{\mathbb E}^{n,i}(X_+) \xrightarrow{\gamma^{\mathbb E}_\sigma} \tilde{\mathbb E}^{n+8,i+4}(H_S \wedge X_+). \end{align*} $$
$$ \begin{align*}\tilde{\mathbb E}^{n,i}(X_+) \xrightarrow{\gamma^{\mathbb E}_\sigma} \tilde{\mathbb E}^{n+8,i+4}(H_S \wedge X_+). \end{align*} $$
When
![]() $\mathbb E$
is
$\mathbb E$
is
![]() $\mathrm {Sp}$
-oriented, one has
$\mathrm {Sp}$
-oriented, one has
![]() $\sigma ^{\mathbb E}_S=b_1(\mathfrak U_1).b_1(\mathfrak U_2)$
, where
$\sigma ^{\mathbb E}_S=b_1(\mathfrak U_1).b_1(\mathfrak U_2)$
, where
![]() $\mathfrak U_i$
is the tautological symplectic bundle on the i-factor of
$\mathfrak U_i$
is the tautological symplectic bundle on the i-factor of
![]() $H_S$
. Then given an additive
$H_S$
. Then given an additive
![]() $\mathrm {Sp}$
-operation
$\mathrm {Sp}$
-operation
![]() $\theta :\mathbf {KSp}_0 \rightarrow \mathbb E^{n,i}$
as above, we define an associated ‘desuspended’
$\theta :\mathbf {KSp}_0 \rightarrow \mathbb E^{n,i}$
as above, we define an associated ‘desuspended’
![]() $\mathrm {Sp}$
-operation
$\mathrm {Sp}$
-operation
![]() $\omega _H(\theta )$
on
$\omega _H(\theta )$
on
![]() $\mathbb E$
of degree
$\mathbb E$
of degree
![]() $(n-8,i-4)$
by the following commutative diagram:
$(n-8,i-4)$
by the following commutative diagram:

Then a stable
![]() $\mathrm {Sp}$
-operation is nothing but a sequence
$\mathrm {Sp}$
-operation is nothing but a sequence
![]() $(\Theta _n)_{n \geq 0}$
of unstable (necessarily additive)
$(\Theta _n)_{n \geq 0}$
of unstable (necessarily additive)
![]() $\mathrm {Sp}$
-operations such that
$\mathrm {Sp}$
-operations such that
![]() $\Theta _n=\omega _H(\Theta _{n+1})$
. Our next result is the computation of the desuspension of every
$\Theta _n=\omega _H(\Theta _{n+1})$
. Our next result is the computation of the desuspension of every
![]() $\mathrm {Sp}$
-operations on MW-motivic cohomology.
$\mathrm {Sp}$
-operations on MW-motivic cohomology.
Theorem C See Theorem 4.3.2
Let R be a ring of coefficients and S a scheme such that one of the following conditions hold:
-
(a)
 $R=\mathbb Z$
, S is a scheme over a perfect field k of characteristic
$R=\mathbb Z$
, S is a scheme over a perfect field k of characteristic
 $\neq 2,3$
.
$\neq 2,3$
. -
(b)
 $R=\mathbb Q$
, S is a
$R=\mathbb Q$
, S is a
 $\mathbb Z[{1}/{2}]$
-scheme.
$\mathbb Z[{1}/{2}]$
-scheme.
Then for any integer
![]() $n \geq 0$
, the following relation holds:
$n \geq 0$
, the following relation holds:
 $$ \begin{align*}\omega_H\left(\tilde{\chi}_{2n+4}^R\right)=\psi_{2n+4}.\tilde{\chi}_{2n}^R, \end{align*} $$
$$ \begin{align*}\omega_H\left(\tilde{\chi}_{2n+4}^R\right)=\psi_{2n+4}.\tilde{\chi}_{2n}^R, \end{align*} $$
where
![]() $\tilde {\chi }_{2n}^R$
is the additive
$\tilde {\chi }_{2n}^R$
is the additive
![]() $\mathrm {Sp}$
-operation on
$\mathrm {Sp}$
-operation on
![]() $\mathbf {H}_{\mathrm {MW}}$
of degree
$\mathbf {H}_{\mathrm {MW}}$
of degree
![]() $(8n,4n)$
obtained in Theorem B and
$(8n,4n)$
obtained in Theorem B and
![]() $\psi _{2n+4}$
is the image in
$\psi _{2n+4}$
is the image in
![]() $\mathbf {H}_{\mathrm {MW}}^{00}(S)$
of the following quadratic form (seen in
$\mathbf {H}_{\mathrm {MW}}^{00}(S)$
of the following quadratic form (seen in
![]() $\operatorname {\mathrm {GW}}(\mathbb Z)$
):
$\operatorname {\mathrm {GW}}(\mathbb Z)$
):
 $$ \begin{align*}\psi_{2n+4}=\begin{cases} \frac 12(2n+4)(2n+3)(2n+2)(2n+1).h & \text{if }n \text{ is even.} \\ (2n+4)(2n+2).\big((2n^2+4n+1).h-\epsilon\big) & \text{if }n \text{ is odd.} \\ \end{cases} \end{align*} $$
$$ \begin{align*}\psi_{2n+4}=\begin{cases} \frac 12(2n+4)(2n+3)(2n+2)(2n+1).h & \text{if }n \text{ is even.} \\ (2n+4)(2n+2).\big((2n^2+4n+1).h-\epsilon\big) & \text{if }n \text{ is odd.} \\ \end{cases} \end{align*} $$
Note in particular that
![]() $\operatorname {\mathrm {rk}}(\psi _{2n+4})=(2n+4)(2n+3)(2n+2)(2n+1)$
; consequently, this result is coherent with the one obtained by Riou (in [Reference Riou30]).Footnote 6 The main ingredient of this computation is Theorem A.
$\operatorname {\mathrm {rk}}(\psi _{2n+4})=(2n+4)(2n+3)(2n+2)(2n+1)$
; consequently, this result is coherent with the one obtained by Riou (in [Reference Riou30]).Footnote 6 The main ingredient of this computation is Theorem A.
As a corollary, we obtain the computation of all stable
![]() $\mathrm {Sp}$
-operations on rational MW-motivic cohomology and ultimately deduce the announced construction of the Borel character:
$\mathrm {Sp}$
-operations on rational MW-motivic cohomology and ultimately deduce the announced construction of the Borel character:
Theorem D See Theorem 4.4.6, 5.5.1, Paragraph 5.1.4
Denote by
![]() $[-,-]$
maps in
$[-,-]$
maps in
![]() $\operatorname {\mathrm {SH}}(S)$
.
$\operatorname {\mathrm {SH}}(S)$
.
-
(1) Let S be the spectrum of
 $\mathbb Z[{1}/{2}]$
or of a field of characteristic not
$\mathbb Z[{1}/{2}]$
or of a field of characteristic not
 $2$
. Then for any integer
$2$
. Then for any integer
 $n \in \mathbb Z$
, one has canonical isomorphisms where the left-hand side denotes the stable symplectic operations on rational MW-motivic cohomology (whose
$n \in \mathbb Z$
, one has canonical isomorphisms where the left-hand side denotes the stable symplectic operations on rational MW-motivic cohomology (whose $$ \begin{align*} \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\widetilde{\mathrm{CH}}{}^{2n}_{\mathbb Q}\big) \simeq & \big[\mathbf{KSp}_S,\mathbf{H}_{\mathrm{MW}} {\mathbb Q}_S(2n)[4n]\big] \\ & \simeq \begin{cases} \big[\mathbf{KSp}_S,\mathbf{H}_{\mathrm M} {\mathbb Q}_S(2n)[4n]\big] \simeq \mathbb Q & n=2i, \\ \operatorname{\mathrm{GW}}(S)_{\mathbb Q}=\mathbb Q\oplus \operatorname{\mathrm{W}}(S)_{\mathbb Q} & n=2i+1, \end{cases} \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\widetilde{\mathrm{CH}}{}^{2n}_{\mathbb Q}\big) \simeq & \big[\mathbf{KSp}_S,\mathbf{H}_{\mathrm{MW}} {\mathbb Q}_S(2n)[4n]\big] \\ & \simeq \begin{cases} \big[\mathbf{KSp}_S,\mathbf{H}_{\mathrm M} {\mathbb Q}_S(2n)[4n]\big] \simeq \mathbb Q & n=2i, \\ \operatorname{\mathrm{GW}}(S)_{\mathbb Q}=\mathbb Q\oplus \operatorname{\mathrm{W}}(S)_{\mathbb Q} & n=2i+1, \end{cases} \end{align*} $$
 $(4n,2n)$
part is given by rational Chow-Witt groups
$(4n,2n)$
part is given by rational Chow-Witt groups
 $\widetilde {\mathrm {CH}}{}^{2n}_{\mathbb Q}$
).
$\widetilde {\mathrm {CH}}{}^{2n}_{\mathbb Q}$
).
-
(2) Let S be a
 $\mathbb Z[{1}/{2}]$
-scheme. Define the Borel character as the following map: where
$\mathbb Z[{1}/{2}]$
-scheme. Define the Borel character as the following map: where $$ \begin{align*} \operatorname{\mathrm{bo}}_t:\mathbf{GW}^{\mathbb Q}_{S} \xrightarrow{\ (\operatorname{\mathrm{bo}}_{2n})_{n \in \mathbb Z}\ } & \bigoplus_{n \text{ even}} \mathbf{H}_{\mathrm{MW}} {\mathbb Q}_S(2n)[4n] \oplus \bigoplus_{n \text{ odd}} \mathbf{H}_{\mathrm M} {\mathbb Q}_S(2n)[4n], \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{bo}}_t:\mathbf{GW}^{\mathbb Q}_{S} \xrightarrow{\ (\operatorname{\mathrm{bo}}_{2n})_{n \in \mathbb Z}\ } & \bigoplus_{n \text{ even}} \mathbf{H}_{\mathrm{MW}} {\mathbb Q}_S(2n)[4n] \oplus \bigoplus_{n \text{ odd}} \mathbf{H}_{\mathrm M} {\mathbb Q}_S(2n)[4n], \end{align*} $$
 $\operatorname {\mathrm {bo}}_{2n}$
is the stable operation corresponding to the unit in
$\operatorname {\mathrm {bo}}_{2n}$
is the stable operation corresponding to the unit in $$ \begin{align*}\mathbf{GW}_S \simeq \mathbf{KSp}_S(-2)[-4] \rightarrow \mathbf{H}_{\mathrm M} \mathbb Q_S(2n)[4n] \end{align*} $$
$$ \begin{align*}\mathbf{GW}_S \simeq \mathbf{KSp}_S(-2)[-4] \rightarrow \mathbf{H}_{\mathrm M} \mathbb Q_S(2n)[4n] \end{align*} $$
 $\mathbb Q$
(respectively
$\mathbb Q$
(respectively
 $\operatorname {\mathrm {GW}}(S)_{\mathbb Q}$
) under the above isomorphism if n is odd (respectively even) when
$\operatorname {\mathrm {GW}}(S)_{\mathbb Q}$
) under the above isomorphism if n is odd (respectively even) when
 $S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
and obtained by pullback in general. Then
$S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
and obtained by pullback in general. Then
 $\operatorname {\mathrm {bo}}$
is an isomorphism of ring spectra and the following diagram commutes:
$\operatorname {\mathrm {bo}}$
is an isomorphism of ring spectra and the following diagram commutes: 
The right vertical map is obtained by forgetting the Witt part in degrees 0 modulo 4, and the left-hand vertical map is the forgetful functor. In fact, the Borel character is the sum of the Chern character in even degrees and a Witt part, concentrated in degrees 0 modulo 4. Moreover, note that from the results of [Reference Déglise, Fasel, Khan and Jin15], the Witt part is only visible on the characteristic zero part of the scheme S. This is because it exists, as a morphism of ring spectra, only modulo torsion.
To conclude this introduction, let us mention that even though the Borel character is a stable and rational phenomenon, the methods and results of this article apply more generally to unstable and integral situations. Indeed, we note that for a given scheme X, one needs only to invert finitely many quadratic forms
![]() $\psi _{2n+4}$
appearing in Theorem C in order to get the Borel character. Presumably, this yields finer results than simply tensoring with
$\psi _{2n+4}$
appearing in Theorem C in order to get the Borel character. Presumably, this yields finer results than simply tensoring with
![]() $\mathbb Q$
. This is somewhat illustrated by the following theorem, which shows that Milnor-Witt K-theory embeds into suitable Hermitian K-theory groups modulo torsion.
$\mathbb Q$
. This is somewhat illustrated by the following theorem, which shows that Milnor-Witt K-theory embeds into suitable Hermitian K-theory groups modulo torsion.
Theorem E see Theorem 6.1.1
For any
![]() $n\geq 2$
and any finitely generated field extension of k, the composite
$n\geq 2$
and any finitely generated field extension of k, the composite
 $$ \begin{align*} \mathrm{K}^{\mathrm{MW}}_n(L) \xrightarrow{\varepsilon_{n,n}} \operatorname{\mathrm{GW}}_n^n(L) \xrightarrow{\tilde{\chi}_{n,n}} \mathrm{K}^{\mathrm{MW}}_n(L) \end{align*} $$
$$ \begin{align*} \mathrm{K}^{\mathrm{MW}}_n(L) \xrightarrow{\varepsilon_{n,n}} \operatorname{\mathrm{GW}}_n^n(L) \xrightarrow{\tilde{\chi}_{n,n}} \mathrm{K}^{\mathrm{MW}}_n(L) \end{align*} $$
is multiplication by
![]() $\psi _{\mu (n)}! \in \operatorname {\mathrm {GW}}(k)$
, where
$\psi _{\mu (n)}! \in \operatorname {\mathrm {GW}}(k)$
, where
![]() $\mu (n)$
is the smallest integer of the form
$\mu (n)$
is the smallest integer of the form
![]() $2+4r$
greater than or equal to n and we put
$2+4r$
greater than or equal to n and we put
This result can be seen as a generalisation of a theorem of Suslin ([Reference Suslin37]), stating that Milnor K-theory embeds into (Quillen) K-theory modulo torsion.
Linked and further works
Our result on computing the ternary laws of MW-motivic cohomology owes much to the reading of [Reference Levine20], as the reader will see in the text. The results of Ananyevskiy’s thesis, published in [Reference Ananyevskiy2], are especially linked to the results obtained here. Indeed, Ananyevskiy computes the ternary laws (without the abstract theory explained here) associated with higher Witt groups in Lemma 8.2 of [Reference Ananyevskiy2] and build the minus part of our Borel character in Theorem 1.1 of [Reference Ananyevskiy2].
We plan to come back to explicit computations of the Borel character in future work, in collaboration with Fangzhou Jin and Adeel Khan. In particular, we will study the natural question that arises with the analogy between the Chern and Borel characters: finding the quadratic analogue of the Grothendieck-Riemann-Roch formula. We also plan to compute the ternary laws associated to some well-known cohomology theories, such as higher Grothendieck-Witt groups.
Plan
In Section 2, we recall Panin and Walter’s theory of generalised orientations and Borel classes and introduce Walter’s notion of ternary laws.
In Section 3, we compute the ternary laws associated with MW-motivic cohomology, as explained in Theorem A. The proof reduces to compute the relevant Borel classes either in Chow groups or in Witt-cohomology. The last part is the core of the proof and occupies Subsection 3.2, complemented with Appendix A containing an ‘elementary’ computation of some threefold tensor product of symplectic bundles that is central in our computations.
Section 4 is devoted to the implementation of Riou’s method for determining cohomological operations in the symplectic case. The first subsection is devoted to proving Theorem B. The second subsection gives some abstract considerations to determine stable
![]() $\mathrm {Sp}$
-operations in the general case. The core of the section is the third subsection, which computes the obstruction to stabilisation for MW-motivic cohomology, as explained in Theorem C.
$\mathrm {Sp}$
-operations in the general case. The core of the section is the third subsection, which computes the obstruction to stabilisation for MW-motivic cohomology, as explained in Theorem C.
In Section 5, we define the Borel character (Definition 5.1.3) and prove that it is an isomorphism of ring spectra. On the model of the proof of Theorem A, we treat the plus part and minus part separately. The plus part can be reduced to the classical case of the Chern character, and the minus part can be treated using properties of periodic ring spectra as recalled in Subsection 5.2 and the ideas of [Reference Ananyevskiy, Levine and Panin4] suitably extended to arbitrary base
![]() $\mathbb Z[{1}/{2}]$
-schemes.
$\mathbb Z[{1}/{2}]$
-schemes.
Subsection 6.1 contains the proof and statement of Theorem E, based on ideas of [Reference Asok and Fasel6].
1 Notation and conventions
We will fix a quasi-compact and quasi-separated base scheme B, which in practice will be the spectrum of either a perfect field denoted by k, the ring
![]() $\mathbb Z[{1}/{2}]$
or
$\mathbb Z[{1}/{2}]$
or
![]() $\mathbb Z$
. We work with the category
$\mathbb Z$
. We work with the category
![]() $\mathscr Sch_B$
of quasi-compact and quasi-separated B-schemes; all schemes are supposed to be in
$\mathscr Sch_B$
of quasi-compact and quasi-separated B-schemes; all schemes are supposed to be in
![]() $\mathscr Sch_B$
. For certain results, we will also restrict our attention to regular finite-dimensional B-schemes, and we denote by
$\mathscr Sch_B$
. For certain results, we will also restrict our attention to regular finite-dimensional B-schemes, and we denote by
![]() $\mathscr Reg_B$
the corresponding category.
$\mathscr Reg_B$
the corresponding category.
Unless explicitly stated, we will consider (ring) spectra
![]() $\; \mathbb E$
over B and look at them as absolute (ring) spectra over
$\; \mathbb E$
over B and look at them as absolute (ring) spectra over
![]() $\mathscr Sch_B$
by putting
$\mathscr Sch_B$
by putting
![]() $\mathbb E_X=f^*\mathbb E$
for any
$\mathbb E_X=f^*\mathbb E$
for any
![]() $f:X \rightarrow B$
. Here are the examples that will appear in the present article:
$f:X \rightarrow B$
. Here are the examples that will appear in the present article:
![]() $\underline{\text{The case }B=\operatorname {\mathrm {Spec}}(\mathbb Z)}$
: the absolute ring spectra
$\underline{\text{The case }B=\operatorname {\mathrm {Spec}}(\mathbb Z)}$
: the absolute ring spectra
![]() $\mathbf {H}_{\mathrm M} \mathbb Z$
(respectively
$\mathbf {H}_{\mathrm M} \mathbb Z$
(respectively
![]() $\mathbf {H}_{\mathrm M} \mathbb Q$
,
$\mathbf {H}_{\mathrm M} \mathbb Q$
,
![]() $\mathbf {KGL}$
), representing integral motivic cohomology (respectively rational motivic cohomology, homotopy-invariant K-theory; see [Reference Spitzweck36], respectively [Reference Cisinski and Déglise11], [Reference Cisinski10]).
$\mathbf {KGL}$
), representing integral motivic cohomology (respectively rational motivic cohomology, homotopy-invariant K-theory; see [Reference Spitzweck36], respectively [Reference Cisinski and Déglise11], [Reference Cisinski10]).
We will also define the rational Milnor-Witt motivic ring spectrum as
where
![]() $\mathbb S$
is the motivic sphere spectrum. We refer the reader to [Reference Déglise, Fasel, Khan and Jin15, Def. 6.1] for a better definition. It coincides with the above one according to Corollary 6.2 of op. cit. Note also that according to [Reference Déglise, Fasel, Khan and Jin15, Cor. 8.9], one has for any regular scheme S
$\mathbb S$
is the motivic sphere spectrum. We refer the reader to [Reference Déglise, Fasel, Khan and Jin15, Def. 6.1] for a better definition. It coincides with the above one according to Corollary 6.2 of op. cit. Note also that according to [Reference Déglise, Fasel, Khan and Jin15, Cor. 8.9], one has for any regular scheme S
 $$ \begin{align} \mathbf{H}_{\mathrm{MW}}^{2n,n}(S,\mathbb Q)\simeq \widetilde{\mathrm{CH}}{}^{n}(X)_{\mathbb Q}=\operatorname{\mathrm{CH}}^n(X)_{\mathbb Q} \oplus H^n_{\mathrm{Zar}}(S_{\mathbb Q},\operatorname{{\underline W}}_{\mathbb Q}), \end{align} $$
$$ \begin{align} \mathbf{H}_{\mathrm{MW}}^{2n,n}(S,\mathbb Q)\simeq \widetilde{\mathrm{CH}}{}^{n}(X)_{\mathbb Q}=\operatorname{\mathrm{CH}}^n(X)_{\mathbb Q} \oplus H^n_{\mathrm{Zar}}(S_{\mathbb Q},\operatorname{{\underline W}}_{\mathbb Q}), \end{align} $$
where
![]() $\operatorname {{\underline W}}_{\mathbb Q}$
is the Zariski sheaf over
$\operatorname {{\underline W}}_{\mathbb Q}$
is the Zariski sheaf over
![]() $S_{\mathbb Q}$
associated to the Witt functor.
$S_{\mathbb Q}$
associated to the Witt functor.
![]() $\underline{\text{The case } B=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])}$
: the absolute ring spectra
$\underline{\text{The case } B=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])}$
: the absolute ring spectra
![]() $\mathbf {GW}$
(respectively
$\mathbf {GW}$
(respectively
![]() $\mathbf {W}$
), representing higher Grothendieck-Witt groups [Reference Schlichting33, §9] (also called Hermitian K-theory, (respectively Balmer’s derived Witt groups) over regular schemes.
$\mathbf {W}$
), representing higher Grothendieck-Witt groups [Reference Schlichting33, §9] (also called Hermitian K-theory, (respectively Balmer’s derived Witt groups) over regular schemes.
For the definition of
![]() $\mathbf {GW}$
we refer the reader to [Reference Panin and Walter28] and [Reference Schlichting and Tripathi34].Footnote 7 To fix our conventions, let us recall that for a regular scheme S:
$\mathbf {GW}$
we refer the reader to [Reference Panin and Walter28] and [Reference Schlichting and Tripathi34].Footnote 7 To fix our conventions, let us recall that for a regular scheme S:
 $$ \begin{align} \mathbf{GW}^{n,i}(S)=GW_{2i-n}^i(S)=\begin{cases} KO_{2i-n}(S) & i \cong 0 \mod 4, \\ KSp_{2i-n}(S) & i \cong 2 \mod 4, \end{cases} \end{align} $$
$$ \begin{align} \mathbf{GW}^{n,i}(S)=GW_{2i-n}^i(S)=\begin{cases} KO_{2i-n}(S) & i \cong 0 \mod 4, \\ KSp_{2i-n}(S) & i \cong 2 \mod 4, \end{cases} \end{align} $$
where
![]() $KO_*$
(respectively
$KO_*$
(respectively
![]() $KSp_*$
) denotes the higher Hermitian K-theory of orthogonal bundles (respectively symplectic bundles) with the canonical duality (see again [Reference Schlichting33]). For the definition of
$KSp_*$
) denotes the higher Hermitian K-theory of orthogonal bundles (respectively symplectic bundles) with the canonical duality (see again [Reference Schlichting33]). For the definition of
![]() $\mathbf {W}$
, we refer to [Reference Ananyevskiy, Levine and Panin4, Def. 3]:
$\mathbf {W}$
, we refer to [Reference Ananyevskiy, Levine and Panin4, Def. 3]:
![]() $\mathbf {W}=\mathbf {GW}[\eta ^{-1}]$
where
$\mathbf {W}=\mathbf {GW}[\eta ^{-1}]$
where
![]() $\eta $
is the (algebraic) Hopf map.
$\eta $
is the (algebraic) Hopf map.
![]() $\underline{\text{The case } B=\operatorname {\mathrm {Spec}}(k),\ k\ \text{perfect field of characteristic not } 2} $
: the absolute ring spectrum
$\underline{\text{The case } B=\operatorname {\mathrm {Spec}}(k),\ k\ \text{perfect field of characteristic not } 2} $
: the absolute ring spectrum
![]() $\mathbf {H}_{\mathrm {MW}} \mathbb Z$
representing integral Milnor-Witt cohomology as defined in [Reference Déglise and Fasel14]. We will also consider
$\mathbf {H}_{\mathrm {MW}} \mathbb Z$
representing integral Milnor-Witt cohomology as defined in [Reference Déglise and Fasel14]. We will also consider
![]() ${\mathbf H}\, \underline {\mathrm {K}}^{\mathrm {MW}}$
(respectively
${\mathbf H}\, \underline {\mathrm {K}}^{\mathrm {MW}}$
(respectively
![]() ${\mathbf H} \operatorname {{\underline W}}$
) the spectrum associated with the unramified Milnor-Witt K-theory (respectively Witt theory), which represents Chow-Witt groups (respectively unramified Witt cohomology) according to [Reference Morel25]. In particular, we have for any smooth k-scheme S:
${\mathbf H} \operatorname {{\underline W}}$
) the spectrum associated with the unramified Milnor-Witt K-theory (respectively Witt theory), which represents Chow-Witt groups (respectively unramified Witt cohomology) according to [Reference Morel25]. In particular, we have for any smooth k-scheme S:
 $$ \begin{align} \mathbf{H}_{\mathrm{MW}}^{2n,n}(S) \simeq H^n_{\mathrm{Zar}}(S,\underline{\mathrm{K}}^{\mathrm{MW}}_n) \simeq \widetilde{\mathrm{CH}}{}^n(S). \end{align} $$
$$ \begin{align} \mathbf{H}_{\mathrm{MW}}^{2n,n}(S) \simeq H^n_{\mathrm{Zar}}(S,\underline{\mathrm{K}}^{\mathrm{MW}}_n) \simeq \widetilde{\mathrm{CH}}{}^n(S). \end{align} $$
Finally, we will use Morel’s plus/minus decomposition of the
![]() $\mathbb Z[{1}/{2}]$
-linear stable homotopy category (see, e.g., [Reference Cisinski and Déglise11, Paragraphe 16.2.1.] or [Reference Ananyevskiy, Levine and Panin4, Rem. 4]). Recall in particular the identifications (see [Reference Cisinski and Déglise11, Theorem 16.2.13] for the second one)
$\mathbb Z[{1}/{2}]$
-linear stable homotopy category (see, e.g., [Reference Cisinski and Déglise11, Paragraphe 16.2.1.] or [Reference Ananyevskiy, Levine and Panin4, Rem. 4]). Recall in particular the identifications (see [Reference Cisinski and Déglise11, Theorem 16.2.13] for the second one)
 $$ \begin{align} \begin{aligned} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S&=\mathbb S_{S,\mathbb Q+} \oplus \mathbb S_{S,\mathbb Q-} \\ \mathbf{H}_{\mathrm M} \mathbb Q_S & \simeq \mathbb S_{S,\mathbb Q+}. \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S&=\mathbb S_{S,\mathbb Q+} \oplus \mathbb S_{S,\mathbb Q-} \\ \mathbf{H}_{\mathrm M} \mathbb Q_S & \simeq \mathbb S_{S,\mathbb Q+}. \end{aligned} \end{align} $$
2 Weak orientations
2.1 Definitions and basic properties
2.1.1. Given a vector bundle V over a scheme X, we let
![]() $\operatorname {\mathrm {Th}}(V)=V/V^\times $
be its Thom space in
$\operatorname {\mathrm {Th}}(V)=V/V^\times $
be its Thom space in
![]() $\operatorname {\mathrm {SH}}(X)$
. Given a spectrum
$\operatorname {\mathrm {SH}}(X)$
. Given a spectrum
![]() $\mathbb E$
and integers
$\mathbb E$
and integers
![]() $(n,i)\in \mathbb Z^2$
, we put as usual:
$(n,i)\in \mathbb Z^2$
, we put as usual:
 $$ \begin{align*}\mathbb E^{n,i}\big(\operatorname{\mathrm{Th}}(V)\big)=\operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(X)}(\operatorname{\mathrm{Th}}(V),\mathbb E_X(i)[n]). \end{align*} $$
$$ \begin{align*}\mathbb E^{n,i}\big(\operatorname{\mathrm{Th}}(V)\big)=\operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(X)}(\operatorname{\mathrm{Th}}(V),\mathbb E_X(i)[n]). \end{align*} $$
Recall that the Thom space functor is a monoidal functor with respect to direct sums of vector bundles and tensor products of spectra:
![]() $\operatorname {\mathrm {Th}}(E \oplus F) \simeq \operatorname {\mathrm {Th}}(E) \otimes \operatorname {\mathrm {Th}}(F)$
. In particular, tensor products of morphisms induce an exterior product
$\operatorname {\mathrm {Th}}(E \oplus F) \simeq \operatorname {\mathrm {Th}}(E) \otimes \operatorname {\mathrm {Th}}(F)$
. In particular, tensor products of morphisms induce an exterior product
 $$ \begin{align*}\mathbb E^{n,i}\big(\operatorname{\mathrm{Th}}(E)\big) \otimes \mathbb E^{m,j}\big(\operatorname{\mathrm{Th}}(F)\big) \rightarrow \mathbb E^{m+n,i+j}\big(\operatorname{\mathrm{Th}}(E \oplus F)\big), x \otimes y \mapsto x \cdot y. \end{align*} $$
$$ \begin{align*}\mathbb E^{n,i}\big(\operatorname{\mathrm{Th}}(E)\big) \otimes \mathbb E^{m,j}\big(\operatorname{\mathrm{Th}}(F)\big) \rightarrow \mathbb E^{m+n,i+j}\big(\operatorname{\mathrm{Th}}(E \oplus F)\big), x \otimes y \mapsto x \cdot y. \end{align*} $$
2.1.2. We will consider the following
![]() $\mathbb Z$
-graded algebraic subgroups of
$\mathbb Z$
-graded algebraic subgroups of
![]() $\mathrm {GL}_*$
:
$\mathrm {GL}_*$
:
The nth graded part of
![]() $\mathrm {Sp}_*$
is
$\mathrm {Sp}_*$
is
![]() $\mathrm {Sp}_{2n}$
so that the first map is homogeneous of degree
$\mathrm {Sp}_{2n}$
so that the first map is homogeneous of degree
![]() $2$
. All other maps are homogeneous of degree
$2$
. All other maps are homogeneous of degree
![]() $1$
. They are all classical algebraic groups except
$1$
. They are all classical algebraic groups except
![]() $\mathrm {SL}^c_*$
, which was introduced in [Reference Panin and Walter28, Definition 3.3]. Recall that its nth graded part is definedFootnote 8 as the kernel of the map
$\mathrm {SL}^c_*$
, which was introduced in [Reference Panin and Walter28, Definition 3.3]. Recall that its nth graded part is definedFootnote 8 as the kernel of the map
Letting
![]() $G=G_*$
be one of these groups, there is a classical correspondence between G-torsors over a given scheme X and vector bundles E over X equipped with extra structures. We summarise the situation in the following table:
$G=G_*$
be one of these groups, there is a classical correspondence between G-torsors over a given scheme X and vector bundles E over X equipped with extra structures. We summarise the situation in the following table:

Recall that our symplectic forms are always assumed to be nondegenerate. For short, we will say G-bundle for a bundle equipped with the corresponding extra structure. Morphisms of G-bundles over X are morphisms of vector bundles over X that preserve the extra structure in the obvious sense.
One can check that the category
![]() ${\mathscr V}(X,G)$
of G-bundles over X is additive. Let
${\mathscr V}(X,G)$
of G-bundles over X is additive. Let
![]() $r=2$
(respectively
$r=2$
(respectively
![]() $r=1$
) when
$r=1$
) when
![]() $G=\mathrm {Sp}_*$
(respectively in the other cases). The constant bundle of rank r admits a canonical G-bundle structure and the corresponding object is denoted by
$G=\mathrm {Sp}_*$
(respectively in the other cases). The constant bundle of rank r admits a canonical G-bundle structure and the corresponding object is denoted by
. In particular, given a (ring) spectrum
![]() $\mathbb E$
over X, one gets an isomorphism
$\mathbb E$
over X, one gets an isomorphism

The following definition is a slight extension of the original one due to Panin and Walter (our reference text will be [Reference Ananyevskiy3]).
Definition 2.1.3. Consider the above notations. Let
![]() $\mathbb E$
be a ring spectrum over B, with unit
$\mathbb E$
be a ring spectrum over B, with unit
![]() $1_X$
over a B-scheme X. An absolute G-orientation
$1_X$
over a B-scheme X. An absolute G-orientation
![]() $\mathfrak t$
of
$\mathfrak t$
of
![]() $\mathbb E$
is the data for every G-bundle
$\mathbb E$
is the data for every G-bundle
![]() $\mathfrak V$
of rank r over a scheme X in
$\mathfrak V$
of rank r over a scheme X in
![]() $\mathscr Sch_B$
of a class
$\mathscr Sch_B$
of a class
![]() $\mathfrak t(\mathfrak V) \in \mathbb E^{2r,r}(\operatorname {\mathrm {Th}}(\mathfrak V))$
satisfying the following properties:
$\mathfrak t(\mathfrak V) \in \mathbb E^{2r,r}(\operatorname {\mathrm {Th}}(\mathfrak V))$
satisfying the following properties:
-
• Isomorphisms compatibility.
 $\phi ^*\mathfrak t(\mathfrak W)=\mathfrak t(\mathfrak V)$
for
$\phi ^*\mathfrak t(\mathfrak W)=\mathfrak t(\mathfrak V)$
for
 $\phi :\mathfrak V \xrightarrow \sim \mathfrak W$
.
$\phi :\mathfrak V \xrightarrow \sim \mathfrak W$
. -
• Pullbacks compatibility.
 $f^*\mathfrak t(\mathfrak V)=\mathfrak t(f^{-1}\mathfrak V)$
for
$f^*\mathfrak t(\mathfrak V)=\mathfrak t(f^{-1}\mathfrak V)$
for
 $f:Y \rightarrow X$
in
$f:Y \rightarrow X$
in
 $\mathscr Sch_B$
.
$\mathscr Sch_B$
. -
• Products compatibility.
 $\mathfrak t(\mathfrak V \oplus \mathfrak W)=\mathfrak t(\mathfrak V) \cdot \mathfrak t(\mathfrak W)$
.
$\mathfrak t(\mathfrak V \oplus \mathfrak W)=\mathfrak t(\mathfrak V) \cdot \mathfrak t(\mathfrak W)$
. -
• Normalisation.
 corresponds to
corresponds to
 $1_X$
via the isomorphism
$1_X$
via the isomorphism
 $\sigma $
.
$\sigma $
.
In this situation, we also say that
![]() $(\mathbb E,\mathfrak t)$
is absolutely G-oriented.
$(\mathbb E,\mathfrak t)$
is absolutely G-oriented.
2.1.4.
Thom isomorphisms. This definition is tailored to generalise the classical notion of orientation in motivic homotopy theory, which corresponds in the above term to a
![]() $\mathrm {GL}$
-orientation.
$\mathrm {GL}$
-orientation.
More generally, under the assumptions of the above definition, given any G-bundle
![]() $\mathfrak V$
of rank r over X, the properties stated above imply that
$\mathfrak V$
of rank r over X, the properties stated above imply that
![]() $\mathbb E^{**}(\operatorname {\mathrm {Th}}(\mathfrak V))$
is a free rank 1 bigraded
$\mathbb E^{**}(\operatorname {\mathrm {Th}}(\mathfrak V))$
is a free rank 1 bigraded
![]() $\mathbb E^{**}(X)$
-module with base
$\mathbb E^{**}(X)$
-module with base
![]() $\mathfrak t(\mathfrak V)$
(see [Reference Ananyevskiy3, Corollary 3.9]).
$\mathfrak t(\mathfrak V)$
(see [Reference Ananyevskiy3, Corollary 3.9]).
In other words, the cup product with the Thom class induces a Thom isomorphism:
as in the classical oriented case (see, e.g., [Reference Déglise12, §2.2]).
Remark 2.1.5. When
![]() $\mathscr Sch_B$
is the category of smooth B-schemes of finite type, we will simply say that
$\mathscr Sch_B$
is the category of smooth B-schemes of finite type, we will simply say that
![]() $\mathbb E$
is G-oriented, and
$\mathbb E$
is G-oriented, and
![]() $\mathfrak t$
is a G-orientation. This is the original definition of [Reference Panin and Walter28] and [Reference Ananyevskiy3].
$\mathfrak t$
is a G-orientation. This is the original definition of [Reference Panin and Walter28] and [Reference Ananyevskiy3].
In case
![]() $G=GL_*$
, a G-orientation uniquely determines an absolute G-orientation. Indeed, according to [Reference Déglise12], a G-orientation over a scheme B is determined by a class
$G=GL_*$
, a G-orientation uniquely determines an absolute G-orientation. Indeed, according to [Reference Déglise12], a G-orientation over a scheme B is determined by a class
 $c \in \tilde {\mathbb E}^{2,1}(\mathbb P^\infty _B)$
whose restriction to
$c \in \tilde {\mathbb E}^{2,1}(\mathbb P^\infty _B)$
whose restriction to
![]() $\mathbb P^1_B$
is the suspension of
$\mathbb P^1_B$
is the suspension of
![]() $1_B$
. Thus, a G-orientation of
$1_B$
. Thus, a G-orientation of
![]() $\mathbb E$
over B induces by pullback a G-orientation over any B-scheme X and therefore an absolute G-orientation.
$\mathbb E$
over B induces by pullback a G-orientation over any B-scheme X and therefore an absolute G-orientation.
A similar remark holds when
![]() $G=\mathrm {Sp}_*$
. Indeed, one can show that a G-orientation over a scheme B is determined by a class
$G=\mathrm {Sp}_*$
. Indeed, one can show that a G-orientation over a scheme B is determined by a class
 $b \in \tilde {\mathbb E}^{4,2}(\mathrm {H}\mathbb {P}^\infty _B)$
whose restriction to
$b \in \tilde {\mathbb E}^{4,2}(\mathrm {H}\mathbb {P}^\infty _B)$
whose restriction to
![]() $\mathrm {H}\mathbb {P}^1_B$
is the suspension of
$\mathrm {H}\mathbb {P}^1_B$
is the suspension of
![]() $1_B$
; see [Reference Panin and Walter27]. For the comfort of the reader, we give an argument in Remark 2.2.5 that shows that an orientation determines a class b with the expected property.
$1_B$
; see [Reference Panin and Walter27]. For the comfort of the reader, we give an argument in Remark 2.2.5 that shows that an orientation determines a class b with the expected property.
On the other hand, we are not aware of an similar statement for
![]() $\mathrm {SL}$
or
$\mathrm {SL}$
or
![]() $\mathrm {SL}^c$
orientations.
$\mathrm {SL}^c$
orientations.
Remark 2.1.6. As proved in [Reference Ananyevskiy3, Lem. 1.4], one deduces from the above definition that given a map
![]() $\phi :G \rightarrow H$
from Diagram (2.1.2.a), an H-orientation
$\phi :G \rightarrow H$
from Diagram (2.1.2.a), an H-orientation
![]() $\mathfrak t$
on a ring spectrum
$\mathfrak t$
on a ring spectrum
![]() $\mathbb E$
induces a G-orientation on
$\mathbb E$
induces a G-orientation on
![]() $\mathbb E$
, namely,
$\mathbb E$
, namely,
![]() $\mathfrak t \circ \phi _*$
, where
$\mathfrak t \circ \phi _*$
, where
![]() $\phi _*:{\mathscr V}(X,G) \rightarrow {\mathscr V}(X,H)$
is the additive functor induced by
$\phi _*:{\mathscr V}(X,G) \rightarrow {\mathscr V}(X,H)$
is the additive functor induced by
![]() $\phi $
. We state the following relations between orientations for further use:
$\phi $
. We state the following relations between orientations for further use:
Example 2.1.7. Here are examples among the spectra that will appear in this work.
-
• The absolute ring spectra
 $\mathbf {H}_{\mathrm M} R$
and
$\mathbf {H}_{\mathrm M} R$
and
 $\mathbf {KGL}$
are oriented (this is classical; see e.g., [Reference Déglise12]).
$\mathbf {KGL}$
are oriented (this is classical; see e.g., [Reference Déglise12]). -
• The absolute ring spectra
 $\mathbf {GW}$
and
$\mathbf {GW}$
and
 $\mathbf {W}$
are
$\mathbf {W}$
are
 $\mathrm {SL}^c$
-oriented. The case of Hermitian K-theory is proved in [Reference Panin and Walter28, Th. 5.1]. The case of derived Witt groups follows from that of Hermitian K-theory, given [Reference Ananyevskiy, Levine and Panin4, Def. 3], which gives a morphism of absolute ring spectra
$\mathrm {SL}^c$
-oriented. The case of Hermitian K-theory is proved in [Reference Panin and Walter28, Th. 5.1]. The case of derived Witt groups follows from that of Hermitian K-theory, given [Reference Ananyevskiy, Levine and Panin4, Def. 3], which gives a morphism of absolute ring spectra
 $\mathbf {GW}\rightarrow \mathbf {W}$
.
$\mathbf {GW}\rightarrow \mathbf {W}$
.
Definition 2.1.8. Let
![]() $(\mathbb E,\mathfrak t)$
be a G-oriented ring spectrum as in the previous definition.
$(\mathbb E,\mathfrak t)$
be a G-oriented ring spectrum as in the previous definition.
Then for any scheme X and any G-bundle
![]() $\mathfrak V$
with underlying vector bundle V of rank r, we define the associated Euler class by the formula
$\mathfrak V$
with underlying vector bundle V of rank r, we define the associated Euler class by the formula
where
![]() $s:X \rightarrow V$
(respectively
$s:X \rightarrow V$
(respectively
![]() $\pi :V \rightarrow \operatorname {\mathrm {Th}}(V)$
) is the zero section (respectively canonical projection map).
$\pi :V \rightarrow \operatorname {\mathrm {Th}}(V)$
) is the zero section (respectively canonical projection map).
When the orientation
![]() $\mathfrak t$
is clear from the context, we simply write
$\mathfrak t$
is clear from the context, we simply write
![]() $e(\mathfrak V)$
. We will apply the same convention for all other characteristic classes associated with
$e(\mathfrak V)$
. We will apply the same convention for all other characteristic classes associated with
![]() $\mathrm {Sp}$
-orientations. This is harmless in this work because we will never consider two different such orientations on our ring spectra.
$\mathrm {Sp}$
-orientations. This is harmless in this work because we will never consider two different such orientations on our ring spectra.
One immediately deduces from the properties of Thom classes the following (usual) properties of Euler classes.
Proposition 2.1.9. Consider the assumptions of the previous definition. Then Euler classes satisfy the following formulas:
-
• Invariance under isomorphisms.
 $\phi ^*e(\mathfrak V)=e(\mathfrak W)$
for
$\phi ^*e(\mathfrak V)=e(\mathfrak W)$
for
 $\phi :\mathfrak V \xrightarrow \sim \mathfrak W$
.
$\phi :\mathfrak V \xrightarrow \sim \mathfrak W$
. -
• Pullbacks compatibility.
 $f^*e(\mathfrak V)=e(f^{-1}\mathfrak V)$
for
$f^*e(\mathfrak V)=e(f^{-1}\mathfrak V)$
for
 $f:Y \rightarrow X$
in
$f:Y \rightarrow X$
in
 $\mathscr Sch_B$
.
$\mathscr Sch_B$
. -
• Products compatibility.
 $e(\mathfrak V \oplus \mathfrak W)=e(\mathfrak V) \cdot e(\mathfrak W)$
.
$e(\mathfrak V \oplus \mathfrak W)=e(\mathfrak V) \cdot e(\mathfrak W)$
. -
• Vanishing.
 $e(\mathfrak V)=0$
whenever
$e(\mathfrak V)=0$
whenever
 $\mathfrak V$
contains a trivial G-bundle as a direct factor.
$\mathfrak V$
contains a trivial G-bundle as a direct factor.
2.1.10.
Gysin morphisms. More generally, it is possible to extend the notions of Gysin maps (e.g., [Reference Déglise12, §3]) to a G-oriented ring spectrum
![]() $(\mathbb E,\mathfrak t)$
over B. We plan to come back to this point in a future work. Let us give the example of closed immersions because it is deeply linked with Euler classes.
$(\mathbb E,\mathfrak t)$
over B. We plan to come back to this point in a future work. Let us give the example of closed immersions because it is deeply linked with Euler classes.
Let
![]() $i:Z \rightarrow X$
be a closed immersion between smooth S-schemes with normal bundle
$i:Z \rightarrow X$
be a closed immersion between smooth S-schemes with normal bundle
![]() $N_i$
. A G-orientation
$N_i$
. A G-orientation
![]() $\sigma $
on i is a G-bundle
$\sigma $
on i is a G-bundle
![]() $\mathcal N_i$
over Z whose underlying vector bundle is
$\mathcal N_i$
over Z whose underlying vector bundle is
![]() $N_i$
. One defines the Gysin morphism associated to
$N_i$
. One defines the Gysin morphism associated to
![]() $(i,\sigma )$
:
$(i,\sigma )$
:
 $$ \begin{align*}i_*^\sigma:\mathbb E^{**}(Z) \xrightarrow{\theta_{\mathcal N_i}^{\mathfrak t}} \mathbb E^{**}(\operatorname{\mathrm{Th}}(N_i)) \xrightarrow{\tau_i} {\mathbb E}^{**}(X/X-Z) \rightarrow \mathbb E^{**}(X), \end{align*} $$
$$ \begin{align*}i_*^\sigma:\mathbb E^{**}(Z) \xrightarrow{\theta_{\mathcal N_i}^{\mathfrak t}} \mathbb E^{**}(\operatorname{\mathrm{Th}}(N_i)) \xrightarrow{\tau_i} {\mathbb E}^{**}(X/X-Z) \rightarrow \mathbb E^{**}(X), \end{align*} $$
where the first map is the Thom isomorphism (Paragraph 2.1.4), the second one Morel-Voevodsky’s purity isomorphism and the last one is obtained through the canonical map
![]() $X \rightarrow (X/X-Z)$
. If
$X \rightarrow (X/X-Z)$
. If
![]() $N_i$
is of rank c, the Gysin map is homogeneous of bidegree
$N_i$
is of rank c, the Gysin map is homogeneous of bidegree
![]() $(2c,c)$
.
$(2c,c)$
.
Given a G-bundle
![]() $\mathfrak V=(V,\psi )$
, the zero section
$\mathfrak V=(V,\psi )$
, the zero section
![]() $s_0:X \rightarrow V$
obviously admits a canonical G-orientation
$s_0:X \rightarrow V$
obviously admits a canonical G-orientation
![]() $can$
. Then it follows from the above definition (and the fact that
$can$
. Then it follows from the above definition (and the fact that
![]() $\tau _{s_0}$
is essentially the identity through the latter identification) that
$\tau _{s_0}$
is essentially the identity through the latter identification) that
2.2 Borel classes
2.2.1. Symplectic bundles. Let us be more specific about symplectic vector bundles, introduced in Paragraph 2.1.2.
First recall that any vector bundle
![]() $V/X$
admits a symplectification
$V/X$
admits a symplectification
 $$ \begin{align*}\mathfrak H(V):=\left(V \oplus V^\vee,\begin{pmatrix} 0 & 1 \\ -\mathrm{can} & 0 \end{pmatrix}\right), \end{align*} $$
$$ \begin{align*}\mathfrak H(V):=\left(V \oplus V^\vee,\begin{pmatrix} 0 & 1 \\ -\mathrm{can} & 0 \end{pmatrix}\right), \end{align*} $$
where
![]() $\mathrm {can}:V\to V^{\vee \vee }$
is the usual canonical isomorphism. Using the notation of loc. cit., we get in particular
$\mathrm {can}:V\to V^{\vee \vee }$
is the usual canonical isomorphism. Using the notation of loc. cit., we get in particular
![]() , which we will simply denote by
, which we will simply denote by
![]() $\mathfrak H$
in the sequel. To comply with the classical notations, the direct sum of symplectic vector bundles will be denoted by
$\mathfrak H$
in the sequel. To comply with the classical notations, the direct sum of symplectic vector bundles will be denoted by
![]() $\perp $
. In particular,
$\perp $
. In particular,
![]() $\mathfrak H(\mathbb A^n)=\mathfrak H^{\perp ,n}$
. More generally,
$\mathfrak H(\mathbb A^n)=\mathfrak H^{\perp ,n}$
. More generally,
![]() $\mathfrak H$
sends
$\mathfrak H$
sends
![]() $\oplus $
to
$\oplus $
to
![]() $\perp $
.
$\perp $
.
2.2.2. As explained by Panin and Walter,
![]() $\mathrm {Sp}$
-oriented ring spectra are analogous to (
$\mathrm {Sp}$
-oriented ring spectra are analogous to (
![]() $\mathrm {GL}$
-)oriented ones. Let us first recall the
$\mathrm {GL}$
-)oriented ones. Let us first recall the
![]() $\mathrm {Sp}$
-projective bundle theorem. Consider an
$\mathrm {Sp}$
-projective bundle theorem. Consider an
![]() $\mathrm {Sp}$
-bundle
$\mathrm {Sp}$
-bundle
![]() $(V,\phi )$
over a scheme X. In [Reference Panin and Walter26] Panin and Walter introduced the projective
$(V,\phi )$
over a scheme X. In [Reference Panin and Walter26] Panin and Walter introduced the projective
![]() $\mathrm {Sp}$
-bundle
$\mathrm {Sp}$
-bundle
![]() $\mathrm {H}\mathbb {P}(V,\phi )$
as the open subscheme of the Grassmannian scheme
$\mathrm {H}\mathbb {P}(V,\phi )$
as the open subscheme of the Grassmannian scheme
![]() $\mathrm {Gr}(2,V)$
on which the restriction of
$\mathrm {Gr}(2,V)$
on which the restriction of
![]() $\phi $
to the canonical subbundle of rank
$\phi $
to the canonical subbundle of rank
![]() $2$
is nondegenerate.
$2$
is nondegenerate.
We let U be the tautological rank
![]() $2$
bundle on
$2$
bundle on
![]() $\mathrm {H}\mathbb {P}(V,\psi )$
. By definition, it is equipped with a symplectic structure
$\mathrm {H}\mathbb {P}(V,\psi )$
. By definition, it is equipped with a symplectic structure
![]() $\psi $
coming from the restriction of
$\psi $
coming from the restriction of
![]() $\phi $
and we set
$\phi $
and we set
![]() $\mathfrak U=(U,\psi )$
.
$\mathfrak U=(U,\psi )$
.
The following
![]() $\mathrm {Sp}$
-projective bundle theorem is due to Panin and Walter; see [Reference Panin and Walter26, Th. 8.2] for a proof.
$\mathrm {Sp}$
-projective bundle theorem is due to Panin and Walter; see [Reference Panin and Walter26, Th. 8.2] for a proof.
Theorem 2.2.3. Consider the above notations and assume that V has rank
![]() $2n$
. Let
$2n$
. Let
![]() $p:\mathrm {H}\mathbb {P}(V,\psi ) \rightarrow X$
be the canonical projection,
$p:\mathrm {H}\mathbb {P}(V,\psi ) \rightarrow X$
be the canonical projection,
![]() $\mathbb E$
be an an
$\mathbb E$
be an an
![]() $\mathrm {Sp}$
-oriented ring spectrum and
$\mathrm {Sp}$
-oriented ring spectrum and
![]() $b=e(\mathfrak U)$
be the associated Euler class (Definition 2.1.8).
$b=e(\mathfrak U)$
be the associated Euler class (Definition 2.1.8).
Then the following map is an isomorphism of bi-graded
![]() $\mathbb E^{**}(X)$
-modules:
$\mathbb E^{**}(X)$
-modules:
 $$ \begin{align*}\bigoplus_{i=0}^{n-1} \mathbb E^{**}(X) \rightarrow \mathbb E^{**}\big(\mathrm{H}\mathbb{P}(V,\psi)\big), x_i \mapsto p^*(x_i).b^i. \end{align*} $$
$$ \begin{align*}\bigoplus_{i=0}^{n-1} \mathbb E^{**}(X) \rightarrow \mathbb E^{**}\big(\mathrm{H}\mathbb{P}(V,\psi)\big), x_i \mapsto p^*(x_i).b^i. \end{align*} $$
2.2.5.
Borel classes. It is easy to derive from the previous theorem the theory of Borel classes. Under the above notation, they are the classes
![]() $b_i(V,\psi ) \in \mathbb E^{4i,2i}(X)$
for
$b_i(V,\psi ) \in \mathbb E^{4i,2i}(X)$
for
![]() $i \geq 0$
uniquely determined by the following relations:
$i \geq 0$
uniquely determined by the following relations:
 $$ \begin{align} \sum_{i=0}^n (-1)^i.b_i(V,\psi).b^{n-i}=0, b_0(V,\psi)=1, \forall i>n, b_i(V,\psi)=0. \end{align} $$
$$ \begin{align} \sum_{i=0}^n (-1)^i.b_i(V,\psi).b^{n-i}=0, b_0(V,\psi)=1, \forall i>n, b_i(V,\psi)=0. \end{align} $$
In addition, they satisfy the following relations:
-
(1)
 $\operatorname {\mathrm {rk}}(V)=2n$
,
$\operatorname {\mathrm {rk}}(V)=2n$
,
 $b_n(V,\psi )=e(V,\psi )$
.
$b_n(V,\psi )=e(V,\psi )$
. -
(2) Invariance under isomorphisms.
 $b_i(\mathfrak V)=b_i(\mathfrak W)$
for
$b_i(\mathfrak V)=b_i(\mathfrak W)$
for
 $\phi :\mathfrak V \xrightarrow \sim \mathfrak W$
.
$\phi :\mathfrak V \xrightarrow \sim \mathfrak W$
. -
(3) Pullbacks compatibility.
 $f^*b_i(\mathfrak V)=b_i(f^{-1}\mathfrak V)$
for
$f^*b_i(\mathfrak V)=b_i(f^{-1}\mathfrak V)$
for
 $f:Y \rightarrow X$
in
$f:Y \rightarrow X$
in
 $\mathscr Sch_B$
.
$\mathscr Sch_B$
. -
(4) Trivial bundles.
 $b_i\big [\mathfrak H(\mathbb A^n)\big ]=0$
for
$b_i\big [\mathfrak H(\mathbb A^n)\big ]=0$
for
 $i>0$
,
$i>0$
,
 $n>0$
.
$n>0$
. -
(5) Whitney sum formula.
 $b_t(\mathfrak V \perp \mathfrak W)=b_t(\mathfrak V) \cdot b_t(\mathfrak W)$
in
$b_t(\mathfrak V \perp \mathfrak W)=b_t(\mathfrak V) \cdot b_t(\mathfrak W)$
in
 $\mathbb E^{**}(X)[[t]]$
, where
$\mathbb E^{**}(X)[[t]]$
, where
 $b_t$
denotes the total Borel class (2.2.4.b)
$b_t$
denotes the total Borel class (2.2.4.b) $$ \begin{align} b_t(\mathfrak V)=\sum_i b_i(\mathfrak V).t^i. \end{align} $$
$$ \begin{align} b_t(\mathfrak V)=\sum_i b_i(\mathfrak V).t^i. \end{align} $$
One can reformulate the two last properties by saying that the total Borel class
![]() $b_t$
factors through the
$b_t$
factors through the
![]() $0$
th symplectic K-theory group,
$0$
th symplectic K-theory group,
![]() $\mathbf {KSp}_0(X)$
, and actually induces a morphism of abelian groups, sending sums to products:
$\mathbf {KSp}_0(X)$
, and actually induces a morphism of abelian groups, sending sums to products:
 $$ \begin{align} b_t:\mathbf{KSp}_0(X)/\mathbb Z\big[\mathfrak H\big] \rightarrow \mathbb E^{**}(X)[[t]]^\times. \end{align} $$
$$ \begin{align} b_t:\mathbf{KSp}_0(X)/\mathbb Z\big[\mathfrak H\big] \rightarrow \mathbb E^{**}(X)[[t]]^\times. \end{align} $$
Remark 2.2.5. The situation is therefore very similar to classical oriented ring spectra. In particular, the infinite symplectic projective space
![]() $\mathrm {H}\mathbb {P}^\infty _S$
(obtained as the colimit of the
$\mathrm {H}\mathbb {P}^\infty _S$
(obtained as the colimit of the
![]() $HP^n_S$
) plays a role similar to
$HP^n_S$
) plays a role similar to
![]() $\mathbb P^\infty _S$
. First, it classifies the symplectic bundles of rank
$\mathbb P^\infty _S$
. First, it classifies the symplectic bundles of rank
![]() $2$
over S. Second, according to the preceding theorem, its cohomology is given by a power series ring
$2$
over S. Second, according to the preceding theorem, its cohomology is given by a power series ring
where b corresponds to the Borel class
![]() $b_1(\mathfrak U)$
of the tautological rank
$b_1(\mathfrak U)$
of the tautological rank
![]() $2$
symplectic bundle
$2$
symplectic bundle
![]() $\mathfrak U$
. Besides,
$\mathfrak U$
. Besides,
![]() $b=b_1(\mathfrak U)$
, seen as a class in the reduced cohomology
$b=b_1(\mathfrak U)$
, seen as a class in the reduced cohomology
 $\tilde {\mathbb E}^{4,2}(\mathrm {H}\mathbb {P}^\infty _S)$
, satisfies a normalisation property analogous to that of an orientation in the classical sense (see, e.g., [Reference Déglise12, Def. 2.1.2]). More precisely, its restriction to
$\tilde {\mathbb E}^{4,2}(\mathrm {H}\mathbb {P}^\infty _S)$
, satisfies a normalisation property analogous to that of an orientation in the classical sense (see, e.g., [Reference Déglise12, Def. 2.1.2]). More precisely, its restriction to
![]() $\mathrm {H}\mathbb {P}^1_S$
is the suspension of the unit element
$\mathrm {H}\mathbb {P}^1_S$
is the suspension of the unit element
![]() $1 \in \mathbb E^{00}(S)$
. In other words, it corresponds to
$1 \in \mathbb E^{00}(S)$
. In other words, it corresponds to
![]() $1$
under the isomorphism
$1$
under the isomorphism
 $$ \begin{align*}\tilde{E}^{4,2}(\mathrm{H}\mathbb{P}^1_S) \simeq \mathbb E^{00}(S). \end{align*} $$
$$ \begin{align*}\tilde{E}^{4,2}(\mathrm{H}\mathbb{P}^1_S) \simeq \mathbb E^{00}(S). \end{align*} $$
To get that property, one must recall that the decomposition
![]() $\mathrm {H}\mathbb {P}^1_S=X_0 \sqcup X_2$
from [Reference Panin and Walter26], where
$\mathrm {H}\mathbb {P}^1_S=X_0 \sqcup X_2$
from [Reference Panin and Walter26], where
![]() $X_2$
is closed, of codimension
$X_2$
is closed, of codimension
![]() $2$
, and
$2$
, and
![]() $X_0$
is open. Moreover
$X_0$
is open. Moreover
![]() $X_2$
is isomorphic to
$X_2$
is isomorphic to
![]() $\mathbb A^2_S$
. Note also that according to [Reference Asok, Doran and Fasel5, proof of 2.1.2, Rem. 2.1.3],
$\mathbb A^2_S$
. Note also that according to [Reference Asok, Doran and Fasel5, proof of 2.1.2, Rem. 2.1.3],
![]() $X_0$
is
$X_0$
is
![]() $\mathbb A^1$
-contractible and the normal bundle
$\mathbb A^1$
-contractible and the normal bundle
![]() $N_2$
of
$N_2$
of
![]() $i:X_2 \rightarrow \mathrm {H}\mathbb {P}^1_S$
is trivial. One deduces a homotopy exact sequence
$i:X_2 \rightarrow \mathrm {H}\mathbb {P}^1_S$
is trivial. One deduces a homotopy exact sequence
 $$ \begin{align*}* \rightarrow \mathrm{H}\mathbb{P}^1_S \xrightarrow{i^!} \operatorname{\mathrm{Th}}(N_2) \end{align*} $$
$$ \begin{align*}* \rightarrow \mathrm{H}\mathbb{P}^1_S \xrightarrow{i^!} \operatorname{\mathrm{Th}}(N_2) \end{align*} $$
so that
![]() $i^!$
induces the above isomorphism:
$i^!$
induces the above isomorphism:
 $$ \begin{align*}i_*:\mathbb E^{00}(X_2)\simeq \tilde{\mathbb E}^{4,2}(\operatorname{\mathrm{Th}}(N_2)) \rightarrow \tilde{E}^{4,2}(\mathrm{H}\mathbb{P}^1_S), \end{align*} $$
$$ \begin{align*}i_*:\mathbb E^{00}(X_2)\simeq \tilde{\mathbb E}^{4,2}(\operatorname{\mathrm{Th}}(N_2)) \rightarrow \tilde{E}^{4,2}(\mathrm{H}\mathbb{P}^1_S), \end{align*} $$
after identifying
![]() $\mathbb E^{00}(X_2)$
with
$\mathbb E^{00}(X_2)$
with
![]() $\mathbb E^{00}(S)$
. In particular, the required normalisation can be formulated as the equality in
$\mathbb E^{00}(S)$
. In particular, the required normalisation can be formulated as the equality in
 $\tilde {\mathbb E}^{4,2}(\mathrm {H}\mathbb {P}^1_S)$
:
$\tilde {\mathbb E}^{4,2}(\mathrm {H}\mathbb {P}^1_S)$
:
where
![]() $\mathfrak U=(U,\psi )$
is the canonical rank
$\mathfrak U=(U,\psi )$
is the canonical rank
![]() $2$
symplectic bundle over
$2$
symplectic bundle over
![]() $\mathrm {H}\mathbb {P}^1_S$
. To prove that equality, we first note that
$\mathrm {H}\mathbb {P}^1_S$
. To prove that equality, we first note that
![]() $\mathfrak U/\mathrm {H}\mathbb {P}^1_S$
admits a section s whose zero locus is
$\mathfrak U/\mathrm {H}\mathbb {P}^1_S$
admits a section s whose zero locus is
![]() $X_2$
. In particular, the normal bundle of i is isomorphic to the the restriction of the normal bundle of s restricted to
$X_2$
. In particular, the normal bundle of i is isomorphic to the the restriction of the normal bundle of s restricted to
![]() $X_2$
: in other words:
$X_2$
: in other words:
![]() $N_i=U|_{X_2}$
. In particular, i admits a canonical
$N_i=U|_{X_2}$
. In particular, i admits a canonical
![]() $\mathrm {Sp}$
-orientation (in the sense of 2.1.10), which is the restriction of
$\mathrm {Sp}$
-orientation (in the sense of 2.1.10), which is the restriction of
![]() $\psi $
to
$\psi $
to
![]() $X_2$
. According to the normalisation property of Thom classes (Definition 2.1.3), it follows that
$X_2$
. According to the normalisation property of Thom classes (Definition 2.1.3), it follows that
![]() $i_*$
agrees with the Gysin map
$i_*$
agrees with the Gysin map
![]() $i_*^\psi $
defined in 2.1.10. Then the above equality follows from the computation
$i_*^\psi $
defined in 2.1.10. Then the above equality follows from the computation
The first equality is obtained by the projection formula for the Gysin morphism defined in Paragraph 2.1.10 (use the proof of [Reference Déglise12, Proposition 3.1.4]), the second one from the last assertion of Paragraph 2.1.10 and the last one by definition of Borel classes.
2.2.6.
The symplectic splitting principle. As for the classical Chern classes (see, e.g., [Reference Fulton16, Section 3.2]), one derives from the previous theorem a splitting principle. Given any symplectic bundle
![]() $\mathfrak V$
over a scheme X, there exists an affine morphism
$\mathfrak V$
over a scheme X, there exists an affine morphism
![]() $p:X' \rightarrow X$
inducing a monomorphism
$p:X' \rightarrow X$
inducing a monomorphism
![]() $p^*:\mathbb E^{**}(X) \rightarrow \mathbb E^{**}(X')$
with the property that
$p^*:\mathbb E^{**}(X) \rightarrow \mathbb E^{**}(X')$
with the property that
![]() $p^{-1}(\mathfrak V)$
splits as a direct sum of rank
$p^{-1}(\mathfrak V)$
splits as a direct sum of rank
![]() $2$
symplectic bundles:
$2$
symplectic bundles:
![]() $p^{-1}(\mathfrak V)=\mathcal X_1 \perp \ldots \perp \mathcal X_n$
.
$p^{-1}(\mathfrak V)=\mathcal X_1 \perp \ldots \perp \mathcal X_n$
.
One defines the Borel roots of
![]() $\mathfrak V$
as the Borel classes
$\mathfrak V$
as the Borel classes
![]() $\xi _i=b_1(\mathcal X_i)$
so that by the preceding Whitney sum formula the Borel classes of
$\xi _i=b_1(\mathcal X_i)$
so that by the preceding Whitney sum formula the Borel classes of
![]() $\mathfrak V$
are the elementary symmetric polynomials in the variables
$\mathfrak V$
are the elementary symmetric polynomials in the variables
![]() $\xi _i$
.
$\xi _i$
.
As in the classical case, one can compute universal formulas involving Borel classes of
![]() $\mathfrak V$
by introducing Borel roots
$\mathfrak V$
by introducing Borel roots
![]() $\xi _i$
, which reduce to rank
$\xi _i$
, which reduce to rank
![]() $2$
symplectic bundles, compute as if
$2$
symplectic bundles, compute as if
![]() $\mathfrak V$
was completely split and then express the resulting formula in terms of the elementary symmetric polynomials in the
$\mathfrak V$
was completely split and then express the resulting formula in terms of the elementary symmetric polynomials in the
![]() $\xi _i$
. This principle will be used repeatedly in Section 3.
$\xi _i$
. This principle will be used repeatedly in Section 3.
A particular instance of Borel classes will be useful in the sequel (see Subsection 3.2).
Definition 2.2.7. Consider the notations of the previous paragraph. Given an arbitrary vector bundle V over a scheme X and an integer
![]() $i\geq 0$
, one defines its ith Pontryagin class associated with the
$i\geq 0$
, one defines its ith Pontryagin class associated with the
![]() $\mathrm {Sp}$
-oriented ring spectrum
$\mathrm {Sp}$
-oriented ring spectrum
![]() $\mathbb E$
as
$\mathbb E$
as
 $$ \begin{align*}p_i(V)=b_i\big(\mathfrak H(V)\big). \end{align*} $$
$$ \begin{align*}p_i(V)=b_i\big(\mathfrak H(V)\big). \end{align*} $$
Beware that we do not follow here the conventions of [Reference Ananyevskiy1, Def. 7] for which one uses a different numbering and sign:
 $p_i(V)=(-1)^i.b_{2i}\big (\mathfrak H(V)\big )$
.
$p_i(V)=(-1)^i.b_{2i}\big (\mathfrak H(V)\big )$
.
2.2.8. For the next formula, we need some notation. Let
![]() $u \in \mathcal O_X(X)^\times $
be a global unit on a scheme X. Let us consider the isomorphism
$u \in \mathcal O_X(X)^\times $
be a global unit on a scheme X. Let us consider the isomorphism
![]() $\gamma _u:\mathbb A^1_X \rightarrow \mathbb A^1_X$
obtained by multiplication by u. It induces a morphism of Thom spaces:
$\gamma _u:\mathbb A^1_X \rightarrow \mathbb A^1_X$
obtained by multiplication by u. It induces a morphism of Thom spaces:
![]() $\gamma _u:\operatorname {\mathrm {Th}}(\mathbb A^1_X) \rightarrow \operatorname {\mathrm {Th}}(\mathbb A^1_X)$
. Following Morel (e.g., [Reference Morel24, Lemma 6.1.3]), we denote by
$\gamma _u:\operatorname {\mathrm {Th}}(\mathbb A^1_X) \rightarrow \operatorname {\mathrm {Th}}(\mathbb A^1_X)$
. Following Morel (e.g., [Reference Morel24, Lemma 6.1.3]), we denote by
![]() $\langle u \rangle $
the corresponding automorphism of
$\langle u \rangle $
the corresponding automorphism of
![]() in the stable homotopy category over X. These elements satisfy the following formulas in the group
in the stable homotopy category over X. These elements satisfy the following formulas in the group
![]() :
:
-
(U1)
 $\langle u \rangle \cdot \langle v \rangle =\langle uv \rangle $
.
$\langle u \rangle \cdot \langle v \rangle =\langle uv \rangle $
. -
(U2)
 $\forall i, \langle u^{2i} \rangle =1$
.
$\forall i, \langle u^{2i} \rangle =1$
.
The following proposition is mainly due to [Reference Ananyevskiy3]. At present, we do not know whether it is true for a general
![]() $\mathrm {Sp}$
-oriented spectrum.
$\mathrm {Sp}$
-oriented spectrum.
Proposition 2.2.9. Let
![]() $(\mathbb E,\mathfrak t)$
be an
$(\mathbb E,\mathfrak t)$
be an
![]() $\mathrm {SL}$
-oriented ring spectrum,
$\mathrm {SL}$
-oriented ring spectrum,
![]() $u \in \mathcal O_X(X)^\times $
be a unit and
$u \in \mathcal O_X(X)^\times $
be a unit and
![]() $i \geq 0$
an integer. We write
$i \geq 0$
an integer. We write
![]() $\mathfrak t$
for the induced
$\mathfrak t$
for the induced
![]() $\mathrm {Sp}$
-orientation on
$\mathrm {Sp}$
-orientation on
![]() $\mathbb E$
(Remark 2.1.6).
$\mathbb E$
(Remark 2.1.6).
-
(1) For any
 $\mathrm {Sp}$
-bundle
$\mathrm {Sp}$
-bundle
 $(V,\psi )$
of rank
$(V,\psi )$
of rank
 $2$
:
$2$
:
 $\mathfrak t(V,u.\psi ) =\langle u \rangle .\mathfrak t(V,\psi )$
.
$\mathfrak t(V,u.\psi ) =\langle u \rangle .\mathfrak t(V,\psi )$
. -
(2) For any
 $\mathrm {Sp}$
-vector bundle
$\mathrm {Sp}$
-vector bundle
 $(V,\psi )$
over X:
$(V,\psi )$
over X:
 $b_i(V,u.\psi )=\langle u^i\rangle .b_i(V,\psi )$
.
$b_i(V,u.\psi )=\langle u^i\rangle .b_i(V,\psi )$
.
Here,
![]() $u.\psi $
is simply the composition
$u.\psi $
is simply the composition
![]() $\gamma _u \circ \psi $
.
$\gamma _u \circ \psi $
.
Proof. Let then
![]() $(V,\psi )$
be a symplectic bundle. The symplectic form
$(V,\psi )$
be a symplectic bundle. The symplectic form
![]() $\psi $
corresponds to a trivialisation of the determinant
$\psi $
corresponds to a trivialisation of the determinant
![]() $\psi :\det V\simeq \mathbb A^1_X$
, and the symplectic form
$\psi :\det V\simeq \mathbb A^1_X$
, and the symplectic form
![]() $u.\psi $
corresponds to the composite
$u.\psi $
corresponds to the composite
 $$ \begin{align*} \det V\stackrel \psi\to \mathbb A^1_X\stackrel {\cdot u}\to \mathbb A^1_X. \end{align*} $$
$$ \begin{align*} \det V\stackrel \psi\to \mathbb A^1_X\stackrel {\cdot u}\to \mathbb A^1_X. \end{align*} $$
The claim now follows from [Reference Ananyevskiy3, Lemma 7.3]. For (2), we can simply use the symplectic splitting principle 2.2.6.
Remark 2.2.10. Extending the notations of Paragraph 2.2.8, it will be convenient to introduce the following notationFootnote 9:
-
•
 $\langle u_1,...,u_n \rangle =\langle u_1\rangle + \ldots + \langle u_n \rangle $
.
$\langle u_1,...,u_n \rangle =\langle u_1\rangle + \ldots + \langle u_n \rangle $
. -
•
 $\epsilon =-\langle -1 \rangle $
.
$\epsilon =-\langle -1 \rangle $
. -
•
 $h=\langle 1,-1 \rangle $
.
$h=\langle 1,-1 \rangle $
.
Extending a classical terminology from the theory of quadratic forms, we will say that a cohomology class
![]() $x \in \mathbb E^{n,i}(X)$
is hyperbolic if it is of the form
$x \in \mathbb E^{n,i}(X)$
is hyperbolic if it is of the form
using the
![]() -module structure on
-module structure on
![]() $\mathbb E^{**}(X)$
.
$\mathbb E^{**}(X)$
.
2.3 Walter’s ternary laws
2.3.1. It appears clearly from the previous section that Borel classes are to
![]() $\mathrm {Sp}$
-oriented spectra what Chern classes are to oriented spectra. It is therefore natural to look for an analogue of the theory of formal group law associated to any oriented ring spectrum.
$\mathrm {Sp}$
-oriented spectra what Chern classes are to oriented spectra. It is therefore natural to look for an analogue of the theory of formal group law associated to any oriented ring spectrum.
As observed by Walter,Footnote 10 the first technical problem that arises is the fact that symplectic bundles are not stable under tensor product. However, if you consider an odd number of symplectic bundles, then their tensor product is equipped with a canonical symplectic form – the tensor product of the symplectic forms of each bundle. The second technical problem is that a triple product of symplectic bundles of rank
![]() $2$
is of rank
$2$
is of rank
![]() $8$
. This means that we will have to take into account four nontrivial Borel classes
$8$
. This means that we will have to take into account four nontrivial Borel classes
![]() $b_i$
for
$b_i$
for
![]() $i=1,\ldots , 4$
. Once taking into account these differences, we can mimic the construction of the associated formal group law in classical orientation theory as follows.
$i=1,\ldots , 4$
. Once taking into account these differences, we can mimic the construction of the associated formal group law in classical orientation theory as follows.
Let us fix an
![]() $\mathrm {Sp}$
-oriented ring spectra
$\mathrm {Sp}$
-oriented ring spectra
![]() $(\mathbb E,\mathfrak t)$
over an arbitrary scheme X (Definition 2.1.3). Put
$(\mathbb E,\mathfrak t)$
over an arbitrary scheme X (Definition 2.1.3). Put
![]() $\mathbb E^{**}=\mathbb E^{**}(X)$
.
$\mathbb E^{**}=\mathbb E^{**}(X)$
.
We consider the following triple product as an ind-smooth X-scheme:
![]() $P=\mathrm {H}\mathbb {P}^\infty _X \times _X \mathrm {H}\mathbb {P}^\infty _X \times _X \mathrm {H}\mathbb {P}^\infty _X$
. For
$P=\mathrm {H}\mathbb {P}^\infty _X \times _X \mathrm {H}\mathbb {P}^\infty _X \times _X \mathrm {H}\mathbb {P}^\infty _X$
. For
![]() $i=1, 2, 3$
, let
$i=1, 2, 3$
, let
![]() $\mathfrak U_i$
be the respective canonical rank
$\mathfrak U_i$
be the respective canonical rank
![]() $2$
symplectic bundle on the ith coordinate, and let
$2$
symplectic bundle on the ith coordinate, and let
![]() $x,y,z=b_1(\mathfrak U_1), b_1(\mathfrak U_2), b_1(\mathfrak U_3)$
. According to the symplectic projective bundle theorem 2.2.3, one gets the following isomorphism of
$x,y,z=b_1(\mathfrak U_1), b_1(\mathfrak U_2), b_1(\mathfrak U_3)$
. According to the symplectic projective bundle theorem 2.2.3, one gets the following isomorphism of
![]() $\mathbb E^{**}$
-bigraded rings:
$\mathbb E^{**}$
-bigraded rings:
the ring of power series in three variables. The next definition follows considerations initiated by Walter.
Definition 2.3.2. Consider an
![]() $\mathrm {Sp}$
-oriented spectrum
$\mathrm {Sp}$
-oriented spectrum
![]() $\mathbb E$
. We respectively associate to
$\mathbb E$
. We respectively associate to
![]() $\mathbb E$
the lth ternary laws for
$\mathbb E$
the lth ternary laws for
![]() $l \in \{1,2,3,4\}$
and the total ternary law:
$l \in \{1,2,3,4\}$
and the total ternary law:
 $$ \begin{align*} F_l(x,y,z)&=b_l(\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3) \in \mathbb E^{**}[[x,y,z]], \\ F_t(x,y,z)&=b_t(\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3) \in \mathbb E^{**}[[x,y,z]][t]. \end{align*} $$
$$ \begin{align*} F_l(x,y,z)&=b_l(\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3) \in \mathbb E^{**}[[x,y,z]], \\ F_t(x,y,z)&=b_t(\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3) \in \mathbb E^{**}[[x,y,z]][t]. \end{align*} $$
We will call
![]() $F_t(x,y,z)$
the formal ternary law (sometimes abbreviated FTL) associated with the
$F_t(x,y,z)$
the formal ternary law (sometimes abbreviated FTL) associated with the
![]() $\mathrm {Sp}$
-oriented spectrum
$\mathrm {Sp}$
-oriented spectrum
![]() $\mathbb E$
. In the sequel, we will also generically write the lth ternary law as
$\mathbb E$
. In the sequel, we will also generically write the lth ternary law as
 $$ \begin{align*}F_l(x,y,z)=\sum_{i,j,k} a_{ijk}^l.x^iy^jz^k. \end{align*} $$
$$ \begin{align*}F_l(x,y,z)=\sum_{i,j,k} a_{ijk}^l.x^iy^jz^k. \end{align*} $$
Note that by construction, x,y and z have bidegree
![]() $(4,2)$
, when viewed as elements of
$(4,2)$
, when viewed as elements of
![]() $\mathbb E^{**}(P)$
. Thus, the bidegree of
$\mathbb E^{**}(P)$
. Thus, the bidegree of
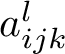 $a_{ijk}^l$
, as an element of the ring of coefficients
$a_{ijk}^l$
, as an element of the ring of coefficients
![]() $\mathbb E^{**}$
, is given by
$\mathbb E^{**}$
, is given by
 $$ \begin{align} \deg(a_{ijk}^l)=(l-i-j-k).(4,2). \end{align} $$
$$ \begin{align} \deg(a_{ijk}^l)=(l-i-j-k).(4,2). \end{align} $$
As in the case of formal group laws associated with oriented ring spectra, the ternary laws play a universal role: given any rank
![]() $2$
symplectic bundles
$2$
symplectic bundles
![]() $\mathfrak V_1, \mathfrak V_2, \mathfrak V_3$
over a scheme X, one always gets the computation
$\mathfrak V_1, \mathfrak V_2, \mathfrak V_3$
over a scheme X, one always gets the computation
the substitution being legitimate because the Borel classes
![]() $b_1(\mathfrak V_i)$
are nilpotent ([Reference Panin and Walter26, Theorem 8.6]).
$b_1(\mathfrak V_i)$
are nilpotent ([Reference Panin and Walter26, Theorem 8.6]).
2.3.3. It is legitimate to look for an analogue of the axioms satisfied by formal group laws in the case of ternary laws. Commutativity is obvious, because the tensor product of three symplectic bundles is commutative. Explicitly, the coefficient
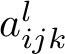 $a^l_{ijk}$
above is independent of the order of the indices
$a^l_{ijk}$
above is independent of the order of the indices
![]() $i,j,k$
:
$i,j,k$
:
 $$ \begin{align} a_{ijk}^l=a_{jik}^l=a_{ikj}^l. \end{align} $$
$$ \begin{align} a_{ijk}^l=a_{jik}^l=a_{ikj}^l. \end{align} $$
In other words, the power series
![]() $F_l(x,y,z)$
is symmetric in the variables
$F_l(x,y,z)$
is symmetric in the variables
![]() $x,y,z$
. To give examples of ternary laws, it is useful to consider a basis for the symmetric polynomials in
$x,y,z$
. To give examples of ternary laws, it is useful to consider a basis for the symmetric polynomials in
![]() $x,y,z$
. We choose the monomial basis denoted by
$x,y,z$
. We choose the monomial basis denoted by
 $$ \begin{align} \sigma(x^iy^jz^k)=\sum_{(a,b,c)} x^ay^bz^c, \end{align} $$
$$ \begin{align} \sigma(x^iy^jz^k)=\sum_{(a,b,c)} x^ay^bz^c, \end{align} $$
where the sum runs over the monomials
![]() $x^ay^bz^c$
in the orbit of
$x^ay^bz^c$
in the orbit of
![]() $x^iy^jz^k$
under the action of the permutations of the variables
$x^iy^jz^k$
under the action of the permutations of the variables
![]() $x,y,z$
. So taking into account the commutativity constraints, ternary laws can be written as
$x,y,z$
. So taking into account the commutativity constraints, ternary laws can be written as
 $$ \begin{align*}F_l(x,y,z)=\sum_{i \geq j \geq k} a_{ijk}^l.\sigma(x^iy^jz^k). \end{align*} $$
$$ \begin{align*}F_l(x,y,z)=\sum_{i \geq j \geq k} a_{ijk}^l.\sigma(x^iy^jz^k). \end{align*} $$
The analogue of the relation
![]() $F(x,0)=x$
is already more involved as shown by the following formula due to Walter.
$F(x,0)=x$
is already more involved as shown by the following formula due to Walter.
Proposition 2.3.4. Let
![]() $\mathbb E$
be an
$\mathbb E$
be an
![]() $\mathrm {SL}$
-oriented ring spectrum over a scheme X. We apply the previous definition to the induced
$\mathrm {SL}$
-oriented ring spectrum over a scheme X. We apply the previous definition to the induced
![]() $\mathrm {Sp}$
-orientation on
$\mathrm {Sp}$
-orientation on
![]() $\mathbb E$
(Remark 2.1.6). Then one gets the following computations:
$\mathbb E$
(Remark 2.1.6). Then one gets the following computations:
-
(1) Using the notation of 2.2.10 and the
 -module structure on
-module structure on
 $\mathbb E^{**}(X)$
: In terms of coefficients:
$\mathbb E^{**}(X)$
: In terms of coefficients: $$ \begin{align*}F_t(x,0,0)=1+2h.xt+2(h-\epsilon).x^2t^2+2h.x^3t^3+x^4t^4. \end{align*} $$
$$ \begin{align*}F_t(x,0,0)=1+2h.xt+2(h-\epsilon).x^2t^2+2h.x^3t^3+x^4t^4. \end{align*} $$
 $$ \begin{align*} &i \neq l \Rightarrow a^l_{i00}=0, \\ &a^1_{100}=a^3_{300}=2h, a^2_{200}=2(h-\epsilon), a^4_{400}=1. \end{align*} $$
$$ \begin{align*} &i \neq l \Rightarrow a^l_{i00}=0, \\ &a^1_{100}=a^3_{300}=2h, a^2_{200}=2(h-\epsilon), a^4_{400}=1. \end{align*} $$
-
(2)
 $F_4(x,x,0)=0$
. In terms of coefficients:
$F_4(x,x,0)=0$
. In terms of coefficients:
 $\sum _{i=0}^r a^4_{i,r-i,0}=0$
.
$\sum _{i=0}^r a^4_{i,r-i,0}=0$
.
Proof. Consider the first assertion. We write
![]() $\mathfrak U=(U,\psi )$
for
$\mathfrak U=(U,\psi )$
for
![]() $\mathfrak U_1$
. Given that
$\mathfrak U_1$
. Given that
![]() $b_i(\mathfrak H)=0$
for
$b_i(\mathfrak H)=0$
for
![]() $i>0$
(Paragraph 2.2.4), we get
$i>0$
(Paragraph 2.2.4), we get
 $$ \begin{align*}F_l(x,0,0)=b_l\big((U,\psi) \otimes \mathfrak H \otimes \mathfrak H\big). \end{align*} $$
$$ \begin{align*}F_l(x,0,0)=b_l\big((U,\psi) \otimes \mathfrak H \otimes \mathfrak H\big). \end{align*} $$
Let
![]() $h^\prime $
be the symmetric bundle on
$h^\prime $
be the symmetric bundle on
![]() $\mathbb Z^2$
given by the matrix
$\mathbb Z^2$
given by the matrix
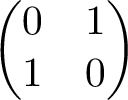 $\displaystyle {\begin {pmatrix} 0 & 1 \\ 1 & 0\end {pmatrix}}$
. A direct computation shows that
$\displaystyle {\begin {pmatrix} 0 & 1 \\ 1 & 0\end {pmatrix}}$
. A direct computation shows that
![]() $\mathfrak H \otimes \mathfrak H$
is isometric to
$\mathfrak H \otimes \mathfrak H$
is isometric to
![]() $h^\prime \perp h^\prime $
; that is,
$h^\prime \perp h^\prime $
; that is,
![]() $\mathfrak H \otimes \mathfrak H=2h^\prime $
. We have
$\mathfrak H \otimes \mathfrak H=2h^\prime $
. We have
![]() $h^\prime +1= h+1$
as illustrated by the following computation:
$h^\prime +1= h+1$
as illustrated by the following computation:
 $$ \begin{align*} \begin{pmatrix} 1 & 0 & 1 \\ 1 & -1 & 1 \\ 0 & 1 & -1 \end{pmatrix}\begin{pmatrix} 0 & 1 & 0 \\ 1 & 0 & 0 \\ 0 & 0 & 1\end{pmatrix} \begin{pmatrix} 1 & 1 & 0 \\ 0 & -1 & 1\\ 1 & 1 & -1\end{pmatrix}=\begin{pmatrix} 1 & 0 & 0 \\ 0 & -1 & 0 \\ 0 & 0 & 1\end{pmatrix}. \end{align*} $$
$$ \begin{align*} \begin{pmatrix} 1 & 0 & 1 \\ 1 & -1 & 1 \\ 0 & 1 & -1 \end{pmatrix}\begin{pmatrix} 0 & 1 & 0 \\ 1 & 0 & 0 \\ 0 & 0 & 1\end{pmatrix} \begin{pmatrix} 1 & 1 & 0 \\ 0 & -1 & 1\\ 1 & 1 & -1\end{pmatrix}=\begin{pmatrix} 1 & 0 & 0 \\ 0 & -1 & 0 \\ 0 & 0 & 1\end{pmatrix}. \end{align*} $$
It follows that
![]() $\mathfrak H \otimes \mathfrak H+2= 2h^\prime +2=2h+2$
. We then obtain
$\mathfrak H \otimes \mathfrak H+2= 2h^\prime +2=2h+2$
. We then obtain
 $$ \begin{align*} b_t\big(((U,\psi) \otimes \mathfrak H \otimes \mathfrak H)\perp(U,\psi)\perp(U,\psi)\big)=b_t\big((2h.(U,\psi))\perp(U,\psi)\perp(U,\psi)\big). \end{align*} $$
$$ \begin{align*} b_t\big(((U,\psi) \otimes \mathfrak H \otimes \mathfrak H)\perp(U,\psi)\perp(U,\psi)\big)=b_t\big((2h.(U,\psi))\perp(U,\psi)\perp(U,\psi)\big). \end{align*} $$
We now observe that if
![]() $(V_1,\phi _1)$
and
$(V_1,\phi _1)$
and
![]() $(V_2,\phi _2)$
are symplectic bundles on
$(V_2,\phi _2)$
are symplectic bundles on
![]() $\mathrm {H}\mathbb {P}^\infty _X$
such that
$\mathrm {H}\mathbb {P}^\infty _X$
such that
then
![]() $b_t(V_1,\phi _1)=b_t(V_2,\phi _2)$
. Indeed, we have
$b_t(V_1,\phi _1)=b_t(V_2,\phi _2)$
. Indeed, we have
and the claim follows from the fact that
![]() $(1-xt)$
is not a zero divisor in
$(1-xt)$
is not a zero divisor in
![]() $\mathbb E^{**}(X)[[x]][t]$
. Thus,
$\mathbb E^{**}(X)[[x]][t]$
. Thus,
 $$ \begin{align*} b_t\big((U,\psi) \otimes \mathfrak H \otimes \mathfrak H\big)=b_t\big(2h.(U,\psi)\big)=b_t\big((U,\psi)\perp (U,\psi)\perp (U,-\psi)\perp (U,-\psi)). \end{align*} $$
$$ \begin{align*} b_t\big((U,\psi) \otimes \mathfrak H \otimes \mathfrak H\big)=b_t\big(2h.(U,\psi)\big)=b_t\big((U,\psi)\perp (U,\psi)\perp (U,-\psi)\perp (U,-\psi)). \end{align*} $$
According to Proposition 2.2.9, one gets
and then
 $$ \begin{align*}b_t\big((U_{,}\psi) \otimes \mathfrak H \otimes \mathfrak H\big)=(1+x.t)^2.\big(1-(\epsilon x).t\big)^2 \end{align*} $$
$$ \begin{align*}b_t\big((U_{,}\psi) \otimes \mathfrak H \otimes \mathfrak H\big)=(1+x.t)^2.\big(1-(\epsilon x).t\big)^2 \end{align*} $$
so that expanding the last term gives the desired result.
To get the second point, we have to compute
![]() $b_4(\mathfrak U \otimes \mathfrak U \otimes \mathfrak H)$
. We claim that the bundle
$b_4(\mathfrak U \otimes \mathfrak U \otimes \mathfrak H)$
. We claim that the bundle
![]() $\mathfrak U \otimes \mathfrak U \otimes \mathfrak H$
has a nowhere vanishing section. Indeed, consider the short exact sequence
$\mathfrak U \otimes \mathfrak U \otimes \mathfrak H$
has a nowhere vanishing section. Indeed, consider the short exact sequence
Because
![]() $\mathfrak U$
is symplectic, we have an isomorphism
$\mathfrak U$
is symplectic, we have an isomorphism
![]() $\det (\mathfrak U)\simeq \mathcal {O}$
and it follows that
$\det (\mathfrak U)\simeq \mathcal {O}$
and it follows that
![]() $\mathfrak U \otimes \mathfrak U$
has a nowhere vanishing section. So does
$\mathfrak U \otimes \mathfrak U$
has a nowhere vanishing section. So does
![]() $\mathfrak H$
, and finally we see that
$\mathfrak H$
, and finally we see that
![]() $\mathfrak U \otimes \mathfrak U \otimes \mathfrak H$
has a nowhere vanishing section. Consequently, its Euler class vanishes; hence the result (according to point (1) in Paragraph 2.2.4).
$\mathfrak U \otimes \mathfrak U \otimes \mathfrak H$
has a nowhere vanishing section. Consequently, its Euler class vanishes; hence the result (according to point (1) in Paragraph 2.2.4).
Remark 2.3.5. If X is a scheme over
![]() $\mathbb Z[{1}/{2}]$
, we have directly
$\mathbb Z[{1}/{2}]$
, we have directly
![]() $\mathfrak H\otimes \mathfrak H=2h$
and the proof of the first assertion is slightly easier.
$\mathfrak H\otimes \mathfrak H=2h$
and the proof of the first assertion is slightly easier.
Remark 2.3.6. There are also formulas encoding the associativity of the ternary tensor product of symplectic bundles. Such a formula can be expressed by considering five rank
![]() $2$
symplectic bundles. We work over
$2$
symplectic bundles. We work over
![]() $P=(\mathrm {H}\mathbb {P}^\infty )^5$
and write
$P=(\mathrm {H}\mathbb {P}^\infty )^5$
and write
![]() $\mathfrak U_i$
for the pullback of universal rank
$\mathfrak U_i$
for the pullback of universal rank
![]() $2$
vector
$2$
vector
![]() $\mathrm {Sp}$
-bundle over the ith copy of P. The formula then amounts to the equality of total Borel classes:
$\mathrm {Sp}$
-bundle over the ith copy of P. The formula then amounts to the equality of total Borel classes:
Each of these Borel classes can be computed in terms of the total ternary law
![]() $F_t(x,y,z)$
using the symplectic splitting principle (Remark 2.2.6) and introducing three Borel roots for each of the rank
$F_t(x,y,z)$
using the symplectic splitting principle (Remark 2.2.6) and introducing three Borel roots for each of the rank
![]() $8 \mathrm {Sp}$
-bundles
$8 \mathrm {Sp}$
-bundles
![]() $\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3$
and
$\mathfrak U_1 \otimes \mathfrak U_2 \otimes \mathfrak U_3$
and
![]() $\mathfrak U_2 \otimes \mathfrak U_3 \otimes \mathfrak U_4$
. We will not use these formulas in the sequel, but we will come back to this in further work.
$\mathfrak U_2 \otimes \mathfrak U_3 \otimes \mathfrak U_4$
. We will not use these formulas in the sequel, but we will come back to this in further work.
2.3.7.
The additive oriented case. Suppose that
![]() $\mathbb E$
is
$\mathbb E$
is
![]() $\mathrm {GL}$
-oriented and consider the induced
$\mathrm {GL}$
-oriented and consider the induced
![]() $\mathrm {Sp}$
-orientation (Remark 2.1.6). In this case, one can express the total ternary law
$\mathrm {Sp}$
-orientation (Remark 2.1.6). In this case, one can express the total ternary law
![]() $F_t(x,y,z)$
in terms of the formal group law associated with the
$F_t(x,y,z)$
in terms of the formal group law associated with the
![]() $\mathrm {GL}$
-orientation. We assume for simplicity that the formal group law associated with the
$\mathrm {GL}$
-orientation. We assume for simplicity that the formal group law associated with the
![]() $\mathrm {GL}$
-orientation is the additive one. In this case, the Borel classes can be expressed in terms of Chern classes as follows:
$\mathrm {GL}$
-orientation is the additive one. In this case, the Borel classes can be expressed in terms of Chern classes as follows:
This is easily seen for a symplectic bundle of rank
![]() $2$
, using the fact that its first Chern class is trivial.Footnote 11 The result for symplectic bundles of higher rank follows from the splitting principle. So, using the notations of the above definition, one can use the following equality:
$2$
, using the fact that its first Chern class is trivial.Footnote 11 The result for symplectic bundles of higher rank follows from the splitting principle. So, using the notations of the above definition, one can use the following equality:
 $$ \begin{align} c_t(U_1 \otimes U_2 \otimes U_3)=\sum_{i=2l}F_l(x,y,z).t^i, \end{align} $$
$$ \begin{align} c_t(U_1 \otimes U_2 \otimes U_3)=\sum_{i=2l}F_l(x,y,z).t^i, \end{align} $$
where
![]() $\mathfrak U_i=(U_i,\psi _i)$
,
$\mathfrak U_i=(U_i,\psi _i)$
,
![]() $x=c_2(U_1)$
,
$x=c_2(U_1)$
,
![]() $y=c_2(U_2)$
,
$y=c_2(U_2)$
,
![]() $z=c_2(U_3)$
. Using the splitting principle to compute the left-hand side (and the fact the formal group law underlying
$z=c_2(U_3)$
. Using the splitting principle to compute the left-hand side (and the fact the formal group law underlying
![]() $\mathbb E$
is additive), one derives from the previous equality the following one:
$\mathbb E$
is additive), one derives from the previous equality the following one:
 $$ \begin{align*} &\prod_{e_1,e_2,e_3 \in \{\pm 1\}} (e_1u_1+e_2u_2+e_3u_3)=\sum_{i=2l} F_l(x,y,z).t^i, \\ &\quad x=-u_1^2, y=-u_2^2, z=-u_3^2. \end{align*} $$
$$ \begin{align*} &\prod_{e_1,e_2,e_3 \in \{\pm 1\}} (e_1u_1+e_2u_2+e_3u_3)=\sum_{i=2l} F_l(x,y,z).t^i, \\ &\quad x=-u_1^2, y=-u_2^2, z=-u_3^2. \end{align*} $$
The result of this computation yields the following example of FTL.
Proposition 2.3.8. Consider a
![]() $\mathrm {GL}$
-orientated ring spectrum
$\mathrm {GL}$
-orientated ring spectrum
![]() $\mathbb E$
whose associated FGL is additive. Then, using notations (2.3.3.b), the FTL associated with the naturally induced
$\mathbb E$
whose associated FGL is additive. Then, using notations (2.3.3.b), the FTL associated with the naturally induced
![]() $\mathrm {Sp}$
-orientation on
$\mathrm {Sp}$
-orientation on
![]() $\mathbb E$
(see Remark 2.1.6) is
$\mathbb E$
(see Remark 2.1.6) is
 $$ \begin{align*}F_l(x,y,z)=\begin{cases} 4.\sigma(x), & l=1, \\ 6.\sigma(x^2)+4.\sigma(xy), & l=2, \\ 4.\sigma(x^3)-4.\sigma(x^2y)+40.xyz, & l=3, \\ \sigma(x^4)-4.\sigma(x^3y)+6.\sigma(x^2y^2)+4.\sigma(x^2yz), & l=4. \end{cases} \end{align*} $$
$$ \begin{align*}F_l(x,y,z)=\begin{cases} 4.\sigma(x), & l=1, \\ 6.\sigma(x^2)+4.\sigma(xy), & l=2, \\ 4.\sigma(x^3)-4.\sigma(x^2y)+40.xyz, & l=3, \\ \sigma(x^4)-4.\sigma(x^3y)+6.\sigma(x^2y^2)+4.\sigma(x^2yz), & l=4. \end{cases} \end{align*} $$
3 Ternary laws associated with Chow-Witt groups
3.1 General principles
3.1.1. The aim of this section is to compute the ternary laws (Definition 2.3.2) associated with Chow-Witt groups or, equivalently, Milnor-Witt motivic cohomology. So, in the entire section we will work with the ring of coefficients
![]() $A=\mathbb E^{**}$
of some
$A=\mathbb E^{**}$
of some
![]() $\mathrm {SL}$
-oriented ring spectrum
$\mathrm {SL}$
-oriented ring spectrum
![]() $\mathbb E$
. The ternary laws live in the ring of formal power series
$\mathbb E$
. The ternary laws live in the ring of formal power series
![]() $A[[x,y,z]]$
.
$A[[x,y,z]]$
.
One can already obtain the following simple lemma that follows from the formula for the degree of the coefficients of the ternary laws (2.3.2.a) and Proposition 2.3.4.
Lemma 3.1.2. Let
![]() $\mathbb E$
be an
$\mathbb E$
be an
![]() $\mathrm {SL}$
-oriented theory over a scheme X, with coefficients ring
$\mathrm {SL}$
-oriented theory over a scheme X, with coefficients ring
![]() $\mathbb E^{**}=\mathbb E^{**}(X)$
. We assume
$\mathbb E^{**}=\mathbb E^{**}(X)$
. We assume
![]() $\mathbb E^{n,i}=0$
in degree
$\mathbb E^{n,i}=0$
in degree
![]() $m.(4,2)$
for
$m.(4,2)$
for
![]() $m\neq 0$
.
$m\neq 0$
.
Then the FTL associated with
![]() $\mathbb E$
has the following form:
$\mathbb E$
has the following form:
 $$ \begin{align*}F_l(x,y,z)= \begin{cases} 2h.\sigma(x), & l=1, \\ 2(h-\epsilon).\sigma(x^2)+a_{110}^2.\sigma(xy), & l=2, \\ 2h.\sigma(x^3)+a^3_{210}.\sigma(x^2y)+a^3_{111}.xyz, & l=3, \\ \sigma(x^4)+a^4_{310}.\sigma(x^3y)+a^4_{220}.\sigma(x^2y^2)+a^4_{211}.\sigma(x^2yz), & l=4 \end{cases} \end{align*} $$
$$ \begin{align*}F_l(x,y,z)= \begin{cases} 2h.\sigma(x), & l=1, \\ 2(h-\epsilon).\sigma(x^2)+a_{110}^2.\sigma(xy), & l=2, \\ 2h.\sigma(x^3)+a^3_{210}.\sigma(x^2y)+a^3_{111}.xyz, & l=3, \\ \sigma(x^4)+a^4_{310}.\sigma(x^3y)+a^4_{220}.\sigma(x^2y^2)+a^4_{211}.\sigma(x^2yz), & l=4 \end{cases} \end{align*} $$
using convention (2.3.3.b) for
![]() $\sigma $
.
$\sigma $
.
3.2 The case of Witt groups
3.2.1. We will now determine the ternary laws
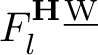 $F_l^{{\mathbf H} \operatorname {{\underline W}}}$
in the case of Witt unramified cohomology, represented by
$F_l^{{\mathbf H} \operatorname {{\underline W}}}$
in the case of Witt unramified cohomology, represented by
![]() ${\mathbf H} \operatorname {{\underline W}}$
. The underlying category of schemes
${\mathbf H} \operatorname {{\underline W}}$
. The underlying category of schemes
![]() $\mathscr Sch$
is that of k-schemes for a fixed base field k of characteristic different from
$\mathscr Sch$
is that of k-schemes for a fixed base field k of characteristic different from
![]() $2$
(see also Notation section).
$2$
(see also Notation section).
We can apply Lemma 3.1.2 to this ring spectrum. Recall, moreover, that on
![]() ${\mathbf H} \operatorname {{\underline W}}$
one gets
${\mathbf H} \operatorname {{\underline W}}$
one gets
![]() $\epsilon =1$
and
$\epsilon =1$
and
![]() $h=0$
. Let us restate the lemma in our particular case to clarify the situation:
$h=0$
. Let us restate the lemma in our particular case to clarify the situation:
 $$ \begin{align*}F^{{\mathbf H} \operatorname{{\underline W}}}_l(x,y,z)= \begin{cases} 0, & l=1, \\ -2.\sigma(x^2)+a_{110}^2.\sigma(xy), & l=2, \\ a^3_{210}.\sigma(x^2y)+a^3_{111}.xyz, & l=3, \\ \sigma(x^4)+a^4_{310}.\sigma(x^3y)+a^4_{220}.\sigma(x^2y^2)+a^4_{211}.\sigma(x^2yz), & l=4. \end{cases} \end{align*} $$
$$ \begin{align*}F^{{\mathbf H} \operatorname{{\underline W}}}_l(x,y,z)= \begin{cases} 0, & l=1, \\ -2.\sigma(x^2)+a_{110}^2.\sigma(xy), & l=2, \\ a^3_{210}.\sigma(x^2y)+a^3_{111}.xyz, & l=3, \\ \sigma(x^4)+a^4_{310}.\sigma(x^3y)+a^4_{220}.\sigma(x^2y^2)+a^4_{211}.\sigma(x^2yz), & l=4. \end{cases} \end{align*} $$
Our first step consists in computing
![]() $F_l^W(x,y,0)$
. We use in particular the following proposition, which is a combination of results of Levine and Ananyevskiy.
$F_l^W(x,y,0)$
. We use in particular the following proposition, which is a combination of results of Levine and Ananyevskiy.
Proposition 3.2.2. Assume
![]() $\mathrm {char}(k) \neq 2$
. Let
$\mathrm {char}(k) \neq 2$
. Let
![]() $P=(\mathrm {H}\mathbb {P}^\infty )^3$
and
$P=(\mathrm {H}\mathbb {P}^\infty )^3$
and
![]() $\mathfrak U_i=(U_i,\psi _i)$
be the tautological rank
$\mathfrak U_i=(U_i,\psi _i)$
be the tautological rank
![]() $2$
symplectic bundle on the ith coordinate of P (as in 2.3.1). Put
$2$
symplectic bundle on the ith coordinate of P (as in 2.3.1). Put
![]() $x=b_1(\mathfrak U_1)$
,
$x=b_1(\mathfrak U_1)$
,
![]() $y=b_1(\mathfrak U_2)$
. One can compute the following Pontryagin classes (Definition 2.2.7) associated with
$y=b_1(\mathfrak U_2)$
. One can compute the following Pontryagin classes (Definition 2.2.7) associated with
![]() ${\mathbf H} \operatorname {{\underline W}}$
:
${\mathbf H} \operatorname {{\underline W}}$
:
 $$ \begin{align*}p_l^W(U_1 \otimes U_2)=\begin{cases} -2.(x^2+y^2), & l=2, \\ 0, & l=3, \\ (x^4+y^4)-2.x^2y^2, & l=4. \end{cases} \end{align*} $$
$$ \begin{align*}p_l^W(U_1 \otimes U_2)=\begin{cases} -2.(x^2+y^2), & l=2, \\ 0, & l=3, \\ (x^4+y^4)-2.x^2y^2, & l=4. \end{cases} \end{align*} $$
For the computation of the odd classes, see [Reference Ananyevskiy3, Corollary 7.9]; for the even ones, see [Reference Levine20, Prop. 9.1]. According to our definition, we get
So the above proposition already gives us the following relations (corresponding to
![]() $l=2,3,4$
):
$l=2,3,4$
):
To get the remaining coefficients, we use the symmetric product
![]() $\operatorname {\mathrm {Sym}}^*$
of symplectic vector bundles. In fact, the next proposition allows determining
$\operatorname {\mathrm {Sym}}^*$
of symplectic vector bundles. In fact, the next proposition allows determining
![]() $F_l^W(x,x,x)$
, and this will allow us to conclude.
$F_l^W(x,x,x)$
, and this will allow us to conclude.
Proposition 3.2.3. Assume that
![]() $6 \in k^\times $
.
$6 \in k^\times $
.
Let
![]() $\mathfrak U=(U,\psi )$
be the tautological rank
$\mathfrak U=(U,\psi )$
be the tautological rank
![]() $2$
symplectic bundle on
$2$
symplectic bundle on
![]() $\mathrm {H}\mathbb {P}^\infty $
and x be its first Borel class. Then the following computations hold in
$\mathrm {H}\mathbb {P}^\infty $
and x be its first Borel class. Then the following computations hold in
![]() $H^*(\mathrm {H}\mathbb {P}^\infty ,\operatorname {{\underline W}})$
:
$H^*(\mathrm {H}\mathbb {P}^\infty ,\operatorname {{\underline W}})$
:
-
(1)
 $b_i^W(\operatorname {\mathrm {Sym}}^3 \mathfrak U)=\begin {cases} (-3+\langle 3\rangle ).x, & i=1,\\ (-4+\langle 3\rangle ).x^2, & i=2, \\ 0, & i>2. \end {cases}$
$b_i^W(\operatorname {\mathrm {Sym}}^3 \mathfrak U)=\begin {cases} (-3+\langle 3\rangle ).x, & i=1,\\ (-4+\langle 3\rangle ).x^2, & i=2, \\ 0, & i>2. \end {cases}$
-
(2)
 $b_i^W(\mathfrak U^{\otimes ,3})=\begin {cases} 0, & i=1,\\ -6.x^2, & i=2, \\ -8.x^3, & i=3, \\ -3.x^4, & i=4. \end {cases}$
$b_i^W(\mathfrak U^{\otimes ,3})=\begin {cases} 0, & i=1,\\ -6.x^2, & i=2, \\ -8.x^3, & i=3, \\ -3.x^4, & i=4. \end {cases}$
Proof. Our main tool will be the following computation of symplectic bundles (A.0.5.a)Footnote 12:
Note that applying point (2) of Proposition 2.2.9, we get
We start with the proof of (1). We first do the computation of the left-hand column. The symplectic bundle
![]() $\operatorname {\mathrm {Sym}}^3\mathfrak U$
is of rank
$\operatorname {\mathrm {Sym}}^3\mathfrak U$
is of rank
![]() $4$
, so that we already get the required vanishing (by definition, see (2.2.4.a)). We apply the symplectic splitting principle to that bundle (Paragraph 2.2.6): in particular, after pullback along an affine morphism
$4$
, so that we already get the required vanishing (by definition, see (2.2.4.a)). We apply the symplectic splitting principle to that bundle (Paragraph 2.2.6): in particular, after pullback along an affine morphism
![]() $p:X' \rightarrow \mathrm {H}\mathbb {P}^\infty $
,
$p:X' \rightarrow \mathrm {H}\mathbb {P}^\infty $
,
![]() $\operatorname {\mathrm {Sym}}^3\mathfrak U$
admits a splitting as two rank
$\operatorname {\mathrm {Sym}}^3\mathfrak U$
admits a splitting as two rank
![]() $2$
symplectic bundles, whose Euler classes we denote respectively
$2$
symplectic bundles, whose Euler classes we denote respectively
![]() $\alpha $
and
$\alpha $
and
![]() $\beta $
. Pulling back the decomposition (3.2.3.a) to
$\beta $
. Pulling back the decomposition (3.2.3.a) to
![]() $X'$
yields a decomposition of
$X'$
yields a decomposition of
![]() $\mathfrak U^{\otimes ,3}$
into a sum of four rank
$\mathfrak U^{\otimes ,3}$
into a sum of four rank
![]() $2$
symplectic bundles and applying the Whitney sum formula of Paragraph 2.2.4, we get
$2$
symplectic bundles and applying the Whitney sum formula of Paragraph 2.2.4, we get
Because we already know
![]() $F_1^W$
and
$F_1^W$
and
![]() $F_2^W$
(Paragraph 3.2.1 and relation (3.2.2.a)), we obtain the following equation by computing the coefficients of t and
$F_2^W$
(Paragraph 3.2.1 and relation (3.2.2.a)), we obtain the following equation by computing the coefficients of t and
![]() $t^2$
:
$t^2$
:
 $$ \begin{align*} \alpha+\beta+(\langle 2\rangle+\langle 6\rangle).x & = 0 \\ \alpha\beta+(\alpha+\beta)(\langle 2\rangle + \langle 6\rangle).x+\langle 3\rangle.x^2 &=-6.x^2. \end{align*} $$
$$ \begin{align*} \alpha+\beta+(\langle 2\rangle+\langle 6\rangle).x & = 0 \\ \alpha\beta+(\alpha+\beta)(\langle 2\rangle + \langle 6\rangle).x+\langle 3\rangle.x^2 &=-6.x^2. \end{align*} $$
Using the relation satisfied by the Borel roots
![]() $\alpha $
and
$\alpha $
and
![]() $\beta $
, we deduce that
$\beta $
, we deduce that
To conclude the proof of (1), it therefore suffices to show the following equality in
![]() $W(k)$
:
$W(k)$
:
We need only to prove it either for a finite field (if k is of positive characteristic) or for
![]() $\mathbb Q$
in case k is of characteristic zero. The first case is obvious because both forms have the same rank and same discriminant and the second case is obtained via a comparison of residues.
$\mathbb Q$
in case k is of characteristic zero. The first case is obvious because both forms have the same rank and same discriminant and the second case is obtained via a comparison of residues.
To get (2), it suffices now to finish the computation of (3.2.3.b). But using the computation above, we obtain
Then, an easy computation allows us to conclude.
3.2.4. The case
![]() $l=3$
and
$l=3$
and
![]() $l=4$
of point (2) in the preceding computation together with relations (3.2.2.b) and (3.2.2.c) yields
$l=4$
of point (2) in the preceding computation together with relations (3.2.2.b) and (3.2.2.c) yields
Let us write the final result for the FTL associated with
![]() ${\mathbf H} \operatorname {{\underline W}}$
:
${\mathbf H} \operatorname {{\underline W}}$
:
 $$ \begin{align} F_t^{{\mathbf H} \operatorname{{\underline W}}}(x,y,z)=-2\sigma(x^2).t^2-8.xyz.t^3+\lbrack\sigma(x^4)-2\sigma(x^2y^2)\rbrack.t^4. \end{align} $$
$$ \begin{align} F_t^{{\mathbf H} \operatorname{{\underline W}}}(x,y,z)=-2\sigma(x^2).t^2-8.xyz.t^3+\lbrack\sigma(x^4)-2\sigma(x^2y^2)\rbrack.t^4. \end{align} $$
3.3 Final case
We will now assemble our knowledge of ternary laws in Chow groups (Proposition 2.3.8), represented over k by the unramified Milnor K-theory
![]() ${\mathbf H}\, \mathrm {K}^{\mathrm {M}}$
, and Witt cohomology from the preceding section. Indeed, recall that there is canonical map of sheaves
${\mathbf H}\, \mathrm {K}^{\mathrm {M}}$
, and Witt cohomology from the preceding section. Indeed, recall that there is canonical map of sheaves
We use the following lemma.
Lemma 3.3.1. Let
![]() $P=(\mathrm {H}\mathbb {P}^\infty _k)^3$
. Then the morphism induced by
$P=(\mathrm {H}\mathbb {P}^\infty _k)^3$
. Then the morphism induced by
![]() $\varphi $
on cohomology
$\varphi $
on cohomology
 $$ \begin{align*}\varphi_*:\widetilde{\mathrm{CH}}{}^*(P) \rightarrow \operatorname{\mathrm{CH}}^*(P) \oplus H^*(P,\operatorname{\mathrm{W}}) \end{align*} $$
$$ \begin{align*}\varphi_*:\widetilde{\mathrm{CH}}{}^*(P) \rightarrow \operatorname{\mathrm{CH}}^*(P) \oplus H^*(P,\operatorname{\mathrm{W}}) \end{align*} $$
is injective.
The proof immediately follows from the symplectic projective bundle formula 2.2.3 and the fact that
![]() $\varphi $
is compatible with the
$\varphi $
is compatible with the
![]() $\mathrm {Sp}$
-orientations on each ring spectra.
$\mathrm {Sp}$
-orientations on each ring spectra.
This lemma allows us to combine the computations obtained for
![]() ${\mathbf H}\, \mathrm {K}^{\mathrm {M}}$
and
${\mathbf H}\, \mathrm {K}^{\mathrm {M}}$
and
![]() ${\mathbf H} \operatorname {{\underline W}}$
, respectively.
${\mathbf H} \operatorname {{\underline W}}$
, respectively.
Theorem 3.3.2. Let k be a field such that
![]() $6 \in k^\times $
. Then the ternary laws associated with the
$6 \in k^\times $
. Then the ternary laws associated with the
![]() $\mathrm {Sp}$
-oriented ring spectra
$\mathrm {Sp}$
-oriented ring spectra
![]() ${\mathbf H}\, \mathrm {K}^{\mathrm {MW}}$
(Chow-Witt groups) or with
${\mathbf H}\, \mathrm {K}^{\mathrm {MW}}$
(Chow-Witt groups) or with
![]() $\mathbf {H}_{\mathrm {MW}}$
(Milnor-Witt motivic cohomology) over k are
$\mathbf {H}_{\mathrm {MW}}$
(Milnor-Witt motivic cohomology) over k are
 $$ \begin{align*}F_l(x,y,z)= \begin{cases} 2h.\sigma(x), & l=1, \\ 2(h-\epsilon).\sigma(x^2)+2h.\sigma(xy), & l=2, \\ 2h.\sigma(x^3)-2h.\sigma(x^2y)+8(2h-\epsilon).xyz, & l=3, \\ \sigma(x^4)-2h.\sigma(x^3y)+2(h-\epsilon).\sigma(x^2y^2)+2h.\sigma(x^2yz), & l=4, \end{cases} \end{align*} $$
$$ \begin{align*}F_l(x,y,z)= \begin{cases} 2h.\sigma(x), & l=1, \\ 2(h-\epsilon).\sigma(x^2)+2h.\sigma(xy), & l=2, \\ 2h.\sigma(x^3)-2h.\sigma(x^2y)+8(2h-\epsilon).xyz, & l=3, \\ \sigma(x^4)-2h.\sigma(x^3y)+2(h-\epsilon).\sigma(x^2y^2)+2h.\sigma(x^2yz), & l=4, \end{cases} \end{align*} $$
Thus, by analogy with the classical oriented case, we introduce the following definition.
Definition 3.3.3. Let A be the ring of endomorphisms of the sphere spectrum in
![]() $\operatorname {\mathrm {SH}}(\mathbb Z)$
. We define the additive ternary laws as the power series
$\operatorname {\mathrm {SH}}(\mathbb Z)$
. We define the additive ternary laws as the power series
![]() $F_l(x,y,z),\; l=1,2,3,4$
with coefficients in A defined by the formulas of the above theorem.
$F_l(x,y,z),\; l=1,2,3,4$
with coefficients in A defined by the formulas of the above theorem.
We will say that an
![]() $\mathrm {Sp}$
-oriented ring spectra
$\mathrm {Sp}$
-oriented ring spectra
![]() $\mathbb E$
has the additive ternary laws if for any scheme S in
$\mathbb E$
has the additive ternary laws if for any scheme S in
![]() $\mathscr Sch$
the associated ternary laws on
$\mathscr Sch$
the associated ternary laws on
![]() $\mathbb E_X$
are the additive ternary laws through the canonical map
$\mathbb E_X$
are the additive ternary laws through the canonical map
![]() $A \rightarrow \mathbb E^{**}(X)$
.
$A \rightarrow \mathbb E^{**}(X)$
.
Remark 3.3.4. The computations of the previous section for the unramified Witt ring spectrum
![]() ${\mathbf H} \operatorname {{\underline W}}$
over k show that
${\mathbf H} \operatorname {{\underline W}}$
over k show that
![]() ${\mathbf H} \operatorname {{\underline W}}$
has the additive ternary laws. Note, however, that
${\mathbf H} \operatorname {{\underline W}}$
has the additive ternary laws. Note, however, that
![]() $h=0$
and
$h=0$
and
![]() $\epsilon =-1$
in
$\epsilon =-1$
in
![]() $W(k)$
so that the formula simplifies to (3.2.4.c).
$W(k)$
so that the formula simplifies to (3.2.4.c).
With rational coefficients, the preceding theorem can be generalised.
Corollary 3.3.5. The
![]() $\mathrm {Sp}$
-oriented ring spectrum
$\mathrm {Sp}$
-oriented ring spectrum
![]() $\mathbf {H}_{\mathrm {MW}}\mathbb Q$
over
$\mathbf {H}_{\mathrm {MW}}\mathbb Q$
over
![]() $\mathbb Z$
has the additive ternary laws.
$\mathbb Z$
has the additive ternary laws.
Proof. One uses the decomposition
where
![]() $\nu :\operatorname {\mathrm {Spec}}{\mathbb Z} \rightarrow \operatorname {\mathrm {Spec}}{\mathbb Q}$
is the canonical open immersion (see [Reference Déglise, Fasel, Khan and Jin15, Cor. 6.2]).
$\nu :\operatorname {\mathrm {Spec}}{\mathbb Z} \rightarrow \operatorname {\mathrm {Spec}}{\mathbb Q}$
is the canonical open immersion (see [Reference Déglise, Fasel, Khan and Jin15, Cor. 6.2]).
4 Symplectic operations
4.1 Unstable and additive operations
4.1.1. Let S be any
![]() $\mathbb Z[{1}/{2}]$
-scheme. Using a method of Morel and Voevodsky, Panin and Walter proved in [Reference Panin and Walter28, Theorem 8.2] that there exists a canonical weak
$\mathbb Z[{1}/{2}]$
-scheme. Using a method of Morel and Voevodsky, Panin and Walter proved in [Reference Panin and Walter28, Theorem 8.2] that there exists a canonical weak
![]() $\mathbb A^1$
-homotopy equivalence
$\mathbb A^1$
-homotopy equivalence
Moreover, when S is regular, they also proved that one gets an isomorphism of abelian groups (we refer the reader to [Reference Schlichting and Tripathi34, Th. 1.3])
where
![]() $[-,-]$
denotes the set of morphisms in the unpointed
$[-,-]$
denotes the set of morphisms in the unpointed
![]() $\mathbb A^1$
-homotopy category over S. Concretely, to a symplectic vector bundle
$\mathbb A^1$
-homotopy category over S. Concretely, to a symplectic vector bundle
![]() $\mathfrak V$
over X of rank
$\mathfrak V$
over X of rank
![]() $2r$
the above isomorphism associates the couple
$2r$
the above isomorphism associates the couple
![]() $(\gamma _{\mathfrak V},r)$
where
$(\gamma _{\mathfrak V},r)$
where
![]() $\gamma _{\mathfrak V}:S \rightarrow BSp_S$
is the map classifying
$\gamma _{\mathfrak V}:S \rightarrow BSp_S$
is the map classifying
![]() $\mathfrak V$
and
$\mathfrak V$
and
![]() $2r$
is the rank of
$2r$
is the rank of
![]() $\mathfrak V$
.
$\mathfrak V$
.
In this section, we will use Riou’s method to classify the following ‘operations’.
Definition 4.1.2. Let
![]() $\mathbb E$
be a spectrum over a regular scheme S. Let
$\mathbb E$
be a spectrum over a regular scheme S. Let
![]() $(n,i)$
be a couple of integers. An
$(n,i)$
be a couple of integers. An
![]() $\mathrm {Sp}$
-operation (respectively additive
$\mathrm {Sp}$
-operation (respectively additive
![]() $\, \mathrm {Sp}$
-operation)
$\, \mathrm {Sp}$
-operation)
![]() $\Theta $
of degree
$\Theta $
of degree
![]() $(n,i)$
with values in
$(n,i)$
with values in
![]() $\mathbb E$
will be a morphism of presheaves of sets (respectively abelian groups) on
$\mathbb E$
will be a morphism of presheaves of sets (respectively abelian groups) on
![]() $\mathscr Sm_S$
:
$\mathscr Sm_S$
:
We denote the set (respectively abelian group) of such operations by
![]() $\operatorname {\mathrm {Hom}}_{\mathrm {Sets}}(KSp_0,\mathbb E^{n,i})$
(respectively
$\operatorname {\mathrm {Hom}}_{\mathrm {Sets}}(KSp_0,\mathbb E^{n,i})$
(respectively
![]() $\operatorname {\mathrm {Hom}}_{\mathrm {Ab}}(KSp_0,\mathbb E^{n,i})$
).
$\operatorname {\mathrm {Hom}}_{\mathrm {Ab}}(KSp_0,\mathbb E^{n,i})$
).
4.1.3. Let us now consider an
![]() $\mathrm {Sp}$
-oriented ring spectrum
$\mathrm {Sp}$
-oriented ring spectrum
![]() $\mathbb E$
over a regular scheme S. Let us put
$\mathbb E$
over a regular scheme S. Let us put
![]() $\mathbb E^{**}=\mathbb E^{**}(S)$
as a bigraded ring.
$\mathbb E^{**}=\mathbb E^{**}(S)$
as a bigraded ring.
Recall that, according to [Reference Panin and Walter28],Footnote 13 one can compute the
![]() $\mathbb E$
-cohomology of symplectic Grassmanians as
$\mathbb E$
-cohomology of symplectic Grassmanians as
 $$ \begin{align} \mathbb E^{n,i}(HGr_S)=\big(E^{**}[[b_r,r \geq 1]]\big)^{(n,i)}, \end{align} $$
$$ \begin{align} \mathbb E^{n,i}(HGr_S)=\big(E^{**}[[b_r,r \geq 1]]\big)^{(n,i)}, \end{align} $$
where the exponent on the right-hand side denotes the subgroup of elements of degree
![]() $(n,i)$
with the convention that
$(n,i)$
with the convention that
![]() $b_r$
is of degree
$b_r$
is of degree
![]() $(4r,2r)$
. Explicitly, an element of the right-hand side is a formal power series of the form
$(4r,2r)$
. Explicitly, an element of the right-hand side is a formal power series of the form
 $$ \begin{align} F=\sum_\alpha \left(a_\alpha.{\textstyle \prod}_{i \in \mathbb N^*} b_i^{\alpha(i)}\right), \end{align} $$
$$ \begin{align} F=\sum_\alpha \left(a_\alpha.{\textstyle \prod}_{i \in \mathbb N^*} b_i^{\alpha(i)}\right), \end{align} $$
where
![]() $\mathbb N^*=(\mathbb N-\{0\})$
and
$\mathbb N^*=(\mathbb N-\{0\})$
and
![]() $\alpha $
runs over the applications
$\alpha $
runs over the applications
![]() $\alpha :\mathbb N^* \rightarrow \mathbb N$
with finite support and
$\alpha :\mathbb N^* \rightarrow \mathbb N$
with finite support and
![]() $a_\alpha $
is an element of
$a_\alpha $
is an element of
![]() $\mathbb E^{**}$
of degree
$\mathbb E^{**}$
of degree
![]() $(n-4.|\alpha |,i-2.|\alpha |)$
.
$(n-4.|\alpha |,i-2.|\alpha |)$
.
Using the method of [Reference Riou30], one deduces a complete description of the
![]() $\mathrm {Sp}$
-operations with values in
$\mathrm {Sp}$
-operations with values in
![]() $\mathbb E$
.
$\mathbb E$
.
Theorem 4.1.4. Consider the above notations. Then the canonical map
 $$ \begin{align*}\operatorname{\mathrm{Hom}}_{{\mathscr H}(S)}\big(\mathbb Z \times HGr,\Omega^{\infty}(\mathbb E(i)[n])\big) \rightarrow \operatorname{\mathrm{Hom}}_{\mathrm{Sets}}(KSp_0,\mathbb E^{n,i}), \phi \mapsto \phi_* \end{align*} $$
$$ \begin{align*}\operatorname{\mathrm{Hom}}_{{\mathscr H}(S)}\big(\mathbb Z \times HGr,\Omega^{\infty}(\mathbb E(i)[n])\big) \rightarrow \operatorname{\mathrm{Hom}}_{\mathrm{Sets}}(KSp_0,\mathbb E^{n,i}), \phi \mapsto \phi_* \end{align*} $$
is bijective. Moreover, via the identification of the left-hand side with the set
![]() $\mathbb E^{n,i}(HGr_S)^{\mathbb Z}$
, a sequence of formal power series
$\mathbb E^{n,i}(HGr_S)^{\mathbb Z}$
, a sequence of formal power series
![]() $(F_r)_{r \in \mathbb Z}$
of the form (4.1.3.b) corresponds to the operation that sends a symplectic bundle
$(F_r)_{r \in \mathbb Z}$
of the form (4.1.3.b) corresponds to the operation that sends a symplectic bundle
![]() $\mathfrak V$
of rank
$\mathfrak V$
of rank
![]() $2r$
over a smooth S-scheme X to the (well-definedFootnote 14) element of
$2r$
over a smooth S-scheme X to the (well-definedFootnote 14) element of
![]() $\mathbb E^{n,i}(X)$
:
$\mathbb E^{n,i}(X)$
:
 $$ \begin{align*}\Theta_{F_*}([\mathfrak V]):=F_r\big(b_1(\mathfrak V),\ldots,b_r(\mathfrak V),0,\ldots\big), \end{align*} $$
$$ \begin{align*}\Theta_{F_*}([\mathfrak V]):=F_r\big(b_1(\mathfrak V),\ldots,b_r(\mathfrak V),0,\ldots\big), \end{align*} $$
where
![]() $b_i(\mathfrak V)$
denotes the Borel classes of
$b_i(\mathfrak V)$
denotes the Borel classes of
![]() $\mathfrak V$
(Paragraph 2.2.4).
$\mathfrak V$
(Paragraph 2.2.4).
Note, moreover, that the map corresponding to
![]() $\Theta _{F_*}$
is pointed (for the obvious base points) if and only if
$\Theta _{F_*}$
is pointed (for the obvious base points) if and only if
![]() $F_0=0$
.
$F_0=0$
.
Proof. This is a consequence of [Reference Riou30, Proposition 1.2.9] applied to the inductive system of smooth schemes
![]() $HGr_\bullet =(HGr_{n,d})_{n,d \in \mathbb N}$
and to the H-group
$HGr_\bullet =(HGr_{n,d})_{n,d \in \mathbb N}$
and to the H-group
![]() $E=\Omega ^\infty (\mathbb E(i)[n])$
. Indeed, formula (4.1.3.a) implies that “
$E=\Omega ^\infty (\mathbb E(i)[n])$
. Indeed, formula (4.1.3.a) implies that “
![]() $HGr_\bullet $
does not unveil phantoms in E” in the sense of [Reference Riou30, Definition 1.2.2]. Moreover, “
$HGr_\bullet $
does not unveil phantoms in E” in the sense of [Reference Riou30, Definition 1.2.2]. Moreover, “
![]() $\pi _0 HGr$
is generated by
$\pi _0 HGr$
is generated by
![]() $HGr$
” in the sense of [Reference Riou30, Definition 1.2.5] by application of [Reference Panin and Walter28, Theorem 8.1].
$HGr$
” in the sense of [Reference Riou30, Definition 1.2.5] by application of [Reference Panin and Walter28, Theorem 8.1].
4.1.5. According to [Reference Riou30, Lem. 6.2.2.2], for any integer
![]() $n>0$
there exists a unique group morphism, natural in commutative ring A, of the form
$n>0$
there exists a unique group morphism, natural in commutative ring A, of the form
such that
![]() $\psi ^A_n(1+a.t)=a^n$
and that vanishes on
$\psi ^A_n(1+a.t)=a^n$
and that vanishes on
![]() $1+t^{n+1}.A[[t]]$
. By analogy with the oriented case, one defines an additive
$1+t^{n+1}.A[[t]]$
. By analogy with the oriented case, one defines an additive
![]() $\mathrm {Sp}$
-operation
$\mathrm {Sp}$
-operation
![]() $\tilde {\chi }_{2n}^{\mathbb E}$
of degree
$\tilde {\chi }_{2n}^{\mathbb E}$
of degree
![]() $(4n,2n)$
with values in
$(4n,2n)$
with values in
![]() $\mathbb E$
. Given any symplectic bundle
$\mathbb E$
. Given any symplectic bundle
![]() $\mathfrak V$
on a smooth S-scheme X, one sets
$\mathfrak V$
on a smooth S-scheme X, one sets
 $$ \begin{align} \tilde{\chi}_{2n}^{\mathbb E}([\mathfrak V])=\psi_n^{\mathbb E^{**}}(b_t(\mathfrak V)), \end{align} $$
$$ \begin{align} \tilde{\chi}_{2n}^{\mathbb E}([\mathfrak V])=\psi_n^{\mathbb E^{**}}(b_t(\mathfrak V)), \end{align} $$
where
![]() $b_t(\mathfrak V)$
is the total Borel class of
$b_t(\mathfrak V)$
is the total Borel class of
![]() $\mathfrak V$
, Formula (2.2.4.b). (The latter formula shows that
$\mathfrak V$
, Formula (2.2.4.b). (The latter formula shows that
![]() $\tilde {\chi }_{2n}^{\mathbb E}$
is indeed additive.) We also put
$\tilde {\chi }_{2n}^{\mathbb E}$
is indeed additive.) We also put
![]() $\tilde {\chi }^E_0=1$
. Note in particular that when
$\tilde {\chi }^E_0=1$
. Note in particular that when
![]() $\mathfrak V=\mathfrak U$
has rank
$\mathfrak V=\mathfrak U$
has rank
![]() $2$
, one gets by construction for
$2$
, one gets by construction for
![]() $n \geq 0$
$n \geq 0$
Recall from Panin-Walter’s symplectic projective bundle theorem (Theorem 2.2.3) that one obtains an isomorphism of bigraded
![]() $\mathbb E^{**}$
-modules
$\mathbb E^{**}$
-modules
where the indeterminate b has degree
![]() $(4,2)$
. As in [Reference Riou30, Prop. 6.2.2.1], one can compute all of the additive
$(4,2)$
. As in [Reference Riou30, Prop. 6.2.2.1], one can compute all of the additive
![]() $\mathrm {Sp}$
-operations with values in
$\mathrm {Sp}$
-operations with values in
![]() $\mathbb E$
.
$\mathbb E$
.
Theorem 4.1.6. Consider the above notations. Let
![]() $\mathfrak U$
be the tautological symplectic bundle of rank
$\mathfrak U$
be the tautological symplectic bundle of rank
![]() $2$
on
$2$
on
![]() $\mathrm {H}\mathbb {P}^\infty _S$
. Then the canonical morphism of graded
$\mathrm {H}\mathbb {P}^\infty _S$
. Then the canonical morphism of graded
![]() $\mathbb E^{**}$
-modules, where b is assigned the bidegree
$\mathbb E^{**}$
-modules, where b is assigned the bidegree
![]() $(4,2)$
,
$(4,2)$
,
is an isomorphism. Moreover, for
![]() $n \geq 0$
, the additive
$n \geq 0$
, the additive
![]() $\mathrm {Sp}$
-operation
$\mathrm {Sp}$
-operation
![]() $\tilde {\chi }_{2n}^{\mathbb E}$
defined above is sent to
$\tilde {\chi }_{2n}^{\mathbb E}$
defined above is sent to
![]() $b^n$
via this isomorphism.
$b^n$
via this isomorphism.
Proof. The injectivity of the map follows from the symplectic bundle principle (Paragraph 2.2.6) and the universal property of
![]() $\mathrm {H}\mathbb {P}^\infty \simeq B\mathrm {Sp}_2$
. The last assertion follows from relation (4.1.5.b). It remains to prove surjectivity. One considers a power series
$\mathrm {H}\mathbb {P}^\infty \simeq B\mathrm {Sp}_2$
. The last assertion follows from relation (4.1.5.b). It remains to prove surjectivity. One considers a power series
![]() $\sum _n \lambda _n.b^n$
. Then the infinite sum
$\sum _n \lambda _n.b^n$
. Then the infinite sum
 $$ \begin{align*}\sum_n \lambda_n.\tilde{\chi}_{2n}^{\mathbb E} \end{align*} $$
$$ \begin{align*}\sum_n \lambda_n.\tilde{\chi}_{2n}^{\mathbb E} \end{align*} $$
gives a well-defined additive operation. Indeed, given any scheme X and a symplectic bundle
![]() $\mathfrak V$
over X, it follows from the nilpotency of Borel classes ([Reference Panin and Walter26, Theorem 8.6]) that there exists an integer
$\mathfrak V$
over X, it follows from the nilpotency of Borel classes ([Reference Panin and Walter26, Theorem 8.6]) that there exists an integer
![]() $N>0$
such that for all
$N>0$
such that for all
![]() $n>N$
,
$n>N$
,
![]() $\chi _{2n}^{\mathbb E}(\mathfrak V)=0$
.
$\chi _{2n}^{\mathbb E}(\mathfrak V)=0$
.
4.1.7. The lemma of Riou used in the above proof is a smart way of constructing
![]() $\tilde {\chi }_{2n}$
. A more classical way is to use the symplectic splitting principle 2.2.6. Indeed,
$\tilde {\chi }_{2n}$
. A more classical way is to use the symplectic splitting principle 2.2.6. Indeed,
![]() $\tilde {\chi }_{2n}(\mathfrak V)$
is uniquely defined in terms of the Borel roots
$\tilde {\chi }_{2n}(\mathfrak V)$
is uniquely defined in terms of the Borel roots
![]() $\xi _i$
of
$\xi _i$
of
![]() $\mathfrak V$
by the property
$\mathfrak V$
by the property
 $$ \begin{align*}\tilde{\chi}_{2n}(\mathfrak V)=\sum_i \xi_i^n. \end{align*} $$
$$ \begin{align*}\tilde{\chi}_{2n}(\mathfrak V)=\sum_i \xi_i^n. \end{align*} $$
Thus, one can express
![]() $\tilde {\chi }_{2n}(\mathfrak V)$
in terms of the Borel classes of
$\tilde {\chi }_{2n}(\mathfrak V)$
in terms of the Borel classes of
![]() $\mathfrak V$
using the fact that
$\mathfrak V$
using the fact that
![]() $b_i=b_i(\mathfrak V)$
is the ith elementary symmetric function in the
$b_i=b_i(\mathfrak V)$
is the ith elementary symmetric function in the
![]() $\xi _i$
and using the classical expression of the symmetric power sum polynomials in terms of the elementary symmetric functions. For example, a classical formula in terms of determinant (see [Reference Macdonald and Zelevinsky22, I.2, p. 28]) is
$\xi _i$
and using the classical expression of the symmetric power sum polynomials in terms of the elementary symmetric functions. For example, a classical formula in terms of determinant (see [Reference Macdonald and Zelevinsky22, I.2, p. 28]) is

A more useful formula is given by Newton’s relations:
Example 4.1.8. Let us assume that either k is a perfect field k and
![]() $R=\mathbb Z$
or
$R=\mathbb Z$
or
![]() $k=\mathbb Z[{1}/{2}]$
and
$k=\mathbb Z[{1}/{2}]$
and
![]() $R=\mathbb Q$
. Given any k-scheme X, we define the (Eilenberg-MacLane) Milnor-Witt
$R=\mathbb Q$
. Given any k-scheme X, we define the (Eilenberg-MacLane) Milnor-Witt
![]() $(n,i)$
-space over S by the formula
$(n,i)$
-space over S by the formula
 $$ \begin{align*}K(\tilde R_S(i),n)=\Omega^\infty\big(\mathbf{H}_{\mathrm{MW}} R_S(i)[n]\big). \end{align*} $$
$$ \begin{align*}K(\tilde R_S(i),n)=\Omega^\infty\big(\mathbf{H}_{\mathrm{MW}} R_S(i)[n]\big). \end{align*} $$
It is the analogue of the
![]() $(n,i)$
th Eilenberg-MacLane motivic space
$(n,i)$
th Eilenberg-MacLane motivic space
![]() $K(R(i),n)$
(see [Reference Voevodsky39, §6.1] for
$K(R(i),n)$
(see [Reference Voevodsky39, §6.1] for
![]() $(n,i)=(2n,n)$
). According to the absolute purity theorem for Milnor-Witt motivic cohomology (see [Reference Déglise, Fasel, Khan and Jin15]), this space is stable under arbitrary pullback between regular k-schemes.
$(n,i)=(2n,n)$
). According to the absolute purity theorem for Milnor-Witt motivic cohomology (see [Reference Déglise, Fasel, Khan and Jin15]), this space is stable under arbitrary pullback between regular k-schemes.
The previous theorem applied to the
![]() $\mathrm {Sp}$
-oriented ring spectrum
$\mathrm {Sp}$
-oriented ring spectrum
![]() $\mathbf {H}_{\mathrm {MW}} R_S$
gives a canonical additive
$\mathbf {H}_{\mathrm {MW}} R_S$
gives a canonical additive
![]() $\mathrm {Sp}$
-operation of degree
$\mathrm {Sp}$
-operation of degree
![]() $(2n,n)$
:
$(2n,n)$
:
 $$ \begin{align*}\tilde{\chi}^R_{2n}:KSp_0 \rightarrow H_{MW}^{4n,2n}(-,R) \simeq \widetilde{\mathrm{CH}}{}^{2n}_R, \end{align*} $$
$$ \begin{align*}\tilde{\chi}^R_{2n}:KSp_0 \rightarrow H_{MW}^{4n,2n}(-,R) \simeq \widetilde{\mathrm{CH}}{}^{2n}_R, \end{align*} $$
the last isomorphism being (1.0.0.c) and (1.0.0.a). It corresponds to a morphism of H-groups
 $$ \begin{align*}\mathbb Z \times B\mathrm{Sp}_S \rightarrow K(\tilde R_S(2n),4n). \end{align*} $$
$$ \begin{align*}\mathbb Z \times B\mathrm{Sp}_S \rightarrow K(\tilde R_S(2n),4n). \end{align*} $$
These operations form a base of all the additive
![]() $\mathrm {Sp}$
-operations over S with values in Chow-Witt groups
$\mathrm {Sp}$
-operations over S with values in Chow-Witt groups
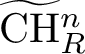 $\widetilde {\mathrm {CH}}{}^n_R$
.
$\widetilde {\mathrm {CH}}{}^n_R$
.
4.2 Stable operations and Hermitian K-theory
4.2.1. We now study stable operations over a regular scheme S, still following the method of Riou. A technical difference between symplectic K-theory and usual K-theory is that the former is
![]() $(8,4)$
-periodic, whereas the latter is
$(8,4)$
-periodic, whereas the latter is
![]() $(2,1)$
periodic. Therefore, the natural sphere that comes into play is
$(2,1)$
periodic. Therefore, the natural sphere that comes into play is
![]() .Footnote 15
.Footnote 15
Given any spectrum
![]() $\mathbb E$
over S, we get a tautological stability isomorphism for any smooth S-scheme X:
$\mathbb E$
over S, we get a tautological stability isomorphism for any smooth S-scheme X:
 $$ \begin{align*}\sigma^{\mathbb E}:\mathbb E^{n-8,i-4}(X) \xrightarrow \sim \tilde{\mathbb E}^{n,i}(H \wedge X_+), \end{align*} $$
$$ \begin{align*}\sigma^{\mathbb E}:\mathbb E^{n-8,i-4}(X) \xrightarrow \sim \tilde{\mathbb E}^{n,i}(H \wedge X_+), \end{align*} $$
where
![]() $\tilde {\mathbb E}$
is the associated reduced cohomology theory. When
$\tilde {\mathbb E}$
is the associated reduced cohomology theory. When
![]() $\mathbb E$
admits a ring structure, this isomorphism can be expressed by the cup-product with a tautological class
$\mathbb E$
admits a ring structure, this isomorphism can be expressed by the cup-product with a tautological class
![]() $\sigma _X \in \tilde {\mathbb E}^{8,4}(H)$
. When
$\sigma _X \in \tilde {\mathbb E}^{8,4}(H)$
. When
![]() $\mathbb E$
is in addition
$\mathbb E$
is in addition
![]() $\mathrm {Sp}$
-oriented, this class is induced by
$\mathrm {Sp}$
-oriented, this class is induced by
![]() $b_1(\mathfrak U_1) \cdot b_1(\mathfrak U_2) \in \mathbb E^{8,4}(\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1)$
, where
$b_1(\mathfrak U_1) \cdot b_1(\mathfrak U_2) \in \mathbb E^{8,4}(\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1)$
, where
![]() $\mathfrak U_i$
is the pullback of the tautological symplectic bundle on the ith factor (apply Remark 2.2.5).
$\mathfrak U_i$
is the pullback of the tautological symplectic bundle on the ith factor (apply Remark 2.2.5).
Similarly, the multiplication map in symplectic K-theory
 $$ \begin{align*}\mathbf{KSp}_0(X) \xrightarrow{(\mathfrak U_i-\mathfrak H) \cdot (\mathfrak U_i-\mathfrak H)} \mathbf{KSp}_0(\mathrm{H}\mathbb{P}^1 \times \mathrm{H}\mathbb{P}^1 \times X) \end{align*} $$
$$ \begin{align*}\mathbf{KSp}_0(X) \xrightarrow{(\mathfrak U_i-\mathfrak H) \cdot (\mathfrak U_i-\mathfrak H)} \mathbf{KSp}_0(\mathrm{H}\mathbb{P}^1 \times \mathrm{H}\mathbb{P}^1 \times X) \end{align*} $$
induces, according to the symplectic bundle theorem, an isomorphism
 $$ \begin{align*}\sigma^{\mathbf{KSp}}:\mathbf{KSp}_0(X) \rightarrow\tilde{\mathbf{KSp}}_0(H \wedge X_+). \end{align*} $$
$$ \begin{align*}\sigma^{\mathbf{KSp}}:\mathbf{KSp}_0(X) \rightarrow\tilde{\mathbf{KSp}}_0(H \wedge X_+). \end{align*} $$
Given an
![]() $\mathrm {Sp}$
-operation
$\mathrm {Sp}$
-operation
![]() $\theta $
with values in a spectrum
$\theta $
with values in a spectrum
![]() $\mathbb E$
of degree
$\mathbb E$
of degree
![]() $(n,i)$
, we define a new associated
$(n,i)$
, we define a new associated
![]() $\mathrm {Sp}$
-operation
$\mathrm {Sp}$
-operation
![]() $\omega _H(\theta )$
with values in
$\omega _H(\theta )$
with values in
![]() $\mathbb E$
of degree
$\mathbb E$
of degree
![]() $(n-8,i-4)$
by the following commutative diagram:
$(n-8,i-4)$
by the following commutative diagram:

In particular, we get two projective systems indexed by integers
![]() $r \geq 0$
:
$r \geq 0$
:
 $$ \begin{align*} \ldots \operatorname{\mathrm{Hom}}_{\mathrm{Set}}\big(KSp_0,\mathbb E^{n+8r,i+4r}\big) \xleftarrow{\omega_H} \operatorname{\mathrm{Hom}}_{\mathrm{Set}}\big(KSp_0,\mathbb E^{n+8r+8,i+4r+4}\big) \ldots \\ \ldots \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}\big(KSp_0,\mathbb E^{n+8r,i+4r}\big) \xleftarrow{\omega_H} \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}\big(KSp_0,\mathbb E^{n+8r+8,i+4r+4}\big) \ldots \end{align*} $$
$$ \begin{align*} \ldots \operatorname{\mathrm{Hom}}_{\mathrm{Set}}\big(KSp_0,\mathbb E^{n+8r,i+4r}\big) \xleftarrow{\omega_H} \operatorname{\mathrm{Hom}}_{\mathrm{Set}}\big(KSp_0,\mathbb E^{n+8r+8,i+4r+4}\big) \ldots \\ \ldots \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}\big(KSp_0,\mathbb E^{n+8r,i+4r}\big) \xleftarrow{\omega_H} \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}\big(KSp_0,\mathbb E^{n+8r+8,i+4r+4}\big) \ldots \end{align*} $$
Their projective limits agree as stable operations must be additive (see [Reference Vishik38, Proposition 3.5]).
Definition 4.2.2. Consider the above notations. We define the abelian group of stable
![]() $\mathrm {Sp}$
-operations with values in
$\mathrm {Sp}$
-operations with values in
![]() $\mathbb E$
of degree
$\mathbb E$
of degree
![]() $(n,i)$
as the projective limit of one of the two projective system above:
$(n,i)$
as the projective limit of one of the two projective system above:
 $$ \begin{align*} \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\mathbb E^{n,i}\big) &=\lim_{r \in \mathbb N} \operatorname{\mathrm{Hom}}_{\mathrm{Sets}}\big(KSp_0,\mathbb E^{n+8r,i+4r}\big) \\ &\simeq \lim_{r \in \mathbb N} \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}\big(KSp_0,\mathbb E^{n+8r,i+4r}\big). \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\mathbb E^{n,i}\big) &=\lim_{r \in \mathbb N} \operatorname{\mathrm{Hom}}_{\mathrm{Sets}}\big(KSp_0,\mathbb E^{n+8r,i+4r}\big) \\ &\simeq \lim_{r \in \mathbb N} \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}\big(KSp_0,\mathbb E^{n+8r,i+4r}\big). \end{align*} $$
In particular, such an operation is a sequence
![]() $(\Theta _r)_{r \in \mathbb N}$
of additive
$(\Theta _r)_{r \in \mathbb N}$
of additive
![]() $\mathrm {Sp}$
-operations
$\mathrm {Sp}$
-operations
![]() $\Theta _r:\mathbf {KSp} \rightarrow \mathbb E^{n+8r,i+4r}$
such that for any
$\Theta _r:\mathbf {KSp} \rightarrow \mathbb E^{n+8r,i+4r}$
such that for any
![]() $r \geq 0$
,
$r \geq 0$
,
![]() $\Theta _r=\omega _H(\Theta _{r+1})$
.
$\Theta _r=\omega _H(\Theta _{r+1})$
.
4.2.3. Recall from [Reference Riou29, 6.1] that a naive H-spectrum over a scheme S is the datum of a sequence
![]() $(E_n,\sigma _n)_{n \in \mathbb N}$
such that
$(E_n,\sigma _n)_{n \in \mathbb N}$
such that
![]() $E_n$
is an object of
$E_n$
is an object of
![]() ${{\mathscr H}_{\bullet }}(S)$
and
${{\mathscr H}_{\bullet }}(S)$
and
![]() $\sigma _n:H \wedge E_n \rightarrow E_n$
is a map in
$\sigma _n:H \wedge E_n \rightarrow E_n$
is a map in
![]() ${{\mathscr H}_{\bullet }}(S)$
whose adjoint map
${{\mathscr H}_{\bullet }}(S)$
whose adjoint map
![]() $E_n \rightarrow \Omega _H E_n$
is an isomorphism. Every spectrum
$E_n \rightarrow \Omega _H E_n$
is an isomorphism. Every spectrum
![]() $\mathbb E$
if
$\mathbb E$
if
![]() $\operatorname {\mathrm {SH}}(S)$
determines a naive H-spectrum whose nth term is
$\operatorname {\mathrm {SH}}(S)$
determines a naive H-spectrum whose nth term is
![]() $E_n=\Omega ^\infty \Sigma _H^n \mathbb E$
. Reciprocally, any naive H-spectrum
$E_n=\Omega ^\infty \Sigma _H^n \mathbb E$
. Reciprocally, any naive H-spectrum
![]() $(E_n,\sigma _n)$
admits a lifting to an object of
$(E_n,\sigma _n)$
admits a lifting to an object of
![]() $\operatorname {\mathrm {SH}}(S)$
, and the lifting is unique provided
$\operatorname {\mathrm {SH}}(S)$
, and the lifting is unique provided
 $$ \begin{align*}\mathbf{R}^1 \lim_{n \in \mathbb N} \operatorname{\mathrm{Hom}}_{{{\mathscr H}_{\bullet}}(S)}(S^1 \wedge E_n,E_n)=0. \end{align*} $$
$$ \begin{align*}\mathbf{R}^1 \lim_{n \in \mathbb N} \operatorname{\mathrm{Hom}}_{{{\mathscr H}_{\bullet}}(S)}(S^1 \wedge E_n,E_n)=0. \end{align*} $$
An important example for us is provided by the naive H-spectrum over any
![]() $\mathbb Z[{1}/{2}]$
-scheme S,
$\mathbb Z[{1}/{2}]$
-scheme S,
whose transition maps are all equal to the multiplication by the element
![]() $\sigma ^2 \in [H,\mathbb Z\times B\mathrm {Sp}]_*$
corresponding to the element
$\sigma ^2 \in [H,\mathbb Z\times B\mathrm {Sp}]_*$
corresponding to the element
![]() $[\mathfrak U_1-\mathfrak H].[\mathfrak U_2-\mathfrak H]$
in
$[\mathfrak U_1-\mathfrak H].[\mathfrak U_2-\mathfrak H]$
in
![]() $\mathbf {KSp}_0(\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1)$
, where
$\mathbf {KSp}_0(\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1)$
, where
![]() $\mathfrak U_i$
is the tautological symplectic bundle on the ith factor of
$\mathfrak U_i$
is the tautological symplectic bundle on the ith factor of
![]() $\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1$
. As explained above, this naive H-spectrum lifts as an object of
$\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1$
. As explained above, this naive H-spectrum lifts as an object of
![]() $\operatorname {\mathrm {SH}}(S)$
. Over
$\operatorname {\mathrm {SH}}(S)$
. Over
![]() $S=\operatorname {\mathrm {Spec}}{\mathbb Z[{1}/{2}]}$
, the ambiguity in this lifting vanishes according to [Reference Panin and Walter28, Theorem 13.2], thus giving a canonical spectrum
$S=\operatorname {\mathrm {Spec}}{\mathbb Z[{1}/{2}]}$
, the ambiguity in this lifting vanishes according to [Reference Panin and Walter28, Theorem 13.2], thus giving a canonical spectrum
![]() $\mathbf {KSp}_{\mathbb Z[{1}/{2}]}$
. Over an arbitrary
$\mathbf {KSp}_{\mathbb Z[{1}/{2}]}$
. Over an arbitrary
![]() $\mathbb Z[{1}/{2}]$
-scheme S, we define
$\mathbb Z[{1}/{2}]$
-scheme S, we define
![]() $\mathbf {KSp}_S$
by pullback. According to [Reference Schlichting and Tripathi34], one gets a canonical isomorphism in
$\mathbf {KSp}_S$
by pullback. According to [Reference Schlichting and Tripathi34], one gets a canonical isomorphism in
![]() $\operatorname {\mathrm {SH}}(S)$
:
$\operatorname {\mathrm {SH}}(S)$
:
Let us recall the following proposition from [Reference Riou29, Lem. 6.4].
Proposition 4.2.4. Given any spectrum
![]() $\mathbb E$
over S, there is a short exact sequence
$\mathbb E$
over S, there is a short exact sequence
 $$ \begin{align*} 0 \rightarrow &\mathbf{R}^1 \lim_{r \in \mathbb N} \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}(KSp_0,\mathbb E^{n+8r-1,i+4r}) & \longrightarrow \operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\big(\mathbf{KSp}_S,\mathbb E(i)[n]\big) \\ & \longrightarrow \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\mathbb E^{n,i}\big) \rightarrow 0, \end{align*} $$
$$ \begin{align*} 0 \rightarrow &\mathbf{R}^1 \lim_{r \in \mathbb N} \operatorname{\mathrm{Hom}}_{\mathrm{Ab}}(KSp_0,\mathbb E^{n+8r-1,i+4r}) & \longrightarrow \operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\big(\mathbf{KSp}_S,\mathbb E(i)[n]\big) \\ & \longrightarrow \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\mathbb E^{n,i}\big) \rightarrow 0, \end{align*} $$
where the transition maps in the left-hand side projective system are given by the desuspension maps
![]() $\omega _H$
(Paragraph 4.2.1).
$\omega _H$
(Paragraph 4.2.1).
4.2.5. Let k be a perfect field of characteristic different from
![]() $2$
. According to [Reference Déglise and Fasel13, Proposition 4.1.2], we get the following vanishing of the Milnor-Witt motivic cohomology groups of k with integral coefficients:
$2$
. According to [Reference Déglise and Fasel13, Proposition 4.1.2], we get the following vanishing of the Milnor-Witt motivic cohomology groups of k with integral coefficients:
Regarding rational motivic cohomology, we get from [Reference Déglise, Fasel, Khan and Jin15] that
 $$ \begin{align*}H^{n,m}_{MW}(\mathbb Z[{1}/{2}],\mathbb Q)=K_{2m-n}^{(m)}(\mathbb Z[{1}/{2}]) \otimes \mathbb Q \oplus H^{n-m}_{\mathrm{Zar}}(\mathbb Q,{\mathbf H} \operatorname{{\underline W}} \otimes \mathbb Q), \end{align*} $$
$$ \begin{align*}H^{n,m}_{MW}(\mathbb Z[{1}/{2}],\mathbb Q)=K_{2m-n}^{(m)}(\mathbb Z[{1}/{2}]) \otimes \mathbb Q \oplus H^{n-m}_{\mathrm{Zar}}(\mathbb Q,{\mathbf H} \operatorname{{\underline W}} \otimes \mathbb Q), \end{align*} $$
where
![]() ${\mathbf H} \operatorname {{\underline W}}$
is the unramified Witt sheaf over
${\mathbf H} \operatorname {{\underline W}}$
is the unramified Witt sheaf over
![]() $\mathscr Sm_{\mathbb Q}$
. Given Borel’s computations of the K-theory of integers, these groups vanish in the same range as in the previous case.Footnote 16
$\mathscr Sm_{\mathbb Q}$
. Given Borel’s computations of the K-theory of integers, these groups vanish in the same range as in the previous case.Footnote 16
Corollary 4.2.6. Assume that we are in one of the following cases:
-
• S is the spectrum of a perfect field k of characteristic not
 $2$
, and
$2$
, and
 $R=\mathbb Z$
;
$R=\mathbb Z$
; -
• S is the spectrum of
 $\mathbb Z[{1}/{2}]$
, and
$\mathbb Z[{1}/{2}]$
, and
 $R=\mathbb Q$
.
$R=\mathbb Q$
.
Then for any integer n, the following canonical map, appearing in the previous proposition, is an isomorphism:
 $$ \begin{align*}\operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\Big(\mathbf{KSp}_S,\mathbf{H}_{\mathrm{MW}} R(2n)[4n]\Big) \xrightarrow \sim \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\widetilde{\mathrm{CH}}{}^{4n}_R\big). \end{align*} $$
$$ \begin{align*}\operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\Big(\mathbf{KSp}_S,\mathbf{H}_{\mathrm{MW}} R(2n)[4n]\Big) \xrightarrow \sim \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\widetilde{\mathrm{CH}}{}^{4n}_R\big). \end{align*} $$
4.2.7. Indeed, in view of the vanishing recalled before the corollary, the projective system appearing on the left-hand side of the short exact sequence in the previous section is just
![]() $0$
at each degree. Besides, one can give another expression for the projective system of the right-hand side appearing in Definition 4.2.2. Using Theorem 4.1.6, the above vanishing and the fact that
$0$
at each degree. Besides, one can give another expression for the projective system of the right-hand side appearing in Definition 4.2.2. Using Theorem 4.1.6, the above vanishing and the fact that
 $$ \begin{align*}H^{0,0}_{MW}(S,R) \simeq GW(S)_R, \end{align*} $$
$$ \begin{align*}H^{0,0}_{MW}(S,R) \simeq GW(S)_R, \end{align*} $$
we get that the two above groups are given by the projective limit of a tower, indexed by
![]() $r \geq 0$
, of the form
$r \geq 0$
, of the form
 $$ \begin{align} \ldots \leftarrow \operatorname{\mathrm{GW}}(S)_R.\tilde{\chi}^R_{2n+4r} \xleftarrow{\omega_H} \operatorname{\mathrm{GW}}(S)_R.\tilde{\chi}^R_{2n+4r+4} \leftarrow \ldots \end{align} $$
$$ \begin{align} \ldots \leftarrow \operatorname{\mathrm{GW}}(S)_R.\tilde{\chi}^R_{2n+4r} \xleftarrow{\omega_H} \operatorname{\mathrm{GW}}(S)_R.\tilde{\chi}^R_{2n+4r+4} \leftarrow \ldots \end{align} $$
where we have denoted by
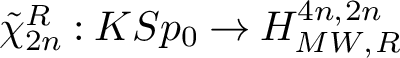 $\tilde {\chi }^R_{2n}:KSp_0 \rightarrow H_{MW,R}^{4n,2n}$
the additive
$\tilde {\chi }^R_{2n}:KSp_0 \rightarrow H_{MW,R}^{4n,2n}$
the additive
![]() $\mathrm {Sp}$
-operations of Example 4.1.8. Note that the desuspension operators
$\mathrm {Sp}$
-operations of Example 4.1.8. Note that the desuspension operators
![]() $\omega _H$
(Paragraph 4.2.1) are
$\omega _H$
(Paragraph 4.2.1) are
![]() $GW(S)$
-linear (because all morphisms involved in Diagram (4.2.1.a) are
$GW(S)$
-linear (because all morphisms involved in Diagram (4.2.1.a) are
![]() $GW(S)$
-linear). The next subsection is devoted to computing explicitly the transition maps in the above projective system.
$GW(S)$
-linear). The next subsection is devoted to computing explicitly the transition maps in the above projective system.
4.3 Stabilisation for Milnor-Witt motivic cohomology
4.3.1. Consider the notations and assumptions of Corollary 4.2.6 and Paragraph 4.2.7. According to formula (4.2.7.a), we know a priori that for any
![]() $n \geq 0$
there exists an isomorphism class of quadratic form
$n \geq 0$
there exists an isomorphism class of quadratic form
![]() $\psi _{2n+4} \in \operatorname {\mathrm {GW}}(S)_R$
such that
$\psi _{2n+4} \in \operatorname {\mathrm {GW}}(S)_R$
such that
 $$ \begin{align} \omega_H\left(\tilde{\chi}_{2n+4}^R\right)=\psi_{2n+4}.\tilde{\chi}_{2n}^R. \end{align} $$
$$ \begin{align} \omega_H\left(\tilde{\chi}_{2n+4}^R\right)=\psi_{2n+4}.\tilde{\chi}_{2n}^R. \end{align} $$
For normalisation purposes, we put
![]() $\psi _0=\psi _2=1$
.
$\psi _0=\psi _2=1$
.
Theorem 4.3.2. Consider the above notations. We assume one of the following hypotheses:
-
(a) S is the spectrum of a perfect field k such that
 $6 \in k^\times $
, and
$6 \in k^\times $
, and
 $R=\mathbb Z$
;
$R=\mathbb Z$
; -
(b) S is the spectrum of
 $\mathbb Z[{1}/{2}]$
, and
$\mathbb Z[{1}/{2}]$
, and
 $R=\mathbb Q$
.
$R=\mathbb Q$
.
Then for any integer
![]() $n \geq 0$
the quadratic form appearing in relation (4.3.1.a) is
$n \geq 0$
the quadratic form appearing in relation (4.3.1.a) is
 $$ \begin{align} \psi_{2n+4}=\begin{cases} \frac 12(2n+4)(2n+3)(2n+2)(2n+1).h & \text{if }n\geq 0 \text{is even,} \\ (2n+4)(2n+2).\big((2n^2+4n+1).h-\epsilon\big) & \text{if }n \text{is odd.} \\ \end{cases} \end{align} $$
$$ \begin{align} \psi_{2n+4}=\begin{cases} \frac 12(2n+4)(2n+3)(2n+2)(2n+1).h & \text{if }n\geq 0 \text{is even,} \\ (2n+4)(2n+2).\big((2n^2+4n+1).h-\epsilon\big) & \text{if }n \text{is odd.} \\ \end{cases} \end{align} $$
Proof. To determine an additive
![]() $\mathrm {Sp}$
-operation over S, we know from Theorem 4.1.6 that we need only to apply it to the element
$\mathrm {Sp}$
-operation over S, we know from Theorem 4.1.6 that we need only to apply it to the element
![]() $[\mathfrak U] \in KSp_0(\mathrm {H}\mathbb {P}^\infty _S)$
. Going back to the defining diagram (4.2.1.a) for
$[\mathfrak U] \in KSp_0(\mathrm {H}\mathbb {P}^\infty _S)$
. Going back to the defining diagram (4.2.1.a) for
![]() $\omega _H$
and using the fact that
$\omega _H$
and using the fact that
![]() $\tilde {\chi }_{2n}^R(\mathfrak U)=b_1(\mathfrak U)^n=u^n$
, we get the following relation in the cohomology group
$\tilde {\chi }_{2n}^R(\mathfrak U)=b_1(\mathfrak U)^n=u^n$
, we get the following relation in the cohomology group
 $H_{MW}^{4n+8,2n+4}(\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^\infty _S)$
:
$H_{MW}^{4n+8,2n+4}(\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^\infty _S)$
:
 $$ \begin{align} \tilde{\chi}_{2n+4}^R\big((\mathfrak U_1-\mathfrak H)(\mathfrak U_2-\mathfrak H)\mathfrak U\big)=\psi_{2n+4}.u_1u_2u^n, \end{align} $$
$$ \begin{align} \tilde{\chi}_{2n+4}^R\big((\mathfrak U_1-\mathfrak H)(\mathfrak U_2-\mathfrak H)\mathfrak U\big)=\psi_{2n+4}.u_1u_2u^n, \end{align} $$
where we denoted
![]() $u_1$
and
$u_1$
and
![]() $u_2$
for the first Borel class of the tautological symplectic bundle on the first and second coordinates of
$u_2$
for the first Borel class of the tautological symplectic bundle on the first and second coordinates of
![]() $\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^\infty _S$
.
$\mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^1 \times \mathrm {H}\mathbb {P}^\infty _S$
.
Using the computation of the ternary laws for Milnor-Witt cohomology, it is possible to determine
![]() $\psi _{2n}$
. However, it is possible to substantially simplify this computation by remembering that the class
$\psi _{2n}$
. However, it is possible to substantially simplify this computation by remembering that the class
![]() $\psi _{2n}$
in
$\psi _{2n}$
in
![]() $GW(S)_R$
is determined by its rank and its class in the Witt ring
$GW(S)_R$
is determined by its rank and its class in the Witt ring
![]() $W(S)_R$
. On the other hand, we have two canonical maps
$W(S)_R$
. On the other hand, we have two canonical maps
according to [Reference Déglise and Fasel14, Section 4.3.1] and [Reference Déglise and Fasel13, (proof of) Proposition 4.1.2] under assumption (a) and [Reference Déglise, Fasel, Khan and Jin15, Cor. 6.2] under assumption (b). These maps induce respectively the rank and the projection map on the cohomology groups in degree
![]() $(0,0)$
. Thus, we need only to specialise our computations either to motivic cohomology or to unramified Witt cohomology. This will be done in Proposition 4.3.5 and Corollary 4.3.8.
$(0,0)$
. Thus, we need only to specialise our computations either to motivic cohomology or to unramified Witt cohomology. This will be done in Proposition 4.3.5 and Corollary 4.3.8.
4.3.3. We consider the hypothesis of Theorem 4.3.2. Recall from [Reference Riou30, Proposition 6.2.2.1] that there are canonical operations
 $$ \begin{align*}\chi_i:\mathbb Z \times B\mathrm{GL} \rightarrow K\big(R(i),2i\big) \end{align*} $$
$$ \begin{align*}\chi_i:\mathbb Z \times B\mathrm{GL} \rightarrow K\big(R(i),2i\big) \end{align*} $$
where
 $K(R(i),2i)=\Omega ^\infty \big (\mathbf {H}_{\mathrm M} R_S(i)[2i]\big )$
is the motivic Eilenberg-MacLane space of degree
$K(R(i),2i)=\Omega ^\infty \big (\mathbf {H}_{\mathrm M} R_S(i)[2i]\big )$
is the motivic Eilenberg-MacLane space of degree
![]() $(2i,i)$
.Footnote 17
$(2i,i)$
.Footnote 17
On the other hand, we defined additive
![]() $\mathrm {Sp}$
-operations by applying Theorem 4.1.6 to the motivic cohomology ring spectrum
$\mathrm {Sp}$
-operations by applying Theorem 4.1.6 to the motivic cohomology ring spectrum
![]() $\mathbf {H}_{\mathrm M} R_S$
:
$\mathbf {H}_{\mathrm M} R_S$
:
 $$ \begin{align*}\tilde{\chi}_{2n}^M:\mathbb Z \times B\mathrm{Sp} \rightarrow K\big(R(2i),4i\big). \end{align*} $$
$$ \begin{align*}\tilde{\chi}_{2n}^M:\mathbb Z \times B\mathrm{Sp} \rightarrow K\big(R(2i),4i\big). \end{align*} $$
Lemma 4.3.4. Consider the above notations and assumptions. Let
![]() $f:B\mathrm {Sp} \rightarrow B\mathrm {GL}$
be the canonical forgetful map. Then for any
$f:B\mathrm {Sp} \rightarrow B\mathrm {GL}$
be the canonical forgetful map. Then for any
![]() $n>0$
one has
$n>0$
one has
Note that by definition,
![]() $\tilde {\chi }_0=1=\chi _0$
.
$\tilde {\chi }_0=1=\chi _0$
.
Proof. Let us denote by
![]() $b_i^M$
the Borel classes associated with the canonical
$b_i^M$
the Borel classes associated with the canonical
![]() $\mathrm {Sp}$
-orientation of
$\mathrm {Sp}$
-orientation of
![]() $\mathbf {H}_{\mathrm M} R_S$
. We prove the result by induction on
$\mathbf {H}_{\mathrm M} R_S$
. We prove the result by induction on
![]() $n\geq 1$
.
$n\geq 1$
.
For
![]() $n=1$
and a symplectic bundle
$n=1$
and a symplectic bundle
![]() $(U,\psi )$
, we get from formulas (4.1.7.a) and (2.3.7.a)
$(U,\psi )$
, we get from formulas (4.1.7.a) and (2.3.7.a)
![]() ${\tilde {\chi }}^M_2(U,\psi )=b^M_1(U,\psi )=-c_2(U)$
. On the other hand, using [Reference Riou30, Remark 6.2.2.3], we obtain
${\tilde {\chi }}^M_2(U,\psi )=b^M_1(U,\psi )=-c_2(U)$
. On the other hand, using [Reference Riou30, Remark 6.2.2.3], we obtain
![]() $\chi _{2}=c_1^2(U)-2c_2(U)=-2c_2(U)$
. These two equalities allow us to conclude. Then the induction step is provided by the following computation (where we suppress f for readability):
$\chi _{2}=c_1^2(U)-2c_2(U)=-2c_2(U)$
. These two equalities allow us to conclude. Then the induction step is provided by the following computation (where we suppress f for readability):
 $$ \begin{align*} \chi_{2n+2} & =-\sum_{i=1}^{n} c_{2i}\chi_{2n-2i+2}-(2n+2)c_{2n+2} \\ & = -2.\sum_{i=1}^{n} (-1)^ib^M_{i}{\tilde{\chi}}^M_{2n-2i+2}-(2n+2)(-1)^{n+1}b^M_{n+1} \\ & = -2\left(\sum_{i=1}^{n} (-1)^ib^M_{i}{\tilde{\chi}}^M_{2n-2i+2}+(-1)^{n+1}(n+1)b^M_{n+1}\right) = 2{\tilde{\chi}}^M_{2n+2}, \end{align*} $$
$$ \begin{align*} \chi_{2n+2} & =-\sum_{i=1}^{n} c_{2i}\chi_{2n-2i+2}-(2n+2)c_{2n+2} \\ & = -2.\sum_{i=1}^{n} (-1)^ib^M_{i}{\tilde{\chi}}^M_{2n-2i+2}-(2n+2)(-1)^{n+1}b^M_{n+1} \\ & = -2\left(\sum_{i=1}^{n} (-1)^ib^M_{i}{\tilde{\chi}}^M_{2n-2i+2}+(-1)^{n+1}(n+1)b^M_{n+1}\right) = 2{\tilde{\chi}}^M_{2n+2}, \end{align*} $$
where the first (respectively second, last) equality follows from [Reference Riou30, Remark 6.2.2.3] (respectively (2.3.7.a) and the induction hypothesis, (4.1.7.b)).
Proposition 4.3.5. For any
![]() $n>0$
, we have
$n>0$
, we have
 $$ \begin{align*}\omega_H({\tilde{\chi}}^M_{2n+4})=(2n+4)(2n+3)(2n+2)(2n+1){\tilde{\chi}}^M_{2n}. \end{align*} $$
$$ \begin{align*}\omega_H({\tilde{\chi}}^M_{2n+4})=(2n+4)(2n+3)(2n+2)(2n+1){\tilde{\chi}}^M_{2n}. \end{align*} $$
Consequently,
![]() $\operatorname {\mathrm {rk}}(\psi _{2n+4})=(2n+4)(2n+3)(2n+2)(2n+1)$
for any
$\operatorname {\mathrm {rk}}(\psi _{2n+4})=(2n+4)(2n+3)(2n+2)(2n+1)$
for any
![]() $n\geq 0$
.
$n\geq 0$
.
Proof. According to the plus part of formula (4.2.7.a), we have a priori
 $$ \begin{align*}\omega_H({\tilde{\chi}}^M_{2n+4})= r_{2n+4}.{\tilde{\chi}}^M_{2n}, \end{align*} $$
$$ \begin{align*}\omega_H({\tilde{\chi}}^M_{2n+4})= r_{2n+4}.{\tilde{\chi}}^M_{2n}, \end{align*} $$
where
![]() $r_{2n+4}$
is an element of
$r_{2n+4}$
is an element of
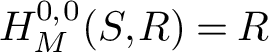 $H^{0,0}_M(S,R)=R$
.Footnote 18 We know from [Reference Riou30, Lemma 6.2.3.2] that
$H^{0,0}_M(S,R)=R$
.Footnote 18 We know from [Reference Riou30, Lemma 6.2.3.2] that
![]() $\Omega _{\mathbb P^1}(\chi _{2n+4})=(2n+4)\chi _{2n+3}$
. Therefore, because
$\Omega _{\mathbb P^1}(\chi _{2n+4})=(2n+4)\chi _{2n+3}$
. Therefore, because
![]() $2$
is a nonzero divisor in R, the proposition follows the previous lemma and the obvious fact that
$2$
is a nonzero divisor in R, the proposition follows the previous lemma and the obvious fact that
![]() $\omega _H({\tilde {\chi }}^M_{2n+4} \circ f) =\Omega _{\mathbb P^1}^4(\tilde {\chi }^M_{2n+4}) \circ f$
. Beware the particular case
$\omega _H({\tilde {\chi }}^M_{2n+4} \circ f) =\Omega _{\mathbb P^1}^4(\tilde {\chi }^M_{2n+4}) \circ f$
. Beware the particular case
![]() $n=0$
, as
$n=0$
, as
![]() $\tilde {\chi }_0=\chi _0$
.
$\tilde {\chi }_0=\chi _0$
.
4.3.6. The main point to prove the above theorem is to determine the Witt part
![]() $\bar \psi _{2n+4} \in \operatorname {\mathrm {W}}(S)_R$
of the quadratic form
$\bar \psi _{2n+4} \in \operatorname {\mathrm {W}}(S)_R$
of the quadratic form
![]() $\psi _{2n+4} \in \operatorname {\mathrm {GW}}(S)_R$
of Paragraph 4.3.1. So we consider the assumptions of this theorem and we let
$\psi _{2n+4} \in \operatorname {\mathrm {GW}}(S)_R$
of Paragraph 4.3.1. So we consider the assumptions of this theorem and we let
![]() $\tilde {\chi }^W_{2n+4}$
(respectively
$\tilde {\chi }^W_{2n+4}$
(respectively
![]() $b_i^W$
) be the
$b_i^W$
) be the
![]() $\mathrm {Sp}$
-operation (respectively Borel class) associated with the
$\mathrm {Sp}$
-operation (respectively Borel class) associated with the
![]() $\mathrm {Sp}$
-oriented ring spectrum
$\mathrm {Sp}$
-oriented ring spectrum
![]() ${\mathbf H} \operatorname {{\underline W}}_{R,S}$
. Specialising relation (4.3.2.b), we get for any
${\mathbf H} \operatorname {{\underline W}}_{R,S}$
. Specialising relation (4.3.2.b), we get for any
![]() $n \geq 0$
,
$n \geq 0$
,
 $$ \begin{align*}{\tilde{\chi}}_{2n+4}^W\big((\mathfrak U_1-\mathfrak H)(\mathfrak U_2-\mathfrak H)\mathfrak U\big)=\bar \psi_{2n+4}.u_1u_2u^n, \end{align*} $$
$$ \begin{align*}{\tilde{\chi}}_{2n+4}^W\big((\mathfrak U_1-\mathfrak H)(\mathfrak U_2-\mathfrak H)\mathfrak U\big)=\bar \psi_{2n+4}.u_1u_2u^n, \end{align*} $$
where
![]() $u_1=b_1^W(\mathfrak U_1)$
,
$u_1=b_1^W(\mathfrak U_1)$
,
![]() $u_2=b_1^W(\mathfrak U_2)$
,
$u_2=b_1^W(\mathfrak U_2)$
,
![]() $u=b_1^W(\mathfrak U)$
.
$u=b_1^W(\mathfrak U)$
.
Proposition 4.3.7. Under the above assumptions, we have:
 $$ \begin{align*} {\tilde{\chi}}^{W}_{2n+4}(\mathfrak U_1\mathfrak U_2\mathfrak U)= \begin{cases} 4u^{n+2} & \text{if }n \text{is even,} \\ -(2n+4)(2n+2)u_1u_2u^{n} & \text{if }n \text{ is odd.} \end{cases} \end{align*} $$
$$ \begin{align*} {\tilde{\chi}}^{W}_{2n+4}(\mathfrak U_1\mathfrak U_2\mathfrak U)= \begin{cases} 4u^{n+2} & \text{if }n \text{is even,} \\ -(2n+4)(2n+2)u_1u_2u^{n} & \text{if }n \text{ is odd.} \end{cases} \end{align*} $$
Proof. Put
![]() $b_i^W=b_i^W(\mathfrak U_1\mathfrak U_2\mathfrak U)$
and
$b_i^W=b_i^W(\mathfrak U_1\mathfrak U_2\mathfrak U)$
and
![]() ${\tilde {\chi }}^{W}_{2n+4}={\tilde {\chi }}^{W}_{2n+4}(\mathfrak U_1\mathfrak U_2\mathfrak U)$
. Let us first start by computing the Borel classes using the ternary law of unramified Witt theory, Formula (3.2.4.c) and the relation
${\tilde {\chi }}^{W}_{2n+4}={\tilde {\chi }}^{W}_{2n+4}(\mathfrak U_1\mathfrak U_2\mathfrak U)$
. Let us first start by computing the Borel classes using the ternary law of unramified Witt theory, Formula (3.2.4.c) and the relation
![]() $u_i^2=0$
:
$u_i^2=0$
:
 $$ \begin{align*}b_i^W= \begin{cases} 0 & i=1, i>4, \\ -2u^2 & i=2, \\ -8u_1u_2u & i=3, \\ u^4 & i=4. \end{cases} \end{align*} $$
$$ \begin{align*}b_i^W= \begin{cases} 0 & i=1, i>4, \\ -2u^2 & i=2, \\ -8u_1u_2u & i=3, \\ u^4 & i=4. \end{cases} \end{align*} $$
We derive from this computation and Newton’s identity relation (4.1.7.b) the following relation for
![]() $n>2$
:
$n>2$
:
 $$ \begin{align} {\tilde{\chi}}^{W}_{2n+4} =2u^2.\tilde{\chi}^{W}_{2n} -8u_1u_2u.\tilde{\chi}^{W}_{2n-2} -u^4.\tilde{\chi}^{W}_{2n-4}. \end{align} $$
$$ \begin{align} {\tilde{\chi}}^{W}_{2n+4} =2u^2.\tilde{\chi}^{W}_{2n} -8u_1u_2u.\tilde{\chi}^{W}_{2n-2} -u^4.\tilde{\chi}^{W}_{2n-4}. \end{align} $$
On the other hand, one can express the first
![]() $\mathrm {Sp}$
-operations using again the first computation and Formula (4.1.7.a):
$\mathrm {Sp}$
-operations using again the first computation and Formula (4.1.7.a):
 $$ \begin{align*}\tilde{\chi}^W_{2n+4}= \begin{cases} 4u^2 & n=0, \\ -24u_1u_2u & n=1, \\ 4u^4 & n=2. \end{cases} \end{align*} $$
$$ \begin{align*}\tilde{\chi}^W_{2n+4}= \begin{cases} 4u^2 & n=0, \\ -24u_1u_2u & n=1, \\ 4u^4 & n=2. \end{cases} \end{align*} $$
Finally, one proves the lemma by induction on n using relation (4.3.7.a).
Corollary 4.3.8. For any
![]() $n\geq 0$
, we have
$n\geq 0$
, we have
 $$ \begin{align*}\omega_H(\tilde{\chi}^W_{2n+4})= \begin{cases} 0 & \text{if }n \text{ is even,} \\ -(2n+4)(2n+2).\tilde{\chi}^W_{2n} & \text{if }n \text{ is odd}. \end{cases} \end{align*} $$
$$ \begin{align*}\omega_H(\tilde{\chi}^W_{2n+4})= \begin{cases} 0 & \text{if }n \text{ is even,} \\ -(2n+4)(2n+2).\tilde{\chi}^W_{2n} & \text{if }n \text{ is odd}. \end{cases} \end{align*} $$
In other words, the image of
![]() $\psi _{2n+4}$
in
$\psi _{2n+4}$
in
![]() $W(S)_R$
is
$W(S)_R$
is
![]() $0$
for n even and
$0$
for n even and
![]() $-(2n+4)(2n+2)$
if n is odd.
$-(2n+4)(2n+2)$
if n is odd.
Proof. Using the additivity of
![]() ${\tilde {\chi }}_{2n+4}^W$
, we find
${\tilde {\chi }}_{2n+4}^W$
, we find
 $$ \begin{align*} {\tilde{\chi}}_{2n+4}^W\big((\mathfrak U_1-\mathfrak H)(\mathfrak U_2-\mathfrak H)\mathfrak U\big)={\tilde{\chi}}_{2n+4}^W(\mathfrak U_1\mathfrak U_2\mathfrak U)-\sum_{i=1}^2{\tilde{\chi}}_{2n+4}^W(\mathfrak H\mathfrak U_i\mathfrak U)+{\tilde{\chi}}_{2n+4}^W(\mathfrak H\mathfrak H\mathfrak U). \end{align*} $$
$$ \begin{align*} {\tilde{\chi}}_{2n+4}^W\big((\mathfrak U_1-\mathfrak H)(\mathfrak U_2-\mathfrak H)\mathfrak U\big)={\tilde{\chi}}_{2n+4}^W(\mathfrak U_1\mathfrak U_2\mathfrak U)-\sum_{i=1}^2{\tilde{\chi}}_{2n+4}^W(\mathfrak H\mathfrak U_i\mathfrak U)+{\tilde{\chi}}_{2n+4}^W(\mathfrak H\mathfrak H\mathfrak U). \end{align*} $$
To compute the second term, it suffices to replace
![]() $u_i$
by
$u_i$
by
![]() $0$
in the expression of Proposition 4.3.7, and the third term is obtained by setting
$0$
in the expression of Proposition 4.3.7, and the third term is obtained by setting
![]() $u_1=u_2=0$
. A direct computation allows to conclude.
$u_1=u_2=0$
. A direct computation allows to conclude.
4.4 Rational stable
 $\mathrm {Sp}$
-operations
$\mathrm {Sp}$
-operations
4.4.1. Consider again the notation of Paragraph 4.3.1. The next step is to provide conditions under which inverting the quadratic forms
![]() $\psi _{2n}$
in the Grothendieck-Witt ring
$\psi _{2n}$
in the Grothendieck-Witt ring
![]() $\operatorname {\mathrm {GW}}(S)_R$
is sensible. For n even, the forms are hyperbolic and inverting them would erase all quadratic information. Thus, we are led to consider the multiplicative system
$\operatorname {\mathrm {GW}}(S)_R$
is sensible. For n even, the forms are hyperbolic and inverting them would erase all quadratic information. Thus, we are led to consider the multiplicative system
![]() $S_\psi $
of
$S_\psi $
of
![]() $\operatorname {\mathrm {GW}}(S)_R$
generated by
$\operatorname {\mathrm {GW}}(S)_R$
generated by
![]() $\{\psi _{2n}\vert n \text { odd}\}$
. Note that
$\{\psi _{2n}\vert n \text { odd}\}$
. Note that
so we restrict our attention to the case of a perfect field k.
Lemma 4.4.2. Let P be an ordering of k and let
![]() $s_P:\mathrm {W}(k)\to \mathbb Z$
be the corresponding signature homomorphism; that is, the homomorphism characterised by
$s_P:\mathrm {W}(k)\to \mathbb Z$
be the corresponding signature homomorphism; that is, the homomorphism characterised by
 $$ \begin{align*} s_P(\langle a\rangle)=\begin{cases} 1 & \text{ if }a \text{ is positive w.r.t. }P, \\ -1 & \text{ if }a \text{ is negative w.r.t. }P.\end{cases} \end{align*} $$
$$ \begin{align*} s_P(\langle a\rangle)=\begin{cases} 1 & \text{ if }a \text{ is positive w.r.t. }P, \\ -1 & \text{ if }a \text{ is negative w.r.t. }P.\end{cases} \end{align*} $$
Then
![]() $s_P(\psi _{2n+4})=-(2n+4)(2n+2)\neq 0$
for any odd
$s_P(\psi _{2n+4})=-(2n+4)(2n+2)\neq 0$
for any odd
![]() $n\in \mathbb N$
.
$n\in \mathbb N$
.
Proof. It suffices to observe that
![]() $1$
is a square and then is positive w.r.t. P. It follows that
$1$
is a square and then is positive w.r.t. P. It follows that
![]() $-1$
is negative and we conclude.
$-1$
is negative and we conclude.
Proposition 4.4.3. Let
![]() $\mathcal P$
be the set of orderings of k. Then,
$\mathcal P$
be the set of orderings of k. Then,
 $$ \begin{align*} S_{\psi}^{-1}\mathrm{W}(k)\simeq \bigoplus_{P\in\mathcal P}\mathbb Q. \end{align*} $$
$$ \begin{align*} S_{\psi}^{-1}\mathrm{W}(k)\simeq \bigoplus_{P\in\mathcal P}\mathbb Q. \end{align*} $$
Proof. The above lemma shows that the map
 $$ \begin{align*} \mathrm{W}(k)\xrightarrow{\sum s_P} \bigoplus_{P\in\mathcal P} \mathbb Z \end{align*} $$
$$ \begin{align*} \mathrm{W}(k)\xrightarrow{\sum s_P} \bigoplus_{P\in\mathcal P} \mathbb Z \end{align*} $$
induces a well-defined map as in the statement; that is, we have a commutative diagram

Besides, the kernel and cokernel of the top homomorphism are
![]() $2$
-primary torsion (see Theorem 6.1 and the paragraph preceding this theorem in [Reference Scharlau32]). It follows immediately that the bottom map is surjective. Let now y be in the kernel of
$2$
-primary torsion (see Theorem 6.1 and the paragraph preceding this theorem in [Reference Scharlau32]). It follows immediately that the bottom map is surjective. Let now y be in the kernel of
 $$ \begin{align*} S_{\psi}^{-1}\mathrm{W}(k)\xrightarrow{\sum s_P} \bigoplus_{P\in\mathcal P}\mathbb Q. \end{align*} $$
$$ \begin{align*} S_{\psi}^{-1}\mathrm{W}(k)\xrightarrow{\sum s_P} \bigoplus_{P\in\mathcal P}\mathbb Q. \end{align*} $$
We may write
![]() $y=\frac xs$
with
$y=\frac xs$
with
![]() $s\in S_{\psi }$
,
$s\in S_{\psi }$
,
![]() $x\in W(k)$
. One deduces
$x\in W(k)$
. One deduces
 $$ \begin{align*}\sum s_P(x)\bigg(\sum s_P(s)\bigg)^{-1}=0,\end{align*} $$
$$ \begin{align*}\sum s_P(x)\bigg(\sum s_P(s)\bigg)^{-1}=0,\end{align*} $$
thus showing that
![]() $\sum s_P(x)=0$
. It follows that
$\sum s_P(x)=0$
. It follows that
![]() $2^rx=0$
for some
$2^rx=0$
for some
![]() $r\in \mathbb N$
. Now, we have
$r\in \mathbb N$
. Now, we have
![]() $8\vert (2n+4)(2n+2)$
in
$8\vert (2n+4)(2n+2)$
in
![]() $\mathrm {W}(k)$
and it follows that for any
$\mathrm {W}(k)$
and it follows that for any
![]() $r\in \mathbb N$
there exists an odd n such that
$r\in \mathbb N$
there exists an odd n such that
![]() $2^r\vert (\psi _{2n+4}\cdot \psi _{2n}\cdot \ldots \psi _2)$
. The map is thus injective.
$2^r\vert (\psi _{2n+4}\cdot \psi _{2n}\cdot \ldots \psi _2)$
. The map is thus injective.
Corollary 4.4.4. The signature and rank homomorphisms induce an isomorphism
 $$ \begin{align*} S_{\psi}^{-1}\mathrm{GW}(k)\simeq \mathbb Q\oplus \bigoplus_{P\in\mathcal P}\mathbb Q\simeq \mathrm{GW}(k)\otimes \mathbb Q. \end{align*} $$
$$ \begin{align*} S_{\psi}^{-1}\mathrm{GW}(k)\simeq \mathbb Q\oplus \bigoplus_{P\in\mathcal P}\mathbb Q\simeq \mathrm{GW}(k)\otimes \mathbb Q. \end{align*} $$
Proof. We have an exact sequence of
![]() $\operatorname {\mathrm {GW}}(k)$
-modules
$\operatorname {\mathrm {GW}}(k)$
-modules
Localisation being exact, we deduce an exact sequence of
 $S_{\psi }^{-1}\mathrm {GW}(k)$
-modules. The above proposition shows that
$S_{\psi }^{-1}\mathrm {GW}(k)$
-modules. The above proposition shows that
 $$ \begin{align*} S_{\psi}^{-1}\mathrm{GW}(k)\simeq S_{\psi}^{-1}\mathbb Z\oplus \bigoplus_{P\in\mathcal P}\mathbb Q \end{align*} $$
$$ \begin{align*} S_{\psi}^{-1}\mathrm{GW}(k)\simeq S_{\psi}^{-1}\mathbb Z\oplus \bigoplus_{P\in\mathcal P}\mathbb Q \end{align*} $$
and we are left to prove that
 $S_{\psi }^{-1}\mathbb Z\simeq \mathbb Q$
. Let p be an odd prime. Then,
$S_{\psi }^{-1}\mathbb Z\simeq \mathbb Q$
. Let p be an odd prime. Then,
![]() $p-2$
is odd and
$p-2$
is odd and
![]() $(2n+4)(2n+2)=4p(p-1)$
and therefore p is invertible. Because we already know that
$(2n+4)(2n+2)=4p(p-1)$
and therefore p is invertible. Because we already know that
![]() $2$
is also invertible, the result follows.
$2$
is also invertible, the result follows.
4.4.5. Let
![]() $S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
. We are now in a position to classify all stable symplectic operations with values in rational Milnor-Witt motivic cohomology
$S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
. We are now in a position to classify all stable symplectic operations with values in rational Milnor-Witt motivic cohomology
![]() $\mathbf {H}_{\mathrm {MW}} \mathbb Q_S$
of degree
$\mathbf {H}_{\mathrm {MW}} \mathbb Q_S$
of degree
![]() $(4n,2n)$
for
$(4n,2n)$
for
![]() $n \in \mathbb Z$
.
$n \in \mathbb Z$
.
We first consider the case
![]() $n=0$
. Recall the decomposition in plus and minus parts of Milnor-Witt motivic cohomology:
$n=0$
. Recall the decomposition in plus and minus parts of Milnor-Witt motivic cohomology:
We consider the additive symplectic operation
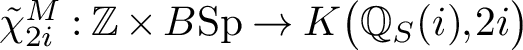 $\tilde {\chi }_{2i}^M:\mathbb Z \times B\mathrm {Sp} \rightarrow K\big (\mathbb Q_S(i),2i\big )$
defined in Paragraph 4.3.3. According to Proposition 4.3.5, we get a stable symplectic operation (Definition 4.2.2) on rational motivic cohomology by considering the following sequence:
$\tilde {\chi }_{2i}^M:\mathbb Z \times B\mathrm {Sp} \rightarrow K\big (\mathbb Q_S(i),2i\big )$
defined in Paragraph 4.3.3. According to Proposition 4.3.5, we get a stable symplectic operation (Definition 4.2.2) on rational motivic cohomology by considering the following sequence:
 $$ \begin{align*}\left(\tilde{\chi}^M_0,\frac 2 {4!} \tilde{\chi}^M_4,\ldots,\frac 2 {4n!} \tilde{\chi}^M_{4n},\ldots\right). \end{align*} $$
$$ \begin{align*}\left(\tilde{\chi}^M_0,\frac 2 {4!} \tilde{\chi}^M_4,\ldots,\frac 2 {4n!} \tilde{\chi}^M_{4n},\ldots\right). \end{align*} $$
Applying Corollary 4.2.6, this uniquely corresponds to a map
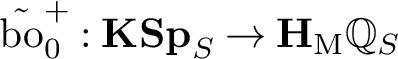 $\tilde {\operatorname {\mathrm {bo}}}^+_0:\mathbf {KSp}_S\rightarrow \mathbf {H}_{\mathrm M} \mathbb Q_S$
and we deduce a map
$\tilde {\operatorname {\mathrm {bo}}}^+_0:\mathbf {KSp}_S\rightarrow \mathbf {H}_{\mathrm M} \mathbb Q_S$
and we deduce a map
 $$ \begin{align*}\tilde{\operatorname{\mathrm{bo}}}_0:\mathbf{KSp}_S\xrightarrow{\tilde{\operatorname{\mathrm{bo}}}^+_0} \mathbf{H}_{\mathrm M} \mathbb Q_S \xrightarrow{i_+} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S, \end{align*} $$
$$ \begin{align*}\tilde{\operatorname{\mathrm{bo}}}_0:\mathbf{KSp}_S\xrightarrow{\tilde{\operatorname{\mathrm{bo}}}^+_0} \mathbf{H}_{\mathrm M} \mathbb Q_S \xrightarrow{i_+} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S, \end{align*} $$
where the last map is the canonical inclusion. For any
![]() $n \in \mathbb Z$
, we put
$n \in \mathbb Z$
, we put
 $$ \begin{align*}\tilde{\operatorname{\mathrm{bo}}}_{4n}:\mathbf{KSp}_S \simeq \mathbf{KSp}_S(4n)[8n] \xrightarrow{\tilde{\operatorname{\mathrm{bo}}}_0(4n)[8n]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(4n)[8n]. \end{align*} $$
$$ \begin{align*}\tilde{\operatorname{\mathrm{bo}}}_{4n}:\mathbf{KSp}_S \simeq \mathbf{KSp}_S(4n)[8n] \xrightarrow{\tilde{\operatorname{\mathrm{bo}}}_0(4n)[8n]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(4n)[8n]. \end{align*} $$
By definition, when
![]() $n>0$
, it is induced as above by the following stable symplectic operation with values in rational motivic cohomology of degree
$n>0$
, it is induced as above by the following stable symplectic operation with values in rational motivic cohomology of degree
![]() $(4n,8n)$
:
$(4n,8n)$
:
 $$ \begin{align} \left(\frac 2 {4n!} \tilde{\chi}^M_{4n},\frac 2 {(4n+4)!} \tilde{\chi}^M_{4n+4},\ldots\right). \end{align} $$
$$ \begin{align} \left(\frac 2 {4n!} \tilde{\chi}^M_{4n},\frac 2 {(4n+4)!} \tilde{\chi}^M_{4n+4},\ldots\right). \end{align} $$
For
![]() $n<0$
, just add enough zeroes at the start.
$n<0$
, just add enough zeroes at the start.
Next we consider the case
![]() $n=2$
. Let us consider the following product:
$n=2$
. Let us consider the following product:
Applying the previous corollary, this product of quadratic forms is invertible in
![]() $\mathbf {H}_{\mathrm {MW}}^{00}(S,\mathbb Q)=\operatorname {\mathrm {GW}}(S)_{\mathbb Q}=\operatorname {\mathrm {GW}}(\mathbb Q)_{\mathbb Q}$
. Thus, using Theorem 4.3.2, we can introduce the following stable symplectic operation with values in rational Milnor-Witt cohomology over S:
$\mathbf {H}_{\mathrm {MW}}^{00}(S,\mathbb Q)=\operatorname {\mathrm {GW}}(S)_{\mathbb Q}=\operatorname {\mathrm {GW}}(\mathbb Q)_{\mathbb Q}$
. Thus, using Theorem 4.3.2, we can introduce the following stable symplectic operation with values in rational Milnor-Witt cohomology over S:
 $$ \begin{align} \left(\tilde{\chi}^{\mathbb Q}_2,\frac 1 {\psi_6!} \tilde{\chi}^{\mathbb Q}_6,\ldots,\frac 1 {\psi_{2+4n}!} \tilde{\chi}^{\mathbb Q}_{2+4n},\ldots\right). \end{align} $$
$$ \begin{align} \left(\tilde{\chi}^{\mathbb Q}_2,\frac 1 {\psi_6!} \tilde{\chi}^{\mathbb Q}_6,\ldots,\frac 1 {\psi_{2+4n}!} \tilde{\chi}^{\mathbb Q}_{2+4n},\ldots\right). \end{align} $$
Applying again Corollary 4.2.6, it uniquely corresponds to a morphism
 $$ \begin{align*}\tilde{\operatorname{\mathrm{bo}}}_2:\mathbf{KSp}_S \rightarrow \mathbf{H}_{\mathrm{MW}} {\mathbb Q}_S(2)[4]. \end{align*} $$
$$ \begin{align*}\tilde{\operatorname{\mathrm{bo}}}_2:\mathbf{KSp}_S \rightarrow \mathbf{H}_{\mathrm{MW}} {\mathbb Q}_S(2)[4]. \end{align*} $$
For
![]() $n \in \mathbb Z$
, we put
$n \in \mathbb Z$
, we put
 $$ \begin{align*}\tilde{\operatorname{\mathrm{bo}}}_{2+4n}:\mathbf{KSp}_S \simeq \mathbf{KSp}_S(4n)[8n] \xrightarrow{\tilde{\operatorname{\mathrm{bo}}}_2(4n)[8n]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2+4n)[4+8n], \end{align*} $$
$$ \begin{align*}\tilde{\operatorname{\mathrm{bo}}}_{2+4n}:\mathbf{KSp}_S \simeq \mathbf{KSp}_S(4n)[8n] \xrightarrow{\tilde{\operatorname{\mathrm{bo}}}_2(4n)[8n]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2+4n)[4+8n], \end{align*} $$
which by definition is induced by the following stable symplectic operation for
![]() $n>0$
:
$n>0$
:
 $$ \begin{align} \left(\frac 1 {\psi_{2+4n}!} \tilde{\chi}^{\mathbb Q}_{2+4n},\frac 1 {\psi_{6+4n}!} \tilde{\chi}^{\mathbb Q}_{6+4n},\ldots\right). \end{align} $$
$$ \begin{align} \left(\frac 1 {\psi_{2+4n}!} \tilde{\chi}^{\mathbb Q}_{2+4n},\frac 1 {\psi_{6+4n}!} \tilde{\chi}^{\mathbb Q}_{6+4n},\ldots\right). \end{align} $$
Given now any
![]() $\mathbb Z[{1}/{2}]$
-scheme S, the operations
$\mathbb Z[{1}/{2}]$
-scheme S, the operations
![]() $\tilde {\operatorname {\mathrm {bo}}}_{2n}$
defined above can be defined over S by taking pullback along the unique morphism
$\tilde {\operatorname {\mathrm {bo}}}_{2n}$
defined above can be defined over S by taking pullback along the unique morphism
![]() $S \rightarrow \operatorname {\mathrm {Spec}}\left (\mathbb Z[{1}/{2}]\right )$
.
$S \rightarrow \operatorname {\mathrm {Spec}}\left (\mathbb Z[{1}/{2}]\right )$
.
Theorem 4.4.6. Let
![]() $S=\operatorname {\mathrm {Spec}}\left (\mathbb Z[{1}/{2}]\right )$
or
$S=\operatorname {\mathrm {Spec}}\left (\mathbb Z[{1}/{2}]\right )$
or
![]() $S=\operatorname {\mathrm {Spec}}(k)$
with k a perfect field of characteristic not
$S=\operatorname {\mathrm {Spec}}(k)$
with k a perfect field of characteristic not
![]() $2$
.
$2$
.
-
(1) Let
 $n \in \mathbb Z$
be an even integer. Then one has canonical isomorphisms where the first isomorphism is defined in Corollary 4.2.6 and the second one is the projection on the plus part. Moreover, these
$n \in \mathbb Z$
be an even integer. Then one has canonical isomorphisms where the first isomorphism is defined in Corollary 4.2.6 and the second one is the projection on the plus part. Moreover, these $$ \begin{align*} \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\widetilde{\mathrm{CH}}{}^{2n}_{\mathbb Q}\big) &\simeq \operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\left(\mathbf{KSp}_S,\mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n]\right) \\ &\simeq \operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\left(\mathbf{KSp}_S,\mathbf{H}_{\mathrm M} \mathbb Q_S(2n)[4n]\right) \simeq \mathbb Q, \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{Hom}}_{\mathrm{St}}\big(KSp_0,\widetilde{\mathrm{CH}}{}^{2n}_{\mathbb Q}\big) &\simeq \operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\left(\mathbf{KSp}_S,\mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n]\right) \\ &\simeq \operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\left(\mathbf{KSp}_S,\mathbf{H}_{\mathrm M} \mathbb Q_S(2n)[4n]\right) \simeq \mathbb Q, \end{align*} $$
 $\mathbb Q$
-vector spaces are generated by the stable operation
$\mathbb Q$
-vector spaces are generated by the stable operation
 $\tilde {\operatorname {\mathrm {bo}}}_{2n}$
defined above.
$\tilde {\operatorname {\mathrm {bo}}}_{2n}$
defined above.
-
(2) Let
 $n \in \mathbb Z$
be an odd integer. Then one has canonical isomorphisms where the first isomorphism is defined in Corollary 4.2.6 and these
$n \in \mathbb Z$
be an odd integer. Then one has canonical isomorphisms where the first isomorphism is defined in Corollary 4.2.6 and these $$ \begin{align*} \operatorname{\mathrm{Hom}}_{\mathrm{St}}\left(KSp_0,\widetilde{\mathrm{CH}}{}^{2n}_{\mathbb Q}\right) &\simeq \operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\left(\mathbf{KSp}_S,\mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n]\right) \\ &\simeq \operatorname{\mathrm{GW}}(S)_{\mathbb Q}=\mathbb Q \oplus \operatorname{\mathrm{W}}(S)_{\mathbb Q}, \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{Hom}}_{\mathrm{St}}\left(KSp_0,\widetilde{\mathrm{CH}}{}^{2n}_{\mathbb Q}\right) &\simeq \operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\left(\mathbf{KSp}_S,\mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n]\right) \\ &\simeq \operatorname{\mathrm{GW}}(S)_{\mathbb Q}=\mathbb Q \oplus \operatorname{\mathrm{W}}(S)_{\mathbb Q}, \end{align*} $$
 $\operatorname {\mathrm {GW}}(S)_{\mathbb Q}$
-modules are generated by the stable operation
$\operatorname {\mathrm {GW}}(S)_{\mathbb Q}$
-modules are generated by the stable operation
 $\tilde {\operatorname {\mathrm {bo}}}_{2n}$
defined above.
$\tilde {\operatorname {\mathrm {bo}}}_{2n}$
defined above.
Proof. Each statement follows simply from Corollary 4.2.6 and the computation of the projective system (4.2.7.a), whose transition maps are given by Theorem 4.3.2 (note that by
![]() $(8,4)$
-periodicity of
$(8,4)$
-periodicity of
![]() $\mathbf {KSp}_S$
, one can reduce to the case
$\mathbf {KSp}_S$
, one can reduce to the case
![]() $n \geq 0$
). By construction, we have for any symplectic bundle
$n \geq 0$
). By construction, we have for any symplectic bundle
![]() $\mathfrak U$
over S equalities
$\mathfrak U$
over S equalities
 $\tilde {\operatorname {\mathrm {bo}}}_0(\mathfrak U)=\tilde {\chi }_0^{\mathbb Q}(\mathfrak U)=\operatorname {\mathrm {rk}}(\mathfrak U)$
and
$\tilde {\operatorname {\mathrm {bo}}}_0(\mathfrak U)=\tilde {\chi }_0^{\mathbb Q}(\mathfrak U)=\operatorname {\mathrm {rk}}(\mathfrak U)$
and
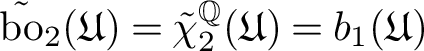 $\tilde {\operatorname {\mathrm {bo}}}_2(\mathfrak U)=\tilde {\chi }_2^{\mathbb Q}(\mathfrak U)=b_1(\mathfrak U)$
– apply (4.1.7.a). This implies that the operations
$\tilde {\operatorname {\mathrm {bo}}}_2(\mathfrak U)=\tilde {\chi }_2^{\mathbb Q}(\mathfrak U)=b_1(\mathfrak U)$
– apply (4.1.7.a). This implies that the operations
![]() $\tilde {\operatorname {\mathrm {bo}}}_{2n}$
are nonzero.
$\tilde {\operatorname {\mathrm {bo}}}_{2n}$
are nonzero.
4.4.7. Assume
![]() $S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
(or
$S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
(or
![]() $\operatorname {\mathrm {Spec}}(k)$
). Recall from [Reference Riou30, Definition 6.2.3.9] that for any
$\operatorname {\mathrm {Spec}}(k)$
). Recall from [Reference Riou30, Definition 6.2.3.9] that for any
![]() $n \geq 0$
, one has that
$n \geq 0$
, one has that
is generated by the nth component of the Chern character map
![]() $\operatorname {\mathrm {ch}}_n:\mathbf {KGL} \rightarrow \mathbf {H}_{\mathrm M} \mathbb Q_S$
(see footnote 17 for the case
$\operatorname {\mathrm {ch}}_n:\mathbf {KGL} \rightarrow \mathbf {H}_{\mathrm M} \mathbb Q_S$
(see footnote 17 for the case
![]() $S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
). Using the notations of Paragraph 4.3.3, the map
$S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
). Using the notations of Paragraph 4.3.3, the map
![]() $\operatorname {\mathrm {ch}}_n$
can be viewed as an H-stable operation as
$\operatorname {\mathrm {ch}}_n$
can be viewed as an H-stable operation as
 $$ \begin{align} \left(\frac 1 {n!}.\chi_n,\frac 1 {(n+4)!}.\chi_{n+4},\ldots\right). \end{align} $$
$$ \begin{align} \left(\frac 1 {n!}.\chi_n,\frac 1 {(n+4)!}.\chi_{n+4},\ldots\right). \end{align} $$
Proposition 4.4.8. Under the assumptions of the previous theorem, the following assertions hold:
-
(1) For any even integer n, the following diagram is commutative:
where f is the forgetful map and
 $i_+$
the inclusion of the plus part.
$i_+$
the inclusion of the plus part.
-
(2) For any integer n, the following diagram is commutative:
where f is the forgetful map and
 $p_+$
the projection onto the plus part.
$p_+$
the projection onto the plus part.
Proof. The first point follows directly from comparing formulas (4.4.5.a) and (4.4.7.a) using Lemma 4.3.4. Consider the second point. The case n even is implied by the first point. The case n odd reduces to
![]() $n>0$
(in fact,
$n>0$
(in fact,
![]() $n=1$
is enough). Then one can compare formulas (4.4.5.d) and (4.4.7.a) using Lemma 4.3.4 and the fact that
$n=1$
is enough). Then one can compare formulas (4.4.5.d) and (4.4.7.a) using Lemma 4.3.4 and the fact that
![]() $\operatorname {\mathrm {rk}}(\psi _{2n}!)=(2n)!/2$
(use Formula (4.3.2.a)).
$\operatorname {\mathrm {rk}}(\psi _{2n}!)=(2n)!/2$
(use Formula (4.3.2.a)).
5 The Borel character
5.1 Definition and main theorem
5.1.1. We rephrase in the next statement the main theorem of the previous section, Theorem 4.4.6, in terms of higher Grothendieck-Witt groups. Let S be a
![]() $\mathbb Z[{1}/{2}]$
-scheme S and
$\mathbb Z[{1}/{2}]$
-scheme S and
![]() $n \in \mathbb Z$
be an integer. Using the isomorphism (4.2.3.a) and the notations of Paragraph 4.4.5, we introduce the following maps. When n is even,
$n \in \mathbb Z$
be an integer. Using the isomorphism (4.2.3.a) and the notations of Paragraph 4.4.5, we introduce the following maps. When n is even,
 $$ \begin{align*} \operatorname{\mathrm{bo}}_{2n}:&\mathbf{GW}_S \simeq \mathbf{KSp}_S(-2)[-4] \\ &\xrightarrow{\tilde{\operatorname{\mathrm{bo}}}_{2+2n}(-2)[-4]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2+2n)[4+4n](-2)[-4] \simeq \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n]. \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{bo}}_{2n}:&\mathbf{GW}_S \simeq \mathbf{KSp}_S(-2)[-4] \\ &\xrightarrow{\tilde{\operatorname{\mathrm{bo}}}_{2+2n}(-2)[-4]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2+2n)[4+4n](-2)[-4] \simeq \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n]. \end{align*} $$
and when n is odd.
 $$ \begin{align*} \operatorname{\mathrm{bo}}_{2n}:&\mathbf{GW}_S \simeq \mathbf{KSp}_S(-2)[-4] \\ &\xrightarrow{p_+ \circ \operatorname{\mathrm{bo}}_{2+2n}(-2)[-4]} \mathbf{H}_{\mathrm M} \mathbb Q_S(2+2n)[4+4n](-2)[-4] \simeq \mathbf{H}_{\mathrm M} \mathbb Q_S(2n)[4n]/ \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{bo}}_{2n}:&\mathbf{GW}_S \simeq \mathbf{KSp}_S(-2)[-4] \\ &\xrightarrow{p_+ \circ \operatorname{\mathrm{bo}}_{2+2n}(-2)[-4]} \mathbf{H}_{\mathrm M} \mathbb Q_S(2+2n)[4+4n](-2)[-4] \simeq \mathbf{H}_{\mathrm M} \mathbb Q_S(2n)[4n]/ \end{align*} $$
Note that is follows from the construction of the stable
![]() $\mathrm {Sp}$
-operation
$\mathrm {Sp}$
-operation
![]() $\tilde {\operatorname {\mathrm {bo}}}$
(see loc. cit.) that for any integer
$\tilde {\operatorname {\mathrm {bo}}}$
(see loc. cit.) that for any integer
![]() $n \in \mathbb Z$
, one has
$n \in \mathbb Z$
, one has
 $$ \begin{align} \begin{aligned} \operatorname{\mathrm{bo}}_{4n}:&\mathbf{GW}_S \simeq \mathbf{GW}_S(4n)[8n] \xrightarrow{\operatorname{\mathrm{bo}}_0(4n)[8n]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(4n)[8n], \\ \operatorname{\mathrm{bo}}_{2+4n}:&\mathbf{GW}_S \simeq \mathbf{GW}_S(4n)[8n] \xrightarrow{\operatorname{\mathrm{bo}}_2(4n)[8n]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2+4n)[4+4n], \end{aligned} \end{align} $$
$$ \begin{align} \begin{aligned} \operatorname{\mathrm{bo}}_{4n}:&\mathbf{GW}_S \simeq \mathbf{GW}_S(4n)[8n] \xrightarrow{\operatorname{\mathrm{bo}}_0(4n)[8n]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(4n)[8n], \\ \operatorname{\mathrm{bo}}_{2+4n}:&\mathbf{GW}_S \simeq \mathbf{GW}_S(4n)[8n] \xrightarrow{\operatorname{\mathrm{bo}}_2(4n)[8n]} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2+4n)[4+4n], \end{aligned} \end{align} $$
where the first isomorphism in each line is obtained by the periodicity of Hermitian K-theory.Footnote 19
Proposition 5.1.2. Consider the assumptions of the previous theorem. Then for any integer
![]() $n \in \mathbb Z$
, one has
$n \in \mathbb Z$
, one has
 $$ \begin{align*}\operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\left(\mathbf{GW}_S,\mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n]\right)= \begin{cases} \mathbb Q.(i_+ \operatorname{\mathrm{bo}}_{2n}) & |n| \text{ odd}, \\ \operatorname{\mathrm{GW}}(S)_{\mathbb Q}.\operatorname{\mathrm{bo}}_{2n} & |n| \text{ even}. \end{cases} \end{align*} $$
$$ \begin{align*}\operatorname{\mathrm{Hom}}_{\operatorname{\mathrm{SH}}(S)}\left(\mathbf{GW}_S,\mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n]\right)= \begin{cases} \mathbb Q.(i_+ \operatorname{\mathrm{bo}}_{2n}) & |n| \text{ odd}, \\ \operatorname{\mathrm{GW}}(S)_{\mathbb Q}.\operatorname{\mathrm{bo}}_{2n} & |n| \text{ even}. \end{cases} \end{align*} $$
In the odd case, any map
![]() $\mathbf {GW}_S \rightarrow \mathbf {H}_{\mathrm {MW}} \mathbb Q_S(2n)[4n]$
is zero on the minus part. The map
$\mathbf {GW}_S \rightarrow \mathbf {H}_{\mathrm {MW}} \mathbb Q_S(2n)[4n]$
is zero on the minus part. The map
![]() $\operatorname {\mathrm {bo}}_0:\mathbf {GW}_S \rightarrow \mathbf {H}_{\mathrm {MW}} \mathbb Q_S$
is the unique map such that
$\operatorname {\mathrm {bo}}_0:\mathbf {GW}_S \rightarrow \mathbf {H}_{\mathrm {MW}} \mathbb Q_S$
is the unique map such that
![]() $bo_0(1_{GW})=1_{\mathbf {H}_{\mathrm {MW}}}$
.
$bo_0(1_{GW})=1_{\mathbf {H}_{\mathrm {MW}}}$
.
Proof. Everything except the statement about
![]() $\operatorname {\mathrm {bo}}_0$
follows from Theorem 4.4.6. By definition of
$\operatorname {\mathrm {bo}}_0$
follows from Theorem 4.4.6. By definition of
![]() $\operatorname {\mathrm {bo}}_0$
, we get the following commutative diagram:
$\operatorname {\mathrm {bo}}_0$
, we get the following commutative diagram:

where
![]() $(1)$
is the exterior product with the tautological symplectic bundle
$(1)$
is the exterior product with the tautological symplectic bundle
![]() $\mathfrak H$
on
$\mathfrak H$
on
![]() $\mathrm {H}\mathbb {P}^1$
and (2) is the projection on the factor
$\mathrm {H}\mathbb {P}^1$
and (2) is the projection on the factor
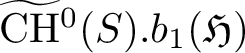 $\widetilde {\mathrm {CH}}{}^0(S).b_1(\mathfrak H)$
, using the symplectic projective bundle theorem for Chow-Witt groups (here
$\widetilde {\mathrm {CH}}{}^0(S).b_1(\mathfrak H)$
, using the symplectic projective bundle theorem for Chow-Witt groups (here
![]() $b_1$
denotes the Borel class for Chow-Witt groups). Thus, the statement simply follows from the fact that
$b_1$
denotes the Borel class for Chow-Witt groups). Thus, the statement simply follows from the fact that
![]() $\tilde {\operatorname {\mathrm {bo}}}_{2*}=\tilde {\chi }_2=b_1$
– formula (4.4.5.c) (respectively (4.1.7.a)) for the first (respectively second) equality.
$\tilde {\operatorname {\mathrm {bo}}}_{2*}=\tilde {\chi }_2=b_1$
– formula (4.4.5.c) (respectively (4.1.7.a)) for the first (respectively second) equality.
Definition 5.1.3. Let S be a
![]() $\mathbb Z[{1}/{2}]$
-scheme. We define the Borel character over S as the map
$\mathbb Z[{1}/{2}]$
-scheme. We define the Borel character over S as the map
 $$ \begin{align*} \operatorname{\mathrm{bo}}_t:\mathbf{GW}^{\mathbb Q}_{S} \xrightarrow{\ (\operatorname{\mathrm{bo}}_{2n})_{n \in \mathbb Z}\ } & \bigoplus_{n \text{ even}} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n] \oplus \bigoplus_{n \text{ odd}} \mathbf{H}_{\mathrm M} \mathbb Q_S(2n)[4n] \\ & \!\!\!\simeq \bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q+}(2n)[4n] \oplus \bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q-}(4n)[8n]. \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{bo}}_t:\mathbf{GW}^{\mathbb Q}_{S} \xrightarrow{\ (\operatorname{\mathrm{bo}}_{2n})_{n \in \mathbb Z}\ } & \bigoplus_{n \text{ even}} \mathbf{H}_{\mathrm{MW}} \mathbb Q_S(2n)[4n] \oplus \bigoplus_{n \text{ odd}} \mathbf{H}_{\mathrm M} \mathbb Q_S(2n)[4n] \\ & \!\!\!\simeq \bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q+}(2n)[4n] \oplus \bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q-}(4n)[8n]. \end{align*} $$
Note that the map
![]() $(bo_{2n})_{n \in \mathbb Z}$
lands into a direct sum rather than a product because the corresponding product is in fact isomorphic to the above direct sum. This follows because
$(bo_{2n})_{n \in \mathbb Z}$
lands into a direct sum rather than a product because the corresponding product is in fact isomorphic to the above direct sum. This follows because
![]() $\operatorname {\mathrm {SH}}(\mathbb Z[{1}/{2}])$
is compactly generated by objects of the form
$\operatorname {\mathrm {SH}}(\mathbb Z[{1}/{2}])$
is compactly generated by objects of the form
![]() $\Sigma ^\infty X_+(q)[p]$
for X smooth over
$\Sigma ^\infty X_+(q)[p]$
for X smooth over
![]() $\mathbb Z[{1}/{2}]$
and from the fact that
$\mathbb Z[{1}/{2}]$
and from the fact that
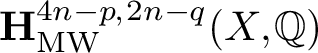 $\mathbf {H}_{\mathrm {MW}}^{4n-p,2n-q}(X,\mathbb Q)$
vanishes for
$\mathbf {H}_{\mathrm {MW}}^{4n-p,2n-q}(X,\mathbb Q)$
vanishes for
![]() $n>>0$
or
$n>>0$
or
![]() $n<<0$
. The second isomorphism follows from the identifications (1.0.0.d).
$n<<0$
. The second isomorphism follows from the identifications (1.0.0.d).
5.1.4. Note that, according to the properties of
![]() $\tilde {\operatorname {\mathrm {bo}}}_{2n}$
,
$\tilde {\operatorname {\mathrm {bo}}}_{2n}$
,
![]() $\operatorname {\mathrm {bo}}_t$
is compatible with pullbacks: it is a morphism of
$\operatorname {\mathrm {bo}}_t$
is compatible with pullbacks: it is a morphism of
![]() $\mathbb Z[{1}/{2}]$
-absolute spectra. Using Proposition 4.4.8, we immediately get the commutativity of the following square:
$\mathbb Z[{1}/{2}]$
-absolute spectra. Using Proposition 4.4.8, we immediately get the commutativity of the following square:

where f is the natural forgetful map and the right-hand vertical map is obtained by the projection on the plus part for the even integer m,
![]() $0$
for m odd. In particular, for odd n, the map
$0$
for m odd. In particular, for odd n, the map
![]() $\operatorname {\mathrm {bo}}_{2n}=\operatorname {\mathrm {ch}}_{2n} \circ f$
(apply point (1) of Proposition 4.4.8).
$\operatorname {\mathrm {bo}}_{2n}=\operatorname {\mathrm {ch}}_{2n} \circ f$
(apply point (1) of Proposition 4.4.8).
In the remainder of this section, we will prove that for any
![]() $\mathbb Z[{1}/{2}]$
-scheme S, the Borel character
$\mathbb Z[{1}/{2}]$
-scheme S, the Borel character
![]() $bo_t$
is an isomorphism of ring spectra (see Theorem 5.5.1).
$bo_t$
is an isomorphism of ring spectra (see Theorem 5.5.1).
5.2 Principle of proof and periodic spectra
5.2.1.
Principle of proof of Theorem 5.5.1
. To prove that the Borel character
![]() $\operatorname {\mathrm {bo}}_t$
is an isomorphism over any scheme S, by compatibility with pullbacks (see Paragraph 5.1.4), it is sufficient to consider the case
$\operatorname {\mathrm {bo}}_t$
is an isomorphism over any scheme S, by compatibility with pullbacks (see Paragraph 5.1.4), it is sufficient to consider the case
![]() $S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
. Moreover, we can always use Morel’s decomposition of
$S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
. Moreover, we can always use Morel’s decomposition of
![]() $\operatorname {\mathrm {SH}}(S)_{\mathbb Q}$
into its plus and minus parts.
$\operatorname {\mathrm {SH}}(S)_{\mathbb Q}$
into its plus and minus parts.
That being said, we will prove that
![]() $\operatorname {\mathrm {bo}}_t$
is an isomorphism by constructing an explicit inverse
$\operatorname {\mathrm {bo}}_t$
is an isomorphism by constructing an explicit inverse
![]() $\operatorname {\mathrm {bo}}^{\prime }_t$
using the theory of periodic ring spectra (see Definition below). The advantage of this construction is that
$\operatorname {\mathrm {bo}}^{\prime }_t$
using the theory of periodic ring spectra (see Definition below). The advantage of this construction is that
![]() $\operatorname {\mathrm {bo}}^{\prime }_t$
will clearly be a morphism of ring spectra. We will construct
$\operatorname {\mathrm {bo}}^{\prime }_t$
will clearly be a morphism of ring spectra. We will construct
![]() $\operatorname {\mathrm {bo}}^{\prime }_t$
by separating the plus part (Subsection 5.3) and the minus part (Subsection 5.4).
$\operatorname {\mathrm {bo}}^{\prime }_t$
by separating the plus part (Subsection 5.3) and the minus part (Subsection 5.4).
The following result is classical (see, e.g., [Reference Gepner and Snaith17, Prop. 2.6]) in (motivic) homotopy theory.
Proposition 5.2.2. Let
![]() $\mathbb E$
be a motivic ring spectrum over S. Consider a pair of integers
$\mathbb E$
be a motivic ring spectrum over S. Consider a pair of integers
![]() $(n,i) \in \mathbb Z^2$
. Then the following conditions are equivalent:
$(n,i) \in \mathbb Z^2$
. Then the following conditions are equivalent:
-
(i) There exists an element
 $\rho \in \mathbb E_{n,i}(S)$
, invertible for the cup product on
$\rho \in \mathbb E_{n,i}(S)$
, invertible for the cup product on
 $\mathbb E^{**}$
.
$\mathbb E^{**}$
. -
(ii) There exists an isomorphism
 $\tilde {\rho }:\mathbb E(i)[n] \rightarrow \mathbb E$
.
$\tilde {\rho }:\mathbb E(i)[n] \rightarrow \mathbb E$
.
Definition 5.2.3. A pair
![]() $(\mathbb E,\rho )$
satisfying the equivalent conditions of the above proposition will be called an
$(\mathbb E,\rho )$
satisfying the equivalent conditions of the above proposition will be called an
![]() $(n,i)$
-periodic ring spectrum over S.
$(n,i)$
-periodic ring spectrum over S.
We obviously get a
![]() $\mathscr Sch_B$
-fibred category of periodic ring spectra. A B-absolute
$\mathscr Sch_B$
-fibred category of periodic ring spectra. A B-absolute
![]() $(n,i)$
-periodic ring spectrum
$(n,i)$
-periodic ring spectrum
![]() $(\mathbb E_{,}\rho )$
is a section of this
$(\mathbb E_{,}\rho )$
is a section of this
![]() $\mathscr Sch_B$
-fibred category.Footnote 20
$\mathscr Sch_B$
-fibred category.Footnote 20
Given such a periodic absolute ring spectrum, we get a universal morphism of absolute ring spectra:
 $$ \begin{align} \sigma_\rho:\bigoplus_{r \in \mathbb Z} \mathbb S_S(ri)[rn] \xrightarrow{\sum_r \rho^r} \mathbb E_S \end{align} $$
$$ \begin{align} \sigma_\rho:\bigoplus_{r \in \mathbb Z} \mathbb S_S(ri)[rn] \xrightarrow{\sum_r \rho^r} \mathbb E_S \end{align} $$
with source the
![]() $(n,i)$
-periodisation of the sphere spectrum.
$(n,i)$
-periodisation of the sphere spectrum.
Example 5.2.4.
-
(1) The K-theory spectrum
 $\mathbf {KGL}$
together with the Bott element
$\mathbf {KGL}$
together with the Bott element
 $\beta $
is
$\beta $
is
 $(2,1)$
-periodic, as an absolute ring spectrum over
$(2,1)$
-periodic, as an absolute ring spectrum over
 $\mathbb Z$
. Note for normalisation purposes that we consider
$\mathbb Z$
. Note for normalisation purposes that we consider
 $\beta _{\mathbb Z}$
as the element of
$\beta _{\mathbb Z}$
as the element of
 $\mathbf {KGL}_{2,1}(\mathbb Z)$
uniquely defined by the following property: where
$\mathbf {KGL}_{2,1}(\mathbb Z)$
uniquely defined by the following property: where
 $\mathcal O(-1)$
is the tautological line bundle on
$\mathcal O(-1)$
is the tautological line bundle on
 $\mathbb P^1_S$
.
$\mathbb P^1_S$
.
Note that it follows from the relation
 $\operatorname {\mathrm {ch}}_n(\beta ^i)=\delta _{ni}$
that the rational and plus parts of the periodisation map are an isomorphism of ring spectra with inverse the Chern character map:
$\operatorname {\mathrm {ch}}_n(\beta ^i)=\delta _{ni}$
that the rational and plus parts of the periodisation map are an isomorphism of ring spectra with inverse the Chern character map: $$ \begin{align*}\sigma_\beta^{\mathbb Q+}:\bigoplus_{n \in \mathbb Z} \mathbf{H}_{\mathrm M}\mathbb Q_S(n)[2n] \rightarrow \mathbf{KGL}_{S,\mathbb Q} \end{align*} $$
$$ \begin{align*}\sigma_\beta^{\mathbb Q+}:\bigoplus_{n \in \mathbb Z} \mathbf{H}_{\mathrm M}\mathbb Q_S(n)[2n] \rightarrow \mathbf{KGL}_{S,\mathbb Q} \end{align*} $$
 $$ \begin{align*}\operatorname{\mathrm{ch}}_t:\mathbf{KGL}_S \xrightarrow{(\operatorname{\mathrm{ch}}_n)} \bigoplus_{n \in \mathbb Z} \mathbf{H}_{\mathrm M}\mathbb Q_S(n)[2n]. \end{align*} $$
$$ \begin{align*}\operatorname{\mathrm{ch}}_t:\mathbf{KGL}_S \xrightarrow{(\operatorname{\mathrm{ch}}_n)} \bigoplus_{n \in \mathbb Z} \mathbf{H}_{\mathrm M}\mathbb Q_S(n)[2n]. \end{align*} $$
-
(2) By construction (see [Reference Panin and Walter28]), the Hermitian K-theory spectrum
 $\mathbf {GW}$
is
$\mathbf {GW}$
is
 $(8,4)$
-periodic, as an absolute ring spectrum over
$(8,4)$
-periodic, as an absolute ring spectrum over
 $\mathbb Z[{1}/{2}]$
. We will consider the periodicity element
$\mathbb Z[{1}/{2}]$
. We will consider the periodicity element
 $\kappa \in \mathbf {GW}_{8,4}(\mathbb Z[{1}/{2}])$
characterised by the propertyFootnote 21 where
$\kappa \in \mathbf {GW}_{8,4}(\mathbb Z[{1}/{2}])$
characterised by the propertyFootnote 21 where
 $P=\mathrm {H}\mathbb {P}^1_{\mathbb Z[{1}/{2}]} \times \mathrm {H}\mathbb {P}^1_{\mathbb Z[{1}/{2}]}$
,
$P=\mathrm {H}\mathbb {P}^1_{\mathbb Z[{1}/{2}]} \times \mathrm {H}\mathbb {P}^1_{\mathbb Z[{1}/{2}]}$
,
 $\mathfrak U$
is the tautological symplectic bundle on
$\mathfrak U$
is the tautological symplectic bundle on
 $\mathrm {H}\mathbb {P}^1_{\mathbb Z[{1}/{2}]}$
(see Paragraph 2.2.2) and
$\mathrm {H}\mathbb {P}^1_{\mathbb Z[{1}/{2}]}$
(see Paragraph 2.2.2) and
 $\mathfrak U \otimes \mathfrak U$
is the external product, seen as a quadratic bundle. Explicitly,
$\mathfrak U \otimes \mathfrak U$
is the external product, seen as a quadratic bundle. Explicitly,
 $\kappa =(\mathfrak U_1-\mathfrak H)\otimes (\mathfrak U_2-\mathfrak H)$
.It follows from our conventions that for any
$\kappa =(\mathfrak U_1-\mathfrak H)\otimes (\mathfrak U_2-\mathfrak H)$
.It follows from our conventions that for any
 $\mathbb Z[{1}/{2}]$
-scheme S, the forgetful map
$\mathbb Z[{1}/{2}]$
-scheme S, the forgetful map
 $f:\mathbf {GW}_S \rightarrow \mathbf {KGL}_S$
sends
$f:\mathbf {GW}_S \rightarrow \mathbf {KGL}_S$
sends
 $\kappa _S$
to
$\kappa _S$
to
 $\beta _S^4$
.
$\beta _S^4$
.
-
(3) The
 $\mathbb Z[1/2]$
-absolute ring spectrum
$\mathbb Z[1/2]$
-absolute ring spectrum
 $\mathbf {W}$
, representing Balmer’s higher Witt groups, together with the Hopf map
$\mathbf {W}$
, representing Balmer’s higher Witt groups, together with the Hopf map
 $\eta $
is
$\eta $
is
 $(1,1)$
-periodic (see Subsection 5.4).
$(1,1)$
-periodic (see Subsection 5.4).
5.3 The plus part
5.3.1. Given an arbitrary
![]() $\mathbb Z[{1}/{2}]$
-scheme S, it follows from [Reference Schlichting33, Th. 6.1] and [Reference Röndigs and Østvær31, Th. 3.4] that one has a canonical distinguished triangle:
$\mathbb Z[{1}/{2}]$
-scheme S, it follows from [Reference Schlichting33, Th. 6.1] and [Reference Röndigs and Østvær31, Th. 3.4] that one has a canonical distinguished triangle:
 $$ \begin{align*}\mathbf{GW}_S(1)[1] \xrightarrow{\eta} \mathbf{GW}_S \xrightarrow f \mathbf{KGL}_S \xrightarrow{h \circ \gamma_{\beta'}} \mathbf{GW}_S(1)[2], \end{align*} $$
$$ \begin{align*}\mathbf{GW}_S(1)[1] \xrightarrow{\eta} \mathbf{GW}_S \xrightarrow f \mathbf{KGL}_S \xrightarrow{h \circ \gamma_{\beta'}} \mathbf{GW}_S(1)[2], \end{align*} $$
where
![]() $\eta $
is the Hopf map, f the forgetful map,
$\eta $
is the Hopf map, f the forgetful map,
![]() $h:\mathbf {KGL}_S \rightarrow \mathbf {GW}_S$
is the ‘hyperbolisation’ map and
$h:\mathbf {KGL}_S \rightarrow \mathbf {GW}_S$
is the ‘hyperbolisation’ map and
![]() $\gamma _{\beta '}$
is the multiplication by the inverse of the Bott element
$\gamma _{\beta '}$
is the multiplication by the inverse of the Bott element
![]() $\beta $
(Example 5.2.4). Because
$\beta $
(Example 5.2.4). Because
![]() $\eta _+=0$
and
$\eta _+=0$
and
![]() $\mathbf {KGL}_{S-}=0$
, we immediately deduce the following result.
$\mathbf {KGL}_{S-}=0$
, we immediately deduce the following result.
Lemma 5.3.2. The following exact sequence is split exact in
![]() $\operatorname {\mathrm {SH}}(S)_+$
:
$\operatorname {\mathrm {SH}}(S)_+$
:
 $$ \begin{align*}0 \rightarrow \mathbf{GW}_{S+} \xrightarrow f \mathbf{KGL}_S[2^{-1}] \xrightarrow{h \circ \gamma_{\beta'}} \mathbf{GW}_{S+}(1)[2] \rightarrow 0. \end{align*} $$
$$ \begin{align*}0 \rightarrow \mathbf{GW}_{S+} \xrightarrow f \mathbf{KGL}_S[2^{-1}] \xrightarrow{h \circ \gamma_{\beta'}} \mathbf{GW}_{S+}(1)[2] \rightarrow 0. \end{align*} $$
In other words,
![]() $\mathbf {KGL}_S[2^{-1}] \simeq \mathbf {GW}_{S+} \oplus \mathbf {GW}_{S+}(1)[2]$
.
$\mathbf {KGL}_S[2^{-1}] \simeq \mathbf {GW}_{S+} \oplus \mathbf {GW}_{S+}(1)[2]$
.
Moreover, there is a canonical splitting of the above exact sequence. Indeed, according to [Reference Röndigs and Østvær31, Lemma 3.6.], one gets the relation in
![]() $\operatorname {\mathrm {End}}_{\operatorname {\mathrm {SH}}(S)_+}(\mathbf {GW}_{S+})$
:
$\operatorname {\mathrm {End}}_{\operatorname {\mathrm {SH}}(S)_+}(\mathbf {GW}_{S+})$
:
because, by design,
![]() $\epsilon _+=-1$
.
$\epsilon _+=-1$
.
5.3.3. By construction of the Hermitian K-theory spectrum and the forgetful map f (see [Reference Röndigs and Østvær31, Prop. 3.3]), we get the following commutative diagram:

where
![]() $\gamma _{\beta ^2}$
is multiplication by
$\gamma _{\beta ^2}$
is multiplication by
![]() $\beta ^2$
and f on the right-hand side is the map forgetting the Hermitian structure. With this notation, we can define the following element in the positive part of Hermitian K-theory:
$\beta ^2$
and f on the right-hand side is the map forgetting the Hermitian structure. With this notation, we can define the following element in the positive part of Hermitian K-theory:
 $$ \begin{align*}\rho_S=\frac 12.\phi([\mathfrak H]) \in \mathbf{GW}^+_{4,2}(S). \end{align*} $$
$$ \begin{align*}\rho_S=\frac 12.\phi([\mathfrak H]) \in \mathbf{GW}^+_{4,2}(S). \end{align*} $$
This element is stable under pullback, so we can erase the base S to simplify notation. It follows from the above commutative square that
![]() $f(\rho )=\beta ^2$
.
$f(\rho )=\beta ^2$
.
The same construction can be done replacing in degree
![]() $(-4,-2)$
by replacing
$(-4,-2)$
by replacing
![]() $\beta $
with
$\beta $
with
![]() $\beta ^{-1}$
. Thus, we get an element
$\beta ^{-1}$
. Thus, we get an element
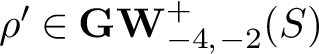 $\rho ' \in \mathbf {GW}^+_{-4,-2}(S)$
such that
$\rho ' \in \mathbf {GW}^+_{-4,-2}(S)$
such that
![]() $f(\rho ')=\beta ^{-2}$
.
$f(\rho ')=\beta ^{-2}$
.
Let us remark that the forgetful map
![]() $f:\mathbf {GW}_S \rightarrow \mathbf {KGL}_S$
is a morphism of ring spectra. This implies that
$f:\mathbf {GW}_S \rightarrow \mathbf {KGL}_S$
is a morphism of ring spectra. This implies that
![]() $f(\rho .\rho ')=1$
. Because f is a monomorphism on the plus part, due to (5.3.2.a), we deduce that
$f(\rho .\rho ')=1$
. Because f is a monomorphism on the plus part, due to (5.3.2.a), we deduce that
![]() $\rho $
is invertible. Thus,
$\rho $
is invertible. Thus,
![]() $(\mathbf {GW}_+,\rho )$
is a periodic absolute
$(\mathbf {GW}_+,\rho )$
is a periodic absolute
![]() $(4,2)$
-spectrum over
$(4,2)$
-spectrum over
![]() $\mathbb Z[{1}/{2}]$
. In particular, we get a canonical map by taking the plus part of (5.2.3.a):
$\mathbb Z[{1}/{2}]$
. In particular, we get a canonical map by taking the plus part of (5.2.3.a):
 $$ \begin{align*}\bigoplus_{n \in \mathbb Z} \mathbb S_{S,+}(2n)[4n] \xrightarrow{\sum_n \rho^n} \mathbf{GW}_{S+}. \end{align*} $$
$$ \begin{align*}\bigoplus_{n \in \mathbb Z} \mathbb S_{S,+}(2n)[4n] \xrightarrow{\sum_n \rho^n} \mathbf{GW}_{S+}. \end{align*} $$
Note, moreover, that we get the following relations for any integer
![]() $n \in \mathbb Z$
:
$n \in \mathbb Z$
:
 $$ \begin{align} f(\rho^n)=\beta^{2n}, \quad h(\beta^{n})=\begin{cases} 2.\rho^i & n=2i, \\ 0 & n \text{ odd}. \end{cases} \end{align} $$
$$ \begin{align} f(\rho^n)=\beta^{2n}, \quad h(\beta^{n})=\begin{cases} 2.\rho^i & n=2i, \\ 0 & n \text{ odd}. \end{cases} \end{align} $$
The first relation follows because f is a morphism of ring spectra and the second one from the first and the fact that
![]() $h \circ \gamma _{\beta '} \circ f=0$
(the above lemma). We immediately deduce from these relations the following lemma.
$h \circ \gamma _{\beta '} \circ f=0$
(the above lemma). We immediately deduce from these relations the following lemma.
Lemma 5.3.4. The following diagram is commutative in
![]() $\operatorname {\mathrm {SH}}(S)_+$
:
$\operatorname {\mathrm {SH}}(S)_+$
:

As an application, we get the following result that concludes the ‘plus part’ of Theorem 5.5.1.
Proposition 5.3.5. Consider the above notation. The morphism of rational ring spectra
 $$ \begin{align*}\sigma_\rho:\bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q+}(2n)[4n] \rightarrow \mathbf{GW}_{S,\mathbb Q+} \end{align*} $$
$$ \begin{align*}\sigma_\rho:\bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q+}(2n)[4n] \rightarrow \mathbf{GW}_{S,\mathbb Q+} \end{align*} $$
is an isomorphism, and the following relation holds:
The first assertion follows from the preceding lemma and point (1) of Example 5.2.4. The second assertion follows easily from relation (5.3.3.a), the commutativity of the square in Paragraph 5.1.4 and Example 5.2.4(1).
5.4 The minus part
5.4.1. Let S be a
![]() $\mathbb Z[{1}/{2}]$
-scheme. According to our conventions, there exists a natural map
$\mathbb Z[{1}/{2}]$
-scheme. According to our conventions, there exists a natural map
that induces an isomorphism
In particular,
![]() $\mathbf {W}_S[1/2]$
is
$\mathbf {W}_S[1/2]$
is
![]() $(1,1)$
-periodic with respect to
$(1,1)$
-periodic with respect to
![]() $\eta $
and
$\eta $
and
![]() $(8,4)$
-periodic with respect to
$(8,4)$
-periodic with respect to
![]() $\kappa _- \in \mathbf {W}_{8,4}$
, which is the image of
$\kappa _- \in \mathbf {W}_{8,4}$
, which is the image of
![]() $\kappa $
under the canonical projection:
$\kappa $
under the canonical projection:
![]() $\mathbf {GW}_S \rightarrow \mathbf {GW}_{S-}$
. The next result generalises the fundamental result of [Reference Ananyevskiy, Levine and Panin4] to an arbitrary base
$\mathbf {GW}_S \rightarrow \mathbf {GW}_{S-}$
. The next result generalises the fundamental result of [Reference Ananyevskiy, Levine and Panin4] to an arbitrary base
![]() $\mathbb Z[{1}/{2}]$
-scheme.
$\mathbb Z[{1}/{2}]$
-scheme.
Proposition 5.4.2. The canonical map associated to
![]() $\kappa _- \in \mathbf {W}_{8,4}(S)$
as in (5.2.3.a),
$\kappa _- \in \mathbf {W}_{8,4}(S)$
as in (5.2.3.a),
 $$ \begin{align*}\sigma_{\kappa_-}:\bigoplus_{n \in \mathbb Z} \mathbb S_{Q-}(4n)[8n] \rightarrow \mathbf{W}_S[1/2], \end{align*} $$
$$ \begin{align*}\sigma_{\kappa_-}:\bigoplus_{n \in \mathbb Z} \mathbb S_{Q-}(4n)[8n] \rightarrow \mathbf{W}_S[1/2], \end{align*} $$
is an isomorphism of ring spectra.
Proof. The map
![]() $\sigma _{\kappa _-}$
is compatible with base change. Recall from [Reference Cisinski and Déglise11, Proposition 4.3.17] that the family of functors
$\sigma _{\kappa _-}$
is compatible with base change. Recall from [Reference Cisinski and Déglise11, Proposition 4.3.17] that the family of functors
![]() $x^*$
for
$x^*$
for
![]() $x \in S$
a point of X is conservative. Thus, we are reduced to the case where S is the spectrum of a field (of characteristic different from
$x \in S$
a point of X is conservative. Thus, we are reduced to the case where S is the spectrum of a field (of characteristic different from
![]() $2$
). Then the result can be reduced to [Reference Ananyevskiy, Levine and Panin4, Corollary 3.5]. In fact, our isomorphism is the inverse of that of loc. cit.. Indeed, the stable operations
$2$
). Then the result can be reduced to [Reference Ananyevskiy, Levine and Panin4, Corollary 3.5]. In fact, our isomorphism is the inverse of that of loc. cit.. Indeed, the stable operations
![]() $\rho _m^{st}$
that compose the latter are defined by the relation
$\rho _m^{st}$
that compose the latter are defined by the relation
![]() $\rho _m^{st}(\kappa _-^n)=\delta _{nm}.\kappa _-^n$
; see [Reference Ananyevskiy, Levine and Panin4, Definition 2.5], given that the element
$\rho _m^{st}(\kappa _-^n)=\delta _{nm}.\kappa _-^n$
; see [Reference Ananyevskiy, Levine and Panin4, Definition 2.5], given that the element
![]() $\beta $
in loc. cit. is our element
$\beta $
in loc. cit. is our element
![]() $\kappa _-$
.
$\kappa _-$
.
Here is the last result needed to conclude the proof of Theorem 5.5.1.
Proposition 5.4.3. For any integers
![]() $n,i \in \mathbb Z$
, the following relation holds in
$n,i \in \mathbb Z$
, the following relation holds in
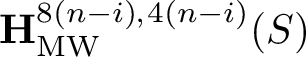 $\mathbf {H}_{\mathrm {MW}}^{8(n-i),4(n-i)}(S)$
:
$\mathbf {H}_{\mathrm {MW}}^{8(n-i),4(n-i)}(S)$
:
Proof. Note first that by compatibility with pullbacks, it is sufficient to treat the case where
![]() $S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
. According to the first of the relations (5.1.1.a), it is sufficient to treat the case
$S=\operatorname {\mathrm {Spec}}(\mathbb Z[{1}/{2}])$
. According to the first of the relations (5.1.1.a), it is sufficient to treat the case
![]() $n=0$
. The case
$n=0$
. The case
![]() $i=0$
follows from the last assertion of Proposition 5.1.2. The vanishing for
$i=0$
follows from the last assertion of Proposition 5.1.2. The vanishing for
![]() $n=0$
,
$n=0$
,
![]() $i\neq 0$
follows as MW-motivic cohomology of
$i\neq 0$
follows as MW-motivic cohomology of
![]() $\mathbb Z[{1}/{2}]$
vanishes in degree
$\mathbb Z[{1}/{2}]$
vanishes in degree
![]() $(8r,4r)$
for
$(8r,4r)$
for
![]() $r=(n-i)\neq 0$
, as recalled in Paragraph 4.2.5.
$r=(n-i)\neq 0$
, as recalled in Paragraph 4.2.5.
5.5 Conclusion
Putting together Propositions 5.3.5, 5.4.2 and 5.4.3, we get the main theorem of this section.
Theorem 5.5.1. Let S be an arbitrary
![]() $\mathbb Z[{1}/{2}]$
-scheme. Then the Borel character
$\mathbb Z[{1}/{2}]$
-scheme. Then the Borel character
![]() $\operatorname {\mathrm {bo}}_t$
(Definition 5.1.3) is an isomorphism of ring spectra with reciprocal isomorphism
$\operatorname {\mathrm {bo}}_t$
(Definition 5.1.3) is an isomorphism of ring spectra with reciprocal isomorphism
 $$ \begin{align*}\sigma_\rho+\sigma_{\kappa_-}:\bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q+}(2n)[4n] \oplus \bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q-}(4n)[8n] \rightarrow \mathbf{GW}_{S,\mathbb Q}. \end{align*} $$
$$ \begin{align*}\sigma_\rho+\sigma_{\kappa_-}:\bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q+}(2n)[4n] \oplus \bigoplus_{n \in \mathbb Z} \mathbb S_{S,\mathbb Q-}(4n)[8n] \rightarrow \mathbf{GW}_{S,\mathbb Q}. \end{align*} $$
Note that taking into account the relation
![]() $\rho ^2=\kappa _+$
and the identifications (1.0.0.d), one can rewrite the preceding isomorphism as
$\rho ^2=\kappa _+$
and the identifications (1.0.0.d), one can rewrite the preceding isomorphism as
 $$ \begin{align*}\sigma_\kappa+\sigma_{\kappa_+}.\rho:\bigoplus_{n \in \mathbb Z} \mathbf{H}_{\mathrm{MW}} \mathbb Q_{S}(4n)[8n] \oplus \bigoplus_{n \in \mathbb Z} \mathbf{H}_{\mathrm M} \mathbb Q_S(4n+2)[8n+4] \rightarrow \mathbf{GW}_{S,\mathbb Q}. \end{align*} $$
$$ \begin{align*}\sigma_\kappa+\sigma_{\kappa_+}.\rho:\bigoplus_{n \in \mathbb Z} \mathbf{H}_{\mathrm{MW}} \mathbb Q_{S}(4n)[8n] \oplus \bigoplus_{n \in \mathbb Z} \mathbf{H}_{\mathrm M} \mathbb Q_S(4n+2)[8n+4] \rightarrow \mathbf{GW}_{S,\mathbb Q}. \end{align*} $$
6 Applications
6.1 A Suslin-type homomorphism
In this subsection k is a perfect field of characteristic different from
![]() $2$
. We begin with the construction of a slightly different model of the spectrum
$2$
. We begin with the construction of a slightly different model of the spectrum
![]() $\mathbf {GW}$
. With this in mind, we can use [Reference Schlichting and Tripathi34, Theorem 1.3] (in the spirit of [Reference Asok and Fasel6, §2.2]) to observe that the orthogonal Grassmannian
$\mathbf {GW}$
. With this in mind, we can use [Reference Schlichting and Tripathi34, Theorem 1.3] (in the spirit of [Reference Asok and Fasel6, §2.2]) to observe that the orthogonal Grassmannian
![]() $OGr$
constructed in loc. cit. admits explicit deloopings
$OGr$
constructed in loc. cit. admits explicit deloopings
![]() $\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr)$
for
$\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr)$
for
![]() $n\in \mathbb N$
satisfying
$n\in \mathbb N$
satisfying
![]() $[\Sigma _{S^1}^i(X_+),\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr)]_{\mathbb A^1}\simeq \operatorname {\mathrm {GW}}^n_i(X)$
for any smooth scheme X. In particular,
$[\Sigma _{S^1}^i(X_+),\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr)]_{\mathbb A^1}\simeq \operatorname {\mathrm {GW}}^n_i(X)$
for any smooth scheme X. In particular,
 $\Omega _{\mathbb P^1}^{-2}(\mathbb Z\times OGr)\simeq \mathbb Z\times HGr$
. This allows one to define a spectrum whose term in degree n is
$\Omega _{\mathbb P^1}^{-2}(\mathbb Z\times OGr)\simeq \mathbb Z\times HGr$
. This allows one to define a spectrum whose term in degree n is
![]() $\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr)$
and whose bonding maps are the adjoints of the equivalences
$\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr)$
and whose bonding maps are the adjoints of the equivalences
 $$ \begin{align*} \Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr)\simeq \Omega_{\mathbb P^1}\Omega_{\mathbb P^1}^{-n-1}(\mathbb Z\times OGr). \end{align*} $$
$$ \begin{align*} \Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr)\simeq \Omega_{\mathbb P^1}\Omega_{\mathbb P^1}^{-n-1}(\mathbb Z\times OGr). \end{align*} $$
We still denote this spectrum by
![]() $\mathbf {GW}$
, because it is canonically isomorphic to the one we considered before. The unit map
$\mathbf {GW}$
, because it is canonically isomorphic to the one we considered before. The unit map
of this spectrum was explicitly constructed in [Reference Asok and Fasel6], yielding explicit morphisms
 $$ \begin{align*} \varepsilon_n:(\mathbb P^1)^{\wedge n}\to \Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr) \end{align*} $$
$$ \begin{align*} \varepsilon_n:(\mathbb P^1)^{\wedge n}\to \Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr) \end{align*} $$
for each
![]() $n\in \mathbb N$
. For an integer
$n\in \mathbb N$
. For an integer
![]() $n\in \mathbb N$
, we define
$n\in \mathbb N$
, we define
![]() $\mu (n)\in \mathbb N$
to be the smallest integer of the form
$\mu (n)\in \mathbb N$
to be the smallest integer of the form
![]() $4m+2$
greater than or equal to n. Note in particular that
$4m+2$
greater than or equal to n. Note in particular that
![]() $\mu (4n+2)=4n+2$
for any
$\mu (4n+2)=4n+2$
for any
![]() $n\in \mathbb N$
. We can define an operation
$n\in \mathbb N$
. We can define an operation
 $$ \begin{align*} \tilde{\chi}_{n}:\Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr)\to K\big(\tilde{\mathbb Z}(n),2n\big) \end{align*} $$
$$ \begin{align*} \tilde{\chi}_{n}:\Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr)\to K\big(\tilde{\mathbb Z}(n),2n\big) \end{align*} $$
for any
![]() $n\in \mathbb N$
using the commutative diagram
$n\in \mathbb N$
using the commutative diagram

in which the vertical maps are isomorphisms. Altogether, we get a composite
 $$ \begin{align*} (\mathbb P^1)^{\wedge n}\stackrel{\varepsilon_n}\to \Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr)\stackrel{\tilde{\chi}_{n}}\to K\big(\tilde{\mathbb Z}(n),2n\big) \end{align*} $$
$$ \begin{align*} (\mathbb P^1)^{\wedge n}\stackrel{\varepsilon_n}\to \Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr)\stackrel{\tilde{\chi}_{n}}\to K\big(\tilde{\mathbb Z}(n),2n\big) \end{align*} $$
that induces homomorphisms of sheaves
 $$ \begin{align*} \boldsymbol{\pi}^{{\mathbb A}^1}_i((\mathbb P^1)^{\wedge n})\to \boldsymbol{\pi}^{{\mathbb A}^1}_i(\Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr))\to \boldsymbol{\pi}^{{\mathbb A}^1}_i(K\big(\tilde{\mathbb Z}(n),2n\big)) \end{align*} $$
$$ \begin{align*} \boldsymbol{\pi}^{{\mathbb A}^1}_i((\mathbb P^1)^{\wedge n})\to \boldsymbol{\pi}^{{\mathbb A}^1}_i(\Omega_{\mathbb P^1}^{-n}(\mathbb Z\times OGr))\to \boldsymbol{\pi}^{{\mathbb A}^1}_i(K\big(\tilde{\mathbb Z}(n),2n\big)) \end{align*} $$
for each
![]() $i\in \mathbb N$
. Because
$i\in \mathbb N$
. Because
![]() $[\Sigma _{S^1}^i(X_+),\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr)]_{\mathbb A^1}\simeq \operatorname {\mathrm {GW}}_i^n(X)$
for any smooth scheme X, we see that
$[\Sigma _{S^1}^i(X_+),\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr)]_{\mathbb A^1}\simeq \operatorname {\mathrm {GW}}_i^n(X)$
for any smooth scheme X, we see that
 $\boldsymbol {\pi }^{{\mathbb A}^1}_i(\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr))$
is the sheaf associated to the presheaf
$\boldsymbol {\pi }^{{\mathbb A}^1}_i(\Omega _{\mathbb P^1}^{-n}(\mathbb Z\times OGr))$
is the sheaf associated to the presheaf
![]() $\operatorname {\mathrm {GW}}_i^n$
, and
$\operatorname {\mathrm {GW}}_i^n$
, and
 $\boldsymbol {\pi }^{{\mathbb A}^1}_i(K\big (\tilde {\mathbb Z}(n),2n\big ))$
is the sheaf associated to the presheaf
$\boldsymbol {\pi }^{{\mathbb A}^1}_i(K\big (\tilde {\mathbb Z}(n),2n\big ))$
is the sheaf associated to the presheaf
 $X\mapsto \mathrm {H}^{2n-i}_{\mathrm {MW}}(X,\mathbb Z)$
. Setting
$X\mapsto \mathrm {H}^{2n-i}_{\mathrm {MW}}(X,\mathbb Z)$
. Setting
![]() $i=n$
above and considering sections on a (finitely generated) field extension
$i=n$
above and considering sections on a (finitely generated) field extension
![]() $L/k$
, we then obtain a string of homomorphisms
$L/k$
, we then obtain a string of homomorphisms
 $$ \begin{align*} \boldsymbol{\pi}^{{\mathbb A}^1}_i((\mathbb P^1)^{\wedge n})(L)\xrightarrow{\varepsilon_{n,n}} \operatorname{\mathrm{GW}}_n^n(L)\xrightarrow{\tilde{\chi}_{n,n}} \mathrm{H}_{\mathrm{MW}}^{n,n}(L,\mathbb Z). \end{align*} $$
$$ \begin{align*} \boldsymbol{\pi}^{{\mathbb A}^1}_i((\mathbb P^1)^{\wedge n})(L)\xrightarrow{\varepsilon_{n,n}} \operatorname{\mathrm{GW}}_n^n(L)\xrightarrow{\tilde{\chi}_{n,n}} \mathrm{H}_{\mathrm{MW}}^{n,n}(L,\mathbb Z). \end{align*} $$
Suppose that
![]() $n\geq 2$
. In light of [Reference Morel25, Corollary 6.43] and [Reference Déglise and Fasel13, Theorem 4.2.3], we finally obtain homomorphisms
$n\geq 2$
. In light of [Reference Morel25, Corollary 6.43] and [Reference Déglise and Fasel13, Theorem 4.2.3], we finally obtain homomorphisms
 $$ \begin{align*} \mathrm{K}^{\mathrm{MW}}_n(L)\xrightarrow{\varepsilon_{n,n}} \operatorname{\mathrm{GW}}_n^n(L)\xrightarrow{\tilde{\chi}_{n,n}}\mathrm{K}^{\mathrm{MW}}_n(L). \end{align*} $$
$$ \begin{align*} \mathrm{K}^{\mathrm{MW}}_n(L)\xrightarrow{\varepsilon_{n,n}} \operatorname{\mathrm{GW}}_n^n(L)\xrightarrow{\tilde{\chi}_{n,n}}\mathrm{K}^{\mathrm{MW}}_n(L). \end{align*} $$
We note that the first map coincides (up to a unit) with the map
![]() $\mathrm {K}^{\mathrm {MW}}_{n}(L)\to \operatorname {\mathrm {GW}}_{n}^n(L)$
induced by the identity
$\mathrm {K}^{\mathrm {MW}}_{n}(L)\to \operatorname {\mathrm {GW}}_{n}^n(L)$
induced by the identity
![]() $\mathrm {K}^{\mathrm {MW}}_1(L)=\operatorname {\mathrm {GW}}^1_1(L)$
and the ring structure on both sides ([Reference Asok and Fasel6, Theorem 4.2.2]).
$\mathrm {K}^{\mathrm {MW}}_1(L)=\operatorname {\mathrm {GW}}^1_1(L)$
and the ring structure on both sides ([Reference Asok and Fasel6, Theorem 4.2.2]).
Theorem 6.1.1. For any
![]() $n\geq 2$
and any finitely generated field extension of k, the composite
$n\geq 2$
and any finitely generated field extension of k, the composite
 $$ \begin{align*} \mathrm{K}^{\mathrm{MW}}_n(L)\stackrel{\varepsilon_{n,n}}\to \operatorname{\mathrm{GW}}_n^n(L)\stackrel{\tilde{\chi}_{n,n}}\to\mathrm{K}^{\mathrm{MW}}_n(L) \end{align*} $$
$$ \begin{align*} \mathrm{K}^{\mathrm{MW}}_n(L)\stackrel{\varepsilon_{n,n}}\to \operatorname{\mathrm{GW}}_n^n(L)\stackrel{\tilde{\chi}_{n,n}}\to\mathrm{K}^{\mathrm{MW}}_n(L) \end{align*} $$
is multiplication by
![]() $\psi _{\mu (n)}!\in \operatorname {\mathrm {GW}}(k)$
according to formula (4.4.5.b), where
$\psi _{\mu (n)}!\in \operatorname {\mathrm {GW}}(k)$
according to formula (4.4.5.b), where
![]() $\mu (n)$
is the smallest integer congruent to 2 modulo 4 and greater than n.
$\mu (n)$
is the smallest integer congruent to 2 modulo 4 and greater than n.
Proof. We have adjunctions
 $$ \begin{align*} \mathcal H_{\mathbb A^1}(k) \leftrightarrows \mathrm{D}_{\mathbb A^1}^{\mathrm{eff}}(k) \leftrightarrows \widetilde{\mathrm{DM}}^{\mathrm{eff}}(k), \end{align*} $$
$$ \begin{align*} \mathcal H_{\mathbb A^1}(k) \leftrightarrows \mathrm{D}_{\mathbb A^1}^{\mathrm{eff}}(k) \leftrightarrows \widetilde{\mathrm{DM}}^{\mathrm{eff}}(k), \end{align*} $$
the first one being the classical Dold-Kan correspondence and the second one being the adjunction of [Reference Déglise and Fasel13, §3.2.4]. We can thus consider the resulting adjunction
 $$ \begin{align*} \mathcal H_{\mathbb A^1}(k)\leftrightarrows \widetilde{\mathrm{DM}}^{\mathrm{eff}}(k). \end{align*} $$
$$ \begin{align*} \mathcal H_{\mathbb A^1}(k)\leftrightarrows \widetilde{\mathrm{DM}}^{\mathrm{eff}}(k). \end{align*} $$
The image of the object
![]() $(\mathbb P^1)^{\wedge n}$
of
$(\mathbb P^1)^{\wedge n}$
of
![]() $\mathcal H_{\mathbb A^1}(k)$
is precisely
$\mathcal H_{\mathbb A^1}(k)$
is precisely
![]() $\tilde {\mathbb Z}(n)[2n]$
and the unit of the adjunction induces a map
$\tilde {\mathbb Z}(n)[2n]$
and the unit of the adjunction induces a map
 $$ \begin{align*} \eta_n:(\mathbb P^1)^{\wedge n}\to K\big(\tilde{\mathbb Z}(n),2n\big), \end{align*} $$
$$ \begin{align*} \eta_n:(\mathbb P^1)^{\wedge n}\to K\big(\tilde{\mathbb Z}(n),2n\big), \end{align*} $$
which is in fact the degree n morphism of the unit map of the spectrum
![]() $\mathbf {H}_{\mathrm {MW}}$
. It is an explicit generator of
$\mathbf {H}_{\mathrm {MW}}$
. It is an explicit generator of
 $[(\mathbb P^1)^{\wedge n},K\big (\tilde {\mathbb Z}(n),2n\big )]\simeq \operatorname {\mathrm {GW}}(k)$
and we claim that it induces an isomorphism upon applying
$[(\mathbb P^1)^{\wedge n},K\big (\tilde {\mathbb Z}(n),2n\big )]\simeq \operatorname {\mathrm {GW}}(k)$
and we claim that it induces an isomorphism upon applying
 $\boldsymbol {\pi }^{{\mathbb A}^1}_n$
. In view of the Hurewicz homomorphism in
$\boldsymbol {\pi }^{{\mathbb A}^1}_n$
. In view of the Hurewicz homomorphism in
![]() $\mathbb A^1$
-homotopy theory and [Reference Morel25, Theorem 6.37], we are reduced to show that the unit map
$\mathbb A^1$
-homotopy theory and [Reference Morel25, Theorem 6.37], we are reduced to show that the unit map
 $$ \begin{align*} C^{\mathbb A^1}_*((\mathbb P^1)^{\wedge n})\to \tilde{\mathbb Z}(n)[2n] \end{align*} $$
$$ \begin{align*} C^{\mathbb A^1}_*((\mathbb P^1)^{\wedge n})\to \tilde{\mathbb Z}(n)[2n] \end{align*} $$
in the adjunction
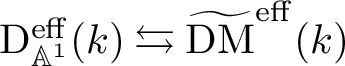 $\mathrm {D}_{\mathbb A^1}^{\mathrm {eff}}(k) \leftrightarrows \widetilde {\mathrm {DM}}^{\mathrm {eff}}(k)$
induces an isomorphism upon applying
$\mathrm {D}_{\mathbb A^1}^{\mathrm {eff}}(k) \leftrightarrows \widetilde {\mathrm {DM}}^{\mathrm {eff}}(k)$
induces an isomorphism upon applying
![]() $\mathrm {H}_{n}$
. This is tantamount to showing that the unit map
$\mathrm {H}_{n}$
. This is tantamount to showing that the unit map
 $$ \begin{align*} C^{\mathbb A^1}_*((\mathbb{G}_m)^{\wedge n})\to \tilde{\mathbb Z}(n)[n] \end{align*} $$
$$ \begin{align*} C^{\mathbb A^1}_*((\mathbb{G}_m)^{\wedge n})\to \tilde{\mathbb Z}(n)[n] \end{align*} $$
induces an isomorphism on
![]() $\mathrm {H}_0$
, which is the case by [Reference Déglise and Fasel13, Theorem 4.2.3].
$\mathrm {H}_0$
, which is the case by [Reference Déglise and Fasel13, Theorem 4.2.3].
Now, we have a commutative diagram

by (a repeated use of) Theorem 4.3.2 and
![]() $\tilde {\chi }_2\circ \varepsilon _2$
corresponds to
$\tilde {\chi }_2\circ \varepsilon _2$
corresponds to
![]() $b_1(\mathfrak U)$
, which is in fact
$b_1(\mathfrak U)$
, which is in fact
![]() $\eta _2$
. Thus,
$\eta _2$
. Thus,
![]() $\tilde {\chi }_{n}\circ \varepsilon _{n}$
corresponds to
$\tilde {\chi }_{n}\circ \varepsilon _{n}$
corresponds to
![]() $\psi _{\mu (n)}!\eta _2$
and consequently we have
$\psi _{\mu (n)}!\eta _2$
and consequently we have
The right-hand side is in fact the composite
 $$ \begin{align*} (\mathbb P^1)^{\wedge n}\xrightarrow{\psi_{\mu(n)}!} (\mathbb P^1)^{\wedge n}\xrightarrow{\eta_n} K\big(\tilde{\mathbb Z}(\mu(n)),2\mu(n)\big), \end{align*} $$
$$ \begin{align*} (\mathbb P^1)^{\wedge n}\xrightarrow{\psi_{\mu(n)}!} (\mathbb P^1)^{\wedge n}\xrightarrow{\eta_n} K\big(\tilde{\mathbb Z}(\mu(n)),2\mu(n)\big), \end{align*} $$
where the first map (still denoted by
![]() $\psi _{\mu (n)}!$
) is obtained via the isomorphism
$\psi _{\mu (n)}!$
) is obtained via the isomorphism
![]() $[(\mathbb P^1)^{\wedge n},(\mathbb P^1)^{\wedge n}]_{\mathbb A^1}\simeq \operatorname {\mathrm {GW}}(k)$
of [Reference Morel25, Corollary 6.43].
$[(\mathbb P^1)^{\wedge n},(\mathbb P^1)^{\wedge n}]_{\mathbb A^1}\simeq \operatorname {\mathrm {GW}}(k)$
of [Reference Morel25, Corollary 6.43].
Remark 6.1.2. Let
be the unit map of the spectrum representing K-theory. Using the operations
![]() $\chi $
from K-theory to motivic cohomology, one can repeat the above construction to get homomorphisms
$\chi $
from K-theory to motivic cohomology, one can repeat the above construction to get homomorphisms
 $$ \begin{align*} \mathrm{K}^{\mathrm{MW}}_n(L)\stackrel{\psi_{n,n}}\to \operatorname{\mathrm{K}}_n(L)\stackrel{\tilde{\chi}_{n,n}}\to\mathrm{K}^{\mathrm{M}}_n(L). \end{align*} $$
$$ \begin{align*} \mathrm{K}^{\mathrm{MW}}_n(L)\stackrel{\psi_{n,n}}\to \operatorname{\mathrm{K}}_n(L)\stackrel{\tilde{\chi}_{n,n}}\to\mathrm{K}^{\mathrm{M}}_n(L). \end{align*} $$
The first map factors through Milnor K-theory and we get a commutative diagram

We note that the composite is then equal to
![]() $\mu (n)!$
, showing that the homomorphism
$\mu (n)!$
, showing that the homomorphism
![]() $\operatorname {\mathrm {K}}_{n}(L)\to \mathrm {K}^{\mathrm {M}}_{n}(L)$
is not optimal, in comparison with the map
$\operatorname {\mathrm {K}}_{n}(L)\to \mathrm {K}^{\mathrm {M}}_{n}(L)$
is not optimal, in comparison with the map
![]() $\operatorname {\mathrm {K}}_{n}(L)\to \mathrm {K}^{\mathrm {M}}_{n}(L)$
defined by Suslin in [Reference Suslin37]. If
$\operatorname {\mathrm {K}}_{n}(L)\to \mathrm {K}^{\mathrm {M}}_{n}(L)$
defined by Suslin in [Reference Suslin37]. If
![]() $n=\mu (n)$
, we guess that the above homomorphism coincides with the one defined by Suslin, up to a sign.
$n=\mu (n)$
, we guess that the above homomorphism coincides with the one defined by Suslin, up to a sign.
Remark 6.1.3. The homomorphism
![]() $\psi _{n,n}:\mathrm {K}^{\mathrm {MW}}_n(L)\to \operatorname {\mathrm {GW}}_{n}^n(L)$
is actually an isomorphism for
$\psi _{n,n}:\mathrm {K}^{\mathrm {MW}}_n(L)\to \operatorname {\mathrm {GW}}_{n}^n(L)$
is actually an isomorphism for
![]() $n\leq 3$
by [Reference Asok and Fasel6, §4]. An unpublished result of O. Röndigs states that this homomorphism is an isomorphism for
$n\leq 3$
by [Reference Asok and Fasel6, §4]. An unpublished result of O. Röndigs states that this homomorphism is an isomorphism for
![]() $n=4$
as well.
$n=4$
as well.
Corollary 6.1.4. Let L be a field and let
![]() $n\geq 2$
be such that
$n\geq 2$
be such that
![]() $\mathrm {K}^{\mathrm {MW}}_n(L)$
is
$\mathrm {K}^{\mathrm {MW}}_n(L)$
is
![]() $\psi _{\mu (n)}!$
-torsion free. Then,
$\psi _{\mu (n)}!$
-torsion free. Then,
![]() $\mathrm {K}^{\mathrm {MW}}_n(L)$
injects into
$\mathrm {K}^{\mathrm {MW}}_n(L)$
injects into
![]() $\operatorname {\mathrm {GW}}_n^n(L)$
.
$\operatorname {\mathrm {GW}}_n^n(L)$
.
Remark 6.1.5. As an example, we may consider
![]() $\mathbb R$
, or actually any real closed field. Indeed, we know from [Reference Kolderup19, Proposition 2.2] that
$\mathbb R$
, or actually any real closed field. Indeed, we know from [Reference Kolderup19, Proposition 2.2] that
![]() $\mathrm {K}^{\mathrm {MW}}_n(\mathbb R)\simeq \mathbb Z\oplus D$
, where D is a uniquely divisible group. It follows that
$\mathrm {K}^{\mathrm {MW}}_n(\mathbb R)\simeq \mathbb Z\oplus D$
, where D is a uniquely divisible group. It follows that
![]() $\mathrm {K}^{\mathrm {MW}}_n(\mathbb R)$
always injects into
$\mathrm {K}^{\mathrm {MW}}_n(\mathbb R)$
always injects into
![]() $\operatorname {\mathrm {GW}}_n^n(\mathbb R)$
, extending [Reference Asok and Fasel6, Example 4.3.3]. Another example is given by
$\operatorname {\mathrm {GW}}_n^n(\mathbb R)$
, extending [Reference Asok and Fasel6, Example 4.3.3]. Another example is given by
![]() $F=\mathbb Q$
for
$F=\mathbb Q$
for
![]() $n\geq 3$
by [Reference Kolderup19, Proposition 2.4] or by any algebraically closed field with
$n\geq 3$
by [Reference Kolderup19, Proposition 2.4] or by any algebraically closed field with
![]() $n\geq 2$
.
$n\geq 2$
.
Appendix A. The threefold product
The purpose of this appendix is to explicitly decompose the threefold product
where
![]() $(U,\varphi )$
is a symplectic bundle of rank
$(U,\varphi )$
is a symplectic bundle of rank
![]() $2$
. The first lemma is obvious and we omit its proof.
$2$
. The first lemma is obvious and we omit its proof.
Lemma A.0.1. Let U be a rank
![]() $2$
bundle and let
$2$
bundle and let
![]() $i:\wedge ^2U\to U\otimes U$
be the homomorphism given on sections by
$i:\wedge ^2U\to U\otimes U$
be the homomorphism given on sections by
![]() $s_1\wedge s_2\mapsto s_1\otimes s_2-s_2\otimes s_1$
. Then, we have an exact sequence
$s_1\wedge s_2\mapsto s_1\otimes s_2-s_2\otimes s_1$
. Then, we have an exact sequence
 $$ \begin{align*} 0\to \wedge^2U\stackrel{i}\to U^{\otimes 2}\to \mathrm{Sym}^2U\to 0. \end{align*} $$
$$ \begin{align*} 0\to \wedge^2U\stackrel{i}\to U^{\otimes 2}\to \mathrm{Sym}^2U\to 0. \end{align*} $$
Tensoring the above by U (say on the right), we obtain an exact sequence
 $$ \begin{align*} 0\to (\wedge^2U)\otimes U\xrightarrow{i\otimes 1} U^{\otimes 3}\to (\mathrm{Sym}^2U)\otimes U\to 0. \end{align*} $$
$$ \begin{align*} 0\to (\wedge^2U)\otimes U\xrightarrow{i\otimes 1} U^{\otimes 3}\to (\mathrm{Sym}^2U)\otimes U\to 0. \end{align*} $$
Now, we may define a homomorphism
![]() $j:(\wedge ^2U)\otimes U\to (\mathrm {Sym}^2U)\otimes U$
on sections by
$j:(\wedge ^2U)\otimes U\to (\mathrm {Sym}^2U)\otimes U$
on sections by
Lemma A.0.2. We have an exact sequence
 $$ \begin{align*} 0\to (\wedge^2U)\otimes U\stackrel{j}\to (\mathrm{Sym}^2U)\otimes U\to \mathrm{Sym}^3U\to 0. \end{align*} $$
$$ \begin{align*} 0\to (\wedge^2U)\otimes U\stackrel{j}\to (\mathrm{Sym}^2U)\otimes U\to \mathrm{Sym}^3U\to 0. \end{align*} $$
Proof. We may work locally and thus suppose that
![]() $U=R^2$
(where R is a local ring) with basis
$U=R^2$
(where R is a local ring) with basis
![]() $e,f$
. We then have a basis
$e,f$
. We then have a basis
![]() $e\wedge f$
of
$e\wedge f$
of
![]() $(\wedge ^2U)$
and a basis
$(\wedge ^2U)$
and a basis
![]() $(e\wedge f)\otimes e, (e\wedge f)\otimes f$
of
$(e\wedge f)\otimes e, (e\wedge f)\otimes f$
of
![]() $(\wedge ^2U)\otimes U$
. Their respective images are
$(\wedge ^2U)\otimes U$
. Their respective images are
![]() $(e e)\otimes f-(e f)\otimes e$
and
$(e e)\otimes f-(e f)\otimes e$
and
![]() $(e f)\otimes f-(f f)\otimes e$
, which are linearly independent. Now, the composite of the morphisms are clearly trivial and therefore the sequence is exact by an easy dimension count.
$(e f)\otimes f-(f f)\otimes e$
, which are linearly independent. Now, the composite of the morphisms are clearly trivial and therefore the sequence is exact by an easy dimension count.
Lemma A.0.3. Suppose that k is of characteristic different from
![]() $2$
. Then, the restriction of
$2$
. Then, the restriction of
![]() $\varphi ^{\otimes 3}$
to
$\varphi ^{\otimes 3}$
to
![]() $(\wedge ^2U)\otimes U$
along
$(\wedge ^2U)\otimes U$
along
is isometric to
![]() $\langle 2\rangle \varphi :U\to U^\vee $
. In particular, it is nondegenerate.
$\langle 2\rangle \varphi :U\to U^\vee $
. In particular, it is nondegenerate.
Proof. Again, we suppose that we work over a local ring R and thus that U has a basis
![]() $e,f$
. The form
$e,f$
. The form
![]() $\varphi $
is then characterised by
$\varphi $
is then characterised by
![]() $\psi :\wedge ^2U\to R$
given by
$\psi :\wedge ^2U\to R$
given by
![]() $\psi (e\wedge f)=\varphi (e,f)$
. An easy computation shows that
$\psi (e\wedge f)=\varphi (e,f)$
. An easy computation shows that
is characterised by
![]() $((e\wedge f)\otimes e)\wedge ((e\wedge f)\otimes f)\mapsto 2\varphi (e,f)^3$
. We can now define an isomorphism
$((e\wedge f)\otimes e)\wedge ((e\wedge f)\otimes f)\mapsto 2\varphi (e,f)^3$
. We can now define an isomorphism
![]() $U\to (\wedge ^2U)\otimes U$
by
$U\to (\wedge ^2U)\otimes U$
by
![]() $s\mapsto \psi ^{-1}(1)\otimes s$
and check that the restriction is precisely
$s\mapsto \psi ^{-1}(1)\otimes s$
and check that the restriction is precisely
![]() $\langle 2\rangle \varphi $
.
$\langle 2\rangle \varphi $
.
Remark A.0.4. In characteristic
![]() $2$
, the above lemma shows that
$2$
, the above lemma shows that
![]() $(\wedge ^2U)\otimes U$
is a sublagrangian of
$(\wedge ^2U)\otimes U$
is a sublagrangian of
![]() $(U^{\otimes 3},\varphi ^{\otimes 3})$
.
$(U^{\otimes 3},\varphi ^{\otimes 3})$
.
Consequently, we see that if k is of characteristic different from
![]() $2$
, then we get a decomposition
$2$
, then we get a decomposition
![]() $(U^{\otimes 3},\varphi ^{\otimes 3})\simeq (U,\langle 2\rangle \varphi )\perp ((\mathrm {Sym}^2U)\otimes U,\varphi ^\prime )$
for some form
$(U^{\otimes 3},\varphi ^{\otimes 3})\simeq (U,\langle 2\rangle \varphi )\perp ((\mathrm {Sym}^2U)\otimes U,\varphi ^\prime )$
for some form
![]() $\varphi ^\prime $
that we now determine. We have an obvious homomorphism
$\varphi ^\prime $
that we now determine. We have an obvious homomorphism
![]() $\mathrm {Sym}^2U\to U^{\otimes 2}$
(always under the hypothesis that k is of characteristic different from
$\mathrm {Sym}^2U\to U^{\otimes 2}$
(always under the hypothesis that k is of characteristic different from
![]() $2$
) given by
$2$
) given by
![]() $s_1 s_2\mapsto s_1\otimes s_2+s_2\otimes s_1$
. This induces a section of
$s_1 s_2\mapsto s_1\otimes s_2+s_2\otimes s_1$
. This induces a section of
![]() $ U^{\otimes 3}\to (\mathrm {Sym}^2U)\otimes U$
and we may consider the form induced by this section. However, it is easier to consider the composite of
$ U^{\otimes 3}\to (\mathrm {Sym}^2U)\otimes U$
and we may consider the form induced by this section. However, it is easier to consider the composite of
 $(\wedge ^2U)\otimes U\stackrel {j}\to (\mathrm {Sym}^2U)\otimes U$
with this section and the form induced on
$(\wedge ^2U)\otimes U\stackrel {j}\to (\mathrm {Sym}^2U)\otimes U$
with this section and the form induced on
![]() $(\wedge ^2U)\otimes U$
.
$(\wedge ^2U)\otimes U$
.
Lemma A.0.5. Suppose that k is of characteristic different from
![]() $2,3$
. The symplectic form induced on
$2,3$
. The symplectic form induced on
![]() $\wedge ^2U\otimes U$
by
$\wedge ^2U\otimes U$
by
![]() $(U^{\otimes 3},\varphi ^{\otimes 3})$
under the homomorphism
$(U^{\otimes 3},\varphi ^{\otimes 3})$
under the homomorphism
defined by
![]() $(s_1\wedge s_2)\otimes s_3\mapsto s_1\otimes s_3\otimes s_2+s_3\otimes s_1\otimes s_2-s_2\otimes s_3\otimes s_1-s_3\otimes s_2\otimes s_1$
is isometric to
$(s_1\wedge s_2)\otimes s_3\mapsto s_1\otimes s_3\otimes s_2+s_3\otimes s_1\otimes s_2-s_2\otimes s_3\otimes s_1-s_3\otimes s_2\otimes s_1$
is isometric to
![]() $\langle 6\rangle (U,\varphi )$
.
$\langle 6\rangle (U,\varphi )$
.
Proof. The same proof as in Lemma A.0.3 shows that the form on
![]() $\wedge ^2U\otimes U$
is locally characterised by
$\wedge ^2U\otimes U$
is locally characterised by
![]() $((e\wedge f)\otimes e)\wedge ((e\wedge f)\otimes f)\mapsto 6\varphi (e,f)^3$
.
$((e\wedge f)\otimes e)\wedge ((e\wedge f)\otimes f)\mapsto 6\varphi (e,f)^3$
.
As a consequence, we have
where
![]() $\psi $
is induced by
$\psi $
is induced by
![]() $(U^{\otimes 3},\varphi ^{\otimes 3})$
under the choice of a reasonable section
$(U^{\otimes 3},\varphi ^{\otimes 3})$
under the choice of a reasonable section
![]() $U^{\otimes 3}\to \mathrm {Sym}^3U$
. There is a canonical such map given on sections by
$U^{\otimes 3}\to \mathrm {Sym}^3U$
. There is a canonical such map given on sections by
 $$ \begin{align*} s_1\otimes s_2\otimes s_3\mapsto \sum_{\sigma\in S_3}s_{\sigma(1)}\otimes s_{\sigma(2)}\otimes s_{\sigma(3)} \end{align*} $$
$$ \begin{align*} s_1\otimes s_2\otimes s_3\mapsto \sum_{\sigma\in S_3}s_{\sigma(1)}\otimes s_{\sigma(2)}\otimes s_{\sigma(3)} \end{align*} $$
under the hypothesis that
![]() $\mathrm {char}(k)\neq 2,3$
.
$\mathrm {char}(k)\neq 2,3$
.
Acknowledgments
The authors are grateful to F. Jin and A. Khan for discussions during our collaboration on absolute purity theorems [Reference Déglise, Fasel, Khan and Jin15] that were linked with the present work. The second author warmly thanks I. Panin for a very interesting conversation on the analogue of the Chern character linking Grothendieck-Witt groups and Chow-Witt groups. This conversation led to many considerations appearing in this work. We express our gratitude to C. Walter for sharing with us his ideas and a preliminary work on ternary laws. We also want to thank A. Ananyevskiy, A. Asok, J. Hornbostel, M. Levine and M. Wendt for discussions about the subject of this article. Finally, we thank the referee for a very careful reading that helped us to get the following well-rounded version.
F. Déglise received support from the French ‘Investissements d’Avenir’ program, project ISITE-BFC (contract ANR-lS-IDEX-OOOB).