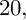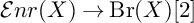1 Introduction
Let S be a complex Enriques surface, and let
$\pi \colon X\to S$
be its K3 étale double cover. J.-L. Colliot-Thélène asked whether the induced map of Brauer groups
$\pi ^*\colon \mathrm {{Br}}(S)\simeq {\mathbb Z}/2\to \mathrm {{Br}}(X)$
is injective or zeroFootnote
1
. Beauville has given a necessary and sufficient condition for the injectivity of
$\pi ^*$
[Reference BeauvilleB, Cor. 5.7] and showed that the Enriques surfaces S for which this map is zero form a countable union of hypersurfaces in the moduli space of Enriques surfaces [Reference BeauvilleB, Cor. 6.5]. Enriques surfaces with injective
$\pi ^*$
are used in explicit constructions of Enriques surfaces over
${\mathbb Q}$
for which the Brauer–Manin obstruction fails to control weak approximation [Reference Harari and SkorobogatovHS1] and the Hasse principle [Reference Várilly-Alvarado and VirayVV]). Enriques surfaces over
${\mathbb Q}$
such that the map
$\pi ^*$
is zero have been constructed in [Reference Hulek and SchüttHS2, Reference Garbagnati and SchüttGS].
From a different perspective, one can start with a K3 surface X and consider the set
$\mathcal F(X)\subset \mathrm {Aut}(X)$
of fixed point free involutions
$\sigma \colon X\to X$
, which are precisely the involutions such that the quotient
$X/\sigma $
is an Enriques surface.
In this paper, we are interested in the map
$$ \begin{align*} \Phi_X\colon{\mathcal F}(X) \longrightarrow \mathrm{{Br}}(X)[2], \end{align*} $$
which sends
$\sigma \in \mathcal F(X)$
to
$\pi ^*(b_S)$
, where
$\pi \colon X\to X/\sigma =S$
is the quotient morphism, and
$b_S$
is the unique nonzero element of
$\mathrm {{Br}}(S)$
. A combination of results of Beauville and of Keum and Ohashi show that
$\mathrm {{Im}}(\Phi _X)$
depends only on the isomorphism class of the transcendental lattice
$T(X)$
of X (see Corollary 2.6). A description of all lattices
$T(X)$
such that
${\mathcal F}(X)\neq \emptyset $
can be found in [Reference Brandhorst, Sonel and VenianiBSV, Th. 1.6].
Let
${\mathcal Enr}(X)$
be the set of Enriques quotients of X, considered up to isomorphism of varieties. Equivalently,
${\mathcal Enr}(X)$
is the set of conjugacy classes of
$\mathrm {Aut}(X)$
contained in
$\mathcal F(X)$
(see [Reference OhashiO1, Prop. 2.1]). Ohashi proved that the set
${\mathcal Enr}(X)$
is always finite [Reference OhashiO1, Cor. 0.4] although its size is not bounded [Reference OhashiO1, Th. 0.1]. The map
$\Phi _X$
is
$\mathrm { Aut}(X)$
-equivariant, where
$\mathrm {Aut}(X)$
acts on
$\mathcal F(X)$
by conjugation, so
$\Phi _X$
descends to a map
$$ \begin{align*}\varphi_X\colon{\mathcal Enr}(X) \longrightarrow \mathrm{{Br}}(X)[2]/\mathrm{ Aut}(X).\\[-4pt]\end{align*} $$
The action of
$\mathrm {Aut}(X)$
on
$\mathrm {{Br}}(X)[2]$
factors through the action of the group of Hodge isometries of the integral Hodge structure on
$T(X)$
, so when
$\mathrm {Aut}_{\mathrm {Hdg}}(T(X))=\{\pm 1\}$
the action of
$\mathrm {Aut}(X)$
on
$\mathrm {{Br}}(X)[2]$
is trivial. In such a generic situation,
$\varphi _X$
is a map
${\mathcal Enr}(X) \to \mathrm {{Br}}(X)[2]$
. In this case, the set
${\mathcal Enr}(X)$
depends only on the isomorphism class of the lattice
$T(X)$
(see the discussion after Theorem 2.5).
Examples show that the set
${\mathcal Enr}(X)$
can be empty or very large, so in general
$\varphi _X$
is neither surjective nor injective. A very general Enriques surface S (corresponding to the points of the moduli space outside a countable union of hypersurfaces) is the unique Enriques quotient of its K3 cover X; by Beauville, in this case,
$\varphi _X({\mathcal Enr}(X))$
is a certain nonzero element of
$\mathrm {{Br}}(X)[2]$
.
The aim of this paper is to clarify the structure of
$\Phi _X$
and
$\varphi _X$
in some favourable situations. Keum [Reference KeumK, Th. 2] proved that every Kummer surface is a double cover of some Enriques surface. His method can be used to prove the following.
Theorem A. Let X be a Kummer surface. Then, for every
$\alpha \in \mathrm {{Br}}(X)$
of order
$2,$
there is an Enriques quotient
$\pi _S\colon X\to S$
such that
$\alpha =\pi _S^*(b_S)$
.
In other words, for Kummer surfaces, the set
$\mathrm {{Br}}(X)[2]\setminus \{0\}$
is contained in the image of
$\Phi _X$
. As a kind of partial converse, in Corollary 2.7, we show that if X is a K3 surface such that the abelian group
$\mathrm {{Br}}(X)[2]$
is generated by the image of
$\Phi _X$
, then the transcendental lattice of X is divisible by 2 as an even lattice. We do not know if there exist Kummer surfaces such that
$\Phi _X^{-1}(0)$
is non-empty. At the end of §2, we give examples of non-Kummer K3 surfaces such that
$\mathrm {{Im}}(\Phi _X)=\{0\}$
.
In two generic cases, Ohashi classified all Enriques quotients of a given K3 surface. Combining Theorem A with his results [Reference OhashiO1, Th. 4.1], [Reference OhashiO2, Th. 1.1] we obtain the following corollary.
Corollary B. Let X be the Kummer surface attached to any of the following abelian surfaces:
-
(i) a product of two non-isogenous elliptic curves;
-
(ii) the Jacobian J of a curve of genus
$2$
such that
$\mathrm {NS\,}(J)\cong {\mathbb Z}$
.
Then
$\varphi _X$
is a bijection between
${\mathcal Enr}(X)$
and
$\mathrm {{Br}}(X)[2]\setminus \{0\}$
.
For some K3 surfaces of maximal Picard rank, the following result gives information about the fibers of
$\varphi _X$
. Its proof uses a certain Galois action on
$\mathrm {{Br}}(X)[2]$
constructed by the second named author in [Reference ValloniV].
Theorem C. Let X be a K3 surface of Picard rank
$20$
. Let
$E={\mathbb Q}(\sqrt {-d})$
, where d is the discriminant of the transcendental lattice
$T(X)$
. Assume that
$\mathrm {{End}}_{\mathrm {Hdg}} (T(X))$
is the ring of integers
${\mathcal O}_E\subset E$
and, moreover,
$2$
is inert in E and
$E \neq {\mathbb Q}(\sqrt {-3})$
. Then
$\mathrm {Aut}_{\mathrm {Hdg}} (T(X))=\{\pm 1\}$
and the fibers of
$\varphi _X\colon {\mathcal Enr}(X) \to \mathrm {{Br}}(X)[2]$
above the nonzero points have the same cardinality.
The conditions in Theorem C are easy to check. Let
$$ \begin{align*} \left(\begin{array}{cc} 2a&b\\b&2c\end{array}\right) \end{align*} $$
be the Gram matrix of
$T(X)$
, where
$a,b,c\in {\mathbb Z}$
, so that
$-d=b^2-4ac<0$
. Write
$-d=f^2D$
, where
$f\in {\mathbb Z}$
and D is the discriminant of E. By [Reference ValloniV, Th. 3.2] we have
$\mathrm {{End}}_{\mathrm {Hdg}} (T(X))={\mathcal O}_E$
if and only if
$f=\mathrm {gcd}(a,b,c)$
. Next,
$2$
is inert in E if and only if
$D\equiv 5 \bmod 8$
. If f is odd, so that
$-d\equiv 5 \bmod 8$
, we have
${\mathcal Enr}(X)=\emptyset $
by [Reference Shimada and VenianiS], so in this case, the fibers of
$\varphi _X$
are empty. Using Theorem A, it is easy to see that for each
$D\equiv 5 \bmod 8$
,
$D\neq -3$
, there are infinitely many pairwise non-isomorphic K3 surfaces of Picard rank
$20$
with complex multiplication by
${\mathcal O}_{{\mathbb Q}(\sqrt {D})}$
such that the fibers of
$\varphi _X$
above the nonzero points of
$\mathrm {{Br}}(X)[2]$
have the same positive number of elements.
It would be interesting to describe the K3 surfaces X such that
$\Phi _X$
is surjective onto
$\mathrm {{Br}}(X)[2]$
or onto
$\mathrm {{Br}}(X)[2]\setminus \{0\}$
. In this direction, we have the following result, whose proof uses Nikulin’s theory of lattices [Reference NikulinN] and surjectivity of the period map for K3 surfaces.
Theorem D. Let X be a K3 surface such that
$\mathrm {{rk}}(\mathrm {NS\,}(X))\geq 12$
. Then there exist infinitely many K3 surfaces Y such that:
-
(1)
$T(X)_{\mathbb Q} \cong T(Y)_{\mathbb Q}$
as polarized Hodge structures. -
(2) The discriminants of
$T(Y)$
are pairwise different. -
(3) There is a natural isomorphism
$\mathrm {{Br}}(X)[2]\cong \mathrm {{Br}}(Y)[2]$
under which
$$ \begin{align*} \mathrm{{Im}}(\Phi_X)\setminus\{0\}=\mathrm{{Im}}(\Phi_Y)\setminus\{0\}. \end{align*} $$
We recall results of Beauville, Keum, and Ohashi, and then prove some useful lemmas in §2. Theorem A and Corollary B are proved in §3, Theorem C is proved in §4, and Theorem D in §5.
2 Lattices and the topology of Enriques quotients
A lattice L is a free finitely generated abelian group with a non-degenerate integral symmetric bilinear form. Write
$L(2)$
for the same group with the form
$2(x.y)$
.
For a lattice L, we denote by
$A_L=L^*/L$
the discriminant group of L. If L is even, then
$q_L\colon A_L\to {\mathbb Q}/2{\mathbb Z}$
is the associated quadratic form.
If
$L\subset M$
are lattices, we denote by
$L^{\perp }_M$
the orthogonal complement to L in M. It is clear that
$L^{\perp }_M$
is a primitive sublattice of M.
Let
$\mathrm {U}$
be the hyperbolic plane. Write
$\mathrm {U}={\mathbb Z} e\oplus {\mathbb Z} f$
, where
$(e^2)=(f^2)=0$
,
$(e.f)=1$
. We denote by
$\mathrm {E}_8$
the negative-definite, even, unimodular lattice of the root system
$\mathrm { E}_8$
. Write
$$ \begin{align*} \Lambda=\mathrm{E}_8^{\oplus 2}\oplus \mathrm{U}^{\oplus 3}, \quad\quad M=\mathrm{U}(2)\oplus \mathrm{E}_8(2), \quad\quad N=\mathrm{U}\oplus \mathrm{U}(2)\oplus \mathrm{ E}_8(2). \end{align*} $$
Here,
$\Lambda $
is the K3 lattice. Let
$\iota \colon \Lambda \to \Lambda $
be the involution permuting two copies of
$\mathrm {E}_8\oplus \mathrm {U}$
, and acting as
$-1$
on the third copy of
$\mathrm {U}$
. Then
$\Lambda ^+\cong M$
and
$\Lambda ^-\cong N$
, where
$\Lambda ^{\pm }$
is the
$\pm 1$
-eigenspace of
$\iota $
. By [Reference HuybrechtsH2, (vii) on p. 305], for any Enriques quotient
$\pi _S\colon X\to S=X/\sigma $
, the induced map
$$ \begin{align*} \pi_S\colon \mathrm{H}^2(S,{\mathbb Z})/_{\mathrm{tors}}\,\longrightarrow \mathrm{ H}^2(X,{\mathbb Z})\end{align*} $$
can be identified with the composition
$$ \begin{align*} \mathrm{H}^2(S,{\mathbb Z})/_{\mathrm{tors}}\simeq\mathrm{U}\oplus \mathrm{E}_8\stackrel{\mathrm{diag}}\longrightarrow (\mathrm{U}\oplus {\mathrm{E}}_8)^{\oplus 2}\subset \Lambda\simeq{\mathrm{H}}^2(X,{\mathbb Z}). \end{align*} $$
Here, the fixed point free involution
$\sigma \colon X\to X$
induces the involution
$\iota $
on
$\Lambda $
.
The lattice N has a canonical character
$N\to {\mathbb Z}/2$
which will play a crucial role in what follows.
Lemma 2.1. The homomorphism
$\varepsilon \colon N\to {\mathbb Z}/2$
given by
$\varepsilon (x):=\big (x.(e+f)\big )\bmod 2$
, where e and f are standard generators of
$\mathrm {U}\subset N$
, does not depend on the embedding of lattices
$\mathrm {U}\hookrightarrow N$
. Hence,
$\alpha ^*(\varepsilon )=\varepsilon $
for any
$\alpha \in \mathrm {Aut}(N)$
.
Proof. Let
$e',f'$
be standard generators of
$\mathrm {U}$
embedded in N. Write
$e'=ae+bf+u$
,
$f'=ce+df+w$
, where
$a,b,c,d\in {\mathbb Z}$
and
$u,w\in \mathrm {U}(2)\oplus \mathrm { E}_8(2)$
. We have
$2ab+(u^2)=2cd+(w^2)=0$
and
$ad+bc+(u.w)=1$
. Since
$(u^2)$
and
$(w^2)$
are divisible by 4, and
$(u.w)$
is even, we see that
$ab$
is even,
$cd$
is even, and
$ad+bc$
is odd. It follows that either
$a,d$
are odd and
$b,c$
are even, or
$a,d$
are even and
$b,c$
are odd. In both cases,
$e'+f'$
equals
$e+f$
modulo
$2 \mathrm {U} \oplus \mathrm {U}(2)\oplus \mathrm {E}_8(2)$
, hence the result.
Lemma 2.2. If
$x\in N$
is such that
$(x^2)\equiv 2\bmod 4$
, then
$\varepsilon (x)=0$
.
Proof. Write
$x=ae+bf+u$
, where
$a,b\in {\mathbb Z}$
and
$u\in \mathrm {U}(2)\oplus \mathrm {E}_8(2)$
. Then a and b are both odd, hence
$\varepsilon (x)\equiv a+b\equiv 0\bmod 2$
.
Lemma 2.3. Let L be a sublattice of N. If the restriction of
$\varepsilon \colon N\to {\mathbb Z}/2$
to L is nonzero, then
$L^{\perp }_N=L'(2)$
for some even lattice
$L'$
.
Proof. Suppose
$\varepsilon (x)\neq 0$
for some
$x\in L$
. Writing
$x=ae+bf+u$
, where
$a,b\in {\mathbb Z}$
and
$u\in \mathrm {U}(2)\oplus \mathrm {E}_8(2)$
, we see that a and b have opposite pairity. If
$y=ce+df+w \in L^{\perp }_N$
, where
$c,d\in {\mathbb Z}$
and
$w\in \mathrm {U}(2)\oplus \mathrm {E}_8(2)$
, then
$ad+bc$
is even, which implies that either c or d is even. Then
$(y^2)=2cd +(w^2)$
is divisible by
$4$
, hence
$L^{\perp }_N=L'(2)$
for some even lattice
$L'$
.
The importance of the character
$\varepsilon \colon N\to {\mathbb Z}/2$
has been revealed by Beauville. Namely, let
$\pi _S\colon X\to S=X/\sigma $
be an Enriques quotient of a K3 surface X. Let
$T(X)\subset \Lambda $
be the transcendental lattice of X. Recall the canonical isomorphism
$$ \begin{align*} \mathrm{{Br}}(X)\cong\mathrm{{Hom}}(T(X),{\mathbb Q}/{\mathbb Z}) \end{align*} $$
(see [Reference Colliot-Thélène and SkorobogatovCS, (5.5) on p. 130, p. 142]). It is well known that the involution
$\sigma $
is not symplectic [Reference HuybrechtsH2, Cor. 15.1.5 and (ii) on p. 356], so it acts on
$\mathrm {H}^0(X,\Omega ^2_X)$
as
$-1$
. Therefore,
$\sigma ^*=\iota $
acts on
$T(X)$
as
$-1$
, so
$T(X)\subset N$
.
Theorem 2.4 (Beauville).
Let
$\pi _S\colon X\to S$
be an Enriques quotient of a K3 surface X. Then
$\pi _S^*(b_S)\in \mathrm {{Br}}(X)[2]$
is the restriction of
$\varepsilon \colon N\to {\mathbb Z}/2$
to
$T(X)$
.
Proof. See [Reference BeauvilleB, Prop s. 3.4 and 5.3].
An embedding
$T(X)\subset N$
coming from an Enriques quotient of X is clearly primitive. The orthogonal complement
$T(X)^{\perp }_N\subset N$
contains no
$(-2)$
-elements x, because by Riemann–Roch either x or
$-x$
is effective, but
$\sigma ^*$
preserves effectivity. In fact, these are the only conditions. Horikawa’s theorem on the surjectivity of the period map for Enriques surfaces [Reference HorikawaH1] leads to the following result. See [Reference KeumK, Th. 1], which was extended in [Reference OhashiO2, Prop. 2.1].
Theorem 2.5 (Keum, Ohashi).
Let X be a K3 surface. Associating to an Enriques quotient of X a primitive embedding
$T(X)\subset N$
defines a bijection between
${\mathcal Enr}(X)$
and the set of equivalence classes of primitive embeddings of
$T(X)$
into N without
$(-2)$
-elements in the orthogonal complement. Here the embeddings
$i_1$
and
$i_2$
are equivalent if there is an automorphism
$\tilde \phi $
of the lattice N and a
$\phi \in \mathrm {Aut}_{\mathrm {Hdg}}(T(X))$
such that
$i_2\circ \phi =\tilde \phi \circ i_1$
.
If
$\mathrm {Aut}_{\mathrm {Hdg}}(T(X))=\{\pm 1\}$
(which holds, e.g., when the Picard number of X is odd), the set
$\mathcal Enr(X)$
depends only on the lattice
$T(X)$
.
Corollary 2.6. For any K3 surface
$X,$
the following statements hold.
-
(i)
$\mathrm {{Im}}(\Phi _X)\setminus \{0\}$
is the set of nonzero
$\alpha \in \mathrm {{Br}}(X)[2]\cong \mathrm {{Hom}}(T(X),{\mathbb Z}/2)$
, for which there exists a primitive embedding
$i\colon T(X)\hookrightarrow N$
such that
$\alpha =i^*(\varepsilon )$
. -
(ii)
$0\in \mathrm {{Im}}(\Phi _X)$
if and only if there exists a primitive embedding
$i\colon T(X)\hookrightarrow N$
without
$(-2)$
-elements in the orthogonal complement such that
$i^*(\varepsilon )=0$
. -
(iii) If
$x\in T(X)$
is such that
$(x^2)\equiv 2\bmod 4$
, then
$\alpha (x)=0$
for any
$\alpha \in \mathrm {{Im}}(\Phi _X)$
.
Proof. Parts (i) and (ii) formally follow from Theorems 2.4 and 2.5 and Lemma 2.3. In particular, Lemma 2.3 implies that
$i(T(X))^{\perp }_N$
does not contain
$(-2)$
-classes. Part (iii) follows from Lemma 2.2.
Corollary 2.7. If X is a K3 surface such that the abelian group
$\mathrm {{Br}}(X)[2]$
is generated by the image of
$\Phi _X$
, then there is an even lattice
$T'$
such that
$T(X)\cong T'(2)$
.
Proof. It is enough to show that for every
$x\in T(X)$
we have
$(x^2)\equiv 0\bmod 4$
. Suppose that there is an element
$y\in T(X)$
such that
$(y^2)\equiv 2\bmod 4$
. Then y is not divisible by 2 in
$T(X)$
. By Corollary 2.6(iii), the nonzero class of y in
$T(X)/2T(X)$
is in the kernel of every
$\alpha \in \mathrm {{Im}}(\Phi _X)$
. Thus
$\mathrm {{Im}}(\Phi _X)$
is contained in a proper subgroup of
$\mathrm {{Br}}(X)[2]$
.
Corollary 2.8. Let X be a K3 surface such that
$T(X)$
has a basis
$e_1,\ldots ,e_n$
with
$(e_i^2)\equiv 2 \bmod 4$
for
$i=1,\ldots ,n$
. Then either
$\mathcal Enr(X)=\emptyset $
or
$\mathrm {{Im}}(\Phi _X)=\{0\}$
.
Proof. Suppose that a nonzero
$\alpha \in \mathrm {{Hom}}(T(X),{\mathbb Z}/2)$
is in the image of
$\Phi _X$
. By Theorem 2.4, there is a primitive embedding
$i\colon T(X)\to N$
such that
$i^*(\varepsilon )=\alpha $
. By Lemma 2.2, we have
$\alpha (e_i)=0$
for
$i=1,\ldots ,n$
, hence
$\alpha (T(X))=0$
which is a contradiction.
This can be used to give examples of K3 surfaces X such that
$\mathrm {{Im}}(\Phi _X)=\{0\}$
. For example, one can take the K3 surface X of Picard rank 20 with transcendental lattice
$$ \begin{align*} \left(\begin{array}{cc}2&0\\ 0&2c\end{array}\right) \end{align*} $$
with
$c=3, 5, 7$
. Indeed, by [Reference SertözSV, Table 3.1] in these cases, we have
$|\mathcal Enr(X)|=1$
.
3 Kummer surfaces
Proof of Theorem A
By Corollary 2.6(i), it is enough to construct, for any nonzero
$\alpha \in \mathrm {{Hom}}(T(X),{\mathbb Z}/2)$
, a primitive embedding
$i\colon T(X)\hookrightarrow N=\mathrm {U}\oplus \mathrm {U}(2)\oplus \mathrm {E}_8(2)$
such that
$\varepsilon (x)=\alpha (x)$
for any
$x\in T(X)$
. We use Morrison’s classification of transcendental lattices of Kummer surfaces (see [Reference HuybrechtsH2, Cor. 14.3.20]). For each of them, Keum [Reference KeumK, pp. 106–108] constructed a primitive embedding into N; we follow the same strategy to construct all
$2^n-1$
embeddings, where
$n=\mathrm {{rk}}(T(X))$
. We keep the notation of [Reference KeumK], in particular,
$e,f$
is a standard basis of
$\mathrm {U}$
and
$h,k$
is a standard basis of
$\mathrm {U}(2)$
. We denote by
$\rho $
the Picard rank of X.
In the proof below, we shall use the following particular case of a result of Nikulin.
Lemma 3.1. Any even negative-definite lattice of rank at most
$4$
has a primitive embedding in
$\mathrm {E}_8$
.
Proof. This follows from [Reference NikulinN, Th. 1.12.4] using the fact that
$\mathrm {E}_8$
is a unique even unimodular negative-definite lattice of rank 8.
$\rho =20$
In this case, the lattice
$T={\mathbb Z} x\oplus {\mathbb Z} y$
is positive-definite with Gram matrix
$$ \begin{align*} \left(\begin{array}{cc}4a&2b\\2b&4c \end{array}\right),\end{align*} $$
where
$a,b,c\in {\mathbb Z}$
. The three primitive embeddings can be given by sending
$x,y$
to the following two elements of N:
$$ \begin{align*} (e+2af,2bf+h+ck), \!\quad (2bf+h+ak,e+2cf), \!\quad (e+2af, e+(2b-2a)f+h+(c-b+a)k). \end{align*} $$
$\rho =19$
Now T has signature
$(2,1)$
. We can choose an integral basis
$x,y,t$
of T so that the Gram matrix is
$$ \begin{align*}\left(\begin{array}{ccc}4a&2d&2l\\2d&4b&2m\\2l&2m&4c \end{array}\right),\end{align*} $$
where
$a,b,c,d,l,m\in {\mathbb Z}$
and
$a,b,c<0$
. The embeddings we need to construct are numbered by the nonzero vectors
$(v_1,v_2,v_3)\in ({\mathbb F}_2)^3$
given by evaluating
$\varepsilon $
on the images of
$x,y,t$
in this order. By symmetry it is enough to construct embeddings labeled
$(1,0,0)$
,
$(1,1,0)$
, and
$(1,1,1)$
. The first two can be given by sending
$x,y,t$
to the following three elements of N, where w is a primitive element of
$\mathrm {E}_8(2)$
such that
$(w^2)=4c$
:
$$ \begin{align*} (e+2af,2df+h+bk,2lf+mk+w); \end{align*} $$
$$ \begin{align*} (e+2af,e+(2d-2a)f+h+(b-d+a)k,2lf+(m-l)k+w). \end{align*} $$
Next, we deal with
$(1,1,1)$
. Without loss of generality, we can assume
$m>0$
. Take
$$ \begin{align*} (e+k+ah,e+2mf+(d-m)h+w', e+lh+w), \end{align*} $$
where
${\mathbb Z} w'\oplus {\mathbb Z} w$
is a primitive sublattice of
$\mathrm {E}_8(2)$
such that
$(w^{\prime 2})=4b-4m<0$
,
$(w^2)=4c<0$
,
$(w'.w)=0$
.
$\rho =18$
Here, the lattice T is the orthogonal direct sum of
${\mathbb Z} x\oplus {\mathbb Z} y$
with signature
$(1,1)$
and Gram matrix
$$ \begin{align*} \left(\begin{array}{cc}4a&2b\\2b&4c\end{array}\right) \end{align*} $$
and
$\mathrm {U}(2)={\mathbb Z} r\oplus {\mathbb Z} s$
. Without loss of generality, we assume that
$a,c<0$
and
$b>0$
. Let w and u be primitive vectors of
$\mathrm {E}_8(2)$
such that
$(w^2)=4c<0$
and
$(u^2)=4(a-b+c)<0$
. We label the embeddings in the same way as above. Up to exchanging the roles of x and y, and of r and s, it is enough to construct embeddings with the following labels:
$$ \begin{align*} (1,0,0,0), (1,1,0,0), (1,0,1,0), (0,0,1,0), (0,0,1,1), (1,1,1,0), (1,0,1,1), (1,1,1,1). \end{align*} $$
Let us first construct primitive embeddings with labels
$(1,0,0,0)$
and
$(1,1,0,0)$
by taking the direct sum of a primitive embedding
${\mathbb Z} x\oplus {\mathbb Z} y$
into
$\mathrm { U}\oplus \mathrm {E}_8(2)$
and the identity embedding
$\mathrm {U}(2)\tilde \longrightarrow \mathrm {U}(2)$
. We send
$x,y$
to
$$ \begin{align*} (e+2af,2bf+w), \quad (e+2af,e+(2b-2a)f+u). \end{align*} $$
The embedding with label
$(1,0,1,0)$
can be obtained by sending
$x,y,r,s$
to
$$ \begin{align*} (e+2af-ak,2bf-bk+w,e+h,k). \end{align*} $$
For
$(0,0,1,0)$
, we take
$(h+w_1,bk+w_2,e,2e+2f+w_3)$
, where
${\mathbb Z} w_1\oplus {\mathbb Z} w_2\oplus {\mathbb Z} w_3$
is a primitive sublattice of
$\mathrm {E}_8(2)$
with diagonal Gram matrix such that
$(w_1^2)=4a<0$
,
$(w_2^2)=4c<0$
,
$(w_3^2)=-8$
.
For
$(0,0,1,1)$
, we take
$(h+w_1,bk+w_2,e,e+2f+w_3)$
, where
${\mathbb Z} w_1\oplus {\mathbb Z} w_2\oplus {\mathbb Z} w_3$
is a primitive sublattice of
$\mathrm {E}_8(2)$
with diagonal Gram matrix such that
$(w_1^2)=4a<0$
,
$(w_2^2)=4c<0$
,
$(w_3^2)=-4$
.
For
$(1,1,1,0)$
, we take
$(e+2af-ak,e+(2b-2a)f+(a-b)k+u,e+h,k)$
.
For
$(1,0,1,1)$
, we take
$(e+2af-ak,2bf-bk+w,e+h,e+k+h+w')$
, where
${\mathbb Z} w\oplus {\mathbb Z} w'$
is a primitive sublattice of
$\mathrm {E}_8(2)$
such that
$(w^2)=4c<0$
,
$(w^{\prime 2})=-4$
,
$(w.w')=0$
.
For
$(1,1,1,1)$
, we take
$(e+2af-ak,e+(2b-2a)f+(a-b)k+u,e+h,e+k+h+w')$
, where
${\mathbb Z} u\oplus {\mathbb Z} w'$
is a primitive sublattice of
$\mathrm {E}_8(2)$
such that
$(u^2)=4(a-b+c)<0$
,
$(w^{\prime 2})=-4$
,
$(u.w')=0$
.
$\rho =17$
Here, we have
$T=\mathrm {U}(2)\oplus \mathrm {U}(2)\oplus (-4m)$
, where
$m\geq 1$
. A standard basis is
$\{x,y,x',y',t\}$
. Up to swapping the two copies of
$\mathrm {U}(2)$
and swapping the elements of a standard basis of each
$\mathrm {U}(2)$
it is enough to construct embeddings with the following labels:
$$ \begin{align*} (1,0,0,0,0), (1,1,0,0,0), (1,0,0,0,1), (1,1,0,0,1), (0,0,0,0,1), \end{align*} $$
$$ \begin{align*} (1,1,1,1,0), (1,1,1,1,1), (1,0,1,0,0), (1,1,1,0,0), (1,1,1,0,1), (1,0,1,0,1). \end{align*} $$
The first five embeddings are obtained as direct sums of a primitive embedding of
$\mathrm {U}(2)\oplus (-4m)$
into
$\mathrm {U}\oplus \mathrm {E}_8(2)$
and the identity embedding
$\mathrm { U}(2)\tilde \longrightarrow \mathrm {U}(2)$
. The respective primitive embeddings of
$\mathrm {U}(2)\oplus (-4m)$
into
$\mathrm {U}\oplus \mathrm {E}_8(2)$
are given by sending
$x,y,t$
to the following triples:
$$ \begin{align*} (e,2e+2f+u_1, v_1), (e,e+2f+u_2,v_2), (e,2e+2f+u_3,e+v_3), (e,e+2f+u_4,e+v_4). \end{align*} $$
Here,
${\mathbb Z} u_i\oplus {\mathbb Z} v_i$
is a primitive sublattice of
$\mathrm {E}_8(2)$
such that:
$(u_1^2)=-8$
,
$(v_1^2)=-4m$
,
$(u_1.v_1)=0$
;
$(u_2^2)=-4$
,
$(v_2^2)=-4m$
,
$(u_2.v_2)=0$
;
$(u_3^2)=-8$
,
$(v_3^2)=-4m$
,
$(u_3.v_3)=-2$
;
$(u_4^2)=-4$
,
$(v_4^2)=-4m$
,
$(u_4.v_4)=-2$
.
The embedding labeled
$(0,0,0,0,1)$
can be obtained by sending
$x,y,t$
to
$$ \begin{align*} (2e+2f+w_0,2e+2f+w_1,e+w_2), \end{align*} $$
where
$w_0,w_1,w_2$
generate a primitive sublattice of
$\mathrm {E}_8(2)$
with Gram matrix
$$ \begin{align*} \left(\begin{array}{ccc}-8&-6&-2\\-6&-8&-2\\-2&-2&-4m \end{array}\right). \end{align*} $$
Indeed, this matrix is negative-definite.
To construct the last six embeddings, we exhibit the images of
$x,y,x',y',t$
. In the case of
$(1,1,1,1,0)$
, we consider
$$ \begin{align*} (e,e+2f+k+w_0,e-h,e-h-k+w_1,w_2), \end{align*} $$
where
$w_0,w_1,w_2$
generate a primitive sublattice of
$\mathrm {E}_8(2)$
with diagonal Gram matrix such that
$(w_0^2)=(w_1^2)=-4$
and
$(w_2^2)=-4m$
.
In the case of
$(1,1,1,1,1)$
, we take
$$ \begin{align*} (e,e+2f+k+w_0,e-h,e-h-k+w_1,e+w_2), \end{align*} $$
where
$w_0,w_1,w_2$
generate a primitive sublattice of
$\mathrm {E}_8(2)$
with the negative-definite Gram matrix
$$ \begin{align*} \left(\begin{array}{ccc}-4&0&-2\\0&-4&0\\-2&0&-4m \end{array}\right). \end{align*} $$
In the case of
$(1,0,1,0,0)$
, we take
$(e,2f+k,e-h,-k,w)$
, where w is a primitive element of
$\mathrm {E}_8(2)$
with
$(w^2)=-4m$
.
For
$(1,1,1,0,0)$
, we take
$(e,e+2f+k+u_2,e-h,-k,v_2)$
.
For
$(1,1,1,0,1)$
, we take
$(e,e+2f+k+u_4,e-h,-k,e+v_4)$
.
For
$(1,0,1,0,1)$
, we take
$(e,2e+2f+k+u_3,e-h,-k,e+v_3)$
.
Proof of Corollary B
(i) Let
$E_1$
and
$E_2$
be non-isogenous elliptic curves, and let
$X=\mathrm {Kum}(E_1\times E_2)$
. By [Reference OhashiO1, §4], we have
$\mathrm {Aut}_{\mathrm {Hdg}}(T(X))=\{\pm 1\}$
and
$|\mathcal Enr(X)|=15$
. (The 15 Enriques involutions can be described geometrically as the Lieberman involutions and the Kondo–Mukai involutions.) We have
$\mathrm {{rk}}(T(X))=4$
, hence
$|\mathrm {{Br}}(X)[2]\setminus \{0\}|=15$
.
(ii) Let C be a smooth projective curve of genus 2 such that
$\mathrm {NS\,}(\mathrm {Jac}(C))\cong {\mathbb Z}$
. Let
$X=\mathrm {Kum}(\mathrm {Jac}(C))$
. Condition
$\mathrm {Aut}_{\mathrm {Hdg}}(T(X))=\{\pm 1\}$
is satisfied since the Picard rank of X is odd. Ohashi [Reference OhashiO2] shows that
$|\mathcal Enr(X)|=31$
and describes these 31 involutions geometrically. In this case
$\mathrm {{rk}}(T(X))=5$
, so
$|\mathrm {{Br}}(X)[2]\setminus \{0\}|=31$
.
Taking into account (i) and (ii), Corollary B follows from Theorem A since a surjective map of finite sets of the same cardinality is a bijection.
4 Singular K3 surfaces
K3 surfaces over
$\overline {\mathbb Q}$
For a variety X over
$\overline {\mathbb Q}$
and an element
$g \in \mathrm {{Gal}}(\overline {\mathbb Q}/{\mathbb Q})$
, we define
$X^g=X\times _{\overline {\mathbb Q}, g}\overline {\mathbb Q}$
. Then, we have a morphism
$g\colon X\to X^g$
making the following diagram commutative:
Here, the vertical arrows are structure morphisms. A morphism of
$\overline {\mathbb Q}$
-varieties
$\phi \colon X\to Y$
gives rise to a morphism of
$\overline {\mathbb Q}$
-varieties
$\phi ^g=g\phi g^{-1}\colon X^g\to Y^g$
.
Let
$K\subset \overline {\mathbb Q}$
be a subfield, and let
$G_K=\mathrm {{Gal}}(\overline {\mathbb Q}/K)$
.
Definition 4.1. Let X be a variety over
$\overline {\mathbb Q}$
.
-
(i) The field of moduli of X over K is the subfield
$K(X)\subset \overline {\mathbb Q}$
fixed by the group
$\{g\in G_K | X\cong X^g \}$
. -
(ii) Let
$B \subset \mathrm {{Br}}(X)$
be a finite subgroup. The field of moduli of the pair
$(X, B)$
over K is the subfield
$K(X,B)\subset \overline {\mathbb Q}$
fixed by the group
$$ \begin{align*}\{g\in G_K| \exists \, \text{an isomorphism} \, f \colon X^g\rightarrow X \, \text{such that} \, (g^* \circ f^*)|_B= \mathrm{id}_B \}.\end{align*} $$
Let us fix an embedding
$\overline {\mathbb Q}\subset {\mathbb C}$
. For a K3 surface X over
$\overline {\mathbb Q}$
we write
$T(X)$
for the transcendental lattice of
$X_{\mathbb C}$
. One has natural isomorphisms ([Reference Colliot-Thélène and SkorobogatovCS, Prop. 5.2.3 and p. 142])
$$ \begin{align*}\mathrm{{Br}}(X) \cong \mathrm{{Br}}(X_{\mathbb C}) \cong \mathrm{{Hom}}(T(X), {\mathbb Q} / {\mathbb Z}). \end{align*} $$
Remark 4.2. Let X be a K3 surface over
$\overline {\mathbb Q}$
of Picard rank at least
$12$
. According to [Reference ValloniV, Rem. 6.1(2), p. 32] a Hodge isometry
$h \colon T(X^g) \tilde \rightarrow T(X)$
exists if and only if
$X \cong X^g$
. It follows that in this case
$K(X,B)$
is the fixed field of the group
$$ \begin{align*}\{g\in G_K | \exists \, \text{a Hodge isometry} \, h \colon T(X^g) \rightarrow T(X) \, \text{such that} \, (g^* \circ h^*)|_B = \mathrm{id}_B \}.\end{align*} $$
For a K3 surface over
$\overline {\mathbb Q}$
, we have
$\mathrm {Aut}(X)=\mathrm {Aut}(X_{\mathbb C})$
, since
$Aut_{X/\overline {\mathbb Q}}$
is a discrete group scheme. Hence, the set of conjugacy classes of fixed point free involutions
$\mathcal Enr(X)\subset \mathrm {Aut}(X)$
coincides with
$\mathcal Enr(X_{\mathbb C})$
.
Proposition 4.3. Let X be a K3 surface over
$\overline {\mathbb Q}$
such that
$\mathrm {Aut}_{\mathrm {Hdg}}(T(X))=\{\pm 1\}$
. The Galois group
$G_{K(X)}$
acts naturally on
${\mathcal Enr}(X)$
and on
$\mathrm {{Br}}(X)[2]$
so that the map
$\varphi _X\colon {\mathcal Enr}(X) \to \mathrm {{Br}}(X)[2]$
is
$G_{K(X)}$
-equivariant.
Proof. Write
$K := K(X)$
. We use
$\sigma $
and
$\tau $
to denote arbitrary elements of
$G_K$
. By Definition 4.1(i), we can find an isomorphism
$f_{\sigma ,\tau } \colon X^{\sigma } \tilde \longrightarrow X^{\tau }$
.
Let us denote the conjugacy class of
$\psi \in \mathrm {Aut}(X)$
by
$[\psi ]$
.
A fixed point free involution
$\iota \colon X\to X$
gives rise to a fixed point free involution
$\iota ^{\sigma }=\sigma \iota \sigma ^{-1}\colon X^{\sigma }\rightarrow X^{\sigma }$
, and one has
$(\iota ^{\sigma })^{\tau }=\iota ^{\tau \sigma }$
. We define an action of
$G_K$
on
${\mathcal Enr}(X)$
by making
$\sigma $
send
$[\iota ]$
to
$[f_{1,\sigma }^{-1}\iota ^{\sigma } f_{1,\sigma }]$
. This class depends neither on the choice of
$\iota $
in its conjugacy class, nor on the choice of
$f_{1,\sigma }$
. We have
$$ \begin{align*} [f_{1,\tau}^{-1}(f_{1,\sigma}^{-1}\iota^{\sigma} f_{1,\sigma})^{\tau} f_{1,\tau}]= [(f_{1,\sigma}^{\tau} f_{1,\tau})^{-1}\iota^{\tau\sigma}(f_{1,\sigma}^{\tau} f_{1,\tau})] =[f_{1,\tau\sigma}^{-1}\iota^{\tau\sigma} f_{1,\tau\sigma}], \end{align*} $$
because
$f_{1,\tau \sigma }$
and
$f_{1,\sigma }^{\tau } f_{1,\tau }$
are both isomorphisms
$X\tilde \longrightarrow X^{\tau \sigma }$
, so replacing one of them by the other does not change the conjugacy class.
Let us now define an action of
$G_K$
on
$\mathrm {{Br}}(X)[2]$
by making
$\sigma \in G_{K}$
act as
$f_{1,\sigma }^*(\sigma ^{-1})^*$
which is induced by
$\sigma ^{-1}f_{1,\sigma }\colon X\to X^{\sigma }\to X$
. This action on
$\mathrm {{Br}}(X)[2]$
does not depend on the choice of
$f_{1,\sigma }$
. Indeed,
$f_{1,\sigma }$
is well defined up to an automorphism of X, but the action of
$\mathrm {Aut}(X)$
on
$\mathrm {{Br}}(X)[2]$
factors through the action of
$\mathrm {Aut}_{\mathrm { Hdg}}(T(X))$
. The latter group is
$\{\pm 1\}$
by assumption, so
$\mathrm {Aut}(X)$
acts on
$\mathrm {{Br}}(X)[2]$
trivially. The map
$(f_{1,\sigma })^{\tau }=\tau f_{1,\sigma }\tau ^{-1}$
is an isomorphism
$X^{\tau }\tilde \longrightarrow X^{\tau \sigma }$
, hence
$(f_{1,\sigma })^{\tau } f_{1,\tau }$
is an isomorphism
$X\to X^{\tau \sigma }$
, so for the purpose of calculating the induced action of
$\mathrm {{Br}}(X)[2]$
, we can replace it with
$f_{1,\tau \sigma }$
. This shows that sending
$\sigma \in G_{K}$
to the map induced on
$\mathrm {{Br}}(X)[2]$
by
$\sigma ^{-1}f_{1,\sigma }$
is indeed an action.
We have a commutative diagram
where the vertical maps are quotients by the respective fixed point free involutions. Thus the image of the nonzero element of
$\mathrm {{Br}}(X/\iota )$
in
$\mathrm {{Br}}(X)[2]$
followed by the action of
$\sigma $
on
$\mathrm {{Br}}(X)[2]$
is the same as the image of the nonzero element of
$\mathrm {{Br}}(X/(f_{1,\sigma }^{-1}\iota ^{\sigma } f_{1,\sigma }))$
in
$\mathrm {{Br}}(X)[2]$
. This proves that
$\varphi _X$
is
$G_{K}$
-equivariant.
Moduli fields of singular K3 surfaces
Let X be a singular K3 surface, that is, a K3 surface of maximal Picard rank
$20$
. It is well known that every singular K3 surface is defined over
$\overline {\mathbb Q}$
and has complex multiplication by the imaginary quadratic field
$E = \mathrm {{End}}_{\mathrm {Hdg}} (T(X)_{\mathbb Q})$
. Assume that
$\mathrm {{End}}_{\mathrm {Hdg}}(T(X))$
is the ring of integers
$\mathcal {O}_E\subset E$
. In this situation, the results of [Reference ValloniV] give explicit descriptions of the moduli fields
$E(X)$
and
$E(X, \mathrm {{Br}}(X)[n])$
which we now recall.
The group
$\mathrm {{Br}}(X)\cong \mathrm {{Hom}}(T(X),{\mathbb Q}/{\mathbb Z})$
is naturally an
$\mathcal {O}_E$
-module. Let
$K_n/E$
be the ray class field of E with modulus
$n\mathcal {O}_E,$
and let
$\mathrm {Cl}_n(E) \cong \mathrm {Gal}(K_n/E)$
. The complex conjugation c acts on
$\mathrm {Cl}_n(E)$
. Let
$\mathrm {Cl}_n(E)^c$
be the c-invariant subgroup of
$\mathrm {Cl}_n(E)$
. Define
$\widetilde {K}_n \subset K_n$
as the fixed field of
$\mathrm {Cl}_n(E)^c$
, so that
$\mathrm {Gal}(\widetilde {K}_n/E) \cong \mathrm {Cl}_n(E)/ \mathrm {Cl}_n(E)^c$
. Note that
$K_1$
is the Hilbert class field of E and
$\mathrm {Cl}_1(E) = \mathrm {Cl}(E)$
is the usual class group. The complex conjugation c acts on
$\mathrm {Cl}(E)$
as
$-1$
.
Theorem 4.4. Let X be a singular K3 surface. Then
$\widetilde {K}_n=E(X, \mathrm {{Br}}(X)[n])$
.
Proof. See [Reference ValloniV, Th. 11.2 and Rem. 9.2 on p. 41].
In particular, we have
$\widetilde {K}_1=E(X)$
. If n divides m, then
$\widetilde {K}_n \subset \widetilde {K}_m$
.
Proof of Theorem C
The assumptions of Theorem C imply that
$\mathrm {Aut}_{\mathrm {Hdg}}(T(X))={\mathcal O}_E^{\times }=\{\pm 1\}$
, so we can apply Proposition 4.3. Let
$\rho $
be the representation of
$G_{\widetilde {K}_1}$
in
$\mathrm {{Br}}(X)[2] \cong ({\mathbb Z}/2)^2$
constructed in the proof of Proposition 4.3. It is enough to show that under our assumptions one has
$|\rho (G_{\widetilde {K}_1})| = 3$
. Then
$G_{\widetilde {K}_1}$
acts transitively on
$\mathrm {{Br}}(X)[2]\setminus \{0\}$
, so in view of the
$G_{\widetilde {K}_1}$
-equivariance established in Proposition 4.3 this will imply Theorem C. By Theorem 4.4, we need to prove that
$[\widetilde K_2:\widetilde K_1]=3$
.
The following exact sequence describes the ray class group
$\mathrm {Cl}_2(E)$
:
$$ \begin{align*} 0 \rightarrow \frac{\mathcal{O}_E^{\times}}{\{ x \in \mathcal{O}_E^{\times} | x \equiv 1 \, \mathrm{mod} \, 2 \}} \rightarrow (\mathcal{O}_E/2)^{\times} \rightarrow \mathrm{Cl}_2(E) \rightarrow \mathrm{Cl}(E) \rightarrow 0. \end{align*} $$
Under our assumptions, we have
$\mathcal {O}_E^{\times } =\{ x \in \mathcal {O}_E^{\times } | x \equiv 1 \, \mathrm {mod} \, 2 \} = \{ \pm 1 \}$
. Since
$2$
is inert in E, we have
$\mathcal {O}_E / 2 \cong \mathbb {F}_4$
, and thus the sequence above becomes
$$ \begin{align*} 0 \rightarrow {\mathbb F}_4^{\times} \rightarrow \mathrm{Cl}_2(E) \rightarrow \mathrm{Cl}(E) \rightarrow 0. \end{align*} $$
This is a sequence of G-modules, where
$G = \{1, c \}$
. We have
$({\mathbb F}_4^{\times })^c =\{1\}$
and
$\mathrm {H}^1(G, \mathbb {F}_4^{\times }) = 0$
, and hence
$\mathrm {Cl}_2(E)^c = \mathrm {Cl}(E)^c$
. From this, we obtain the exact sequence
$$ \begin{align*} 0 \rightarrow {\mathbb F}_4^{\times} \rightarrow \mathrm{Gal}(\widetilde K_2/E) \rightarrow \mathrm{Gal}(\widetilde K_1/E) \rightarrow 0. \end{align*} $$
Thus,
$[\widetilde K_2:\widetilde K_1]=3$
, as required.
Remark 4.5. When
$2$
is split, a similar argument shows that the
$G_{\widetilde {K}_1}$
-action on
$\mathrm {{Br}}(X)[2]$
is trivial.
5 Constructing Enriques involutions
For a finite abelian group
$G,$
we write
$\ell (G)$
for the minimal number of generators of G. For a prime p we denote by
$G_p$
the p-primary subgroup of G. Recall that for a lattice L we write
$A_L=L^*/L$
for the discriminant group of L. When L is even, we denote by
$q_L\colon A_L\to {\mathbb Q}/2{\mathbb Z}$
the finite quadratic form of L.
We need to recall fundamental results of Nikulin about the existence of lattices and their primitive embeddings.
Let
$q \colon A \rightarrow {\mathbb Q} / 2 {\mathbb Z}$
be a finite quadratic form. The signature
$\mathrm {sign}(q) \in {\mathbb Z} / 8 {\mathbb Z}$
of q is defined as
$(t_+ - t_-) \bmod 8$
, where
$(t_+, t_-)$
is the signature of any even lattice whose discriminant form is isomorphic to
$(A,q)$
(such a lattice always exists and, moreover, this notion is well-defined). One also has
$$ \begin{align} \mathrm{sign}(q \oplus q') = \mathrm{sign}(q) + \mathrm{sign}(q'). \end{align} $$
Write
$A = \bigoplus _p A_p$
, where p ranges over the prime numbers. Then one has quadratic forms
$q_p \colon A_p \rightarrow {\mathbb Q}_p / {\mathbb Z}_p$
when p is odd and
$q_2 \colon A_2 \rightarrow {\mathbb Q}_2/ 2 {\mathbb Z}_2$
when
$p=2$
. It is clear that q is the orthogonal direct sum of the forms
$q_p$
.
For an odd prime p, a finite abelian p-group
$A_p$
, and a quadratic form
$q_p\colon A_p\to {\mathbb Q}_p/{\mathbb Z}_p$
, Nikulin [Reference NikulinN, Th. 1.9.1] showed that there is a unique
${\mathbb Z}_p$
-lattice
$K(q_p)$
of rank
$\ell (A_p)$
whose quadratic form is isomorphic to
$q_p$
.
When
$p=2$
, the same result of Nikulin says the following. Let
$q^{(2)}_{\theta } (2)$
be the discriminant quadratic form of the rank one
${\mathbb Z}_2$
-lattice
$(2 \theta )$
, where
$\theta \in {\mathbb Z}_2^{\times }$
. For a finite abelian 2-group
$A_2$
and a quadratic form
$q_2\colon A_2\to {\mathbb Q}_2/2{\mathbb Z}_2$
we have the following alternative. If
$q_2$
splits as an orthogonal direct sum
$q_2=q^{(2)}_{\theta } (2) \oplus q^{\prime }_2$
, then there are precisely two even
${\mathbb Z}_2$
-lattices of rank
$\ell (A_2)$
whose quadratic form is isomorphic to
$q_2$
. If such a splitting of
$q_2$
does not exist, there is a unique
${\mathbb Z}_2$
-lattice
$K(q_2)$
of rank
$\ell (A_2)$
whose quadratic form is isomorphic to
$q_2$
. The following result is [Reference NikulinN, Th. 1.10.1].
Theorem 5.1 (Nikulin).
An even lattice with signature
$(t_+, t_-)$
and quadratic form
$q\colon A\to {\mathbb Q}/2{\mathbb Z}$
exists if and only if the following conditions are satisfied:
-
(1)
$t_+ - t_- \equiv \mathrm {sign}(q) \mod 8$
; -
(2)
$t_+, t_- \geq 0$
and
$t_+ + t_- \geq \ell (A)$
; -
(3)
$(-1)^{t_-} |A_p| \equiv \mathrm {discr} K(q_p) \bmod {\mathbb Z}_p^{\times 2}$
for the odd primes p such that
$t_+ + t_- = \ell (A_p)$
; -
(4)
$|A_2| \equiv \pm \mathrm {discr} K(q_2) \bmod {\mathbb Z}_2^{\times 2}$
if
$t_+ + t_- = \ell (A_2)$
and
$q_2 \neq q_{\theta }^{(2)}(2) \oplus q_2'$
for any
$\theta $
and
$q_2'$
.
The following result is a consequence of [Reference NikulinN, Prop. 1.15.1] where we took into account that N is the unique lattice of signature
$(2,10)$
whose quadratic form is isomorphic to
$q_N$
(see [Reference NikulinN, Cor. 1.13.4].
Theorem 5.2 (Nikulin).
Let L be an even lattice with signature
$(2_+, k_-)$
and quadratic form
$q_L\colon A_L\to {\mathbb Q}/2{\mathbb Z}$
. The existence of a primitive embedding
$L \hookrightarrow N$
is equivalent to the existence of the following data:
-
• subgroups
$H_L \subset A_L$
and
$H_N \subset A_N$
; -
• an isomorphism of finite quadratic forms
$\gamma \colon (H_L,q_L|_{H_L}) \xrightarrow {\sim } (H_N,q_N|_{H_N})$
; -
• an even negative-definite lattice K of rank
$10-k$
; -
• an isomorphism of finite quadratic forms
$\delta $
from
$(A_K,-q_K)$
to the restriction of
$q_L\oplus -q_N$
to
$\Gamma _{\gamma }^{\perp } / \Gamma _{\gamma }$
, where the isotropic subgroup
$\Gamma _{\gamma }\subset A_L\oplus A_N$
is the graph of
$\gamma $
in
$H_L\oplus H_N\subset A_L\oplus A_N$
.
Moreover, if
$i \colon L \hookrightarrow N$
is a primitive embedding associated to
$(H_L, H_N, \gamma, K, \delta )$
, then
$K \cong i(L)^{\perp }$
.
Remark 5.3.
-
(1) If
$f \colon \widetilde {K} \to K$
is an isomorphism of lattices and
$\bar {f} \colon A_{\widetilde {K}} \to A_K$
is the induced isomorphism, then the primitive embeddings
$L\hookrightarrow N$
associated to
$(H_L, H_N, \gamma , K, \delta )$
and to
$(H_L, H_N, \gamma , \widetilde {K},\delta \circ \bar {f})$
are isomorphic. -
(2) An analog of Theorem 5.2 gives the conditions for the existence of a primitive embedding of
$L\otimes {\mathbb Z}_p$
into
$N\otimes {\mathbb Z}_p$
, for any prime p. The analog of (1) also holds in this context.
Definition 5.4. Let L be a lattice such that
$0<\mathrm {{rk}}(L) \leq 10$
. We say that a sublattice
$L' \subset L$
of finite index satisfies condition
$(*)$
if
$$ \begin{align*} \mathrm{gcd}( 2\mathrm{discr}(L), [L \colon L']) = 1, \end{align*} $$
and for each prime p not dividing
$ 2\mathrm {discr}(L),$
we have
$\ell (A_{L',p}) < 12 - \mathrm {{rk}}(L')$
.
Proposition 5.5. Any lattice L such that
$0<\mathrm {{rk}}(L) \leq 10$
contains infinitely many distinct sublattices
$L'\subset L$
satisfying condition
$(*)$
.
Proof. Let p be any odd prime not dividing
$\mathrm {discr}(L)$
. As is well known (see, e.g., [Reference NikulinN, Cor. 1.9.3]), the unimodular p-adic lattice
$L\otimes {\mathbb Z}_p$
has an orthogonal
${\mathbb Z}_p$
-basis
$v_1,\ldots ,v_n$
such that
$(v_i^2)\in {\mathbb Z}_p^{\times }$
for
$i=1,\ldots , n$
. The images of
$v_1,\ldots ,v_n$
in
$(L\otimes {\mathbb Z}_p)/p\cong L/p$
form a basis of this
${\mathbb F}_p$
-vector space. Let
$L'\subset L$
be the inverse image of the hyperplane spanned by the images of
$v_2,\ldots ,v_n$
. Thus
$[L:L']=p$
, so that
$\mathrm {discr}(L')=p^2\mathrm {discr}(L)$
. Since p does not divide
$\mathrm { discr}(L)$
, we have a canonical isomorphism
$A_{L'}\cong A_L\oplus A_{L',p}$
. It is enough to check that
$\ell (A_{L',p})=1$
, which says that
$A_{L',p}$
is cyclic. It is clear that
$A_{L',p}\cong A_{L'\otimes {\mathbb Z}_p}$
, so it is enough to prove that
$\mathrm {{Hom}}_{{\mathbb Z}_p}(L'\otimes {\mathbb Z}_p,{\mathbb Z}_p)/(L'\otimes {\mathbb Z}_p)\cong {\mathbb Z}/p^2$
. The
${\mathbb Z}_p$
-module
$L'\otimes {\mathbb Z}_p$
is freely generated by
$pv_1,v_2,\ldots ,v_n$
, hence the
${\mathbb Z}_p$
-module
$\mathrm {{Hom}}_{{\mathbb Z}_p}(L'\otimes {\mathbb Z}_p,{\mathbb Z}_p)\subset L'\otimes {\mathbb Q}_p$
is freely generated by
$p^{-1}v_1,v_2,\ldots ,v_n$
, which implies the result.
Condition
$(*)$
implies that
$[L \colon L']$
is odd, and hence the inclusion
$L' \subset L$
induces a natural isomorphism
$$ \begin{align} \mathrm{{Hom}}(L', {\mathbb Z} / 2 {\mathbb Z}) \cong \mathrm{{Hom}}(L, {\mathbb Z} / 2 {\mathbb Z}). \end{align} $$
Recall that for a primitive embedding
$i\colon L\hookrightarrow N$
we denote by
$i^*(\varepsilon )$
the precomposition of the character
$\varepsilon \colon N\to {\mathbb Z}/2$
with i.
Theorem 5.6. Let
$L' \subset L$
be an inclusion of even lattices of signature
$(2_+, k_-)$
, where
$0\leq k\leq 8$
. Then we have the following statements.
-
(a) If
$L' \subset L$
satisfies condition
$(*)$
, then for any primitive embedding
$i\colon L\hookrightarrow N$
with
$i^*(\varepsilon )\neq 0$
there exists a primitive embedding
$i'\colon L'\hookrightarrow N$
such that
$i^{\prime *}(\varepsilon )=i^*(\varepsilon )$
under the identification (2). -
(b) If
$[L \colon L']$
is odd, then for any primitive embedding
$i'\colon L'\hookrightarrow N$
with
$i^{\prime *}(\varepsilon )\neq 0$
there exists a primitive embedding
$i\colon L\hookrightarrow N$
such that
$i^{\prime *}(\varepsilon )=i^*(\varepsilon )$
under the identification (2).
Proof. (a) Let
$i\colon L\hookrightarrow N$
be a primitive embedding such that
$i^*(\varepsilon )\neq 0$
. Then
$K:=i(L)^{\perp }_N$
is an even negative-definite lattice of rank
$10-k$
. By Theorem 5.2, the embedding i corresponds to some datum
$(H_L, H_N, \gamma , K, \delta )$
.
Since
$L' \subset L$
satisfies condition
$(*)$
, the index
$[L:L']$
is coprime to
$|A_L|$
, hence
$A_{L'}$
canonically isomorphic to
$A_L \oplus A_{\mathrm {new}}$
, where
$|A_{\mathrm {new}}|=[L:L']^2$
. Then
$q_{L'}$
is an orthogonal direct sum
$q_{L'}\cong q_L\oplus q_{\mathrm {new}}$
, where
$q_{\mathrm {new}}$
is a quadratic form on
$A_{\mathrm {new}}$
.
We claim that there is a negative-definite lattice
$K'$
of rank
$10 - k$
such that
$A_{K'}\cong A_K \oplus A_{\mathrm {new}}$
and
$q_{K'}\cong q_K\oplus -q_{\mathrm {new}}$
. Since
$L'$
is a sublattice of L of finite index and
$\mathrm {{rk}}(K)=10-k$
, we have
$$ \begin{align*} \mathrm{sign}(q_L)\equiv \mathrm{sign}(q_{L'}) \bmod 8, \quad \quad k-10\equiv\mathrm{sign}(q_K) \bmod 8. \end{align*} $$
Since
$q_{L'}\cong q_L \oplus q_{\mathrm {new}}$
, we have that
$\mathrm {sign}(q_{L'}) = \mathrm {sign}(q_{L}) + \mathrm {sign}(q_{\mathrm {new}})$
by (1). Thus
$\mathrm {sign}(q_{\mathrm {new}}) \equiv 0 \bmod 8$
, which implies property (1) of Theorem 5.1.
By condition
$(*)$
, we know that
$|A_{\mathrm {new}}|$
is odd and coprime to
$|A_L|$
. For any odd prime p, the
${\mathbb Z}_p$
-lattices
$L \otimes {\mathbb Z}_p$
and
$K \otimes {\mathbb Z}_p$
are orthogonal complements of each other in the unimodular
${\mathbb Z}_p$
-lattice
$N \otimes {\mathbb Z}_p$
, hence
$|A_{L,p}|=|A_{K,p}|$
. Thus,
$|A_K|$
and
$|A_{\mathrm {new}}|$
are coprime. This implies
$$ \begin{align*} \ell(A_K \oplus A_{\mathrm{new}}) = \max\{\ell(A_K), \ell(A_{\mathrm{new}})\}\leq 10-k, \end{align*} $$
since
$\ell (A_K)\leq \mathrm {{rk}}(K)=10-k$
and
$\ell (A_{\mathrm {new}})\leq \ell (A_{L'})< 12-\mathrm {{rk}}(L)$
by condition
$(*)$
. Thus, property (2) of Theorem 5.1 also holds.
We now check properties (3) and (4) taking into account the coprimality of
$|A_K|$
and
$|A_{\mathrm {new}}|$
. If p divides
$|A_K|$
, then (3) and (4) hold because they hold for
$A_K$
. If p divides
$|A_{\mathrm {new}}|$
, then
$\ell ( A_{\mathrm {new}}) < \mathrm {{rk}}(K')$
by condition
$(*)$
, so there is nothing to check.
Theorem 5.1 now implies the existence of
$K'$
with required properties.
Let us construct a datum defining the desired primitive embedding
$L' \hookrightarrow N$
. Since
$2A_N=0$
, we have
$2H_N=0$
and thus
$2H_L=0$
, so that
$H_L \subset A_{L,2}$
. In view of the canonical isomorphism
$A_{L,2} \cong A_{L',2}$
, we can keep the same
$H_{L'}=H_L$
,
$H_N$
and
$\gamma '=\gamma $
as the first three entries of our datum.
Recall that
$A_{L'}\cong A_L \oplus A_{\mathrm {new}}$
. We have
$$ \begin{align*}\Gamma_{\gamma'} = \Gamma_{\gamma} \oplus 0 \subset \Gamma_{\gamma'}^{\perp} = \Gamma_{\gamma}^{\perp} \oplus A_{\mathrm{new}} \subset (A_L \oplus A_N) \oplus A_{\mathrm{new}},\end{align*} $$
hence
$\Gamma _{\gamma '}^{\perp } / \Gamma _{\gamma '} = \Gamma _{\gamma }^{\perp } / \Gamma _{\gamma }\oplus A_{\mathrm {new}} \cong A_K \oplus A_{\mathrm {new}}$
. The restriction of
$$ \begin{align*} q_{L'}\oplus -q_N\cong(q_L\oplus -q_N)\oplus q_{\mathrm{ new}} \end{align*} $$
to
$\Gamma _{\gamma '}^{\perp } / \Gamma _{\gamma '}$
is isomorphic to
$-q_K\oplus q_{\mathrm {new}}$
via the isomorphism
$\delta ':=(\delta , \mathrm {id})$
.
Take a negative-definite lattice
$K'$
of rank
$10 - k$
as above, that is, such that
$A_{K'}\cong A_K \oplus A_{\mathrm {new}}$
and
$q_{K'}\cong q_K\oplus -q_{\mathrm {new}}$
. Let
$i'\colon L'\hookrightarrow N$
be a primitive embedding associated to the datum
$(H_{L'}, H_{N}, \gamma ', K', \delta ')$
.
To prove that
$i^{\prime *}(\varepsilon )=i(\varepsilon )$
under the natural identification (2), it is enough to show that the induced embeddings of
${\mathbb Z}_2$
-lattices
$i_2\colon L\otimes {\mathbb Z}_2\hookrightarrow N\otimes {\mathbb Z}_2$
and
$i^{\prime }_2\colon L'\otimes {\mathbb Z}_2\hookrightarrow N\otimes {\mathbb Z}_2$
are isomorphic.
First, we claim that
$K \otimes {\mathbb Z}_2$
and
$K' \otimes {\mathbb Z}_2$
are isomorphic
${\mathbb Z}_2$
-lattices. Since K and
$K'$
are negative-definite of the same rank, and
$|A_{K'}| = |A_K| \cdot |A_{\mathrm {new}}|$
, we have
$\mathrm {discr}(K')= \mathrm {discr}(K)\cdot |A_{\mathrm {new}}|$
. Since
$|A_{\mathrm {new}}|$
is a square of an odd integer, the even
$2$
-adic lattices
$K \otimes {\mathbb Z}_2$
and
$K' \otimes {\mathbb Z}_2$
have the same rank, the same discriminant form, and the same discriminant modulo
${\mathbb Z}_2^{\times 2}$
. This implies that the
${\mathbb Z}_2$
-lattices
$K \otimes {\mathbb Z}_2$
and
$K' \otimes {\mathbb Z}_2$
are isomorphic (see [Reference NikulinNik79, Cor. 1.9.3]).
It remains to show that after tensoring with
${\mathbb Z}_2$
the data
$(H_{L}, H_{N}, \gamma , K, \delta )$
and
$(H_{L'}, H_{N}, \gamma ', K', \delta ')$
give rise to isomorphic embeddings of
$L' \otimes {\mathbb Z}_2 \cong L \otimes {\mathbb Z}_2$
into
$N\otimes {\mathbb Z}_2$
. The first three entries of each datum are the same. By Remark 5.3, it is enough to find an isomorphism of
${\mathbb Z}_2$
-lattices
$f \colon K' \otimes {\mathbb Z}_2 \rightarrow K \otimes {\mathbb Z}_2$
such that
$\delta ^{\prime }_2 =\delta _2 \circ \bar {f}$
. The existence of such an f follows from [Reference NikulinN, Th. 1.9.5]. This concludes the proof of (a).
(b) Write
$A := A_L = A_2 \oplus A_{\mathrm {odd}}$
, where
$A_2$
is the 2-primary subgroup of A. Similarly, write
$A' := A_{L'} = A^{\prime }_2 \oplus A^{\prime }_{\mathrm {odd}}$
. It is clear that
$A_2\cong A^{\prime }_2$
. Then
$q_{L'}$
is an orthogonal direct sum of quadratic forms
$q_{L,2}$
on
$A_2$
and
$q_{\mathrm {odd}}$
on
$A^{\prime }_{\mathrm {odd}}$
.
The overlattice L of
$L'$
defines an isotropic subgroup
$I \subset A'$
, where
$|I|=[L:L']$
, so that
$q_L$
is the quadratic form induced by
$q_{L'}$
on
$A= I^{\perp }/I$
. Since
$[L \colon L']$
is odd by assumption, we have
$I \subset A^{\prime }_{\mathrm {odd}}$
. Thus
$I^{\perp } = A_2 \oplus I^{\perp }_{\mathrm {odd}}$
, where
$I^{\perp }_{\mathrm {odd}}=I^{\perp }\cap A^{\prime }_{\mathrm {odd}}$
. This shows that
$A= A_2 \oplus (I^{\perp }_{\mathrm {odd}}/I)$
.
Let
$i'\colon L'\hookrightarrow N$
be a primitive embedding such that
$i^{\prime *}(\varepsilon )\neq 0$
. Then
$K':=i(L')^{\perp }_N$
is an even negative-definite lattice of rank
$10-k$
. Let
$(H_{L'}, H_N, \gamma ', K', \delta ')$
be a datum associated to
$i'\colon L'\hookrightarrow N$
as in Theorem 5.2. In particular,
$\delta '$
is an isomorphism of
$-q_{K'}$
with the restriction of
$q_{L'}\oplus -q_N$
to
$\Gamma _{\gamma '}^{\perp } / \Gamma _{\gamma '}$
. Since
$2A_N=0$
, we have
$2H_{L'}=0$
, so that
$H_{L'} \subset A^{\prime }_2=A_2$
. Hence
$\Gamma _{\gamma '} \subset A_2\oplus A_N \subset A' \oplus A_N$
and thus
$\Gamma _{\gamma '}^{\perp } = (\Gamma _{\gamma '}^{\perp })_2 \oplus A^{\prime }_{\mathrm {odd}}$
, where
$(\Gamma _{\gamma '}^{\perp })_2=\Gamma _{\gamma '}^{\perp }\cap (A_2 \oplus A_N)$
. This shows that
$\delta '$
identifies the finite quadratic form
$-q_{K'}$
on
$A_{K'}$
with the restriction of
$(q_{L,2}\oplus -q_N)\oplus q_{\mathrm {odd}}$
to
$\big ((\Gamma _{\gamma '}^{\perp }\big )_2/\Gamma _{\gamma '}) \oplus A^{\prime }_{\mathrm {odd}}$
.
The isotropic subgroup
$I \subset A^{\prime }_{\mathrm {odd}}$
gives rise, via
$\delta '$
, to an isotropic subgroup in
$A_{K'}$
. The latter defines an overlattice
$K' \subset K$
with
$[K:K']=[L:L']$
, so that
$\delta '$
induces an isomorphism
$\delta $
of the quadratic form
$-q_K$
on
$A_K$
with the restriction of
$(q_{L,2}\oplus -q_N)\oplus q_{\mathrm {odd}}$
to
$\big ( (\Gamma _{\gamma '}^{\perp })_2/\Gamma _{\gamma '}\big )\oplus (I^{\perp }_{\mathrm {odd}}/I)$
. Let
$i\colon L\hookrightarrow N$
be a primitive embedding associated to the datum
$(H_{L}, H_N, \gamma , K, \delta )$
, where
$H_L=H_{L'}$
and
$\gamma =\gamma '$
.
To complete the proof of (b), it remains to show that i and
$i'$
induce isomorphic embeddings of
${\mathbb Z}_2$
-lattices. This is proved by the same arguments as in (a).
Corollary 5.7. Let L be an even lattice of signature
$(2_+, k_-)$
, where
$0\leq k\leq 8$
. Write
${\mathcal S}(L)$
for the set of nonzero homomorphisms
$\alpha \colon L\to {\mathbb Z}/2$
such that there is a primitive embedding
$i\colon L\hookrightarrow N$
with
$\alpha =i^*(\varepsilon )$
. Let
$L'$
be a sublattice of L that satisfies condition
$(*)$
. Then, under the natural identification
$\mathrm {{Hom}}(L,{\mathbb Z}/2) \cong \mathrm {{Hom}}(L', {\mathbb Z}/2)$
, we have
${\mathcal S}(L)={\mathcal S}(L')$
.
Proof. Part (a) of Theorem 5.6 implies
${\mathcal S}(L)\subset {\mathcal S}(L')$
, whereas part (b) implies
${\mathcal S}(L')\subset {\mathcal S}(L)$
since
$[L:L']$
is odd.
Proof of Theorem D
By Proposition 5.5, there are infinitely many sublattices
$T \subset T(X)$
with pairwise different discriminants that satisfy condition
$(*)$
. Endow T with the Hodge structure coming from
$T(X)$
. Since
$\mathrm {{rk}}(T) \leq 10$
, by [Reference NikulinN, Th. 1.14.4], there exists a unique primitive embedding of the lattice T into the K3 lattice
$\Lambda $
. We equip
$\Lambda $
with the Hodge structure induced by the Hodge structure on T so that
$T^{\perp }_{\Lambda }\subset \Lambda ^{(1,1)}$
. By the surjectivity of the period map, there is a K3 surface Y together with a Hodge isometry between
$\Lambda $
and
$\mathrm {H}^2(Y,{\mathbb Z})$
. The transcendental lattice
$T(Y)$
is the orthogonal complement to
$\mathrm {H}^2(Y,{\mathbb Z})^{(1,1)}$
, hence
$T(Y)\cong T$
.
Applying Corollary 5.7 with
$L=T(X)$
, we obtain
${\mathcal S}(T(X))={\mathcal S}(T(Y))$
. Now Corollary 2.6(i) (whose proof uses Lemma 2.3) gives
$\mathrm {{Im}}(\Phi _X)\setminus \{0\}=\mathrm {{Im}}(\Phi _Y)\setminus \{0\}$
.
Acknowledgments
This note was written while A.N.S. was a fellow of FRIAS, University of Freiburg. He is grateful to FRIAS for hospitality and excellent working conditions. We would like to thank the referee for careful reading of the paper and helpful suggestions.
