Article contents
On functionally constant invariants of the Riemann tensor
Published online by Cambridge University Press: 24 October 2008
Abstract
In any V4 two quadratic invariants of the Riemann tensor, J4 and K4, say, are known which are functionally constant, i.e. the Hamiltonian derivatives of which vanish identically. Certain natural generalizations, J2n and K2n, of degree n of these are considered, J2n being defined in any Vm but K2n only in a V2n. It is shown by methods having purely local character that J2n is functionally constant in any V2n, and that K2n vanishes identically when n is odd or when n is even and the V2n is either conformally flat or admits a hypersurface-orthogonal Killing vector; whilst in a V4n it is functionally constant. These results follow upon a prior spinorial treatment of the special case n = 3 which leads to an explicit expression for a pseudo-vector density of which the scalar density 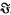 6 is the divergence. Finally, it is shown that for appropriate values of n there exist functionally constant invariants other than J2n and K2n
6 is the divergence. Finally, it is shown that for appropriate values of n there exist functionally constant invariants other than J2n and K2n
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 68 , Issue 1 , July 1970 , pp. 179 - 185
- Copyright
- Copyright © Cambridge Philosophical Society 1970
References
REFERENCES
- 8
- Cited by


