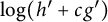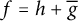1 results
Integral mean estimates for univalent and locally univalent harmonic mappings
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 67 / Issue 3 / September 2024
- Published online by Cambridge University Press:
- 15 January 2024, pp. 655-669
- Print publication:
- September 2024
-
- Article
-
- You have access
- HTML
- Export citation
-
We verify a long-standing conjecture on the membership of univalent harmonic mappings in the Hardy space, whenever the functions have a “nice” analytic part. We also produce a coefficient estimate for these functions, which is in a sense best possible. The problem is then explored in a new direction, without the additional hypothesis. Interestingly, our ideas extend to certain classes of locally univalent harmonic mappings. Finally, we prove a Baernstein-type extremal result for the function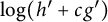
$\log (h'+cg')$, when 
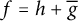$f=h+\overline {g}$ is a close-to-convex harmonic function, and c is a constant. This leads to a sharp coefficient inequality for these functions.
