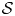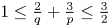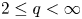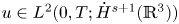1 results
Fractal dimension of potential singular points set in the Navier–Stokes equations under supercritical regularity
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 154 / Issue 3 / June 2024
- Published online by Cambridge University Press:
- 18 April 2023, pp. 727-745
- Print publication:
- June 2024
-
- Article
-
- You have access
- HTML
- Export citation
-
The main objective of this paper is to answer the questions posed by Robinson and Sadowski [22, p. 505, Commun. Math. Phys., 2010] for the Navier–Stokes equations. Firstly, we prove that the upper box dimension of the potential singular points set $\mathcal {S}$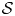
of suitable weak solution $u$
belonging to $L^{q}(0,T;L^{p}(\mathbb {R}^{3}))$
for $1\leq \frac {2}{q}+\frac {3}{p}\leq \frac 32$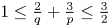
with $2\leq q<\infty$
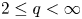and $2< p<\infty$
is at most $\max \{p,q\}(\frac {2}{q}+\frac {3}{p}-1)$
in this system. Secondly, it is shown that $1-2s$
dimension Hausdorff measure of potential singular points set of suitable weak solutions satisfying $u\in L^{2}(0,T;\dot {H}^{s+1}(\mathbb {R}^{3}))$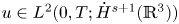
for $0\leq s\leq \frac 12$
is zero, whose proof relies on Caffarelli–Silvestre's extension. Inspired by Barker–Wang's recent work [1], this further allows us to discuss the Hausdorff dimension of potential singular points set of suitable weak solutions if the gradient of the velocity is under some supercritical regularity.
