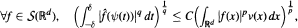Skip to main content Accessibility help

We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.

Close cookie message
×
  1. Home
  2. Search

Refine search

Refine search

Access:
Content type:
Publication date:
Apply   From and To filters
Subject: Show more
Journals Show more
Publishers: Show more
Societies Show more
Series: Show more
Collections: Show more

Actions for selected content:

Select all | Deselect all
  • View selected items
  • Export citations
  • Download PDF (zip)
  • Save to Kindle
  • Save to Dropbox
  • Save to Google Drive

Save Search

You can save your searches here and later view and run them again in "My saved searches".

Please provide a title, maximum of 40 characters.
Cancel
×

2 results

  • Transference of Vector-valued Multipliers on Weighted Lp-spaces

  • Oscar Blasco, Paco Villarroya
  • Journal:
    Canadian Journal of Mathematics / Volume 65 / Issue 3 / 01 June 2013
    Published online by Cambridge University Press:
    20 November 2018, pp. 510-543
    Print publication:
    01 June 2013
    • Article
      • You have access
    • PDF
    • Export citation

  • New transference results for Fourier multiplier operators defined by regulated symbols are presented. We prove restriction and extension of multipliers between weighted Lebesgue spaces with two different weights, which belong to a class more general than periodic weights, and two different exponents of integrability that can be below one.

    We also develop some ad-hoc methods that apply to weights defined by the product of periodic weights with functions of power type. Our vector-valued approach allows us to extend our results to transference of maximal multipliers and provide transference of Littlewood–Paley inequalities.

  • Weighted Restriction for Curves

  • Joseph D. Lakey
  • Journal:
    Canadian Mathematical Bulletin / Volume 36 / Issue 1 / 01 March 1993
    Published online by Cambridge University Press:
    20 November 2018, pp. 87-95
    Print publication:
    01 March 1993
    • Article
      • You have access
    • PDF
    • Export citation

  • We prove weighted norm inequalities for the Fourier transform of the form

    where v is a nonnegative weight function on ℝd and ψ: [— 1,1 ] —> ℝd is a nondegenerate curve. Our results generalize unweighted (i.e. v = 1) restriction theorems of M. Christ, and two-dimensional weighted restriction theorems of C. Carton-Lebrun and H. Heinig.