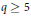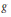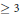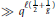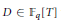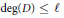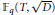1 results
Exponents of Class Groups of Quadratic Function Fields over Finite Fields
-
- Journal:
- Canadian Mathematical Bulletin / Volume 44 / Issue 4 / 01 December 2001
- Published online by Cambridge University Press:
- 20 November 2018, pp. 398-407
- Print publication:
- 01 December 2001
-
- Article
-
- You have access
- Export citation
-
We find a lower bound on the number of imaginary quadratic extensions of the function field
${{\mathbb{F}}_{q}}\left( T \right)$ whose class groups have an element of a fixed order.More precisely, let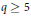
$q\,\ge \,5$ be a power of an odd prime and let 
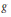$g$ be a fixed positive integer 
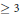$\ge \,3$. There are 
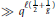$\gg \,{{q}^{\ell \left( \frac{1}{2}+\frac{1}{g} \right)}}$ polynomials 
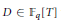$D\,\in \,{{\mathbb{F}}_{q}}\left[ T \right]$ with 
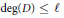$\deg \left( D \right)\,\le \,\ell $ such that the class groups of the quadratic extensions 
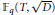${{\mathbb{F}}_{q}}\left( T,\,\sqrt{D} \right)$ have an element of order 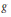$g$.
