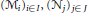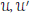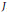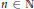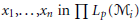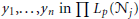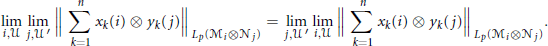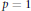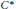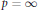1 results
Fubini's Theorem for Ultraproducts of Noncommutative Lp-Spaces
-
- Journal:
- Canadian Journal of Mathematics / Volume 56 / Issue 5 / 01 October 2004
- Published online by Cambridge University Press:
- 20 November 2018, pp. 983-1021
- Print publication:
- 01 October 2004
-
- Article
-
- You have access
- Export citation
-
Let
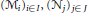${{({{\mathcal{M}}_{i}})}_{i}}{{_{\in }}_{I}},{{({{\mathcal{N}}_{j}})}_{j\in J}}$
be families of von Neumann algebras and
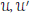$\mathcal{U},\,{\mathcal{U}}'$
be ultrafilters in 
$I$,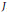 
$J$, respectively. Let 
$1\,\le \,p\,<\,\infty $ and 
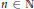$n\,\in \,\mathbb{N}$. Let
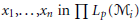${{x}_{1}},...,{{x}_{n}}\,\text{in}\,\prod {{L}_{p}}({{\mathcal{M}}_{i}})$ and 
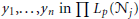${{y}_{1}},...,{{y}_{n\,}}\text{in }\Pi \,{{L}_{p}}({{\mathcal{N}}_{j}})$
be bounded families. We show the following equality
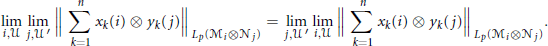$$\underset{i,\mathcal{U}}{\mathop{\lim }}\,\,\underset{j,{\mathcal{U}}'}{\mathop{\lim }}\,\,{{\left\| \sum\limits_{k=1}^{n}{{{x}_{k}}(i)\,\otimes \,{{y}_{k}}(j)} \right\|}_{{{L}_{p}}({{\mathcal{M}}_{i}}\otimes {{\mathcal{N}}_{j}})}}=\,\underset{j,{\mathcal{U}}'}{\mathop{\lim }}\,\,\underset{i,\mathcal{U}}{\mathop{\lim }}\,\,{{\left\| \sum\limits_{k=1}^{n}{{{x}_{k}}(i)\,\,\otimes \,{{y}_{k}}(j)} \right\|}_{{{L}_{p}}({{\mathcal{M}}_{i}}\otimes {{\mathcal{N}}_{j}})}}$$For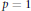
$p\,=\,1$ this Fubini type result is related to the local reflexivity of duals of 
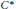${{C}^{*}}$
-algebras. This fails for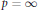 
$P=\infty $.
