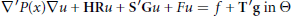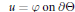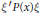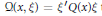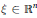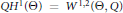1 results
Existence of Weak Solutions of Linear Subelliptic Dirichlet Problems with Rough Coefficients
-
- Journal:
- Canadian Journal of Mathematics / Volume 64 / Issue 6 / 01 December 2012
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1395-1414
- Print publication:
- 01 December 2012
-
- Article
-
- You have access
- Export citation
-
This article gives an existence theory for weak solutions of second order non-elliptic linear Dirichlet problems of the form
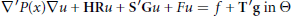$${\nabla }'P\left( x \right)\nabla u+\text{HR}u+\text{{S}'G}u\text{+ F}u\text{=}\text{f}\,\text{+}\,\text{{T}'g}\,\text{in}\,\Theta$$
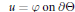$$u=\varphi \,\,on\,\,\partial \Theta$$The principal part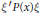
${\xi }'P\left( x \right)\xi$ of the above equation is assumed to be comparable to a quadratic form 
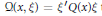$\mathcal{Q}\left( x,\xi \right)={\xi }'Q\left( x \right)\xi$ that may vanish for non-zero 
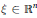$\xi \in {{\mathbb{R}}^{n}}$. This is achieved using techniques of functional analysis applied to the degenerate Sobolev spaces 
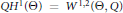$Q{{H}^{1}}\left( \Theta \right)={{W}^{1,2}}\left( \Theta ,Q \right)$ and 
$QH_{0}^{1}\left( \Theta \right)=W_{0}^{1,2}\left( \Theta ,Q \right)$ as defined in previous works. E.T. Sawyer and R.L. Wheeden (2010) have given a regularity theory for a subset of the class of equations dealt with here.
