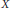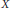J. Elton used an application of Ramsey theory to show that if $X$ is an infinite dimensional Banach space, then ${{c}_{0}}$ embeds in $X$, ${{\ell }_{1}}$ embeds in 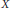$X$, or there is a subspace of 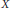$X$ that fails to have the Dunford–Pettis property. Bessaga and Pelczynski showed that if ${{c}_{0}}$ embeds i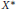n ${{X}^{*}}$ , then 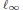${{\ell }_{\infty }}$ embeds in 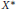${{X}^{*}}.$ Emmanuele and John showed that if ${{c}_{0}}$ embeds in 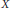$K\left( X,\,Y \right)$, then 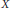$K\left( X,\,Y \right)$ is not complemented in 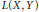$L\left( X,\,Y \right)$. Classical results from Schauder basis theory are used in a study of Dunford–Pettis sets and strong Dunford–Pettis sets to extend each of the preceding theorems. The space 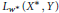${{L}_{{{w}^{*}}}}\left( {{X}^{*}},\,Y \right)$ of 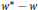${{w}^{*}}\,-\,w$ continuous operators is also studied.