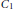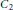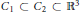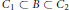1 results
Optimal Roughening of Convex Bodies
-
- Journal:
- Canadian Journal of Mathematics / Volume 64 / Issue 5 / 01 October 2012
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1058-1074
- Print publication:
- 01 October 2012
-
- Article
-
- You have access
- Export citation
-
A body moves in a rarefied medium composed of point particles at rest. The particles make elastic reflections when colliding with the body surface and do not interact with each other. We consider a generalization of Newton’s minimal resistance problem: given two bounded convex bodies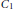
${{C}_{1}}$ and 
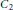${{C}_{2}}$ such that 
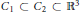${{C}_{1}}\,\subset \,{{C}_{2}}\,\subset \,{{\mathbb{R}}^{3}}$ and 
$\partial {{C}_{1}}\,\cap \,\partial {{C}_{2}}\,=\,\varnothing $, minimize the resistance in the class of connected bodies 
$B$ such that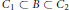 
${{C}_{1}}\,\subset \,B\,\subset \,{{C}_{2}}$. We prove that the infimum of resistance is zero; that is, there exist “almost perfectly streamlined” bodies.
