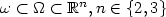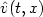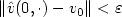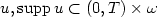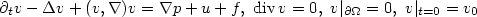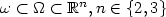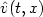We study the local exact controllability problem for the Navier-Stokes equations that describe an incompressible fluid flow in a bounded domain Ω with control distributed in a subdomain $\omega\subset\Omega\subset \mathbb{R}^n, n\in\{2,3\}$. The result that we obtained in this paper is as follows. Suppose that $\hat v(t,x)$ is a given solution of the Navier-Stokes equations. Let $ v_0(x)$ be a given initial condition and $\Vert \hat v(0,\cdot) - v_0 \Vert < \varepsilon$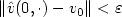 where ε is small enough. 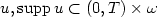Then there exists a locally distributed control $u, \text{supp}\, u\subset (0,T)\times \omega $ such that the solution v(t,x) of the Navier-Stokes equations: $$ \partial_tv-\Delta v+(v,\nabla)v=\nabla p+u+f, \,\, \text{\rm div}\, v=0,\,\, v\vert_{\partial\Omega}=0, \,\, v \vert_{t=0} = v_0 $$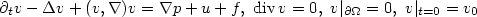 coincides with $\hat v(t,x)$ 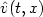at the instant T : $v(T,x) \equiv \hat v(T,x)$.