We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
For every fixed-point expression e of alternation-depth r,
we construct a new fixed-point expression e' of alternation-depth 2
and size ${\cal O}(r\cdot|e|)$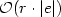
. Expression e' is equivalent to e
whenever operators are distributive and the underlying
complete lattice has a co-continuous least upper bound. We
alternation-depth but also w.r.t. the increase in size of the
resulting expression.
