We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let λ > 0 and let
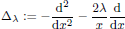
be the Bessel operator on ℝ+ := (0,∞). We show that the oscillation operator 𝒪(RΔλ,∗) and variation operator 𝒱ρ(RΔλ,∗) of the Riesz transform RΔλ associated with Δλ are both bounded on Lp(ℝ+, dmλ) for p ∈ (1,∞), from L1(ℝ+, dmλ) to L1,∞(ℝ+, dmλ), and from L∞(ℝ+, dmλ) to BMO(ℝ+, dmλ), where ρ ∈ (2,∞) and dmλ(x) := x2λ dx. As an application, we give the corresponding Lp-estimates for β-jump operators and the number of up-crossings.
