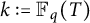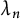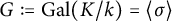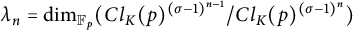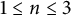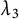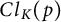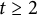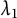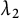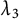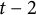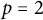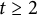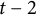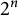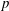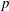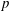We study the Galois module structure of the class groups of the Artin–Schreier extensions K over k of extension degree p, where 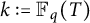 $k:={\mathbb F}_q(T)$ is the rational function field and p is a prime number. The structure of the p-part
$k:={\mathbb F}_q(T)$ is the rational function field and p is a prime number. The structure of the p-part  $Cl_K(p)$ of the ideal class group of K as a finite G-module is determined by the invariant
$Cl_K(p)$ of the ideal class group of K as a finite G-module is determined by the invariant 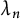 ${\lambda }_n$, where
${\lambda }_n$, where 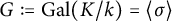 $G:=\operatorname {\mathrm {Gal}}(K/k)=\langle {\sigma } \rangle $ is the Galois group of K over k, and
$G:=\operatorname {\mathrm {Gal}}(K/k)=\langle {\sigma } \rangle $ is the Galois group of K over k, and 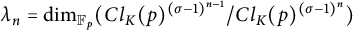 ${\lambda }_n = \dim _{{\mathbb F}_p}(Cl_K(p)^{({\sigma }-1)^{n-1}}/Cl_K(p)^{({\sigma }-1)^{n}})$. We find infinite families of the Artin–Schreier extensions over k whose ideal class groups have guaranteed prescribed
${\lambda }_n = \dim _{{\mathbb F}_p}(Cl_K(p)^{({\sigma }-1)^{n-1}}/Cl_K(p)^{({\sigma }-1)^{n}})$. We find infinite families of the Artin–Schreier extensions over k whose ideal class groups have guaranteed prescribed  ${\lambda }_n$-rank for
${\lambda }_n$-rank for 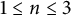 $1 \leq n \leq 3$. We find an algorithm for computing
$1 \leq n \leq 3$. We find an algorithm for computing 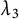 ${\lambda }_3$-rank of
${\lambda }_3$-rank of 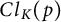 $Cl_K(p)$. Using this algorithm, for a given integer
$Cl_K(p)$. Using this algorithm, for a given integer 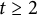 $t \ge 2$, we get infinite families of the Artin–Schreier extensions over k whose
$t \ge 2$, we get infinite families of the Artin–Schreier extensions over k whose 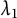 ${\lambda }_1$-rank is t,
${\lambda }_1$-rank is t, 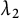 ${\lambda }_2$-rank is
${\lambda }_2$-rank is  $t-1$, and
$t-1$, and 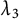 ${\lambda }_3$-rank is
${\lambda }_3$-rank is 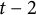 $t-2$. In particular, in the case where
$t-2$. In particular, in the case where 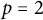 $p=2$, for a given positive integer
$p=2$, for a given positive integer 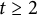 $t \ge 2$, we obtain an infinite family of the Artin–Schreier quadratic extensions over k whose
$t \ge 2$, we obtain an infinite family of the Artin–Schreier quadratic extensions over k whose 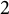 $2$-class group rank (resp.
$2$-class group rank (resp.  $2^2$-class group rank and
$2^2$-class group rank and 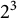 $2^3$-class group rank) is exactly t (resp.
$2^3$-class group rank) is exactly t (resp.  $t-1$ and
$t-1$ and 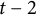 $t-2$). Furthermore, we also obtain a similar result on the
$t-2$). Furthermore, we also obtain a similar result on the 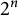 $2^n$-ranks of the divisor class groups of the Artin–Schreier quadratic extensions over k.
$2^n$-ranks of the divisor class groups of the Artin–Schreier quadratic extensions over k.
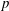 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}p$-cyclic McKay correspondence via motivic integration
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}p$-cyclic McKay correspondence via motivic integration