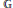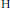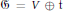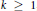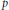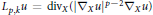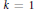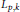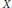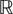We prove the existence of nontrivial ground state solutions of the critical quasilinear Hénon equation $\displaystyle -\Delta _p u=|x|^{\alpha _1}|u|^{p^{*}(\alpha _1)-2}u-|x|^{\alpha _2}|u|^{p^{*}(\alpha _2)-2}u\ \ {\rm in}\ \mathbb {R}^{N}.$ It is a new problem in the sense that the signs of the coefficients of critical terms are opposite.

Let 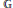$\mathbb{G}$ be a step-two nilpotent group of 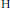$\text{H}$-type with Lie algebra 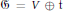$\mathfrak{G}\,=\,V\,\oplus \,\text{t}$. We define a class of vector fields $X\,=\,\left\{ {{X}_{j}} \right\}$ on 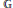$\mathbb{G}$ depending on a real parameter 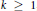$k\,\ge \,1$, and we consider the corresponding 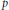$p$-Laplacian operator 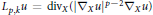${{L}_{p,\,k}}u\,=\,di{{v}_{X}}\left( {{\left| {{\nabla }_{X}}u \right|}^{p-2}}{{\nabla }_{X}}u \right)$. For 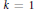$k\,=\,1$ the vector fields $X\,=\,\left\{ {{X}_{j}} \right\}$ are the left invariant vector fields corresponding to an orthonormal basis of $V$; for $\mathbb{G}$ being the Heisenberg group the vector fields are the Greiner fields. In this paper we obtain the fundamental solution for the operator 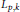${{L}_{p,\,k}}$ and as an application, we get a Hardy type inequality associated with 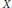$X$.

In this paper we show existence of positive solutions for a class of quasi-linear problems with Neumann boundary conditions defined in a half-space and involving the critical exponent.