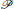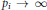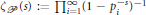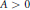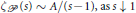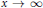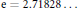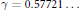1 results
On Mertens' Theorem for Beurling Primes
-
- Journal:
- Canadian Mathematical Bulletin / Volume 56 / Issue 4 / 01 December 2013
- Published online by Cambridge University Press:
- 20 November 2018, pp. 829-843
- Print publication:
- 01 December 2013
-
- Article
-
- You have access
- Export citation
-
Let
$1\,<\,{{p}_{1}}\,\le \,{{p}_{2}}\,\le \,{{p}_{3}}\,\le \,...$ be an infinite sequence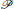 
$P$ of real numbers for which 
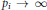${{p}_{i}}\,\to \,\infty $, and associate with this sequence the Beurling zeta function
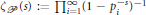$\zeta P\left( s \right)\,:=\,{{\prod\nolimits_{i=1}^{\infty }{\left( 1\,-\,p_{i}^{-s} \right)}}^{-1}}$. Suppose that for some constant 
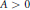$A\,>\,0$, we have 
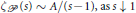$\zeta P\left( s \right)\tilde{\ }A/\left( s-1 \right),\ \text{as}\,s\,\downarrow \,1$. We prove that 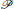$P$ satisfies an analogue of a classical theorem of Mertens: 
${{\prod{_{{{p}_{i}}\le x}\left( 1\,-\,{1}/{{{p}_{i}}}\; \right)}}^{-1}}\,\sim \,A{{\text{e}}^{\gamma }}\,\log \,x$, as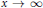 
$x\,\to \,\infty $. Here 
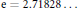$\text{e}\,\text{=}\,\text{2}\text{.71828}...$ is the base of the natural logarithm and 
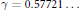$\gamma \,=\,0.57721...$ is the usual Euler–Mascheroni constant. This strengthens a recent theorem of Olofsson.
