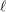1 Introduction
Let
![]() $C/K$
be a proper, smooth geometrically irreducible curve, with
$C/K$
be a proper, smooth geometrically irreducible curve, with
![]() $K$
a number field, let
$K$
a number field, let
![]() $f:E\rightarrow U\subset C$
be a non-isotrivial family of elliptic curves over a non-empty open subset
$f:E\rightarrow U\subset C$
be a non-isotrivial family of elliptic curves over a non-empty open subset
![]() $U$
of
$U$
of
![]() $C$
, and let
$C$
, and let
![]() $L=\text{R}^{1}f_{\ast }(\overline{\mathbf{Q}}_{\ell })$
be the associated rank-two lisse
$L=\text{R}^{1}f_{\ast }(\overline{\mathbf{Q}}_{\ell })$
be the associated rank-two lisse
![]() $\ell$
-adic sheaf on
$\ell$
-adic sheaf on
![]() $U$
. The following properties hold.
$U$
. The following properties hold.
(a) There is an isomorphism
 $\bigwedge ^{2}L\cong \overline{\mathbf{Q}}_{\ell }(1)$
.
$\bigwedge ^{2}L\cong \overline{\mathbf{Q}}_{\ell }(1)$
.(b) There exists a proper smooth model
 ${\mathcal{C}}$
of
${\mathcal{C}}$
of
 $C$
over
$C$
over
 $\operatorname{Spec}{\mathcal{O}}_{K}[1/N]$
, an open subset
$\operatorname{Spec}{\mathcal{O}}_{K}[1/N]$
, an open subset
 ${\mathcal{U}}$
of
${\mathcal{U}}$
of
 ${\mathcal{C}}$
extending
${\mathcal{C}}$
extending
 $U$
, and a lisse sheaf
$U$
, and a lisse sheaf
 ${\mathcal{L}}$
on
${\mathcal{L}}$
on
 ${\mathcal{U}}$
extending
${\mathcal{U}}$
extending
 $L$
.
$L$
.(c) For every closed point
 $x$
of
$x$
of
 ${\mathcal{U}}$
, the trace of the Frobenius element on
${\mathcal{U}}$
, the trace of the Frobenius element on
 ${\mathcal{L}}_{x}$
is a rational number.
${\mathcal{L}}_{x}$
is a rational number.(d) There exists a point
 $x$
of
$x$
of
 $C_{\overline{K}}$
at which
$C_{\overline{K}}$
at which
 $L_{\overline{K}}$
does not have potentially good reduction, i.e., the restriction to the inertia subgroup at
$L_{\overline{K}}$
does not have potentially good reduction, i.e., the restriction to the inertia subgroup at
 $x$
of the representation of
$x$
of the representation of
 $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U_{\overline{K}})$
associated to
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U_{\overline{K}})$
associated to
 $L_{\overline{K}}$
does not act through a finite order quotient.
$L_{\overline{K}}$
does not act through a finite order quotient.
To see (d), take
![]() $x$
to be a pole of the
$x$
to be a pole of the
![]() $j$
-invariant of
$j$
-invariant of
![]() $E$
.
$E$
.
The purpose of this paper is to prove the following converse of the above statement.
Theorem 1. Let
![]() $C/K$
be as above, and let
$C/K$
be as above, and let
![]() $L$
be an irreducible rank-two lisse
$L$
be an irreducible rank-two lisse
![]() $\overline{\mathbf{Q}}_{\ell }$
-sheaf over an open subset
$\overline{\mathbf{Q}}_{\ell }$
-sheaf over an open subset
![]() $U\subset C$
. Assume conditions (a)–(d) above hold. Then there exists a family of elliptic curves
$U\subset C$
. Assume conditions (a)–(d) above hold. Then there exists a family of elliptic curves
![]() $f:E\rightarrow U$
and an isomorphism
$f:E\rightarrow U$
and an isomorphism
![]() $L\cong \text{R}^{1}f_{\ast }(\overline{\mathbf{Q}}_{\ell })$
.
$L\cong \text{R}^{1}f_{\ast }(\overline{\mathbf{Q}}_{\ell })$
.
Remark 2. The Fontaine–Mazur conjecture predicts that representations of
![]() $G_{K}$
satisfying certain natural conditions should appear in the étale cohomology of algebraic varieties. It seems reasonable to expect some kind of generalization of this conjecture to higher-dimensional bases. Our theorem can be viewed as confirmation of a very simple case of this.
$G_{K}$
satisfying certain natural conditions should appear in the étale cohomology of algebraic varieties. It seems reasonable to expect some kind of generalization of this conjecture to higher-dimensional bases. Our theorem can be viewed as confirmation of a very simple case of this.
Remark 3. One can prove a version of Theorem 1 where
![]() $C$
is replaced with a higher-dimensional variety. We just treat the case of curves to keep the exposition simpler.
$C$
is replaced with a higher-dimensional variety. We just treat the case of curves to keep the exposition simpler.
Remark 4. The theorem is not true if one assumes only (a)–(c). Recall that a fake elliptic curve is an abelian surface
![]() $A$
such that
$A$
such that
![]() $\operatorname{End}(A)$
is an order
$\operatorname{End}(A)$
is an order
![]() $R$
in a non-split quaternion algebra that is split at infinity. The moduli space of fake elliptic curves corresponding to
$R$
in a non-split quaternion algebra that is split at infinity. The moduli space of fake elliptic curves corresponding to
![]() $R$
is a proper curve. We therefore can construct a family
$R$
is a proper curve. We therefore can construct a family
![]() $f:A\rightarrow C$
of fake elliptic curves for some
$f:A\rightarrow C$
of fake elliptic curves for some
![]() $C$
as above. The sheaf
$C$
as above. The sheaf
![]() $\text{R}^{1}f_{\ast }\overline{\mathbf{Q}}_{\ell }$
decomposes as
$\text{R}^{1}f_{\ast }\overline{\mathbf{Q}}_{\ell }$
decomposes as
![]() $L^{\oplus 2}$
for some rank-two lisse sheaf
$L^{\oplus 2}$
for some rank-two lisse sheaf
![]() $L$
. This
$L$
. This
![]() $L$
satisfies (a)–(c) but not (d), and thus does not come from a non-isotrivial family of elliptic curves. (Note that one can take
$L$
satisfies (a)–(c) but not (d), and thus does not come from a non-isotrivial family of elliptic curves. (Note that one can take
![]() $f$
so that
$f$
so that
![]() $L$
is not isotrivial, in which case
$L$
is not isotrivial, in which case
![]() $L$
does not come from any family of elliptic curves.)
$L$
does not come from any family of elliptic curves.)
Question 5. Suppose that for each prime number
![]() $\ell$
we have an irreducible rank-two
$\ell$
we have an irreducible rank-two
![]() $\mathbf{Q}_{\ell }$
sheaf
$\mathbf{Q}_{\ell }$
sheaf
![]() $L_{\ell }$
satisfying (a)–(c) such that
$L_{\ell }$
satisfying (a)–(c) such that
![]() $\{L_{\ell }\}$
forms a compatible system (meaning that the
$\{L_{\ell }\}$
forms a compatible system (meaning that the
![]() ${\mathcal{U}}$
in part (b) can be chosen uniformly and that the traces of Frobenius elements are independent of
${\mathcal{U}}$
in part (b) can be chosen uniformly and that the traces of Frobenius elements are independent of
![]() $\ell$
). Does the system come from a family of elliptic curves? Note that the fake elliptic curve counterexample does not apply here: if
$\ell$
). Does the system come from a family of elliptic curves? Note that the fake elliptic curve counterexample does not apply here: if
![]() $\ell$
ramifies in
$\ell$
ramifies in
![]() $R\otimes \mathbf{Q}$
then
$R\otimes \mathbf{Q}$
then
![]() $\text{R}^{1}f_{\ast }\mathbf{Q}_{\ell }$
does not decompose as
$\text{R}^{1}f_{\ast }\mathbf{Q}_{\ell }$
does not decompose as
![]() $L^{\oplus 2}$
.
$L^{\oplus 2}$
.
1.1 Summary of the proof
The basic idea is to use Drinfeld’s results on the global Langlands program to construct an elliptic curve over
![]() ${\mathcal{C}}_{\mathbf{F}_{v}}$
for most places
${\mathcal{C}}_{\mathbf{F}_{v}}$
for most places
![]() $v$
of
$v$
of
![]() ${\mathcal{O}}_{K}$
, and then piece these together to get one over
${\mathcal{O}}_{K}$
, and then piece these together to get one over
![]() ${\mathcal{C}}$
. More precisely, we proceed as follows.
${\mathcal{C}}$
. More precisely, we proceed as follows.
∙ We first show that we are free to pass to finite covers of
 $C$
. The main content here is a descent result that shows that if
$C$
. The main content here is a descent result that shows that if
 $L$
comes from an elliptic curve over a cover of
$L$
comes from an elliptic curve over a cover of
 $C$
then it comes from an elliptic curve over
$C$
then it comes from an elliptic curve over
 $C$
. Using this, we replace
$C$
. Using this, we replace
 $C$
with a cover so that
$C$
with a cover so that
 $L/\ell ^{3}L$
is trivial (after replacing
$L/\ell ^{3}L$
is trivial (after replacing
 $L$
with an integral form).
$L$
with an integral form).∙ We next consider
 $L$
over
$L$
over
 ${\mathcal{C}}_{\mathbf{F}_{v}}$
and use Drinfeld’s results on the global Langlands program to produce a
${\mathcal{C}}_{\mathbf{F}_{v}}$
and use Drinfeld’s results on the global Langlands program to produce a
 $\mathbf{GL}_{2}$
-type abelian variety
$\mathbf{GL}_{2}$
-type abelian variety
 $A_{v}$
realizing
$A_{v}$
realizing
 $L$
.
$L$
.∙ Using hypotheses (c) and (d), we descend the coefficient field of
 $A_{v}$
to
$A_{v}$
to
 $\mathbf{Q}$
, obtaining an elliptic curve
$\mathbf{Q}$
, obtaining an elliptic curve
 $E_{v}$
. (It is likely this could be obtained directly from Drinfeld’s proof.)
$E_{v}$
. (It is likely this could be obtained directly from Drinfeld’s proof.)∙ We next consider a certain moduli space
 ${\mathcal{M}}$
of maps
${\mathcal{M}}$
of maps
 ${\mathcal{U}}\rightarrow Y(\ell ^{3})$
. From the previous step (and the triviality of
${\mathcal{U}}\rightarrow Y(\ell ^{3})$
. From the previous step (and the triviality of
 $L/\ell ^{3}L$
), we see that
$L/\ell ^{3}L$
), we see that
 ${\mathcal{M}}$
has
${\mathcal{M}}$
has
 $\mathbf{F}_{v}$
-points for infinitely many
$\mathbf{F}_{v}$
-points for infinitely many
 $v$
. Since
$v$
. Since
 ${\mathcal{M}}$
is of finite type over
${\mathcal{M}}$
is of finite type over
 ${\mathcal{O}}_{K}$
, it therefore has a
${\mathcal{O}}_{K}$
, it therefore has a
 $\overline{K}$
-point. This yields an elliptic curve
$\overline{K}$
-point. This yields an elliptic curve
 $E_{\overline{K}}$
over
$E_{\overline{K}}$
over
 $U_{\overline{K}}$
realizing
$U_{\overline{K}}$
realizing
 $L_{\overline{K}}$
.
$L_{\overline{K}}$
.∙ Our hypotheses imply that
 $L_{\overline{K}}$
is irreducible. A simple representation theory argument thus shows that there is a finite extension
$L_{\overline{K}}$
is irreducible. A simple representation theory argument thus shows that there is a finite extension
 $K^{\prime }/K$
such that
$K^{\prime }/K$
such that
 $E$
descends to
$E$
descends to
 $U_{K^{\prime }}$
and its Tate module agrees with
$U_{K^{\prime }}$
and its Tate module agrees with
 $L_{K^{\prime }}$
. We have already shown that it suffices to prove the result over a finite cover of
$L_{K^{\prime }}$
. We have already shown that it suffices to prove the result over a finite cover of
 $C$
, so we are now finished.
$C$
, so we are now finished.
We note that we use Faltings’ proof of the Tate conjecture in the third and fifth steps.
1.2 Outline
In § 2 we recall the relevant background material. In § 3, we prove a few descent results for abelian varieties. In § 4, we package Drinfeld’s results on the global Langlands program into the form we need; in particular, we use the results of § 3 to produce elliptic curves (as opposed to
![]() $\mathbf{GL}_{2}$
-type abelian varieties). In § 5, we construct a mapping space parametrizing maps between two affine curves. Finally, in § 6, we prove Theorem 1.
$\mathbf{GL}_{2}$
-type abelian varieties). In § 5, we construct a mapping space parametrizing maps between two affine curves. Finally, in § 6, we prove Theorem 1.
2 Background
2.1 Abelian varieties
Let
![]() $A$
be an abelian variety over a field
$A$
be an abelian variety over a field
![]() $K$
such that
$K$
such that
![]() $\operatorname{End}_{K}(A)\otimes \mathbf{Q}$
contains a number field
$\operatorname{End}_{K}(A)\otimes \mathbf{Q}$
contains a number field
![]() $F$
. Let
$F$
. Let
![]() $V_{\ell }(A)$
denote the rational Tate module of
$V_{\ell }(A)$
denote the rational Tate module of
![]() $A$
at the rational prime
$A$
at the rational prime
![]() $\ell$
. This is a module over
$\ell$
. This is a module over
![]() $F\otimes \mathbf{Q}_{\ell }=\prod _{w\mid \ell }F_{w}$
, and thus decomposes as
$F\otimes \mathbf{Q}_{\ell }=\prod _{w\mid \ell }F_{w}$
, and thus decomposes as
![]() $\bigoplus _{w\mid \ell }V_{w}(A)$
where each
$\bigoplus _{w\mid \ell }V_{w}(A)$
where each
![]() $V_{w}(A)$
is a continuous representation of
$V_{w}(A)$
is a continuous representation of
![]() $G_{K}$
over the field
$G_{K}$
over the field
![]() $F_{w}$
. We recall the following standard results.
$F_{w}$
. We recall the following standard results.
Proposition 6. Let
![]() $\unicode[STIX]{x1D70E}\in \operatorname{End}_{K}(A)$
commute with
$\unicode[STIX]{x1D70E}\in \operatorname{End}_{K}(A)$
commute with
![]() $F$
. Then the characteristic polynomial of
$F$
. Then the characteristic polynomial of
![]() $\unicode[STIX]{x1D70E}$
on
$\unicode[STIX]{x1D70E}$
on
![]() $V_{w}(A)$
(regarded as an
$V_{w}(A)$
(regarded as an
![]() $F_{w}$
-vector space) has coefficients in
$F_{w}$
-vector space) has coefficients in
![]() $F$
and is independent of
$F$
and is independent of
![]() $w$
. In particular, each
$w$
. In particular, each
![]() $V_{w}(A)$
has the same dimension over
$V_{w}(A)$
has the same dimension over
![]() $F_{w}$
.
$F_{w}$
.
Proof. See [Reference ShimuraShi67, § 11.10] and (for
![]() $F=\mathbf{Q}$
) [Reference MilneMil, Proposition 9.23].◻
$F=\mathbf{Q}$
) [Reference MilneMil, Proposition 9.23].◻
Proposition 7. Assume
![]() $K$
is a number field and
$K$
is a number field and
![]() $\operatorname{End}_{K}(A)\otimes \mathbf{Q}=F$
. Let
$\operatorname{End}_{K}(A)\otimes \mathbf{Q}=F$
. Let
![]() $w$
be a place of
$w$
be a place of
![]() $F$
above a prime
$F$
above a prime
![]() $p$
. Then
$p$
. Then
![]() $\operatorname{End}_{\mathbf{Q}_{p}[G_{K}]}(V_{w}(A))=F_{w}$
. In particular,
$\operatorname{End}_{\mathbf{Q}_{p}[G_{K}]}(V_{w}(A))=F_{w}$
. In particular,
![]() $V_{w}(A)$
is absolutely irreducible as a representation of
$V_{w}(A)$
is absolutely irreducible as a representation of
![]() $G_{K}$
over
$G_{K}$
over
![]() $F_{w}$
.
$F_{w}$
.
Proof. We have
where the first equality is the Tate conjecture proved by Faltings [Reference Faltings, Wüstholz, Grunewald, Schappacher and StuhlerFWGSS92, Theorem 1, p. 211]. Since the endmost spaces have the same dimension, we conclude that the containments are equalities, and so
![]() $\operatorname{End}_{\mathbf{Q}_{p}[G_{K}]}(V_{w}(A))=F_{w}$
.◻
$\operatorname{End}_{\mathbf{Q}_{p}[G_{K}]}(V_{w}(A))=F_{w}$
.◻
2.2 Arithmetic fundamental groups
Let
![]() $X$
be an affine normal integral scheme of finite type over
$X$
be an affine normal integral scheme of finite type over
![]() $\mathbf{Z}$
and consider
$\mathbf{Z}$
and consider
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
. For each closed point
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
. For each closed point
![]() $x$
of
$x$
of
![]() $X$
there is a conjugacy class of Frobenius elements
$X$
there is a conjugacy class of Frobenius elements
![]() $F_{x}$
. We recall the following generalization of the Chebotarev density theorem.
$F_{x}$
. We recall the following generalization of the Chebotarev density theorem.
Proposition 8. The elements
![]() $\{F_{x}\}_{x\in X}$
are dense in
$\{F_{x}\}_{x\in X}$
are dense in
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
.
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
.
Proof. This follows from [Reference SerreSer65, Theorem 7]. ◻
Corollary 9. Suppose that
![]() $\unicode[STIX]{x1D70C}_{1}$
and
$\unicode[STIX]{x1D70C}_{1}$
and
![]() $\unicode[STIX]{x1D70C}_{2}$
are semi-simple continuous representations
$\unicode[STIX]{x1D70C}_{2}$
are semi-simple continuous representations
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)\rightarrow \mathbf{GL}_{n}(\overline{\mathbf{Q}}_{\ell })$
such that
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)\rightarrow \mathbf{GL}_{n}(\overline{\mathbf{Q}}_{\ell })$
such that
![]() $\operatorname{tr}(\unicode[STIX]{x1D70C}_{1}(F_{x}))=\operatorname{tr}(\unicode[STIX]{x1D70C}_{2}(F_{x}))$
for all
$\operatorname{tr}(\unicode[STIX]{x1D70C}_{1}(F_{x}))=\operatorname{tr}(\unicode[STIX]{x1D70C}_{2}(F_{x}))$
for all
![]() $x$
. Then
$x$
. Then
![]() $\unicode[STIX]{x1D70C}_{1}$
and
$\unicode[STIX]{x1D70C}_{1}$
and
![]() $\unicode[STIX]{x1D70C}_{2}$
are equivalent.
$\unicode[STIX]{x1D70C}_{2}$
are equivalent.
2.3 Ramification of characters
Lemma 10. Let
![]() $C$
be a curve over a number field
$C$
be a curve over a number field
![]() $K$
, and let
$K$
, and let
![]() $U$
be an open subset. Suppose that
$U$
be an open subset. Suppose that
![]() $\unicode[STIX]{x1D6FC}:\unicode[STIX]{x1D70B}_{1}(U)\rightarrow \overline{\mathbf{Q}}_{\ell }^{\times }$
is a continuous homomorphism. Then for every point
$\unicode[STIX]{x1D6FC}:\unicode[STIX]{x1D70B}_{1}(U)\rightarrow \overline{\mathbf{Q}}_{\ell }^{\times }$
is a continuous homomorphism. Then for every point
![]() $x$
of
$x$
of
![]() $C_{\overline{K}}$
, the inertia subgroup of
$C_{\overline{K}}$
, the inertia subgroup of
![]() $\unicode[STIX]{x1D70B}_{1}(U_{\overline{K}})$
at
$\unicode[STIX]{x1D70B}_{1}(U_{\overline{K}})$
at
![]() $x$
has finite image under
$x$
has finite image under
![]() $\unicode[STIX]{x1D6FC}$
.
$\unicode[STIX]{x1D6FC}$
.
Proof. We are free to replace
![]() $K$
with a finite extension, so we may as well assume
$K$
with a finite extension, so we may as well assume
![]() $x$
is a
$x$
is a
![]() $K$
-point. The decomposition group at
$K$
-point. The decomposition group at
![]() $x$
has the form
$x$
has the form
![]() $\widehat{\mathbf{Z}}\rtimes G_{K}$
, where
$\widehat{\mathbf{Z}}\rtimes G_{K}$
, where
![]() $\widehat{\mathbf{Z}}$
is the geometric inertia group and
$\widehat{\mathbf{Z}}$
is the geometric inertia group and
![]() $G_{K}$
acts on it through the cyclotomic character
$G_{K}$
acts on it through the cyclotomic character
![]() $\unicode[STIX]{x1D712}$
. Let
$\unicode[STIX]{x1D712}$
. Let
![]() $T$
be a topological generator of
$T$
be a topological generator of
![]() $\widehat{\mathbf{Z}}$
, written multiplicatively. Then for
$\widehat{\mathbf{Z}}$
, written multiplicatively. Then for
![]() $\unicode[STIX]{x1D70E}\in G_{K}$
we have
$\unicode[STIX]{x1D70E}\in G_{K}$
we have
![]() $\unicode[STIX]{x1D6FC}(T)=\unicode[STIX]{x1D6FC}(\unicode[STIX]{x1D70E}T\unicode[STIX]{x1D70E}^{-1})=\unicode[STIX]{x1D6FC}(T^{\unicode[STIX]{x1D712}(\unicode[STIX]{x1D70E})})=\unicode[STIX]{x1D6FC}(T)^{\unicode[STIX]{x1D712}(\unicode[STIX]{x1D70E})}$
. It follows that
$\unicode[STIX]{x1D6FC}(T)=\unicode[STIX]{x1D6FC}(\unicode[STIX]{x1D70E}T\unicode[STIX]{x1D70E}^{-1})=\unicode[STIX]{x1D6FC}(T^{\unicode[STIX]{x1D712}(\unicode[STIX]{x1D70E})})=\unicode[STIX]{x1D6FC}(T)^{\unicode[STIX]{x1D712}(\unicode[STIX]{x1D70E})}$
. It follows that
![]() $\unicode[STIX]{x1D6FC}(T)$
has finite order.◻
$\unicode[STIX]{x1D6FC}(T)$
has finite order.◻
2.4 Some lemmas from representation theory
Lemma 11. Let
![]() $\unicode[STIX]{x1D70C}$
and
$\unicode[STIX]{x1D70C}$
and
![]() $\unicode[STIX]{x1D70C}^{\prime }$
be finite-dimensional representations of a group
$\unicode[STIX]{x1D70C}^{\prime }$
be finite-dimensional representations of a group
![]() $G$
with equal determinant. Suppose there exists a normal subgroup
$G$
with equal determinant. Suppose there exists a normal subgroup
![]() $H$
of
$H$
of
![]() $G$
such that
$G$
such that
![]() $\unicode[STIX]{x1D70C}|_{H}$
and
$\unicode[STIX]{x1D70C}|_{H}$
and
![]() $\unicode[STIX]{x1D70C}^{\prime }|_{H}$
are absolutely irreducible and isomorphic. Then there exists a finite-index subgroup
$\unicode[STIX]{x1D70C}^{\prime }|_{H}$
are absolutely irreducible and isomorphic. Then there exists a finite-index subgroup
![]() $G^{\prime }$
of
$G^{\prime }$
of
![]() $G$
such that
$G$
such that
![]() $\unicode[STIX]{x1D70C}|_{G^{\prime }}$
and
$\unicode[STIX]{x1D70C}|_{G^{\prime }}$
and
![]() $\unicode[STIX]{x1D70C}^{\prime }|_{G^{\prime }}$
are isomorphic.
$\unicode[STIX]{x1D70C}^{\prime }|_{G^{\prime }}$
are isomorphic.
Proof. Let
![]() $V$
and
$V$
and
![]() $V^{\prime }$
be the spaces for
$V^{\prime }$
be the spaces for
![]() $\unicode[STIX]{x1D70C}$
and
$\unicode[STIX]{x1D70C}$
and
![]() $\unicode[STIX]{x1D70C}^{\prime }$
and let
$\unicode[STIX]{x1D70C}^{\prime }$
and let
![]() $f:V\rightarrow V^{\prime }$
be an isomorphism of
$f:V\rightarrow V^{\prime }$
be an isomorphism of
![]() $H$
representations. One easily verifies that for
$H$
representations. One easily verifies that for
![]() $g\in G$
the endomorphism
$g\in G$
the endomorphism
![]() $g^{-1}f^{-1}gf$
of
$g^{-1}f^{-1}gf$
of
![]() $V$
commutes with
$V$
commutes with
![]() $H$
, and is therefore given by multiplication by some scalar
$H$
, and is therefore given by multiplication by some scalar
![]() $\unicode[STIX]{x1D712}(g)$
. Thus we have
$\unicode[STIX]{x1D712}(g)$
. Thus we have
![]() $f^{-1}gf=\unicode[STIX]{x1D712}(g)g$
for all
$f^{-1}gf=\unicode[STIX]{x1D712}(g)g$
for all
![]() $g\in G$
. It follows easily from this that
$g\in G$
. It follows easily from this that
![]() $\unicode[STIX]{x1D712}$
is a homomorphism, i.e.,
$\unicode[STIX]{x1D712}$
is a homomorphism, i.e.,
![]() $\unicode[STIX]{x1D712}(gg^{\prime })=\unicode[STIX]{x1D712}(g)\unicode[STIX]{x1D712}(g^{\prime })$
. We thus see that
$\unicode[STIX]{x1D712}(gg^{\prime })=\unicode[STIX]{x1D712}(g)\unicode[STIX]{x1D712}(g^{\prime })$
. We thus see that
![]() $\unicode[STIX]{x1D712}\otimes \unicode[STIX]{x1D70C}$
and
$\unicode[STIX]{x1D712}\otimes \unicode[STIX]{x1D70C}$
and
![]() $\unicode[STIX]{x1D70C}^{\prime }$
are isomorphic as representations of
$\unicode[STIX]{x1D70C}^{\prime }$
are isomorphic as representations of
![]() $G$
. Taking determinants, we see that
$G$
. Taking determinants, we see that
![]() $\unicode[STIX]{x1D712}^{n}$
is trivial, where
$\unicode[STIX]{x1D712}^{n}$
is trivial, where
![]() $n$
is the dimension of
$n$
is the dimension of
![]() $\unicode[STIX]{x1D70C}$
. The result follows by taking
$\unicode[STIX]{x1D70C}$
. The result follows by taking
![]() $G^{\prime }$
to be the kernel of
$G^{\prime }$
to be the kernel of
![]() $\unicode[STIX]{x1D712}$
; this has finite index since the image of
$\unicode[STIX]{x1D712}$
; this has finite index since the image of
![]() $\unicode[STIX]{x1D712}$
is contained in the group of
$\unicode[STIX]{x1D712}$
is contained in the group of
![]() $n$
th roots of unity of
$n$
th roots of unity of
![]() $k$
. (In fact, the index of
$k$
. (In fact, the index of
![]() $G^{\prime }$
divides
$G^{\prime }$
divides
![]() $n$
.)◻
$n$
.)◻
Lemma 12. Let
![]() $G$
be a group and
$G$
be a group and
![]() $H$
a normal subgroup of
$H$
a normal subgroup of
![]() $G$
. Consider a two-dimensional irreducible representation
$G$
. Consider a two-dimensional irreducible representation
![]() $\unicode[STIX]{x1D70C}:G\rightarrow \mathbf{GL}_{2}(\overline{\mathbf{Q}}_{\ell })$
. Assume that for some element
$\unicode[STIX]{x1D70C}:G\rightarrow \mathbf{GL}_{2}(\overline{\mathbf{Q}}_{\ell })$
. Assume that for some element
![]() $h\in H$
, the matrix
$h\in H$
, the matrix
![]() $\unicode[STIX]{x1D70C}(h)$
is a non-trivial unipotent element. Then
$\unicode[STIX]{x1D70C}(h)$
is a non-trivial unipotent element. Then
![]() $\unicode[STIX]{x1D70C}|_{H}$
is irreducible.
$\unicode[STIX]{x1D70C}|_{H}$
is irreducible.
Proof. Suppose not. Then by the existence of
![]() $h$
, there exists a unique one-dimensional subspace
$h$
, there exists a unique one-dimensional subspace
![]() $V$
invariant under
$V$
invariant under
![]() $H$
. But since
$H$
. But since
![]() $H$
is normal in
$H$
is normal in
![]() $G$
, it follows that
$G$
, it follows that
![]() $V$
is invariant under
$V$
is invariant under
![]() $G$
as well. This contradicts the supposition.◻
$G$
as well. This contradicts the supposition.◻
3 Descent results for abelian varieties
For this section, fix a finitely generated field
![]() $K$
. We consider the following condition on a continuous representation
$K$
. We consider the following condition on a continuous representation
![]() $\unicode[STIX]{x1D70C}:G_{K}\rightarrow \mathbf{GL}_{n}(\overline{\mathbf{Q}}_{\ell })$
.
$\unicode[STIX]{x1D70C}:G_{K}\rightarrow \mathbf{GL}_{n}(\overline{\mathbf{Q}}_{\ell })$
.
(∗) There exists an integral scheme
 $X$
of finite type over
$X$
of finite type over
 $\operatorname{Spec}(\mathbf{Z})$
with function field
$\operatorname{Spec}(\mathbf{Z})$
with function field
 $K$
and a lisse
$K$
and a lisse
 $\overline{\mathbf{Q}}_{\ell }$
-sheaf
$\overline{\mathbf{Q}}_{\ell }$
-sheaf
 $L$
on
$L$
on
 $X$
with generic fiber
$X$
with generic fiber
 $\unicode[STIX]{x1D70C}$
such that at every closed point
$\unicode[STIX]{x1D70C}$
such that at every closed point
 $x$
of
$x$
of
 $X$
the trace of the Frobenius element on
$X$
the trace of the Frobenius element on
 $L_{x}$
is rational.
$L_{x}$
is rational.
In our proof of Theorem 1, we will show that
![]() $\unicode[STIX]{x1D70C}$
comes from an elliptic curve over some finite extension of
$\unicode[STIX]{x1D70C}$
comes from an elliptic curve over some finite extension of
![]() $K^{\prime }$
. We use the following result to conclude that we actually get an elliptic curve over
$K^{\prime }$
. We use the following result to conclude that we actually get an elliptic curve over
![]() $K$
.
$K$
.
Proposition 13. Let
![]() $\unicode[STIX]{x1D70C}:G_{K}\rightarrow \mathbf{GL}_{2}(\overline{\mathbf{Q}}_{\ell })$
be a Galois representation satisfying condition (
$\unicode[STIX]{x1D70C}:G_{K}\rightarrow \mathbf{GL}_{2}(\overline{\mathbf{Q}}_{\ell })$
be a Galois representation satisfying condition (
![]() $\ast$
). Suppose that there exists a finite extension
$\ast$
). Suppose that there exists a finite extension
![]() $K^{\prime }/K$
such that
$K^{\prime }/K$
such that
![]() $\unicode[STIX]{x1D70C}|_{K^{\prime }}$
comes from a non-CM elliptic curve
$\unicode[STIX]{x1D70C}|_{K^{\prime }}$
comes from a non-CM elliptic curve
![]() $E$
. Then
$E$
. Then
![]() $\unicode[STIX]{x1D70C}$
comes from an elliptic curve.Footnote
1
$\unicode[STIX]{x1D70C}$
comes from an elliptic curve.Footnote
1
We proceed with a number of lemmas.
Lemma 14. Let
![]() $\unicode[STIX]{x1D70C}:G\rightarrow \mathbf{GL}_{n}(\overline{\mathbf{Q}}_{\ell })$
a continuous representation of the profinite group
$\unicode[STIX]{x1D70C}:G\rightarrow \mathbf{GL}_{n}(\overline{\mathbf{Q}}_{\ell })$
a continuous representation of the profinite group
![]() $G$
. Suppose there exists an open subgroup
$G$
. Suppose there exists an open subgroup
![]() $H$
of
$H$
of
![]() $G$
such that
$G$
such that
![]() $\unicode[STIX]{x1D70C}(H)$
is contained in
$\unicode[STIX]{x1D70C}(H)$
is contained in
![]() $\mathbf{GL}_{n}(\mathbf{Z}_{\ell })$
and contains an open subgroup of
$\mathbf{GL}_{n}(\mathbf{Z}_{\ell })$
and contains an open subgroup of
![]() $\mathbf{GL}_{n}(\mathbf{Z}_{\ell })$
. Suppose also that there is a dense set of elements
$\mathbf{GL}_{n}(\mathbf{Z}_{\ell })$
. Suppose also that there is a dense set of elements
![]() $\{g_{i}\}_{i\in I}$
of
$\{g_{i}\}_{i\in I}$
of
![]() $G$
such that
$G$
such that
![]() $\operatorname{tr}\unicode[STIX]{x1D70C}(g_{i})\in \mathbf{Q}_{\ell }$
for all
$\operatorname{tr}\unicode[STIX]{x1D70C}(g_{i})\in \mathbf{Q}_{\ell }$
for all
![]() $i\in I$
. Then
$i\in I$
. Then
![]() $\unicode[STIX]{x1D70C}(G)$
is contained in
$\unicode[STIX]{x1D70C}(G)$
is contained in
![]() $\mathbf{GL}_{n}(\mathbf{Q}_{\ell })$
.
$\mathbf{GL}_{n}(\mathbf{Q}_{\ell })$
.
Proof. Let
![]() $e_{ij}$
be the
$e_{ij}$
be the
![]() $n\times n$
matrix with a 1 in the
$n\times n$
matrix with a 1 in the
![]() $(i,j)$
position and 0 elsewhere. By assumption, there exists
$(i,j)$
position and 0 elsewhere. By assumption, there exists
![]() $m$
such that
$m$
such that
![]() $\unicode[STIX]{x1D70C}(H)$
contains
$\unicode[STIX]{x1D70C}(H)$
contains
![]() $1+\ell ^{m}e_{ij}$
for all
$1+\ell ^{m}e_{ij}$
for all
![]() $i,j$
, and so
$i,j$
, and so
![]() $e_{ij}\in \mathbf{Q}_{\ell }[\unicode[STIX]{x1D70C}(G)]$
. For any
$e_{ij}\in \mathbf{Q}_{\ell }[\unicode[STIX]{x1D70C}(G)]$
. For any
![]() $A\in \unicode[STIX]{x1D70C}(G)$
we have
$A\in \unicode[STIX]{x1D70C}(G)$
we have
![]() $A_{i,j}=\operatorname{tr}(e_{ii}Ae_{jj})\in \operatorname{tr}(\mathbf{Q}_{\ell }[\unicode[STIX]{x1D70C}(G)])=\mathbf{Q}_{\ell }$
, where the last equality follows from the fact that
$A_{i,j}=\operatorname{tr}(e_{ii}Ae_{jj})\in \operatorname{tr}(\mathbf{Q}_{\ell }[\unicode[STIX]{x1D70C}(G)])=\mathbf{Q}_{\ell }$
, where the last equality follows from the fact that
![]() $\text{tr}\circ \unicode[STIX]{x1D70C}:G\rightarrow \mathbf{Q}_{\ell }$
is continuous and
$\text{tr}\circ \unicode[STIX]{x1D70C}:G\rightarrow \mathbf{Q}_{\ell }$
is continuous and
![]() $\operatorname{tr}(\unicode[STIX]{x1D70C}(g_{i}))\in \mathbf{Q}_{\ell }$
for all
$\operatorname{tr}(\unicode[STIX]{x1D70C}(g_{i}))\in \mathbf{Q}_{\ell }$
for all
![]() $i$
. It thus follows that
$i$
. It thus follows that
![]() $\unicode[STIX]{x1D70C}(G)\subset \mathbf{GL}_{n}(\mathbf{Q}_{\ell })$
as claimed.◻
$\unicode[STIX]{x1D70C}(G)\subset \mathbf{GL}_{n}(\mathbf{Q}_{\ell })$
as claimed.◻
The following lemma is basically [Reference TaylorTay02, Corollary 2.4].
Lemma 15. Let
![]() $\unicode[STIX]{x1D70C}:G_{K}\rightarrow \mathbf{GL}_{2}(\overline{\mathbf{Q}}_{\ell })$
be a continuous irreducible Galois representation. Suppose that there is a finite separable extension
$\unicode[STIX]{x1D70C}:G_{K}\rightarrow \mathbf{GL}_{2}(\overline{\mathbf{Q}}_{\ell })$
be a continuous irreducible Galois representation. Suppose that there is a finite separable extension
![]() $K^{\prime }/K$
such that
$K^{\prime }/K$
such that
![]() $\unicode[STIX]{x1D70C}|_{G_{K^{\prime }}}$
comes from a non-CM elliptic curve
$\unicode[STIX]{x1D70C}|_{G_{K^{\prime }}}$
comes from a non-CM elliptic curve
![]() $E$
. Then there is an abelian variety
$E$
. Then there is an abelian variety
![]() $A/K$
with
$A/K$
with
![]() $F=\operatorname{End}(A)\otimes \mathbf{Q}$
a number field of degree
$F=\operatorname{End}(A)\otimes \mathbf{Q}$
a number field of degree
![]() $\dim (A)$
such that
$\dim (A)$
such that
![]() $\unicode[STIX]{x1D70C}\cong \overline{\mathbf{Q}}_{\ell }\otimes _{F_{w}}V_{w}(A)$
for some place
$\unicode[STIX]{x1D70C}\cong \overline{\mathbf{Q}}_{\ell }\otimes _{F_{w}}V_{w}(A)$
for some place
![]() $w$
of
$w$
of
![]() $A$
.
$A$
.
Proof. Consider
![]() $B=\operatorname{Res}_{K}^{K^{\prime }}E$
, an abelian variety over
$B=\operatorname{Res}_{K}^{K^{\prime }}E$
, an abelian variety over
![]() $K$
of dimension
$K$
of dimension
![]() $[K^{\prime }:K]$
[Reference Bosch, Lütkebohmert and RaynaudBLR90, § 7.6]. Write
$[K^{\prime }:K]$
[Reference Bosch, Lütkebohmert and RaynaudBLR90, § 7.6]. Write
![]() $B=\prod _{i=1}^{r}B_{i}$
(up to isogeny) where each
$B=\prod _{i=1}^{r}B_{i}$
(up to isogeny) where each
![]() $B_{i}$
is a power of a simple abelian variety. Thus
$B_{i}$
is a power of a simple abelian variety. Thus
where
![]() $D_{i}=\operatorname{End}_{K}(B_{i})\otimes \mathbf{Q}$
is a simple algebra. We have
$D_{i}=\operatorname{End}_{K}(B_{i})\otimes \mathbf{Q}$
is a simple algebra. We have
where the latter follows from Faltings’ proof of the Tate conjecture [Reference Faltings, Wüstholz, Grunewald, Schappacher and StuhlerFWGSS92, Theorem 1, p. 211] since
![]() $E$
is non-CM. We thus see that
$E$
is non-CM. We thus see that
![]() $\unicode[STIX]{x1D70C}$
occurs uniquely in
$\unicode[STIX]{x1D70C}$
occurs uniquely in
![]() $\overline{\mathbf{Q}}_{\ell }\otimes V_{\ell }(B_{i})$
for some
$\overline{\mathbf{Q}}_{\ell }\otimes V_{\ell }(B_{i})$
for some
![]() $i$
. Let
$i$
. Let
![]() $A$
be this
$A$
be this
![]() $B_{i}$
. Since
$B_{i}$
. Since
![]() $A$
is a power of a simple abelian variety and
$A$
is a power of a simple abelian variety and
![]() $\unicode[STIX]{x1D70C}$
occurs uniquely in its Tate module,
$\unicode[STIX]{x1D70C}$
occurs uniquely in its Tate module,
![]() $A$
must itself be simple. The endomorphism ring
$A$
must itself be simple. The endomorphism ring
![]() $D_{i}$
must preserve
$D_{i}$
must preserve
![]() $\unicode[STIX]{x1D70C}$
, and thus the action of
$\unicode[STIX]{x1D70C}$
, and thus the action of
![]() $\unicode[STIX]{x1D70C}$
comes from an algebra homomorphism
$\unicode[STIX]{x1D70C}$
comes from an algebra homomorphism
![]() $\unicode[STIX]{x1D713}:D_{i}\rightarrow \overline{\mathbf{Q}}_{\ell }$
, which is injective since
$\unicode[STIX]{x1D713}:D_{i}\rightarrow \overline{\mathbf{Q}}_{\ell }$
, which is injective since
![]() $D_{i}$
is simple. We thus see that
$D_{i}$
is simple. We thus see that
![]() $D_{i}=F$
is a number field, and
$D_{i}=F$
is a number field, and
![]() $\unicode[STIX]{x1D713}$
corresponds to a place
$\unicode[STIX]{x1D713}$
corresponds to a place
![]() $w$
of
$w$
of
![]() $F$
above
$F$
above
![]() $\ell$
. Since
$\ell$
. Since
![]() $V_{w}(A)\otimes _{F_{w}}\overline{\mathbf{Q}}_{\ell }$
contains
$V_{w}(A)\otimes _{F_{w}}\overline{\mathbf{Q}}_{\ell }$
contains
![]() $\unicode[STIX]{x1D70C}$
and is absolutely irreducible by Proposition 7, it is equal to
$\unicode[STIX]{x1D70C}$
and is absolutely irreducible by Proposition 7, it is equal to
![]() $\unicode[STIX]{x1D70C}$
. Therefore
$\unicode[STIX]{x1D70C}$
. Therefore
![]() $V_{w}(A)$
is two dimensional over
$V_{w}(A)$
is two dimensional over
![]() $F_{w}$
, and so
$F_{w}$
, and so
![]() $[F:\mathbf{Q}]=\dim (A)$
by Proposition 6.◻
$[F:\mathbf{Q}]=\dim (A)$
by Proposition 6.◻
Lemma 16. Proposition 13 holds if
![]() $K^{\prime }/K$
is separable.
$K^{\prime }/K$
is separable.
Proof. By Lemma 15, we can find an abelian variety
![]() $A/K$
with
$A/K$
with
![]() $\operatorname{End}(A)\otimes \mathbf{Q}=F$
a number field of degree
$\operatorname{End}(A)\otimes \mathbf{Q}=F$
a number field of degree
![]() $\dim (A)$
, and a place
$\dim (A)$
, and a place
![]() $w_{0}$
of
$w_{0}$
of
![]() $F$
such that
$F$
such that
![]() $\unicode[STIX]{x1D70C}\cong \overline{\mathbf{Q}}_{\ell }\otimes _{F_{w_{0}}}V_{w_{0}}(A)$
.
$\unicode[STIX]{x1D70C}\cong \overline{\mathbf{Q}}_{\ell }\otimes _{F_{w_{0}}}V_{w_{0}}(A)$
.
Choose an integral scheme
![]() $X$
of finite type over
$X$
of finite type over
![]() $\operatorname{Spec}(\mathbf{Z})$
with fraction field
$\operatorname{Spec}(\mathbf{Z})$
with fraction field
![]() $K$
and a family of abelian varieties
$K$
and a family of abelian varieties
![]() ${\mathcal{A}}\rightarrow X$
extending
${\mathcal{A}}\rightarrow X$
extending
![]() $A$
. The representation of
$A$
. The representation of
![]() $G_{K}$
on
$G_{K}$
on
![]() $V_{w}(A)$
factors through
$V_{w}(A)$
factors through
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
, for all
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
, for all
![]() $w$
. Since
$w$
. Since
![]() $V_{w_{0}}(A)$
satisfies (
$V_{w_{0}}(A)$
satisfies (
![]() $\ast$
), we can replace
$\ast$
), we can replace
![]() $X$
with a dense open subscheme such that the Frobenius elements in
$X$
with a dense open subscheme such that the Frobenius elements in
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
have rational traces on
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
have rational traces on
![]() $V_{w_{0}}(A)$
. By Proposition 6, it follows that the Frobenius elements in
$V_{w_{0}}(A)$
. By Proposition 6, it follows that the Frobenius elements in
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
have rational traces on
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
have rational traces on
![]() $V_{w}(A)$
for all
$V_{w}(A)$
for all
![]() $w$
.
$w$
.
By assumption,
![]() $\unicode[STIX]{x1D70C}|_{G_{K^{\prime }}}\cong \overline{\mathbf{Q}}_{\ell }\,\otimes \,V_{\ell }(E)$
for some non-CM elliptic curve
$\unicode[STIX]{x1D70C}|_{G_{K^{\prime }}}\cong \overline{\mathbf{Q}}_{\ell }\,\otimes \,V_{\ell }(E)$
for some non-CM elliptic curve
![]() $E/K^{\prime }$
. As above, pick an integral scheme
$E/K^{\prime }$
. As above, pick an integral scheme
![]() $X^{\prime }$
of finite type over
$X^{\prime }$
of finite type over
![]() $\operatorname{Spec}(\mathbf{Z})$
with fraction field
$\operatorname{Spec}(\mathbf{Z})$
with fraction field
![]() $K^{\prime }$
such that there is an elliptic curve
$K^{\prime }$
such that there is an elliptic curve
![]() ${\mathcal{E}}\rightarrow X^{\prime }$
extending
${\mathcal{E}}\rightarrow X^{\prime }$
extending
![]() $E$
. Further shrinking
$E$
. Further shrinking
![]() $X,X^{\prime }$
we can assume
$X,X^{\prime }$
we can assume
![]() $X^{\prime }$
maps to
$X^{\prime }$
maps to
![]() $X$
inducing the inclusion
$X$
inducing the inclusion
![]() $K\subset K^{\prime }$
.
$K\subset K^{\prime }$
.
Pick a place
![]() $w\mid p$
of
$w\mid p$
of
![]() $F$
. As we saw above, Frobenius elements of
$F$
. As we saw above, Frobenius elements of
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
have equal traces on
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X)$
have equal traces on
![]() $\unicode[STIX]{x1D70C}\cong \overline{\mathbf{Q}}_{\ell }\otimes _{F_{w_{0}}}V_{w_{0}}(A)$
and
$\unicode[STIX]{x1D70C}\cong \overline{\mathbf{Q}}_{\ell }\otimes _{F_{w_{0}}}V_{w_{0}}(A)$
and
![]() $\overline{\mathbf{Q}}_{p}\otimes _{F_{w}}V_{w}(A)$
. Similarly, the traces of Frobenius elements of
$\overline{\mathbf{Q}}_{p}\otimes _{F_{w}}V_{w}(A)$
. Similarly, the traces of Frobenius elements of
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X^{\prime })$
on
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X^{\prime })$
on
![]() $V_{\ell }(E)$
and
$V_{\ell }(E)$
and
![]() $V_{p}(E)$
are equal. We thus see by Corollary 9 that
$V_{p}(E)$
are equal. We thus see by Corollary 9 that
![]() $\overline{\mathbf{Q}}_{p}\otimes _{F_{w}}V_{w}(A)$
is isomorphic to
$\overline{\mathbf{Q}}_{p}\otimes _{F_{w}}V_{w}(A)$
is isomorphic to
![]() $\overline{\mathbf{Q}}_{p}\otimes _{\mathbf{Q}_{p}}V_{p}(E)$
as representations of
$\overline{\mathbf{Q}}_{p}\otimes _{\mathbf{Q}_{p}}V_{p}(E)$
as representations of
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X^{\prime })$
. By the Tate conjecture proved by Faltings [Reference Faltings, Wüstholz, Grunewald, Schappacher and StuhlerFWGSS92, Theorem 1, p. 211], the image of
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(X^{\prime })$
. By the Tate conjecture proved by Faltings [Reference Faltings, Wüstholz, Grunewald, Schappacher and StuhlerFWGSS92, Theorem 1, p. 211], the image of
![]() $G_{K^{\prime }}$
in
$G_{K^{\prime }}$
in
![]() $\mathbf{GL}(V_{p}(E))$
contains an open subgroup of
$\mathbf{GL}(V_{p}(E))$
contains an open subgroup of
![]() $\mathbf{GL}_{2}(\mathbf{Z}_{p})$
.
$\mathbf{GL}_{2}(\mathbf{Z}_{p})$
.
It follows that the conditions of Lemma 14 are fulfilled for
![]() $V_{w}(A)\,\otimes _{F_{w}}\,\overline{\mathbf{Q}}_{p}$
, and so
$V_{w}(A)\,\otimes _{F_{w}}\,\overline{\mathbf{Q}}_{p}$
, and so
![]() $V_{w}(A)\,\otimes _{F_{w}}\,\overline{\mathbf{Q}}_{p}$
is defined over
$V_{w}(A)\,\otimes _{F_{w}}\,\overline{\mathbf{Q}}_{p}$
is defined over
![]() $\mathbf{Q}_{p}$
; that is, there exists some representation
$\mathbf{Q}_{p}$
; that is, there exists some representation
![]() $V$
of
$V$
of
![]() $G_{K}$
over
$G_{K}$
over
![]() $\mathbf{Q}_{p}$
such that
$\mathbf{Q}_{p}$
such that
![]() $V_{w}(A)\,\otimes _{F_{w}}\,\overline{\mathbf{Q}}_{p}\cong \overline{\mathbf{Q}}_{p}\otimes _{\mathbf{Q}_{p}}V$
. Since
$V_{w}(A)\,\otimes _{F_{w}}\,\overline{\mathbf{Q}}_{p}\cong \overline{\mathbf{Q}}_{p}\otimes _{\mathbf{Q}_{p}}V$
. Since
![]() $V_{w}(A)$
and
$V_{w}(A)$
and
![]() $V\otimes _{\mathbf{Q}_{p}}F_{w}$
have the same character, and are irreducible, it follows that they are isomorphic. We thus see that
$V\otimes _{\mathbf{Q}_{p}}F_{w}$
have the same character, and are irreducible, it follows that they are isomorphic. We thus see that
![]() $\operatorname{End}_{\mathbf{Q}_{p}[G_{K}]}(V_{w}(A))\cong \operatorname{End}_{\mathbf{Q}_{p}}(F_{w})$
, where on the right side we are taking endomorphisms of
$\operatorname{End}_{\mathbf{Q}_{p}[G_{K}]}(V_{w}(A))\cong \operatorname{End}_{\mathbf{Q}_{p}}(F_{w})$
, where on the right side we are taking endomorphisms of
![]() $F_{w}$
as a vector space. By Proposition 7 we have that
$F_{w}$
as a vector space. By Proposition 7 we have that
![]() $\operatorname{End}_{\mathbf{Q}_{p}[G_{K}]}(V_{w}(A))\cong F_{w}$
, and so
$\operatorname{End}_{\mathbf{Q}_{p}[G_{K}]}(V_{w}(A))\cong F_{w}$
, and so
![]() $\operatorname{End}_{\mathbf{Q}_{p}}(F_{w})=F_{w}$
, which implies
$\operatorname{End}_{\mathbf{Q}_{p}}(F_{w})=F_{w}$
, which implies
![]() $F_{w}=\mathbf{Q}_{p}$
. We thus see that all places of
$F_{w}=\mathbf{Q}_{p}$
. We thus see that all places of
![]() $F$
are split, and so
$F$
are split, and so
![]() $F=\mathbf{Q}$
. Thus
$F=\mathbf{Q}$
. Thus
![]() $A$
is actually an elliptic curve, and the proof is complete.◻
$A$
is actually an elliptic curve, and the proof is complete.◻
Lemma 17. Proposition 13 holds if
![]() $K^{\prime }/K$
is purely inseparable.
$K^{\prime }/K$
is purely inseparable.
Proof. It suffices to treat the case where
![]() $(K^{\prime })^{p}=K$
. Let
$(K^{\prime })^{p}=K$
. Let
![]() $E/K^{\prime }$
be the elliptic curve giving rise to
$E/K^{\prime }$
be the elliptic curve giving rise to
![]() $\unicode[STIX]{x1D70C}|_{K^{\prime }}$
. Let
$\unicode[STIX]{x1D70C}|_{K^{\prime }}$
. Let
![]() $E^{(p)}=E\times _{K^{\prime },F_{0}}K^{\prime }$
where
$E^{(p)}=E\times _{K^{\prime },F_{0}}K^{\prime }$
where
![]() $F_{0}:K^{\prime }\rightarrow K^{\prime }$
is the absolute Frobenius map. Then
$F_{0}:K^{\prime }\rightarrow K^{\prime }$
is the absolute Frobenius map. Then
![]() $E^{(p)}$
is defined over
$E^{(p)}$
is defined over
![]() $K$
, and there is a canonical isogeny (relative Frobenius map)
$K$
, and there is a canonical isogeny (relative Frobenius map)
![]() $F:E\rightarrow E^{(p)}$
defined over
$F:E\rightarrow E^{(p)}$
defined over
![]() $K^{\prime }$
inducing an isomorphism on rational
$K^{\prime }$
inducing an isomorphism on rational
![]() $\ell$
-adic Tate modules. Thus
$\ell$
-adic Tate modules. Thus
![]() $V_{\ell }(E^{(p)})|_{G_{K^{\prime }}}\cong \unicode[STIX]{x1D70C}|_{G_{K^{\prime }}}$
and so
$V_{\ell }(E^{(p)})|_{G_{K^{\prime }}}\cong \unicode[STIX]{x1D70C}|_{G_{K^{\prime }}}$
and so
![]() $V_{\ell }(E^{(p)})\cong \unicode[STIX]{x1D70C}$
since
$V_{\ell }(E^{(p)})\cong \unicode[STIX]{x1D70C}$
since
![]() $K^{\prime }/K$
is purely inseparable.◻
$K^{\prime }/K$
is purely inseparable.◻
Proposition 13 in general follows from the previous two lemmas. We now prove a slightly different descent result. Recall that for an elliptic curve
![]() $E$
and a number field
$E$
and a number field
![]() $F$
, one has an abelian variety
$F$
, one has an abelian variety
![]() $E\otimes {\mathcal{O}}_{F}$
with multiplication by
$E\otimes {\mathcal{O}}_{F}$
with multiplication by
![]() ${\mathcal{O}}_{F}$
: as an abelian variety,
${\mathcal{O}}_{F}$
: as an abelian variety,
![]() $E\otimes {\mathcal{O}}_{F}$
is simply
$E\otimes {\mathcal{O}}_{F}$
is simply
![]() $E^{n}$
where
$E^{n}$
where
![]() $n=[F:\mathbf{Q}]$
.
$n=[F:\mathbf{Q}]$
.
Proposition 18. Let
![]() $k$
be a finite field, let
$k$
be a finite field, let
![]() $C/k$
be a proper smooth geometrically irreducible curve, and let
$C/k$
be a proper smooth geometrically irreducible curve, and let
![]() $f:A\rightarrow U$
be a family of
$f:A\rightarrow U$
be a family of
![]() $g$
-dimensional abelian varieties over a non-empty open subset
$g$
-dimensional abelian varieties over a non-empty open subset
![]() $U$
of
$U$
of
![]() $C$
such that
$C$
such that
![]() $\operatorname{End}(A)\otimes \mathbf{Q}$
contains a number field
$\operatorname{End}(A)\otimes \mathbf{Q}$
contains a number field
![]() $F$
of degree
$F$
of degree
![]() $g$
. For a finite place
$g$
. For a finite place
![]() $v$
of
$v$
of
![]() $F$
, let
$F$
, let
![]() ${\mathcal{L}}_{v}$
be the
${\mathcal{L}}_{v}$
be the
![]() $v$
-adic Tate module of
$v$
-adic Tate module of
![]() $A$
. Assume that for all closed points
$A$
. Assume that for all closed points
![]() $x$
of
$x$
of
![]() $U$
, the trace of the Frobenius element at
$U$
, the trace of the Frobenius element at
![]() $x$
on
$x$
on
![]() ${\mathcal{L}}_{v,x}$
belongs to
${\mathcal{L}}_{v,x}$
belongs to
![]() $\mathbf{Q}$
. Also assume that there is some place of
$\mathbf{Q}$
. Also assume that there is some place of
![]() $C$
where
$C$
where
![]() $A$
does not have potentially good reduction. Then there exists an elliptic curve
$A$
does not have potentially good reduction. Then there exists an elliptic curve
![]() $E\rightarrow U$
such that
$E\rightarrow U$
such that
![]() $A$
is isogenous to
$A$
is isogenous to
![]() $E\otimes {\mathcal{O}}_{F}$
.
$E\otimes {\mathcal{O}}_{F}$
.
Proof. Let
![]() $\ell$
be a rational prime that splits completely in
$\ell$
be a rational prime that splits completely in
![]() $F$
. Then the
$F$
. Then the
![]() $\ell$
-adic Tate module
$\ell$
-adic Tate module
![]() ${\mathcal{L}}_{\ell }$
of
${\mathcal{L}}_{\ell }$
of
![]() $A$
decomposes as
$A$
decomposes as
![]() $\bigoplus _{v\mid \ell }{\mathcal{L}}_{v}$
. The
$\bigoplus _{v\mid \ell }{\mathcal{L}}_{v}$
. The
![]() ${\mathcal{L}}_{v}$
form a compatible system with coefficients in
${\mathcal{L}}_{v}$
form a compatible system with coefficients in
![]() $F$
, so for each closed point
$F$
, so for each closed point
![]() $x\in U$
there exists
$x\in U$
there exists
![]() $\unicode[STIX]{x1D6FC}\in F$
such that the trace of the Frobenius element at
$\unicode[STIX]{x1D6FC}\in F$
such that the trace of the Frobenius element at
![]() $x$
on
$x$
on
![]() ${\mathcal{L}}_{v,x}$
is the image of
${\mathcal{L}}_{v,x}$
is the image of
![]() $\unicode[STIX]{x1D6FC}$
in
$\unicode[STIX]{x1D6FC}$
in
![]() $F_{v}$
. By our assumptions,
$F_{v}$
. By our assumptions,
![]() $\unicode[STIX]{x1D6FC}$
is a rational number, and so if
$\unicode[STIX]{x1D6FC}$
is a rational number, and so if
![]() $v,w\mid \ell$
then the traces of Frobenius elements on
$v,w\mid \ell$
then the traces of Frobenius elements on
![]() ${\mathcal{L}}_{v,x}$
and
${\mathcal{L}}_{v,x}$
and
![]() ${\mathcal{L}}_{w,x}$
are the same element of
${\mathcal{L}}_{w,x}$
are the same element of
![]() $\mathbf{Q}_{\ell }\subset F_{v},F_{w}$
. It follows that the characters of
$\mathbf{Q}_{\ell }\subset F_{v},F_{w}$
. It follows that the characters of
![]() ${\mathcal{L}}_{v}$
and
${\mathcal{L}}_{v}$
and
![]() ${\mathcal{L}}_{w}$
are equal at Frobenius elements, and so
${\mathcal{L}}_{w}$
are equal at Frobenius elements, and so
![]() ${\mathcal{L}}_{v}\cong {\mathcal{L}}_{w}$
. Thus
${\mathcal{L}}_{v}\cong {\mathcal{L}}_{w}$
. Thus
![]() $\dim (\operatorname{End}({\mathcal{L}}_{\ell }))\geqslant g^{2}$
. By Faltings’ isogeny theorem, it follows that
$\dim (\operatorname{End}({\mathcal{L}}_{\ell }))\geqslant g^{2}$
. By Faltings’ isogeny theorem, it follows that
![]() $\dim (\operatorname{End}(A)\otimes \mathbf{Q})\geqslant g^{2}$
.
$\dim (\operatorname{End}(A)\otimes \mathbf{Q})\geqslant g^{2}$
.
Let
![]() $C^{\prime }\rightarrow C$
be a cover such that
$C^{\prime }\rightarrow C$
be a cover such that
![]() $A$
has semi-stable reduction, and let
$A$
has semi-stable reduction, and let
![]() $x$
be a point at which
$x$
be a point at which
![]() $A^{\prime }$
(the pullback of
$A^{\prime }$
(the pullback of
![]() $A$
) has bad reduction. Let
$A$
) has bad reduction. Let
![]() ${\mathcal{A}}^{\prime }$
be the Néron model of
${\mathcal{A}}^{\prime }$
be the Néron model of
![]() $A^{\prime }$
over
$A^{\prime }$
over
![]() $C^{\prime }$
, and let
$C^{\prime }$
, and let
![]() $T$
be the torus quotient of the identity component of
$T$
be the torus quotient of the identity component of
![]() ${\mathcal{A}}_{x}^{\prime }$
. The dimension
${\mathcal{A}}_{x}^{\prime }$
. The dimension
![]() $h$
of
$h$
of
![]() $T$
is at least 1, and at most
$T$
is at least 1, and at most
![]() $g$
. Under the map
$g$
. Under the map
![]() $\operatorname{End}(A)\otimes \mathbf{Q}\rightarrow \operatorname{End}(T)\otimes \mathbf{Q}\subset M_{h}(\mathbf{Q})$
, the field
$\operatorname{End}(A)\otimes \mathbf{Q}\rightarrow \operatorname{End}(T)\otimes \mathbf{Q}\subset M_{h}(\mathbf{Q})$
, the field
![]() $F$
must inject, and so
$F$
must inject, and so
![]() $h=g$
. Thus
$h=g$
. Thus
![]() $T$
is the entire identity component of
$T$
is the entire identity component of
![]() ${\mathcal{A}}_{x}^{\prime }$
, and so the map
${\mathcal{A}}_{x}^{\prime }$
, and so the map
![]() $\operatorname{End}(A)\otimes \mathbf{Q}\rightarrow \operatorname{End}(T)\otimes \mathbf{Q}\subset M_{g}(\mathbf{Q})$
is injective. Combined with the previous paragraph, we find that
$\operatorname{End}(A)\otimes \mathbf{Q}\rightarrow \operatorname{End}(T)\otimes \mathbf{Q}\subset M_{g}(\mathbf{Q})$
is injective. Combined with the previous paragraph, we find that
![]() $\dim (\operatorname{End}(A)\,\otimes \,\mathbf{Q})=g^{2}$
, and so the map
$\dim (\operatorname{End}(A)\,\otimes \,\mathbf{Q})=g^{2}$
, and so the map
![]() $\operatorname{End}(A)\otimes \mathbf{Q}\rightarrow M_{g}(\mathbf{Q})$
is an isomorphism. The statement now follows by projecting under an idempotent.◻
$\operatorname{End}(A)\otimes \mathbf{Q}\rightarrow M_{g}(\mathbf{Q})$
is an isomorphism. The statement now follows by projecting under an idempotent.◻
4 Drinfeld’s work on the global Langlands program
Proposition 19. Let
![]() $k$
be a finite field, and
$k$
be a finite field, and
![]() $C/k$
be a smooth proper geometrically irreducible curve. Let
$C/k$
be a smooth proper geometrically irreducible curve. Let
![]() $L$
be an irreducible rank-two lisse
$L$
be an irreducible rank-two lisse
![]() $\overline{\mathbf{Q}}_{\ell }$
-sheaf over a non-empty open subset
$\overline{\mathbf{Q}}_{\ell }$
-sheaf over a non-empty open subset
![]() $U\subset C$
, such that the following hold.
$U\subset C$
, such that the following hold.
(a) There is an isomorphism
 $\bigwedge ^{2}L\cong \overline{\mathbf{Q}}_{\ell }(1)$
.
$\bigwedge ^{2}L\cong \overline{\mathbf{Q}}_{\ell }(1)$
.(b) For every closed point
 $x$
of
$x$
of
 $C$
, the trace of the Frobenius element on
$C$
, the trace of the Frobenius element on
 $L_{x}$
is a rational number.
$L_{x}$
is a rational number.(c) There exists a point
 $x$
of
$x$
of
 $C_{\overline{k}}$
at which
$C_{\overline{k}}$
at which
 $L_{\overline{k}}$
does not have potentially good reduction.
$L_{\overline{k}}$
does not have potentially good reduction.
Then there exists an elliptic curve
![]() $f:E\rightarrow U$
such that
$f:E\rightarrow U$
such that
![]() $\text{R}^{1}f_{\ast }(\overline{\mathbf{Q}}_{\ell })\cong L$
.
$\text{R}^{1}f_{\ast }(\overline{\mathbf{Q}}_{\ell })\cong L$
.
Proof. By Drinfeld’s theorem ([Reference DrinfedDri83, Main theorem, Remark 5], see also [Reference DrinfeldDri78]) there is a cuspidal automorphic representation
![]() $\unicode[STIX]{x1D70B}$
of
$\unicode[STIX]{x1D70B}$
of
![]() $\mathbf{GL}_{2}(\mathbf{A}_{k(C)})$
which is compatible with
$\mathbf{GL}_{2}(\mathbf{A}_{k(C)})$
which is compatible with
![]() $L$
. Since inertia at
$L$
. Since inertia at
![]() $x$
does not have finite order,
$x$
does not have finite order,
![]() $\unicode[STIX]{x1D70B}$
must be special at
$\unicode[STIX]{x1D70B}$
must be special at
![]() $x$
. It follows by another theorem of Drinfeld [Reference DrinfeldDri77, Theorem 1] that there exists a number field
$x$
. It follows by another theorem of Drinfeld [Reference DrinfeldDri77, Theorem 1] that there exists a number field
![]() $E$
and a
$E$
and a
![]() $\mathbf{GL}_{2}(E)$
-type abelian variety
$\mathbf{GL}_{2}(E)$
-type abelian variety
![]() $A$
over
$A$
over
![]() $U$
which is compatible with
$U$
which is compatible with
![]() $\unicode[STIX]{x1D70B}$
and thus also with
$\unicode[STIX]{x1D70B}$
and thus also with
![]() $L$
, in the sense that the
$L$
, in the sense that the
![]() $\ell$
-adic Tate module
$\ell$
-adic Tate module
![]() $L^{\prime }$
of
$L^{\prime }$
of
![]() $A$
is isomorphic with
$A$
is isomorphic with
![]() $L$
when tensored up to
$L$
when tensored up to
![]() $\overline{\mathbf{Q}}_{\ell }$
; that is,
$\overline{\mathbf{Q}}_{\ell }$
; that is,
![]() $L^{\prime }\otimes _{E}\overline{\mathbf{Q}}_{\ell }\cong L$
. By Proposition 18, we may take
$L^{\prime }\otimes _{E}\overline{\mathbf{Q}}_{\ell }\cong L$
. By Proposition 18, we may take
![]() $A$
to be an elliptic curve.◻
$A$
to be an elliptic curve.◻
Remark 20. By following Drinfeld’s proof carefully, one may directly see that we can take
![]() $E$
to be the field generated by the Frobenius traces of
$E$
to be the field generated by the Frobenius traces of
![]() $L$
, and is thus
$L$
, and is thus
![]() $\mathbf{Q}$
, which can replace the use of Proposition 18.
$\mathbf{Q}$
, which can replace the use of Proposition 18.
5 Mapping spaces
Let
![]() $S$
be a noetherian scheme. For
$S$
be a noetherian scheme. For
![]() $i=1,2$
, let
$i=1,2$
, let
![]() $C_{i}$
be a proper smooth scheme over
$C_{i}$
be a proper smooth scheme over
![]() $S$
with geometric fibers irreducible curves, let
$S$
with geometric fibers irreducible curves, let
![]() $Z_{i}$
be a closed subscheme of
$Z_{i}$
be a closed subscheme of
![]() $C_{i}$
that is a finite union of sections
$C_{i}$
that is a finite union of sections
![]() $S\rightarrow C_{i}$
, and let
$S\rightarrow C_{i}$
, and let
![]() $U_{i}$
be the complement of
$U_{i}$
be the complement of
![]() $Z_{i}$
in
$Z_{i}$
in
![]() $C_{i}$
. Fix
$C_{i}$
. Fix
![]() $d\geqslant 1$
.
$d\geqslant 1$
.
Proposition 21. There exists a scheme
![]() ${\mathcal{M}}$
of finite type over
${\mathcal{M}}$
of finite type over
![]() $S$
and a map
$S$
and a map
![]() $\unicode[STIX]{x1D719}:(U_{1})_{{\mathcal{M}}}\rightarrow (U_{2})_{{\mathcal{M}}}$
with the following property: if
$\unicode[STIX]{x1D719}:(U_{1})_{{\mathcal{M}}}\rightarrow (U_{2})_{{\mathcal{M}}}$
with the following property: if
![]() $k$
is a field,
$k$
is a field,
![]() $s\in S(k)$
, and
$s\in S(k)$
, and
![]() $f:U_{1,s}\rightarrow U_{2,s}$
is a map of curves over
$f:U_{1,s}\rightarrow U_{2,s}$
is a map of curves over
![]() $k$
of degree
$k$
of degree
![]() $d$
(meaning the corresponding function field extension has degree
$d$
(meaning the corresponding function field extension has degree
![]() $d$
), then there exists
$d$
), then there exists
![]() $t\in {\mathcal{M}}(k)$
over
$t\in {\mathcal{M}}(k)$
over
![]() $s$
such that
$s$
such that
![]() $f=\unicode[STIX]{x1D719}_{t}$
.
$f=\unicode[STIX]{x1D719}_{t}$
.
Proof. Let
![]() $P$
be the image of a section
$P$
be the image of a section
![]() $S\rightarrow C_{1}$
. Define
$S\rightarrow C_{1}$
. Define
![]() $\widetilde{P}$
to be the
$\widetilde{P}$
to be the
![]() $d$
th nilpotent thickening of
$d$
th nilpotent thickening of
![]() $P$
. Precisely, if
$P$
. Precisely, if
![]() $P$
is defined by the ideal sheaf
$P$
is defined by the ideal sheaf
![]() ${\mathcal{I}}_{P}$
then
${\mathcal{I}}_{P}$
then
![]() $\widetilde{P}$
is defined by
$\widetilde{P}$
is defined by
![]() ${\mathcal{I}}_{P}^{d}$
. Let
${\mathcal{I}}_{P}^{d}$
. Let
![]() $Q$
be the image of a section
$Q$
be the image of a section
![]() $S\rightarrow C_{2}$
, and define
$S\rightarrow C_{2}$
, and define
![]() $\widetilde{Q}$
similarly. Let
$\widetilde{Q}$
similarly. Let
![]() $0\leqslant e\leqslant d$
be an integer. For an
$0\leqslant e\leqslant d$
be an integer. For an
![]() $S$
-scheme
$S$
-scheme
![]() $S^{\prime }$
, let
$S^{\prime }$
, let
![]() ${\mathcal{C}}_{P,Q,e}(S^{\prime })$
be the set of maps
${\mathcal{C}}_{P,Q,e}(S^{\prime })$
be the set of maps
![]() $f:\widetilde{P}_{S^{\prime }}\rightarrow \widetilde{Q}_{S^{\prime }}$
such that
$f:\widetilde{P}_{S^{\prime }}\rightarrow \widetilde{Q}_{S^{\prime }}$
such that
![]() $f^{\ast }({\mathcal{I}}_{Q})\subset {\mathcal{I}}_{P}^{e}$
. As these are finite schemes over
$f^{\ast }({\mathcal{I}}_{Q})\subset {\mathcal{I}}_{P}^{e}$
. As these are finite schemes over
![]() $S$
, this functor is represented by a scheme
$S$
, this functor is represented by a scheme
![]() ${\mathcal{C}}_{P,Q,E}$
of finite type over
${\mathcal{C}}_{P,Q,E}$
of finite type over
![]() $S$
.
$S$
.
Write
![]() $Z_{1}=\coprod _{i=1}^{n}P_{i}$
and
$Z_{1}=\coprod _{i=1}^{n}P_{i}$
and
![]() $Z_{2}=\coprod _{j=1}^{m}Q_{j}$
. (Here
$Z_{2}=\coprod _{j=1}^{m}Q_{j}$
. (Here
![]() $\coprod$
denotes disjoint union.) By a ramification datum we mean a tuple
$\coprod$
denotes disjoint union.) By a ramification datum we mean a tuple
![]() $\unicode[STIX]{x1D70C}=(\unicode[STIX]{x1D70C}_{1},\ldots ,\unicode[STIX]{x1D70C}_{n})$
where each
$\unicode[STIX]{x1D70C}=(\unicode[STIX]{x1D70C}_{1},\ldots ,\unicode[STIX]{x1D70C}_{n})$
where each
![]() $\unicode[STIX]{x1D70C}_{i}$
is either null (denoted
$\unicode[STIX]{x1D70C}_{i}$
is either null (denoted
![]() $\emptyset$
), or a pair
$\emptyset$
), or a pair
![]() $(k_{i},e_{i})$
, where
$(k_{i},e_{i})$
, where
![]() $1\leqslant k_{i}\leqslant m$
and
$1\leqslant k_{i}\leqslant m$
and
![]() $0\leqslant e_{i}\leqslant d$
, such that the following condition holds: for any
$0\leqslant e_{i}\leqslant d$
, such that the following condition holds: for any
![]() $1\leqslant j\leqslant m$
, we have
$1\leqslant j\leqslant m$
, we have
![]() $\sum _{k_{i}=j}e_{i}=d$
(the sum taken over
$\sum _{k_{i}=j}e_{i}=d$
(the sum taken over
![]() $i$
for which
$i$
for which
![]() $\unicode[STIX]{x1D70C}_{i}\neq \emptyset$
). Obviously, there are only finitely many ramification data. For a ramification datum
$\unicode[STIX]{x1D70C}_{i}\neq \emptyset$
). Obviously, there are only finitely many ramification data. For a ramification datum
![]() $\unicode[STIX]{x1D70C}$
, define
$\unicode[STIX]{x1D70C}$
, define
![]() ${\mathcal{C}}_{\unicode[STIX]{x1D70C}}=\prod _{\unicode[STIX]{x1D70C}_{i}\neq \emptyset }{\mathcal{C}}_{P_{i},Q_{k_{i}},e_{i}}$
. Finally, define
${\mathcal{C}}_{\unicode[STIX]{x1D70C}}=\prod _{\unicode[STIX]{x1D70C}_{i}\neq \emptyset }{\mathcal{C}}_{P_{i},Q_{k_{i}},e_{i}}$
. Finally, define
![]() ${\mathcal{C}}=\coprod _{\unicode[STIX]{x1D70C}}{\mathcal{C}}_{\unicode[STIX]{x1D70C}}$
, where the union is taken over all ramification data
${\mathcal{C}}=\coprod _{\unicode[STIX]{x1D70C}}{\mathcal{C}}_{\unicode[STIX]{x1D70C}}$
, where the union is taken over all ramification data
![]() $\unicode[STIX]{x1D70C}$
.
$\unicode[STIX]{x1D70C}$
.
For an
![]() $S$
-scheme
$S$
-scheme
![]() $S^{\prime }$
, let
$S^{\prime }$
, let
![]() ${\mathcal{A}}(S^{\prime })$
be the set of morphisms
${\mathcal{A}}(S^{\prime })$
be the set of morphisms
![]() $(C_{1})_{S^{\prime }}\rightarrow (C_{2})_{S^{\prime }}$
having degree
$(C_{1})_{S^{\prime }}\rightarrow (C_{2})_{S^{\prime }}$
having degree
![]() $d$
in each geometric fiber. The theory of the Hilbert scheme shows that
$d$
in each geometric fiber. The theory of the Hilbert scheme shows that
![]() ${\mathcal{A}}$
is represented by a scheme of finite type over
${\mathcal{A}}$
is represented by a scheme of finite type over
![]() $S$
. For
$S$
. For
![]() $1\leqslant i\leqslant n$
, let
$1\leqslant i\leqslant n$
, let
![]() ${\mathcal{B}}_{i}(S^{\prime })$
be the set of all maps
${\mathcal{B}}_{i}(S^{\prime })$
be the set of all maps
![]() $(\widetilde{P}_{i})_{S^{\prime }}\rightarrow (C_{2})_{S^{\prime }}$
. This is easily seen to be a scheme of finite type over
$(\widetilde{P}_{i})_{S^{\prime }}\rightarrow (C_{2})_{S^{\prime }}$
. This is easily seen to be a scheme of finite type over
![]() $S$
. Let
$S$
. Let
![]() ${\mathcal{B}}=\coprod _{i=1}^{n}\widetilde{{\mathcal{B}}}_{i}$
.
${\mathcal{B}}=\coprod _{i=1}^{n}\widetilde{{\mathcal{B}}}_{i}$
.
We have restriction maps
![]() ${\mathcal{A}}\rightarrow {\mathcal{B}}$
and
${\mathcal{A}}\rightarrow {\mathcal{B}}$
and
![]() ${\mathcal{C}}\rightarrow {\mathcal{B}}$
. Define
${\mathcal{C}}\rightarrow {\mathcal{B}}$
. Define
![]() ${\mathcal{M}}$
to be the fiber product
${\mathcal{M}}$
to be the fiber product
![]() ${\mathcal{A}}\times _{{\mathcal{B}}}{\mathcal{C}}$
, which is a scheme of finite type over
${\mathcal{A}}\times _{{\mathcal{B}}}{\mathcal{C}}$
, which is a scheme of finite type over
![]() $S$
. We can write
$S$
. We can write
![]() ${\mathcal{M}}=\coprod _{\unicode[STIX]{x1D70C}}{\mathcal{M}}_{\unicode[STIX]{x1D70C}}$
, where
${\mathcal{M}}=\coprod _{\unicode[STIX]{x1D70C}}{\mathcal{M}}_{\unicode[STIX]{x1D70C}}$
, where
![]() ${\mathcal{M}}_{\unicode[STIX]{x1D70C}}={\mathcal{A}}\times _{{\mathcal{B}}}{\mathcal{C}}_{\unicode[STIX]{x1D70C}}$
. If
${\mathcal{M}}_{\unicode[STIX]{x1D70C}}={\mathcal{A}}\times _{{\mathcal{B}}}{\mathcal{C}}_{\unicode[STIX]{x1D70C}}$
. If
![]() $s\in S(k)$
then
$s\in S(k)$
then
![]() ${\mathcal{M}}_{\unicode[STIX]{x1D70C},s}(k)$
is the set of degree
${\mathcal{M}}_{\unicode[STIX]{x1D70C},s}(k)$
is the set of degree
![]() $d$
maps
$d$
maps
![]() $f:C_{1,s}\rightarrow C_{2,s}$
satisfying the following conditions at the
$f:C_{1,s}\rightarrow C_{2,s}$
satisfying the following conditions at the
![]() $P_{i}$
: if
$P_{i}$
: if
![]() $\unicode[STIX]{x1D70C}_{i}=\emptyset$
then there is no condition at
$\unicode[STIX]{x1D70C}_{i}=\emptyset$
then there is no condition at
![]() $P_{i}$
; otherwise,
$P_{i}$
; otherwise,
![]() $f(P_{i})=Q_{k_{i}}$
and the ramification index
$f(P_{i})=Q_{k_{i}}$
and the ramification index
![]() $e(P_{i}\mid Q_{k_{i}})$
is at least
$e(P_{i}\mid Q_{k_{i}})$
is at least
![]() $e_{i}$
. (In fact, the ramification index is exactly
$e_{i}$
. (In fact, the ramification index is exactly
![]() $e_{i}$
, since the total ramification is
$e_{i}$
, since the total ramification is
![]() $d$
and the
$d$
and the
![]() $e_{i}$
with
$e_{i}$
with
![]() $k_{i}=j$
add up to
$k_{i}=j$
add up to
![]() $d$
.) It is clear that such a map carries
$d$
.) It is clear that such a map carries
![]() $U_{1,s}$
into
$U_{1,s}$
into
![]() $U_{2,s}$
, and that any map
$U_{2,s}$
, and that any map
![]() $U_{1,s}\rightarrow U_{2,s}$
of degree
$U_{1,s}\rightarrow U_{2,s}$
of degree
![]() $d$
comes from a point of some
$d$
comes from a point of some
![]() ${\mathcal{M}}_{\unicode[STIX]{x1D70C},s}$
. Thus every map
${\mathcal{M}}_{\unicode[STIX]{x1D70C},s}$
. Thus every map
![]() $f:U_{1,s}\rightarrow U_{2,s}$
comes from some
$f:U_{1,s}\rightarrow U_{2,s}$
comes from some
![]() $k$
-point of
$k$
-point of
![]() ${\mathcal{M}}_{s}$
. Finally, note that the universal map
${\mathcal{M}}_{s}$
. Finally, note that the universal map
![]() $(C_{1})_{{\mathcal{M}}}\rightarrow (C_{2})_{{\mathcal{M}}}$
carries
$(C_{1})_{{\mathcal{M}}}\rightarrow (C_{2})_{{\mathcal{M}}}$
carries
![]() $(U_{1})_{{\mathcal{M}}}$
to
$(U_{1})_{{\mathcal{M}}}$
to
![]() $(U_{2})_{{\mathcal{M}}}$
, as this can be checked at field points of
$(U_{2})_{{\mathcal{M}}}$
, as this can be checked at field points of
![]() ${\mathcal{M}}$
. This proves the proposition.◻
${\mathcal{M}}$
. This proves the proposition.◻
6 Proof of Theorem 1
Keep notation as in Theorem 1, and put
![]() $S=\operatorname{Spec}({\mathcal{O}}_{K}[1/N])$
.
$S=\operatorname{Spec}({\mathcal{O}}_{K}[1/N])$
.
Lemma 22. The restriction of
![]() $L$
to any open subgroup of
$L$
to any open subgroup of
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U_{K})$
is irreducible.
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U_{K})$
is irreducible.
Proof. Suppose not. Then there exists an open normal subgroup
![]() $H$
of
$H$
of
![]() $G=\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U_{K})$
such that
$G=\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U_{K})$
such that
![]() $L|_{H}$
is reducible. It is semi-simple by Lemma 12, and therefore a sum of two characters. Since characters have finite ramification by Lemma 10, we have contradicted assumption (d).◻
$L|_{H}$
is reducible. It is semi-simple by Lemma 12, and therefore a sum of two characters. Since characters have finite ramification by Lemma 10, we have contradicted assumption (d).◻
Choose
![]() $r\gg 0$
so that
$r\gg 0$
so that
![]() $X=X(\ell ^{r})$
has genus at least 2 and
$X=X(\ell ^{r})$
has genus at least 2 and
![]() $Y=Y(\ell ^{r})$
is a fine moduli space; in fact,
$Y=Y(\ell ^{r})$
is a fine moduli space; in fact,
![]() $r=3$
suffices for any
$r=3$
suffices for any
![]() $\ell$
. We replace
$\ell$
. We replace
![]() ${\mathcal{L}}$
with a rank-two
${\mathcal{L}}$
with a rank-two
![]() ${\mathcal{O}}_{E}$
-sheaf, where
${\mathcal{O}}_{E}$
-sheaf, where
![]() $E/\mathbf{Q}_{\ell }$
is a finite extension. Proposition 13 and Lemma 22 show that it suffices to prove the proposition after passing to a finite cover of
$E/\mathbf{Q}_{\ell }$
is a finite extension. Proposition 13 and Lemma 22 show that it suffices to prove the proposition after passing to a finite cover of
![]() $C$
. By passing to an appropriate cover, we can therefore assume that
$C$
. By passing to an appropriate cover, we can therefore assume that
![]() ${\mathcal{L}}/\ell ^{r}$
is trivial. The image of the Galois representation
${\mathcal{L}}/\ell ^{r}$
is trivial. The image of the Galois representation
![]() $\unicode[STIX]{x1D70C}:\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}({\mathcal{U}})\rightarrow \mathbf{GL}_{2}({\mathcal{O}}_{E})$
has order
$\unicode[STIX]{x1D70C}:\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}({\mathcal{U}})\rightarrow \mathbf{GL}_{2}({\mathcal{O}}_{E})$
has order
![]() $M\cdot \ell ^{\infty }$
(in the sense of profinite groups) for some positive integer
$M\cdot \ell ^{\infty }$
(in the sense of profinite groups) for some positive integer
![]() $M$
. By enlarging
$M$
. By enlarging
![]() $N$
, we can assume that
$N$
, we can assume that
![]() $M\cdot \ell \mid N$
and that the complement of
$M\cdot \ell \mid N$
and that the complement of
![]() $U$
in
$U$
in
![]() $C$
spreads out to a divisor on
$C$
spreads out to a divisor on
![]() ${\mathcal{C}}$
that is smooth over
${\mathcal{C}}$
that is smooth over
![]() $S$
.
$S$
.
We make the following definitions.
∙ Let
 $D$
be an integer greater than
$D$
be an integer greater than
 $(g(C)-1)/(g(X)-1)$
, where
$(g(C)-1)/(g(X)-1)$
, where
 $g(-)$
denotes genus. Let
$g(-)$
denotes genus. Let
 ${\mathcal{M}}_{d}$
be the space of maps
${\mathcal{M}}_{d}$
be the space of maps
 ${\mathcal{U}}\rightarrow Y$
of degree
${\mathcal{U}}\rightarrow Y$
of degree
 $d$
, in the sense of Proposition 21, and let
$d$
, in the sense of Proposition 21, and let
 ${\mathcal{M}}=\coprod _{d=1}^{D}{\mathcal{M}}_{d}$
.
${\mathcal{M}}=\coprod _{d=1}^{D}{\mathcal{M}}_{d}$
.∙ Let
 ${\mathcal{T}}$
be the (integral)
${\mathcal{T}}$
be the (integral)
 $\ell$
-adic Tate module of the universal elliptic curve over
$\ell$
-adic Tate module of the universal elliptic curve over
 $Y$
, and let
$Y$
, and let
 ${\mathcal{T}}^{\prime }={\mathcal{T}}\otimes {\mathcal{O}}_{E}$
. Also let
${\mathcal{T}}^{\prime }={\mathcal{T}}\otimes {\mathcal{O}}_{E}$
. Also let
 ${\mathcal{L}}_{n}={\mathcal{L}}/\ell ^{n}{\mathcal{L}}$
and let
${\mathcal{L}}_{n}={\mathcal{L}}/\ell ^{n}{\mathcal{L}}$
and let
 ${\mathcal{T}}_{n}^{\prime }={\mathcal{T}}^{\prime }/\ell ^{n}{\mathcal{T}}^{\prime }$
.
${\mathcal{T}}_{n}^{\prime }={\mathcal{T}}^{\prime }/\ell ^{n}{\mathcal{T}}^{\prime }$
.∙ Let
 $\widetilde{{\mathcal{M}}}_{n}$
be the moduli space of pairs
$\widetilde{{\mathcal{M}}}_{n}$
be the moduli space of pairs
 $(f,\unicode[STIX]{x1D713})$
where
$(f,\unicode[STIX]{x1D713})$
where
 $f\in {\mathcal{M}}$
and
$f\in {\mathcal{M}}$
and
 $\unicode[STIX]{x1D713}$
is an isomorphism of
$\unicode[STIX]{x1D713}$
is an isomorphism of
 ${\mathcal{O}}_{E}$
-sheaves
${\mathcal{O}}_{E}$
-sheaves
 $f^{\ast }({\mathcal{T}}_{n})\rightarrow {\mathcal{L}}_{n}$
.
$f^{\ast }({\mathcal{T}}_{n})\rightarrow {\mathcal{L}}_{n}$
.
Lemma 23. The map
![]() $\unicode[STIX]{x1D70B}:\widetilde{{\mathcal{M}}}_{n}\rightarrow {\mathcal{M}}$
is finite.
$\unicode[STIX]{x1D70B}:\widetilde{{\mathcal{M}}}_{n}\rightarrow {\mathcal{M}}$
is finite.
Proof. Note that for every field-valued point
![]() $f$
of
$f$
of
![]() ${\mathcal{M}}$
there are only finitely many choices for
${\mathcal{M}}$
there are only finitely many choices for
![]() $\unicode[STIX]{x1D713}$
and so the map
$\unicode[STIX]{x1D713}$
and so the map
![]() $\widetilde{{\mathcal{M}}}_{n}\rightarrow {\mathcal{M}}$
is quasi-finite. Thus, to prove the lemma it is sufficient to show that
$\widetilde{{\mathcal{M}}}_{n}\rightarrow {\mathcal{M}}$
is quasi-finite. Thus, to prove the lemma it is sufficient to show that
![]() $\unicode[STIX]{x1D70B}$
is proper.
$\unicode[STIX]{x1D70B}$
is proper.
We use the valuative criterion. Let
![]() $R$
be a discrete valuation ring with fraction field
$R$
be a discrete valuation ring with fraction field
![]() $F$
. Let
$F$
. Let
![]() $f\in {\mathcal{M}}(R)$
and
$f\in {\mathcal{M}}(R)$
and
![]() $(\unicode[STIX]{x1D713},f)\in \widetilde{{\mathcal{M}}}_{n}(F)$
. Thus,
$(\unicode[STIX]{x1D713},f)\in \widetilde{{\mathcal{M}}}_{n}(F)$
. Thus,
![]() $f$
corresponds to a map
$f$
corresponds to a map
![]() $f:U_{R}\rightarrow Y_{R}$
and
$f:U_{R}\rightarrow Y_{R}$
and
![]() $\unicode[STIX]{x1D713}:f^{\ast }({\mathcal{T}}_{n})_{F}\rightarrow ({\mathcal{L}}_{n})_{F}$
is an isomorphism. Since
$\unicode[STIX]{x1D713}:f^{\ast }({\mathcal{T}}_{n})_{F}\rightarrow ({\mathcal{L}}_{n})_{F}$
is an isomorphism. Since
![]() $f^{\ast }({\mathcal{T}}_{n})$
and
$f^{\ast }({\mathcal{T}}_{n})$
and
![]() ${\mathcal{L}}_{n}$
are finite étale sheaves on
${\mathcal{L}}_{n}$
are finite étale sheaves on
![]() $U_{R}$
, which is a normal scheme,
$U_{R}$
, which is a normal scheme,
![]() $\unicode[STIX]{x1D713}$
extends uniquely over
$\unicode[STIX]{x1D713}$
extends uniquely over
![]() $U_{R}$
.◻
$U_{R}$
.◻
Let
![]() ${\mathcal{M}}_{n}$
be the image of
${\mathcal{M}}_{n}$
be the image of
![]() $\widetilde{{\mathcal{M}}}_{n}$
in
$\widetilde{{\mathcal{M}}}_{n}$
in
![]() ${\mathcal{M}}$
, which is closed by Lemma 23. We endow it with the reduced subscheme structure. As the
${\mathcal{M}}$
, which is closed by Lemma 23. We endow it with the reduced subscheme structure. As the
![]() ${\mathcal{M}}_{n}$
form a descending chain of closed subschemes of
${\mathcal{M}}_{n}$
form a descending chain of closed subschemes of
![]() ${\mathcal{M}}$
, they stabilize. Let
${\mathcal{M}}$
, they stabilize. Let
![]() ${\mathcal{M}}_{\infty }$
be
${\mathcal{M}}_{\infty }$
be
![]() ${\mathcal{M}}_{n}$
for
${\mathcal{M}}_{n}$
for
![]() $n\gg 0$
.
$n\gg 0$
.
Lemma 24. The fiber of
![]() ${\mathcal{M}}_{\infty }$
over all closed points of
${\mathcal{M}}_{\infty }$
over all closed points of
![]() $S$
is non-empty.
$S$
is non-empty.
Proof. Let
![]() $s$
be a closed point of
$s$
be a closed point of
![]() $S$
of characteristic
$S$
of characteristic
![]() $p$
. By Lemma 12,
$p$
. By Lemma 12,
![]() ${\mathcal{L}}_{\overline{K}}$
is an irreducible sheaf. Let
${\mathcal{L}}_{\overline{K}}$
is an irreducible sheaf. Let
![]() $\overline{S}$
be the strict Hensilization of
$\overline{S}$
be the strict Hensilization of
![]() $S$
at
$S$
at
![]() $s$
,
$s$
,
![]() $\overline{s}$
the geometric point corresponding to
$\overline{s}$
the geometric point corresponding to
![]() $s$
, and
$s$
, and
![]() $K_{s}$
the fraction field of
$K_{s}$
the fraction field of
![]() $\overline{S}$
. By [Reference Grothendieck and RaynaudSGA1, XIII, 2.10, p. 289],
$\overline{S}$
. By [Reference Grothendieck and RaynaudSGA1, XIII, 2.10, p. 289],
where
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t},(p)}$
denotes the prime to
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t},(p)}$
denotes the prime to
![]() $p$
quotient of
$p$
quotient of
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}$
. We can regard
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}$
. We can regard
![]() ${\mathcal{L}}$
as a representation of
${\mathcal{L}}$
as a representation of
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}({\mathcal{U}})$
and then restrict it to a representation of
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}({\mathcal{U}})$
and then restrict it to a representation of
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}({\mathcal{U}}_{\overline{S}})$
; since the image of the representation has order
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}({\mathcal{U}}_{\overline{S}})$
; since the image of the representation has order
![]() $M\ell ^{\infty }$
, which is prime to
$M\ell ^{\infty }$
, which is prime to
![]() $p$
, it factors through
$p$
, it factors through
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t},(p)}({\mathcal{U}}_{\overline{S}})$
. We thus obtain a representation of
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t},(p)}({\mathcal{U}}_{\overline{S}})$
. We thus obtain a representation of
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t},(p)}({\mathcal{U}}_{\overline{s}})$
. The pullback of this representation to
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t},(p)}({\mathcal{U}}_{\overline{s}})$
. The pullback of this representation to
![]() $\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U_{\overline{K}})$
is irreducible. It follows now that
$\unicode[STIX]{x1D70B}_{1}^{\acute{\text{e}}\text{t}}(U_{\overline{K}})$
is irreducible. It follows now that
![]() ${\mathcal{L}}_{s}$
is an irreducible sheaf on
${\mathcal{L}}_{s}$
is an irreducible sheaf on
![]() ${\mathcal{U}}_{s}$
.
${\mathcal{U}}_{s}$
.
By Proposition 19, we can find a family of elliptic curves
![]() ${\mathcal{E}}\rightarrow {\mathcal{U}}_{s}$
and an isomorphism
${\mathcal{E}}\rightarrow {\mathcal{U}}_{s}$
and an isomorphism
![]() ${\mathcal{L}}_{s}|_{{\mathcal{U}}_{s}}\cong T({\mathcal{E}})\otimes {\mathcal{O}}_{E}$
, where
${\mathcal{L}}_{s}|_{{\mathcal{U}}_{s}}\cong T({\mathcal{E}})\otimes {\mathcal{O}}_{E}$
, where
![]() $T({\mathcal{E}})$
is the relative Tate module of
$T({\mathcal{E}})$
is the relative Tate module of
![]() ${\mathcal{E}}$
. It follows that
${\mathcal{E}}$
. It follows that
![]() $T({\mathcal{E}})/\ell ^{r}$
is the trivial sheaf, and so we can find a basis for
$T({\mathcal{E}})/\ell ^{r}$
is the trivial sheaf, and so we can find a basis for
![]() ${\mathcal{E}}[\ell ^{r}]$
over
${\mathcal{E}}[\ell ^{r}]$
over
![]() ${\mathcal{U}}_{s}$
. We thus have a map
${\mathcal{U}}_{s}$
. We thus have a map
![]() $f:{\mathcal{U}}_{s}\rightarrow Y$
such that
$f:{\mathcal{U}}_{s}\rightarrow Y$
such that
![]() $T({\mathcal{E}})\cong f^{\ast }({\mathcal{T}})$
. Factor
$T({\mathcal{E}})\cong f^{\ast }({\mathcal{T}})$
. Factor
![]() $f$
as
$f$
as
![]() $g\circ F^{n}$
, where
$g\circ F^{n}$
, where
![]() $g:{\mathcal{U}}_{s}\rightarrow Y$
is separable and
$g:{\mathcal{U}}_{s}\rightarrow Y$
is separable and
![]() $F:Y_{\unicode[STIX]{x1D705}(s)}\rightarrow Y_{\unicode[STIX]{x1D705}(s)}$
is the absolute Frobenius element. Note that
$F:Y_{\unicode[STIX]{x1D705}(s)}\rightarrow Y_{\unicode[STIX]{x1D705}(s)}$
is the absolute Frobenius element. Note that
![]() $F^{\ast }({\mathcal{T}})\cong {\mathcal{T}}$
, and so
$F^{\ast }({\mathcal{T}})\cong {\mathcal{T}}$
, and so
![]() $T({\mathcal{E}})\cong g^{\ast }({\mathcal{T}})$
. Let
$T({\mathcal{E}})\cong g^{\ast }({\mathcal{T}})$
. Let
![]() $\overline{g}$
be the extension of
$\overline{g}$
be the extension of
![]() $g$
to a map
$g$
to a map
![]() $C_{s}\rightarrow X$
. Since
$C_{s}\rightarrow X$
. Since
![]() $\overline{g}$
is separable, it has degree
$\overline{g}$
is separable, it has degree
![]() ${\leqslant}D$
. Thus
${\leqslant}D$
. Thus
![]() $\overline{g}$
, and the isomorphism
$\overline{g}$
, and the isomorphism
![]() ${\mathcal{L}}\cong g^{\ast }({\mathcal{T}})\otimes {\mathcal{O}}_{E}$
, define a
${\mathcal{L}}\cong g^{\ast }({\mathcal{T}})\otimes {\mathcal{O}}_{E}$
, define a
![]() $\unicode[STIX]{x1D705}(s)$
points of
$\unicode[STIX]{x1D705}(s)$
points of
![]() ${\mathcal{M}}_{n}$
for all
${\mathcal{M}}_{n}$
for all
![]() $n$
, which proves the lemma.◻
$n$
, which proves the lemma.◻
Proof of Theorem 1.
Since
![]() ${\mathcal{M}}_{\infty }$
is finite type over
${\mathcal{M}}_{\infty }$
is finite type over
![]() $S$
and all of its fibers over closed points are non-empty, it follows that the generic fiber of
$S$
and all of its fibers over closed points are non-empty, it follows that the generic fiber of
![]() ${\mathcal{M}}_{\infty }$
is non-empty. Choose a point
${\mathcal{M}}_{\infty }$
is non-empty. Choose a point
![]() $x$
in
$x$
in
![]() ${\mathcal{M}}_{\infty }(L^{\prime })$
, for some finite extension
${\mathcal{M}}_{\infty }(L^{\prime })$
, for some finite extension
![]() $L^{\prime }/L$
, corresponding to a family of elliptic curves
$L^{\prime }/L$
, corresponding to a family of elliptic curves
![]() ${\mathcal{E}}\rightarrow U_{L^{\prime }}$
. Now,
${\mathcal{E}}\rightarrow U_{L^{\prime }}$
. Now,
![]() $x$
lifts to
$x$
lifts to
![]() $\widetilde{{\mathcal{M}}}_{n}(\overline{L})$
for all
$\widetilde{{\mathcal{M}}}_{n}(\overline{L})$
for all
![]() $n$
. Thus
$n$
. Thus
![]() ${\mathcal{L}}_{n}$
and
${\mathcal{L}}_{n}$
and
![]() $T({\mathcal{E}})\otimes {\mathcal{O}}_{E}/\ell ^{n}$
are isomorphic for all
$T({\mathcal{E}})\otimes {\mathcal{O}}_{E}/\ell ^{n}$
are isomorphic for all
![]() $n$
, as sheaves on
$n$
, as sheaves on
![]() $U_{\overline{L}}$
. It follows that
$U_{\overline{L}}$
. It follows that
![]() ${\mathcal{L}}$
and
${\mathcal{L}}$
and
![]() $T({\mathcal{E}})\otimes {\mathcal{O}}_{E}$
are isomorphic over
$T({\mathcal{E}})\otimes {\mathcal{O}}_{E}$
are isomorphic over
![]() $U_{\overline{L}}$
(by compactness). By Lemma 11,
$U_{\overline{L}}$
(by compactness). By Lemma 11,
![]() ${\mathcal{L}}[1/\ell ]$
and
${\mathcal{L}}[1/\ell ]$
and
![]() $T({\mathcal{E}})\otimes E$
are isomorphic over
$T({\mathcal{E}})\otimes E$
are isomorphic over
![]() $U_{L^{\prime \prime }}$
, for some finite (even quadratic) extension
$U_{L^{\prime \prime }}$
, for some finite (even quadratic) extension
![]() $L^{\prime \prime }$
of
$L^{\prime \prime }$
of
![]() $L^{\prime }$
. Passing to the generic fibers, we see that
$L^{\prime }$
. Passing to the generic fibers, we see that
![]() $\unicode[STIX]{x1D70C}|_{KL^{\prime \prime }}$
comes from an elliptic curve, which completes the proof by Proposition 13.◻
$\unicode[STIX]{x1D70C}|_{KL^{\prime \prime }}$
comes from an elliptic curve, which completes the proof by Proposition 13.◻
Acknowledgement
We thank the referee for helpful comments, which improved the exposition of the paper.