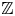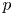Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Mor, Itamar
2024.
The Relative Brauer Group of K(1)-Local Spectra.
International Mathematics Research Notices,
Vol. 2024,
Issue. 15,
p.
11292.
Carmeli, Shachar
Schlank, Tomer M
and
Yanovski, Lior
2024.
Chromatic cyclotomic extensions.
Geometry & Topology,
Vol. 28,
Issue. 8,
p.
3511.