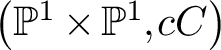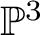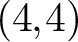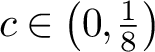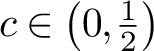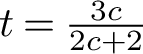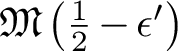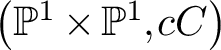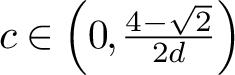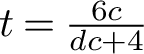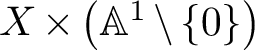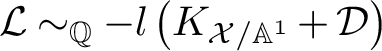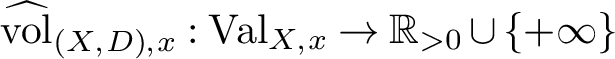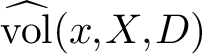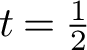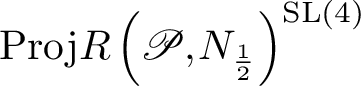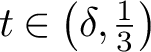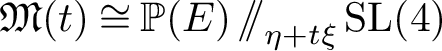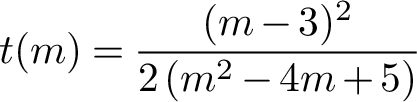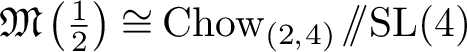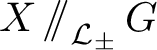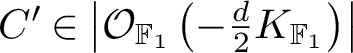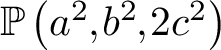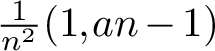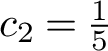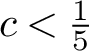1 Introduction
The moduli space
![]() $\mathscr {F}$
of polarized K3 surfaces is often constructed as the arithmetic quotient of an Hermitian symmetric domain, and comes with a natural Baily–Borel compactification
$\mathscr {F}$
of polarized K3 surfaces is often constructed as the arithmetic quotient of an Hermitian symmetric domain, and comes with a natural Baily–Borel compactification
![]() $\mathscr {F} \subset \mathscr {F}^*$
. A long-standing problem has been to compare this compactification with other compactifications which carry a more geometric meaning, such as those coming from geometric invariant theory (GIT). In particular, if
$\mathscr {F} \subset \mathscr {F}^*$
. A long-standing problem has been to compare this compactification with other compactifications which carry a more geometric meaning, such as those coming from geometric invariant theory (GIT). In particular, if
![]() $\mathfrak {M}$
denotes a GIT compactification, there is often a birational period map
$\mathfrak {M}$
denotes a GIT compactification, there is often a birational period map
![]() $\mathfrak {p}: \mathfrak {M} \dashrightarrow \mathscr {F}^*$
thanks to the global Torelli theorem for K3 surfaces, and a natural question is whether this map can be resolved in a modular way.
$\mathfrak {p}: \mathfrak {M} \dashrightarrow \mathscr {F}^*$
thanks to the global Torelli theorem for K3 surfaces, and a natural question is whether this map can be resolved in a modular way.
The case of degree
![]() $2$
K3 surfaces was worked out by Shah [Reference Shah69] and Looijenga [Reference Looijenga58]. In particular, Shah constructs a space
$2$
K3 surfaces was worked out by Shah [Reference Shah69] and Looijenga [Reference Looijenga58]. In particular, Shah constructs a space
![]() $\widehat {\mathfrak {M}}$
as a partial Kirwan desingularization of the GIT quotient
$\widehat {\mathfrak {M}}$
as a partial Kirwan desingularization of the GIT quotient
![]() $\mathfrak {M}$
, which Looijenga shows is simultaneously a small partial resolution of
$\mathfrak {M}$
, which Looijenga shows is simultaneously a small partial resolution of
![]() $\mathscr {F}^*$
(a semitoric compactification, in the language of [Reference Looijenga59]). In particular, there is one space that interpolates between the GIT and Baily–Borel compacitfications. A far-reaching conjectural generalization is proposed by Laza and O’Grady in [Reference Laza and O’Grady42]. When
$\mathscr {F}^*$
(a semitoric compactification, in the language of [Reference Looijenga59]). In particular, there is one space that interpolates between the GIT and Baily–Borel compacitfications. A far-reaching conjectural generalization is proposed by Laza and O’Grady in [Reference Laza and O’Grady42]. When
![]() $\mathscr {F}$
is a type IV locally symmetric variety associated to a lattice of the form
$\mathscr {F}$
is a type IV locally symmetric variety associated to a lattice of the form
![]() $U^2 \oplus D_{N-2}$
(e.g., hyperelliptic quartic K3 surfaces when
$U^2 \oplus D_{N-2}$
(e.g., hyperelliptic quartic K3 surfaces when
![]() $N=18$
, quartic K3 surfaces when
$N=18$
, quartic K3 surfaces when
![]() $N=19$
, or double EPW-sextics when
$N=19$
, or double EPW-sextics when
![]() $N=20$
), they conjecture a systematic way to resolve the period map
$N=20$
), they conjecture a systematic way to resolve the period map
![]() $\mathfrak {p}$
via a series of birational transformations governed by certain divisors present in
$\mathfrak {p}$
via a series of birational transformations governed by certain divisors present in
![]() $\mathscr {F}^*$
. They confirm their conjectures in the case of hyperelliptic quartic K3 surfaces in [Reference Laza and O’Grady43] (i.e., when
$\mathscr {F}^*$
. They confirm their conjectures in the case of hyperelliptic quartic K3 surfaces in [Reference Laza and O’Grady43] (i.e., when
![]() $N=18$
); we briefly review some of their results (see §3.3 for a more detailed discussion).
$N=18$
); we briefly review some of their results (see §3.3 for a more detailed discussion).
Let C be a smooth curve in
![]() $\mathbb {P}^1\times \mathbb {P}^1$
of bidegree
$\mathbb {P}^1\times \mathbb {P}^1$
of bidegree
![]() $(4,4)$
, and let
$(4,4)$
, and let
![]() $\pi : X_C \to \mathbb {P}^1 \times \mathbb {P}^1$
be the double cover of the quadric surface branched along C. The resulting surface
$\pi : X_C \to \mathbb {P}^1 \times \mathbb {P}^1$
be the double cover of the quadric surface branched along C. The resulting surface
![]() $X_C$
is a smooth hyperelliptic polarized K3 surface of degree
$X_C$
is a smooth hyperelliptic polarized K3 surface of degree
![]() $4$
, whose polarization is given by the pullback
$4$
, whose polarization is given by the pullback
![]() $\pi ^*\left(\mathcal {O}_{\mathbb {P}^1}(1) \boxtimes \mathcal {O}_{\mathbb {P}^1}(1)\right)$
. The corresponding period domain gives a moduli space
$\pi ^*\left(\mathcal {O}_{\mathbb {P}^1}(1) \boxtimes \mathcal {O}_{\mathbb {P}^1}(1)\right)$
. The corresponding period domain gives a moduli space
![]() $\mathscr {F} \subset \mathscr {F}^*$
. If
$\mathscr {F} \subset \mathscr {F}^*$
. If
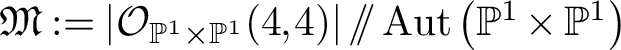 $\mathfrak {M}:=\lvert \mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(4,4)\rvert \mathbin {/\mkern -6mu/}\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
denotes the GIT quotient of
$\mathfrak {M}:=\lvert \mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(4,4)\rvert \mathbin {/\mkern -6mu/}\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
denotes the GIT quotient of
![]() $(4,4)$
curves on
$(4,4)$
curves on
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
, then there is a birational period map
$\mathbb {P}^1 \times \mathbb {P}^1$
, then there is a birational period map
![]() $\mathfrak {p}: \mathfrak {M} \dashrightarrow \mathscr {F}^*$
. In [Reference Laza and O’Grady43], Laza and O’Grady described the birational map
$\mathfrak {p}: \mathfrak {M} \dashrightarrow \mathscr {F}^*$
. In [Reference Laza and O’Grady43], Laza and O’Grady described the birational map
![]() $\mathfrak {p}$
as a series of explicit wall crossings. Let
$\mathfrak {p}$
as a series of explicit wall crossings. Let
![]() $\lambda $
denote the Hodge line bundle on
$\lambda $
denote the Hodge line bundle on
![]() $\mathscr {F}$
, and let
$\mathscr {F}$
, and let
![]() $\Delta $
=
$\Delta $
=
![]() $H/2$
, where H is the Heegner divisor parametrizing periods of K3 surfaces which are double covers of a quadric cone. In this setting, Laza and O’Grady show that one can interpolate between
$H/2$
, where H is the Heegner divisor parametrizing periods of K3 surfaces which are double covers of a quadric cone. In this setting, Laza and O’Grady show that one can interpolate between
![]() $\mathscr {F}^*$
and
$\mathscr {F}^*$
and
![]() $\mathfrak {M}$
by considering
$\mathfrak {M}$
by considering
![]() $\mathscr {F}(\beta ) := \mathrm {Proj} R(\lambda + \beta \Delta )$
and varying
$\mathscr {F}(\beta ) := \mathrm {Proj} R(\lambda + \beta \Delta )$
and varying
![]() $ 0 \leq \beta \leq 1$
. One aspect of their proof is a variation-of-GIT (VGIT) study on the moduli space of
$ 0 \leq \beta \leq 1$
. One aspect of their proof is a variation-of-GIT (VGIT) study on the moduli space of
![]() $(2,4)$
-complete intersection curves in
$(2,4)$
-complete intersection curves in
![]() $\mathbb {P}^3$
. Denoting this space by
$\mathbb {P}^3$
. Denoting this space by
![]() $\mathfrak {M}(t)$
, they show that each step
$\mathfrak {M}(t)$
, they show that each step
![]() $\mathscr {F}(\beta )$
can be realized as the VGIT moduli space
$\mathscr {F}(\beta )$
can be realized as the VGIT moduli space
![]() $\mathfrak {M}(t)$
for some specific
$\mathfrak {M}(t)$
for some specific
![]() $t(\beta )$
.
$t(\beta )$
.
If
 $c\in \left(0,\frac {1}{2}\right)$
is a rational number, then
$c\in \left(0,\frac {1}{2}\right)$
is a rational number, then
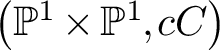 $\left(\mathbb {P}^1 \times \mathbb {P}^1, cC\right)$
is a log Fano pair. Recently, it has become apparent that K-stability provides a natural framework to construct compactifications of moduli spaces of log Fano pairs (see, e.g., [Reference Ascher, DeVleming and Liu6] or §2.4). With this in mind, our goal in this paper is to use this theory to construct alternative compactifications of the moduli space of smooth
$\left(\mathbb {P}^1 \times \mathbb {P}^1, cC\right)$
is a log Fano pair. Recently, it has become apparent that K-stability provides a natural framework to construct compactifications of moduli spaces of log Fano pairs (see, e.g., [Reference Ascher, DeVleming and Liu6] or §2.4). With this in mind, our goal in this paper is to use this theory to construct alternative compactifications of the moduli space of smooth
![]() $(4,4)$
curves. The framework to study K-moduli of log Fano pairs was established in [Reference Ascher, DeVleming and Liu6], where we constructed proper good moduli spaces parametrizing
$(4,4)$
curves. The framework to study K-moduli of log Fano pairs was established in [Reference Ascher, DeVleming and Liu6], where we constructed proper good moduli spaces parametrizing
![]() $\mathbb {Q}$
-Gorenstein smoothable K-polystable log Fano pairs
$\mathbb {Q}$
-Gorenstein smoothable K-polystable log Fano pairs
![]() $(X, cD)$
, where D is a rational multiple of
$(X, cD)$
, where D is a rational multiple of
![]() $-K_X$
and c is a rational number. Furthermore, we showed that the moduli spaces undergo wall crossings as the weight c varies.
$-K_X$
and c is a rational number. Furthermore, we showed that the moduli spaces undergo wall crossings as the weight c varies.
Let
![]() $\overline {\mathcal {K}}_{c}$
be the connected component of the moduli stack parametrizing K-semistable log Fano pairs which admit
$\overline {\mathcal {K}}_{c}$
be the connected component of the moduli stack parametrizing K-semistable log Fano pairs which admit
![]() $\mathbb {Q}$
-Gorenstein smoothings to
$\mathbb {Q}$
-Gorenstein smoothings to
 $\left(\mathbb {P}^1 \times \mathbb {P}^1, c C\right)$
, where C is a
$\left(\mathbb {P}^1 \times \mathbb {P}^1, c C\right)$
, where C is a
![]() $(4,4)$
curve. By [Reference Ascher, DeVleming and Liu6], the moduli stack
$(4,4)$
curve. By [Reference Ascher, DeVleming and Liu6], the moduli stack
![]() $\overline {\mathcal {K}}_c$
admits a proper good moduli space
$\overline {\mathcal {K}}_c$
admits a proper good moduli space
![]() $\overline {K}_c$
. The goal of this paper is to show that this K-moduli space
$\overline {K}_c$
. The goal of this paper is to show that this K-moduli space
![]() $\overline {K}_c$
and the wall crossings obtained by varying the weight vector c coincide with the wall crossings given by the VGIT
$\overline {K}_c$
and the wall crossings obtained by varying the weight vector c coincide with the wall crossings given by the VGIT
![]() $\mathfrak {M}(t)$
under the correspondence
$\mathfrak {M}(t)$
under the correspondence
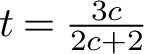 $t=\frac {3c}{2c+2}$
. In particular, varying the weight c on the K-moduli space
$t=\frac {3c}{2c+2}$
. In particular, varying the weight c on the K-moduli space
![]() $\overline {K}_c$
interpolates between
$\overline {K}_c$
interpolates between
![]() $\mathfrak {M}$
and
$\mathfrak {M}$
and
![]() $\mathscr {F}^*$
, and gives the intermediate spaces an alternative modular meaning.
$\mathscr {F}^*$
, and gives the intermediate spaces an alternative modular meaning.
Theorem 1.1. Let
![]() $\overline {\mathcal {K}}_c$
be the moduli stack parametrizing K-semistable (resp.
$\overline {\mathcal {K}}_c$
be the moduli stack parametrizing K-semistable (resp.
![]() $\overline {K}_c$
) log Fano pairs
$\overline {K}_c$
) log Fano pairs
![]() $(X,cD)$
admitting
$(X,cD)$
admitting
![]() $\mathbb {Q}$
-Gorenstein smoothings to
$\mathbb {Q}$
-Gorenstein smoothings to
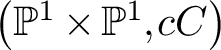 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where C is a smooth
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where C is a smooth
![]() $(4,4)$
curve. Let
$(4,4)$
curve. Let
![]() $\mathscr {M}$
be the GIT quotient stack of
$\mathscr {M}$
be the GIT quotient stack of
![]() $(4,4)$
curves on
$(4,4)$
curves on
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
. Let
$\mathbb {P}^1 \times \mathbb {P}^1$
. Let
![]() $\mathscr {M}(t)$
be the VGIT quotient stack of
$\mathscr {M}(t)$
be the VGIT quotient stack of
![]() $(2,4)$
-complete intersection curves in
$(2,4)$
-complete intersection curves in
![]() $\mathbb {P}^3$
of slope t (see Definition 3.2).
$\mathbb {P}^3$
of slope t (see Definition 3.2).
-
(1) Let
 $c \in \left(0, \frac {1}{8}\right)$
be a rational number. Then there is an isomorphism of Artin stacks
$c \in \left(0, \frac {1}{8}\right)$
be a rational number. Then there is an isomorphism of Artin stacks
 $\overline {\mathcal {K}}_c \cong \mathscr {M}$
. In particular, a
$\overline {\mathcal {K}}_c \cong \mathscr {M}$
. In particular, a
 $(4,4)$
curve C on
$(4,4)$
curve C on
 $\mathbb {P}^1\times \mathbb {P}^1$
is GIT-(poly/semi)semistable if and only if
$\mathbb {P}^1\times \mathbb {P}^1$
is GIT-(poly/semi)semistable if and only if
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
is K-(poly/semi)stable.
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
is K-(poly/semi)stable. -
(2) Let
 $c \in \left(0, \frac {1}{2}\right)$
be a rational number. Then there is an isomorphism of Artin stacks
$c \in \left(0, \frac {1}{2}\right)$
be a rational number. Then there is an isomorphism of Artin stacks
 $\overline {\mathcal {K}}_c\cong \mathscr {M}(t)$
with
$\overline {\mathcal {K}}_c\cong \mathscr {M}(t)$
with
 $t=\frac {3c}{2c+2}$
. Moreover, such isomorphisms commute with the wall-crossing morphisms for K-moduli stacks
$t=\frac {3c}{2c+2}$
. Moreover, such isomorphisms commute with the wall-crossing morphisms for K-moduli stacks
 $\overline {\mathcal {K}}_c$
and GIT moduli stacks
$\overline {\mathcal {K}}_c$
and GIT moduli stacks
 $\mathscr {M}(t)$
.
$\mathscr {M}(t)$
.
Moreover, all isomorphisms descend to the level of good moduli spaces.
We note here that the comparison between K-moduli spaces and (V)GIT moduli spaces in various explicit settings has been studied before, such as [Reference Mabuchi and Mukai60, Reference Odaka, Spotti and Sun65, Reference Spotti and Sun71, Reference Liu and Xu56, Reference Fujita24, Reference Gallardo, Martinez-Garcia and Spotti28, Reference Ascher, DeVleming and Liu6] (see also Remark 2.20).
Combining Theorem 1.1 with the main results in [Reference Laza and O’Grady43], we obtain the following isomorphisms between moduli spaces and their natural polarizations. In particular, the wall-crossing morphisms between our K-moduli spaces
![]() $\overline {K}_c$
form a natural interpolation of the period map
$\overline {K}_c$
form a natural interpolation of the period map
![]() $\mathfrak {p}:\mathfrak {M}\dashrightarrow \mathscr {F}^*$
. For an explicit description of K-moduli wall crossings, see Remarks 5.13 and 5.14.
$\mathfrak {p}:\mathfrak {M}\dashrightarrow \mathscr {F}^*$
. For an explicit description of K-moduli wall crossings, see Remarks 5.13 and 5.14.
Theorem 1.2. Let
![]() $\overline {K}_c$
be the good moduli space parametrizing K-polystable log Fano pairs
$\overline {K}_c$
be the good moduli space parametrizing K-polystable log Fano pairs
![]() $(X,cD)$
admitting
$(X,cD)$
admitting
![]() $\mathbb {Q}$
-Gorenstein smoothings to
$\mathbb {Q}$
-Gorenstein smoothings to
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where C is a smooth
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where C is a smooth
![]() $(4,4)$
curve. Let
$(4,4)$
curve. Let
![]() $\mathfrak {M}(t)$
be the VGIT quotient space of
$\mathfrak {M}(t)$
be the VGIT quotient space of
![]() $(2,4)$
-complete intersection curves in
$(2,4)$
-complete intersection curves in
![]() $\mathbb {P}^3$
of slope t (see Definition 3.2). Then for any rational number
$\mathbb {P}^3$
of slope t (see Definition 3.2). Then for any rational number
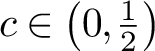 $c\in \left(0,\frac {1}{2}\right)$
, we have
$c\in \left(0,\frac {1}{2}\right)$
, we have
 $$ \begin{align*} \overline{K}_c\cong \mathfrak{M}(t)\cong \mathscr{F}(\beta), \quad \text{where }t=\frac{3c}{2c+2} \text{ and }\beta=\min\left\{1,\frac{1-2c}{6c}\right\}. \end{align*} $$
$$ \begin{align*} \overline{K}_c\cong \mathfrak{M}(t)\cong \mathscr{F}(\beta), \quad \text{where }t=\frac{3c}{2c+2} \text{ and }\beta=\min\left\{1,\frac{1-2c}{6c}\right\}. \end{align*} $$
Moreover, the CM
![]() $\mathbb {Q}$
-line bundle on
$\mathbb {Q}$
-line bundle on
![]() $\overline {K}_c$
, the VGIT polarization on
$\overline {K}_c$
, the VGIT polarization on
![]() $\mathfrak {M}(t)$
, and the Laza–O’Grady polarization on
$\mathfrak {M}(t)$
, and the Laza–O’Grady polarization on
![]() $\mathscr {F}(\beta )$
(i.e., the push-forward of
$\mathscr {F}(\beta )$
(i.e., the push-forward of
![]() $\lambda +\beta \Delta $
under
$\lambda +\beta \Delta $
under
![]() $\mathscr {F}\dashrightarrow \mathscr {F}(\beta )$
) are all proportional up to positive factors.
$\mathscr {F}\dashrightarrow \mathscr {F}(\beta )$
) are all proportional up to positive factors.
As a consequence of these theorems and [Reference Laza and O’Grady43, Theorem 1.1(iv)], we identify the final K-moduli space
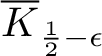 $\overline {K}_{\frac {1}{2}-\epsilon }$
with Looijenga’s semitoric compactification
$\overline {K}_{\frac {1}{2}-\epsilon }$
with Looijenga’s semitoric compactification
![]() $\widehat {\mathscr {F}}$
of
$\widehat {\mathscr {F}}$
of
![]() $\mathscr {F}$
. In part (1) of the following theorem, we give an alternative proof of [Reference Laza and O’Grady43, Second part of Theorem 1.1(iv)] using K-stability. Part (2) suggests that
$\mathscr {F}$
. In part (1) of the following theorem, we give an alternative proof of [Reference Laza and O’Grady43, Second part of Theorem 1.1(iv)] using K-stability. Part (2) suggests that
![]() $\mathscr {F}^*$
can be viewed as a moduli space of log Calabi–Yau pairs, as expected in [Reference Ascher, DeVleming and Liu6, Conjecture 1.8].
$\mathscr {F}^*$
can be viewed as a moduli space of log Calabi–Yau pairs, as expected in [Reference Ascher, DeVleming and Liu6, Conjecture 1.8].
Theorem 1.3. Let
![]() $0<\epsilon ,\epsilon '\ll 1$
be two sufficiently small rational numbers. Then we have isomorphisms
$0<\epsilon ,\epsilon '\ll 1$
be two sufficiently small rational numbers. Then we have isomorphisms
 $\overline {K}_{\frac {1}{2}-\epsilon }\cong \mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)\cong \widehat {\mathscr {F}}$
. Moreover, we have the following:
$\overline {K}_{\frac {1}{2}-\epsilon }\cong \mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)\cong \widehat {\mathscr {F}}$
. Moreover, we have the following:
-
(1) The moduli space
 $\mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)$
parametrizes quartic hyperelliptic K3 surfaces with semilog canonical singularities.
$\mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)$
parametrizes quartic hyperelliptic K3 surfaces with semilog canonical singularities. -
(2) The Hodge line bundle over
 $\overline {K}_{\frac {1}{2}-\epsilon }$
is semiample with ample model
$\overline {K}_{\frac {1}{2}-\epsilon }$
is semiample with ample model
 $\mathscr {F}^*$
.
$\mathscr {F}^*$
.
Finally, we discuss some partial generalizations of Theorem 1.1 to higher-degree curves on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
(see also Remark 6.10).
$\mathbb {P}^1\times \mathbb {P}^1$
(see also Remark 6.10).
Theorem 1.4. Let
![]() $d\geq 3$
be an integer. Let
$d\geq 3$
be an integer. Let
![]() $\overline {\mathcal {K}}_{d,c}$
be the moduli stack parametrizing K-semistable log Fano pairs
$\overline {\mathcal {K}}_{d,c}$
be the moduli stack parametrizing K-semistable log Fano pairs
![]() $(X,cD)$
admitting
$(X,cD)$
admitting
![]() $\mathbb {Q}$
-Gorenstein smoothings to
$\mathbb {Q}$
-Gorenstein smoothings to
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where C is a smooth
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where C is a smooth
![]() $(d,d)$
curve. Let
$(d,d)$
curve. Let
![]() $\mathscr {M}_d$
be the GIT quotient stack of
$\mathscr {M}_d$
be the GIT quotient stack of
![]() $(d,d)$
curves on
$(d,d)$
curves on
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
. Let
$\mathbb {P}^1 \times \mathbb {P}^1$
. Let
![]() $\mathscr {M}_d(t)$
be the VGIT quotient stack of
$\mathscr {M}_d(t)$
be the VGIT quotient stack of
![]() $(2,d)$
complete intersection curves in
$(2,d)$
complete intersection curves in
![]() $\mathbb {P}^3$
of slope
$\mathbb {P}^3$
of slope
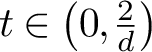 $t\in \left(0, \frac {2}{d}\right)$
(see Definition 6.2).
$t\in \left(0, \frac {2}{d}\right)$
(see Definition 6.2).
-
(1) Let
 $c \in \left(0, \frac {1}{2d}\right)$
be a rational number. Then there is an isomorphism of Artin stacks
$c \in \left(0, \frac {1}{2d}\right)$
be a rational number. Then there is an isomorphism of Artin stacks
 $\overline {\mathcal {K}}_{d,c} \cong \mathscr {M}_d$
. In particular, C is GIT-(poly/semi)semistable on
$\overline {\mathcal {K}}_{d,c} \cong \mathscr {M}_d$
. In particular, C is GIT-(poly/semi)semistable on
 $\mathbb {P}^1\times \mathbb {P}^1$
if and only if
$\mathbb {P}^1\times \mathbb {P}^1$
if and only if
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
is K-(poly/semi)stable.
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
is K-(poly/semi)stable. -
(2) Let
 $c \in \left(0, \frac {4-\sqrt {2}}{2d}\right)$
be a rational number. Then there is an isomorphism of Artin stacks
$c \in \left(0, \frac {4-\sqrt {2}}{2d}\right)$
be a rational number. Then there is an isomorphism of Artin stacks
 $\overline {\mathcal {K}}_{d,c}\cong \mathscr {M}_d(t)$
with
$\overline {\mathcal {K}}_{d,c}\cong \mathscr {M}_d(t)$
with
 $t=\frac {6c}{dc+4}$
. Moreover, such isomorphisms commute with the wall-crossing morphisms for K-moduli stacks
$t=\frac {6c}{dc+4}$
. Moreover, such isomorphisms commute with the wall-crossing morphisms for K-moduli stacks
 $\overline {\mathcal {K}}_{d,c}$
and GIT moduli stacks
$\overline {\mathcal {K}}_{d,c}$
and GIT moduli stacks
 $\mathscr {M}_d(t)$
.
$\mathscr {M}_d(t)$
.
Organization
For the remainder of this paper, c (and thus t and
![]() $\beta $
) will always denote a rational number. This paper is organized as follows. In §2 we recall the definitions of K-stability, normalized volumes, and the CM-line bundle. We also recall the main results of [Reference Ascher, DeVleming and Liu6] and define the relevant moduli functor. In §3 we recall the background on K3 surfaces and review the main results of [Reference Laza and O’Grady43]. In §4 we determine which surfaces can appear as degenerations of
$\beta $
) will always denote a rational number. This paper is organized as follows. In §2 we recall the definitions of K-stability, normalized volumes, and the CM-line bundle. We also recall the main results of [Reference Ascher, DeVleming and Liu6] and define the relevant moduli functor. In §3 we recall the background on K3 surfaces and review the main results of [Reference Laza and O’Grady43]. In §4 we determine which surfaces can appear as degenerations of
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
on the boundary of the K-moduli spaces. Key ingredients are Theorems 4.7 and 4.8, which bound the Gorenstein indices of singular surfaces using normalized volumes. In §5 we compare the GIT compactification with the K-stability compactification, and study the wall crossings that appear for K-moduli. In particular, we present the proofs of Theorems 1.1, 1.2, and 1.3. These are achieved by the index estimates already mentioned, computation of CM line bundles, and a modification of Paul and Tian’s criterion [Reference Paul and Tian67] (see also [Reference Odaka, Spotti and Sun65, Reference Ascher, DeVleming and Liu6]) to work over nonproper bases (see also [Reference Spotti and Sun71]). Note that the VGIT of
$\mathbb {P}^1 \times \mathbb {P}^1$
on the boundary of the K-moduli spaces. Key ingredients are Theorems 4.7 and 4.8, which bound the Gorenstein indices of singular surfaces using normalized volumes. In §5 we compare the GIT compactification with the K-stability compactification, and study the wall crossings that appear for K-moduli. In particular, we present the proofs of Theorems 1.1, 1.2, and 1.3. These are achieved by the index estimates already mentioned, computation of CM line bundles, and a modification of Paul and Tian’s criterion [Reference Paul and Tian67] (see also [Reference Odaka, Spotti and Sun65, Reference Ascher, DeVleming and Liu6]) to work over nonproper bases (see also [Reference Spotti and Sun71]). Note that the VGIT of
![]() $(2,4)$
-complete intersections in
$(2,4)$
-complete intersections in
![]() $\mathbb {P}^3$
for a general slope does not provide a
$\mathbb {P}^3$
for a general slope does not provide a
![]() $\mathbb {Q}$
-Gorenstein flat log Fano family over a proper base, but only such a family over the complete intersection locus as a quasi-projective variety. This creates an issue in that the usual Paul–Tian criterion cannot be directly applied. In order to resolve this issue, we trace the change of K/VGIT stability conditions along their wall crossings, and argue that their polystable replacements indeed coincide. Finally, in §6 we discuss some generalizations for higher-degree curves on
$\mathbb {Q}$
-Gorenstein flat log Fano family over a proper base, but only such a family over the complete intersection locus as a quasi-projective variety. This creates an issue in that the usual Paul–Tian criterion cannot be directly applied. In order to resolve this issue, we trace the change of K/VGIT stability conditions along their wall crossings, and argue that their polystable replacements indeed coincide. Finally, in §6 we discuss some generalizations for higher-degree curves on
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
and prove Theorem 1.4.
$\mathbb {P}^1 \times \mathbb {P}^1$
and prove Theorem 1.4.
2 Preliminaries
Throughout this paper, we work over the field of complex numbers
![]() $\mathbb {C}$
, and all schemes are assumed to be of finite type over
$\mathbb {C}$
, and all schemes are assumed to be of finite type over
![]() $\mathbb {C}$
. A variety is a separated integral scheme of finite type over
$\mathbb {C}$
. A variety is a separated integral scheme of finite type over
![]() $\mathbb {C}$
.
$\mathbb {C}$
.
2.1 K-stability of log Fano pairs
We first recall necessary background to define the K-stability of log Fano pairs:
Definition 2.1. Let X be a normal variety and let D be an effective
![]() $\mathbb {Q}$
-divisor on X. We say such
$\mathbb {Q}$
-divisor on X. We say such
![]() $(X,D)$
is a log pair. If X is projective and
$(X,D)$
is a log pair. If X is projective and
![]() $-(K_X+D)$
is
$-(K_X+D)$
is
![]() $\mathbb {Q}$
-Cartier ample, then the log pair
$\mathbb {Q}$
-Cartier ample, then the log pair
![]() $(X,D)$
is called a log Fano pair. The variety X is a
$(X,D)$
is called a log Fano pair. The variety X is a
![]() $\mathbb {Q}$
-Fano variety if
$\mathbb {Q}$
-Fano variety if
![]() $(X,0)$
is a klt log Fano pair.
$(X,0)$
is a klt log Fano pair.
Next we recall the definition of the K-stability of log Fano pairs:
Definition 2.2 [Reference Tian74, Reference Donaldson20, Reference Li46, Reference Li and Xu49, Reference Odaka and Sun66]
Let
![]() $(X,D)$
be a log Fano pair and let L be an ample line bundle on X such that
$(X,D)$
be a log Fano pair and let L be an ample line bundle on X such that
![]() $L\sim _{\mathbb {Q}}-l(K_X+D)$
for some
$L\sim _{\mathbb {Q}}-l(K_X+D)$
for some
![]() $l\in \mathbb {Q}_{>0}$
.
$l\in \mathbb {Q}_{>0}$
.
-
(a) A normal test configuration
 $(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
of
$(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
of
 $(X,D;L)$
consists of the following data:
$(X,D;L)$
consists of the following data:-
(i) a normal variety
 $\mathcal X$
together with a flat projective morphism
$\mathcal X$
together with a flat projective morphism
 $\pi :\mathcal X\to \mathbb {A}^1$
;
$\pi :\mathcal X\to \mathbb {A}^1$
; -
(ii) a
 $\pi $
-ample line bundle
$\pi $
-ample line bundle
 $\mathcal L$
on
$\mathcal L$
on
 $\mathcal X$
;
$\mathcal X$
; -
(iii) a
 $\mathbb {G}_m$
-action on
$\mathbb {G}_m$
-action on
 $(\mathcal X;\mathcal L)$
such that
$(\mathcal X;\mathcal L)$
such that
 $\pi $
is
$\pi $
is
 $\mathbb {G}_m$
-equivariant with respect to the standard action of
$\mathbb {G}_m$
-equivariant with respect to the standard action of
 $\mathbb {G}_m$
on
$\mathbb {G}_m$
on
 $\mathbb {A}^1$
via multiplication;
$\mathbb {A}^1$
via multiplication; -
(iv)
 $\left(\mathcal X\setminus \mathcal X_0;\mathcal L\rvert _{\mathcal X\setminus \mathcal X_0}\right)$
$\left(\mathcal X\setminus \mathcal X_0;\mathcal L\rvert _{\mathcal X\setminus \mathcal X_0}\right)$
 $\mathbb {G}_m$
-equivariantly isomorphic to
$\mathbb {G}_m$
-equivariantly isomorphic to
 $(X;L)\times \left(\mathbb {A}^1\setminus \{0\}\right)$
; and
$(X;L)\times \left(\mathbb {A}^1\setminus \{0\}\right)$
; and -
(v) an effective
 $\mathbb {Q}$
-divisor
$\mathbb {Q}$
-divisor
 $\mathcal D$
on
$\mathcal D$
on
 $\mathcal X$
such that
$\mathcal X$
such that
 $\mathcal D$
is the Zariski closure of
$\mathcal D$
is the Zariski closure of
 $D\times \left(\mathbb {A}^1\setminus \{0\}\right)$
under the identification between
$D\times \left(\mathbb {A}^1\setminus \{0\}\right)$
under the identification between
 $\mathcal X\setminus \mathcal X_0$
and
$\mathcal X\setminus \mathcal X_0$
and
 $X\times \left(\mathbb {A}^1\setminus \{0\}\right)$
.
$X\times \left(\mathbb {A}^1\setminus \{0\}\right)$
.
A normal test configuration is called a product test configuration if
for some $$ \begin{align*} (\mathcal X,\mathcal D;\mathcal L)\cong\left(X\times\mathbb{A}^1,D\times\mathbb{A}^1;\mathrm{pr}_1^* L\otimes\mathcal{O}_{\mathcal X}(k\mathcal X_0)\right) \end{align*} $$
$$ \begin{align*} (\mathcal X,\mathcal D;\mathcal L)\cong\left(X\times\mathbb{A}^1,D\times\mathbb{A}^1;\mathrm{pr}_1^* L\otimes\mathcal{O}_{\mathcal X}(k\mathcal X_0)\right) \end{align*} $$
 $k\in \mathbb {Z}$
. A product test configuration is called a trivial test configuration if this isomorphism is
$k\in \mathbb {Z}$
. A product test configuration is called a trivial test configuration if this isomorphism is
 $\mathbb {G}_m$
-equivariant with respect to the trivial
$\mathbb {G}_m$
-equivariant with respect to the trivial
 $\mathbb {G}_m$
-action on X and the standard
$\mathbb {G}_m$
-action on X and the standard
 $\mathbb {G}_m$
-action on
$\mathbb {G}_m$
-action on
 $\mathbb {A}^1$
via multiplication.
$\mathbb {A}^1$
via multiplication.
-
-
(b) For a normal test configuration
 $(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
of
$(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
of
 $(X,D)$
, denote its natural compactification over
$(X,D)$
, denote its natural compactification over
 $\mathbb {P}^1$
by
$\mathbb {P}^1$
by
 $\left(\overline {\mathcal X},\overline {\mathcal D};\overline {\mathcal {L}}\right)$
. The generalized Futaki invariant of
$\left(\overline {\mathcal X},\overline {\mathcal D};\overline {\mathcal {L}}\right)$
. The generalized Futaki invariant of
 $(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
is defined by the following intersection formula due to [Reference Wang75, Reference Odaka64]:
$(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
is defined by the following intersection formula due to [Reference Wang75, Reference Odaka64]:  $$ \begin{align*} \mathrm{Fut}(\mathcal X,\mathcal D;\mathcal L):=\frac{1}{(-(K_X+D))^n}\left(\frac{n}{n+1}\cdot\frac{\left(\bar{\mathcal L}^{n+1}\right)}{l^{n+1}}+\frac{\left(\bar{\mathcal L}^n\cdot \left(K_{\bar{\mathcal X}/\mathbb{P}^1}+\bar{\mathcal D}\right)\right)}{l^n}\right). \end{align*} $$
$$ \begin{align*} \mathrm{Fut}(\mathcal X,\mathcal D;\mathcal L):=\frac{1}{(-(K_X+D))^n}\left(\frac{n}{n+1}\cdot\frac{\left(\bar{\mathcal L}^{n+1}\right)}{l^{n+1}}+\frac{\left(\bar{\mathcal L}^n\cdot \left(K_{\bar{\mathcal X}/\mathbb{P}^1}+\bar{\mathcal D}\right)\right)}{l^n}\right). \end{align*} $$
-
(c) The log Fano pair
 $(X,D)$
is said to be:
$(X,D)$
is said to be:-
(i) K-semistable if
 $\mathrm {Fut}(\mathcal X,\mathcal D;\mathcal L)\geq 0$
for any normal test configuration
$\mathrm {Fut}(\mathcal X,\mathcal D;\mathcal L)\geq 0$
for any normal test configuration
 $(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
and any
$(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
and any
 $l\in \mathbb {Q}_{>0}$
such that L is Cartier;
$l\in \mathbb {Q}_{>0}$
such that L is Cartier; -
(ii) K-stable if it is K-semistable and
 $\mathrm {Fut}(\mathcal X,\mathcal D;\mathcal L)=0$
for a normal test configuration
$\mathrm {Fut}(\mathcal X,\mathcal D;\mathcal L)=0$
for a normal test configuration
 $(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
if and only if it is a trivial test configuration; or
$(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
if and only if it is a trivial test configuration; or -
(iii) K-polystable if it is K-semistable and
 $\mathrm {Fut}(\mathcal X,\mathcal D;\mathcal L)=0$
for a normal test configuration
$\mathrm {Fut}(\mathcal X,\mathcal D;\mathcal L)=0$
for a normal test configuration
 $(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
if and only if it is a product test configuration.
$(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
if and only if it is a product test configuration.
-
-
(d) Let
 $(X,D)$
be a klt log Fano pair. Then a normal test configuration
$(X,D)$
be a klt log Fano pair. Then a normal test configuration
 $(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
is called a special test configuration if
$(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
is called a special test configuration if
 $\mathcal L\sim _{\mathbb {Q}}-l\left(K_{\mathcal X/\mathbb {A}^1}+\mathcal D\right)$
and
$\mathcal L\sim _{\mathbb {Q}}-l\left(K_{\mathcal X/\mathbb {A}^1}+\mathcal D\right)$
and
 $(\mathcal X,\mathcal D+\mathcal X_0)$
is plt. In this case, we say that
$(\mathcal X,\mathcal D+\mathcal X_0)$
is plt. In this case, we say that
 $(X,D)$
specially degenerates to
$(X,D)$
specially degenerates to
 $(\mathcal X_0,\mathcal D_0)$
, which is necessarily a klt log Fano pair.
$(\mathcal X_0,\mathcal D_0)$
, which is necessarily a klt log Fano pair.
Remark 2.3.
-
(1) The concept of K-(semi/poly)stability of log Fano pairs can also be defined via test configurations that are possibly nonnormal. For the general definitions we refer to [Reference Ascher, DeVleming and Liu6, Section 2.1]. By [Reference Boucksom, Hisamoto and Jonsson14, Proposition 3.15], we know that generalized Futaki invariants will not increase under normalization of test configurations.
-
(2) Odaka proved in [Reference Odaka63] that any K-semistable log Fano pair is klt. By the work of Li and Xu [Reference Li and Xu49], to test the K-(poly/semi)stability of a klt log Fano pair it suffices to test the sign of generalized Futaki invariants only on special test configurations.
The following lemma is very useful in the proof of Theorem 1.1:
Lemma 2.4.
-
(1) [Reference Kempf35] Let G be a reductive group acting on a polarized projective scheme
 $(Y,L)$
. Let
$(Y,L)$
. Let
 $y\in Y$
be a closed point. Let
$y\in Y$
be a closed point. Let
 $\sigma :\mathbb {G}_m\to G$
be a 1-PS. Denote
$\sigma :\mathbb {G}_m\to G$
be a 1-PS. Denote
 $y'=\lim _{t\to 0}\sigma (t)\cdot y$
. If y is GIT semistable and
$y'=\lim _{t\to 0}\sigma (t)\cdot y$
. If y is GIT semistable and
 $\mu ^{L}(y,\sigma )=0$
, then
$\mu ^{L}(y,\sigma )=0$
, then
 $y'$
is also GIT semistable.
$y'$
is also GIT semistable. -
(2) [Reference Li, Wang and Xu53, Lemma 3.1] Let
 $(X,D)$
be a log Fano pair. Let
$(X,D)$
be a log Fano pair. Let
 $(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
be a normal test configuration of
$(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
be a normal test configuration of
 $(X,D)$
. If
$(X,D)$
. If
 $(X,D)$
is K-semistable and
$(X,D)$
is K-semistable and
 $\mathrm {Fut}(\mathcal X,\mathcal D;\mathcal L)=0$
, then
$\mathrm {Fut}(\mathcal X,\mathcal D;\mathcal L)=0$
, then
 $(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
is a special test configuration and
$(\mathcal X,\mathcal D;\mathcal L)/\mathbb {A}^1$
is a special test configuration and
 $(\mathcal X_0,\mathcal D_0)$
is also K-semistable.
$(\mathcal X_0,\mathcal D_0)$
is also K-semistable.
2.2 Normalized volumes
In this section, we consider a klt singularity
![]() $x\in (X,D)$
– that is, a klt log pair
$x\in (X,D)$
– that is, a klt log pair
![]() $(X,D)$
with a closed point
$(X,D)$
with a closed point
![]() $x\in X$
. Recall that a valuation v on X centered at x is a real valuation of
$x\in X$
. Recall that a valuation v on X centered at x is a real valuation of
![]() $\mathbb {C}(X)$
such that the valuation ring
$\mathbb {C}(X)$
such that the valuation ring
![]() $\mathcal {O}_v$
dominates
$\mathcal {O}_v$
dominates
![]() $\mathcal {O}_{X,x}$
as local rings. The set of such valuations is denoted by
$\mathcal {O}_{X,x}$
as local rings. The set of such valuations is denoted by
![]() $\mathrm {Val}_{X,x}$
.
$\mathrm {Val}_{X,x}$
.
We briefly review normalized volume of valuations as introduced by Chi Li [Reference Li48]. See [Reference Li, Liu and Xu52] for a survey on recent developments.
Definition 2.5. Let
![]() $x\in (X,D)$
be an n-dimensional klt singularity.
$x\in (X,D)$
be an n-dimensional klt singularity.
-
(a) The volume is a function
 $\mathrm {vol}_{X,x}:\mathrm {Val}_{X,x}\to \mathbb {R}_{\geq 0}$
, defined in [Reference Ein, Lazarsfeld and Smith21] as
$\mathrm {vol}_{X,x}:\mathrm {Val}_{X,x}\to \mathbb {R}_{\geq 0}$
, defined in [Reference Ein, Lazarsfeld and Smith21] as  $$ \begin{align*} \mathrm{vol}_{X,x}(v):=\lim_{k\to\infty}\frac{\dim_{\mathbb{C}}\mathcal{O}_{X,x}/\left\{f\in\mathcal{O}_{X,x}\mid v(f)\geq k\right\}}{k^n/n!}. \end{align*} $$
$$ \begin{align*} \mathrm{vol}_{X,x}(v):=\lim_{k\to\infty}\frac{\dim_{\mathbb{C}}\mathcal{O}_{X,x}/\left\{f\in\mathcal{O}_{X,x}\mid v(f)\geq k\right\}}{k^n/n!}. \end{align*} $$
-
(b) The log discrepancy is a function
 $A_{\left(X,D\right)}:\mathrm {Val}_{X,x}\to \mathbb {R}_{>0}\cup \{+\infty \}$
, defined in [Reference Jonsson and Mustaţă34, Reference Boucksom, de Fernex, Favre and Urbinati13]. If
$A_{\left(X,D\right)}:\mathrm {Val}_{X,x}\to \mathbb {R}_{>0}\cup \{+\infty \}$
, defined in [Reference Jonsson and Mustaţă34, Reference Boucksom, de Fernex, Favre and Urbinati13]. If
 $v=a\cdot \mathrm {ord}_E$
, where
$v=a\cdot \mathrm {ord}_E$
, where
 $a\in \mathbb {R}_{>0}$
and E is a prime divisor over X centered at x, then where
$a\in \mathbb {R}_{>0}$
and E is a prime divisor over X centered at x, then where $$ \begin{align*} A_{\left(X,D\right)}(v)=a(1+\mathrm{ord}_E(K_{Y}-\pi^*(K_X+D))), \end{align*} $$
$$ \begin{align*} A_{\left(X,D\right)}(v)=a(1+\mathrm{ord}_E(K_{Y}-\pi^*(K_X+D))), \end{align*} $$
 $\pi :Y\to X$
provides a birational model Y of X containing E as a divisor. In this paper, we deal only with divisorial valuations.
$\pi :Y\to X$
provides a birational model Y of X containing E as a divisor. In this paper, we deal only with divisorial valuations.
-
(c) The normalized volume is a function
 $\widehat {\mathrm {vol}}_{\left(X,D\right),x}:\mathrm {Val}_{X,x}\to \mathbb {R}_{>0}\cup \{+\infty \}$
, defined in [Reference Li48] as
$\widehat {\mathrm {vol}}_{\left(X,D\right),x}:\mathrm {Val}_{X,x}\to \mathbb {R}_{>0}\cup \{+\infty \}$
, defined in [Reference Li48] as  $$ \begin{align*} \widehat{\mathrm{vol}}_{\left(X,D\right),x}(v):=\begin{cases} A_{\left(X,D\right)}(v)^n\cdot\mathrm{vol}_{X,x}(v) & \text{if } A_{\left(X,D\right)}(v)<+\infty,\\ +\infty & \text{if }A_{\left(X,D\right)}(v)=+\infty. \end{cases} \end{align*} $$
$$ \begin{align*} \widehat{\mathrm{vol}}_{\left(X,D\right),x}(v):=\begin{cases} A_{\left(X,D\right)}(v)^n\cdot\mathrm{vol}_{X,x}(v) & \text{if } A_{\left(X,D\right)}(v)<+\infty,\\ +\infty & \text{if }A_{\left(X,D\right)}(v)=+\infty. \end{cases} \end{align*} $$
The local volume of a klt singularity
 $x\in (X,D)$
is defined as Note that the existence of a normalized volume minimizer is proven in [Reference Blum9]. From [Reference Li and Xu50] we know that
$x\in (X,D)$
is defined as Note that the existence of a normalized volume minimizer is proven in [Reference Blum9]. From [Reference Li and Xu50] we know that $$ \begin{align*} \widehat{\mathrm{vol}}(x,X,D):=\min_{v\in\mathrm{Val}_{X,x}}\widehat{\mathrm{vol}}_{\left(X,D\right),x}(v). \end{align*} $$
$$ \begin{align*} \widehat{\mathrm{vol}}(x,X,D):=\min_{v\in\mathrm{Val}_{X,x}}\widehat{\mathrm{vol}}_{\left(X,D\right),x}(v). \end{align*} $$
 $\widehat {\mathrm {vol}}(x,X,D)$
can be approximated by the normalized volume of divisorial valuations.
$\widehat {\mathrm {vol}}(x,X,D)$
can be approximated by the normalized volume of divisorial valuations.
The following theorem from [Reference Li and Liu51], generalizing [Reference Fujita25, Theorem 1.1] and [Reference Liu54, Theorem 1.2], is crucial. Note that it also follows from the valuative criterion for K-semistability by Fujita [Reference Fujita26] and C. Li [Reference Li47].
Theorem 2.6 [Reference Li and Liu51, Proposition 4.6]
Let
![]() $(X,D)$
be a K-semistable log Fano pair of dimension n. Then for any closed point
$(X,D)$
be a K-semistable log Fano pair of dimension n. Then for any closed point
![]() $x\in X$
, we have
$x\in X$
, we have
 $$ \begin{align*} (-K_X-D)^n\leq \left(1+\frac{1}{n}\right)^n\widehat{\mathrm{vol}}(x,X,D). \end{align*} $$
$$ \begin{align*} (-K_X-D)^n\leq \left(1+\frac{1}{n}\right)^n\widehat{\mathrm{vol}}(x,X,D). \end{align*} $$
2.3 CM line bundles
The Chow–Mumford (CM) line bundle of a flat family of polarized projective varieties was introduced algebraically by Tian [Reference Tian74] as a functorial line bundle over the base. We start with the definition of CM line bundles due to Paul and Tian [Reference Paul and Tian67, Reference Paul and Tian68] using the Knudsen–Mumford expansion (see also [Reference Fujiki and Schumacher23, Reference Fine and Ross22]). In order to define CM line bundles for families of log Fano pairs over reduced bases, we need to use the concept of relative Mumford divisors from [Reference Kollár39, Definition 1] (see also [Reference Kollár38]).
Definition 2.7 Relative Mumford divisors
Let
![]() $f: \mathcal X\to T$
be a morphism between schemes. Assume that f has
$f: \mathcal X\to T$
be a morphism between schemes. Assume that f has
![]() $S_2$
fibers of pure dimension n. A closed subscheme
$S_2$
fibers of pure dimension n. A closed subscheme
![]() $\mathcal D$
of
$\mathcal D$
of
![]() $\mathcal X$
is a relative Mumford divisor over T if there is an open subset
$\mathcal X$
is a relative Mumford divisor over T if there is an open subset
![]() $\mathcal {U}\subset \mathcal X$
such that:
$\mathcal {U}\subset \mathcal X$
such that:
-
(1)
 $\textrm {codim}_{\mathcal X_t} \left(\mathcal X_t\setminus \mathcal {U}_t\right)\geq 2$
for any
$\textrm {codim}_{\mathcal X_t} \left(\mathcal X_t\setminus \mathcal {U}_t\right)\geq 2$
for any
 $t\in T$
,
$t\in T$
, -
(2)
 $\mathcal D\rvert _{\mathcal {U}}$
is a relative Cartier divisor,
$\mathcal D\rvert _{\mathcal {U}}$
is a relative Cartier divisor, -
(3)
 $\mathcal D$
is the scheme-theoretic closure of
$\mathcal D$
is the scheme-theoretic closure of
 $\mathcal D\rvert _{\mathcal {U}}$
, and
$\mathcal D\rvert _{\mathcal {U}}$
, and -
(4)
 $\mathcal X_t$
is smooth at generic points of
$\mathcal X_t$
is smooth at generic points of
 $\textrm {Supp}(\mathcal D_t)$
for any
$\textrm {Supp}(\mathcal D_t)$
for any
 $t\in T$
.
$t\in T$
.
A relative Mumford
![]() $\mathbb {Q}$
-divisor is a formal
$\mathbb {Q}$
-divisor is a formal
![]() $\mathbb {Q}$
-linear combination of relative Mumford divisors.
$\mathbb {Q}$
-linear combination of relative Mumford divisors.
Definition 2.8 Log CM line bundle
Let
![]() $f:\mathcal X\to T$
be a proper flat morphism of connected schemes. Assume that f has
$f:\mathcal X\to T$
be a proper flat morphism of connected schemes. Assume that f has
![]() $S_2$
fibers of pure dimension n. Let
$S_2$
fibers of pure dimension n. Let
![]() $\mathcal L$
be an f-ample line bundle on
$\mathcal L$
be an f-ample line bundle on
![]() $\mathcal X$
.
$\mathcal X$
.
A result of Knudsen and Mumford [Reference Knudsen and Mumford36] says that there exist line bundles
![]() $\lambda _j=\lambda _{j}(\mathcal X,\mathcal L)$
on T such that for all k,
$\lambda _j=\lambda _{j}(\mathcal X,\mathcal L)$
on T such that for all k,
 $$ \begin{align*} \det f_!\left(\mathcal L^k\right)=\lambda_{n+1}^{\binom{k}{n+1}}\otimes\lambda_n^{\binom{k}{n}}\otimes\dotsb\otimes\lambda_0. \end{align*} $$
$$ \begin{align*} \det f_!\left(\mathcal L^k\right)=\lambda_{n+1}^{\binom{k}{n+1}}\otimes\lambda_n^{\binom{k}{n}}\otimes\dotsb\otimes\lambda_0. \end{align*} $$
By flatness, the Hilbert polynomial
 $\chi \left(\mathcal X_t,\mathcal L_t^k\right)=a_0 k^n+a_1 k^{n-1}+ O\left(k^{n-2}\right)$
. Then the CM line bundle of the data
$\chi \left(\mathcal X_t,\mathcal L_t^k\right)=a_0 k^n+a_1 k^{n-1}+ O\left(k^{n-2}\right)$
. Then the CM line bundle of the data
![]() $(f:\mathcal X\to T,\mathcal L)$
is defined as
$(f:\mathcal X\to T,\mathcal L)$
is defined as
 $$ \begin{align*} \lambda_{\mathrm{CM},f,\mathcal L}:=\lambda_{n+1}^{\mu+n(n+1)}\otimes\lambda_n^{-2(n+1)}, \end{align*} $$
$$ \begin{align*} \lambda_{\mathrm{CM},f,\mathcal L}:=\lambda_{n+1}^{\mu+n(n+1)}\otimes\lambda_n^{-2(n+1)}, \end{align*} $$
where
 $\mu =\mu (\mathcal X,\mathcal L):=\frac {2a_1}{a_0}$
. The Chow line bundle is defined as
$\mu =\mu (\mathcal X,\mathcal L):=\frac {2a_1}{a_0}$
. The Chow line bundle is defined as
Let
 $\mathcal D:=\sum _{i=1}^{k} c_i\mathcal D_i$
be a relative Mumford
$\mathcal D:=\sum _{i=1}^{k} c_i\mathcal D_i$
be a relative Mumford
![]() $\mathbb {Q}$
-divisor on
$\mathbb {Q}$
-divisor on
![]() $\mathcal X$
over T, where each
$\mathcal X$
over T, where each
![]() $\mathcal D_i$
is a relative Mumford divisor and
$\mathcal D_i$
is a relative Mumford divisor and
![]() $c_i\in [0,1]\cap \mathbb {Q}$
. We also assume that each
$c_i\in [0,1]\cap \mathbb {Q}$
. We also assume that each
![]() $\mathcal D_i$
is flat over T.
$\mathcal D_i$
is flat over T.
The log CM
![]() $\mathbb {Q}$
-line bundle of the data
$\mathbb {Q}$
-line bundle of the data
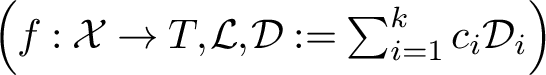 $\left(f:\mathcal X\to T, \mathcal L,\mathcal D:=\sum _{i=1}^k c_i\mathcal D_i\right)$
is defined as
$\left(f:\mathcal X\to T, \mathcal L,\mathcal D:=\sum _{i=1}^k c_i\mathcal D_i\right)$
is defined as
 $$ \begin{align*} \lambda_{\mathrm{CM},f,\mathcal D,\mathcal L}:=\lambda_{\mathrm{CM},f,\mathcal L}-\frac{n\left(\mathcal L_t^{n-1}\cdot\mathcal D_t\right)}{\left(\mathcal L_t^n\right)}\lambda_{\operatorname{Chow},f,\mathcal L}+(n+1)\lambda_{\operatorname{Chow},f\rvert_{\mathcal D},\mathcal L\rvert_{\mathcal D}}, \end{align*} $$
$$ \begin{align*} \lambda_{\mathrm{CM},f,\mathcal D,\mathcal L}:=\lambda_{\mathrm{CM},f,\mathcal L}-\frac{n\left(\mathcal L_t^{n-1}\cdot\mathcal D_t\right)}{\left(\mathcal L_t^n\right)}\lambda_{\operatorname{Chow},f,\mathcal L}+(n+1)\lambda_{\operatorname{Chow},f\rvert_{\mathcal D},\mathcal L\rvert_{\mathcal D}}, \end{align*} $$
where
 $$ \begin{align*} \left(\mathcal L_t^{n-1}\cdot\mathcal D_t\right):=\sum_{i=1}^k c_i \left(\mathcal L_t^{n-1}\cdot\mathcal D_{i,t}\right),\quad \lambda_{\operatorname{Chow},f\rvert_{\mathcal D},\mathcal L\rvert_{\mathcal D}}:=\bigotimes_{i=1}^k\lambda_{\operatorname{Chow},f\rvert_{\mathcal D_i},\mathcal L\rvert_{\mathcal D_i}}^{\otimes c_i}. \end{align*} $$
$$ \begin{align*} \left(\mathcal L_t^{n-1}\cdot\mathcal D_t\right):=\sum_{i=1}^k c_i \left(\mathcal L_t^{n-1}\cdot\mathcal D_{i,t}\right),\quad \lambda_{\operatorname{Chow},f\rvert_{\mathcal D},\mathcal L\rvert_{\mathcal D}}:=\bigotimes_{i=1}^k\lambda_{\operatorname{Chow},f\rvert_{\mathcal D_i},\mathcal L\rvert_{\mathcal D_i}}^{\otimes c_i}. \end{align*} $$
Note that if T is not connected, then we define the log CM line bundle on each connected component of T as in Definition 2.8.
Next, we recall the concept of
![]() $\mathbb {Q}$
-Gorenstein flat families of log Fano pairs over reduced base schemes:
$\mathbb {Q}$
-Gorenstein flat families of log Fano pairs over reduced base schemes:
Definition 2.9. Let T be a reduced scheme. Let
![]() $f:\mathcal X\to T$
be a proper flat morphism with normal, geometrically connected fibers of pure dimension n. Let
$f:\mathcal X\to T$
be a proper flat morphism with normal, geometrically connected fibers of pure dimension n. Let
![]() $\mathcal D$
be an effective relative Mumford
$\mathcal D$
be an effective relative Mumford
![]() $\mathbb {Q}$
-divisor on
$\mathbb {Q}$
-divisor on
![]() $\mathcal X$
over T. We say that
$\mathcal X$
over T. We say that
![]() $f:(\mathcal X,\mathcal D)\to T$
is a
$f:(\mathcal X,\mathcal D)\to T$
is a
![]() $\mathbb {Q}$
-Gorenstein flat family of log Fano pairs if
$\mathbb {Q}$
-Gorenstein flat family of log Fano pairs if
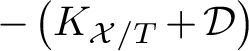 $-\left(K_{\mathcal X/T}+\mathcal D\right)$
is
$-\left(K_{\mathcal X/T}+\mathcal D\right)$
is
![]() $\mathbb {Q}$
-Cartier and f-ample.
$\mathbb {Q}$
-Cartier and f-ample.
We consider the following class of log Fano pairs as objects of our moduli problems:
Definition 2.10. Let
![]() $c,r$
be positive rational numbers such that
$c,r$
be positive rational numbers such that
 $c<\min \left\{1, r^{-1}\right\}$
. A log Fano pair
$c<\min \left\{1, r^{-1}\right\}$
. A log Fano pair
![]() $(X,cD)$
is
$(X,cD)$
is
![]() $\mathbb {Q}$
-Gorenstein smoothable if there exists a
$\mathbb {Q}$
-Gorenstein smoothable if there exists a
![]() $\mathbb {Q}$
-Gorenstein flat family of log Fano pairs
$\mathbb {Q}$
-Gorenstein flat family of log Fano pairs
![]() $\pi :(\mathcal X,c\mathcal D)\to B$
over a pointed smooth curve
$\pi :(\mathcal X,c\mathcal D)\to B$
over a pointed smooth curve
![]() $(0\in B)$
such that the following hold:
$(0\in B)$
such that the following hold:
-
•
 $\mathcal D$
is a relative Mumford divisor over B;
$\mathcal D$
is a relative Mumford divisor over B; -
• both
 $-K_{\mathcal X/B}$
and
$-K_{\mathcal X/B}$
and
 $\mathcal D$
are
$\mathcal D$
are
 $\mathbb {Q}$
-Cartier and
$\mathbb {Q}$
-Cartier and
 $\pi $
-ample, and
$\pi $
-ample, and
 $\mathcal D\sim _{\mathbb {Q},\pi }-rK_{\mathcal X/B}$
;
$\mathcal D\sim _{\mathbb {Q},\pi }-rK_{\mathcal X/B}$
; -
• both
 $\pi $
and
$\pi $
and
 $\pi \rvert _{\mathcal D}$
are smooth morphisms over
$\pi \rvert _{\mathcal D}$
are smooth morphisms over
 $B\setminus \{0\}$
; and
$B\setminus \{0\}$
; and -
•
 $(\mathcal X_0,c\mathcal D_0)\cong (X,cD)$
, and X has klt singularities.
$(\mathcal X_0,c\mathcal D_0)\cong (X,cD)$
, and X has klt singularities.
A
![]() $\mathbb {Q}$
-Gorenstein flat family of log Fano pairs
$\mathbb {Q}$
-Gorenstein flat family of log Fano pairs
![]() $f:(\mathcal X,c\mathcal D)\to T$
over a reduced scheme T is called a
$f:(\mathcal X,c\mathcal D)\to T$
over a reduced scheme T is called a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family if
$\mathbb {Q}$
-Gorenstein smoothable log Fano family if
![]() $\mathcal D$
is a
$\mathcal D$
is a
![]() $\mathbb {Q}$
-Cartier relative Mumford divisor over T, and all fibers of f are
$\mathbb {Q}$
-Cartier relative Mumford divisor over T, and all fibers of f are
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano pairs.
$\mathbb {Q}$
-Gorenstein smoothable log Fano pairs.
Lemma 2.11. For
![]() $c,r\in \mathbb {Q}_{>0}$
with
$c,r\in \mathbb {Q}_{>0}$
with
![]() $cr<1$
, let
$cr<1$
, let
![]() $(\mathcal X,c\mathcal D)\to B$
be a
$(\mathcal X,c\mathcal D)\to B$
be a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family over a smooth curve B, where
$\mathbb {Q}$
-Gorenstein smoothable log Fano family over a smooth curve B, where
![]() $\mathcal D\sim _{\mathbb {Q},B}-rK_{\mathcal X/B}$
. Then the function
$\mathcal D\sim _{\mathbb {Q},B}-rK_{\mathcal X/B}$
. Then the function
![]() $B\ni b\mapsto h^0\left(\mathcal X_b,\mathcal {O}_{\mathcal X_b}(m\mathcal D_b)\right)$
is constant for any
$B\ni b\mapsto h^0\left(\mathcal X_b,\mathcal {O}_{\mathcal X_b}(m\mathcal D_b)\right)$
is constant for any
![]() $m\in \mathbb {Z}$
.
$m\in \mathbb {Z}$
.
Proof. By inversion of adjunction, we know that
![]() $\mathcal X$
has klt singularities. Since
$\mathcal X$
has klt singularities. Since
![]() $\mathcal D$
and
$\mathcal D$
and
![]() $\mathcal D_b$
are
$\mathcal D_b$
are
![]() $\mathbb {Q}$
-Cartier Weil divisors on
$\mathbb {Q}$
-Cartier Weil divisors on
![]() $\mathcal X$
and
$\mathcal X$
and
![]() $\mathcal X_b$
, respectively, we know that both
$\mathcal X_b$
, respectively, we know that both
![]() $\mathcal {O}_{\mathcal X}(m\mathcal D)$
and
$\mathcal {O}_{\mathcal X}(m\mathcal D)$
and
![]() $\mathcal {O}_{\mathcal X_b}(m\mathcal D_b)$
are Cohen–Macaulay for any
$\mathcal {O}_{\mathcal X_b}(m\mathcal D_b)$
are Cohen–Macaulay for any
![]() $m\in \mathbb {Z}$
by [Reference Kollár and Mori40, Corollary 5.25]. Hence
$m\in \mathbb {Z}$
by [Reference Kollár and Mori40, Corollary 5.25]. Hence
![]() $\mathcal {O}_{\mathcal X_b}(m\mathcal D_b)\cong \mathcal {O}_{\mathcal X}(m\mathcal D)\otimes \mathcal {O}_{\mathcal X_b}$
for any
$\mathcal {O}_{\mathcal X_b}(m\mathcal D_b)\cong \mathcal {O}_{\mathcal X}(m\mathcal D)\otimes \mathcal {O}_{\mathcal X_b}$
for any
![]() $m\in \mathbb {Z}$
. By Kawamata–Viehweg vanishing, we know that
$m\in \mathbb {Z}$
. By Kawamata–Viehweg vanishing, we know that
![]() $H^i\left(\mathcal X_b,\mathcal {O}_{\mathcal X_b}(m\mathcal D_b)\right)=0$
for any
$H^i\left(\mathcal X_b,\mathcal {O}_{\mathcal X_b}(m\mathcal D_b)\right)=0$
for any
![]() $b\in B$
and
$b\in B$
and
![]() $m,i\geq 1$
. Hence the statement for
$m,i\geq 1$
. Hence the statement for
![]() $m>0$
follows from the semicontinuity theorem and flatness of
$m>0$
follows from the semicontinuity theorem and flatness of
![]() $\mathcal {O}_{\mathcal X}(m\mathcal D)$
over B, and it is obvious for
$\mathcal {O}_{\mathcal X}(m\mathcal D)$
over B, and it is obvious for
![]() $m\leq 0$
.
$m\leq 0$
.
Proposition 2.12. Let
![]() $f:(\mathcal X,c\mathcal D)\to T$
be a
$f:(\mathcal X,c\mathcal D)\to T$
be a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family over a reduced scheme T. Then
$\mathbb {Q}$
-Gorenstein smoothable log Fano family over a reduced scheme T. Then
![]() $\mathcal D$
is flat over T.
$\mathcal D$
is flat over T.
Proof. For simplicity, we assume that T is connected. By [Reference Kollár37, Theorem 4.33], there exists a locally closed decomposition
![]() $T'\to T$
such that for any morphism
$T'\to T$
such that for any morphism
![]() $q:W\to T$
, the divisorial pullback
$q:W\to T$
, the divisorial pullback
![]() $f_W: (\mathcal X_W, \mathcal D_W)\to W$
of
$f_W: (\mathcal X_W, \mathcal D_W)\to W$
of
![]() $f:(\mathcal X,\mathcal D)\to T$
satisfies that
$f:(\mathcal X,\mathcal D)\to T$
satisfies that
![]() $\mathcal D_W$
is flat over W if and only if q factors as
$\mathcal D_W$
is flat over W if and only if q factors as
![]() $q: W\to T'\to T$
. It is clear that
$q: W\to T'\to T$
. It is clear that
![]() $\mathcal D$
is flat over T if and only if
$\mathcal D$
is flat over T if and only if
![]() $T'=T$
. Thus it suffices to show that for any morphism
$T'=T$
. Thus it suffices to show that for any morphism
![]() $B\to T$
from a smooth curve B, the divisorial pullback
$B\to T$
from a smooth curve B, the divisorial pullback
![]() $f_B:(\mathcal X_B,\mathcal D_B)\to B$
of f satisfies the demand that
$f_B:(\mathcal X_B,\mathcal D_B)\to B$
of f satisfies the demand that
![]() $\mathcal D_B$
be flat over B. It is clear that
$\mathcal D_B$
be flat over B. It is clear that
![]() $f_B$
is also a
$f_B$
is also a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family. By the proof of Lemma 2.11, we know that
$\mathbb {Q}$
-Gorenstein smoothable log Fano family. By the proof of Lemma 2.11, we know that
![]() $\mathcal {O}_{\mathcal X_B}(-\mathcal D_B)$
is flat over B, and its fiber over
$\mathcal {O}_{\mathcal X_B}(-\mathcal D_B)$
is flat over B, and its fiber over
![]() $b\in B$
is isomorphic to
$b\in B$
is isomorphic to
![]() $\mathcal {O}_{\mathcal X_{B,b}}\left(-\mathcal D_{B,b}\right)$
, which is
$\mathcal {O}_{\mathcal X_{B,b}}\left(-\mathcal D_{B,b}\right)$
, which is
![]() $S_2$
. Hence [Reference Kollár37, Definition-Lemma 4.19] implies that
$S_2$
. Hence [Reference Kollár37, Definition-Lemma 4.19] implies that
![]() $\mathcal D_B\to B$
is flat. This finishes the proof.
$\mathcal D_B\to B$
is flat. This finishes the proof.
Definition 2.13. We define the CM
![]() $\mathbb {Q}$
-line bundle of a
$\mathbb {Q}$
-line bundle of a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family
$\mathbb {Q}$
-Gorenstein smoothable log Fano family
![]() $f:(\mathcal X,c\mathcal D)\to T$
over a reduced scheme T to be
$f:(\mathcal X,c\mathcal D)\to T$
over a reduced scheme T to be
![]() $\lambda _{\mathrm {CM},f,c\mathcal D}:=l^{-n}\lambda _{\mathrm {CM},f,c\mathcal D,\mathcal L}$
, where
$\lambda _{\mathrm {CM},f,c\mathcal D}:=l^{-n}\lambda _{\mathrm {CM},f,c\mathcal D,\mathcal L}$
, where
 $\mathcal L:=-l\left(K_{\mathcal X/T}+c\mathcal D\right)$
is an f-ample Cartier divisor on
$\mathcal L:=-l\left(K_{\mathcal X/T}+c\mathcal D\right)$
is an f-ample Cartier divisor on
![]() $\mathcal X$
for some
$\mathcal X$
for some
![]() $l\in \mathbb {Z}_{>0}$
. Here
$l\in \mathbb {Z}_{>0}$
. Here
![]() $\mathcal D$
is flat over T by Proposition 2.12.
$\mathcal D$
is flat over T by Proposition 2.12.
Note that the CM
![]() $\mathbb {Q}$
-line bundle of a
$\mathbb {Q}$
-line bundle of a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family is functorial under reduced base change, by the functoriality of Chow line bundles and
$\mathbb {Q}$
-Gorenstein smoothable log Fano family is functorial under reduced base change, by the functoriality of Chow line bundles and
![]() $\lambda _n$
, according to [Reference Knudsen and Mumford36, Reference Codogni and Patakfalvi17].
$\lambda _n$
, according to [Reference Knudsen and Mumford36, Reference Codogni and Patakfalvi17].
We can now recall the definition of the Hodge line bundle for a smoothable log Calabi–Yau fibration of Fano type. From the definition it is clear that the Hodge line bundle is functorial under reduced base change.
Definition 2.14. For
![]() $c,r\in \mathbb {Q}_{>0}$
with
$c,r\in \mathbb {Q}_{>0}$
with
![]() $cr<1$
, let
$cr<1$
, let
![]() $f:(\mathcal X,c\mathcal D)\to T$
be a
$f:(\mathcal X,c\mathcal D)\to T$
be a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family over a reduced scheme T, where
$\mathbb {Q}$
-Gorenstein smoothable log Fano family over a reduced scheme T, where
![]() $\mathcal D\sim _{\mathbb {Q},f} -rK_{\mathcal X/T}$
. The Hodge
$\mathcal D\sim _{\mathbb {Q},f} -rK_{\mathcal X/T}$
. The Hodge
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
![]() $\lambda _{\mathrm {Hodge},f,r^{-1}\mathcal D}$
is defined as the
$\lambda _{\mathrm {Hodge},f,r^{-1}\mathcal D}$
is defined as the
![]() $\mathbb {Q}$
-linear equivalence class of
$\mathbb {Q}$
-linear equivalence class of
![]() $\mathbb {Q}$
-Cartier
$\mathbb {Q}$
-Cartier
![]() $\mathbb {Q}$
-divisors on T such that
$\mathbb {Q}$
-divisors on T such that
 $$ \begin{align*} K_{\mathcal X/T}+r^{-1}\mathcal D\sim_{\mathbb{Q}}f^*\lambda_{\mathrm{Hodge},f,r^{-1}\mathcal D}. \end{align*} $$
$$ \begin{align*} K_{\mathcal X/T}+r^{-1}\mathcal D\sim_{\mathbb{Q}}f^*\lambda_{\mathrm{Hodge},f,r^{-1}\mathcal D}. \end{align*} $$
The following proposition relates CM
![]() $\mathbb {Q}$
-line bundles and the Hodge
$\mathbb {Q}$
-line bundles and the Hodge
![]() $\mathbb {Q}$
-line bundle:
$\mathbb {Q}$
-line bundle:
Proposition 2.15 [Reference Ascher, DeVleming and Liu6, Proposition 2.25]
With the notation of Definition 2.14, for any rational number
![]() $0\leq c<r^{-1}$
we have
$0\leq c<r^{-1}$
we have
The next criterion is important when checking K-stability in explicit families. It is a partial generalization of [Reference Paul and Tian67, Theorem 1] and [Reference Odaka, Spotti and Sun65, Theorem 3.4].
Theorem 2.16 [Reference Ascher, DeVleming and Liu6, Theorem 2.22]
Let
![]() $f:(\mathcal X,c\mathcal D)\to T$
be a
$f:(\mathcal X,c\mathcal D)\to T$
be a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family over a normal projective variety T. Let G be a reductive group acting on
$\mathbb {Q}$
-Gorenstein smoothable log Fano family over a normal projective variety T. Let G be a reductive group acting on
![]() $\mathcal X$
and T such that
$\mathcal X$
and T such that
![]() $\mathcal D$
is G-invariant and f is G-equivariant. Assume in addition that:
$\mathcal D$
is G-invariant and f is G-equivariant. Assume in addition that:
-
(a) if
 $\mathrm {Aut}(\mathcal X_t,\mathcal D_t)$
is finite for
$\mathrm {Aut}(\mathcal X_t,\mathcal D_t)$
is finite for
 $t\in T$
, then the stabilizer subgroup
$t\in T$
, then the stabilizer subgroup
 $G_t$
is also finite;
$G_t$
is also finite; -
(b) if
 $(\mathcal X_t,\mathcal D_t)\cong \left(\mathcal X_{t'}, \mathcal D_{t'}\right)$
for
$(\mathcal X_t,\mathcal D_t)\cong \left(\mathcal X_{t'}, \mathcal D_{t'}\right)$
for
 $t,t'\in T$
, then
$t,t'\in T$
, then
 $t'\in G\cdot t$
; and
$t'\in G\cdot t$
; and -
(c)
 $\lambda _{\mathrm {CM},f,c\mathcal D}$
is an ample
$\lambda _{\mathrm {CM},f,c\mathcal D}$
is an ample
 $\mathbb {Q}$
-line bundle on T.
$\mathbb {Q}$
-line bundle on T.
Then
![]() $t\in T$
is GIT-(poly/semi)stable with respect to the G-linearized
$t\in T$
is GIT-(poly/semi)stable with respect to the G-linearized
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
![]() $\lambda _{\mathrm {CM},f,c\mathcal D}$
if
$\lambda _{\mathrm {CM},f,c\mathcal D}$
if
![]() $(\mathcal X_t, c\mathcal D_t)$
is a K-(poly/semi)stable log Fano pair.
$(\mathcal X_t, c\mathcal D_t)$
is a K-(poly/semi)stable log Fano pair.
The following proposition provides an intersection formula for log CM line bundles. For the case without divisors this was proven by Paul and Tian [Reference Paul and Tian67]. The current statement follows from [Reference Codogni and Patakfalvi17, Proposition 3.7].
Proposition 2.17 [Reference Ascher, DeVleming and Liu6, Proposition 2.23]
Let
![]() $f:(\mathcal X,c\mathcal D)\to T$
be a
$f:(\mathcal X,c\mathcal D)\to T$
be a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family of relative dimension n over a normal proper variety T. Then
$\mathbb {Q}$
-Gorenstein smoothable log Fano family of relative dimension n over a normal proper variety T. Then
 $$ \begin{align} \mathrm{c}_1\left(\lambda_{\mathrm{CM},f,c\mathcal D}\right)=-f_*\left(\left(-K_{\mathcal X/T}-c\mathcal D\right)^{n+1}\right). \end{align} $$
$$ \begin{align} \mathrm{c}_1\left(\lambda_{\mathrm{CM},f,c\mathcal D}\right)=-f_*\left(\left(-K_{\mathcal X/T}-c\mathcal D\right)^{n+1}\right). \end{align} $$
2.4 K-moduli spaces of log Fano pairs
In this subsection, we gather recent results on the construction of K-moduli spaces of log Fano pairs.
In [Reference Ascher, DeVleming and Liu6], we construct K-moduli stacks (resp., proper good moduli spaces) of
![]() $\mathbb {Q}$
-Gorenstein smoothable K-semistable (resp., K-polystable) log Fano pairs
$\mathbb {Q}$
-Gorenstein smoothable K-semistable (resp., K-polystable) log Fano pairs
![]() $(X,cD)$
, where
$(X,cD)$
, where
![]() $D \sim _{\mathbb {Q}} -rK_X$
and c is a rational number.
$D \sim _{\mathbb {Q}} -rK_X$
and c is a rational number.
Theorem 2.18 [Reference Ascher, DeVleming and Liu6, Theorem 3.1 and Remark 3.25]
Let
![]() $\chi _0$
be the Hilbert polynomial of an anticanonically polarized Fano manifold. Fix
$\chi _0$
be the Hilbert polynomial of an anticanonically polarized Fano manifold. Fix
![]() $r\in \mathbb {Q}_{>0}$
and a rational number
$r\in \mathbb {Q}_{>0}$
and a rational number
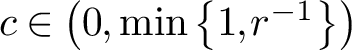 $c\in \left(0,\min \left\{1,r^{-1}\right\}\right)$
. Consider the following moduli pseudo-functor over reduced base S:
$c\in \left(0,\min \left\{1,r^{-1}\right\}\right)$
. Consider the following moduli pseudo-functor over reduced base S:
 $$ \begin{align*} &\mathcal{K}\mathcal M_{\chi_0,r,c}(S)\\ &=\left\{(\mathcal X,\mathcal D)/S\left| \begin{array}{l}(\mathcal X,c\mathcal D)/S\text{ is a }\mathbb{Q}\text{-Gorenstein smoothable log Fano family,}\\ \mathcal D\sim_{S,\mathbb{Q}}-rK_{\mathcal X/S},~\text{each fiber }(\mathcal X_s,c\mathcal D_s) \text{ is K-semistable,}\\ \text{and } \chi\left(\mathcal X_s,\mathcal{O}_{\mathcal X_s}\left(-kK_{\mathcal X_s}\right)\right)=\chi_0(k) \text{ for }k \text{ sufficiently divisible.}\end{array}\right.\right\}. \end{align*} $$
$$ \begin{align*} &\mathcal{K}\mathcal M_{\chi_0,r,c}(S)\\ &=\left\{(\mathcal X,\mathcal D)/S\left| \begin{array}{l}(\mathcal X,c\mathcal D)/S\text{ is a }\mathbb{Q}\text{-Gorenstein smoothable log Fano family,}\\ \mathcal D\sim_{S,\mathbb{Q}}-rK_{\mathcal X/S},~\text{each fiber }(\mathcal X_s,c\mathcal D_s) \text{ is K-semistable,}\\ \text{and } \chi\left(\mathcal X_s,\mathcal{O}_{\mathcal X_s}\left(-kK_{\mathcal X_s}\right)\right)=\chi_0(k) \text{ for }k \text{ sufficiently divisible.}\end{array}\right.\right\}. \end{align*} $$
Then there exists a reduced Artin stack
![]() $\mathcal {K}\mathcal M_{\chi _0,r,c}$
(called a K-moduli stack) of finite type over
$\mathcal {K}\mathcal M_{\chi _0,r,c}$
(called a K-moduli stack) of finite type over
![]() $\mathbb {C}$
representing this moduli pseudo-functor. In particular, the
$\mathbb {C}$
representing this moduli pseudo-functor. In particular, the
![]() $\mathbb {C}$
-points of
$\mathbb {C}$
-points of
![]() $\mathcal {K}\mathcal M_{\chi _0,r,c}$
parametrize K-semistable
$\mathcal {K}\mathcal M_{\chi _0,r,c}$
parametrize K-semistable
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano pairs
$\mathbb {Q}$
-Gorenstein smoothable log Fano pairs
![]() $(X,cD)$
with Hilbert polynomial
$(X,cD)$
with Hilbert polynomial
![]() $\chi (X,\mathcal {O}_X(-mK_X))=\chi _0(m)$
for sufficiently divisible m and
$\chi (X,\mathcal {O}_X(-mK_X))=\chi _0(m)$
for sufficiently divisible m and
![]() $D\sim _{\mathbb {Q}}-rK_X$
.
$D\sim _{\mathbb {Q}}-rK_X$
.
Moreover, the Artin stack
![]() $\mathcal {K}\mathcal M_{\chi _0,r,c}$
admits a good moduli space
$\mathcal {K}\mathcal M_{\chi _0,r,c}$
admits a good moduli space
![]() $KM_{\chi _0,r,c}$
(called a K-moduli space) as a proper reduced scheme of finite type over
$KM_{\chi _0,r,c}$
(called a K-moduli space) as a proper reduced scheme of finite type over
![]() $\mathbb {C}$
, whose closed points parametrize K-polystable log Fano pairs.
$\mathbb {C}$
, whose closed points parametrize K-polystable log Fano pairs.
By [Reference Ascher, DeVleming and Liu6, Proposition 3.35], we know that the universal log Fano family over
![]() $\mathcal {K}\mathcal M_{\chi _0,r,c}$
provides a CM
$\mathcal {K}\mathcal M_{\chi _0,r,c}$
provides a CM
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
![]() $\lambda _c$
and a Hodge
$\lambda _c$
and a Hodge
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
![]() $\lambda _{c,\mathrm {Hodge}}$
over
$\lambda _{c,\mathrm {Hodge}}$
over
![]() $\mathcal {K}\mathcal M_{\chi _0,r,c}$
which descend to
$\mathcal {K}\mathcal M_{\chi _0,r,c}$
which descend to
![]() $\mathbb {Q}$
-line bundles
$\mathbb {Q}$
-line bundles
![]() $\Lambda _c$
and
$\Lambda _c$
and
![]() $\Lambda _{c,\mathrm {Hodge}}$
over the good moduli space
$\Lambda _{c,\mathrm {Hodge}}$
over the good moduli space
![]() $KM_{\chi _0,r,c}$
. Recently, it was shown by [Reference Xu and Zhuang76] on the positivity of the CM that these K-moduli spaces are projective with ample CM
$KM_{\chi _0,r,c}$
. Recently, it was shown by [Reference Xu and Zhuang76] on the positivity of the CM that these K-moduli spaces are projective with ample CM
![]() $\mathbb {Q}$
-line bundles.
$\mathbb {Q}$
-line bundles.
Theorem 2.19 [Reference Xu and Zhuang76, Theorem 7.10]
The CM
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
![]() $\Lambda _c$
over
$\Lambda _c$
over
![]() $KM_{\chi _0,r,c}$
is ample. Hence
$KM_{\chi _0,r,c}$
is ample. Hence
![]() $KM_{\chi _0,r,c}$
is a projective scheme.
$KM_{\chi _0,r,c}$
is a projective scheme.
Remark 2.20. The explicit study of K-moduli originated in [Reference Mabuchi and Mukai60] with the case of degree
![]() $4$
del Pezzo surfaces, and del Pezzo surfaces of other degree were later studied in [Reference Odaka, Spotti and Sun65]. Since then, this area has seen rapid growth (see, e.g., [Reference Spotti and Sun71, Reference Liu and Xu56, Reference Fujita24, Reference Gallardo, Martinez-Garcia and Spotti28, Reference Ascher, DeVleming and Liu6, Reference Liu55]). In all of the aforementioned cases, the smoothable condition was necessary.
$4$
del Pezzo surfaces, and del Pezzo surfaces of other degree were later studied in [Reference Odaka, Spotti and Sun65]. Since then, this area has seen rapid growth (see, e.g., [Reference Spotti and Sun71, Reference Liu and Xu56, Reference Fujita24, Reference Gallardo, Martinez-Garcia and Spotti28, Reference Ascher, DeVleming and Liu6, Reference Liu55]). In all of the aforementioned cases, the smoothable condition was necessary.
If we drop the
![]() $\mathbb {Q}$
-Gorenstein smoothable condition, then K-moduli stacks and spaces of log Fano pairs with fixed numerical conditions (such as volume and finite coefficient set) exist as Artin stacks and projective schemes, respectively. For a precise statement, see, for example, [Reference Xu and Zhuang76, Theorem 2.21] and [Reference Liu, Xu and Zhuang57, Theorem 1.3]. These follow from recent works [Reference Jiang33, Reference Codogni and Patakfalvi17, Reference Blum and Xu10, Reference Alper, Blum, Halpern-Leistner and Xu4, Reference Blum, Liu and Xu12, Reference Xu15, Reference Xu and Zhuang76, Reference Xu and Zhuang77, Reference Blum, Halpern-Leistner, Liu and Xu11, Reference Liu, Xu and Zhuang57]. Since the work establishing the properness of K-moduli spaces [Reference Liu, Xu and Zhuang57] appeared after the first version of this article was posted on arXiv, we restrict our discussion to the smoothable components of K-moduli spaces with reduced structure.
$\mathbb {Q}$
-Gorenstein smoothable condition, then K-moduli stacks and spaces of log Fano pairs with fixed numerical conditions (such as volume and finite coefficient set) exist as Artin stacks and projective schemes, respectively. For a precise statement, see, for example, [Reference Xu and Zhuang76, Theorem 2.21] and [Reference Liu, Xu and Zhuang57, Theorem 1.3]. These follow from recent works [Reference Jiang33, Reference Codogni and Patakfalvi17, Reference Blum and Xu10, Reference Alper, Blum, Halpern-Leistner and Xu4, Reference Blum, Liu and Xu12, Reference Xu15, Reference Xu and Zhuang76, Reference Xu and Zhuang77, Reference Blum, Halpern-Leistner, Liu and Xu11, Reference Liu, Xu and Zhuang57]. Since the work establishing the properness of K-moduli spaces [Reference Liu, Xu and Zhuang57] appeared after the first version of this article was posted on arXiv, we restrict our discussion to the smoothable components of K-moduli spaces with reduced structure.
The following result shows that any K-moduli stack
![]() $\mathcal {K}\mathcal M_{\chi _0,r,c}$
parametrizing
$\mathcal {K}\mathcal M_{\chi _0,r,c}$
parametrizing
![]() $2$
-dimensional
$2$
-dimensional
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano pairs is always smooth. For the special case of plane curves on
$\mathbb {Q}$
-Gorenstein smoothable log Fano pairs is always smooth. For the special case of plane curves on
![]() $\mathbb {P}^2$
, see [Reference Ascher, DeVleming and Liu6, Proposition 4.6].
$\mathbb {P}^2$
, see [Reference Ascher, DeVleming and Liu6, Proposition 4.6].
Theorem 2.21. Let
![]() $\chi _0$
be the Hilbert polynomial of an anticanonically polarized smooth del Pezzo surface. Fix
$\chi _0$
be the Hilbert polynomial of an anticanonically polarized smooth del Pezzo surface. Fix
![]() $r\in \mathbb {Q}_{>0}$
and a rational number
$r\in \mathbb {Q}_{>0}$
and a rational number
 $c\in \left(0,\min \left\{1,r^{-1}\right\}\right)$
. Then the K-moduli stack
$c\in \left(0,\min \left\{1,r^{-1}\right\}\right)$
. Then the K-moduli stack
![]() $\mathcal {K}\mathcal M_{\chi _0,r,c}$
is isomorphic to the quotient stack of a smooth scheme by a projective general linear group. In particular,
$\mathcal {K}\mathcal M_{\chi _0,r,c}$
is isomorphic to the quotient stack of a smooth scheme by a projective general linear group. In particular,
![]() $\mathcal {K}\mathcal M_{\chi _0,r,c}$
is smooth and
$\mathcal {K}\mathcal M_{\chi _0,r,c}$
is smooth and
![]() $KM_{\chi _0,r,c}$
is normal.
$KM_{\chi _0,r,c}$
is normal.
Proof. Fix a sufficiently divisible
![]() $m\in \mathbb {Z}_{>0}$
. Denote
$m\in \mathbb {Z}_{>0}$
. Denote
Recall that in [Reference Ascher, DeVleming and Liu6, Section 3.1], we construct a locally closed subscheme
![]() $Z^{\mathrm {klt}}$
of the relative Hilbert scheme
$Z^{\mathrm {klt}}$
of the relative Hilbert scheme
 $\mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)\times \mathrm {Hilb}_{\tilde {\chi }}\left(\mathbb {P}^{N_m}\right)$
which parametrizes
$\mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)\times \mathrm {Hilb}_{\tilde {\chi }}\left(\mathbb {P}^{N_m}\right)$
which parametrizes
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano pairs
$\mathbb {Q}$
-Gorenstein smoothable log Fano pairs
![]() $(X,cD)$
such that they are embedded into
$(X,cD)$
such that they are embedded into
![]() $\mathbb {P}^{N_m}$
by
$\mathbb {P}^{N_m}$
by
![]() $\lvert -mK_X\rvert $
and X is klt. Denote by Z the dense open subscheme of
$\lvert -mK_X\rvert $
and X is klt. Denote by Z the dense open subscheme of
![]() $Z^{\mathrm {klt}}$
parametrizing
$Z^{\mathrm {klt}}$
parametrizing
![]() $(X,D)$
, where both X and D are smooth. Let
$(X,D)$
, where both X and D are smooth. Let
![]() $Z_c^\circ $
be the Zariski open subset of
$Z_c^\circ $
be the Zariski open subset of
![]() $Z^{\mathrm {klt}}$
parametrizing K-semistable log Fano pairs
$Z^{\mathrm {klt}}$
parametrizing K-semistable log Fano pairs
![]() $(X,cD)$
. Denote by
$(X,cD)$
. Denote by
![]() $Z_c^{\mathrm {red}}$
the reduced scheme supported on
$Z_c^{\mathrm {red}}$
the reduced scheme supported on
![]() $Z_c^\circ $
. Then
$Z_c^\circ $
. Then
![]() $\mathcal {K}\mathcal M_{\chi _0,r,c}$
is defined as the quotient stack
$\mathcal {K}\mathcal M_{\chi _0,r,c}$
is defined as the quotient stack
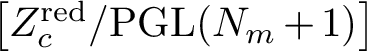 $\left[Z_c^{\mathrm {red}}/\mathrm {PGL}(N_m+1)\right]$
. Hence it suffices to show that
$\left[Z_c^{\mathrm {red}}/\mathrm {PGL}(N_m+1)\right]$
. Hence it suffices to show that
![]() $Z^{\mathrm {klt}}$
is smooth, which would then imply that
$Z^{\mathrm {klt}}$
is smooth, which would then imply that
![]() $Z_c^{\mathrm {red}}$
is smooth. The following argument is inspired by [Reference Ascher, DeVleming and Liu6, Lemma 9.7].
$Z_c^{\mathrm {red}}$
is smooth. The following argument is inspired by [Reference Ascher, DeVleming and Liu6, Lemma 9.7].
Denote by
![]() $Z^{\mathbb {Q}\mathrm {F}}$
the locally closed subscheme of
$Z^{\mathbb {Q}\mathrm {F}}$
the locally closed subscheme of
 $\mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)$
parametrizing
$\mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)$
parametrizing
![]() $\mathbb {Q}$
-Gorenstein smoothable
$\mathbb {Q}$
-Gorenstein smoothable
![]() $\mathbb {Q}$
-Fano varieties X that are embedded into
$\mathbb {Q}$
-Fano varieties X that are embedded into
![]() $\mathbb {P}^{N_m}$
by
$\mathbb {P}^{N_m}$
by
![]() $\lvert -mK_X\rvert $
. Since we are in dimension
$\lvert -mK_X\rvert $
. Since we are in dimension
![]() $2$
, any point
$2$
, any point
![]() $\mathrm {Hilb}(X)\in Z^{\mathbb {Q}\mathrm {F}}$
corresponds to a log del Pezzo surface X with only T-singularities. Hence X has unobstructed
$\mathrm {Hilb}(X)\in Z^{\mathbb {Q}\mathrm {F}}$
corresponds to a log del Pezzo surface X with only T-singularities. Hence X has unobstructed
![]() $\mathbb {Q}$
-Gorenstein deformations by [Reference Hacking29, Theorem 8.2], [Reference Hacking and Prokhorov31, Proposition 3.1], and [Reference Akhtar, Coates, Corti, Heuberger, Kasprzyk, Oneto, Petracci, Prince and Tveiten1, Lemma 6]. Thus
$\mathbb {Q}$
-Gorenstein deformations by [Reference Hacking29, Theorem 8.2], [Reference Hacking and Prokhorov31, Proposition 3.1], and [Reference Akhtar, Coates, Corti, Heuberger, Kasprzyk, Oneto, Petracci, Prince and Tveiten1, Lemma 6]. Thus
![]() $Z^{\mathbb {Q}\mathrm {F}}$
is a smooth scheme. Denote by
$Z^{\mathbb {Q}\mathrm {F}}$
is a smooth scheme. Denote by
![]() $Z^{\textrm {sm}}$
the Zariski open subset of
$Z^{\textrm {sm}}$
the Zariski open subset of
![]() $Z^{\mathbb {Q}\mathrm {F}}$
parametrizing smooth Fano manifolds X such that there exists a smooth divisor
$Z^{\mathbb {Q}\mathrm {F}}$
parametrizing smooth Fano manifolds X such that there exists a smooth divisor
![]() $D\sim _{\mathbb {Q}}-rK_X$
. The openness of
$D\sim _{\mathbb {Q}}-rK_X$
. The openness of
![]() $Z^{\textrm {sm}}$
follows from the openness of smoothness,
$Z^{\textrm {sm}}$
follows from the openness of smoothness,
![]() $H^0(X,\mathcal {O}_X(D))$
being constant since
$H^0(X,\mathcal {O}_X(D))$
being constant since
![]() $H^i(X,\mathcal {O}_X(D))=0$
for
$H^i(X,\mathcal {O}_X(D))=0$
for
![]() $i \geq 1$
by Kodaira vanishing, and the fact that smooth families of Fano manifolds have locally constant Picard groups. Denote
$i \geq 1$
by Kodaira vanishing, and the fact that smooth families of Fano manifolds have locally constant Picard groups. Denote
![]() $Z^{\mathrm {bs}}:=\overline {Z^{\textrm {sm}}}\cap Z^{\mathbb {Q}\mathrm {F}}$
. Hence
$Z^{\mathrm {bs}}:=\overline {Z^{\textrm {sm}}}\cap Z^{\mathbb {Q}\mathrm {F}}$
. Hence
![]() $Z^{\mathrm {bs}}$
is the disjoint union of some connected components of
$Z^{\mathrm {bs}}$
is the disjoint union of some connected components of
![]() $Z^{\mathbb {Q}\mathrm {F}}$
. Denote the first projection by
$Z^{\mathbb {Q}\mathrm {F}}$
. Denote the first projection by
 $\mathrm {pr}_1: Z^{\mathrm {klt}}\to \mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)$
. Clearly
$\mathrm {pr}_1: Z^{\mathrm {klt}}\to \mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)$
. Clearly
 $\mathrm {pr}_1\left(Z^{\mathrm {klt}}\right)$
is contained in
$\mathrm {pr}_1\left(Z^{\mathrm {klt}}\right)$
is contained in
![]() $Z^{\mathbb {Q}\mathrm {F}}$
. We claim that
$Z^{\mathbb {Q}\mathrm {F}}$
. We claim that
 $\mathrm {pr}_1\left(Z^{\mathrm {klt}}\right)=Z^{\mathrm {bs}}$
, and that the restriction morphism
$\mathrm {pr}_1\left(Z^{\mathrm {klt}}\right)=Z^{\mathrm {bs}}$
, and that the restriction morphism
![]() $\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
is proper and smooth.
$\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
is proper and smooth.
We first show that
 $\mathrm {pr}_1\left(Z^{\mathrm {klt}}\right)=Z^{\mathrm {bs}}$
and
$\mathrm {pr}_1\left(Z^{\mathrm {klt}}\right)=Z^{\mathrm {bs}}$
and
![]() $\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
is proper. Since Z is a dense open subset of
$\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
is proper. Since Z is a dense open subset of
![]() $Z^{\mathrm {klt}}$
, we know that
$Z^{\mathrm {klt}}$
, we know that
 $$ \begin{align*} Z^{\textrm{sm}}=\mathrm{pr}_1(Z)\subset\mathrm{pr}_1\left(Z^{\mathrm{klt}}\right)\subset \overline{\mathrm{pr}_1(Z)}\cap Z^{\mathbb{Q}\mathrm{F}}=\overline{Z^{\textrm{sm}}}\cap Z^{\mathbb{Q}\mathrm{F}}=Z^{\mathrm{bs}}. \end{align*} $$
$$ \begin{align*} Z^{\textrm{sm}}=\mathrm{pr}_1(Z)\subset\mathrm{pr}_1\left(Z^{\mathrm{klt}}\right)\subset \overline{\mathrm{pr}_1(Z)}\cap Z^{\mathbb{Q}\mathrm{F}}=\overline{Z^{\textrm{sm}}}\cap Z^{\mathbb{Q}\mathrm{F}}=Z^{\mathrm{bs}}. \end{align*} $$
Hence the surjectivity of
![]() $\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
would follow from its properness. We will verify properness by checking the existence part of the valuative criterion. Let
$\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
would follow from its properness. We will verify properness by checking the existence part of the valuative criterion. Let
![]() $0\in B$
be a pointed curve with
$0\in B$
be a pointed curve with
![]() $B^\circ :=B\setminus \{0\}$
. Consider two morphisms
$B^\circ :=B\setminus \{0\}$
. Consider two morphisms
![]() $f^\circ : B^\circ \to Z^{\mathrm {klt}}$
and
$f^\circ : B^\circ \to Z^{\mathrm {klt}}$
and
![]() $g:B\to Z^{\mathbb {Q}\mathrm {F}}$
such that
$g:B\to Z^{\mathbb {Q}\mathrm {F}}$
such that
![]() $g\rvert _{B^\circ }=\mathrm {pr}_1\circ f^\circ $
. It suffices to show that
$g\rvert _{B^\circ }=\mathrm {pr}_1\circ f^\circ $
. It suffices to show that
![]() $f^\circ $
extends to
$f^\circ $
extends to
![]() $f:B\to Z^{\mathrm {klt}}$
such that
$f:B\to Z^{\mathrm {klt}}$
such that
![]() $g=\mathrm {pr}_1\circ f$
. We have a
$g=\mathrm {pr}_1\circ f$
. We have a
![]() $\mathbb {Q}$
-Gorenstein smoothable family
$\mathbb {Q}$
-Gorenstein smoothable family
![]() $p:\mathcal X\to B$
induced by g, and a
$p:\mathcal X\to B$
induced by g, and a
![]() $\mathbb {Q}$
-Cartier Weil divisor
$\mathbb {Q}$
-Cartier Weil divisor
![]() $\mathcal D^\circ $
on
$\mathcal D^\circ $
on
![]() $\mathcal X^\circ :=p^{-1}(B^\circ )$
induced by
$\mathcal X^\circ :=p^{-1}(B^\circ )$
induced by
![]() $f^\circ $
whose support does not contain any fiber
$f^\circ $
whose support does not contain any fiber
![]() $\mathcal X_b$
, and
$\mathcal X_b$
, and
![]() $\mathcal D^\circ \sim _{\mathbb {Q},B^\circ }-rK_{\mathcal X^\circ /B^\circ }$
. We define
$\mathcal D^\circ \sim _{\mathbb {Q},B^\circ }-rK_{\mathcal X^\circ /B^\circ }$
. We define
![]() $\mathcal D:=\overline {\mathcal D^\circ }$
. Then, by taking Zariski closure, it is clear that
$\mathcal D:=\overline {\mathcal D^\circ }$
. Then, by taking Zariski closure, it is clear that
![]() $\mathcal D\sim _{\mathbb {Q}, B}-rK_{\mathcal X/B}$
, since
$\mathcal D\sim _{\mathbb {Q}, B}-rK_{\mathcal X/B}$
, since
![]() $\mathcal X_0$
is a
$\mathcal X_0$
is a
![]() $\pi $
-linearly trivial Cartier prime divisor on
$\pi $
-linearly trivial Cartier prime divisor on
![]() $\mathcal X$
. Thus
$\mathcal X$
. Thus
![]() $(\mathcal X,\mathcal D)\to B$
is a
$(\mathcal X,\mathcal D)\to B$
is a
![]() $\mathbb {Q}$
-Gorenstein smoothable log Fano family. This finishes proving the properness and surjectivity of
$\mathbb {Q}$
-Gorenstein smoothable log Fano family. This finishes proving the properness and surjectivity of
![]() $\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
.
$\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
.
Finally, we will show that
![]() $\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
is a smooth morphism. Indeed, we will show that it is a smooth
$\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
is a smooth morphism. Indeed, we will show that it is a smooth
![]() $\mathbb {P}^{N_r}$
-fibration, where
$\mathbb {P}^{N_r}$
-fibration, where
![]() $N_r:=\chi _0(r)-1$
. If
$N_r:=\chi _0(r)-1$
. If
![]() $\mathrm {Hilb}(X,D)\in \mathrm {pr}_1^{-1}(Z^{\textrm {sm}})$
, then we know that
$\mathrm {Hilb}(X,D)\in \mathrm {pr}_1^{-1}(Z^{\textrm {sm}})$
, then we know that
![]() $h^0(X,\mathcal {O}_X(D))=\chi (X,\mathcal {O}_X(D))=\chi _0(r)$
, since
$h^0(X,\mathcal {O}_X(D))=\chi (X,\mathcal {O}_X(D))=\chi _0(r)$
, since
![]() $H^i(X,\mathcal {O}_X(D))=0$
for any
$H^i(X,\mathcal {O}_X(D))=0$
for any
![]() $i\geq 1$
by Kodaira vanishing. Hence the fiber over
$i\geq 1$
by Kodaira vanishing. Hence the fiber over
![]() $\mathrm {Hilb}(X)\in Z^{\textrm {sm}}$
is isomorphic to
$\mathrm {Hilb}(X)\in Z^{\textrm {sm}}$
is isomorphic to
![]() $\mathbb {P}(H^0(X,\mathcal {O}_X(D)))\cong \mathbb {P}^{N_r}$
. Hence we may restrict to the case when
$\mathbb {P}(H^0(X,\mathcal {O}_X(D)))\cong \mathbb {P}^{N_r}$
. Hence we may restrict to the case when
![]() $\mathrm {Hilb}(X)\in Z^{\mathrm {bs}}\setminus Z^{\textrm {sm}}$
. Assume that
$\mathrm {Hilb}(X)\in Z^{\mathrm {bs}}\setminus Z^{\textrm {sm}}$
. Assume that
![]() $(X,D)\in Z^{\mathrm {klt}}$
is
$(X,D)\in Z^{\mathrm {klt}}$
is
![]() $\mathbb {Q}$
-Gorenstein smoothable where
$\mathbb {Q}$
-Gorenstein smoothable where
![]() $\pi : (\mathcal X,\mathcal D)\to B$
is a
$\pi : (\mathcal X,\mathcal D)\to B$
is a
![]() $\mathbb {Q}$
-Gorenstein smoothing over a pointed curve
$\mathbb {Q}$
-Gorenstein smoothing over a pointed curve
![]() $0\in B$
with
$0\in B$
with
![]() $(\mathcal X_0,\mathcal D_0)\cong (X,D)$
. Then by Lemma 2.11, we know that
$(\mathcal X_0,\mathcal D_0)\cong (X,D)$
. Then by Lemma 2.11, we know that
![]() $\pi _*\mathcal {O}_{\mathcal X}(\mathcal D)$
is locally free with fiber over
$\pi _*\mathcal {O}_{\mathcal X}(\mathcal D)$
is locally free with fiber over
![]() $b\in B$
isomorphic to
$b\in B$
isomorphic to
![]() $H^0\left(\mathcal X_b,\mathcal {O}_{\mathcal X_b}(\mathcal D_b)\right)$
. Hence it is easy to conclude that for any effective Weil divisor
$H^0\left(\mathcal X_b,\mathcal {O}_{\mathcal X_b}(\mathcal D_b)\right)$
. Hence it is easy to conclude that for any effective Weil divisor
![]() $D'\sim D$
, the pair
$D'\sim D$
, the pair
![]() $(X,D')$
is also
$(X,D')$
is also
![]() $\mathbb {Q}$
-Gorenstein smoothable. Since the Weil divisor class group
$\mathbb {Q}$
-Gorenstein smoothable. Since the Weil divisor class group
![]() $\mathrm {Cl}(X)$
of X is finitely generated, we know that there are only finitely many Weil divisor classes
$\mathrm {Cl}(X)$
of X is finitely generated, we know that there are only finitely many Weil divisor classes
![]() $[D]$
such that
$[D]$
such that
![]() $[D]= -r[K_X]$
in
$[D]= -r[K_X]$
in
![]() $\mathrm {Cl}(X)\otimes _{\mathbb {Z}} \mathbb {Q}$
. Hence the fiber
$\mathrm {Cl}(X)\otimes _{\mathbb {Z}} \mathbb {Q}$
. Hence the fiber
![]() $\mathrm {pr}_1^{-1}(\mathrm {Hilb}(X))$
is isomorphic to a disjoint union of finitely many copies of
$\mathrm {pr}_1^{-1}(\mathrm {Hilb}(X))$
is isomorphic to a disjoint union of finitely many copies of
![]() $\mathbb {P}^{N_r}$
. However, since
$\mathbb {P}^{N_r}$
. However, since
![]() $\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
is proper with connected fibers over a dense open subset
$\mathrm {pr}_1:Z^{\mathrm {klt}}\to Z^{\mathrm {bs}}$
is proper with connected fibers over a dense open subset
![]() $Z^{\textrm {sm}}$
and
$Z^{\textrm {sm}}$
and
![]() $Z^{\mathrm {bs}}$
is normal, taking Stein factorization yields that
$Z^{\mathrm {bs}}$
is normal, taking Stein factorization yields that
![]() $\mathrm {pr}_1$
has connected fibers everywhere. Hence
$\mathrm {pr}_1$
has connected fibers everywhere. Hence
![]() $\mathrm {pr}_1^{-1}(\mathrm {Hilb}(X))\cong \mathbb {P}^{N_r}$
for any
$\mathrm {pr}_1^{-1}(\mathrm {Hilb}(X))\cong \mathbb {P}^{N_r}$
for any
![]() $\mathrm {Hilb}(X)\in Z^{\mathrm {bs}}$
. Therefore,
$\mathrm {Hilb}(X)\in Z^{\mathrm {bs}}$
. Therefore,
![]() $\mathrm {pr}_1$
has smooth fibers and smooth base, which implies that
$\mathrm {pr}_1$
has smooth fibers and smooth base, which implies that
![]() $Z^{\mathrm {klt}}$
is Cohen–Macaulay. Hence, miracle flatness implies that
$Z^{\mathrm {klt}}$
is Cohen–Macaulay. Hence, miracle flatness implies that
![]() $\mathrm {pr}_1$
is flat and hence smooth. The proof is finished.
$\mathrm {pr}_1$
is flat and hence smooth. The proof is finished.
3 Overview of previous results, Laza–O’Grady, and VGIT
We refer the reader to [Reference Laza and O’Grady42, Reference Laza and O’Grady44, Reference Laza and O’Grady43] for more details.
3.1 Hyperelliptic K3 surfaces of degree
 $4$
$4$
A K3 surface X is a connected projective surface with Du Val singularities such that
![]() $\omega _X\cong \mathcal {O}_X$
and
$\omega _X\cong \mathcal {O}_X$
and
![]() $H^1(X,\mathcal {O}_X)=0$
. A K3 surface X together with an ample line bundle L on X is called a polarized K3 surface
$H^1(X,\mathcal {O}_X)=0$
. A K3 surface X together with an ample line bundle L on X is called a polarized K3 surface
![]() $(X,L)$
of degree
$(X,L)$
of degree
 $\left(L^2\right)$
. A polarized K3 surface
$\left(L^2\right)$
. A polarized K3 surface
![]() $(X,L)$
is hyperelliptic if the map
$(X,L)$
is hyperelliptic if the map
![]() $\varphi _L: X \dashrightarrow \lvert L\rvert ^\vee $
is regular, and is a double cover of its image. All hyperelliptic quartic K3 surfaces are obtained by the following procedure (see [Reference Laza and O’Grady42, Remark 2.1.3]): Consider a normal quadric surface
$\varphi _L: X \dashrightarrow \lvert L\rvert ^\vee $
is regular, and is a double cover of its image. All hyperelliptic quartic K3 surfaces are obtained by the following procedure (see [Reference Laza and O’Grady42, Remark 2.1.3]): Consider a normal quadric surface
![]() $Q \subset \mathbb {P}^3$
, and
$Q \subset \mathbb {P}^3$
, and
 $B \in \left\lvert \omega _Q^{-2}\right\rvert $
with ADE singularities (in particular, GIT stable when
$B \in \left\lvert \omega _Q^{-2}\right\rvert $
with ADE singularities (in particular, GIT stable when
![]() $Q\cong \mathbb {P}^1\times \mathbb {P}^1$
). Then the double cover
$Q\cong \mathbb {P}^1\times \mathbb {P}^1$
). Then the double cover
![]() $\pi : X \to Q$
ramified over B is a hyperelliptic quartic K3 with polarization
$\pi : X \to Q$
ramified over B is a hyperelliptic quartic K3 with polarization
![]() $L = \pi ^*\mathcal {O}_{Q}(1)$
and at worst ADE singularities.
$L = \pi ^*\mathcal {O}_{Q}(1)$
and at worst ADE singularities.
Given a smooth
![]() $(4,4)$
curve C on
$(4,4)$
curve C on
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
, the double cover
$\mathbb {P}^1 \times \mathbb {P}^1$
, the double cover
![]() $\pi : X_C \to \mathbb {P}^1 \times \mathbb {P}^1$
ramified over C is a hyperelliptic polarized K3 surface of degree
$\pi : X_C \to \mathbb {P}^1 \times \mathbb {P}^1$
ramified over C is a hyperelliptic polarized K3 surface of degree
![]() $4$
. The polarization is given by
$4$
. The polarization is given by
![]() $L_C = \pi ^*\mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(1,1)$
. One can ask how the GIT moduli space of
$L_C = \pi ^*\mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(1,1)$
. One can ask how the GIT moduli space of
![]() $(4,4)$
curves on
$(4,4)$
curves on
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
compares to the moduli space of hyperelliptic K3 surfaces of degree
$\mathbb {P}^1 \times \mathbb {P}^1$
compares to the moduli space of hyperelliptic K3 surfaces of degree
![]() $4$
constructed via periods.
$4$
constructed via periods.
3.2 Moduli of K3 surfaces
Let
![]() $\Lambda $
be the lattice
$\Lambda $
be the lattice
![]() $U^2 \oplus D_{16}$
, where U is the hyperbolic plane and
$U^2 \oplus D_{16}$
, where U is the hyperbolic plane and
![]() $D_{16}$
is the negative definite lattice corresponding to Dynkin diagram
$D_{16}$
is the negative definite lattice corresponding to Dynkin diagram
![]() $D_{16}$
. Let
$D_{16}$
. Let
 $\mathscr {D} = \left\{ \lvert \sigma \rvert \in \mathbb {P}(\Lambda \otimes \mathbb {C}) \mid \sigma ^2 = 0, (\sigma + \overline {\sigma })^2> 0\right\}.$
The connected component
$\mathscr {D} = \left\{ \lvert \sigma \rvert \in \mathbb {P}(\Lambda \otimes \mathbb {C}) \mid \sigma ^2 = 0, (\sigma + \overline {\sigma })^2> 0\right\}.$
The connected component
![]() $\mathscr {D}^+$
is a type IV bounded symmetric domain. Let
$\mathscr {D}^+$
is a type IV bounded symmetric domain. Let
![]() $\Gamma (\Lambda ) = O^+(\Lambda ) < O(\Lambda )$
be the index
$\Gamma (\Lambda ) = O^+(\Lambda ) < O(\Lambda )$
be the index
![]() $2$
subgroup mapping
$2$
subgroup mapping
![]() $\mathscr {D}^+$
to itself. We define the locally symmetric variety
$\mathscr {D}^+$
to itself. We define the locally symmetric variety
![]() $\mathscr {F} = \Gamma \setminus \mathscr {D}^+$
, and we let
$\mathscr {F} = \Gamma \setminus \mathscr {D}^+$
, and we let
![]() $\mathscr {F} \subset \mathscr {F}^*$
be its Baily–Borel compactification (see [Reference Laza and Zhang45, Section 3.1]).
$\mathscr {F} \subset \mathscr {F}^*$
be its Baily–Borel compactification (see [Reference Laza and Zhang45, Section 3.1]).
It turns out that
![]() $\mathscr {F}$
can be identified as the period space for hyperelliptic quartic K3 surfaces (see [Reference Laza and O’Grady42, Remark 2.2.4]). The rough idea is that
$\mathscr {F}$
can be identified as the period space for hyperelliptic quartic K3 surfaces (see [Reference Laza and O’Grady42, Remark 2.2.4]). The rough idea is that
![]() $\mathscr {F}$
sits inside a larger period domain
$\mathscr {F}$
sits inside a larger period domain
![]() $\mathscr {F}^\prime $
which serves as a moduli space for quartic K3 surfaces, and
$\mathscr {F}^\prime $
which serves as a moduli space for quartic K3 surfaces, and
![]() $\mathscr {F}$
is naturally isomorphic to a divisor in
$\mathscr {F}$
is naturally isomorphic to a divisor in
![]() $\mathscr {F}^\prime $
whose points correspond to the periods of the hyperelliptic K3 surfaces.
$\mathscr {F}^\prime $
whose points correspond to the periods of the hyperelliptic K3 surfaces.
Let
![]() $\mathfrak {M}$
denote the GIT moduli space of
$\mathfrak {M}$
denote the GIT moduli space of
![]() $(4,4)$
curves on
$(4,4)$
curves on
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
. Shah proved that
$\mathbb {P}^1 \times \mathbb {P}^1$
. Shah proved that
![]() $(4,4)$
curves with ADE singularities are GIT-stable, and by associating to C the corresponding period point of the K3 surface, one obtains a rational period map
$(4,4)$
curves with ADE singularities are GIT-stable, and by associating to C the corresponding period point of the K3 surface, one obtains a rational period map
![]() $\mathfrak {p}: \mathfrak {M} \dashrightarrow \mathscr {F}^*$
([Reference Shah69, Theorem 4.8]). By the global Torelli theorem, the period map
$\mathfrak {p}: \mathfrak {M} \dashrightarrow \mathscr {F}^*$
([Reference Shah69, Theorem 4.8]). By the global Torelli theorem, the period map
![]() $\mathfrak {p}$
is actually birational. Laza and O’Grady showed that the indeterminacy locus of
$\mathfrak {p}$
is actually birational. Laza and O’Grady showed that the indeterminacy locus of
![]() $\mathfrak {p}$
is a subset of
$\mathfrak {p}$
is a subset of
![]() $\mathfrak {M}$
of dimension
$\mathfrak {M}$
of dimension
![]() $7$
(see, e.g., [Reference Laza and O’Grady43, Corollary 4.10]). The goal of their work is to describe this birational map explicitly, as a series of flips and divisorial contractions.
$7$
(see, e.g., [Reference Laza and O’Grady43, Corollary 4.10]). The goal of their work is to describe this birational map explicitly, as a series of flips and divisorial contractions.
The intersection of
![]() $\mathscr {F}$
and the image of the regular locus of
$\mathscr {F}$
and the image of the regular locus of
![]() $\mathfrak {p}$
is
$\mathfrak {p}$
is
![]() $\mathscr {F} \setminus H_h$
, where
$\mathscr {F} \setminus H_h$
, where
![]() $H_h$
is a Heegner divisor. Geometrically, it parametrizes periods of hyperelliptic K3 surfaces which are double covers of a quadric cone, and is defined as follows: The vector
$H_h$
is a Heegner divisor. Geometrically, it parametrizes periods of hyperelliptic K3 surfaces which are double covers of a quadric cone, and is defined as follows: The vector
![]() $w \in \Lambda $
is hyperbolic if
$w \in \Lambda $
is hyperbolic if
![]() $w^2 = -4$
and the divisibility
$w^2 = -4$
and the divisibility
![]() $div(w) = 2$
(the positive generator of
$div(w) = 2$
(the positive generator of
![]() $(w, \Lambda )$
). The Heegner divisor
$(w, \Lambda )$
). The Heegner divisor
![]() $H_h \subset \mathscr {F}$
is the locus of
$H_h \subset \mathscr {F}$
is the locus of
![]() $O^+(\Lambda )$
-equivalence classes of points
$O^+(\Lambda )$
-equivalence classes of points
![]() $[\sigma ] \in \mathscr {D}^+$
such that
$[\sigma ] \in \mathscr {D}^+$
such that
![]() $\sigma ^\perp $
contains a hyperbolic vector.
$\sigma ^\perp $
contains a hyperbolic vector.
3.3 Results of Laza and O’Grady and VGIT for (2,4)-complete intersections in
 $\mathbb {P}^3$
$\mathbb {P}^3$
As mentioned in the introduction, Laza and O’Grady propose a conjectural framework to interpolate between GIT and Baily–Borel compactifications of moduli spaces which are type IV locally symmetric domains
![]() $\mathscr {F}(N)$
associated to lattices of the form
$\mathscr {F}(N)$
associated to lattices of the form
![]() $U^2 \oplus D_{N_2}$
(see [Reference Laza and O’Grady42]). These include, for example, K3 surfaces of degree
$U^2 \oplus D_{N_2}$
(see [Reference Laza and O’Grady42]). These include, for example, K3 surfaces of degree
![]() $4$
(
$4$
(
![]() $N = 19$
) and EPW sextics (
$N = 19$
) and EPW sextics (
![]() $N = 20$
).
$N = 20$
).
Let
![]() $\lambda (N)$
denote the Hodge line bundle on
$\lambda (N)$
denote the Hodge line bundle on
![]() $\mathscr {F}(N)$
and let
$\mathscr {F}(N)$
and let
![]() $\Delta (N)$
denote a geometrically meaningful (e.g., Noether–Lefschetz) divisor. By the work of Baily and Borel, the compact space
$\Delta (N)$
denote a geometrically meaningful (e.g., Noether–Lefschetz) divisor. By the work of Baily and Borel, the compact space
![]() $\mathscr {F}^*(N)$
can always be identified with
$\mathscr {F}^*(N)$
can always be identified with
![]() $\mathrm {Proj} R(\mathscr {F}(N), \lambda (N))$
. Moreover, Looijenga showed that
$\mathrm {Proj} R(\mathscr {F}(N), \lambda (N))$
. Moreover, Looijenga showed that
![]() $\mathrm {Proj} R(\mathscr {F}(N), \lambda (N) + \Delta (N))$
can often be identified with
$\mathrm {Proj} R(\mathscr {F}(N), \lambda (N) + \Delta (N))$
can often be identified with
![]() $\mathfrak {M}$
. The main prediction of Laza and O’Grady is that the ring of sections
$\mathfrak {M}$
. The main prediction of Laza and O’Grady is that the ring of sections
![]() $R(\mathscr {F}(N), \lambda (N) + \beta \Delta (N))$
is finitely generated for
$R(\mathscr {F}(N), \lambda (N) + \beta \Delta (N))$
is finitely generated for
![]() $\beta \in [0, 1] \cap \mathbb {Q}$
. Moreover, they give a prediction for the ‘walls’ where the moduli spaces change, thus predicting a natural interpolation between
$\beta \in [0, 1] \cap \mathbb {Q}$
. Moreover, they give a prediction for the ‘walls’ where the moduli spaces change, thus predicting a natural interpolation between
![]() $\mathscr {F}^*$
and
$\mathscr {F}^*$
and
![]() $\mathfrak {M}$
.
$\mathfrak {M}$
.
From now on, we restrict to the case where
![]() $N=18$
– that is, the case of hyperelliptic quartic K3 surfaces. In this case, if
$N=18$
– that is, the case of hyperelliptic quartic K3 surfaces. In this case, if
![]() $\Delta = H_h /2$
(introduced in §3.2), then it was shown in [Reference Laza and O’Grady42] that
$\Delta = H_h /2$
(introduced in §3.2), then it was shown in [Reference Laza and O’Grady42] that
![]() $\mathfrak {M} \cong \mathrm {Proj} R(\mathscr {F}, \lambda + \Delta )$
. Let
$\mathfrak {M} \cong \mathrm {Proj} R(\mathscr {F}, \lambda + \Delta )$
. Let
![]() $\beta \in [0,1] \cap \mathbb {Q}$
. In [Reference Laza and O’Grady43], Laza and O’Grady prove that the ring of sections
$\beta \in [0,1] \cap \mathbb {Q}$
. In [Reference Laza and O’Grady43], Laza and O’Grady prove that the ring of sections
![]() $R(\mathscr {F}, \lambda + \beta \Delta )$
is finitely generated, and therefore
$R(\mathscr {F}, \lambda + \beta \Delta )$
is finitely generated, and therefore
![]() $\mathscr {F}(\beta ) = \mathrm {Proj} R(\mathscr {F}, \lambda + \beta \Delta )$
can be viewed as a projective variety interpolating between the GIT and Baily–Borel moduli spaces. Moreover, they calculate the set of critical values, and show that the birational period map is the composition of explicitly understood divisorial contractions and flips. In fact, they show that the intermediate spaces arise from variation of GIT (VGIT). They also show that the first step in their program produces
$\mathscr {F}(\beta ) = \mathrm {Proj} R(\mathscr {F}, \lambda + \beta \Delta )$
can be viewed as a projective variety interpolating between the GIT and Baily–Borel moduli spaces. Moreover, they calculate the set of critical values, and show that the birational period map is the composition of explicitly understood divisorial contractions and flips. In fact, they show that the intermediate spaces arise from variation of GIT (VGIT). They also show that the first step in their program produces
 $\widehat {\mathscr {F}}=\mathscr {F}(\epsilon )\to \mathscr {F}^*$
as the
$\widehat {\mathscr {F}}=\mathscr {F}(\epsilon )\to \mathscr {F}^*$
as the
![]() $\mathbb {Q}$
-Cartierization of
$\mathbb {Q}$
-Cartierization of
![]() $H_h \subset \mathscr {F}^*$
for
$H_h \subset \mathscr {F}^*$
for
![]() $0<\epsilon \ll 1$
. In particular, this gives a small partial resolution
$0<\epsilon \ll 1$
. In particular, this gives a small partial resolution
![]() $\widehat {\mathscr {F}}$
of
$\widehat {\mathscr {F}}$
of
![]() $\mathscr {F}^*$
which parametrizes hyperelliptic quartic K3 surfaces with slc singularities. In what follows, we review VGIT and their results in further detail.
$\mathscr {F}^*$
which parametrizes hyperelliptic quartic K3 surfaces with slc singularities. In what follows, we review VGIT and their results in further detail.
We now introduce the VGIT
![]() $\mathscr {M}(t)$
, largely modeled on [Reference Laza and O’Grady43, Section 5]. A smooth
$\mathscr {M}(t)$
, largely modeled on [Reference Laza and O’Grady43, Section 5]. A smooth
![]() $(2,4)$
-complete intersection inside
$(2,4)$
-complete intersection inside
![]() $\mathbb {P}^3$
determines
$\mathbb {P}^3$
determines
![]() $X_C$
, a smooth hyperelliptic K3 of degree
$X_C$
, a smooth hyperelliptic K3 of degree
![]() $4$
. Let U be the parameter space for all
$4$
. Let U be the parameter space for all
![]() $(2,4)$
-complete intersection closed subschemes in
$(2,4)$
-complete intersection closed subschemes in
![]() $\mathbb {P}^3$
. Then U has a natural action of
$\mathbb {P}^3$
. Then U has a natural action of
![]() $\mathrm {SL}(4)$
, though we note that U is not projective. We let E be the vector bundle over
$\mathrm {SL}(4)$
, though we note that U is not projective. We let E be the vector bundle over
![]() $\lvert \mathcal {O}_{\mathbb {P}^3}(2)\rvert $
whose fiber over
$\lvert \mathcal {O}_{\mathbb {P}^3}(2)\rvert $
whose fiber over
![]() $Q \in \lvert \mathcal {O}_{\mathbb {P}^3}(2)\rvert $
is given by
$Q \in \lvert \mathcal {O}_{\mathbb {P}^3}(2)\rvert $
is given by
![]() $H^0\left(Q, \mathcal {O}_Q(4)\right)$
. Then
$H^0\left(Q, \mathcal {O}_Q(4)\right)$
. Then
![]() $U \subseteq \mathbb {P}(E)$
and
$U \subseteq \mathbb {P}(E)$
and
![]() $\textrm {codim}_{\mathbb {P}(E)}\mathbb {P}(E) \setminus U \geq 2$
.
$\textrm {codim}_{\mathbb {P}(E)}\mathbb {P}(E) \setminus U \geq 2$
.
There is a map
![]() $\mathrm {chow}: U \to \operatorname {Chow}$
to the Chow variety parametrizing
$\mathrm {chow}: U \to \operatorname {Chow}$
to the Chow variety parametrizing
![]() $1$
-dimensional cycles inside
$1$
-dimensional cycles inside
![]() $\mathbb {P}^3$
. We denote by
$\mathbb {P}^3$
. We denote by
![]() $\operatorname {Chow}_{\left(2,4\right)}$
the closure of the image of
$\operatorname {Chow}_{\left(2,4\right)}$
the closure of the image of
![]() $\mathrm {chow}$
. Note then that there is a regular embedding
$\mathrm {chow}$
. Note then that there is a regular embedding
Next we describe the the universal family of log Fano pairs over U. We need this to set up the VGIT and in §5.2 to compute the CM line bundle. We begin by considering the following diagram:

We let
![]() $p_1$
(resp.,
$p_1$
(resp.,
![]() $p_2$
) denote the first (resp., second) projection, and let
$p_2$
) denote the first (resp., second) projection, and let
![]() $f: (\mathscr {X}, \mathscr {D}) \to \mathbb {P}(E)$
be the universal family over
$f: (\mathscr {X}, \mathscr {D}) \to \mathbb {P}(E)$
be the universal family over
![]() $\mathbb {P}(E)$
, where we view
$\mathbb {P}(E)$
, where we view
![]() $(\mathscr {X}, \mathscr {D}) \subseteq \mathbb {P}^3 \times \mathbb {P}(E)$
. We let
$(\mathscr {X}, \mathscr {D}) \subseteq \mathbb {P}^3 \times \mathbb {P}(E)$
. We let
![]() $\mathcal {Q} \subset \mathbb {P}^3 \times \mathbb {P}^9$
denote the universal family over
$\mathcal {Q} \subset \mathbb {P}^3 \times \mathbb {P}^9$
denote the universal family over
![]() $\mathbb {P}^9$
with morphism
$\mathbb {P}^9$
with morphism
![]() $\phi : \mathcal {Q} \to \mathbb {P}^9$
, and let
$\phi : \mathcal {Q} \to \mathbb {P}^9$
, and let
![]() $E = \phi _* \mathcal {O}_{\mathcal {Q}}(4,0)$
. Pointwise, we have
$E = \phi _* \mathcal {O}_{\mathcal {Q}}(4,0)$
. Pointwise, we have

Using the notation of Laza and O’Grady (see [Reference Laza and O’Grady43, equation (5.2)]), we denote by
![]() $\eta : = \pi ^*\mathcal {O}_{\mathbb {P}^9}(1)$
and
$\eta : = \pi ^*\mathcal {O}_{\mathbb {P}^9}(1)$
and
![]() $\xi := \mathcal {O}_{\mathbb {P}(E)}(1)$
. We recall the following result of Benoist.
$\xi := \mathcal {O}_{\mathbb {P}(E)}(1)$
. We recall the following result of Benoist.
Proposition 3.1 [Reference Benoist8, Theorem 2.7]
If
![]() $t \in \mathbb {Q}$
, then the
$t \in \mathbb {Q}$
, then the
![]() $\mathbb {Q}$
-Cartier class
$\mathbb {Q}$
-Cartier class
![]() $\eta + t\xi $
on
$\eta + t\xi $
on
![]() $\mathbb {P}(E)$
is ample if and only if
$\mathbb {P}(E)$
is ample if and only if
 $t \in \left(0, \frac {1}{3}\right) \cap \mathbb {Q}$
.
$t \in \left(0, \frac {1}{3}\right) \cap \mathbb {Q}$
.
We now set up the VGIT, following [Reference Laza and O’Grady43, Section 5.1]. Let
![]() $\mathscr {P}$
denote the closure of U in
$\mathscr {P}$
denote the closure of U in
![]() $\mathbb {P}(E) \times \operatorname {Chow}_{\left(2,4\right)}$
. Let
$\mathbb {P}(E) \times \operatorname {Chow}_{\left(2,4\right)}$
. Let
![]() $p_1$
and
$p_1$
and
![]() $p_2$
be the first and second projections from
$p_2$
be the first and second projections from
![]() $\mathscr {P}$
to
$\mathscr {P}$
to
![]() $\mathbb {P}(E)$
and
$\mathbb {P}(E)$
and
![]() $\operatorname {Chow}_{\left(2,4\right)}$
, respectively. The action of
$\operatorname {Chow}_{\left(2,4\right)}$
, respectively. The action of
![]() $\mathrm {SL}(4)$
on
$\mathrm {SL}(4)$
on
![]() $\mathbb {P}^3$
extends to an action on
$\mathbb {P}^3$
extends to an action on
![]() $\mathscr {P}$
. To construct a GIT quotient, we thus need to specify an
$\mathscr {P}$
. To construct a GIT quotient, we thus need to specify an
![]() $\mathrm {SL}(4)$
linearized ample line bundle on
$\mathrm {SL}(4)$
linearized ample line bundle on
![]() $\mathscr {P}$
.
$\mathscr {P}$
.
Fix a rational number
 $0 < \delta < \frac {1}{6}$
. For
$0 < \delta < \frac {1}{6}$
. For
![]() $t \in (\delta , 1/2) \cap \mathbb {Q}$
, consider the
$t \in (\delta , 1/2) \cap \mathbb {Q}$
, consider the
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
 $$ \begin{align*}N_t := \frac{1 - 2t}{1-2\delta} p_1^*(\eta + \delta \xi) + \frac{t - \delta}{2(1-2\delta)} p_2^*L_{\infty} ,\end{align*} $$
$$ \begin{align*}N_t := \frac{1 - 2t}{1-2\delta} p_1^*(\eta + \delta \xi) + \frac{t - \delta}{2(1-2\delta)} p_2^*L_{\infty} ,\end{align*} $$
where
![]() $L_{\infty }$
is the restriction of the natural polarization of the Chow variety to
$L_{\infty }$
is the restriction of the natural polarization of the Chow variety to
![]() $\operatorname {Chow}_{\left(2,4\right)}$
. One can check that
$\operatorname {Chow}_{\left(2,4\right)}$
. One can check that
![]() $N_t$
is ample for
$N_t$
is ample for
 $\delta < t < \frac {1}{2}$
and semiample for
$\delta < t < \frac {1}{2}$
and semiample for
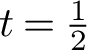 $t = \frac {1}{2}$
.
$t = \frac {1}{2}$
.
Definition 3.2. Let
![]() $\delta \in \mathbb {Q}$
satisfy
$\delta \in \mathbb {Q}$
satisfy
 $0 < \delta < \frac {1}{6}$
. For each
$0 < \delta < \frac {1}{6}$
. For each
 $t \in \left(\delta , \frac {1}{2}\right] \cap \mathbb {Q}$
, we define the VGIT quotient stack
$t \in \left(\delta , \frac {1}{2}\right] \cap \mathbb {Q}$
, we define the VGIT quotient stack
![]() $\mathscr {M}(t)$
of slope t and the VGIT quotient space
$\mathscr {M}(t)$
of slope t and the VGIT quotient space
![]() $\mathfrak {M}(t)$
of slope t:
$\mathfrak {M}(t)$
of slope t:
Remark 3.3.
-
(1) Laza and O’Grady show that the VGIT quotients do not depend on the choice of
 $\delta $
, so the lack of
$\delta $
, so the lack of
 $\delta $
in the notation is justified (see also Theorem 6.6(1)).
$\delta $
in the notation is justified (see also Theorem 6.6(1)). -
(2) Since
 $N_t$
is only semiample for
$N_t$
is only semiample for
 $t = \frac {1}{2}$
, they define
$t = \frac {1}{2}$
, they define
 $\mathfrak {M}\left(\frac {1}{2}\right)$
to be
$\mathfrak {M}\left(\frac {1}{2}\right)$
to be
 $\mathrm {Proj} R\left(\mathscr {P}, N_{\frac {1}{2}}\right)^{\mathrm {SL}(4)}$
and show that this is isomorphic to
$\mathrm {Proj} R\left(\mathscr {P}, N_{\frac {1}{2}}\right)^{\mathrm {SL}(4)}$
and show that this is isomorphic to
 $\operatorname {Chow}_{\left(2,4\right)}\mathbin {/\mkern -6mu/} \mathrm {SL}(4)$
.
$\operatorname {Chow}_{\left(2,4\right)}\mathbin {/\mkern -6mu/} \mathrm {SL}(4)$
.
The following two results from [Reference Laza and O’Grady43] will be required to relate the VGIT moduli spaces and K-moduli spaces:
Proposition 3.4 [Reference Laza and O’Grady43, Proposition 5.4]
Let
![]() $\mathrm {chow}: U \to \operatorname {Chow}_{(2,4)}$
be the Hilbert–Chow morphism and let
$\mathrm {chow}: U \to \operatorname {Chow}_{(2,4)}$
be the Hilbert–Chow morphism and let
![]() $\overline {L}_{\infty }\in \mathrm {Pic} (\mathbb {P}(E))_{\mathbb {Q}}$
be the unique extension of
$\overline {L}_{\infty }\in \mathrm {Pic} (\mathbb {P}(E))_{\mathbb {Q}}$
be the unique extension of
![]() $\mathrm {chow}^*L_\infty $
to
$\mathrm {chow}^*L_\infty $
to
![]() $\mathbb {P}(E)$
. Then
$\mathbb {P}(E)$
. Then
Lemma 3.5 [Reference Laza and O’Grady43, Proposition 5.11]
For each
 $t\in \left(\delta ,\frac {1}{2}\right] \cap \mathbb {Q}$
, the VGIT semistable locus
$t\in \left(\delta ,\frac {1}{2}\right] \cap \mathbb {Q}$
, the VGIT semistable locus
![]() $\mathscr {P}^{\textrm {ss}}(N_t)$
of slope t is a Zariski open subset of U.
$\mathscr {P}^{\textrm {ss}}(N_t)$
of slope t is a Zariski open subset of U.
We now state the main VGIT result of [Reference Laza and O’Grady43], noting that their results also hold for the VGIT quotient stacks. Let
![]() $\mathrm {Hilb}_{\left(2,4\right)}$
denote the closure of U inside the relevant Hilbert scheme, and let
$\mathrm {Hilb}_{\left(2,4\right)}$
denote the closure of U inside the relevant Hilbert scheme, and let
![]() $L_m$
denote the Plücker line bundle corresponding to the mth Hilbert point.
$L_m$
denote the Plücker line bundle corresponding to the mth Hilbert point.
Theorem 3.6 [Reference Laza and O’Grady43, Theorem 5.6]
Let
![]() $\delta $
be as before. The following hold:
$\delta $
be as before. The following hold:
-
(1) For
 $t \in \left(\delta , \frac {1}{3}\right)$
, the moduli space
$t \in \left(\delta , \frac {1}{3}\right)$
, the moduli space
 $\mathfrak {M}(t) \cong \mathbb {P}(E) \mathbin {/\mkern -6mu/}_{\eta + t\xi } \mathrm {SL}(4)$
.
$\mathfrak {M}(t) \cong \mathbb {P}(E) \mathbin {/\mkern -6mu/}_{\eta + t\xi } \mathrm {SL}(4)$
. -
(2) For
 $t \in \left(\delta , \frac {1}{6}\right)$
, we have
$t \in \left(\delta , \frac {1}{6}\right)$
, we have
 $\mathfrak {M}(t) \cong \mathfrak {M}$
.
$\mathfrak {M}(t) \cong \mathfrak {M}$
. -
(3) For
 $m \geq 4$
, we have
$m \geq 4$
, we have
 $\mathrm {Hilb}_{\left(2,4\right)}\mathbin {/\mkern -6mu/}_{L_m} \mathrm {SL}(4) \cong \mathfrak {M}(t(m))$
, where
$\mathrm {Hilb}_{\left(2,4\right)}\mathbin {/\mkern -6mu/}_{L_m} \mathrm {SL}(4) \cong \mathfrak {M}(t(m))$
, where
 $t(m) = \dfrac {(m-3)^2}{2\left(m^2 - 4m + 5\right)}$
.
$t(m) = \dfrac {(m-3)^2}{2\left(m^2 - 4m + 5\right)}$
. -
(4)
 $\mathfrak {M}\left(\frac {1}{2}\right)\cong \operatorname {Chow}_{\left(2,4\right)}\mathbin {/\mkern -6mu/} \mathrm {SL}(4)$
.
$\mathfrak {M}\left(\frac {1}{2}\right)\cong \operatorname {Chow}_{\left(2,4\right)}\mathbin {/\mkern -6mu/} \mathrm {SL}(4)$
.
Before stating their main result, we review some results from VGIT.
3.3.1 Variation of GIT
The general theory of VGIT quotients can be found in [Reference Thaddeus72, Reference Dolgachev and Hu19]. The goal here is to compare
![]() $\mathfrak {M}(t)$
for
$\mathfrak {M}(t)$
for
 $t \in \left(\delta , \frac {1}{2}\right) \cap \mathbb {Q}$
, in particular how varying the line bundle
$t \in \left(\delta , \frac {1}{2}\right) \cap \mathbb {Q}$
, in particular how varying the line bundle
![]() $N_t$
changes the GIT quotient. The main results of VGIT state that this interval can be subdivided into finitely many open chambers, and on each open chamber the space
$N_t$
changes the GIT quotient. The main results of VGIT state that this interval can be subdivided into finitely many open chambers, and on each open chamber the space
![]() $\mathfrak {M}(t)$
remains unchanged ([Reference Thaddeus72, Theorem 2.4] and [Reference Dolgachev and Hu19, Theorem 0.2.3]). The finitely many values where the space
$\mathfrak {M}(t)$
remains unchanged ([Reference Thaddeus72, Theorem 2.4] and [Reference Dolgachev and Hu19, Theorem 0.2.3]). The finitely many values where the space
![]() $\mathfrak {M}(t)$
does change are called walls. Here, there are birational morphisms
$\mathfrak {M}(t)$
does change are called walls. Here, there are birational morphisms
![]() $\mathfrak {M}(t-\epsilon ) \to \mathfrak {M}(t) \leftarrow \mathfrak {M}(t+\epsilon )$
, and there are additionally wall-crossing rational maps
$\mathfrak {M}(t-\epsilon ) \to \mathfrak {M}(t) \leftarrow \mathfrak {M}(t+\epsilon )$
, and there are additionally wall-crossing rational maps
![]() $\mathfrak {M}(t-\epsilon ) \dashrightarrow \mathfrak {M}(t+\epsilon )$
([Reference Thaddeus72, Theorem 3.3]).
$\mathfrak {M}(t-\epsilon ) \dashrightarrow \mathfrak {M}(t+\epsilon )$
([Reference Thaddeus72, Theorem 3.3]).
Later on, we will need the following foundational results in VGIT, and we refer the reader to the survey [Reference Laza41, Sections 3 and 4] and the references therein:
Lemma 3.7. Let
![]() $(X,\mathcal L_0)$
be a polarized projective variety. Let G be a reductive group acting on
$(X,\mathcal L_0)$
be a polarized projective variety. Let G be a reductive group acting on
![]() $(X,\mathcal L_0)$
. Let
$(X,\mathcal L_0)$
. Let
![]() $\mathcal L$
be a G-linearized line bundle on X. For a rational number
$\mathcal L$
be a G-linearized line bundle on X. For a rational number
![]() $0<\epsilon \ll 1$
, consider the G-linearized ample
$0<\epsilon \ll 1$
, consider the G-linearized ample
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
![]() $\mathcal L_{\pm }:= \mathcal L_{0}\otimes \mathcal L^{\otimes (\pm \epsilon )}$
.
$\mathcal L_{\pm }:= \mathcal L_{0}\otimes \mathcal L^{\otimes (\pm \epsilon )}$
.
-
(1) Let
 $X \mathbin {/\mkern -6mu/}_{\mathcal {L}_0} G$
and
$X \mathbin {/\mkern -6mu/}_{\mathcal {L}_0} G$
and
 $X \mathbin {/\mkern -6mu/}_{\mathcal {L}_{\pm }} G$
denote the VGIT quotients. If
$X \mathbin {/\mkern -6mu/}_{\mathcal {L}_{\pm }} G$
denote the VGIT quotients. If
 $X^{\textrm {ss}}(0)$
and
$X^{\textrm {ss}}(0)$
and
 $X^{\textrm {ss}}(\pm )$
denote the respective VGIT semistable loci, then there are open inclusions
$X^{\textrm {ss}}(\pm )$
denote the respective VGIT semistable loci, then there are open inclusions
 $X^{\textrm {ss}}(\pm ) \subseteq X^{\textrm {ss}}(0)$
.
$X^{\textrm {ss}}(\pm ) \subseteq X^{\textrm {ss}}(0)$
. -
(2) For any closed point
 $x\in X^{\textrm {ss}}(0)\setminus X^{\textrm {ss}}(\pm )$
, there exists a
$x\in X^{\textrm {ss}}(0)\setminus X^{\textrm {ss}}(\pm )$
, there exists a
 $1$
-PS
$1$
-PS
 $\sigma $
in G such that
$\sigma $
in G such that  $$ \begin{align*} \mu^{\mathcal L_0}(x, \sigma)=0 \quad\text{and}\quad \mu^{\mathcal L_\pm}(x, \sigma)<0. \end{align*} $$
$$ \begin{align*} \mu^{\mathcal L_0}(x, \sigma)=0 \quad\text{and}\quad \mu^{\mathcal L_\pm}(x, \sigma)<0. \end{align*} $$
Proof. (1) This is the well-known semicontinuity property of semistable loci from [Reference Thaddeus72, Theorem 4.1] and [Reference Dolgachev and Hu19, §3.4] (see also [Reference Laza41, Lemma 3.10]).
(2) By symmetry we may assume that x is VGIT unstable with respect to
![]() $\mathcal L_+$
. Hence by the Hilbert–Mumford numerical criterion, there exists a
$\mathcal L_+$
. Hence by the Hilbert–Mumford numerical criterion, there exists a
![]() $1$
-PS
$1$
-PS
![]() $\sigma _0$
in G such that
$\sigma _0$
in G such that
![]() $\mu ^{\mathcal L_+}(x, \sigma _0)<0$
. Let T be a maximal torus of G containing
$\mu ^{\mathcal L_+}(x, \sigma _0)<0$
. Let T be a maximal torus of G containing
![]() $\sigma _0$
. By [Reference Mumford, Fogarty and Kirwan61, Chapter 2, Proposition 2.14], we know that there exist two rational piecewise linear function
$\sigma _0$
. By [Reference Mumford, Fogarty and Kirwan61, Chapter 2, Proposition 2.14], we know that there exist two rational piecewise linear function
![]() $h_0$
and h on
$h_0$
and h on
![]() $\mathrm {Hom}_{\mathbb {Q}}(\mathbb {G}_m, T)$
such that for any
$\mathrm {Hom}_{\mathbb {Q}}(\mathbb {G}_m, T)$
such that for any
![]() $1$
-PS
$1$
-PS
![]() $\lambda $
in T, we have
$\lambda $
in T, we have
Since
![]() $x\in X^{\textrm {ss}}(0)$
, we know that
$x\in X^{\textrm {ss}}(0)$
, we know that
![]() $h_0(\lambda )\geq 0$
for any
$h_0(\lambda )\geq 0$
for any
![]() $\lambda \in \mathrm {Hom}_{\mathbb {Q}}(\mathbb {G}_m, T)$
. On the other hand,
$\lambda \in \mathrm {Hom}_{\mathbb {Q}}(\mathbb {G}_m, T)$
. On the other hand,
![]() $\mu ^{\mathcal L_+}(x,\sigma _0)=h_0(\sigma _0)+\epsilon h(\sigma _0)<0$
. Hence there exists
$\mu ^{\mathcal L_+}(x,\sigma _0)=h_0(\sigma _0)+\epsilon h(\sigma _0)<0$
. Hence there exists
![]() $\sigma \in \mathrm {Hom}_{\mathbb {Q}}(\mathbb {G}_m, T)$
such that
$\sigma \in \mathrm {Hom}_{\mathbb {Q}}(\mathbb {G}_m, T)$
such that
![]() $h_0(\sigma )=0$
and
$h_0(\sigma )=0$
and
![]() $h(\sigma )<0$
. The proof is finished.
$h(\sigma )<0$
. The proof is finished.
Finally, we state the main result from [Reference Laza and O’Grady43]:
Theorem 3.8 [Reference Laza and O’Grady43, Theorem 1.1]
Let
![]() $\beta \in [0,1]$
and let
$\beta \in [0,1]$
and let
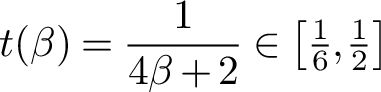 $t(\beta ) = \dfrac {1}{4\beta +2} \in \left[\frac {1}{6}, \frac {1}{2}\right]$
. The period map
$t(\beta ) = \dfrac {1}{4\beta +2} \in \left[\frac {1}{6}, \frac {1}{2}\right]$
. The period map
is the composition of elementary birational maps with eight critical values of
![]() $\beta $
. Moreover, there is an isomorphism
$\beta $
. Moreover, there is an isomorphism
![]() $\mathfrak {M}(t(\beta )) \cong \mathscr {F}(\beta )$
. In particular, the intermediate spaces are the VGIT quotients already described, and are related by elementary birational maps. Finally, the map
$\mathfrak {M}(t(\beta )) \cong \mathscr {F}(\beta )$
. In particular, the intermediate spaces are the VGIT quotients already described, and are related by elementary birational maps. Finally, the map
![]() $\mathscr {F}(1/8) \to \mathscr {F}(0) \cong \mathscr {F}^*$
is the
$\mathscr {F}(1/8) \to \mathscr {F}(0) \cong \mathscr {F}^*$
is the
![]() $\mathbb {Q}$
-Cartierization of
$\mathbb {Q}$
-Cartierization of
![]() $H_h$
.
$H_h$
.
4 Degenerations of
 $\mathbb {P}^1 \times \mathbb {P}^1$
in K-moduli spaces
$\mathbb {P}^1 \times \mathbb {P}^1$
in K-moduli spaces
4.1 K-moduli spaces of curves on
 $\mathbb {P}^1 \times \mathbb {P}^1$
$\mathbb {P}^1 \times \mathbb {P}^1$
In this section, we will define the K-moduli spaces which generically parametrize smooth
![]() $(d,d)$
-curves on
$(d,d)$
-curves on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
.
$\mathbb {P}^1\times \mathbb {P}^1$
.
Proposition 4.1. Let
![]() $d\geq 3$
be an integer. Let C be a
$d\geq 3$
be an integer. Let C be a
![]() $(d,d)$
-curve on
$(d,d)$
-curve on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
. If
$\mathbb {P}^1\times \mathbb {P}^1$
. If
 $\mathrm {lct}\left(\mathbb {P}^1\times \mathbb {P}^1;C\right)>\frac {2}{d}$
(resp.,
$\mathrm {lct}\left(\mathbb {P}^1\times \mathbb {P}^1;C\right)>\frac {2}{d}$
(resp.,
 $\geq \frac {2}{d}$
), then the log Fano pair
$\geq \frac {2}{d}$
), then the log Fano pair
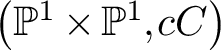 $\left(\mathbb {P}^1 \times \mathbb {P}^1, cC\right)$
is K-stable (resp., K-semistable) for any
$\left(\mathbb {P}^1 \times \mathbb {P}^1, cC\right)$
is K-stable (resp., K-semistable) for any
 $c\in \left(0, \frac {2}{d}\right)$
. In particular,
$c\in \left(0, \frac {2}{d}\right)$
. In particular,
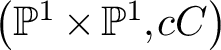 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
is K-stable for any
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
is K-stable for any
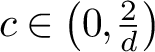 $c\in \left(0, \frac {2}{d}\right)$
if either C is smooth or
$c\in \left(0, \frac {2}{d}\right)$
if either C is smooth or
![]() $d=4$
and C has at worst ADE singularities.
$d=4$
and C has at worst ADE singularities.
Proof. This follows from interpolation (see [Reference Ascher, DeVleming and Liu6, Proposition 2.13] or [Reference Dervan18, Lemma 2.6]), since the pair
 $\left(\mathbb {P}^1 \times \mathbb {P}^1, \frac {2}{d}C\right)$
is klt (resp., lc) and
$\left(\mathbb {P}^1 \times \mathbb {P}^1, \frac {2}{d}C\right)$
is klt (resp., lc) and
![]() $\mathbb {P}^1\times \mathbb {P}^1$
is K-polystable.
$\mathbb {P}^1\times \mathbb {P}^1$
is K-polystable.
We begin to define the K-moduli stack
![]() $\overline {\mathcal {K}}_{d,c}$
and the K-moduli space
$\overline {\mathcal {K}}_{d,c}$
and the K-moduli space
![]() $\overline {K}_{d,c}$
. Let
$\overline {K}_{d,c}$
. Let
![]() $\chi _0(\cdot )$
be the Hilbert polynomial of the polarized Fano manifold
$\chi _0(\cdot )$
be the Hilbert polynomial of the polarized Fano manifold
 $\left(\mathbb {P}^1\times \mathbb {P}^1,-K_{\mathbb {P}^1\times \mathbb {P}^1}\right)$
– that is,
$\left(\mathbb {P}^1\times \mathbb {P}^1,-K_{\mathbb {P}^1\times \mathbb {P}^1}\right)$
– that is,
![]() $\chi _0(m)= 4m^2 +4m+1$
. Consider the K-moduli stack
$\chi _0(m)= 4m^2 +4m+1$
. Consider the K-moduli stack
![]() $\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
and K-moduli space
$\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
and K-moduli space
![]() $KM_{\chi _0, d/2, c}$
, where
$KM_{\chi _0, d/2, c}$
, where
![]() $d\geq 3$
is an integer and
$d\geq 3$
is an integer and
 $c\in \left(0, \frac {2}{d}\right)\cap \mathbb {Q}$
.
$c\in \left(0, \frac {2}{d}\right)\cap \mathbb {Q}$
.
Proposition 4.2. Let
![]() $d\geq 3$
be an integer. The K-moduli stack
$d\geq 3$
be an integer. The K-moduli stack
![]() $\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
and K-moduli space
$\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
and K-moduli space
![]() $KM_{\chi _0, d/2, c}$
are both normal. Moreover, we have the following cases:
$KM_{\chi _0, d/2, c}$
are both normal. Moreover, we have the following cases:
-
(1) If d is odd, then
 $\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
is connected and generically parametrizes
$\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
is connected and generically parametrizes
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where
 $C\in \lvert \mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(d,d)\rvert $
is a smooth curve.
$C\in \lvert \mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(d,d)\rvert $
is a smooth curve. -
(2) If d is even, then
 $\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
has at most two connected components. One of these components generically parametrizes
$\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
has at most two connected components. One of these components generically parametrizes
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where
 $C\in \lvert \mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(d,d)\rvert $
is a smooth curve; the other component, if it exists, generically parametrizes
$C\in \lvert \mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(d,d)\rvert $
is a smooth curve; the other component, if it exists, generically parametrizes
 $(\mathbb {F}_1, cC')$
, where
$(\mathbb {F}_1, cC')$
, where
 $C'\in \left\lvert \mathcal {O}_{\mathbb {F}_1}\left(-\frac {d}{2}K_{\mathbb {F}_1}\right)\right\rvert $
is a smooth curve on the Hirzebruch surface
$C'\in \left\lvert \mathcal {O}_{\mathbb {F}_1}\left(-\frac {d}{2}K_{\mathbb {F}_1}\right)\right\rvert $
is a smooth curve on the Hirzebruch surface
 $\mathbb {F}_1$
.
$\mathbb {F}_1$
.
Proof. The normality of
![]() $\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
and
$\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
and
![]() $KM_{\chi _0, d/2, c}$
is a direct consequence of Theorem 2.21. For the rest, notice that there are only two smooth del Pezzo surfaces of degree
$KM_{\chi _0, d/2, c}$
is a direct consequence of Theorem 2.21. For the rest, notice that there are only two smooth del Pezzo surfaces of degree
![]() $8$
up to isomorphism:
$8$
up to isomorphism:
![]() $\mathbb {P}^1\times \mathbb {P}^1$
and
$\mathbb {P}^1\times \mathbb {P}^1$
and
![]() $\mathbb {F}_1$
. In addition, they are not homeomorphic, since their intersection pairings on
$\mathbb {F}_1$
. In addition, they are not homeomorphic, since their intersection pairings on
![]() $H^2(\cdot , \mathbb {Z})$
are not isomorphic. By Proposition 4.1 we know that
$H^2(\cdot , \mathbb {Z})$
are not isomorphic. By Proposition 4.1 we know that
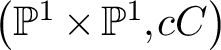 $\left(\mathbb {P}^1\times \mathbb {P}^1,cC\right)$
, where C is a smooth
$\left(\mathbb {P}^1\times \mathbb {P}^1,cC\right)$
, where C is a smooth
![]() $(d,d)$
-curve, is always parametrized by
$(d,d)$
-curve, is always parametrized by
![]() $\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
. If d is odd, then
$\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
. If d is odd, then
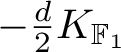 $-\frac {d}{2}K_{\mathbb {F}_1}$
is not represented by any Weil divisor, since it has fractional intersection with the
$-\frac {d}{2}K_{\mathbb {F}_1}$
is not represented by any Weil divisor, since it has fractional intersection with the
![]() $(-1)$
-curve on
$(-1)$
-curve on
![]() $\mathbb {F}_1$
. Hence
$\mathbb {F}_1$
. Hence
![]() $\mathbb {F}_1$
will not appear in
$\mathbb {F}_1$
will not appear in
![]() $\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
when d is odd. The proof is finished.
$\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
when d is odd. The proof is finished.
Definition 4.3. Let
![]() $d\geq 3$
be an integer. For
$d\geq 3$
be an integer. For
 $c\in \left(0, \frac {2}{d}\right)\cap \mathbb {Q}$
, let
$c\in \left(0, \frac {2}{d}\right)\cap \mathbb {Q}$
, let
![]() $\overline {\mathcal {K}}_{d,c}$
denote the connected component of
$\overline {\mathcal {K}}_{d,c}$
denote the connected component of
![]() $\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
where a general point parametrizes
$\mathcal {K}\mathcal M_{\chi _0, d/2, c}$
where a general point parametrizes
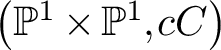 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
, where
![]() $C\in \lvert \mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(d,d)\rvert $
is a smooth curve. In other words,
$C\in \lvert \mathcal {O}_{\mathbb {P}^1\times \mathbb {P}^1}(d,d)\rvert $
is a smooth curve. In other words,
![]() $\overline {\mathcal {K}}_{d,c}$
is the moduli stack parametrizing K-semistable log Fano pairs
$\overline {\mathcal {K}}_{d,c}$
is the moduli stack parametrizing K-semistable log Fano pairs
![]() $(X,cD)$
, where X admits a
$(X,cD)$
, where X admits a
![]() $\mathbb {Q}$
-Gorenstein smoothing to
$\mathbb {Q}$
-Gorenstein smoothing to
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
and the effective
$\mathbb {P}^1 \times \mathbb {P}^1$
and the effective
![]() $\mathbb {Q}$
-Cartier Weil divisor
$\mathbb {Q}$
-Cartier Weil divisor
 $D \sim _{\mathbb {Q}} -\frac {d}{2}K_X$
. We let
$D \sim _{\mathbb {Q}} -\frac {d}{2}K_X$
. We let
![]() $\overline {K}_{d,c}$
denote the good moduli space of
$\overline {K}_{d,c}$
denote the good moduli space of
![]() $\overline {\mathcal {K}}_{d,c}$
. From Theorems 2.19 and 2.21 and Proposition 4.2, we know that
$\overline {\mathcal {K}}_{d,c}$
. From Theorems 2.19 and 2.21 and Proposition 4.2, we know that
![]() $\overline {\mathcal {K}}_{d,c}$
is a connected smooth Artin stack of finite type over
$\overline {\mathcal {K}}_{d,c}$
is a connected smooth Artin stack of finite type over
![]() $\mathbb {C}$
, and
$\mathbb {C}$
, and
![]() $\overline {K}_{d,c}$
is a normal projective variety over
$\overline {K}_{d,c}$
is a normal projective variety over
![]() $\mathbb {C}$
.
$\mathbb {C}$
.
The following theorem is a direct consequence of [Reference Ascher, DeVleming and Liu6, Theorem 1.2] and Proposition 4.1:
Theorem 4.4. Let
![]() $d\geq 3$
be an integer. There exist rational numbers
$d\geq 3$
be an integer. There exist rational numbers
 $$ \begin{align*} 0=c_0 <c_1<c_2<\dotsb< c_k =\frac{2}{d} \end{align*} $$
$$ \begin{align*} 0=c_0 <c_1<c_2<\dotsb< c_k =\frac{2}{d} \end{align*} $$
such that for each
![]() $0\leq i\leq k-1$
, the K-moduli stacks
$0\leq i\leq k-1$
, the K-moduli stacks
![]() $\overline {\mathcal {K}}_{d,c}$
are independent of the choice of
$\overline {\mathcal {K}}_{d,c}$
are independent of the choice of
![]() $c\in (c_i,c_{i+1})$
. For each
$c\in (c_i,c_{i+1})$
. For each
![]() $1\leq i\leq k-1$
and
$1\leq i\leq k-1$
and
![]() $0<\epsilon \ll 1$
, we have open immersions
$0<\epsilon \ll 1$
, we have open immersions
which induce projective birational morphisms
Moreover, all these morphisms have local VGIT presentations as in [Reference Alper, Fedorchuk and Smyth5, (1.2)].
In this paper, we are mainly interested in the case when
![]() $d=4$
, although some results for general d are presented in §6. We always abbreviate
$d=4$
, although some results for general d are presented in §6. We always abbreviate
![]() $\overline {\mathcal {K}}_{4,c}$
and
$\overline {\mathcal {K}}_{4,c}$
and
![]() $\overline {K}_{4,c}$
to
$\overline {K}_{4,c}$
to
![]() $\overline {\mathcal {K}}_{c}$
and
$\overline {\mathcal {K}}_{c}$
and
![]() $\overline {K}_{c}$
, respectively.
$\overline {K}_{c}$
, respectively.
4.2 Classification of degenerations of
 $\mathbb {P}^1 \times \mathbb {P}^1$
$\mathbb {P}^1 \times \mathbb {P}^1$
The goal of this section is to prove Theorem 4.8, which states that if
![]() $(X,cD)$
is a pair parametrized by
$(X,cD)$
is a pair parametrized by
![]() $\overline {\mathcal {K}}_{c}$
for some
$\overline {\mathcal {K}}_{c}$
for some
 $c \in \left(0, \frac {1}{2}\right)$
, then X is isomorphic to either
$c \in \left(0, \frac {1}{2}\right)$
, then X is isomorphic to either
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
or
$\mathbb {P}^1 \times \mathbb {P}^1$
or
![]() $\mathbb {P}(1,1,2)$
. Later on, we will show (in Theorem 4.10) that the same is true in
$\mathbb {P}(1,1,2)$
. Later on, we will show (in Theorem 4.10) that the same is true in
![]() $\overline {\mathcal {K}}_{d,c}$
for
$\overline {\mathcal {K}}_{d,c}$
for
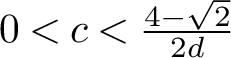 $0 < c < \frac {4-\sqrt {2}}{2d}$
and
$0 < c < \frac {4-\sqrt {2}}{2d}$
and
![]() $d \geq 3$
. First we show that if X is a normal
$d \geq 3$
. First we show that if X is a normal
![]() $\mathbb {Q}$
-Gorenstein deformation of
$\mathbb {Q}$
-Gorenstein deformation of
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
, then
$\mathbb {P}^1 \times \mathbb {P}^1$
, then
![]() $\rho (X) \leq 2$
:
$\rho (X) \leq 2$
:
Proposition 4.5. Let X be a log del Pezzo surface. Suppose that X admits a
![]() $\mathbb {Q}$
-Gorenstein deformation to
$\mathbb {Q}$
-Gorenstein deformation to
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
. Then
$\mathbb {P}^1 \times \mathbb {P}^1$
. Then
![]() $\rho (X) \leq 2$
.
$\rho (X) \leq 2$
.
Proof. Let
![]() $\mathcal X\to T$
be a
$\mathcal X\to T$
be a
![]() $\mathbb {Q}$
-Gorenstein smoothing of X – that is,
$\mathbb {Q}$
-Gorenstein smoothing of X – that is,
![]() $0\in T$
is a smooth germ of pointed curve,
$0\in T$
is a smooth germ of pointed curve,
![]() $\mathcal X_0\cong X$
, and
$\mathcal X_0\cong X$
, and
![]() $\mathcal X_t\cong \mathbb {P}^1\times \mathbb {P}^1$
for
$\mathcal X_t\cong \mathbb {P}^1\times \mathbb {P}^1$
for
![]() $t\in T\setminus \{0\}$
. By passing to a finite cover of
$t\in T\setminus \{0\}$
. By passing to a finite cover of
![]() $0\in T$
, we may assume that
$0\in T$
, we may assume that
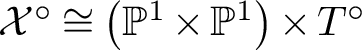 $\mathcal X^\circ \cong \left(\mathbb {P}^1\times \mathbb {P}^1\right)\times T^\circ $
, where
$\mathcal X^\circ \cong \left(\mathbb {P}^1\times \mathbb {P}^1\right)\times T^\circ $
, where
![]() $\mathcal X^\circ :=\mathcal X\setminus \mathcal X_0$
and
$\mathcal X^\circ :=\mathcal X\setminus \mathcal X_0$
and
![]() $T^\circ := T \setminus \{0\}$
. First, using [Reference Hacking29, Lemma 2.11], we show that
$T^\circ := T \setminus \{0\}$
. First, using [Reference Hacking29, Lemma 2.11], we show that
![]() $\mathrm {Cl}(\mathcal {X}) \cong \mathbb {Z}^{2}$
. Indeed, consider the exact sequence
$\mathrm {Cl}(\mathcal {X}) \cong \mathbb {Z}^{2}$
. Indeed, consider the exact sequence
which gives
![]() $\mathrm {Cl}(\mathcal {X}) \cong \mathrm {Cl}(\mathcal {X}^\circ )\cong \mathbb {Z}^{2}$
.
$\mathrm {Cl}(\mathcal {X}) \cong \mathrm {Cl}(\mathcal {X}^\circ )\cong \mathbb {Z}^{2}$
.
Now we follow the proof of [Reference Hacking29, Proposition 6.3]. First note that there is an isomorphism
![]() $\mathrm {Pic}(\mathcal {X}) \to \mathrm {Pic}(X)$
, and so we obtain the inequality
$\mathrm {Pic}(\mathcal {X}) \to \mathrm {Pic}(X)$
, and so we obtain the inequality
with equality if and only if
![]() $\mathcal {X}$
is
$\mathcal {X}$
is
![]() $\mathbb {Q}$
-factorial.
$\mathbb {Q}$
-factorial.
A result of Hacking and Prokhorov now classifies the possible
![]() $\mathbb {Q}$
-Gorenstein smoothings of
$\mathbb {Q}$
-Gorenstein smoothings of
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
(see [Reference Hacking and Prokhorov30, Theorem 1.2] and [Reference Hacking and Prokhorov31, Proposition 2.6]):
$\mathbb {P}^1 \times \mathbb {P}^1$
(see [Reference Hacking and Prokhorov30, Theorem 1.2] and [Reference Hacking and Prokhorov31, Proposition 2.6]):
Proposition 4.6 Hacking and Prokhorov
Let X be a log del Pezzo surface admitting a
![]() $\mathbb {Q}$
-Gorenstein smoothing to
$\mathbb {Q}$
-Gorenstein smoothing to
![]() $\mathbb {P}^1\times \mathbb {P}^1$
. There are two cases:
$\mathbb {P}^1\times \mathbb {P}^1$
. There are two cases:
-
(1) If
 $\rho (X)=1$
, then X is a
$\rho (X)=1$
, then X is a
 $\mathbb {Q}$
-Gorenstein partial smoothing of a weighted projective plane
$\mathbb {Q}$
-Gorenstein partial smoothing of a weighted projective plane
 $\mathbb {P}\left(a^2,b^2,2c^2\right)$
, where
$\mathbb {P}\left(a^2,b^2,2c^2\right)$
, where
 $(a,b,c)\in \mathbb {Z}_{>0}^3$
subject to the equation In particular, the local index
$(a,b,c)\in \mathbb {Z}_{>0}^3$
subject to the equation In particular, the local index $$ \begin{align*} a^2+b^2+2c^2=4abc. \end{align*} $$
$$ \begin{align*} a^2+b^2+2c^2=4abc. \end{align*} $$
 $\mathrm {ind}(x,K_X)$
is odd for any
$\mathrm {ind}(x,K_X)$
is odd for any
 $x\in X$
.
$x\in X$
.
-
(2) If
 $\rho (X)=2$
, then X only has quotient singularities of type
$\rho (X)=2$
, then X only has quotient singularities of type
 $\frac {1}{n^2}(1,an-1)$
, where
$\frac {1}{n^2}(1,an-1)$
, where
 $\gcd (a,n)=1$
.
$\gcd (a,n)=1$
.
Suppose
![]() $x\in X$
is a surface T-singularity. We denote by
$x\in X$
is a surface T-singularity. We denote by
![]() $\mu _x$
the Milnor number of a
$\mu _x$
the Milnor number of a
![]() $\mathbb {Q}$
-Gorenstein smoothing of
$\mathbb {Q}$
-Gorenstein smoothing of
![]() $x\in X$
. If
$x\in X$
. If
![]() $x\in X$
is a cyclic quotient T-singularity of type
$x\in X$
is a cyclic quotient T-singularity of type
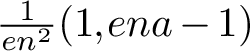 $\frac {1}{en^2}(1,ena-1)$
, then
$\frac {1}{en^2}(1,ena-1)$
, then
![]() $\mu _x=e-1$
.
$\mu _x=e-1$
.
Theorem 4.7. Let
![]() $(X,cD) $
be a K-semistable log Fano pair that admits a
$(X,cD) $
be a K-semistable log Fano pair that admits a
![]() $\mathbb {Q}$
-Gorenstein smoothing to
$\mathbb {Q}$
-Gorenstein smoothing to
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC_t\right)$
, with
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC_t\right)$
, with
 $c\in \left(0,\frac {2}{d}\right)$
and
$c\in \left(0,\frac {2}{d}\right)$
and
![]() $C_t$
a curve of bidgree
$C_t$
a curve of bidgree
![]() $(d,d)$
. Let
$(d,d)$
. Let
![]() $x\in X$
be any singular point.
$x\in X$
be any singular point.
-
(1) If d is even or
 $\mathrm {ind}(x,K_X)$
is odd, then
$\mathrm {ind}(x,K_X)$
is odd, then  $$ \begin{align*} \mathrm{ind}(x,K_X)\leq\begin{cases} \min\left\{\left\lfloor\frac{3}{\sqrt{2}(2-cd)}\right\rfloor,d+1\right\} & \text{if }\mu_x=0,\\ \min\left\{\left\lfloor\frac{3}{2(2-cd)}\right\rfloor,d\right\} & \text{if }\mu_x=1. \end{cases} \end{align*} $$
$$ \begin{align*} \mathrm{ind}(x,K_X)\leq\begin{cases} \min\left\{\left\lfloor\frac{3}{\sqrt{2}(2-cd)}\right\rfloor,d+1\right\} & \text{if }\mu_x=0,\\ \min\left\{\left\lfloor\frac{3}{2(2-cd)}\right\rfloor,d\right\} & \text{if }\mu_x=1. \end{cases} \end{align*} $$
-
(2) If d is odd and
 $\mathrm {ind}(x,K_X)$
is even, then
$\mathrm {ind}(x,K_X)$
is even, then
 $\rho (X)=2$
,
$\rho (X)=2$
,
 $\mu _x=0$
, and
$\mu _x=0$
, and  $$ \begin{align*} \mathrm{ind}(x,K_X)\leq \min\left\{2\left\lfloor\tfrac{3}{2\sqrt{2}(2-cd)}\right\rfloor,2d-2\right\}. \end{align*} $$
$$ \begin{align*} \mathrm{ind}(x,K_X)\leq \min\left\{2\left\lfloor\tfrac{3}{2\sqrt{2}(2-cd)}\right\rfloor,2d-2\right\}. \end{align*} $$
Proof. Define
![]() $\beta :=1-cd/2\in (0,1)$
. We know that an index n point
$\beta :=1-cd/2\in (0,1)$
. We know that an index n point
![]() $x\in X$
is a cyclic quotient singularity of type
$x\in X$
is a cyclic quotient singularity of type
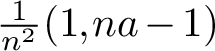 $\frac {1}{n^2}(1,na-1)$
or
$\frac {1}{n^2}(1,na-1)$
or
 $\frac {1}{2n^2}(1, 2na-1)$
, where
$\frac {1}{2n^2}(1, 2na-1)$
, where
![]() $\gcd (a,n)=1$
. If
$\gcd (a,n)=1$
. If
![]() $\mu _x=0$
, then the orbifold group of
$\mu _x=0$
, then the orbifold group of
![]() $x\in X$
has order
$x\in X$
has order
![]() $n^2$
, which implies that
$n^2$
, which implies that
 $\widehat {\mathrm {vol}}(x,X)=\frac {4}{n^2}$
by [Reference Li and Liu51, Proposition 4.10]. Hence Theorem 2.6 implies that
$\widehat {\mathrm {vol}}(x,X)=\frac {4}{n^2}$
by [Reference Li and Liu51, Proposition 4.10]. Hence Theorem 2.6 implies that
 $$ \begin{align*} 8\beta^2 =(-K_X-cD)^2 \leq \frac{9}{4}\widehat{\mathrm{vol}}(x,X)=\frac{9}{n^2}. \end{align*} $$
$$ \begin{align*} 8\beta^2 =(-K_X-cD)^2 \leq \frac{9}{4}\widehat{\mathrm{vol}}(x,X)=\frac{9}{n^2}. \end{align*} $$
This shows that
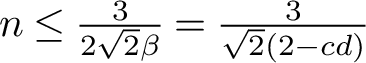 $n\leq \frac {3}{2\sqrt {2} \beta }=\frac {3}{\sqrt {2}(2-cd)}$
. Similarly, if
$n\leq \frac {3}{2\sqrt {2} \beta }=\frac {3}{\sqrt {2}(2-cd)}$
. Similarly, if
![]() $\mu _x=1$
, then
$\mu _x=1$
, then
![]() $x\in X$
has orbifold group of order
$x\in X$
has orbifold group of order
![]() $2n^2$
, which implies that
$2n^2$
, which implies that
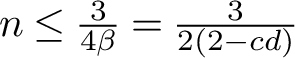 $n\leq \frac {3}{4 \beta }=\frac {3}{2(2-cd)}$
. Hence the first terms in the index upper bounds are verified.
$n\leq \frac {3}{4 \beta }=\frac {3}{2(2-cd)}$
. Hence the first terms in the index upper bounds are verified.
The rest of this proof is devoted to verifying the second terms in the index upper bounds. We know that
![]() $dK_X+2D\sim 0$
when d is odd and
$dK_X+2D\sim 0$
when d is odd and
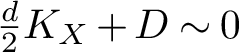 $\frac {d}{2}K_X+D\sim 0$
when d is even. If
$\frac {d}{2}K_X+D\sim 0$
when d is even. If
![]() $x\not \in D$
, then
$x\not \in D$
, then
![]() $n\mid d$
, hence
$n\mid d$
, hence
![]() $n\leq d$
(in fact,
$n\leq d$
(in fact,
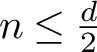 $n\leq \frac d{2}$
if d is even). Hence the second terms are verified for
$n\leq \frac d{2}$
if d is even). Hence the second terms are verified for
![]() $x\not \in D$
.
$x\not \in D$
.
From now on, let us assume
![]() $x\in D$
. Let
$x\in D$
. Let
 $\left(\tilde {x}\in \widetilde {X}\right)$
be the smooth cover of
$\left(\tilde {x}\in \widetilde {X}\right)$
be the smooth cover of
![]() $(x\in X)$
, with
$(x\in X)$
, with
![]() $\widetilde {D}$
being the preimage of D. Assume
$\widetilde {D}$
being the preimage of D. Assume
![]() $\tilde {x}\in \widetilde {X}$
has local coordinates
$\tilde {x}\in \widetilde {X}$
has local coordinates
![]() $(u,v)$
where the cyclic group action is scaling on each coordinate. Let
$(u,v)$
where the cyclic group action is scaling on each coordinate. Let
![]() $u^i v^j$
be a monomial appearing in the equation on
$u^i v^j$
be a monomial appearing in the equation on
![]() $\widetilde {D}$
with minimum
$\widetilde {D}$
with minimum
![]() $i+j=\mathrm {ord}_{\tilde {x}}\widetilde {D}$
.
$i+j=\mathrm {ord}_{\tilde {x}}\widetilde {D}$
.
Case 1. Assume d is even and
![]() $\mu _x=0$
. Then the orbifold group of
$\mu _x=0$
. Then the orbifold group of
![]() $x\in X$
has order
$x\in X$
has order
![]() $n^2$
. Since the finite-degree formula is true in dimension
$n^2$
. Since the finite-degree formula is true in dimension
![]() $2$
by [Reference Li, Liu and Xu52, Theorem 4.15], we have
$2$
by [Reference Li, Liu and Xu52, Theorem 4.15], we have
 $$ \begin{align*} \widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right) =n^2\cdot\widehat{\mathrm{vol}}(x,X,c D). \end{align*} $$
$$ \begin{align*} \widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right) =n^2\cdot\widehat{\mathrm{vol}}(x,X,c D). \end{align*} $$
On the other hand, Theorem 2.6 implies that
 $$ \begin{align*} 8\beta^2=(-K_X-cD)^2\leq \frac{9}{4}\widehat{\mathrm{vol}}(x, X, cD)=\frac{9}{4n^2}\widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right). \end{align*} $$
$$ \begin{align*} 8\beta^2=(-K_X-cD)^2\leq \frac{9}{4}\widehat{\mathrm{vol}}(x, X, cD)=\frac{9}{4n^2}\widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right). \end{align*} $$
So we have
 $$ \begin{align} n\leq \frac{3\sqrt{ \widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right)}}{4\sqrt{2}\beta} \leq \frac{3\left(2-c~\mathrm{ord}_{\tilde{x}}\widetilde{D}\right)}{4\sqrt{2}\beta}. \end{align} $$
$$ \begin{align} n\leq \frac{3\sqrt{ \widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right)}}{4\sqrt{2}\beta} \leq \frac{3\left(2-c~\mathrm{ord}_{\tilde{x}}\widetilde{D}\right)}{4\sqrt{2}\beta}. \end{align} $$
In particular, we have
 $n<\frac {3}{2\sqrt {2}\beta } $
. We know that
$n<\frac {3}{2\sqrt {2}\beta } $
. We know that
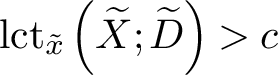 $\mathrm {lct}_{\tilde {x}}\left(\widetilde {X};\widetilde {D}\right)> c$
, and Skoda [Reference Skoda70] implies
$\mathrm {lct}_{\tilde {x}}\left(\widetilde {X};\widetilde {D}\right)> c$
, and Skoda [Reference Skoda70] implies
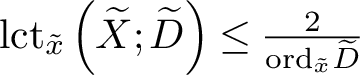 $\mathrm {lct}_{\tilde {x}}\left(\widetilde {X};\widetilde {D}\right)\leq \frac {2}{\mathrm {ord}_{\tilde {x}}\widetilde {D}}$
, so we have
$\mathrm {lct}_{\tilde {x}}\left(\widetilde {X};\widetilde {D}\right)\leq \frac {2}{\mathrm {ord}_{\tilde {x}}\widetilde {D}}$
, so we have
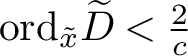 $ \mathrm {ord}_{\tilde {x}}\widetilde {D}<\frac {2}{c}$
. Since
$ \mathrm {ord}_{\tilde {x}}\widetilde {D}<\frac {2}{c}$
. Since
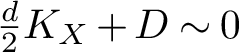 $\frac {d}{2}K_X+D\sim 0$
, we have
$\frac {d}{2}K_X+D\sim 0$
, we have
 $i+(na-1)j\equiv \frac {d}{2}na\mod n^2$
, which implies
$i+(na-1)j\equiv \frac {d}{2}na\mod n^2$
, which implies
![]() $i\equiv j\mod n$
.
$i\equiv j\mod n$
.
If
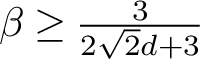 $\beta \geq \frac {3}{2\sqrt {2}d+3}$
, then
$\beta \geq \frac {3}{2\sqrt {2}d+3}$
, then
 $n<\frac {3}{2\sqrt {2}\beta }\leq d+\frac {3}{2\sqrt {2}}$
, which implies
$n<\frac {3}{2\sqrt {2}\beta }\leq d+\frac {3}{2\sqrt {2}}$
, which implies
![]() $n\leq d+1$
. Thus we may assume
$n\leq d+1$
. Thus we may assume
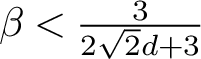 $\beta <\frac {3}{2\sqrt {2}d+3}$
. Then
$\beta <\frac {3}{2\sqrt {2}d+3}$
. Then
 $$ \begin{align*} i+j= \mathrm{ord}_{\tilde{x}}\widetilde{D}<\frac{2}{c}<d+\frac{3}{2\sqrt{2}}. \end{align*} $$
$$ \begin{align*} i+j= \mathrm{ord}_{\tilde{x}}\widetilde{D}<\frac{2}{c}<d+\frac{3}{2\sqrt{2}}. \end{align*} $$
Hence
![]() $i+j\leq d+1$
. Assume to the contrary that
$i+j\leq d+1$
. Assume to the contrary that
![]() $n\geq d+2$
. Then
$n\geq d+2$
. Then
![]() $i\equiv j\mod n$
and
$i\equiv j\mod n$
and
![]() $i+j<n$
implies that
$i+j<n$
implies that
![]() $i=j$
. Hence
$i=j$
. Hence
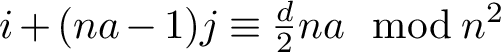 $i+(na-1)j\equiv \frac {d}{2}na\mod n^2$
implies
$i+(na-1)j\equiv \frac {d}{2}na\mod n^2$
implies
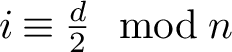 $i\equiv \frac {d}{2}\mod n$
. But since
$i\equiv \frac {d}{2}\mod n$
. But since
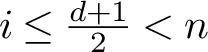 $i\leq \frac {d+1}{2}<n$
, we know that
$i\leq \frac {d+1}{2}<n$
, we know that
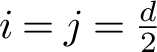 $i=j=\frac {d}{2}$
. Then formula (4.1) implies that
$i=j=\frac {d}{2}$
. Then formula (4.1) implies that
 $$ \begin{align*} n\leq \frac{3(2-c(i+j))}{4\sqrt{2}\beta}=\frac{6\beta}{4\sqrt{2}\beta}<2. \end{align*} $$
$$ \begin{align*} n\leq \frac{3(2-c(i+j))}{4\sqrt{2}\beta}=\frac{6\beta}{4\sqrt{2}\beta}<2. \end{align*} $$
We reach a contradiction.
Case 2. Assume d is even and
![]() $\mu _x=1$
. Then the orbifold group of
$\mu _x=1$
. Then the orbifold group of
![]() $x\in X$
has order
$x\in X$
has order
![]() $n^2$
. By a similar argument as in case 1, we know that
$n^2$
. By a similar argument as in case 1, we know that
 $$ \begin{align*} 8\beta^2=(-K_X-cD)^2\leq \frac{9}{4}\widehat{\mathrm{vol}}(x, X, cD)=\frac{9}{8n^2}\widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right). \end{align*} $$
$$ \begin{align*} 8\beta^2=(-K_X-cD)^2\leq \frac{9}{4}\widehat{\mathrm{vol}}(x, X, cD)=\frac{9}{8n^2}\widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right). \end{align*} $$
Hence
 $$ \begin{align} n\leq \frac{3\sqrt{ \widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right)}}{8\beta} \leq \frac{3\left(2-c~\mathrm{ord}_{\tilde{x}}\widetilde{D}\right)}{8\beta}. \end{align} $$
$$ \begin{align} n\leq \frac{3\sqrt{ \widehat{\mathrm{vol}}\left(\tilde{x},\widetilde{X},c\widetilde{D}\right)}}{8\beta} \leq \frac{3\left(2-c~\mathrm{ord}_{\tilde{x}}\widetilde{D}\right)}{8\beta}. \end{align} $$
In particular, we have
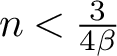 $n<\frac {3}{4\beta }$
.
$n<\frac {3}{4\beta }$
.
If
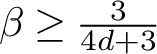 $\beta \geq \frac {3}{4d+3}$
, then
$\beta \geq \frac {3}{4d+3}$
, then
 $n<\frac {3}{4\beta }\leq d+\frac {3}{4}$
, which implies
$n<\frac {3}{4\beta }\leq d+\frac {3}{4}$
, which implies
![]() $n\leq d$
. Thus we may assume
$n\leq d$
. Thus we may assume
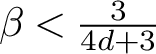 $\beta <\frac {3}{4d+3}$
. Then
$\beta <\frac {3}{4d+3}$
. Then
 $$ \begin{align*} i+j= \mathrm{ord}_{\tilde{x}}\widetilde{D}<\frac{2}{c} <d+\frac{3}{4}. \end{align*} $$
$$ \begin{align*} i+j= \mathrm{ord}_{\tilde{x}}\widetilde{D}<\frac{2}{c} <d+\frac{3}{4}. \end{align*} $$
Hence
![]() $i+j\leq d$
. Assume to the contrary that
$i+j\leq d$
. Assume to the contrary that
![]() $n\geq d+1$
. Then
$n\geq d+1$
. Then
![]() $i\equiv j\mod n$
and
$i\equiv j\mod n$
and
![]() $i+j<n$
implies
$i+j<n$
implies
![]() $i=j$
. Hence
$i=j$
. Hence
 $i+(na-1)j\equiv \frac {d}{2}na\mod n^2$
implies
$i+(na-1)j\equiv \frac {d}{2}na\mod n^2$
implies
 $i\equiv \frac {d}{2}\mod n$
. But since
$i\equiv \frac {d}{2}\mod n$
. But since
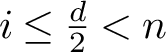 $i\leq \frac {d}{2}<n$
, we know that
$i\leq \frac {d}{2}<n$
, we know that
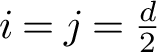 $i=j=\frac {d}{2}$
. Then formula (4.2) implies that
$i=j=\frac {d}{2}$
. Then formula (4.2) implies that
 $$ \begin{align*} n\leq \frac{3(2-c(i+j))}{8\beta}=\frac{6\beta}{8\beta}<1. \end{align*} $$
$$ \begin{align*} n\leq \frac{3(2-c(i+j))}{8\beta}=\frac{6\beta}{8\beta}<1. \end{align*} $$
We reach a contradiction.
Case 3. Assume d is odd and
![]() $\mu _x=0$
. In this case we have
$\mu _x=0$
. In this case we have
![]() $dK_X+2D\sim 0$
, which implies
$dK_X+2D\sim 0$
, which implies
![]() $2(i+(na-1)j)\equiv dna \mod n^2$
. If n is odd, then clearly
$2(i+(na-1)j)\equiv dna \mod n^2$
. If n is odd, then clearly
![]() $i\equiv j\mod n$
. By the same argument as case 1, we know
$i\equiv j\mod n$
. By the same argument as case 1, we know
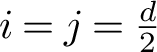 $i=j=\frac {d}{2}$
if
$i=j=\frac {d}{2}$
if
![]() $n\geq d+2$
, hence a contradiction.
$n\geq d+2$
, hence a contradiction.
If n is even, then we do a finer analysis. Since both d and a are odd, from
![]() $2(i+(na-1)j)\equiv dna \mod n^2$
we know that
$2(i+(na-1)j)\equiv dna \mod n^2$
we know that
![]() $i-j\equiv \frac {n}{2}\mod n$
. Thus
$i-j\equiv \frac {n}{2}\mod n$
. Thus
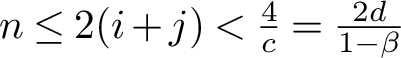 $n\leq 2(i+j)<\frac {4}{c}=\frac {2d}{1-\beta }$
. Besides, formula (4.1) implies that
$n\leq 2(i+j)<\frac {4}{c}=\frac {2d}{1-\beta }$
. Besides, formula (4.1) implies that
 $n<\frac {3}{2\sqrt {2}\beta }$
. Hence
$n<\frac {3}{2\sqrt {2}\beta }$
. Hence
 $$ \begin{align*} n<\min\left\{\frac{2d}{1-\beta},\frac{3}{2\sqrt{2}\beta}\right\}\leq \frac{2\sqrt{2}(2d)+3}{2\sqrt{2}(1-\beta)+2\sqrt{2}\beta}=2d+\frac{3}{2\sqrt{2}}. \end{align*} $$
$$ \begin{align*} n<\min\left\{\frac{2d}{1-\beta},\frac{3}{2\sqrt{2}\beta}\right\}\leq \frac{2\sqrt{2}(2d)+3}{2\sqrt{2}(1-\beta)+2\sqrt{2}\beta}=2d+\frac{3}{2\sqrt{2}}. \end{align*} $$
Thus
![]() $n\leq 2d$
. Assume to the contrary that
$n\leq 2d$
. Assume to the contrary that
![]() $n=2d$
; then
$n=2d$
; then
![]() $i+j\geq \frac {n}{2}=d$
. Hence formula (4.1) implies that
$i+j\geq \frac {n}{2}=d$
. Hence formula (4.1) implies that
 $$ \begin{align*} 2d=n\leq \frac{3(2-c(i+j))}{4\sqrt{2}\beta}\leq \frac{3(2-cd)}{4\sqrt{2}\beta}=\frac{3}{2\sqrt{2}}. \end{align*} $$
$$ \begin{align*} 2d=n\leq \frac{3(2-c(i+j))}{4\sqrt{2}\beta}\leq \frac{3(2-cd)}{4\sqrt{2}\beta}=\frac{3}{2\sqrt{2}}. \end{align*} $$
We reach a contradiction. Thus we have
![]() $n\leq 2d-2$
.
$n\leq 2d-2$
.
Case 4. Assume d is odd and
![]() $\mu _x=1$
. Then by [Reference Hacking and Prokhorov31, Proposition 2.6], we know that
$\mu _x=1$
. Then by [Reference Hacking and Prokhorov31, Proposition 2.6], we know that
![]() $\rho (X)=1$
. So n is odd by Proposition 4.6. Hence
$\rho (X)=1$
. So n is odd by Proposition 4.6. Hence
![]() $2(i+(2na-1)j)\equiv dna \mod n^2$
implies
$2(i+(2na-1)j)\equiv dna \mod n^2$
implies
![]() $i\equiv j\mod n$
. By a similar argument as in case 2, we know
$i\equiv j\mod n$
. By a similar argument as in case 2, we know
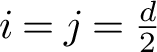 $i=j=\frac {d}{2}$
if
$i=j=\frac {d}{2}$
if
![]() $n\geq d+1$
, hence a contradiction.
$n\geq d+1$
, hence a contradiction.
The index bounds in Theorem 4.7 allow us to limit the surfaces that appear in pairs parametrized by the moduli stack
![]() $\overline {\mathcal {K}}_{c}$
.
$\overline {\mathcal {K}}_{c}$
.
Theorem 4.8. Let
![]() $(X,cD)$
be a K-semistable log Fano pair that admits a
$(X,cD)$
be a K-semistable log Fano pair that admits a
![]() $\mathbb {Q}$
-Gorenstein smoothing to
$\mathbb {Q}$
-Gorenstein smoothing to
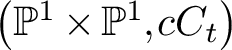 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC_t\right)$
, with
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC_t\right)$
, with
 $c\in \left(0,\frac {1}{2}\right)$
and
$c\in \left(0,\frac {1}{2}\right)$
and
![]() $C_t$
a
$C_t$
a
![]() $(4,4)$
curve. Then X must be isomorphic to either
$(4,4)$
curve. Then X must be isomorphic to either
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
or
$\mathbb {P}^1 \times \mathbb {P}^1$
or
![]() $\mathbb {P}(1,1,2)$
.
$\mathbb {P}(1,1,2)$
.
Proof. By Proposition 4.5, we know that
![]() $\rho (X) \leq 2$
. We start with
$\rho (X) \leq 2$
. We start with
![]() $\rho (X) = 1$
. In this case, by Proposition 4.6 we know that X is a weighted projective space of the form
$\rho (X) = 1$
. In this case, by Proposition 4.6 we know that X is a weighted projective space of the form
 $\mathbb {P}\left(a^2, b^2, 2c^2\right)$
, where
$\mathbb {P}\left(a^2, b^2, 2c^2\right)$
, where
![]() $a^2 + b^2 + 2c^2 = 4abc$
, or a partial smoothing. We begin enumerating the possible integer solutions and see that the first few are
$a^2 + b^2 + 2c^2 = 4abc$
, or a partial smoothing. We begin enumerating the possible integer solutions and see that the first few are
We can exclude the last two (and any with higher index) by the index bound of Theorem 4.7. The first gives
![]() $\mathbb {P}(1,1,2)$
and the second gives
$\mathbb {P}(1,1,2)$
and the second gives
![]() $\mathbb {P}(1,2,9)$
. We now show that the singularity
$\mathbb {P}(1,2,9)$
. We now show that the singularity
 $\frac {1}{9}(1,2)$
cannot appear.
$\frac {1}{9}(1,2)$
cannot appear.
Assume to the contrary that
![]() $x\in X$
is of type
$x\in X$
is of type
 $\frac {1}{9}(1,2)$
. Suppose
$\frac {1}{9}(1,2)$
. Suppose
![]() $D \sim -2K_X$
and consider a smooth covering
$D \sim -2K_X$
and consider a smooth covering
 $\left(\tilde {x} \in \widetilde {X}\right) \to (x \in X)$
. Note that we may assume
$\left(\tilde {x} \in \widetilde {X}\right) \to (x \in X)$
. Note that we may assume
![]() $x \in D$
, because otherwise
$x \in D$
, because otherwise
![]() $\mathrm {ind}(x,K_X) \leq 2$
, and we obtain a contradiction. Consider local coordinates of
$\mathrm {ind}(x,K_X) \leq 2$
, and we obtain a contradiction. Consider local coordinates of
![]() $\tilde {x} \in \widetilde {X}$
, namely
$\tilde {x} \in \widetilde {X}$
, namely
![]() $(u,v)$
. Let
$(u,v)$
. Let
![]() $u^i v^j$
be a monomial appearing in the equation on
$u^i v^j$
be a monomial appearing in the equation on
![]() $\widetilde {D}$
with minimum
$\widetilde {D}$
with minimum
![]() $i+j=\mathrm {ord}_{\tilde {x}}\widetilde {D}$
. Then
$i+j=\mathrm {ord}_{\tilde {x}}\widetilde {D}$
. Then
![]() $i + 2j \equiv 6 \mod 9$
. Since we know that
$i + 2j \equiv 6 \mod 9$
. Since we know that
![]() $(X, cD)$
is klt at x, we have that
$(X, cD)$
is klt at x, we have that
 $$ \begin{align*} \frac{2}{i+j} \geq \mathrm{lct}\left(\widetilde{D}\right)> c,\end{align*} $$
$$ \begin{align*} \frac{2}{i+j} \geq \mathrm{lct}\left(\widetilde{D}\right)> c,\end{align*} $$
and so in particular
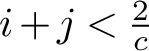 $i + j < \frac {2}{c}$
. By formula (4.1) with
$i + j < \frac {2}{c}$
. By formula (4.1) with
![]() $n=3$
and
$n=3$
and
![]() $\beta =1-2c$
, we have
$\beta =1-2c$
, we have
 $$ \begin{align*}2-(i+j)c \geq 4\sqrt{2}(1-2c).\end{align*} $$
$$ \begin{align*}2-(i+j)c \geq 4\sqrt{2}(1-2c).\end{align*} $$
Since this inequality holds for some
 $0 < c < \frac {1}{2}$
, we have
$0 < c < \frac {1}{2}$
, we have
![]() $i+j\leq 3$
, because otherwise
$i+j\leq 3$
, because otherwise
 $$ \begin{align*} 2-(i+j)c\leq 2-4c<4\sqrt{2}(1-2c), \end{align*} $$
$$ \begin{align*} 2-(i+j)c\leq 2-4c<4\sqrt{2}(1-2c), \end{align*} $$
which contradicts the previous inequality. Putting this together with
![]() $i + 2j = 6 \mod 9$
, we see that
$i + 2j = 6 \mod 9$
, we see that
![]() $(i, j) = (0,3)$
.
$(i, j) = (0,3)$
.
Consider the valuation w on
![]() $\widetilde {X}$
, which is the monomial valuation in the coordinates
$\widetilde {X}$
, which is the monomial valuation in the coordinates
![]() $(u,v)$
of weights
$(u,v)$
of weights
![]() $(1,2)$
. In particular,
$(1,2)$
. In particular,
 $w\left(\widetilde {D}\right) = 6$
. Moreover,
$w\left(\widetilde {D}\right) = 6$
. Moreover,
![]() $A_{\widetilde {X}}(w) = 3$
and
$A_{\widetilde {X}}(w) = 3$
and
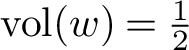 $\mathrm {vol}(w) = \frac {1}{2}$
. Then we note that
$\mathrm {vol}(w) = \frac {1}{2}$
. Then we note that
 $$ \begin{align*} \widehat{\mathrm{vol}}\left(\tilde{x}, \widetilde{X}, c\widetilde{D}\right) \leq \left(A_{\widetilde{X}}(w) - c~w\left(\widetilde{D}\right)\right)^2\mathrm{vol}(w) = \frac{(3-6c)^2}{2}. \end{align*} $$
$$ \begin{align*} \widehat{\mathrm{vol}}\left(\tilde{x}, \widetilde{X}, c\widetilde{D}\right) \leq \left(A_{\widetilde{X}}(w) - c~w\left(\widetilde{D}\right)\right)^2\mathrm{vol}(w) = \frac{(3-6c)^2}{2}. \end{align*} $$
By formula (4.1) we have
 $$ \begin{align*} 4\sqrt{2}(1-2c)\leq \sqrt{\widehat{\mathrm{vol}}\left(\tilde{x}, \widetilde{X}, c\widetilde{D}\right)}\leq \frac{3-6c}{\sqrt{2}}, \end{align*} $$
$$ \begin{align*} 4\sqrt{2}(1-2c)\leq \sqrt{\widehat{\mathrm{vol}}\left(\tilde{x}, \widetilde{X}, c\widetilde{D}\right)}\leq \frac{3-6c}{\sqrt{2}}, \end{align*} $$
which gives
 $4\sqrt {2} \leq \frac {3}{\sqrt {2}}$
, a contradiction. Thus the surface X with a
$4\sqrt {2} \leq \frac {3}{\sqrt {2}}$
, a contradiction. Thus the surface X with a
 $\frac {1}{9}(1,2)$
singularity cannot appear. In particular, the only surface with
$\frac {1}{9}(1,2)$
singularity cannot appear. In particular, the only surface with
![]() $\rho (X) = 1$
is
$\rho (X) = 1$
is
![]() $X \cong \mathbb {P}(1,1,2)$
.
$X \cong \mathbb {P}(1,1,2)$
.
Now we consider
![]() $\rho (X) = 2$
. By Proposition 4.6, we know that the only singular points of X are of the form
$\rho (X) = 2$
. By Proposition 4.6, we know that the only singular points of X are of the form
 $\frac {1}{n^2}(1, na-1)$
, with
$\frac {1}{n^2}(1, na-1)$
, with
![]() $n\leq 5$
. We already excluded
$n\leq 5$
. We already excluded
 $\frac {1}{9}(1,2)$
, so we only need to consider
$\frac {1}{9}(1,2)$
, so we only need to consider
![]() $n = 2, 4, 5$
.
$n = 2, 4, 5$
.
Let us consider
![]() $n=4$
, namely a singularity of type
$n=4$
, namely a singularity of type
 $\frac {1}{16}(1, 3)$
. We show that this singularity cannot occur. As before, consider a smooth covering
$\frac {1}{16}(1, 3)$
. We show that this singularity cannot occur. As before, consider a smooth covering
 $\left(\tilde {x} \in \widetilde {X}\right) \to (x \in X)$
and suppose
$\left(\tilde {x} \in \widetilde {X}\right) \to (x \in X)$
and suppose
![]() $D \sim -2K_X$
. Note that we may assume
$D \sim -2K_X$
. Note that we may assume
![]() $x \in D$
, because otherwise
$x \in D$
, because otherwise
![]() $\mathrm {ind}(x, K_X) \leq 2$
, and we obtain a contradiction. Consider local coordinates of
$\mathrm {ind}(x, K_X) \leq 2$
, and we obtain a contradiction. Consider local coordinates of
![]() $\tilde {x} \in \widetilde {X}$
, namely
$\tilde {x} \in \widetilde {X}$
, namely
![]() $(u,v)$
. Let
$(u,v)$
. Let
![]() $u^i v^j$
be a monomial appearing in the equation on
$u^i v^j$
be a monomial appearing in the equation on
![]() $\widetilde {D}$
with minimum
$\widetilde {D}$
with minimum
![]() $i+j=\mathrm {ord}_{\tilde {x}}\widetilde {D}$
. Then
$i+j=\mathrm {ord}_{\tilde {x}}\widetilde {D}$
. Then
![]() $i + 3j \equiv 8 \mod 16$
, and
$i + 3j \equiv 8 \mod 16$
, and
 $i + j < \frac {2}{c}$
. By formula (4.1) with
$i + j < \frac {2}{c}$
. By formula (4.1) with
![]() $n=4$
and
$n=4$
and
![]() $\beta =1-2c$
, we have
$\beta =1-2c$
, we have
 $$ \begin{align*} 4 \sqrt{2} (1-2c) \leq (2-c(i+j)).\end{align*} $$
$$ \begin{align*} 4 \sqrt{2} (1-2c) \leq (2-c(i+j)).\end{align*} $$
Since this inequality holds for some
 $0 < c < \frac {1}{2}$
, we have
$0 < c < \frac {1}{2}$
, we have
![]() $i+j\leq 3$
by the same reason in
$i+j\leq 3$
by the same reason in
![]() $n=3$
. This contradicts
$n=3$
. This contradicts
![]() $i+3j\equiv 8\mod 16$
. In particular, a singularity of type
$i+3j\equiv 8\mod 16$
. In particular, a singularity of type
 $\frac {1}{16}(1,3)$
cannot occur.
$\frac {1}{16}(1,3)$
cannot occur.
Next let us consider
![]() $n=5$
, namely a singularity of type
$n=5$
, namely a singularity of type
 $\frac {1}{25}(1,4)$
or
$\frac {1}{25}(1,4)$
or
 $\frac {1}{25}(1,9)$
. We again show that these singularities cannot occur. With the same setup as the previous paragraph, we have either
$\frac {1}{25}(1,9)$
. We again show that these singularities cannot occur. With the same setup as the previous paragraph, we have either
![]() $i+4j\equiv 10 \mod 25$
or
$i+4j\equiv 10 \mod 25$
or
![]() $i+9j\equiv 20 \mod 25$
. Moreover, we again have
$i+9j\equiv 20 \mod 25$
. Moreover, we again have
![]() $i+j\leq 3$
by the same reason in
$i+j\leq 3$
by the same reason in
![]() $n=3,4$
, but this contradicts the congruence equations. Therefore, a singularity of type
$n=3,4$
, but this contradicts the congruence equations. Therefore, a singularity of type
 $\frac {1}{25}(1,4)$
or
$\frac {1}{25}(1,4)$
or
 $\frac {1}{25}(1,9)$
cannot occur.
$\frac {1}{25}(1,9)$
cannot occur.
After these discussions, the only case left to study is
![]() $\rho (X)=2$
where X has only singularities of type
$\rho (X)=2$
where X has only singularities of type
 $\frac {1}{4}(1,1)$
. If X is singular, then by [Reference Nakayama62, Table 6 and Theorem 7.15] (see also [Reference Alexeev and Nikulin2]), we know that X is isomorphic to a blowup of
$\frac {1}{4}(1,1)$
. If X is singular, then by [Reference Nakayama62, Table 6 and Theorem 7.15] (see also [Reference Alexeev and Nikulin2]), we know that X is isomorphic to a blowup of
![]() $\mathbb {P}(1,1,4)$
at a smooth point. However, in this case X admits a
$\mathbb {P}(1,1,4)$
at a smooth point. However, in this case X admits a
![]() $\mathbb {Q}$
-Gorenstein smoothing to the Hirzebruch surface
$\mathbb {Q}$
-Gorenstein smoothing to the Hirzebruch surface
![]() $\mathbb {F}_1$
, which is not homeomorphic to
$\mathbb {F}_1$
, which is not homeomorphic to
![]() $\mathbb {P}^1\times \mathbb {P}^1$
. This is a contradiction. Hence X is smooth and isomorphic to
$\mathbb {P}^1\times \mathbb {P}^1$
. This is a contradiction. Hence X is smooth and isomorphic to
![]() $\mathbb {P}^1\times \mathbb {P}^1$
.
$\mathbb {P}^1\times \mathbb {P}^1$
.
Remark 4.9. Let
![]() $(X,cD)$
be a K-semistable log Fano pair that admits a
$(X,cD)$
be a K-semistable log Fano pair that admits a
![]() $\mathbb {Q}$
-Gorenstein smoothing to
$\mathbb {Q}$
-Gorenstein smoothing to
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC_t\right)$
, with
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC_t\right)$
, with
 $c\in \left(0,\frac {1}{2}\right)$
and
$c\in \left(0,\frac {1}{2}\right)$
and
![]() $C_t$
a
$C_t$
a
![]() $(4,4)$
curve. By Theorem 4.8, this implies that X is either
$(4,4)$
curve. By Theorem 4.8, this implies that X is either
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
or
$\mathbb {P}^1 \times \mathbb {P}^1$
or
![]() $\mathbb {P}(1,1,2)$
. Therefore, there exists a closed embedding
$\mathbb {P}(1,1,2)$
. Therefore, there exists a closed embedding
![]() $(X,D)\hookrightarrow \mathbb {P}^3$
such that
$(X,D)\hookrightarrow \mathbb {P}^3$
such that
![]() $X \in \lvert \mathcal {O}_{\mathbb {P}^3}(2)\rvert $
and
$X \in \lvert \mathcal {O}_{\mathbb {P}^3}(2)\rvert $
and
![]() $D\sim -2K_X$
are
$D\sim -2K_X$
are
![]() $(2,4)$
-complete intersections inside
$(2,4)$
-complete intersections inside
![]() $\mathbb {P}^3$
. Hence, all K-semistable pairs
$\mathbb {P}^3$
. Hence, all K-semistable pairs
![]() $(X, cD)$
with
$(X, cD)$
with
 $c \in \left(0,\frac {1}{2}\right)$
are parametrized by a Zariski open subset of U.
$c \in \left(0,\frac {1}{2}\right)$
are parametrized by a Zariski open subset of U.
Theorem 4.10. Let
![]() $(X,cD)$
be a K-semistable log Fano pair that admits a
$(X,cD)$
be a K-semistable log Fano pair that admits a
![]() $\mathbb {Q}$
-Gorenstein smoothing to
$\mathbb {Q}$
-Gorenstein smoothing to
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC_t\right)$
, with
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC_t\right)$
, with
 $c\in \left(0,\frac {4-\sqrt {2}}{2d}\right)$
and
$c\in \left(0,\frac {4-\sqrt {2}}{2d}\right)$
and
![]() $C_t$
a
$C_t$
a
![]() $(d,d)$
curve where
$(d,d)$
curve where
![]() $d \geq 3$
. Then X must be either
$d \geq 3$
. Then X must be either
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
or
$\mathbb {P}^1 \times \mathbb {P}^1$
or
![]() $\mathbb {P}(1,1,2)$
.
$\mathbb {P}(1,1,2)$
.
Proof. By Proposition 4.5,
![]() $\rho (X) \leq 2$
. By the index bound of Theorem 4.7, for
$\rho (X) \leq 2$
. By the index bound of Theorem 4.7, for
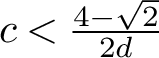 $ c < \frac {4 - \sqrt {2}}{2d}$
we know that
$ c < \frac {4 - \sqrt {2}}{2d}$
we know that
![]() $\mathrm {ind}(x, K_X) < 3$
. If
$\mathrm {ind}(x, K_X) < 3$
. If
![]() $\rho (X)=1$
, then by Proposition 4.6 we know that X is Gorenstein, which implies that
$\rho (X)=1$
, then by Proposition 4.6 we know that X is Gorenstein, which implies that
![]() $X\cong \mathbb {P}(1,1,2)$
. If
$X\cong \mathbb {P}(1,1,2)$
. If
![]() $\rho (X)=2$
, then by Proposition 4.6 we know that either X is smooth, hence isomorphic to
$\rho (X)=2$
, then by Proposition 4.6 we know that either X is smooth, hence isomorphic to
![]() $\mathbb {P}^1\times \mathbb {P}^1$
, or X has only singularities of type
$\mathbb {P}^1\times \mathbb {P}^1$
, or X has only singularities of type
 $\frac {1}{4}(1,1)$
. The latter case cannot happen, by the end of the proof of Theorem 4.8. Therefore, the only surfaces appearing are
$\frac {1}{4}(1,1)$
. The latter case cannot happen, by the end of the proof of Theorem 4.8. Therefore, the only surfaces appearing are
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
and
$\mathbb {P}^1 \times \mathbb {P}^1$
and
![]() $\mathbb {P}(1,1,2)$
.
$\mathbb {P}(1,1,2)$
.
5 Wall crossings for K-moduli and GIT
In this section we prove Theorem 1.1 – that is, for
 $0<c < \frac {1}{2}$
, the K-moduli stack
$0<c < \frac {1}{2}$
, the K-moduli stack
![]() $\overline {\mathcal {K}}_{c}$
coincides with the GIT moduli stack
$\overline {\mathcal {K}}_{c}$
coincides with the GIT moduli stack
![]() $\mathscr {M}(t)$
with
$\mathscr {M}(t)$
with
 $t=\frac {3c}{2c+2}$
(see Definition 3.2). The important observation comes from Theorem 4.8: the surfaces X in the pairs parametrized by
$t=\frac {3c}{2c+2}$
(see Definition 3.2). The important observation comes from Theorem 4.8: the surfaces X in the pairs parametrized by
![]() $\overline {\mathcal {K}}_{c}$
are
$\overline {\mathcal {K}}_{c}$
are
![]() $\mathbb {P}^1 \times \mathbb {P}^1$
or
$\mathbb {P}^1 \times \mathbb {P}^1$
or
![]() $\mathbb {P}(1,1,2)$
, which are quadric surfaces in
$\mathbb {P}(1,1,2)$
, which are quadric surfaces in
![]() $\mathbb {P}^3$
, and the divisors D can therefore be viewed as
$\mathbb {P}^3$
, and the divisors D can therefore be viewed as
![]() $(2,4)$
-complete intersections in
$(2,4)$
-complete intersections in
![]() $\mathbb {P}^3$
.
$\mathbb {P}^3$
.
5.1 The first wall crossing
In this section, we show that GIT-(poly/semi)stability of
![]() $(4,4)$
curves on
$(4,4)$
curves on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
and c-K-(poly/semi)stability coincide for
$\mathbb {P}^1\times \mathbb {P}^1$
and c-K-(poly/semi)stability coincide for
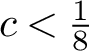 $c < \frac 1{8}$
. Moreover, we show that
$c < \frac 1{8}$
. Moreover, we show that
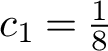 $c_1=\frac {1}{8}$
is the first wall for K-moduli stacks
$c_1=\frac {1}{8}$
is the first wall for K-moduli stacks
![]() $\overline {\mathcal {K}}_c$
.
$\overline {\mathcal {K}}_c$
.
Definition 5.1. A
![]() $(4,4)$
curve C on
$(4,4)$
curve C on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
gives a point
$\mathbb {P}^1\times \mathbb {P}^1$
gives a point
![]() $[C] \in \mathbf {P}_{4,4}:= \mathbb {P}(H^0(\mathbb {P}^1\times \mathbb {P}^1, \mathcal {O}(4,4)))$
. We say C is GIT-(poly/semi)stable if
$[C] \in \mathbf {P}_{4,4}:= \mathbb {P}(H^0(\mathbb {P}^1\times \mathbb {P}^1, \mathcal {O}(4,4)))$
. We say C is GIT-(poly/semi)stable if
![]() $[C]$
is GIT-(poly/semi)stable with respect to the natural
$[C]$
is GIT-(poly/semi)stable with respect to the natural
 $\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
-action on
$\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
-action on
![]() $\left(\mathbf {P}_{4,4}, \mathcal {O}(2)\right)$
. We define the GIT quotient stack
$\left(\mathbf {P}_{4,4}, \mathcal {O}(2)\right)$
. We define the GIT quotient stack
![]() $\mathscr {M}$
and the GIT quotient space
$\mathscr {M}$
and the GIT quotient space
![]() $\mathfrak {M}$
as
$\mathfrak {M}$
as
 $$ \begin{align*} \mathscr{M}:=\left[\mathbf{P}_{4,4}^{\textrm{ss}}/\mathrm{Aut}\left(\mathbb{P}^1\times\mathbb{P}^1\right)\right], \qquad \mathfrak{M}:=\mathbf{P}_{4,4}^{\textrm{ss}}\mathbin{/\mkern-6mu/} \mathrm{Aut}\left(\mathbb{P}^1\times\mathbb{P}^1\right). \end{align*} $$
$$ \begin{align*} \mathscr{M}:=\left[\mathbf{P}_{4,4}^{\textrm{ss}}/\mathrm{Aut}\left(\mathbb{P}^1\times\mathbb{P}^1\right)\right], \qquad \mathfrak{M}:=\mathbf{P}_{4,4}^{\textrm{ss}}\mathbin{/\mkern-6mu/} \mathrm{Aut}\left(\mathbb{P}^1\times\mathbb{P}^1\right). \end{align*} $$
Theorem 5.2. For any
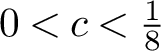 $0 < c < \frac 1{8}$
, a curve
$0 < c < \frac 1{8}$
, a curve
![]() $C \subset \mathbb {P}^1\times \mathbb {P}^1$
of bidgree
$C \subset \mathbb {P}^1\times \mathbb {P}^1$
of bidgree
![]() $(4,4)$
is GIT-(poly/semi)stable if and only if the log Fano pair
$(4,4)$
is GIT-(poly/semi)stable if and only if the log Fano pair
 $\left(\mathbb {P}^1 \times \mathbb {P}^1, cC\right)$
is K-(poly/semi)stable. Moreover, there is an isomorphism of Artin stacks
$\left(\mathbb {P}^1 \times \mathbb {P}^1, cC\right)$
is K-(poly/semi)stable. Moreover, there is an isomorphism of Artin stacks
![]() $\overline {\mathcal {K}}_{c} \cong \mathscr {M}$
.
$\overline {\mathcal {K}}_{c} \cong \mathscr {M}$
.
Proof. We first show that the K-(poly/semi)stability of
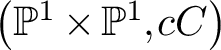 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
implies GIT-(poly/semi)stability of C for any
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
implies GIT-(poly/semi)stability of C for any
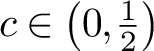 $c\in \left(0,\frac {1}{2}\right)$
. Consider the universal family
$c\in \left(0,\frac {1}{2}\right)$
. Consider the universal family
 $\pi : \left(\mathbb {P}^1 \times \mathbb {P}^1 \times \mathbf {P}_{\left(4,4\right)}, c\mathcal C\right) \to \mathbf {P}_{\left(4,4\right)}$
over the parameter space of
$\pi : \left(\mathbb {P}^1 \times \mathbb {P}^1 \times \mathbf {P}_{\left(4,4\right)}, c\mathcal C\right) \to \mathbf {P}_{\left(4,4\right)}$
over the parameter space of
![]() $(4,4)$
curves on
$(4,4)$
curves on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
. It is clear that
$\mathbb {P}^1\times \mathbb {P}^1$
. It is clear that
![]() $\mathcal C \in \lvert \mathcal {O}(4,4,1)\rvert $
. Hence by Proposition 2.17 we have
$\mathcal C \in \lvert \mathcal {O}(4,4,1)\rvert $
. Hence by Proposition 2.17 we have
 $$ \begin{align*} \lambda_{\mathrm{CM}, \pi, c\mathcal C}& = -\pi_* \left(-K_{\mathbb{P}^1 \times \mathbb{P}^1 \times \mathbf{P}_{\left(4,4\right)}/\mathbf{P}_{\left(4,4\right)}}-c\mathcal C\right)^3 = -\pi_* (\mathcal{O}(2-4c,2-4c,-c))^3 \\ & = -3\left(\mathcal{O}_{\mathbb{P}^1\times\mathbb{P}^1}(2-4c,2-4c)^2\right) \mathcal{O}_{\mathbf{P}_{\left(4,4\right)}}(-c) = \mathcal{O}_{\mathbf{P}_{\left(4,4\right)}}\left(3(2-4c)^2 c\right). \end{align*} $$
$$ \begin{align*} \lambda_{\mathrm{CM}, \pi, c\mathcal C}& = -\pi_* \left(-K_{\mathbb{P}^1 \times \mathbb{P}^1 \times \mathbf{P}_{\left(4,4\right)}/\mathbf{P}_{\left(4,4\right)}}-c\mathcal C\right)^3 = -\pi_* (\mathcal{O}(2-4c,2-4c,-c))^3 \\ & = -3\left(\mathcal{O}_{\mathbb{P}^1\times\mathbb{P}^1}(2-4c,2-4c)^2\right) \mathcal{O}_{\mathbf{P}_{\left(4,4\right)}}(-c) = \mathcal{O}_{\mathbf{P}_{\left(4,4\right)}}\left(3(2-4c)^2 c\right). \end{align*} $$
Hence the CM line bundle
![]() $\lambda _{\mathrm {CM}, \pi , c\mathcal C}$
is ample whenever
$\lambda _{\mathrm {CM}, \pi , c\mathcal C}$
is ample whenever
 $c\in \left(0, \frac {1}{2}\right)$
. Hence the statement of K implying GIT directly follows from Theorem 2.16.
$c\in \left(0, \frac {1}{2}\right)$
. Hence the statement of K implying GIT directly follows from Theorem 2.16.
Next we show the converse – that is, that the GIT-(poly/semi)stability of C implies K-(poly/semi)stability of
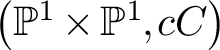 $\left(\mathbb {P}^1\times \mathbb {P}^1,cC\right)$
for
$\left(\mathbb {P}^1\times \mathbb {P}^1,cC\right)$
for
 $c< \frac {1}{8}$
. Indeed, using a similar argument as the proof of [Reference Ascher, DeVleming and Liu6, Theorem 5.2], with a key ingredient from properness of K-moduli spaces, it suffices to show that any pair
$c< \frac {1}{8}$
. Indeed, using a similar argument as the proof of [Reference Ascher, DeVleming and Liu6, Theorem 5.2], with a key ingredient from properness of K-moduli spaces, it suffices to show that any pair
![]() $(X,D)$
appearing in the K-moduli stack
$(X,D)$
appearing in the K-moduli stack
![]() $\overline {\mathcal {K}}_c$
for
$\overline {\mathcal {K}}_c$
for
 $c < \frac {1}{8}$
satisfies the conditions that
$c < \frac {1}{8}$
satisfies the conditions that
![]() $X\cong \mathbb {P}^1\times \mathbb {P}^1$
and D is a
$X\cong \mathbb {P}^1\times \mathbb {P}^1$
and D is a
![]() $(4,4)$
curve. Since
$(4,4)$
curve. Since
![]() $\mathbb {P}^1\times \mathbb {P}^1$
has no nontrivial smooth degeneration, it suffices to show that X is smooth. Assume to the contrary that X is singular at a point
$\mathbb {P}^1\times \mathbb {P}^1$
has no nontrivial smooth degeneration, it suffices to show that X is smooth. Assume to the contrary that X is singular at a point
![]() $x\in X$
. Then by [Reference Li and Liu51] we know that
$x\in X$
. Then by [Reference Li and Liu51] we know that
 $$ \begin{align*} 8(1-2c)^2=(-K_X-cD)^2\leq \frac{9}{4}\widehat{\mathrm{vol}}(x,X,cD)\leq \frac{9}{4}\widehat{\mathrm{vol}}(x,X)\leq \frac{9}{2}. \end{align*} $$
$$ \begin{align*} 8(1-2c)^2=(-K_X-cD)^2\leq \frac{9}{4}\widehat{\mathrm{vol}}(x,X,cD)\leq \frac{9}{4}\widehat{\mathrm{vol}}(x,X)\leq \frac{9}{2}. \end{align*} $$
This implies that
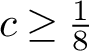 $c\geq \frac {1}{8}$
, which is a contradiction. Hence for
$c\geq \frac {1}{8}$
, which is a contradiction. Hence for
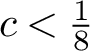 $c < \frac {1}{8}$
, a K-semistable pair
$c < \frac {1}{8}$
, a K-semistable pair
![]() $(X,cD)$
must be isomorphic to
$(X,cD)$
must be isomorphic to
 $\left(\mathbb {P}^1 \times \mathbb {P}^1, cC\right)$
, where C is a
$\left(\mathbb {P}^1 \times \mathbb {P}^1, cC\right)$
, where C is a
![]() $(4,4)$
curve.
$(4,4)$
curve.
Summing up, the equivalence of K-(poly/semi)stability with GIT-(poly/semi)stability yields a morphism
![]() $\phi : \mathscr {M}\to \overline {\mathcal {K}}_{c}$
which descends to an isomorphism
$\phi : \mathscr {M}\to \overline {\mathcal {K}}_{c}$
which descends to an isomorphism
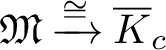 $\mathfrak {M}\xrightarrow {\cong }\overline {K}_c$
. To conclude, it suffices to show that
$\mathfrak {M}\xrightarrow {\cong }\overline {K}_c$
. To conclude, it suffices to show that
![]() $\phi $
is an isomorphism between Artin stacks. The proof is similar to [Reference Ascher, DeVleming and Liu6, Theorem 3.24]. Denote
$\phi $
is an isomorphism between Artin stacks. The proof is similar to [Reference Ascher, DeVleming and Liu6, Theorem 3.24]. Denote
![]() $T:=\mathbf {P}_{4,4}^{\textrm {ss}}$
. Let
$T:=\mathbf {P}_{4,4}^{\textrm {ss}}$
. Let
![]() $\pi :(\mathcal X,\mathcal D)\to T$
be the universal family. Recall from [Reference Ascher, DeVleming and Liu6, Section 3.1] and Theorem 2.21 that
$\pi :(\mathcal X,\mathcal D)\to T$
be the universal family. Recall from [Reference Ascher, DeVleming and Liu6, Section 3.1] and Theorem 2.21 that
![]() $\overline {\mathcal {K}}_c\cong \left[Z_{c}^\circ /\mathrm {PGL}(N_m+1)\right]$
, where
$\overline {\mathcal {K}}_c\cong \left[Z_{c}^\circ /\mathrm {PGL}(N_m+1)\right]$
, where
![]() $Z_{c}^\circ $
is the K-semistable locus in the Hilbert scheme of embedded by mth multiple of anticanonical divisors. Denote by
$Z_{c}^\circ $
is the K-semistable locus in the Hilbert scheme of embedded by mth multiple of anticanonical divisors. Denote by
![]() $\pi ': (\mathcal X',\mathcal D')\to T'$
the universal family over
$\pi ': (\mathcal X',\mathcal D')\to T'$
the universal family over
![]() $T':=Z_c^\circ $
. Let P be the
$T':=Z_c^\circ $
. Let P be the
![]() $\mathrm {PGL}(N_m+1)$
-torsor over T induced from the vector bundle
$\mathrm {PGL}(N_m+1)$
-torsor over T induced from the vector bundle
 $\pi _*\mathcal {O}_{\mathcal X}\left(-mK_{\mathcal X/T}\right)$
. Then from [Reference Ascher, DeVleming and Liu6, Proof of Theorem 3.24] we see that there is an
$\pi _*\mathcal {O}_{\mathcal X}\left(-mK_{\mathcal X/T}\right)$
. Then from [Reference Ascher, DeVleming and Liu6, Proof of Theorem 3.24] we see that there is an
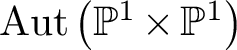 $\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
-equivariant morphism
$\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
-equivariant morphism
![]() $\psi : P\to T'$
whose descent is precisely
$\psi : P\to T'$
whose descent is precisely
![]() $\phi $
. Hence, to show that
$\phi $
. Hence, to show that
![]() $\phi $
is isomorphic it suffices to show that
$\phi $
is isomorphic it suffices to show that
![]() $\psi $
provides an
$\psi $
provides an
 $\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
-torsor. Indeed, since
$\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
-torsor. Indeed, since
![]() $\pi ':\mathcal X'\to T'$
is isotrivial where all fibers are isomorphic to
$\pi ':\mathcal X'\to T'$
is isotrivial where all fibers are isomorphic to
![]() $\mathbb {P}^1\times \mathbb {P}^1$
, we may find an étale covering
$\mathbb {P}^1\times \mathbb {P}^1$
, we may find an étale covering
![]() $\cup _i V_i\twoheadrightarrow T'$
such that there is an isomorphism
$\cup _i V_i\twoheadrightarrow T'$
such that there is an isomorphism
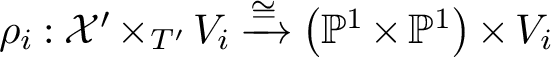 $\rho _i: \mathcal X'\times _{T'} V_i\xrightarrow {\cong } \left(\mathbb {P}^1\times \mathbb {P}^1\right)\times V_i$
. Hence by pushing forward
$\rho _i: \mathcal X'\times _{T'} V_i\xrightarrow {\cong } \left(\mathbb {P}^1\times \mathbb {P}^1\right)\times V_i$
. Hence by pushing forward
![]() $(\mathcal X',\mathcal D')\times _{T'} V_i$
and its natural frame from
$(\mathcal X',\mathcal D')\times _{T'} V_i$
and its natural frame from
![]() $\mathbb {P}^{N_m+1}$
to
$\mathbb {P}^{N_m+1}$
to
 $\left(\mathbb {P}^1\times \mathbb {P}^1\right)\times V_i$
under
$\left(\mathbb {P}^1\times \mathbb {P}^1\right)\times V_i$
under
![]() $\rho _i$
, we obtain a section
$\rho _i$
, we obtain a section
![]() $V_i\to P\times _{T'}V_i$
of
$V_i\to P\times _{T'}V_i$
of
![]() $\psi \times _{T'}V_i$
which trivializes
$\psi \times _{T'}V_i$
which trivializes
![]() $\psi $
. Thus the proof is finished.
$\psi $
. Thus the proof is finished.
The following proposition shows that
 $c_1=\frac {1}{8}$
is the first wall of the K-moduli stacks
$c_1=\frac {1}{8}$
is the first wall of the K-moduli stacks
![]() $\overline {\mathcal {K}}_c$
. Note that it is also proved by Fujita [Reference Fujita27] independently using different methods.
$\overline {\mathcal {K}}_c$
. Note that it is also proved by Fujita [Reference Fujita27] independently using different methods.
Proposition 5.3. Let
![]() $C=4H$
, where H is a smooth
$C=4H$
, where H is a smooth
![]() $(1,1)$
-curve on
$(1,1)$
-curve on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
. Let
$\mathbb {P}^1\times \mathbb {P}^1$
. Let
 $c\in \left(0,\frac {1}{2}\right)$
be a rational number. Then
$c\in \left(0,\frac {1}{2}\right)$
be a rational number. Then
 $\left(\mathbb {P}^1\times \mathbb {P}^1,cC\right)$
is K-semistable (resp., K-polystable) if and only if
$\left(\mathbb {P}^1\times \mathbb {P}^1,cC\right)$
is K-semistable (resp., K-polystable) if and only if
 $c\leq \frac {1}{8}$
(resp.
$c\leq \frac {1}{8}$
(resp.
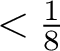 $<\frac {1}{8}$
). Moreover, the K-polystable degeneration of
$<\frac {1}{8}$
). Moreover, the K-polystable degeneration of
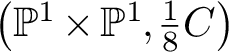 $\left(\mathbb {P}^1\times \mathbb {P}^1,\frac {1}{8}C\right)$
is isomorphic to
$\left(\mathbb {P}^1\times \mathbb {P}^1,\frac {1}{8}C\right)$
is isomorphic to
 $\left(\mathbb {P}(1,1,2), \frac {1}{8}C_0\right)$
, where
$\left(\mathbb {P}(1,1,2), \frac {1}{8}C_0\right)$
, where
![]() $C_0=4 H_0$
and
$C_0=4 H_0$
and
![]() $H_0$
is the section at infinity.
$H_0$
is the section at infinity.
Proof. We first show that
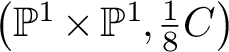 $\left(\mathbb {P}^1\times \mathbb {P}^1,\frac {1}{8}C\right)$
is K-semistable where
$\left(\mathbb {P}^1\times \mathbb {P}^1,\frac {1}{8}C\right)$
is K-semistable where
 $\left(\mathbb {P}(1,1,2), \frac {1}{8}C_0\right)$
is its K-polystable degeneration. Choose an embedding
$\left(\mathbb {P}(1,1,2), \frac {1}{8}C_0\right)$
is its K-polystable degeneration. Choose an embedding
![]() $\mathbb {P}^1\times \mathbb {P}^1\hookrightarrow \mathbb {P}^3$
as a smooth quadric surface. Then H is a hyperplane section of
$\mathbb {P}^1\times \mathbb {P}^1\hookrightarrow \mathbb {P}^3$
as a smooth quadric surface. Then H is a hyperplane section of
![]() $\mathbb {P}^1\times \mathbb {P}^1$
. Pick projective coordinates
$\mathbb {P}^1\times \mathbb {P}^1$
. Pick projective coordinates
![]() $[x_0,x_1,x_2,x_3]$
of
$[x_0,x_1,x_2,x_3]$
of
![]() $\mathbb {P}^3$
such that the hyperplane section through H is given by
$\mathbb {P}^3$
such that the hyperplane section through H is given by
![]() $x_3=0$
. Then the
$x_3=0$
. Then the
![]() $1$
-PS
$1$
-PS
![]() $\sigma : \mathbb {G}_m \to \mathrm {PGL}(4)$
given by
$\sigma : \mathbb {G}_m \to \mathrm {PGL}(4)$
given by
![]() $\sigma (t)[x_0,x_1,x_2,x_3]=[tx_0,tx_1,tx_2,x_3]$
provides a special test configuration of
$\sigma (t)[x_0,x_1,x_2,x_3]=[tx_0,tx_1,tx_2,x_3]$
provides a special test configuration of
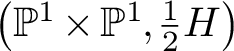 $\left(\mathbb {P}^1\times \mathbb {P}^1, \frac {1}{2}H\right)$
whose central fiber is an ordinary quadric cone with a section at infinity of coefficient
$\left(\mathbb {P}^1\times \mathbb {P}^1, \frac {1}{2}H\right)$
whose central fiber is an ordinary quadric cone with a section at infinity of coefficient
![]() $\frac {1}{2}$
– that is, isomorphic to
$\frac {1}{2}$
– that is, isomorphic to
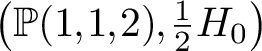 $\left(\mathbb {P}(1,1,2), \frac {1}{2}H_0\right)$
. By [Reference Li and Liu51] we know that
$\left(\mathbb {P}(1,1,2), \frac {1}{2}H_0\right)$
. By [Reference Li and Liu51] we know that
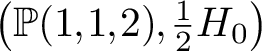 $\left(\mathbb {P}(1,1,2), \frac {1}{2}H_0\right)$
admits a conical Kähler–Einstein metric, hence is K-polystable. The K-semistability of
$\left(\mathbb {P}(1,1,2), \frac {1}{2}H_0\right)$
admits a conical Kähler–Einstein metric, hence is K-polystable. The K-semistability of
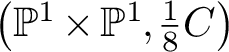 $\left(\mathbb {P}^1\times \mathbb {P}^1,\frac {1}{8}C\right)$
follows from the openness of K-semistability [Reference Blum, Liu and Xu12, Reference Xu15].
$\left(\mathbb {P}^1\times \mathbb {P}^1,\frac {1}{8}C\right)$
follows from the openness of K-semistability [Reference Blum, Liu and Xu12, Reference Xu15].
Next we show that
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
is K-polystable for
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
is K-polystable for
 $c\in \left(0,\frac {1}{8}\right)$
. Clearly, it is K-semistable by interpolation [Reference Ascher, DeVleming and Liu6, Proposition 2.13]. Let
$c\in \left(0,\frac {1}{8}\right)$
. Clearly, it is K-semistable by interpolation [Reference Ascher, DeVleming and Liu6, Proposition 2.13]. Let
![]() $(X,cD)$
be its K-polystable degeneration. By Theorem 5.2, we know that
$(X,cD)$
be its K-polystable degeneration. By Theorem 5.2, we know that
![]() $X\cong \mathbb {P}^1\times \mathbb {P}^1$
. Since
$X\cong \mathbb {P}^1\times \mathbb {P}^1$
. Since
![]() $C=4H$
, we have
$C=4H$
, we have
![]() $D=4H_0$
for some
$D=4H_0$
for some
![]() $(1,1)$
-curve
$(1,1)$
-curve
![]() $H_0$
. If
$H_0$
. If
![]() $H_0$
is reducible, then
$H_0$
is reducible, then
![]() $(X,cD)$
is isomorphic to the self-product of
$(X,cD)$
is isomorphic to the self-product of
 $\left(\mathbb {P}^1, c[0]\right)$
. Since
$\left(\mathbb {P}^1, c[0]\right)$
. Since
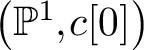 $\left(\mathbb {P}^1, c[0]\right)$
is K-unstable, we know that
$\left(\mathbb {P}^1, c[0]\right)$
is K-unstable, we know that
![]() $(X,cD)$
is also K-unstable by [Reference Zhuang78]. Thus
$(X,cD)$
is also K-unstable by [Reference Zhuang78]. Thus
![]() $H_0$
must be irreducible, which implies that
$H_0$
must be irreducible, which implies that
 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)\cong (X,cD)$
is K-polystable. Thus the proof is finished.
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)\cong (X,cD)$
is K-polystable. Thus the proof is finished.
Remark 5.4.
-
(1) The first K-moduli wall crossing at
 $c_1 = \frac {1}{8}$
has the following diagram: where the composition
$c_1 = \frac {1}{8}$
has the following diagram: where the composition $$ \begin{align*} \overline{K}_{\frac{1}{8}+\epsilon}\xrightarrow{\phi_1^+} \overline{K}_{\frac{1}{8}} \xleftarrow[\cong]{\phi_1^-} \overline{K}_{\frac{1}{8}-\epsilon}= \mathfrak{M}, \end{align*} $$
$$ \begin{align*} \overline{K}_{\frac{1}{8}+\epsilon}\xrightarrow{\phi_1^+} \overline{K}_{\frac{1}{8}} \xleftarrow[\cong]{\phi_1^-} \overline{K}_{\frac{1}{8}-\epsilon}= \mathfrak{M}, \end{align*} $$
 $\left(\phi _1^{-}\right)^{-1}\circ \phi _1^+: \overline {K}_{\frac {1}{8}+\epsilon }\to \mathfrak {M}$
is the Kirwan blowup of the point
$\left(\phi _1^{-}\right)^{-1}\circ \phi _1^+: \overline {K}_{\frac {1}{8}+\epsilon }\to \mathfrak {M}$
is the Kirwan blowup of the point
 $[4H]$
in the GIT quotient
$[4H]$
in the GIT quotient
 $\mathfrak {M}$
. Across this wall, we replace the quadruple
$\mathfrak {M}$
. Across this wall, we replace the quadruple
 $(1,1)$
curve
$(1,1)$
curve
 $4H$
on
$4H$
on
 $\mathbb {P}^1 \times \mathbb {P}^1$
with GIT polystable degree
$\mathbb {P}^1 \times \mathbb {P}^1$
with GIT polystable degree
 $8$
curves on
$8$
curves on
 $\mathbb {P}(1,1,2)$
which do not pass through the singular point
$\mathbb {P}(1,1,2)$
which do not pass through the singular point
 $[0,0,1]$
. This behavior is similar to [Reference Ascher, DeVleming and Liu6, Theorem 1.3].
$[0,0,1]$
. This behavior is similar to [Reference Ascher, DeVleming and Liu6, Theorem 1.3].
-
(2) From Remarks 5.13 and 5.14, we will see that
 $c_2=\frac {1}{5}$
is the second K-moduli wall. Moreover, if a degree
$c_2=\frac {1}{5}$
is the second K-moduli wall. Moreover, if a degree
 $8$
curve D passes through the singular point of
$8$
curve D passes through the singular point of
 $X =\mathbb {P}(1,1,2)$
, then we see that for any
$X =\mathbb {P}(1,1,2)$
, then we see that for any
 $c < \frac {1}{5}$
the pair
$c < \frac {1}{5}$
the pair
 $(X, cD)$
is K-unstable.
$(X, cD)$
is K-unstable.
5.2 Computations on CM line bundles
The main goals of this section are to compute the CM line bundle of the log Fano family from §3.3 and to show that over the complete intersection locus U, the CM
![]() $\mathbb {Q}$
-line bundle is proportional to the VGIT line bundle.
$\mathbb {Q}$
-line bundle is proportional to the VGIT line bundle.
Proposition 5.5. With the notation from §3.3, we have
 $$ \begin{align*} -f_*\left(\left(-K_{\mathscr{X}/\mathbb{P}(E)} - c\mathscr{D}\right)^3\right)= (2-4c)^2(4c+4)\left(\eta+\frac{3c}{2c+2}\xi\right). \end{align*} $$
$$ \begin{align*} -f_*\left(\left(-K_{\mathscr{X}/\mathbb{P}(E)} - c\mathscr{D}\right)^3\right)= (2-4c)^2(4c+4)\left(\eta+\frac{3c}{2c+2}\xi\right). \end{align*} $$
Proof. By construction, we have
 $$ \begin{align*} \mathcal{O}_{\mathbb{P}^3 \times \mathbb{P}(E)}(\mathscr{X}) &= p_1^*\mathcal{O}_{\mathbb{P}^3}(2) \otimes p_2^* \pi^* \mathcal{O}_{\mathbb{P}^9}(1) \\ \mathcal{O}_{\mathscr{X}}(\mathscr{D}) &= p_1^* \mathcal{O}_{\mathbb{P}^3}(4)\rvert_{\mathscr{X}} \otimes p_2^*\mathcal{O}_{\mathbb{P}(E)}(1) \rvert_{\mathscr{X}}. \end{align*} $$
$$ \begin{align*} \mathcal{O}_{\mathbb{P}^3 \times \mathbb{P}(E)}(\mathscr{X}) &= p_1^*\mathcal{O}_{\mathbb{P}^3}(2) \otimes p_2^* \pi^* \mathcal{O}_{\mathbb{P}^9}(1) \\ \mathcal{O}_{\mathscr{X}}(\mathscr{D}) &= p_1^* \mathcal{O}_{\mathbb{P}^3}(4)\rvert_{\mathscr{X}} \otimes p_2^*\mathcal{O}_{\mathbb{P}(E)}(1) \rvert_{\mathscr{X}}. \end{align*} $$
First note that
![]() $K_{\mathscr {X}/\mathbb {P}(E)} = K_{\mathscr {X}} - f^*K_{\mathbb {P}(E)}$
, and by adjunction,
$K_{\mathscr {X}/\mathbb {P}(E)} = K_{\mathscr {X}} - f^*K_{\mathbb {P}(E)}$
, and by adjunction,
 $$ \begin{align*} K_{\mathscr{X}} &= \left(K_{\mathbb{P}^3 \times \mathbb{P}(E)} + \mathscr{X}\right)\big\rvert_{\mathscr{X}} \\ & = \left(p_1^*\mathcal{O}(-4) \otimes p_2^*\mathcal{O}\left(K_{\mathbb{P}(E)}\right) \otimes p_1^*\mathcal{O}(2) \otimes p_2^*\pi^*\mathcal{O}_{\mathbb{P}^9}(1)\right)\big\rvert_{\mathscr{X}} \\ &= \mathcal{O}_{\mathscr{X}}(-2) \otimes p_2^*\mathcal{O}\left(K_{\mathbb{P}(E)}\right)\rvert_{\mathscr{X}} \otimes p_2^*\pi^*\mathcal{O}_{\mathbb{P}^9}(1)\rvert_{\mathscr{X}}. \end{align*} $$
$$ \begin{align*} K_{\mathscr{X}} &= \left(K_{\mathbb{P}^3 \times \mathbb{P}(E)} + \mathscr{X}\right)\big\rvert_{\mathscr{X}} \\ & = \left(p_1^*\mathcal{O}(-4) \otimes p_2^*\mathcal{O}\left(K_{\mathbb{P}(E)}\right) \otimes p_1^*\mathcal{O}(2) \otimes p_2^*\pi^*\mathcal{O}_{\mathbb{P}^9}(1)\right)\big\rvert_{\mathscr{X}} \\ &= \mathcal{O}_{\mathscr{X}}(-2) \otimes p_2^*\mathcal{O}\left(K_{\mathbb{P}(E)}\right)\rvert_{\mathscr{X}} \otimes p_2^*\pi^*\mathcal{O}_{\mathbb{P}^9}(1)\rvert_{\mathscr{X}}. \end{align*} $$
So in particular we have
Since
![]() $\mathscr {D} = \mathcal {O}_{\mathscr {X}}(4) \otimes p_2^*\mathcal {O}_{\mathbb {P}(E)}(1) \rvert _{\mathscr {X}}$
, we see that
$\mathscr {D} = \mathcal {O}_{\mathscr {X}}(4) \otimes p_2^*\mathcal {O}_{\mathbb {P}(E)}(1) \rvert _{\mathscr {X}}$
, we see that
 $$ \begin{align*} \mathcal{O}_{\mathscr{X}}\left(-K_{\mathscr{X}/\mathbb{P}(E)} - c\mathscr{D}\right) = \mathcal{O}_{\mathscr{X}}(2-4c) \otimes f^* \pi^*\mathcal{O}_{\mathbb{P}^9}(-1) \otimes f^* \mathcal{O}_{\mathbb{P}(E)}(-c).\end{align*} $$
$$ \begin{align*} \mathcal{O}_{\mathscr{X}}\left(-K_{\mathscr{X}/\mathbb{P}(E)} - c\mathscr{D}\right) = \mathcal{O}_{\mathscr{X}}(2-4c) \otimes f^* \pi^*\mathcal{O}_{\mathbb{P}^9}(-1) \otimes f^* \mathcal{O}_{\mathbb{P}(E)}(-c).\end{align*} $$
Let
![]() $H_Y$
denote an element of the class
$H_Y$
denote an element of the class
![]() $\mathcal {O}_Y(1)$
for
$\mathcal {O}_Y(1)$
for
![]() $Y = \mathscr {X}, \mathbb {P}^3, \mathbb {P}(E),$
or
$Y = \mathscr {X}, \mathbb {P}^3, \mathbb {P}(E),$
or
![]() $\mathbb {P}^9$
. We compute
$\mathbb {P}^9$
. We compute
 $$ \begin{align*} &-f_*\left(-K_{\mathscr{X}/\mathbb{P}(E)} -c\mathscr{D}\right)^3\\ &= -f_*\left(((2-4c)H_{\mathscr{X}})^3 - 3((2-4c)H_{\mathscr{X}})^2\cdot\left(cf^*H_{\mathbb{P}(E)}+f^*\pi^*H_{\mathbb{P}^9}\right)\right. + \\ & \quad \left. 3\left((2-4c)H_{\mathscr{X}} \cdot \left(cf^*H_{\mathbb{P}(E)}+f^*\pi^*H_{\mathbb{P}^9}\right)^2 \right) - \left(cf^*H_{\mathbb{P}(E)}+f^*\pi^*H_{\mathbb{P}^9}\right) ^3\right) \\ &= -f_*\left((2-4c)^3(\mathscr{X}\rvert_{\mathscr{X}}) - 3(2-4c)^2H_{\mathbb{P}}^3 \cdot (\mathscr{X}\rvert_{\mathscr{X}})\cdot \left(cf^*H_{\mathbb{P}(E)}+f^*\pi^*H_{\mathbb{P}^9}\right)\right)\\ &= -(2-4c)^3 \pi^*H_{\mathbb{P}^9} + 6(2-4c)^2\left(cH_{\mathbb{P}(E)} + \pi^*H_{\mathbb{P}^9}\right). \end{align*} $$
$$ \begin{align*} &-f_*\left(-K_{\mathscr{X}/\mathbb{P}(E)} -c\mathscr{D}\right)^3\\ &= -f_*\left(((2-4c)H_{\mathscr{X}})^3 - 3((2-4c)H_{\mathscr{X}})^2\cdot\left(cf^*H_{\mathbb{P}(E)}+f^*\pi^*H_{\mathbb{P}^9}\right)\right. + \\ & \quad \left. 3\left((2-4c)H_{\mathscr{X}} \cdot \left(cf^*H_{\mathbb{P}(E)}+f^*\pi^*H_{\mathbb{P}^9}\right)^2 \right) - \left(cf^*H_{\mathbb{P}(E)}+f^*\pi^*H_{\mathbb{P}^9}\right) ^3\right) \\ &= -f_*\left((2-4c)^3(\mathscr{X}\rvert_{\mathscr{X}}) - 3(2-4c)^2H_{\mathbb{P}}^3 \cdot (\mathscr{X}\rvert_{\mathscr{X}})\cdot \left(cf^*H_{\mathbb{P}(E)}+f^*\pi^*H_{\mathbb{P}^9}\right)\right)\\ &= -(2-4c)^3 \pi^*H_{\mathbb{P}^9} + 6(2-4c)^2\left(cH_{\mathbb{P}(E)} + \pi^*H_{\mathbb{P}^9}\right). \end{align*} $$
Thus the proof is finished, since
![]() $\eta = \pi ^*H_{\mathbb {P}^9}$
and
$\eta = \pi ^*H_{\mathbb {P}^9}$
and
![]() $\xi = H_Z$
.
$\xi = H_Z$
.
Proposition 5.6. Let
![]() $f_U:(\mathscr {X}_U,\mathscr {D}_U)\to U$
be the restriction of
$f_U:(\mathscr {X}_U,\mathscr {D}_U)\to U$
be the restriction of
![]() $f:(\mathscr {X},\mathscr {D})\to \mathbb {P}(E)$
over
$f:(\mathscr {X},\mathscr {D})\to \mathbb {P}(E)$
over
![]() $U\subset \mathbb {P}(E)$
. We denote the CM
$U\subset \mathbb {P}(E)$
. We denote the CM
![]() $\mathbb {Q}$
-line bundle of
$\mathbb {Q}$
-line bundle of
![]() $f_U$
with coefficient c by
$f_U$
with coefficient c by
![]() ${\lambda _{U,c}:=\lambda _{\mathrm {CM}, f_U, c\mathscr {D}_U}}$
. Denote by
${\lambda _{U,c}:=\lambda _{\mathrm {CM}, f_U, c\mathscr {D}_U}}$
. Denote by
![]() $\eta _U$
and
$\eta _U$
and
![]() $\xi _U$
the restriction of
$\xi _U$
the restriction of
![]() $\eta $
and
$\eta $
and
![]() $\xi $
to U. Then for any
$\xi $
to U. Then for any
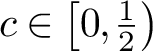 $c\in \left[0,\frac {1}{2}\right)$
, we have
$c\in \left[0,\frac {1}{2}\right)$
, we have
 $$ \begin{align} \lambda_{U,c}=(2-4c)^2(4c+4)\left(\eta_U+\frac{3c}{2c+2}\xi_U\right). \end{align} $$
$$ \begin{align} \lambda_{U,c}=(2-4c)^2(4c+4)\left(\eta_U+\frac{3c}{2c+2}\xi_U\right). \end{align} $$
Proof. We take
![]() $l\in \mathbb {Z}_{>0}$
sufficiently divisible such that
$l\in \mathbb {Z}_{>0}$
sufficiently divisible such that
 $\mathscr {L}:=-l\left(K_{\mathscr {X}/\mathbb {P}(E)}+c\mathscr {D}\right)$
is a Cartier divisor on
$\mathscr {L}:=-l\left(K_{\mathscr {X}/\mathbb {P}(E)}+c\mathscr {D}\right)$
is a Cartier divisor on
![]() $\mathscr {X}$
. From the foregoing computation, we see that
$\mathscr {X}$
. From the foregoing computation, we see that
![]() $\mathscr {L}\sim _{f}\mathcal {O}_X(l(2-4c))$
, which implies that
$\mathscr {L}\sim _{f}\mathcal {O}_X(l(2-4c))$
, which implies that
![]() $\mathscr {L}$
is f-ample. Denote
$\mathscr {L}$
is f-ample. Denote
![]() $\mathscr {L}_{U}:=\mathscr {L}\rvert _{\mathscr {X}_U}$
. Since both
$\mathscr {L}_{U}:=\mathscr {L}\rvert _{\mathscr {X}_U}$
. Since both
![]() $\mathscr {X}$
and
$\mathscr {X}$
and
![]() $\mathbb {P}(E)$
are smooth projective varieties, for
$\mathbb {P}(E)$
are smooth projective varieties, for
![]() $q\gg 1$
and using the Grothendieck–Riemann–Roch theorem we have
$q\gg 1$
and using the Grothendieck–Riemann–Roch theorem we have
 $$ \begin{align*} \mathrm{c}_1\left(f_*\left(\mathscr{L}^{\otimes q}\right)\right)& =\frac{q^{3}}{6}f_*\left(\mathscr{L}^3\right)-\frac{q^2}{4}f_*\left(K_{\mathscr{X}/\mathbb{P}(E)}\cdot\mathscr{L}^2\right)+O(q),\\ \mathrm{c}_1\left(f_*\left(\mathscr{L}^{\otimes q}\otimes\mathcal{O}_{\mathscr{X}}(-\mathscr{D})\right)\right)& =\frac{q^{3}}{6}f_*\left(\mathscr{L}^3\right)-\frac{q^2}{2}f_*\left(\mathscr{D}\cdot\mathscr{L}^2\right)-\frac{q^2}{4}f_*\left(K_{\mathscr{X}/\mathbb{P}(E)}\cdot\mathscr{L}^2\right)+O(q). \end{align*} $$
$$ \begin{align*} \mathrm{c}_1\left(f_*\left(\mathscr{L}^{\otimes q}\right)\right)& =\frac{q^{3}}{6}f_*\left(\mathscr{L}^3\right)-\frac{q^2}{4}f_*\left(K_{\mathscr{X}/\mathbb{P}(E)}\cdot\mathscr{L}^2\right)+O(q),\\ \mathrm{c}_1\left(f_*\left(\mathscr{L}^{\otimes q}\otimes\mathcal{O}_{\mathscr{X}}(-\mathscr{D})\right)\right)& =\frac{q^{3}}{6}f_*\left(\mathscr{L}^3\right)-\frac{q^2}{2}f_*\left(\mathscr{D}\cdot\mathscr{L}^2\right)-\frac{q^2}{4}f_*\left(K_{\mathscr{X}/\mathbb{P}(E)}\cdot\mathscr{L}^2\right)+O(q). \end{align*} $$
Thus
 $\mathrm {c}_1\left((f\rvert _{\mathscr {D}})_*\left(\mathscr {L}\rvert _{\mathscr {D}}^{\otimes q}\right)\right)=\frac {q^2}{2}f_*\left(\mathscr {D}\cdot \mathscr {L}^2\right)+O(q)$
. Since CM line bundles are functorial, by similar arguments to [Reference Ascher, DeVleming and Liu6, Proposition 2.23] we have
$\mathrm {c}_1\left((f\rvert _{\mathscr {D}})_*\left(\mathscr {L}\rvert _{\mathscr {D}}^{\otimes q}\right)\right)=\frac {q^2}{2}f_*\left(\mathscr {D}\cdot \mathscr {L}^2\right)+O(q)$
. Since CM line bundles are functorial, by similar arguments to [Reference Ascher, DeVleming and Liu6, Proposition 2.23] we have
 $$ \begin{align*} \mathrm{c}_1\left(\lambda_{\mathrm{CM}, f_U, c\mathscr{D}_U, \mathscr{L}_U}\right)= -l^2 f_*\left(\left(-K_{\mathscr{X}/\mathbb{P}(E)}-c\mathscr{D}\right)^3\right)\Big\rvert_U. \end{align*} $$
$$ \begin{align*} \mathrm{c}_1\left(\lambda_{\mathrm{CM}, f_U, c\mathscr{D}_U, \mathscr{L}_U}\right)= -l^2 f_*\left(\left(-K_{\mathscr{X}/\mathbb{P}(E)}-c\mathscr{D}\right)^3\right)\Big\rvert_U. \end{align*} $$
Proposition 5.7. The CM
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
![]() $\lambda _{U,c}$
and the VGIT polarization
$\lambda _{U,c}$
and the VGIT polarization
![]() $N_t$
are proportional up to a positive constant when restricted to U where
$N_t$
are proportional up to a positive constant when restricted to U where
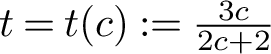 $t=t(c):=\frac {3c}{2c+2}$
.
$t=t(c):=\frac {3c}{2c+2}$
.
Proof. By Proposition 5.6, we see that
![]() $\lambda _{U,c}$
is a positive multiple of
$\lambda _{U,c}$
is a positive multiple of
 $\eta _U + \frac {3c}{2c+2}\xi _U$
. By Proposition 3.4,
$\eta _U + \frac {3c}{2c+2}\xi _U$
. By Proposition 3.4,
 $$ \begin{align*} N_t \rvert_U &= \frac{1 - 2t}{1-2\delta} p_1^*(\eta + \delta \xi) \rvert_U + \frac{t - \delta}{2(1-2\delta)} p_2^*L_{\infty}\rvert_U \\ &= \frac{1 - 2t}{1-2\delta} (\eta_U + \delta \xi_U) + \frac{t - \delta}{2(1-2\delta)} (4 \eta_U + 2 \xi_U ) \\ &= \eta_U + t \xi_U. \end{align*} $$
$$ \begin{align*} N_t \rvert_U &= \frac{1 - 2t}{1-2\delta} p_1^*(\eta + \delta \xi) \rvert_U + \frac{t - \delta}{2(1-2\delta)} p_2^*L_{\infty}\rvert_U \\ &= \frac{1 - 2t}{1-2\delta} (\eta_U + \delta \xi_U) + \frac{t - \delta}{2(1-2\delta)} (4 \eta_U + 2 \xi_U ) \\ &= \eta_U + t \xi_U. \end{align*} $$
Hence for
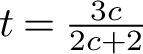 $t = \frac {3c}{2c+2}$
, we see that
$t = \frac {3c}{2c+2}$
, we see that
![]() $\lambda _{U,c}$
is a positive multiple of
$\lambda _{U,c}$
is a positive multiple of
![]() $N_t \rvert _U$
.
$N_t \rvert _U$
.
5.3 K-moduli wall crossings and VGIT
In this section we will prove Theorem 1.1(2) by an inductive argument on walls.
Theorem 5.8 =Theorem 1.1(2)
Let
 $c \in (0, \frac {1}{2})$
be a rational number. Then there is an isomorphism between Artin stacks
$c \in (0, \frac {1}{2})$
be a rational number. Then there is an isomorphism between Artin stacks
![]() $\overline {\mathcal {K}}_c\cong \mathscr {M}(t(c))$
with
$\overline {\mathcal {K}}_c\cong \mathscr {M}(t(c))$
with
 $t(c)=\frac {3c}{2c+2}$
. Moreover, such isomorphisms commute with wall-crossing morphisms.
$t(c)=\frac {3c}{2c+2}$
. Moreover, such isomorphisms commute with wall-crossing morphisms.
We first set up some notation. Recall that the open subset
![]() $U\subset \mathbb {P}(E)$
is defined to be the locus parametrizing
$U\subset \mathbb {P}(E)$
is defined to be the locus parametrizing
![]() $(X, D)$
, where X is a quadric surface in
$(X, D)$
, where X is a quadric surface in
![]() $\mathbb {P}^3$
and D is the complete intersection of X with some quartic surface in
$\mathbb {P}^3$
and D is the complete intersection of X with some quartic surface in
![]() $\mathbb {P}^3$
. Let
$\mathbb {P}^3$
. Let
![]() $U_c^{\mathrm {K}}$
denote the open subset of U parametrizing c-K-semistable log Fano pairs. Let
$U_c^{\mathrm {K}}$
denote the open subset of U parametrizing c-K-semistable log Fano pairs. Let
![]() $U_c^{\mathrm {GIT}}:=\mathscr {P}^{\textrm {ss}}(N_{t})$
denote the VGIT semistable locus in
$U_c^{\mathrm {GIT}}:=\mathscr {P}^{\textrm {ss}}(N_{t})$
denote the VGIT semistable locus in
![]() $\mathscr {P}$
with slope
$\mathscr {P}$
with slope
 $t=t(c)=\frac {3c}{2c+2}$
, which is also contained in U by Lemma 3.5. We say a point
$t=t(c)=\frac {3c}{2c+2}$
, which is also contained in U by Lemma 3.5. We say a point
![]() $[(X,D)]\in U$
is c-GIT-(poly/semi)stable if it is GIT-(poly/semi)stable in
$[(X,D)]\in U$
is c-GIT-(poly/semi)stable if it is GIT-(poly/semi)stable in
![]() $\mathscr {P}$
with slope
$\mathscr {P}$
with slope
![]() $t(c)$
. By Theorem 4.4, we know that there are finitely many walls in
$t(c)$
. By Theorem 4.4, we know that there are finitely many walls in
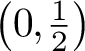 $\left(0,\frac {1}{2}\right)$
for K-moduli stacks
$\left(0,\frac {1}{2}\right)$
for K-moduli stacks
![]() $\overline {\mathcal {K}}_c$
. Denote the sequence of VGIT walls and K-moduli walls by
$\overline {\mathcal {K}}_c$
. Denote the sequence of VGIT walls and K-moduli walls by
 $$ \begin{align*} 0=w_0<w_1<w_2<\dotsb<w_{\ell}=\frac{1}{2}. \end{align*} $$
$$ \begin{align*} 0=w_0<w_1<w_2<\dotsb<w_{\ell}=\frac{1}{2}. \end{align*} $$
That is, either
![]() $c=w_i$
is a wall for K-moduli stacks
$c=w_i$
is a wall for K-moduli stacks
![]() $\overline {\mathcal {K}}_c$
or
$\overline {\mathcal {K}}_c$
or
![]() $t=t(w_i)$
is a wall for VGIT moduli stacks
$t=t(w_i)$
is a wall for VGIT moduli stacks
![]() $\mathscr {M}(t)$
.
$\mathscr {M}(t)$
.
The following proposition allows us to replace K-moduli stacks
![]() $\overline {\mathcal {K}}_c$
by a quotient stack of
$\overline {\mathcal {K}}_c$
by a quotient stack of
![]() $U_c^{\mathrm {K}}$
. An essential ingredient is Theorem 4.8.
$U_c^{\mathrm {K}}$
. An essential ingredient is Theorem 4.8.
Proposition 5.9. There is an isomorphism of stacks
 $\left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]\xrightarrow {\cong } \overline {\mathcal {K}}_c$
. Moreover, we have open immersions
$\left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]\xrightarrow {\cong } \overline {\mathcal {K}}_c$
. Moreover, we have open immersions
![]() $ U_{c-\epsilon }^{\mathrm {K}}\hookrightarrow U_{c}^{\mathrm {K}}\hookleftarrow U_{c+\epsilon }^{\mathrm {K}}$
which descend (via these isomorphisms) to wall-crossing morphisms
$ U_{c-\epsilon }^{\mathrm {K}}\hookrightarrow U_{c}^{\mathrm {K}}\hookleftarrow U_{c+\epsilon }^{\mathrm {K}}$
which descend (via these isomorphisms) to wall-crossing morphisms
![]() $\overline {\mathcal {K}}_{c-\epsilon }\hookrightarrow \overline {\mathcal {K}}_{c}\hookleftarrow \overline {\mathcal {K}}_{c+\epsilon }$
.
$\overline {\mathcal {K}}_{c-\epsilon }\hookrightarrow \overline {\mathcal {K}}_{c}\hookleftarrow \overline {\mathcal {K}}_{c+\epsilon }$
.
Proof. Since
![]() $U_c^{\mathrm {K}}$
parametrizes c-K-semistable log Fano pairs, by the universality of K-moduli stacks we know that there exists a morphism
$U_c^{\mathrm {K}}$
parametrizes c-K-semistable log Fano pairs, by the universality of K-moduli stacks we know that there exists a morphism
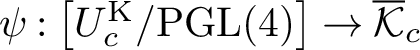 $\psi : \left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]\to \overline {\mathcal {K}}_c$
. In order to show that
$\psi : \left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]\to \overline {\mathcal {K}}_c$
. In order to show that
![]() $\psi $
is an isomorphism, we will construct the inverse morphism
$\psi $
is an isomorphism, we will construct the inverse morphism
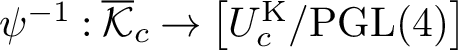 $\psi ^{-1}:\overline {\mathcal {K}}_c\to \left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]$
. We follow notation from Theorem 2.21. Let
$\psi ^{-1}:\overline {\mathcal {K}}_c\to \left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]$
. We follow notation from Theorem 2.21. Let
![]() $T\subset Z_{c}^{\mathrm {red}}$
be the connected component where a general point parametrizes
$T\subset Z_{c}^{\mathrm {red}}$
be the connected component where a general point parametrizes
![]() $\mathbb {P}^1\times \mathbb {P}^1$
. By Definition 4.3 we know that
$\mathbb {P}^1\times \mathbb {P}^1$
. By Definition 4.3 we know that
![]() $\overline {\mathcal {K}}_c\cong \left[T/\mathrm {PGL}(N_m+1)\right]$
. Let
$\overline {\mathcal {K}}_c\cong \left[T/\mathrm {PGL}(N_m+1)\right]$
. Let
 $T'=\mathrm {pr}_1(T)\subset \mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)$
. By Theorems 2.21 and 4.8 we know that
$T'=\mathrm {pr}_1(T)\subset \mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)$
. By Theorems 2.21 and 4.8 we know that
![]() $T'$
is smooth and contains a (possibly empty) smooth divisor
$T'$
is smooth and contains a (possibly empty) smooth divisor
![]() $H'$
parametrizing
$H'$
parametrizing
![]() $\mathbb {P}(1,1,2)$
. Moreover, both
$\mathbb {P}(1,1,2)$
. Moreover, both
![]() $T'\setminus H'$
and
$T'\setminus H'$
and
![]() $H'$
are
$H'$
are
![]() $\mathrm {PGL}(N_m+1)$
-orbits in
$\mathrm {PGL}(N_m+1)$
-orbits in
 $\mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)$
.
$\mathrm {Hilb}_{\chi }\left(\mathbb {P}^{N_m}\right)$
.
In order to construct
![]() $\psi ^{-1}$
, we will first construct a
$\psi ^{-1}$
, we will first construct a
![]() $\mathrm {PGL}(4)$
-torsor
$\mathrm {PGL}(4)$
-torsor
![]() $\mathcal {P}'/T'$
. The argument here is similar to that of [Reference Ascher, DeVleming and Liu6, Proof of Theorem 5.15]. Let
$\mathcal {P}'/T'$
. The argument here is similar to that of [Reference Ascher, DeVleming and Liu6, Proof of Theorem 5.15]. Let
![]() $\pi :(\mathcal X,\mathcal D)\to T$
and
$\pi :(\mathcal X,\mathcal D)\to T$
and
![]() $\pi ':\mathcal X'\to T'$
be the universal families. Since
$\pi ':\mathcal X'\to T'$
be the universal families. Since
![]() $\pi '$
is an isotrivial
$\pi '$
is an isotrivial
![]() $\mathbb {P}^1\times \mathbb {P}^1$
-fibration over
$\mathbb {P}^1\times \mathbb {P}^1$
-fibration over
![]() $T'\setminus H'$
, there exists a flat quasi-finite morphism
$T'\setminus H'$
, there exists a flat quasi-finite morphism
![]() $\widetilde {T}\to T'$
from a smooth variety
$\widetilde {T}\to T'$
from a smooth variety
![]() $\widetilde {T}$
that is étale away from
$\widetilde {T}$
that is étale away from
![]() $H'$
whose image intersects
$H'$
whose image intersects
![]() $H'$
(unless
$H'$
(unless
![]() $H'$
is empty). From the fact that
$H'$
is empty). From the fact that
![]() $T'\setminus H'$
and
$T'\setminus H'$
and
![]() $H'$
are
$H'$
are
![]() $\mathrm {PGL}(N_m+1)$
-orbits, we know that there exists
$\mathrm {PGL}(N_m+1)$
-orbits, we know that there exists
 $T_{i}^{\prime }=g_i\cdot \widetilde {T}$
, where
$T_{i}^{\prime }=g_i\cdot \widetilde {T}$
, where
![]() $g_i\in \mathrm {PGL}(N_m+1)$
, such that
$g_i\in \mathrm {PGL}(N_m+1)$
, such that
![]() $\sqcup _i T_{i}^{\prime }\to T$
is an fppf covering. Moreover, we may assume that
$\sqcup _i T_{i}^{\prime }\to T$
is an fppf covering. Moreover, we may assume that
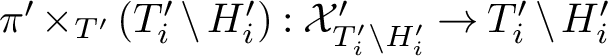 $\pi '\times _{T'} \left(T_{i}^{\prime }\setminus H_{i}^{\prime }\right):\mathcal X^{\prime }_{T_{i}^{\prime }\setminus H_{i}^{\prime }}\to T_{i}^{\prime }\setminus H_{i}^{\prime }$
is a trivial
$\pi '\times _{T'} \left(T_{i}^{\prime }\setminus H_{i}^{\prime }\right):\mathcal X^{\prime }_{T_{i}^{\prime }\setminus H_{i}^{\prime }}\to T_{i}^{\prime }\setminus H_{i}^{\prime }$
is a trivial
![]() $\mathbb {P}^1\times \mathbb {P}^1$
-bundle for each i, where
$\mathbb {P}^1\times \mathbb {P}^1$
-bundle for each i, where
![]() $H_{i}^{\prime }=H'\times _{T'} T_{i}^{\prime }$
. Let
$H_{i}^{\prime }=H'\times _{T'} T_{i}^{\prime }$
. Let
![]() $\mathcal L_{i}^{\prime }$
be the Weil divisorial sheaf on
$\mathcal L_{i}^{\prime }$
be the Weil divisorial sheaf on
 $\mathcal X^{\prime }_{T_{i}^{\prime }}$
as the Zariski closure of
$\mathcal X^{\prime }_{T_{i}^{\prime }}$
as the Zariski closure of
![]() $\mathcal {O}(1,1)$
on
$\mathcal {O}(1,1)$
on
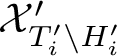 $\mathcal X^{\prime }_{T_{i}^{\prime }\setminus H_{i}^{\prime }}$
. After replacing
$\mathcal X^{\prime }_{T_{i}^{\prime }\setminus H_{i}^{\prime }}$
. After replacing
![]() $T_{i}^{\prime }$
by its Zariski covering, we may assume that
$T_{i}^{\prime }$
by its Zariski covering, we may assume that
 $\mathcal L_i^{\prime [-2]}\cong \omega _{\mathcal X^{\prime }_{T_{i}^{\prime }}/T_{i}^{\prime }}$
. By Kawamata–Viehweg vanishing, we know that
$\mathcal L_i^{\prime [-2]}\cong \omega _{\mathcal X^{\prime }_{T_{i}^{\prime }}/T_{i}^{\prime }}$
. By Kawamata–Viehweg vanishing, we know that
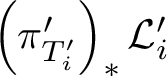 $\left(\pi ^{\prime }_{T_{i}^{\prime }}\right)_*\mathcal L_{i}^{\prime }$
is a rank
$\left(\pi ^{\prime }_{T_{i}^{\prime }}\right)_*\mathcal L_{i}^{\prime }$
is a rank
![]() $4$
vector bundle over
$4$
vector bundle over
![]() $T_{i}^{\prime }$
. Let
$T_{i}^{\prime }$
. Let
![]() $\mathcal {P}_{i}^{\prime }/T_{i}^{\prime }$
be the
$\mathcal {P}_{i}^{\prime }/T_{i}^{\prime }$
be the
![]() $\mathrm {PGL}(4)$
-torsor induced by the projectivized basis of
$\mathrm {PGL}(4)$
-torsor induced by the projectivized basis of
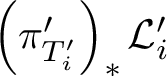 $\left(\pi ^{\prime }_{T_{i}^{\prime }}\right)_*\mathcal L_{i}^{\prime }$
. Since the cocycle condition of
$\left(\pi ^{\prime }_{T_{i}^{\prime }}\right)_*\mathcal L_{i}^{\prime }$
. Since the cocycle condition of
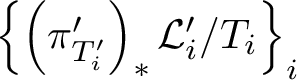 $\left\{\left(\pi ^{\prime }_{T_{i}^{\prime }}\right)_*\mathcal L_{i}^{\prime }/T_i\right\}_i$
is off by
$\left\{\left(\pi ^{\prime }_{T_{i}^{\prime }}\right)_*\mathcal L_{i}^{\prime }/T_i\right\}_i$
is off by
![]() $\pm 1$
, we know that
$\pm 1$
, we know that
![]() $\left\{\mathcal {P}_{i}^{\prime }/T_{i}^{\prime }\right\}$
is an fppf descent datum which descends to a
$\left\{\mathcal {P}_{i}^{\prime }/T_{i}^{\prime }\right\}$
is an fppf descent datum which descends to a
![]() $\mathrm {PGL}(4)$
-torsor
$\mathrm {PGL}(4)$
-torsor
![]() $\mathcal {P}'/T'$
, by [73, Tag 04U1]. It is clear that
$\mathcal {P}'/T'$
, by [73, Tag 04U1]. It is clear that
![]() $\mathcal {P}'/T'$
is
$\mathcal {P}'/T'$
is
![]() $\mathrm {PGL}(N_m+1)$
-equivariant. Denote
$\mathrm {PGL}(N_m+1)$
-equivariant. Denote
![]() $\mathcal {P}:=\mathcal {P}'\times _{T'} T$
. Hence the morphism
$\mathcal {P}:=\mathcal {P}'\times _{T'} T$
. Hence the morphism
![]() $\mathcal {P}\to U_c^{\mathrm {K}}$
given by
$\mathcal {P}\to U_c^{\mathrm {K}}$
given by
![]() $(t,[s_0,s_1,s_2,s_3])\mapsto [s_0,s_1,s_2,s_3](\mathcal X_t,\mathcal D_t)$
induces
$(t,[s_0,s_1,s_2,s_3])\mapsto [s_0,s_1,s_2,s_3](\mathcal X_t,\mathcal D_t)$
induces
 $\psi ^{-1}:\overline {\mathcal {K}}_c\to \left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]$
. The proof is finished.
$\psi ^{-1}:\overline {\mathcal {K}}_c\to \left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]$
. The proof is finished.
In order to prove Theorem 5.8, we run an inductive argument on the walls
![]() $w_i$
. The following proposition is an initial step for the induction:
$w_i$
. The following proposition is an initial step for the induction:
Proposition 5.10. For any
![]() $c\in (0, w_1)$
, we have
$c\in (0, w_1)$
, we have
![]() $U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
.
$U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
.
Proof. Since both
![]() $U_c^{\mathrm {K}}$
and
$U_c^{\mathrm {K}}$
and
![]() $U_c^{\mathrm {GIT}}$
are independent of the choice of
$U_c^{\mathrm {GIT}}$
are independent of the choice of
![]() $c\in (0, w_1)$
, it suffices to show that they are equal for
$c\in (0, w_1)$
, it suffices to show that they are equal for
![]() $0< c\ll 1$
. By Theorem 3.6(2), we know that
$0< c\ll 1$
. By Theorem 3.6(2), we know that
![]() $[(X,D)]\in U_c^{\mathrm {GIT}}$
if and only if
$[(X,D)]\in U_c^{\mathrm {GIT}}$
if and only if
![]() $X\cong \mathbb {P}^1\times \mathbb {P}^1$
and D is a GIT semistable
$X\cong \mathbb {P}^1\times \mathbb {P}^1$
and D is a GIT semistable
![]() $(4,4)$
curve. By Theorem 5.2 and Proposition 5.9, we know that
$(4,4)$
curve. By Theorem 5.2 and Proposition 5.9, we know that
![]() $U_c^{\mathrm {K}}$
consists of exactly the same points as
$U_c^{\mathrm {K}}$
consists of exactly the same points as
![]() $U_c^{\mathrm {GIT}}$
. Hence the proof is finished.
$U_c^{\mathrm {GIT}}$
. Hence the proof is finished.
Next, we divide each induction step into two statements, as Propositions 5.11 and 5.12.
Proposition 5.11. Assume that for any
![]() $c\in (0, w_i)$
we have
$c\in (0, w_i)$
we have
![]() $U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
. Then
$U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
. Then
 $U_{w_i}^{\mathrm {K}}=U_{w_i}^{\mathrm {GIT}}$
.
$U_{w_i}^{\mathrm {K}}=U_{w_i}^{\mathrm {GIT}}$
.
Proof. For simplicity, denote
![]() $w:=w_i$
. We first show that
$w:=w_i$
. We first show that
![]() $U_w^{\mathrm {K}}\subset U_w^{\mathrm {GIT}}$
. Let
$U_w^{\mathrm {K}}\subset U_w^{\mathrm {GIT}}$
. Let
![]() $[(X,D)]$
be a point in
$[(X,D)]$
be a point in
![]() $U_w^{\mathrm {K}}$
. By Proposition 5.9, we know that
$U_w^{\mathrm {K}}$
. By Proposition 5.9, we know that
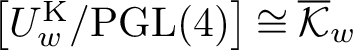 $\left[U_w^{\mathrm {K}}/\mathrm {PGL}(4)\right]\cong \overline {\mathcal {K}}_w$
. By Theorem 4.4, the K-moduli wall-crossing morphism
$\left[U_w^{\mathrm {K}}/\mathrm {PGL}(4)\right]\cong \overline {\mathcal {K}}_w$
. By Theorem 4.4, the K-moduli wall-crossing morphism
![]() $\overline {K}_{w-\epsilon }\to \overline {K}_w$
is surjective, induced by the open immersion
$\overline {K}_{w-\epsilon }\to \overline {K}_w$
is surjective, induced by the open immersion
![]() $U_{w-\epsilon }^{\mathrm {K}}\hookrightarrow U_w^{\mathrm {K}}$
. Hence there exist a w-K-polystable point
$U_{w-\epsilon }^{\mathrm {K}}\hookrightarrow U_w^{\mathrm {K}}$
. Hence there exist a w-K-polystable point
![]() $[(X_0, D_0)]\in U_w^{\mathrm {K}}$
, a
$[(X_0, D_0)]\in U_w^{\mathrm {K}}$
, a
![]() $(w-\epsilon )$
-K-semistable point
$(w-\epsilon )$
-K-semistable point
![]() $[(X',D')]\in U_{w-\epsilon }^{\mathrm {K}}$
, and two
$[(X',D')]\in U_{w-\epsilon }^{\mathrm {K}}$
, and two
![]() $1$
-PSs
$1$
-PSs
![]() $\sigma $
and
$\sigma $
and
![]() $\sigma '$
of
$\sigma '$
of
![]() $\mathrm {SL}(4)$
, such that
$\mathrm {SL}(4)$
, such that
 $$ \begin{align} \lim_{t\to 0 }\sigma(t)\cdot [(X,D)]= [(X_0, D_0)],\qquad \lim_{t\to 0 }\sigma'(t)\cdot [(X',D')]= [(X_0, D_0)]. \end{align} $$
$$ \begin{align} \lim_{t\to 0 }\sigma(t)\cdot [(X,D)]= [(X_0, D_0)],\qquad \lim_{t\to 0 }\sigma'(t)\cdot [(X',D')]= [(X_0, D_0)]. \end{align} $$
In other words,
![]() $(X_0,D_0)$
is the w-K-polystable degeneration of
$(X_0,D_0)$
is the w-K-polystable degeneration of
![]() $(X,D)$
, and the existence of
$(X,D)$
, and the existence of
![]() $(X',D')$
follows from the surjectivity of
$(X',D')$
follows from the surjectivity of
![]() $\overline {K}_{w-\epsilon }\to \overline {K}_w$
. Denote these two special test configurations by
$\overline {K}_{w-\epsilon }\to \overline {K}_w$
. Denote these two special test configurations by
![]() $(\mathcal X, w\mathcal D)$
and
$(\mathcal X, w\mathcal D)$
and
![]() $(\mathcal X',w\mathcal D')$
, respectively. Since
$(\mathcal X',w\mathcal D')$
, respectively. Since
![]() $(X_0, wD_0)$
is K-polystable, we know that
$(X_0, wD_0)$
is K-polystable, we know that
![]() $\mathrm {Fut}(\mathcal X',w\mathcal D')=0$
. Since the generalized Futaki invariant is proportional to the GIT weight of the CM
$\mathrm {Fut}(\mathcal X',w\mathcal D')=0$
. Since the generalized Futaki invariant is proportional to the GIT weight of the CM
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
![]() $\lambda _{U,w}$
, which is again proportional to
$\lambda _{U,w}$
, which is again proportional to
![]() $N_t(w)\rvert _U$
by Proposition 5.7, we have that the GIT weight
$N_t(w)\rvert _U$
by Proposition 5.7, we have that the GIT weight
![]() $\mu ^{N_{t(w)}}([(X',D')], \sigma ')=0$
. By assumption, we have
$\mu ^{N_{t(w)}}([(X',D')], \sigma ')=0$
. By assumption, we have
![]() $[(X',D')]\in U_{w-\epsilon }^{\mathrm {K}}= U_{w-\epsilon }^{\mathrm {GIT}}\subset U_{w}^{\mathrm {GIT}}$
. Hence Lemma 2.4(1) implies that
$[(X',D')]\in U_{w-\epsilon }^{\mathrm {K}}= U_{w-\epsilon }^{\mathrm {GIT}}\subset U_{w}^{\mathrm {GIT}}$
. Hence Lemma 2.4(1) implies that
![]() $[(X_0,D_0)]\in U_{w}^{\mathrm {GIT}}$
, which implies
$[(X_0,D_0)]\in U_{w}^{\mathrm {GIT}}$
, which implies
![]() $[(X,D)]\in U_w^{\mathrm {GIT}}$
by the openness of the GIT semistable locus. Thus we have shown that
$[(X,D)]\in U_w^{\mathrm {GIT}}$
by the openness of the GIT semistable locus. Thus we have shown that
![]() $U_w^{\mathrm {K}}\subset U_w^{\mathrm {GIT}}$
.
$U_w^{\mathrm {K}}\subset U_w^{\mathrm {GIT}}$
.
Next we show the reverse containment
![]() $U_w^{\mathrm {GIT}}\subset U_w^{\mathrm {K}}$
. Let
$U_w^{\mathrm {GIT}}\subset U_w^{\mathrm {K}}$
. Let
![]() $[(X,D)]$
be a point in
$[(X,D)]$
be a point in
![]() $U_w^{\mathrm {GIT}}$
. By almost the same argument as the previous paragraph, except replacing K-stability with GIT stability, we can find
$U_w^{\mathrm {GIT}}$
. By almost the same argument as the previous paragraph, except replacing K-stability with GIT stability, we can find
![]() $[(X_0,D_0)]\in U_w^{\mathrm {GIT}}$
,
$[(X_0,D_0)]\in U_w^{\mathrm {GIT}}$
,
![]() $[(X',D')]\in U_{w-\epsilon }^{\mathrm {GIT}}$
, and two
$[(X',D')]\in U_{w-\epsilon }^{\mathrm {GIT}}$
, and two
![]() $1$
-PSs
$1$
-PSs
![]() $\sigma ,\sigma '$
of
$\sigma ,\sigma '$
of
![]() $\mathrm {SL}(4)$
such that equation (5.2) holds, and
$\mathrm {SL}(4)$
such that equation (5.2) holds, and
Note that the surjectivity of wall-crossing morphisms in VGIT follows from [Reference Laza and O’Grady43] (see Theorem 3.8). By assumption, we have
![]() $[(X',D')]\in U_{w-\epsilon }^{\mathrm {GIT}}=U_{w-\epsilon }^{\mathrm {K}}\subset U_w^{\mathrm {K}}$
. Again using Proposition 5.7, we get
$[(X',D')]\in U_{w-\epsilon }^{\mathrm {GIT}}=U_{w-\epsilon }^{\mathrm {K}}\subset U_w^{\mathrm {K}}$
. Again using Proposition 5.7, we get
![]() $\mathrm {Fut}(\mathcal X',w\mathcal D';\mathcal L)=0$
, where
$\mathrm {Fut}(\mathcal X',w\mathcal D';\mathcal L)=0$
, where
![]() $(\mathcal X',w\mathcal D';\mathcal L)$
is the test configuration of
$(\mathcal X',w\mathcal D';\mathcal L)$
is the test configuration of
![]() $(X',wD',\mathcal {O}_{X'}(1))$
induced by
$(X',wD',\mathcal {O}_{X'}(1))$
induced by
![]() $\sigma '$
. Since
$\sigma '$
. Since
![]() $(X',wD')$
is K-semistable, by [Reference Li and Xu49, Section 8.2] we know that
$(X',wD')$
is K-semistable, by [Reference Li and Xu49, Section 8.2] we know that
![]() $\mathcal X'$
is regular in codimension
$\mathcal X'$
is regular in codimension
![]() $1$
. Since
$1$
. Since
![]() $\mathcal X_0'=X_0$
is Cohen–Macaulay, we know that
$\mathcal X_0'=X_0$
is Cohen–Macaulay, we know that
![]() $\mathcal X'$
is
$\mathcal X'$
is
![]() $S_2$
, which implies that
$S_2$
, which implies that
![]() $\mathcal X'$
is normal. Hence Lemma 2.4(2) implies that
$\mathcal X'$
is normal. Hence Lemma 2.4(2) implies that
![]() $(X_0, wD_0)$
is K-semistable, and so is
$(X_0, wD_0)$
is K-semistable, and so is
![]() $(X,wD)$
by the openness of K-semistability [Reference Blum, Liu and Xu12, Reference Xu15]. The proof is finished.
$(X,wD)$
by the openness of K-semistability [Reference Blum, Liu and Xu12, Reference Xu15]. The proof is finished.
Proposition 5.12. Assume that for any
![]() $c\in (0, w_i]$
we have
$c\in (0, w_i]$
we have
![]() $U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
. Then
$U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
. Then
![]() $U_{c'}^{\mathrm {K}}=U_{c'}^{\mathrm {GIT}}$
for any
$U_{c'}^{\mathrm {K}}=U_{c'}^{\mathrm {GIT}}$
for any
![]() $c'\in (w_i, w_{i+1})$
.
$c'\in (w_i, w_{i+1})$
.
Proof. For simplicity, denote
![]() $w:=w_i$
. Since the K-semistable locus
$w:=w_i$
. Since the K-semistable locus
![]() $U_{c'}^{\mathrm {K}}$
and the GIT semistable locus
$U_{c'}^{\mathrm {K}}$
and the GIT semistable locus
![]() $U_{c'}^{\mathrm {GIT}}$
are independent of the choice of
$U_{c'}^{\mathrm {GIT}}$
are independent of the choice of
![]() $c'\in (w_i,w_{i+1})$
, it suffices to show that
$c'\in (w_i,w_{i+1})$
, it suffices to show that
 $U_{w+\epsilon }^{\mathrm {K}} = U_{w + \epsilon }^{\mathrm {GIT}}$
. We first show
$U_{w+\epsilon }^{\mathrm {K}} = U_{w + \epsilon }^{\mathrm {GIT}}$
. We first show
 $U_{w+\epsilon }^{\mathrm {K}} \subset U_{w + \epsilon }^{\mathrm {GIT}}$
. Assume to the contrary that
$U_{w+\epsilon }^{\mathrm {K}} \subset U_{w + \epsilon }^{\mathrm {GIT}}$
. Assume to the contrary that
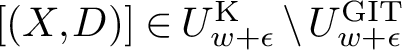 $[(X,D)]\in U_{w+\epsilon }^{\mathrm {K}}\setminus U_{w+\epsilon }^{\mathrm {GIT}}$
. We note that by Proposition 5.9 and Lemma 3.7, there are open immersions
$[(X,D)]\in U_{w+\epsilon }^{\mathrm {K}}\setminus U_{w+\epsilon }^{\mathrm {GIT}}$
. We note that by Proposition 5.9 and Lemma 3.7, there are open immersions
![]() $U_{w+\epsilon }^{\mathrm {K}} \hookrightarrow U_w^{\mathrm {K}}$
and
$U_{w+\epsilon }^{\mathrm {K}} \hookrightarrow U_w^{\mathrm {K}}$
and
 $U_{w+\epsilon }^{\mathrm {GIT}} \hookrightarrow U_w^{\mathrm {GIT}}$
. By assumption, we have
$U_{w+\epsilon }^{\mathrm {GIT}} \hookrightarrow U_w^{\mathrm {GIT}}$
. By assumption, we have
 $[(X,D)]\in U_{w+\epsilon }^{\mathrm {K}}\subset U_w^{\mathrm {K}}=U_w^{\mathrm {GIT}}$
, hence
$[(X,D)]\in U_{w+\epsilon }^{\mathrm {K}}\subset U_w^{\mathrm {K}}=U_w^{\mathrm {GIT}}$
, hence
![]() $[(X,D)]$
is w-GIT semistable but
$[(X,D)]$
is w-GIT semistable but
![]() $(w+\epsilon )$
-GIT unstable. Thus by Lemma 3.7 there exists a 1-PS
$(w+\epsilon )$
-GIT unstable. Thus by Lemma 3.7 there exists a 1-PS
![]() $\sigma : \mathbb {G}_m \to \mathrm {SL}(4)$
such that
$\sigma : \mathbb {G}_m \to \mathrm {SL}(4)$
such that
Denote
![]() $\zeta _0:=\lim _{t\to 0}\sigma (t)\cdot [(X,D)] \in \mathscr {P}$
. Since
$\zeta _0:=\lim _{t\to 0}\sigma (t)\cdot [(X,D)] \in \mathscr {P}$
. Since
![]() $[(X,D)]$
is w-GIT semistable, by Lemma 2.4(1) and equation (5.3) we know that
$[(X,D)]$
is w-GIT semistable, by Lemma 2.4(1) and equation (5.3) we know that
![]() $\zeta _0$
is also w-GIT semistable; in particular,
$\zeta _0$
is also w-GIT semistable; in particular,
![]() $\zeta _0=[(X_0,D_0)]\in U$
. Denote by
$\zeta _0=[(X_0,D_0)]\in U$
. Denote by
![]() $(\mathcal X,w\mathcal D;\mathcal L)/\mathbb {A}^1$
the test configuration of
$(\mathcal X,w\mathcal D;\mathcal L)/\mathbb {A}^1$
the test configuration of
![]() $(X,wD;\mathcal {O}_X(1))$
induced by
$(X,wD;\mathcal {O}_X(1))$
induced by
![]() $\sigma $
. Hence by equation (5.3) and Proposition 5.7, we have
$\sigma $
. Hence by equation (5.3) and Proposition 5.7, we have
![]() $\mathrm {Fut}(\mathcal X,(w+\epsilon )\mathcal D)<0$
. This implies that
$\mathrm {Fut}(\mathcal X,(w+\epsilon )\mathcal D)<0$
. This implies that
![]() $(X,(w+\epsilon )D)$
is K-unstable, which contradicts the assumption that
$(X,(w+\epsilon )D)$
is K-unstable, which contradicts the assumption that
![]() $[(X,D)]\in U_{w+\epsilon }^{\mathrm {K}}$
. Thus we conclude that
$[(X,D)]\in U_{w+\epsilon }^{\mathrm {K}}$
. Thus we conclude that
 $U_{w+\epsilon }^{\mathrm {K}}\subset U_{w+\epsilon }^{\mathrm {GIT}}$
.
$U_{w+\epsilon }^{\mathrm {K}}\subset U_{w+\epsilon }^{\mathrm {GIT}}$
.
Next, if
![]() $[(X,D)] \in U_{w+\epsilon }^{\mathrm {K}}$
is
$[(X,D)] \in U_{w+\epsilon }^{\mathrm {K}}$
is
![]() $(w+\epsilon )$
-K-polystable, then we claim that
$(w+\epsilon )$
-K-polystable, then we claim that
![]() $[(X,D)]$
is
$[(X,D)]$
is
![]() $(w+\epsilon )$
-GIT polystable. We have already shown that
$(w+\epsilon )$
-GIT polystable. We have already shown that
![]() $[(X,D)]$
is
$[(X,D)]$
is
![]() $(w+\epsilon )$
-GIT semistable. Let us take a 1-PS
$(w+\epsilon )$
-GIT semistable. Let us take a 1-PS
![]() $\sigma '$
of
$\sigma '$
of
![]() $\mathrm {SL}(4)$
degenerating
$\mathrm {SL}(4)$
degenerating
![]() $[(X,D)]$
to a
$[(X,D)]$
to a
![]() $(w+\epsilon )$
-GIT polystable point
$(w+\epsilon )$
-GIT polystable point
![]() $[(X',D')]$
. Hence we have
$[(X',D')]$
. Hence we have
![]() $\mu ^{N_{t(w+\epsilon )}}([(X,D)],\sigma ')=0$
. By Proposition 5.7, we have
$\mu ^{N_{t(w+\epsilon )}}([(X,D)],\sigma ')=0$
. By Proposition 5.7, we have
![]() $\mathrm {Fut}(\mathcal X',(w+\epsilon )\mathcal D';\mathcal L')=0$
, where
$\mathrm {Fut}(\mathcal X',(w+\epsilon )\mathcal D';\mathcal L')=0$
, where
![]() $(\mathcal X',(w+\epsilon )\mathcal D';\mathcal L')$
is the test configuration of
$(\mathcal X',(w+\epsilon )\mathcal D';\mathcal L')$
is the test configuration of
![]() $(X,(w+\epsilon )D;\mathcal {O}_X(1))$
induced by
$(X,(w+\epsilon )D;\mathcal {O}_X(1))$
induced by
![]() $\sigma '$
. Since
$\sigma '$
. Since
 $[(X',D')]\in U_{w+\epsilon }^{\mathrm {GIT}}\subset U_w^{\mathrm {GIT}}=U_w^{\mathrm {K}}$
by assumption, we know that
$[(X',D')]\in U_{w+\epsilon }^{\mathrm {GIT}}\subset U_w^{\mathrm {GIT}}=U_w^{\mathrm {K}}$
by assumption, we know that
![]() $(X',wD')$
is K-semistable and hence klt. Thus
$(X',wD')$
is K-semistable and hence klt. Thus
![]() $(\mathcal X',(w+\epsilon )\mathcal D')$
is a special test configuration with vanishing generalized Futaki invariant. Since
$(\mathcal X',(w+\epsilon )\mathcal D')$
is a special test configuration with vanishing generalized Futaki invariant. Since
![]() $(X,(w+\epsilon )D$
is K-polystable, we know that
$(X,(w+\epsilon )D$
is K-polystable, we know that
![]() $(X,D)\cong (X',D')$
, which implies that
$(X,D)\cong (X',D')$
, which implies that
![]() $[(X,D)]$
and
$[(X,D)]$
and
![]() $[(X',D')]$
belong to the same
$[(X',D')]$
belong to the same
![]() $\mathrm {SL}(4)$
-orbit in U. Hence
$\mathrm {SL}(4)$
-orbit in U. Hence
![]() $[(X,D)]$
is
$[(X,D)]$
is
![]() $(w+\epsilon )$
-GIT polystable.
$(w+\epsilon )$
-GIT polystable.
Finally we show that
 $U_{w+\epsilon }^{\mathrm {K}}= U_{w+\epsilon }^{\mathrm {GIT}}$
. Consider the following commutative diagram:
$U_{w+\epsilon }^{\mathrm {K}}= U_{w+\epsilon }^{\mathrm {GIT}}$
. Consider the following commutative diagram:

Since f is an open immersion between smooth varieties, its descent g is separated and representable. By Lemma 5.9 we know that
 $\left[U_{w+\epsilon }^{\mathrm {K}}/\mathrm {PGL}(4)\right]\cong \overline {\mathcal {K}}_{w+\epsilon }$
, and hence g maps closed points to closed points as shown in the previous paragraph and h is quasi-finite. Since the GIT quotients on the third column are isomorphic to the K-moduli space
$\left[U_{w+\epsilon }^{\mathrm {K}}/\mathrm {PGL}(4)\right]\cong \overline {\mathcal {K}}_{w+\epsilon }$
, and hence g maps closed points to closed points as shown in the previous paragraph and h is quasi-finite. Since the GIT quotients on the third column are isomorphic to the K-moduli space
![]() $\overline {K}_{w+\epsilon }$
and the VGIT moduli space
$\overline {K}_{w+\epsilon }$
and the VGIT moduli space
![]() $\mathfrak {M}(t(w+\epsilon ))$
, respectively, they are both proper. Thus h is a finite morphism. Then we apply [Reference Alper3, Proposition 6.4] to conclude that g is a finite morphism as well. In particular, this implies that f is finite, hence surjective. The proof is finished.
$\mathfrak {M}(t(w+\epsilon ))$
, respectively, they are both proper. Thus h is a finite morphism. Then we apply [Reference Alper3, Proposition 6.4] to conclude that g is a finite morphism as well. In particular, this implies that f is finite, hence surjective. The proof is finished.
Proof of Theorem 5.8. By Propositions 5.10, 5.11, and 5.12 on induction of the walls
![]() $\{w_i\}_{i=0}^{\ell }$
, we conclude that
$\{w_i\}_{i=0}^{\ell }$
, we conclude that
![]() $U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
for any
$U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
for any
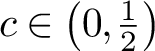 $c\in \left(0,\frac {1}{2}\right)$
. Hence the theorem follows from Proposition 5.9 and the definition
$c\in \left(0,\frac {1}{2}\right)$
. Hence the theorem follows from Proposition 5.9 and the definition
 $\mathscr {M}(t(c))=\left[U_c^{\mathrm {GIT}}/\mathrm {PGL}(4)\right]$
.□
$\mathscr {M}(t(c))=\left[U_c^{\mathrm {GIT}}/\mathrm {PGL}(4)\right]$
.□
Proof of Theorem 1.2. The first isomorphism follows from Theorem 1.1. The second isomorphism follows from Theorem 3.8. For the proportionality statements, the first one between the CM
![]() $\mathbb {Q}$
-line bundle and VGIT polarization follows from Proposition 5.7, and the second one between VGIT polarization and the push-forward of
$\mathbb {Q}$
-line bundle and VGIT polarization follows from Proposition 5.7, and the second one between VGIT polarization and the push-forward of
![]() $\lambda +\beta \Delta $
follows from [Reference Laza and O’Grady43, Proposition 7.6].□
$\lambda +\beta \Delta $
follows from [Reference Laza and O’Grady43, Proposition 7.6].□
Proof of Theorem 1.3. Since there are finitely many K-moduli (resp., GIT) walls for
 $c\in \left(0,\frac {1}{2}\right) \left(\text {resp., }t\in \left(0,\frac {1}{2}\right)\right)$
, we may assume that
$c\in \left(0,\frac {1}{2}\right) \left(\text {resp., }t\in \left(0,\frac {1}{2}\right)\right)$
, we may assume that
![]() $\epsilon $
and
$\epsilon $
and
![]() $\epsilon '$
satisfy the relation
$\epsilon '$
satisfy the relation
 $\epsilon =\frac {3\epsilon '}{2\epsilon '+2}$
– that is,
$\epsilon =\frac {3\epsilon '}{2\epsilon '+2}$
– that is,
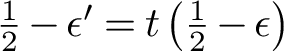 $\frac {1}{2}-\epsilon ' = t\left(\frac {1}{2}-\epsilon \right)$
. By Theorem 1.1, we have
$\frac {1}{2}-\epsilon ' = t\left(\frac {1}{2}-\epsilon \right)$
. By Theorem 1.1, we have
 $\mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)\cong \overline {K}_{\frac {1}{2}-\epsilon }$
. The isomorphism
$\mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)\cong \overline {K}_{\frac {1}{2}-\epsilon }$
. The isomorphism
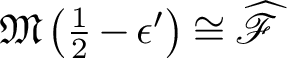 $\mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)\cong \widehat {\mathscr {F}}$
follows from [Reference Laza and O’Grady43, Theorem 1.1].
$\mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)\cong \widehat {\mathscr {F}}$
follows from [Reference Laza and O’Grady43, Theorem 1.1].
For part (1), from these isomorphisms we know that
 $\mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)$
parametrizes K-polystable klt log Fano pairs
$\mathfrak {M}\left(\frac {1}{2}-\epsilon '\right)$
parametrizes K-polystable klt log Fano pairs
 $\left(X,\left(\frac {1}{2}-\epsilon '\right)D\right)$
. By the ACC of log canonical thresholds [Reference Hacon, McKernan and Xu32], we know that
$\left(X,\left(\frac {1}{2}-\epsilon '\right)D\right)$
. By the ACC of log canonical thresholds [Reference Hacon, McKernan and Xu32], we know that
 $\left(X,\frac {1}{2}D\right)$
is log canonical. Hence taking a double cover of X branched along D, we obtain a hyperelliptic K3 surface S with only slc singularities. The proof is finished.
$\left(X,\frac {1}{2}D\right)$
is log canonical. Hence taking a double cover of X branched along D, we obtain a hyperelliptic K3 surface S with only slc singularities. The proof is finished.
For part (2), notice that by taking fiberwise double covers of the universal log Fano family over
 $\overline {\mathcal {K}}_{\frac {1}{2}-\epsilon }$
, we obtain a universal family of slc K3 surfaces
$\overline {\mathcal {K}}_{\frac {1}{2}-\epsilon }$
, we obtain a universal family of slc K3 surfaces
![]() $\mathcal {S}\to \mathcal T$
, where
$\mathcal {S}\to \mathcal T$
, where
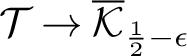 $\mathcal T\to \overline {\mathcal {K}}_{\frac {1}{2}-\epsilon }$
is a
$\mathcal T\to \overline {\mathcal {K}}_{\frac {1}{2}-\epsilon }$
is a
![]() $\boldsymbol {\mu }_2$
-gerbe. In particular, the Hodge line bundle
$\boldsymbol {\mu }_2$
-gerbe. In particular, the Hodge line bundle
![]() $\lambda _{\mathrm {Hodge},\mathcal T}$
of the K3 family
$\lambda _{\mathrm {Hodge},\mathcal T}$
of the K3 family
![]() $\mathcal {S}/\mathcal T$
is the pullback of the Hodge line bundle
$\mathcal {S}/\mathcal T$
is the pullback of the Hodge line bundle
![]() $\lambda _{\mathrm {Hodge}, \frac {1}{2}-\epsilon }$
over
$\lambda _{\mathrm {Hodge}, \frac {1}{2}-\epsilon }$
over
 $\overline {\mathcal {K}}_{\frac {1}{2}-\epsilon }$
. Taking good moduli spaces of
$\overline {\mathcal {K}}_{\frac {1}{2}-\epsilon }$
. Taking good moduli spaces of
![]() $\mathcal T\to T$
and
$\mathcal T\to T$
and
 $\overline {\mathcal {K}}_{\frac {1}{2}-\epsilon }\to \overline {K}_{\frac {1}{2}-\epsilon }$
gives an isomorphism
$\overline {\mathcal {K}}_{\frac {1}{2}-\epsilon }\to \overline {K}_{\frac {1}{2}-\epsilon }$
gives an isomorphism
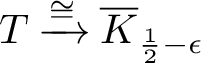 $T\xrightarrow {\cong }\overline {K}_{\frac {1}{2}-\epsilon }$
. Since both spaces are isomorphic to
$T\xrightarrow {\cong }\overline {K}_{\frac {1}{2}-\epsilon }$
. Since both spaces are isomorphic to
![]() $\widehat {\mathscr {F}}$
, we know that
$\widehat {\mathscr {F}}$
, we know that
![]() $\mathscr {F}$
admits an open immersion into T whose complement has codimension at least
$\mathscr {F}$
admits an open immersion into T whose complement has codimension at least
![]() $2$
. In particular, we know that
$2$
. In particular, we know that
![]() $\lambda _{\mathrm {Hodge}, T}\rvert _{\mathscr {F}}=\lambda _{\mathrm {Hodge}, \mathscr {F}}$
, and the conclusion follows from
$\lambda _{\mathrm {Hodge}, T}\rvert _{\mathscr {F}}=\lambda _{\mathrm {Hodge}, \mathscr {F}}$
, and the conclusion follows from
![]() $\mathscr {F}^*=\mathrm {Proj} R\left(\mathscr {F}, \lambda _{\mathrm {Hodge}, \mathscr {F}}\right)$
.□
$\mathscr {F}^*=\mathrm {Proj} R\left(\mathscr {F}, \lambda _{\mathrm {Hodge}, \mathscr {F}}\right)$
.□
Remark 5.13. According to [Reference Laza and O’Grady43], the t-walls for VGIT quotients
![]() $\mathfrak {M}(t)$
and
$\mathfrak {M}(t)$
and
![]() $\beta $
-walls for the Hassett–Keel–Looijenga program for
$\beta $
-walls for the Hassett–Keel–Looijenga program for
![]() $\mathscr {F}(\beta )=\mathrm {Proj} R(\mathscr {F},\lambda +\beta \Delta )$
with
$\mathscr {F}(\beta )=\mathrm {Proj} R(\mathscr {F},\lambda +\beta \Delta )$
with
![]() $N=18$
(under the transformation rule
$N=18$
(under the transformation rule
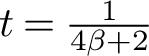 $t=\frac {1}{4\beta +2}$
) are given by
$t=\frac {1}{4\beta +2}$
) are given by
 $$ \begin{align*} t\in \left\{\frac{1}{6}, \frac{1}{4}, \frac{3}{10}, \frac{1}{3}, \frac{5}{14}, \frac{3}{8}, \frac{2}{5}, \frac{1}{2} \right\},\qquad \beta\in \left\{1, \frac{1}{2}, \frac{1}{3}, \frac{1}{4}, \frac{1}{5}, \frac{1}{6}, \frac{1}{8}, 0 \right\}. \end{align*} $$
$$ \begin{align*} t\in \left\{\frac{1}{6}, \frac{1}{4}, \frac{3}{10}, \frac{1}{3}, \frac{5}{14}, \frac{3}{8}, \frac{2}{5}, \frac{1}{2} \right\},\qquad \beta\in \left\{1, \frac{1}{2}, \frac{1}{3}, \frac{1}{4}, \frac{1}{5}, \frac{1}{6}, \frac{1}{8}, 0 \right\}. \end{align*} $$
By the transformation rule
 $t=\frac {3c}{2c+2}$
, we obtain the c-walls for K-moduli stacks
$t=\frac {3c}{2c+2}$
, we obtain the c-walls for K-moduli stacks
![]() $\overline {\mathcal {K}}_c$
:
$\overline {\mathcal {K}}_c$
:
 $$ \begin{align*} c\in \left\{\frac{1}{8}, \frac{1}{5}, \frac{1}{4}, \frac{2}{7}, \frac{5}{16}, \frac{1}{3}, \frac{4}{11}, \frac{1}{2} \right\}. \end{align*} $$
$$ \begin{align*} c\in \left\{\frac{1}{8}, \frac{1}{5}, \frac{1}{4}, \frac{2}{7}, \frac{5}{16}, \frac{1}{3}, \frac{4}{11}, \frac{1}{2} \right\}. \end{align*} $$
Note that
 $c=\frac {1}{2}$
corresponds to the log Calabi–Yau wall crossing
$c=\frac {1}{2}$
corresponds to the log Calabi–Yau wall crossing
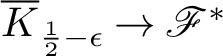 $\overline {K}_{\frac {1}{2}-\epsilon }\to \mathscr {F}^*$
, while the remaining walls are in the log Fano region.
$\overline {K}_{\frac {1}{2}-\epsilon }\to \mathscr {F}^*$
, while the remaining walls are in the log Fano region.
Remark 5.14 Compare [Reference Laza and O’Grady43, Section 6]
Let
![]() $i\in \{1,2,\dotsc , 7\}$
be an index. For the ith K-moduli wall
$i\in \{1,2,\dotsc , 7\}$
be an index. For the ith K-moduli wall
![]() $c_i$
, we have K-moduli wall crossing morphisms
$c_i$
, we have K-moduli wall crossing morphisms
 $$ \begin{align*} \overline{K}_{c_i-\epsilon}\xrightarrow{\phi_i^{-}}\overline{K}_{c_i}\xleftarrow{\phi_i^{+}}\overline{K}_{c_i+\epsilon}. \end{align*} $$
$$ \begin{align*} \overline{K}_{c_i-\epsilon}\xrightarrow{\phi_i^{-}}\overline{K}_{c_i}\xleftarrow{\phi_i^{+}}\overline{K}_{c_i+\epsilon}. \end{align*} $$
Denote by
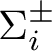 $\Sigma _i^{\pm }$
the closed subset of
$\Sigma _i^{\pm }$
the closed subset of
![]() $\overline {K}_{c_i\pm \epsilon }$
parametrizing pairs that are
$\overline {K}_{c_i\pm \epsilon }$
parametrizing pairs that are
![]() $(c_i\pm \epsilon )$
-K-polystable but not
$(c_i\pm \epsilon )$
-K-polystable but not
![]() $c_i$
-K-polystable. As observed in [Reference Laza and O’Grady43, Section 6], we know that a general point
$c_i$
-K-polystable. As observed in [Reference Laza and O’Grady43, Section 6], we know that a general point
![]() $[(X,D)]$
in
$[(X,D)]$
in
 $\Sigma _i^{-} \left(\text {resp., }\Sigma _i^{+}\right)$
parametrizes a curve D on
$\Sigma _i^{-} \left(\text {resp., }\Sigma _i^{+}\right)$
parametrizes a curve D on
![]() $X\cong \mathbb {P}^1\times \mathbb {P}^1$
(resp.,
$X\cong \mathbb {P}^1\times \mathbb {P}^1$
(resp.,
![]() $X\cong \mathbb {P}(1,1,2)$
). In Table 1, we rephrase results from [Reference Laza and O’Grady43], especially [Reference Laza and O’Grady43, Table 2], to describe the generic singularities (in local analytic form) presented in the curves D. Note that a general curve D in
$X\cong \mathbb {P}(1,1,2)$
). In Table 1, we rephrase results from [Reference Laza and O’Grady43], especially [Reference Laza and O’Grady43, Table 2], to describe the generic singularities (in local analytic form) presented in the curves D. Note that a general curve D in
![]() $\Sigma _i^+$
is smooth when
$\Sigma _i^+$
is smooth when
![]() $i=1$
, and singular only at the cone vertex
$i=1$
, and singular only at the cone vertex
![]() $v=[0,0,1]$
of
$v=[0,0,1]$
of
![]() $\mathbb {P}(1,1,2)$
when
$\mathbb {P}(1,1,2)$
when
![]() $2\leq i\leq 7$
.
$2\leq i\leq 7$
.
Table 1 Singularities along the K-moduli walls

6 Some results for
 $(d,d)$
curves
$(d,d)$
curves
In this section we discuss some generalizations of our results to
![]() $(d,d)$
-curves on
$(d,d)$
-curves on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
, including the proof of Theorem 1.4. We assume
$\mathbb {P}^1\times \mathbb {P}^1$
, including the proof of Theorem 1.4. We assume
![]() $d\geq 3$
throughout this section.
$d\geq 3$
throughout this section.
6.1 VGIT for
 $(2,d)$
complete intersections in
$(2,d)$
complete intersections in
 $\mathbb {P}^3$
$\mathbb {P}^3$
Define
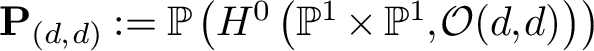 $\mathbf {P}_{\left(d,d\right)}:=\mathbb {P}\left(H^0\left(\mathbb {P}^1\times \mathbb {P}^1,\mathcal {O}(d,d)\right)\right)$
. We say a
$\mathbf {P}_{\left(d,d\right)}:=\mathbb {P}\left(H^0\left(\mathbb {P}^1\times \mathbb {P}^1,\mathcal {O}(d,d)\right)\right)$
. We say a
![]() $(d,d)$
-curve C on
$(d,d)$
-curve C on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
is GIT-(poly/semi)stable if
$\mathbb {P}^1\times \mathbb {P}^1$
is GIT-(poly/semi)stable if
![]() $[C]$
is GIT-(poly/semi)stable with respect to the natural
$[C]$
is GIT-(poly/semi)stable with respect to the natural
 $\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
-action on
$\mathrm {Aut}\left(\mathbb {P}^1\times \mathbb {P}^1\right)$
-action on
 $\left(\mathbf {P}_{\left(d,d\right)},\mathcal {O}(2)\right)$
. We define the GIT moduli stack
$\left(\mathbf {P}_{\left(d,d\right)},\mathcal {O}(2)\right)$
. We define the GIT moduli stack
![]() $\mathscr {M}_d$
and the GIT moduli space
$\mathscr {M}_d$
and the GIT moduli space
![]() $\mathfrak {M}_d$
of degree
$\mathfrak {M}_d$
of degree
![]() $(d,d)$
curves as
$(d,d)$
curves as
 $$ \begin{align*} \mathscr{M}_d:= \left[\mathbf{P}_{\left(d,d\right)}^{\textrm{ss}}/\mathrm{Aut}\left(\mathbb{P}^1\times\mathbb{P}^1\right)\right],\qquad \mathfrak{M}_d:=\mathbf{P}_{(d,d)}^{\textrm{ss}}\mathbin{/\mkern-6mu/}\mathrm{Aut}\left(\mathbb{P}^1\times\mathbb{P}^1\right). \end{align*} $$
$$ \begin{align*} \mathscr{M}_d:= \left[\mathbf{P}_{\left(d,d\right)}^{\textrm{ss}}/\mathrm{Aut}\left(\mathbb{P}^1\times\mathbb{P}^1\right)\right],\qquad \mathfrak{M}_d:=\mathbf{P}_{(d,d)}^{\textrm{ss}}\mathbin{/\mkern-6mu/}\mathrm{Aut}\left(\mathbb{P}^1\times\mathbb{P}^1\right). \end{align*} $$
Next we describe the VGIT of
![]() $(2,d)$
complete intersection curves in
$(2,d)$
complete intersection curves in
![]() $\mathbb {P}^3$
based on [Reference Benoist8, Reference Casalaina-Martin, Jensen and Laza16, Reference Laza and O’Grady43]. Our setup is a direct generalization of §3.3. Let
$\mathbb {P}^3$
based on [Reference Benoist8, Reference Casalaina-Martin, Jensen and Laza16, Reference Laza and O’Grady43]. Our setup is a direct generalization of §3.3. Let
 $$ \begin{align*} \pi:\mathbb{P}(E_d)\to \mathbb{P}\left(H^0\left(\mathbb{P}^3,\mathcal{O}(2)\right)\right)=\mathbb{P}^9 \end{align*} $$
$$ \begin{align*} \pi:\mathbb{P}(E_d)\to \mathbb{P}\left(H^0\left(\mathbb{P}^3,\mathcal{O}(2)\right)\right)=\mathbb{P}^9 \end{align*} $$
be the projective space bundle with fiber
 $\mathbb {P}\left(H^0\left(Q,\mathcal {O}_Q(d)\right)\right)$
over a quadric surface
$\mathbb {P}\left(H^0\left(Q,\mathcal {O}_Q(d)\right)\right)$
over a quadric surface
![]() $[Q]\in \mathbb {P}^9$
. Set
$[Q]\in \mathbb {P}^9$
. Set
![]() $f: (\mathscr {X},\mathscr {D})\to \mathbb {P}(E_d)$
the universal family of quadric surfaces with
$f: (\mathscr {X},\mathscr {D})\to \mathbb {P}(E_d)$
the universal family of quadric surfaces with
![]() $(2,d)$
intersections over
$(2,d)$
intersections over
![]() $\mathbb {P}(E_d)$
. Denote
$\mathbb {P}(E_d)$
. Denote
![]() $\eta :=\pi ^*\mathcal {O}_{\mathbb {P}^9}(1)$
and
$\eta :=\pi ^*\mathcal {O}_{\mathbb {P}^9}(1)$
and
![]() $\xi :=\mathcal {O}_{\mathbb {P}\left(E_d\right)}(1)$
. Then we have the following result of Benoist, where a special case of
$\xi :=\mathcal {O}_{\mathbb {P}\left(E_d\right)}(1)$
. Then we have the following result of Benoist, where a special case of
![]() $d=4$
is stated in Proposition 3.1:
$d=4$
is stated in Proposition 3.1:
Proposition 6.1 [Reference Benoist8, Theorem 2.7]
If
![]() $t \in \mathbb {Q}$
, then the
$t \in \mathbb {Q}$
, then the
![]() $\mathbb {Q}$
-Cartier class
$\mathbb {Q}$
-Cartier class
![]() $\overline {N}_t:=\eta + t\xi $
on
$\overline {N}_t:=\eta + t\xi $
on
![]() $\mathbb {P}(E_d)$
is ample if and only if
$\mathbb {P}(E_d)$
is ample if and only if
 $t \in \left(0, \frac {1}{d-1}\right) \cap \mathbb {Q}$
.
$t \in \left(0, \frac {1}{d-1}\right) \cap \mathbb {Q}$
.
Let
![]() $U_{\left(2,d\right)}\subset \mathbb {P}(E_d)$
be the complete intersection locus as an open subset. Then we know that
$U_{\left(2,d\right)}\subset \mathbb {P}(E_d)$
be the complete intersection locus as an open subset. Then we know that
![]() $\textrm {codim}_{\mathbb {P}(E_d)}\mathbb {P}\left(E_d\right)\setminus U_{\left(2,d\right)}\geq 2$
. There is a birational morphism
$\textrm {codim}_{\mathbb {P}(E_d)}\mathbb {P}\left(E_d\right)\setminus U_{\left(2,d\right)}\geq 2$
. There is a birational morphism
![]() $\mathrm {chow}: U_{\left(2,d\right)}\to \operatorname {Chow}_{\left(2,d\right)}$
as a restriction of the Hilbert–Chow morphism. Hence the graph of
$\mathrm {chow}: U_{\left(2,d\right)}\to \operatorname {Chow}_{\left(2,d\right)}$
as a restriction of the Hilbert–Chow morphism. Hence the graph of
![]() $\mathrm {chow}$
gives a locally closed embedding
$\mathrm {chow}$
gives a locally closed embedding
Denote by
![]() $\mathscr {P}_d$
the closure of
$\mathscr {P}_d$
the closure of
![]() $U_{\left(2,d\right)}$
in
$U_{\left(2,d\right)}$
in
![]() $\mathbb {P}(E_d)\times \operatorname {Chow}_{\left(2,d\right)}$
. Let
$\mathbb {P}(E_d)\times \operatorname {Chow}_{\left(2,d\right)}$
. Let
![]() $p_1$
and
$p_1$
and
![]() $p_2$
be the first and second projections from
$p_2$
be the first and second projections from
![]() $\mathscr {P}_d$
to
$\mathscr {P}_d$
to
![]() $\mathbb {P}(E_d)$
and
$\mathbb {P}(E_d)$
and
![]() $\operatorname {Chow}_{\left(2,d\right)}$
, respectively. The action of
$\operatorname {Chow}_{\left(2,d\right)}$
, respectively. The action of
![]() $\mathrm {SL}(4)$
on
$\mathrm {SL}(4)$
on
![]() $\mathbb {P}^3$
extends naturally to actions on
$\mathbb {P}^3$
extends naturally to actions on
![]() $U_{(2,d)}$
,
$U_{(2,d)}$
,
![]() $\mathbb {P}(E_d)$
,
$\mathbb {P}(E_d)$
,
![]() $\operatorname {Chow}_{\left(2,d\right)}$
, and
$\operatorname {Chow}_{\left(2,d\right)}$
, and
![]() $\mathscr {P}_d$
. Similar to §3.3, we will specify a family of
$\mathscr {P}_d$
. Similar to §3.3, we will specify a family of
![]() $\mathrm {SL}(4)$
-linearized ample
$\mathrm {SL}(4)$
-linearized ample
![]() $\mathbb {Q}$
-line bundles on
$\mathbb {Q}$
-line bundles on
![]() $\mathscr {P}_d$
.
$\mathscr {P}_d$
.
Fix a rational number
 $0 < \delta < \frac {2}{3d}$
. For
$0 < \delta < \frac {2}{3d}$
. For
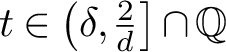 $t \in \left(\delta , \frac {2}{d}\right] \cap \mathbb {Q}$
, consider the
$t \in \left(\delta , \frac {2}{d}\right] \cap \mathbb {Q}$
, consider the
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
 $$ \begin{align*}N_t := \frac{2 - dt}{2-d\delta} p_1^*(\eta + \delta \xi) + \frac{t - \delta}{2-d\delta} p_2^*L_{\infty} ,\end{align*} $$
$$ \begin{align*}N_t := \frac{2 - dt}{2-d\delta} p_1^*(\eta + \delta \xi) + \frac{t - \delta}{2-d\delta} p_2^*L_{\infty} ,\end{align*} $$
where
![]() $L_{\infty }$
is the restriction of the natural polarization of the Chow variety to
$L_{\infty }$
is the restriction of the natural polarization of the Chow variety to
![]() $\operatorname {Chow}_{\left(2,d\right)}$
. Since
$\operatorname {Chow}_{\left(2,d\right)}$
. Since
 $\frac {2}{3d}<\frac {1}{d-1}$
, Proposition 6.1 implies that
$\frac {2}{3d}<\frac {1}{d-1}$
, Proposition 6.1 implies that
![]() $\eta +\delta \xi $
is ample on
$\eta +\delta \xi $
is ample on
![]() $\mathbb {P}(E_d)$
. It is clear that
$\mathbb {P}(E_d)$
. It is clear that
![]() $L_\infty $
is ample on
$L_\infty $
is ample on
![]() $\operatorname {Chow}_{\left(2,d\right)}$
. Hence
$\operatorname {Chow}_{\left(2,d\right)}$
. Hence
![]() $N_t$
is ample for
$N_t$
is ample for
 $\delta <t<\frac {2}{d}$
and semiample for
$\delta <t<\frac {2}{d}$
and semiample for
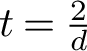 $t=\frac {2}{d}$
.
$t=\frac {2}{d}$
.
Definition 6.2. Let
![]() $\delta \in \mathbb {Q}$
satisfy
$\delta \in \mathbb {Q}$
satisfy
 $0 < \delta < \frac {2}{3d}$
. For each
$0 < \delta < \frac {2}{3d}$
. For each
 $t \in \left(\delta , \frac {2}{d}\right) \cap \mathbb {Q}$
, we define the VGIT quotient stack
$t \in \left(\delta , \frac {2}{d}\right) \cap \mathbb {Q}$
, we define the VGIT quotient stack
![]() $\mathscr {M}_d(t)$
and the VGIT quotient space
$\mathscr {M}_d(t)$
and the VGIT quotient space
![]() $\mathfrak {M}_d(t)$
of slope t to be
$\mathfrak {M}_d(t)$
of slope t to be
This definition a priori depends on the choice of
 $\delta \in \left(0,\frac {2}{3d}\right)$
. Nevertheless, similar to [Reference Laza and O’Grady43], we will show in Theorem 6.6(1) that neither
$\delta \in \left(0,\frac {2}{3d}\right)$
. Nevertheless, similar to [Reference Laza and O’Grady43], we will show in Theorem 6.6(1) that neither
![]() $\mathscr {M}_d(t)$
nor
$\mathscr {M}_d(t)$
nor
![]() $\mathfrak {M}_d(t)$
depends on the choice of
$\mathfrak {M}_d(t)$
depends on the choice of
![]() $\delta $
, and hence they are well defined for all
$\delta $
, and hence they are well defined for all
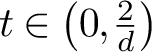 $t\in \left(0,\frac {2}{d}\right)$
. Before stating the main VGIT result (Theorem 6.6), we need some preparation.
$t\in \left(0,\frac {2}{d}\right)$
. Before stating the main VGIT result (Theorem 6.6), we need some preparation.
Lemma 6.3. With notation as before, we have
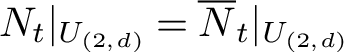 $N_t\rvert _{U_{\left(2,d\right)}}= \overline {N}_t\rvert _{U_{\left(2,d\right)}}$
for any
$N_t\rvert _{U_{\left(2,d\right)}}= \overline {N}_t\rvert _{U_{\left(2,d\right)}}$
for any
 $t\in \left(\delta ,\frac {2}{d}\right]\cap \mathbb {Q}$
.
$t\in \left(\delta ,\frac {2}{d}\right]\cap \mathbb {Q}$
.
Proof. Denote by
![]() $\overline {L}_\infty $
the unique extension of
$\overline {L}_\infty $
the unique extension of
![]() $L_\infty \rvert _{U_{\left(2,d\right)}}$
to
$L_\infty \rvert _{U_{\left(2,d\right)}}$
to
![]() $\mathbb {P}(E_d)$
. By the same argument as [Reference Laza and O’Grady43, Proposition 5.4], we get that
$\mathbb {P}(E_d)$
. By the same argument as [Reference Laza and O’Grady43, Proposition 5.4], we get that
![]() $\overline {L}_\infty =d\eta +2\xi $
. Hence we have
$\overline {L}_\infty =d\eta +2\xi $
. Hence we have
 $$ \begin{align*} N_t\rvert_{U_{\left(2,d\right)}}& =\frac{2 - dt}{2-d\delta} (\eta + \delta \xi)\rvert_{U_{\left(2,d\right)}} + \frac{t - \delta}{2-d\delta} \overline{L}_{\infty}\rvert_{U_{\left(2,d\right)}}\\ & = \frac{2 - dt}{2-d\delta} (\eta + \delta \xi)\rvert_{U_{\left(2,d\right)}} + \frac{t - \delta}{2-d\delta} (d\eta+2\xi)\rvert_{U_{\left(2,d\right)}} = (\eta+t\xi)\rvert_{U_{\left(2,d\right)}}. \end{align*} $$
$$ \begin{align*} N_t\rvert_{U_{\left(2,d\right)}}& =\frac{2 - dt}{2-d\delta} (\eta + \delta \xi)\rvert_{U_{\left(2,d\right)}} + \frac{t - \delta}{2-d\delta} \overline{L}_{\infty}\rvert_{U_{\left(2,d\right)}}\\ & = \frac{2 - dt}{2-d\delta} (\eta + \delta \xi)\rvert_{U_{\left(2,d\right)}} + \frac{t - \delta}{2-d\delta} (d\eta+2\xi)\rvert_{U_{\left(2,d\right)}} = (\eta+t\xi)\rvert_{U_{\left(2,d\right)}}. \end{align*} $$
The proof is finished.
The following lemma is very useful (see [Reference Casalaina-Martin, Jensen and Laza16, Propositions 4.6 and 6.2] and Lemma 3.5 for
![]() $d=3,4$
):
$d=3,4$
):
Lemma 6.4. For each
 $t\in \left(\delta ,\frac {2}{d}\right)\cap \mathbb {Q} \left(\text {resp., }t\in \left(0,\frac {1}{d-1}\right)\cap \mathbb {Q}\right)$
, the VGIT semistable locus
$t\in \left(\delta ,\frac {2}{d}\right)\cap \mathbb {Q} \left(\text {resp., }t\in \left(0,\frac {1}{d-1}\right)\cap \mathbb {Q}\right)$
, the VGIT semistable locus
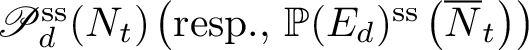 $\mathscr {P}_d^{\textrm {ss}}(N_t) \left(\text {resp., }\mathbb {P}(E_d)^{\textrm {ss}}\left(\overline {N}_t\right)\right)$
of slope t is a Zariski open subset of
$\mathscr {P}_d^{\textrm {ss}}(N_t) \left(\text {resp., }\mathbb {P}(E_d)^{\textrm {ss}}\left(\overline {N}_t\right)\right)$
of slope t is a Zariski open subset of
![]() $U_{\left(2,d\right)}$
.
$U_{\left(2,d\right)}$
.
Proof. We first consider the VGIT semistable locus of
![]() $\mathbb {P}(E_d)$
. Let
$\mathbb {P}(E_d)$
. Let
![]() $([Q], [s])$
be a point in
$([Q], [s])$
be a point in
![]() $\mathbb {P}(E_d)\setminus U_{\left(2,d\right)}$
, where
$\mathbb {P}(E_d)\setminus U_{\left(2,d\right)}$
, where
![]() $Q=(q=0)$
is a nonnormal quadric surface in
$Q=(q=0)$
is a nonnormal quadric surface in
![]() $\mathbb {P}^3$
and
$\mathbb {P}^3$
and
![]() $0\neq s\in H^0\left(Q,\mathcal {O}_Q(d)\right)$
. Let
$0\neq s\in H^0\left(Q,\mathcal {O}_Q(d)\right)$
. Let
 $g\in H^0\left(\mathbb {P}^3, \mathcal {O}_{\mathbb {P}^3}(d)\right)$
be a lifting of s. We choose suitable projective coordinates
$g\in H^0\left(\mathbb {P}^3, \mathcal {O}_{\mathbb {P}^3}(d)\right)$
be a lifting of s. We choose suitable projective coordinates
![]() $[x_0,x_1,x_2,x_3]$
of
$[x_0,x_1,x_2,x_3]$
of
![]() $\mathbb {P}^3$
such that one of the following holds:
$\mathbb {P}^3$
such that one of the following holds:
-
(a)
 $q=x_0 x_1$
;
$q=x_0 x_1$
;
 $g=x_0 h$
, where
$g=x_0 h$
, where
 $h\in \mathbb {C}[x_0,\dotsc , x_3]_{d-1}$
; and
$h\in \mathbb {C}[x_0,\dotsc , x_3]_{d-1}$
; and
 $x_1\nmid h$
; or
$x_1\nmid h$
; or -
(b)
 $q=x_0^2$
; and
$q=x_0^2$
; and
 $g=x_0 h$
, where
$g=x_0 h$
, where
 $h\in \mathbb {C}[x_0,\dotsc , x_3]_{d-1}$
; and
$h\in \mathbb {C}[x_0,\dotsc , x_3]_{d-1}$
; and
 $x_0\nmid h$
.
$x_0\nmid h$
.
Let
![]() $\sigma $
be the
$\sigma $
be the
![]() $1$
-PS in
$1$
-PS in
![]() $\mathrm {SL}(4)$
of weights
$\mathrm {SL}(4)$
of weights
![]() $(-3,1,1,1)$
with respect to the chosen coordinates. By [Reference Benoist8, Proposition 2.15], for any
$(-3,1,1,1)$
with respect to the chosen coordinates. By [Reference Benoist8, Proposition 2.15], for any
 $t\in \left(0,\frac {2}{d}\right]$
we have
$t\in \left(0,\frac {2}{d}\right]$
we have
 $$ \begin{align*} \mu^{\overline{N}_t}(([Q], [s]), \sigma)\leq \mu(q,\sigma)+t\mu(g,\sigma)\leq -2+t(d-4)<0. \end{align*} $$
$$ \begin{align*} \mu^{\overline{N}_t}(([Q], [s]), \sigma)\leq \mu(q,\sigma)+t\mu(g,\sigma)\leq -2+t(d-4)<0. \end{align*} $$
Hence
![]() $([Q], [s])$
is VGIT unstable of slope t, by the Hilbert–Mumford numerical criterion.
$([Q], [s])$
is VGIT unstable of slope t, by the Hilbert–Mumford numerical criterion.
Next we consider the VGIT semistable locus of
![]() $\mathscr {P}_d$
. It is clear that any point z in
$\mathscr {P}_d$
. It is clear that any point z in
![]() $\mathscr {P}_d\setminus U_{\left(2,d\right)}$
has the form
$\mathscr {P}_d\setminus U_{\left(2,d\right)}$
has the form
![]() $z=(([Q],[s]), \mathrm {chow}(\mathscr {C}))$
, where
$z=(([Q],[s]), \mathrm {chow}(\mathscr {C}))$
, where
![]() $([Q],[s])\in \mathbb {P}(E_d)\setminus U_{\left(2,d\right)}$
,
$([Q],[s])\in \mathbb {P}(E_d)\setminus U_{\left(2,d\right)}$
,
![]() $\mathscr {C}\in \mathrm {Hilb}_{\left(2,d\right)}\setminus U_{\left(2,d\right)}$
, and
$\mathscr {C}\in \mathrm {Hilb}_{\left(2,d\right)}\setminus U_{\left(2,d\right)}$
, and
![]() $\mathrm {chow}: \mathrm {Hilb}_{\left(2,d\right)}\to \operatorname {Chow}_{\left(2,d\right)}$
is the Hilbert–Chow morphism. We choose
$\mathrm {chow}: \mathrm {Hilb}_{\left(2,d\right)}\to \operatorname {Chow}_{\left(2,d\right)}$
is the Hilbert–Chow morphism. We choose
![]() $[x_0,\dotsc ,x_3]$
and
$[x_0,\dotsc ,x_3]$
and
![]() $\sigma $
as before. Then
$\sigma $
as before. Then
 $$ \begin{align*} \mu^{N_t}(z, \sigma)=\frac{2-dt}{2-d\delta} \mu^{\overline{N}_{\delta}}(([Q],[s]),\sigma)+\frac{t-\delta}{2-d\delta}\mu^{L_\infty}(\mathrm{chow}(\mathscr{C}),\sigma). \end{align*} $$
$$ \begin{align*} \mu^{N_t}(z, \sigma)=\frac{2-dt}{2-d\delta} \mu^{\overline{N}_{\delta}}(([Q],[s]),\sigma)+\frac{t-\delta}{2-d\delta}\mu^{L_\infty}(\mathrm{chow}(\mathscr{C}),\sigma). \end{align*} $$
From the foregoing argument we get
 $\mu ^{\overline {N}_{\delta }}(([Q],[s]),\sigma )<0$
. By [Reference Laza and O’Grady43, Propostion 5.8] we know that
$\mu ^{\overline {N}_{\delta }}(([Q],[s]),\sigma )<0$
. By [Reference Laza and O’Grady43, Propostion 5.8] we know that
![]() $\mu ^{L_\infty }(\mathrm {chow}(\mathscr {C}),\sigma )<0$
. Hence
$\mu ^{L_\infty }(\mathrm {chow}(\mathscr {C}),\sigma )<0$
. Hence
![]() $\mu ^{N_t}(z,\sigma )<0$
for any
$\mu ^{N_t}(z,\sigma )<0$
for any
 $t\in \left(\delta ,\frac {2}{d}\right)\cap \mathbb {Q}$
, and the proof is finished.
$t\in \left(\delta ,\frac {2}{d}\right)\cap \mathbb {Q}$
, and the proof is finished.
Indeed, we have a stronger result on VGIT semistable loci (see [Reference Laza and O’Grady43, Lemma 6.8] for
![]() $d=4$
):
$d=4$
):
Lemma 6.5. For each
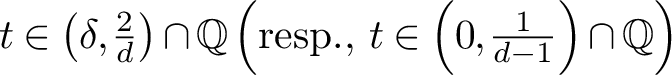 $t\in \left(\delta ,\frac {2}{d}\right)\cap \mathbb {Q} \left(\text {resp., }t\in \left(0,\frac {1}{d-1}\right)\cap \mathbb {Q}\right)$
, any VGIT semistable point in
$t\in \left(\delta ,\frac {2}{d}\right)\cap \mathbb {Q} \left(\text {resp., }t\in \left(0,\frac {1}{d-1}\right)\cap \mathbb {Q}\right)$
, any VGIT semistable point in
 $\mathscr {P}_d^{\textrm {ss}}(N_t) \left(\text {resp., }\mathbb {P}(E_d)^{\textrm {ss}}\left(\overline {N}_t\right)\right)$
of slope t has the form
$\mathscr {P}_d^{\textrm {ss}}(N_t) \left(\text {resp., }\mathbb {P}(E_d)^{\textrm {ss}}\left(\overline {N}_t\right)\right)$
of slope t has the form
![]() $([Q],[s])$
, where
$([Q],[s])$
, where
![]() $\mathrm {rank}(Q)\geq 3$
.
$\mathrm {rank}(Q)\geq 3$
.
Proof. Let
![]() $z=([Q],[s])$
be a point in
$z=([Q],[s])$
be a point in
![]() $U_{\left(2,d\right)}$
, where
$U_{\left(2,d\right)}$
, where
![]() $\mathrm {rank}(Q)\leq 2$
. Hence by Lemma 6.4, it suffices to show the instability of z in
$\mathrm {rank}(Q)\leq 2$
. Hence by Lemma 6.4, it suffices to show the instability of z in
![]() $\mathbb {P}(E_d)$
and
$\mathbb {P}(E_d)$
and
![]() $\mathscr {P}_d$
, respectively. We will assume
$\mathscr {P}_d$
, respectively. We will assume
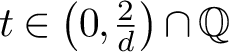 $t\in \left(0,\frac {2}{d}\right)\cap \mathbb {Q}$
throughout the proof. Choose a projective coordinate
$t\in \left(0,\frac {2}{d}\right)\cap \mathbb {Q}$
throughout the proof. Choose a projective coordinate
![]() $[x_0,\dotsc ,x_3]$
such that
$[x_0,\dotsc ,x_3]$
such that
![]() $Q=(q=0)$
is defined by
$Q=(q=0)$
is defined by
![]() $q=x_0^2$
or
$q=x_0^2$
or
![]() $x_0x_1$
. Let
$x_0x_1$
. Let
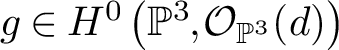 $g\in H^0\left(\mathbb {P}^3, \mathcal {O}_{\mathbb {P}^3}(d)\right)$
be a lifting of s. Let
$g\in H^0\left(\mathbb {P}^3, \mathcal {O}_{\mathbb {P}^3}(d)\right)$
be a lifting of s. Let
![]() $\sigma $
be the
$\sigma $
be the
![]() $1$
-PS in
$1$
-PS in
![]() $\mathrm {SL}(4)$
of weights
$\mathrm {SL}(4)$
of weights
![]() $(-1,-1,1,1)$
with respect to the chosen coordinates. Then by [Reference Benoist8, Proposition 2.15],
$(-1,-1,1,1)$
with respect to the chosen coordinates. Then by [Reference Benoist8, Proposition 2.15],
 $$ \begin{align*} \mu^{\overline{N}_t}(z, \sigma)\leq \mu(q,\sigma)+t\mu(g,\sigma)\leq -2+td<0. \end{align*} $$
$$ \begin{align*} \mu^{\overline{N}_t}(z, \sigma)\leq \mu(q,\sigma)+t\mu(g,\sigma)\leq -2+td<0. \end{align*} $$
Hence z is
![]() $\overline {N}_t$
-unstable in
$\overline {N}_t$
-unstable in
![]() $\mathbb {P}(E_d)$
. It is clear that
$\mathbb {P}(E_d)$
. It is clear that
![]() $\lim _{r\to 0}\lambda (r)\cdot ([Q],[s])=([Q], [g(0,0,x_2,x_3)])$
in
$\lim _{r\to 0}\lambda (r)\cdot ([Q],[s])=([Q], [g(0,0,x_2,x_3)])$
in
![]() $\mathbb {P}(E_d)$
. Hence for general s, we see that
$\mathbb {P}(E_d)$
. Hence for general s, we see that
![]() $\lim _{r\to 0}\lambda (r)\cdot ([Q],[s])$
belongs to
$\lim _{r\to 0}\lambda (r)\cdot ([Q],[s])$
belongs to
![]() $U_{\left(2,d\right)}$
. In particular, Lemma 6.3 implies that
$U_{\left(2,d\right)}$
. In particular, Lemma 6.3 implies that
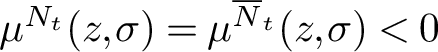 $\mu ^{N_t}(z,\sigma )=\mu ^{\overline {N}_t}(z,\sigma )<0$
, so z is
$\mu ^{N_t}(z,\sigma )=\mu ^{\overline {N}_t}(z,\sigma )<0$
, so z is
![]() $N_t$
-unstable in
$N_t$
-unstable in
![]() $\mathscr {P}_d$
when s is general. Since the GIT unstable locus is closed, we conclude that z is
$\mathscr {P}_d$
when s is general. Since the GIT unstable locus is closed, we conclude that z is
![]() $N_t$
-unstable for any choice of s.
$N_t$
-unstable for any choice of s.
The following theorem is a generalization of [Reference Laza and O’Grady43, Theorem 5.6]:
Theorem 6.6. Let
![]() $\delta $
be as before. The following hold:
$\delta $
be as before. The following hold:
-
(1) The VGIT semistable locus
 $\mathscr {P}_d^{\textrm {ss}}(N_t)$
is independent of the choice of
$\mathscr {P}_d^{\textrm {ss}}(N_t)$
is independent of the choice of
 $\delta $
.
$\delta $
. -
(2) For
 $t \in \left(\delta , \frac {1}{d-1}\right)$
, we have
$t \in \left(\delta , \frac {1}{d-1}\right)$
, we have
 $\mathscr {M}_d(t)\cong \left[\mathbb {P}(E_d)^{\textrm {ss}}\left(\overline {N}_t\right)/\mathrm {PGL}(4)\right]$
and
$\mathscr {M}_d(t)\cong \left[\mathbb {P}(E_d)^{\textrm {ss}}\left(\overline {N}_t\right)/\mathrm {PGL}(4)\right]$
and
 $\mathfrak {M}_d(t) \cong \mathbb {P}(E_d) \mathbin {/\mkern -6mu/}_{\overline {N}_t} \mathrm {SL}(4)$
.
$\mathfrak {M}_d(t) \cong \mathbb {P}(E_d) \mathbin {/\mkern -6mu/}_{\overline {N}_t} \mathrm {SL}(4)$
. -
(3) For
 $t \in \left(\delta , \frac {2}{3d}\right)$
, we have
$t \in \left(\delta , \frac {2}{3d}\right)$
, we have
 $\mathscr {M}_d(t)\cong \mathscr {M}_d$
and
$\mathscr {M}_d(t)\cong \mathscr {M}_d$
and
 $\mathfrak {M}_d(t) \cong \mathfrak {M}_d$
.
$\mathfrak {M}_d(t) \cong \mathfrak {M}_d$
.
Proof. (1) Let
![]() $\delta $
and
$\delta $
and
![]() $\delta '$
be two rational numbers in
$\delta '$
be two rational numbers in
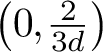 $\left(0, \frac {2}{3d}\right)$
. Denote
$\left(0, \frac {2}{3d}\right)$
. Denote
![]() $G:=\mathrm {SL}(4)$
. Denote the corresponding polarization on
$G:=\mathrm {SL}(4)$
. Denote the corresponding polarization on
![]() $\mathscr {P}_d$
by
$\mathscr {P}_d$
by
![]() $N_t$
and
$N_t$
and
![]() $N_t'$
. Since both GIT semistable loci
$N_t'$
. Since both GIT semistable loci
![]() $\mathscr {P}_d$
with respect to
$\mathscr {P}_d$
with respect to
![]() $N_t$
and
$N_t$
and
![]() $N_t'$
are contained in
$N_t'$
are contained in
![]() $U_{\left(2,d\right)}$
, where their restrictions are the same by Lemmas 6.3 and 6.4, [Reference Casalaina-Martin, Jensen and Laza16, Lemma 4.17] implies that for
$U_{\left(2,d\right)}$
, where their restrictions are the same by Lemmas 6.3 and 6.4, [Reference Casalaina-Martin, Jensen and Laza16, Lemma 4.17] implies that for
![]() $m\in \mathbb {N}$
sufficiently divisible we have
$m\in \mathbb {N}$
sufficiently divisible we have
 $$ \begin{align*} H^0\left(\mathscr{P}_d, N_t^{\otimes m}\right)^G\xrightarrow{\cong } H^0\left(U_{\left(2,d\right)}, N_t\rvert_{U_{\left(2,d\right)}}^{\otimes m}\right)^G=H^0\left(U_{\left(2,d\right)}, N_t'\rvert_{U_{\left(2,d\right)}}^{\otimes m}\right)^G\xleftarrow[]{\cong} H^0\left(\mathscr{P}_d, N_t'^{\otimes m}\right)^G. \end{align*} $$
$$ \begin{align*} H^0\left(\mathscr{P}_d, N_t^{\otimes m}\right)^G\xrightarrow{\cong } H^0\left(U_{\left(2,d\right)}, N_t\rvert_{U_{\left(2,d\right)}}^{\otimes m}\right)^G=H^0\left(U_{\left(2,d\right)}, N_t'\rvert_{U_{\left(2,d\right)}}^{\otimes m}\right)^G\xleftarrow[]{\cong} H^0\left(\mathscr{P}_d, N_t'^{\otimes m}\right)^G. \end{align*} $$
Since both
![]() $\mathscr {P}_d^{\textrm {ss}}(N_t)$
and
$\mathscr {P}_d^{\textrm {ss}}(N_t)$
and
![]() $\mathscr {P}_d^{\textrm {ss}}\left(N_t'\right)$
are unions of nonvanishing loci of G-invariant sections in the first and last terms of this diagram, we know that they are equal. Hence
$\mathscr {P}_d^{\textrm {ss}}\left(N_t'\right)$
are unions of nonvanishing loci of G-invariant sections in the first and last terms of this diagram, we know that they are equal. Hence
![]() $\mathscr {P}_d^{\textrm {ss}}(N_t)$
is independent of the choice of
$\mathscr {P}_d^{\textrm {ss}}(N_t)$
is independent of the choice of
![]() $\delta $
.
$\delta $
.
(2) The proof is similar to that of (1), using Lemmas 6.3 and 6.4 and [Reference Casalaina-Martin, Jensen and Laza16, Lemma 4.17].
(3) By (2) it suffices to show that
 $\left[\mathbb {P}(E_d)^{\textrm {ss}}\left(\overline {N}_t\right)/\mathrm {PGL}(4)\right]\cong \mathscr {M}_d$
for
$\left[\mathbb {P}(E_d)^{\textrm {ss}}\left(\overline {N}_t\right)/\mathrm {PGL}(4)\right]\cong \mathscr {M}_d$
for
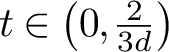 $t\in \left(0, \frac {2}{3d}\right)$
. By Lemma 6.5, we know that any GIT semistable point
$t\in \left(0, \frac {2}{3d}\right)$
. By Lemma 6.5, we know that any GIT semistable point
![]() $z\in \mathbb {P}(E_d)$
with respect to
$z\in \mathbb {P}(E_d)$
with respect to
![]() $\overline {N}_t$
has the form
$\overline {N}_t$
has the form
![]() $z=([Q],[s])$
, where
$z=([Q],[s])$
, where
![]() $\mathrm {rank}(Q)\geq 3$
. We will show that under the assumption
$\mathrm {rank}(Q)\geq 3$
. We will show that under the assumption
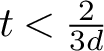 $t<\frac {2}{3d}$
, the quadric surface Q must be smooth. Assume to the contrary that
$t<\frac {2}{3d}$
, the quadric surface Q must be smooth. Assume to the contrary that
![]() $Q=(q=0)$
is singular. Then we may choose a projective coordinate
$Q=(q=0)$
is singular. Then we may choose a projective coordinate
![]() $[x_0,\dotsc ,x_3]$
of
$[x_0,\dotsc ,x_3]$
of
![]() $\mathbb {P}^3$
such that
$\mathbb {P}^3$
such that
![]() $q\in \mathbb {C}[x_1,x_2,x_3]_2$
. Let
$q\in \mathbb {C}[x_1,x_2,x_3]_2$
. Let
![]() $\sigma $
be the
$\sigma $
be the
![]() $1$
-PS in
$1$
-PS in
![]() $\mathrm {SL}(4)$
with weights
$\mathrm {SL}(4)$
with weights
![]() $(3,-1,-1,-1)$
. Let
$(3,-1,-1,-1)$
. Let
 $g\in H^0\left(\mathbb {P}^3,\mathcal {O}_{\mathbb {P}^3}(d)\right)$
be a lifting of s. Then by [Reference Benoist8, Proposition 2.15] we have
$g\in H^0\left(\mathbb {P}^3,\mathcal {O}_{\mathbb {P}^3}(d)\right)$
be a lifting of s. Then by [Reference Benoist8, Proposition 2.15] we have
 $$ \begin{align*} \mu^{\overline{N}_t}(z, \sigma)\leq \mu(q, \sigma)+t\mu(g, \sigma)\leq -2+t\cdot 3d<0. \end{align*} $$
$$ \begin{align*} \mu^{\overline{N}_t}(z, \sigma)\leq \mu(q, \sigma)+t\mu(g, \sigma)\leq -2+t\cdot 3d<0. \end{align*} $$
Hence z is
![]() $\overline {N}_t$
-unstable on
$\overline {N}_t$
-unstable on
![]() $\mathbb {P}(E_d)$
. Since
$\mathbb {P}(E_d)$
. Since
![]() $\sigma $
fixes Q, we know that
$\sigma $
fixes Q, we know that
![]() $\lim _{r\to 0}\sigma (r)\cdot z$
belongs to
$\lim _{r\to 0}\sigma (r)\cdot z$
belongs to
![]() $U_{\left(2,d\right)}$
. Hence
$U_{\left(2,d\right)}$
. Hence
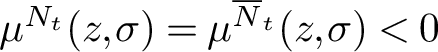 $\mu ^{N_t}(z, \sigma )=\mu ^{\overline {N}_t}(z, \sigma )<0$
, by Lemma 6.3, which implies that z is
$\mu ^{N_t}(z, \sigma )=\mu ^{\overline {N}_t}(z, \sigma )<0$
, by Lemma 6.3, which implies that z is
![]() $N_t$
-unstable on
$N_t$
-unstable on
![]() $\mathscr {P}_d$
. The rest of the proof is similar to [Reference Casalaina-Martin, Jensen and Laza16, Lemma 4.18].
$\mathscr {P}_d$
. The rest of the proof is similar to [Reference Casalaina-Martin, Jensen and Laza16, Lemma 4.18].
Remark 6.7. When
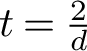 $t=\frac {2}{d}$
, we can define the VGIT quotient stack and space by
$t=\frac {2}{d}$
, we can define the VGIT quotient stack and space by
 $$ \begin{align*} \mathscr{M}_d\left(\tfrac{2}{d}\right):=\left[\operatorname{Chow}_{\left(2,d\right)}^{\textrm{ss}}/\mathrm{PGL}(4)\right],\qquad \mathfrak{M}_d\left(\tfrac{2}{d}\right):=\operatorname{Chow}_{\left(2,d\right)}\mathbin{/\mkern-6mu/} \mathrm{SL}(4). \end{align*} $$
$$ \begin{align*} \mathscr{M}_d\left(\tfrac{2}{d}\right):=\left[\operatorname{Chow}_{\left(2,d\right)}^{\textrm{ss}}/\mathrm{PGL}(4)\right],\qquad \mathfrak{M}_d\left(\tfrac{2}{d}\right):=\operatorname{Chow}_{\left(2,d\right)}\mathbin{/\mkern-6mu/} \mathrm{SL}(4). \end{align*} $$
As in [Reference Laza and O’Grady43], one can show that there are natural wall-crossing morphisms
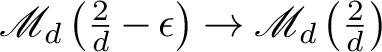 $\mathscr {M}_d\left(\frac {2}{d}-\epsilon \right)\to \mathscr {M}_d\left(\frac {2}{d}\right)$
and
$\mathscr {M}_d\left(\frac {2}{d}-\epsilon \right)\to \mathscr {M}_d\left(\frac {2}{d}\right)$
and
 $\mathfrak {M}_d\left(\frac {2}{d}-\epsilon \right)\to \mathfrak {M}_d\left(\frac {2}{d}\right)$
for
$\mathfrak {M}_d\left(\frac {2}{d}-\epsilon \right)\to \mathfrak {M}_d\left(\frac {2}{d}\right)$
for
![]() $0<\epsilon \ll 1$
. We omit further discussion on the Chow quotient, since it is not directly related to our K-moduli spaces when
$0<\epsilon \ll 1$
. We omit further discussion on the Chow quotient, since it is not directly related to our K-moduli spaces when
![]() $d\neq 4$
(see, e.g., Remark 6.10).
$d\neq 4$
(see, e.g., Remark 6.10).
6.2 Proofs
In this section we prove Theorem 1.4, starting with part (1).
Proof of Theorem 1.4(1). The proof is similar to that of Theorem 5.2. Consider the universal family
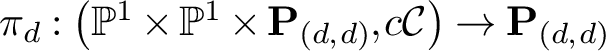 $\pi _d: \left(\mathbb {P}^1 \times \mathbb {P}^1 \times \mathbf {P}_{\left(d,d\right)}, c\mathcal C\right) \to \mathbf {P}_{\left(d,d\right)}$
over the parameter space of
$\pi _d: \left(\mathbb {P}^1 \times \mathbb {P}^1 \times \mathbf {P}_{\left(d,d\right)}, c\mathcal C\right) \to \mathbf {P}_{\left(d,d\right)}$
over the parameter space of
![]() $(d,d)$
-curves on
$(d,d)$
-curves on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
. It is clear that
$\mathbb {P}^1\times \mathbb {P}^1$
. It is clear that
![]() $\mathcal C \in \lvert \mathcal {O}(d,d,1)\rvert $
. Hence by Proposition 2.17, we know that the CM
$\mathcal C \in \lvert \mathcal {O}(d,d,1)\rvert $
. Hence by Proposition 2.17, we know that the CM
![]() $\mathbb {Q}$
-line bundle
$\mathbb {Q}$
-line bundle
![]() $\lambda _{\mathrm {CM}, \pi _d, c\mathcal C}$
is equal to
$\lambda _{\mathrm {CM}, \pi _d, c\mathcal C}$
is equal to
 $\mathcal {O}_{\mathbf {P}_{\left(d,d\right)}}\left(3(2-dc)^2 c\right)$
, which is ample for
$\mathcal {O}_{\mathbf {P}_{\left(d,d\right)}}\left(3(2-dc)^2 c\right)$
, which is ample for
 $c\in \left(0,\frac {2}{d}\right)$
. Hence the K-(poly/semi)stability of
$c\in \left(0,\frac {2}{d}\right)$
. Hence the K-(poly/semi)stability of
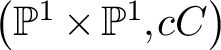 $\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
implies the GIT-(poly/semi)stability of C. For the other direction, let
$\left(\mathbb {P}^1\times \mathbb {P}^1, cC\right)$
implies the GIT-(poly/semi)stability of C. For the other direction, let
![]() $(X,cD)$
be a K-semistable pair parametrized by
$(X,cD)$
be a K-semistable pair parametrized by
![]() $\overline {\mathcal {K}}_{d,c}$
, with
$\overline {\mathcal {K}}_{d,c}$
, with
 $c\in \left(0, \frac {1}{2d}\right)$
. By [Reference Li and Liu51], for any point
$c\in \left(0, \frac {1}{2d}\right)$
. By [Reference Li and Liu51], for any point
![]() $x\in X$
we have
$x\in X$
we have
 $$ \begin{align*} \widehat{\mathrm{vol}}(x,X)\geq \widehat{\mathrm{vol}}(x,X,cD)\geq \frac{4}{9}(-K_X-cD)^2=\frac{32}{9}(1-dc)^2>2. \end{align*} $$
$$ \begin{align*} \widehat{\mathrm{vol}}(x,X)\geq \widehat{\mathrm{vol}}(x,X,cD)\geq \frac{4}{9}(-K_X-cD)^2=\frac{32}{9}(1-dc)^2>2. \end{align*} $$
This implies that any
![]() $x\in X$
is smooth, hence
$x\in X$
is smooth, hence
![]() $X\cong \mathbb {P}^1\times \mathbb {P}^1$
. The rest of the proof is exactly the same as for Theorem 5.2.□
$X\cong \mathbb {P}^1\times \mathbb {P}^1$
. The rest of the proof is exactly the same as for Theorem 5.2.□
Remark 6.8. Similar to Proposition 5.3, we have that
 $c_1=\frac {1}{2d}$
is the first K-moduli wall for
$c_1=\frac {1}{2d}$
is the first K-moduli wall for
![]() $(d,d)$
-curves on
$(d,d)$
-curves on
![]() $\mathbb {P}^1\times \mathbb {P}^1$
, which replaces
$\mathbb {P}^1\times \mathbb {P}^1$
, which replaces
 $\left(\mathbb {P}^1\times \mathbb {P}^1, dH\right)$
by
$\left(\mathbb {P}^1\times \mathbb {P}^1, dH\right)$
by
![]() $(\mathbb {P}(1,1,2), D)$
where H is a smooth
$(\mathbb {P}(1,1,2), D)$
where H is a smooth
![]() $(1,1)$
-curve.
$(1,1)$
-curve.
Next we prove part (2) of Theorem 1.4. Before starting the proof, we need some preparation on CM line bundles as a generalization of Propositions 5.6 and 5.7:
Proposition 6.9. For simplicity, denote
![]() $U:=U_{\left(2,d\right)}$
. Let
$U:=U_{\left(2,d\right)}$
. Let
![]() $f_U:(\mathscr {X}_U,\mathscr {D}_U)\to U$
be the restriction of
$f_U:(\mathscr {X}_U,\mathscr {D}_U)\to U$
be the restriction of
![]() $f:(\mathscr {X},\mathscr {D})\to \mathbb {P}(E_d)$
over
$f:(\mathscr {X},\mathscr {D})\to \mathbb {P}(E_d)$
over
![]() $U\subset \mathbb {P}(E_d)$
. We denote the CM
$U\subset \mathbb {P}(E_d)$
. We denote the CM
![]() $\mathbb {Q}$
-line bundle of
$\mathbb {Q}$
-line bundle of
![]() $f_U$
with coefficient c by
$f_U$
with coefficient c by
![]() $\lambda _{U,c}:=\lambda _{\mathrm {CM}, f_U, c\mathscr {D}_U}$
. Then
$\lambda _{U,c}:=\lambda _{\mathrm {CM}, f_U, c\mathscr {D}_U}$
. Then
![]() $\lambda _{U,c}$
and
$\lambda _{U,c}$
and
![]() $N_t\rvert _U$
are proportional up to a positive constant, where
$N_t\rvert _U$
are proportional up to a positive constant, where
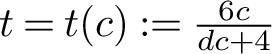 $t=t(c):=\frac {6c}{dc+4}$
and
$t=t(c):=\frac {6c}{dc+4}$
and
 $c\in \left(0,\frac {2}{d}\right)$
.
$c\in \left(0,\frac {2}{d}\right)$
.
Proof. By the same computations as in §5.2, we get
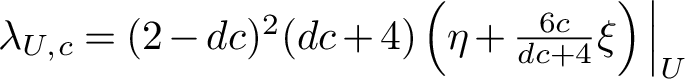 $\lambda _{U,c}={(2-dc)^2(dc+4)}\left(\eta +\frac {6c}{dc+4}\xi \right)\Big \rvert _U\\[3pt]$
.
$\lambda _{U,c}={(2-dc)^2(dc+4)}\left(\eta +\frac {6c}{dc+4}\xi \right)\Big \rvert _U\\[3pt]$
.
Proof of Theorem 1.4(2). We first fix some notation. Let
![]() $U_c^{\mathrm {K}}$
be the open subset of
$U_c^{\mathrm {K}}$
be the open subset of
![]() $U=U_{\left(2,d\right)}$
parametrizing c-K-semistable log Fano pairs. Let
$U=U_{\left(2,d\right)}$
parametrizing c-K-semistable log Fano pairs. Let
![]() $U_c^{\mathrm {GIT}}:=\mathscr {P}_d^{\textrm {ss}}(N_t)$
be the open subset of U parametrizing VGIT semistable points of slope
$U_c^{\mathrm {GIT}}:=\mathscr {P}_d^{\textrm {ss}}(N_t)$
be the open subset of U parametrizing VGIT semistable points of slope
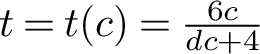 $t=t(c)=\frac {6c}{dc+4}$
. Similar to Proposition 5.9, by Theorem 4.10 we know that
$t=t(c)=\frac {6c}{dc+4}$
. Similar to Proposition 5.9, by Theorem 4.10 we know that
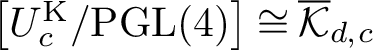 $\left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]\cong \overline {\mathcal {K}}_{d,c}$
as long as
$\left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]\cong \overline {\mathcal {K}}_{d,c}$
as long as
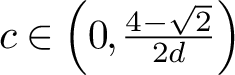 $c\in \left(0, \frac {4-\sqrt {2}}{2d}\right)$
. Hence it suffices to show
$c\in \left(0, \frac {4-\sqrt {2}}{2d}\right)$
. Hence it suffices to show
![]() $U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
for
$U_c^{\mathrm {K}}=U_c^{\mathrm {GIT}}$
for
 $c\in \left(0,\frac {4-\sqrt {2}}{2d}\right)$
.
$c\in \left(0,\frac {4-\sqrt {2}}{2d}\right)$
.
We follow the strategy in the proof of Theorem 5.8 – that is, induction on the walls for K-moduli and VGIT. It suffices to generalize Propositions 5.10, 5.11, and 5.12 to
![]() $(2,d)$
complete intersections under the assumption
$(2,d)$
complete intersections under the assumption
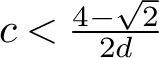 $c<\frac {4-\sqrt {2}}{2d}$
. The generalization of Proposition 5.10 follows from Theorems 1.4(1) and 6.6(3). For Propositions 5.11 and 5.12, we can generalize them using
$c<\frac {4-\sqrt {2}}{2d}$
. The generalization of Proposition 5.10 follows from Theorems 1.4(1) and 6.6(3). For Propositions 5.11 and 5.12, we can generalize them using
 $\left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]\cong \overline {\mathcal {K}}_{d,c}$
, Proposition 6.9, and Theorem 4.4.□
$\left[U_c^{\mathrm {K}}/\mathrm {PGL}(4)\right]\cong \overline {\mathcal {K}}_{d,c}$
, Proposition 6.9, and Theorem 4.4.□
Remark 6.10. If
![]() $d\neq 4$
, then the isomorphism
$d\neq 4$
, then the isomorphism
![]() $\overline {K}_{d,c}\cong \mathfrak {M}_d(t)$
can fail for
$\overline {K}_{d,c}\cong \mathfrak {M}_d(t)$
can fail for
 $c>\frac {4-\sqrt {2}}{2d}$
. For instance, it was observed in [Reference Odaka, Spotti and Sun65, Example 5.8] that
$c>\frac {4-\sqrt {2}}{2d}$
. For instance, it was observed in [Reference Odaka, Spotti and Sun65, Example 5.8] that
![]() $\mathbb {P}(1,2,9)$
appears in the K-moduli space
$\mathbb {P}(1,2,9)$
appears in the K-moduli space
 $\overline {K}_{3,\frac {1}{2}}$
. We will further investigate the case
$\overline {K}_{3,\frac {1}{2}}$
. We will further investigate the case
![]() $d=3$
in a forthcoming work. It would also be interesting to consider more general divisors as well as other del Pezzo surfaces.
$d=3$
in a forthcoming work. It would also be interesting to consider more general divisors as well as other del Pezzo surfaces.
Remark 6.11. In a forthcoming work [Reference Ascher, DeVleming and Liu7], we give a complete description of wall crossing for K-moduli compactifications of
 $\left(\mathbb {P}^3, cS\right)$
, where
$\left(\mathbb {P}^3, cS\right)$
, where
![]() $S\subset \mathbb {P}^3$
is a smooth degree
$S\subset \mathbb {P}^3$
is a smooth degree
![]() $4$
K3 surface. As an application, we prove Laza and O’Grady’s conjecture [Reference Laza and O’Grady42, Reference Laza and O’Grady44] on birational models of moduli of degree
$4$
K3 surface. As an application, we prove Laza and O’Grady’s conjecture [Reference Laza and O’Grady42, Reference Laza and O’Grady44] on birational models of moduli of degree
![]() $4$
K3 surfaces. An essential ingredient is Theorem 1.1, which fully describes the wall-crossing behavior for K-moduli spaces of hyperelliptic quartic K3 surfaces.
$4$
K3 surfaces. An essential ingredient is Theorem 1.1, which fully describes the wall-crossing behavior for K-moduli spaces of hyperelliptic quartic K3 surfaces.
Acknowledgments
We would like to thank David Jensen, Radu Laza, Zhiyuan Li, Xiaowei Wang, and Chenyang Xu for helpful discussions. We also thank the referee for many valuable suggestions. This material is based upon work supported by the National Science Foundation under Grant DMS-1440140 while the authors were in residence at the Mathematical Sciences Research Institute in Berkeley, California, during the spring 2019 semester. The authors were supported in part by the American Institute of Mathematics as part of the AIM SQuaREs program. The first author was partially supported by an NSF Postdoctoral Fellowship and NSF Grant DMS-2001408. The second author was partially supported by the Gamelin Endowed Postdoctoral Fellowship of the MSRI. The third author was partially supported by the Della Pietra Endowed Postdoctoral Fellowship of the MSRI and NSF Grant DMS-2001317.
Competing Interest
None.