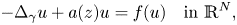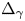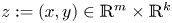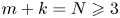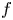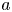1. Introduction
In this paper, we study the existence of nontrivial solutions for the following class of semilinear degenerate elliptic equations
where $\Delta _{\gamma }$![]() given by
given by
is known as the Grushin operator, $\Delta _x$![]() and $\Delta _y$
and $\Delta _y$![]() are the Laplace operators in the variables $x$
are the Laplace operators in the variables $x$![]() and $y$
and $y$![]() , respectively, with $z:=(x,y)\in \mathbb {R}^{m}\times \mathbb {R}^{k}$
, respectively, with $z:=(x,y)\in \mathbb {R}^{m}\times \mathbb {R}^{k}$![]() and $m+k=N\geqslant 3$
and $m+k=N\geqslant 3$![]() . Here, $\gamma \geqslant 0$
. Here, $\gamma \geqslant 0$![]() is a real number and $N_{\gamma }=m+(1+\gamma )k$
is a real number and $N_{\gamma }=m+(1+\gamma )k$![]() is the appropriate homogeneous dimension. The nonlinearity $f:\mathbb {R} \longrightarrow \mathbb {R}$
is the appropriate homogeneous dimension. The nonlinearity $f:\mathbb {R} \longrightarrow \mathbb {R}$![]() and the weight $a:\mathbb {R}^{N}\longrightarrow \mathbb {R}$
and the weight $a:\mathbb {R}^{N}\longrightarrow \mathbb {R}$![]() are continuous functions that satisfy some technical conditions.
are continuous functions that satisfy some technical conditions.
To begin with, we would like to observe that $\Delta _{\gamma }$![]() is elliptic for $x\in \mathbb {R}^{m}$
is elliptic for $x\in \mathbb {R}^{m}$![]() , $|x|\neq 0$
, $|x|\neq 0$![]() and degenerates on the manifold $\{0\}\times \mathbb {R}^{k}$
and degenerates on the manifold $\{0\}\times \mathbb {R}^{k}$![]() . This operator was studied by Grushin in [Reference Grushin20, Reference Grushin21], and it appears in the study of P.D.E. on manifolds. For example, in [Reference Kogoj and Lanconelli24, p. 4641] the authors showed that problem $(P)_a$
. This operator was studied by Grushin in [Reference Grushin20, Reference Grushin21], and it appears in the study of P.D.E. on manifolds. For example, in [Reference Kogoj and Lanconelli24, p. 4641] the authors showed that problem $(P)_a$![]() appears in the study of a P.D.E. in the Heisenberg group, while in [Reference Bhakta and Sandeep9] it was showed a strong relation with a P.D.E. on the Hyperbolic space. Furthermore, if $\gamma =0$
appears in the study of a P.D.E. in the Heisenberg group, while in [Reference Bhakta and Sandeep9] it was showed a strong relation with a P.D.E. on the Hyperbolic space. Furthermore, if $\gamma =0$![]() , the operator $\Delta _{0}$
, the operator $\Delta _{0}$![]() is the well-known classical Laplace operator. On the other hand, $\Delta _{\gamma }$
is the well-known classical Laplace operator. On the other hand, $\Delta _{\gamma }$![]() is contained in a family of operators of the kind
is contained in a family of operators of the kind

which was first introduced and studied by Franchi and Lanconelli [Reference Franchi and Lanconelli18, Reference Franchi and Lanconelli19]. For the interested read we cite for instance [Reference Anh and My5, Reference Kogoj and Lanconelli24–Reference Kogoj and Sonner26, Reference Luyen and Tri32, Reference Rahal and Hamdani37] and references therein.
In the last few years some authors have dedicated a special attention for problems involving the Grushin operator, see for example [Reference Chung14, Reference D'Ambrosio15, Reference Monti33, Reference Monti and Morbidelli34] and more recently [Reference Duong and Nguyen16, Reference Duong and Phan17, Reference Liu, Tang and Wang30, Reference Loiudice31].
The main motivation to study the above problem $(P)_a$![]() comes from of the papers due to [Reference Berestycki and Lions6, Reference Berestycki, Gallouet and Kavian7], that have considered the case $\gamma =0$
comes from of the papers due to [Reference Berestycki and Lions6, Reference Berestycki, Gallouet and Kavian7], that have considered the case $\gamma =0$![]() and $a(z)=1$
and $a(z)=1$![]() for all $z\in \mathbb {R}^{N}$
for all $z\in \mathbb {R}^{N}$![]() . More precisely, in [Reference Berestycki and Lions6], Berestycki and Lions have considered the existence of solution for
. More precisely, in [Reference Berestycki and Lions6], Berestycki and Lions have considered the existence of solution for
by assuming that $N \geqslant 3$![]() and the following conditions on $g$
and the following conditions on $g$![]() :
:
where $G(s)=\int _{0}^{s}g(t)\,{\rm d}t$![]() .
.
In [Reference Berestycki, Gallouet and Kavian7], Berestycki et al. have studied the case where $N=2$![]() and the nonlinearity $g$
and the nonlinearity $g$![]() possesses an exponential growth of the type
possesses an exponential growth of the type
In the two abovementioned papers, the authors obtained a solution for (1.2) by solving in the first moment a minimization problem, where the properties of the radially symmetric functions play an essential rule in their approach. After that, by using Lagrange multipliers, they were able to get a nontrivial solution for (1.2).
A version of the problem (1.2) for the critical case have been made in [Reference Alves, Montenegro and Souto2] for $N \geqslant 3$![]() and $N=2$
and $N=2$![]() , see also [Reference Zhang and Zou41] for $N=3$
, see also [Reference Zhang and Zou41] for $N=3$![]() . The reader can found in [Reference Alves, Figueiredo and Siciliano3, Reference Chang and Wang8, Reference Zhang, do Ó and Squassina42] the same type of results involving the fractional Laplacian operator, more precisely, for a problem like
. The reader can found in [Reference Alves, Figueiredo and Siciliano3, Reference Chang and Wang8, Reference Zhang, do Ó and Squassina42] the same type of results involving the fractional Laplacian operator, more precisely, for a problem like
with $\alpha \in (0,1)$![]() and $N \geqslant 1$
and $N \geqslant 1$![]() . For more details about this subject, we would like to cite the references found in the abovementioned papers.
. For more details about this subject, we would like to cite the references found in the abovementioned papers.
Recently, Alves et al. [Reference Alves, Duarte and Souto1] have proved an abstract theorem that was used to solve a large class of Berestycki–Lions type problems, which includes anisotropic operator, discontinuous nonlinearity, etc. In that paper, the authors have used the deformation lemma together with a notation of Pohozaev set to prove the abstract theorem.
Initially, in the present paper, we will deal with the existence of nontrivial solution for the problem $(P)_1$![]() , that is,
, that is,
for the positive mass case. In this case, the nonlinearity $f$![]() satisfies the following conditions:
satisfies the following conditions:
(f1) $f(s)/s = o(1)$
 as $s \to 0$
as $s \to 0$ ;
;(f2) There exists $q \in (2,2^{*}_{\gamma })$
 such that
\[ \limsup_{|s| \to +\infty}\frac{|f(s)|}{|s|^{q-1}} <{+}\infty, \quad \forall s \in \mathbb{R}, \]where $2^{*}_{\gamma }={2N_{\gamma }}/({N_{\gamma }-2})$
such that
\[ \limsup_{|s| \to +\infty}\frac{|f(s)|}{|s|^{q-1}} <{+}\infty, \quad \forall s \in \mathbb{R}, \]where $2^{*}_{\gamma }={2N_{\gamma }}/({N_{\gamma }-2})$
 ;
;(f3) There exists $s_0>0$
 such that $F(s_0)>\frac {1}{2}|s_0|^{2}$
such that $F(s_0)>\frac {1}{2}|s_0|^{2}$ , where $F(s) = \int _0^{s} f(t)\,{\rm d}t$
, where $F(s) = \int _0^{s} f(t)\,{\rm d}t$ .
.
Using the above assumptions we are ready to state our first result.
Theorem 1.1 (The positive mass case: $a(z)=1$ )
)
Assume $0 \leqslant \gamma < 1$![]() , $(f_1),(f_2)$
, $(f_1),(f_2)$![]() and $(f_3)$
and $(f_3)$![]() . Then problem $(P)_1$
. Then problem $(P)_1$![]() has at least one nonnegative nontrivial weak solution.
has at least one nonnegative nontrivial weak solution.
Our next result is associated with the zero mass case due to [Reference Berestycki and Lions6], more precisely, we intend to find a solution for the following class of problems
where $f$![]() satisfies some conditions that will be fixed later on.
satisfies some conditions that will be fixed later on.
Problems of this type have attracted the attention of many mathematicians with regards to the non-existence of a solution. For instance, if $\Delta _{H}$![]() is the Heisenberg Laplacian on $H^{N}=\mathbb {C}^{N}\times \mathbb {R}$
is the Heisenberg Laplacian on $H^{N}=\mathbb {C}^{N}\times \mathbb {R}$![]() and $u$
and $u$![]() is radial in the variable $x$
is radial in the variable $x$![]() , $u=u(|x|,y)$
, $u=u(|x|,y)$![]() , then $-\Delta _{H}u =\Delta _{x}u+4|x|^ 2\partial _{yy}u$
, then $-\Delta _{H}u =\Delta _{x}u+4|x|^ 2\partial _{yy}u$![]() is a Grushin operator with $\gamma =1$
is a Grushin operator with $\gamma =1$![]() , and so, $(P)_0$
, and so, $(P)_0$![]() is strongly related to equation
is strongly related to equation
In [Reference Birindelli and Prajapat10], Birindelli and Prajapat studied the nonexistence of cylindrical solution for above problem with $f(u)= u^{p}$![]() , $0< p<(Q+2)/(Q-2)$
, $0< p<(Q+2)/(Q-2)$![]() , where $Q$
, where $Q$![]() is the homogeneous dimension of $H^{N}$
is the homogeneous dimension of $H^{N}$![]() . Recently, Yu [Reference Yu39] studied the Liouville type theorem in the Heisenberg group for general nonlinearity. In [Reference Yu40], Yu studied the nonexistence of positive solutions for a general Grushin operator, and the main tool in this paper is the moving plane method. Monti and Morbidelli in [Reference Monti and Morbidelli34] investigated the uniqueness and symmetry of solutions for the problem $(P)_0$
. Recently, Yu [Reference Yu39] studied the Liouville type theorem in the Heisenberg group for general nonlinearity. In [Reference Yu40], Yu studied the nonexistence of positive solutions for a general Grushin operator, and the main tool in this paper is the moving plane method. Monti and Morbidelli in [Reference Monti and Morbidelli34] investigated the uniqueness and symmetry of solutions for the problem $(P)_0$![]() with critical growth and introduced a suitable Kelvin-type transform in this context. Finally, related to the bounded domains, Kogoj and Lanconelli [Reference Kogoj and Lanconelli24] showed regularity, existence and Pohozaev-type nonexistence results for the Dirichlet problem
with critical growth and introduced a suitable Kelvin-type transform in this context. Finally, related to the bounded domains, Kogoj and Lanconelli [Reference Kogoj and Lanconelli24] showed regularity, existence and Pohozaev-type nonexistence results for the Dirichlet problem
where $\Omega$![]() is a bounded open subset of $\mathbb {R}^{N}$
is a bounded open subset of $\mathbb {R}^{N}$![]() , for general nonlinearities $f$
, for general nonlinearities $f$![]() .
.
In order to solve $(P)_0$![]() , we are assuming the following conditions on function $f$
, we are assuming the following conditions on function $f$![]() :
:
(f4) There are $q >2^{*}_{\gamma }$
 and $c,\delta >0$
and $c,\delta >0$ such that
\[ |f(s)| \leqslant c|s|^{q-1}, \quad \mbox{for} \ |s| \leqslant \delta. \]
such that
\[ |f(s)| \leqslant c|s|^{q-1}, \quad \mbox{for} \ |s| \leqslant \delta. \]
(f5) $\displaystyle \lim _{|s| \to +\infty }\frac {f(s)}{|s|^{2^{*}_{\gamma }-1}}=0.$

(f6) There is $s_0>0$
 such that $F(s_0)>0$
such that $F(s_0)>0$ .
.
Theorem 1.2 (The zero mass case: $a(z)=0$ )
)
Assume $0 \leqslant \gamma < 1$![]() , $(f_4),(f_5)$
, $(f_4),(f_5)$![]() and $(f_6)$
and $(f_6)$![]() . Then problem $(P)_0$
. Then problem $(P)_0$![]() has at least one nonnegative nontrivial weak solution.
has at least one nonnegative nontrivial weak solution.
Related to a more general class of degenerate operators, in $2017$![]() , Chen et al. studied in [Reference Chen, Tang and Gao13] (see also [Reference Anh and My5, Reference Kogoj and Lanconelli24, Reference Luyen and Tri32, Reference Rahal and Hamdani37]) the following problem
, Chen et al. studied in [Reference Chen, Tang and Gao13] (see also [Reference Anh and My5, Reference Kogoj and Lanconelli24, Reference Luyen and Tri32, Reference Rahal and Hamdani37]) the following problem
where $\Omega$![]() is a bounded domain in $\mathbb {R}^{N}$
is a bounded domain in $\mathbb {R}^{N}$![]() , $\Delta _{\lambda }$
, $\Delta _{\lambda }$![]() -Laplace is given by (1.1), $a(z)$
-Laplace is given by (1.1), $a(z)$![]() is allowing to be sign-changing and $f$
is allowing to be sign-changing and $f$![]() is a function with a more general super-quadratic growth.
is a function with a more general super-quadratic growth.
If we suppose that $f$![]() satisfies the well-known Ambrosetti–Rabinowitz superlinear condition, that is, there exists $\theta > 2$
satisfies the well-known Ambrosetti–Rabinowitz superlinear condition, that is, there exists $\theta > 2$![]() such that
such that
we are able to establish the existence of nontrivial solution for the problem $(P)_a$![]() for all $\gamma \geqslant 0$
for all $\gamma \geqslant 0$![]() by supposing that
by supposing that
and
In addition to the above conditions, we will consider one of the following conditions:
The function $a$![]() is periodic on variable $y$
is periodic on variable $y$![]() , that is,
, that is,
or the function $a$![]() is coercive on variable $y$
is coercive on variable $y$![]() , that is,
, that is,
Our main result involving the above conditions is the following:
Theorem 1.3 (Nonconstant case)
Assume $\gamma \geqslant 0$![]() , $(f_1),(f_2)$
, $(f_1),(f_2)$![]() and $(f_7)$
and $(f_7)$![]() and let $a:\mathbb {R}^{N}\longrightarrow \mathbb {R}$
and let $a:\mathbb {R}^{N}\longrightarrow \mathbb {R}$![]() be a continuous function satisfying (A1) and (A2). If (A3) or (A4) holds, then problem $(P)_a$
be a continuous function satisfying (A1) and (A2). If (A3) or (A4) holds, then problem $(P)_a$![]() has at least one nonnegative nontrivial weak solution.
has at least one nonnegative nontrivial weak solution.
Theorem 1.3 complements the study made in [Reference Coti Zelati and Rabinowitz12, Reference Kryszewski and Szulkin27, Reference Rabinowitz36], because in those papers it was considered the case $\gamma =0$![]() and $a$
and $a$![]() being a periodic function.
being a periodic function.
Our last result is associated with the behaviour of the solutions at infinite.
Theorem 1.4 Let $u$![]() be a solution of problem $(P)_a$
be a solution of problem $(P)_a$![]() with $a \not =0$
with $a \not =0$![]() . Then $u\in L^{\infty }(\mathbb {R}^{N})$
. Then $u\in L^{\infty }(\mathbb {R}^{N})$![]() and $\lim _{|z|\to \infty }u(z)=0.$
and $\lim _{|z|\to \infty }u(z)=0.$![]()
In what follows, we say that a function $u\in \mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$![]() is a weak solution to problem $(P)_a$
is a weak solution to problem $(P)_a$![]() , with $a\neq 0$
, with $a\neq 0$![]() , if $u\geqslant 0$
, if $u\geqslant 0$![]() a.e. in $\mathbb {R}^{N}$
a.e. in $\mathbb {R}^{N}$![]() , $u \not \equiv 0$
, $u \not \equiv 0$![]() and
and
Similarly, $u\in \mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() is a weak solution to problem $(P)_0$
is a weak solution to problem $(P)_0$![]() if (1.3) holds for all $\varphi \in \mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$
if (1.3) holds for all $\varphi \in \mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() . The spaces $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
. The spaces $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() , $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$
, $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$![]() , $\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$
, $\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() and the notation $\nabla _{\gamma }$
and the notation $\nabla _{\gamma }$![]() are defined in § 2.
are defined in § 2.
Before concluding this section, we would like to point out that in the proof of theorems 1.1, 1.2 and 1.3 we have found some difficulties to apply variational methods when $\gamma >0$![]() , because different from the case $\gamma =0$
, because different from the case $\gamma =0$![]() , the energy functional associated with problem $(P)_a$
, the energy functional associated with problem $(P)_a$![]() is not invariant on the group $O(N)$
is not invariant on the group $O(N)$![]() and it is also not invariant by for some types of translations. These facts bring to the problem some technical difficulties that must be analysed in a very cautious way. Having this in mind, in § 2, we made a careful study about the space functions that will be used in this paper, which includes compactness results and a version of a result due to [Reference Lions29, lemma I.1] for the space $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
and it is also not invariant by for some types of translations. These facts bring to the problem some technical difficulties that must be analysed in a very cautious way. Having this in mind, in § 2, we made a careful study about the space functions that will be used in this paper, which includes compactness results and a version of a result due to [Reference Lions29, lemma I.1] for the space $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() .
.
Throughout the paper, unless explicitly stated, the symbol ${C}$![]() will always denote a generic positive constant, which may vary from line to line. The usual norm in $L^{p}(\mathbb {R}^{N})$
will always denote a generic positive constant, which may vary from line to line. The usual norm in $L^{p}(\mathbb {R}^{N})$![]() will be denoted by $|\cdot |_{p}$
will be denoted by $|\cdot |_{p}$![]() and $H^{1}(\Omega )$
and $H^{1}(\Omega )$![]() will be used to denote standard Sobolev spaces in $\Omega \subset \mathbb {R}^{N}$
will be used to denote standard Sobolev spaces in $\Omega \subset \mathbb {R}^{N}$![]() .
.
2. Function spaces
In this section, we will introduce some notations and preliminary results that will be used later on. First of all, let $\gamma$![]() be a nonnegative real number and $z=(x,y)=(x_1,\ldots,x_m,y_1,\ldots,y_k)\in \mathbb {R}^{m}\times \mathbb {R}^{k}$
be a nonnegative real number and $z=(x,y)=(x_1,\ldots,x_m,y_1,\ldots,y_k)\in \mathbb {R}^{m}\times \mathbb {R}^{k}$![]() with $m, k\geqslant 1$
with $m, k\geqslant 1$![]() and $m+k=N\geqslant 3$
and $m+k=N\geqslant 3$![]() . In what follows, we designate by $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
. In what follows, we designate by $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() the weighted Sobolev space
the weighted Sobolev space
For simplicity, if $u\in \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() , we denote by $\nabla _{\gamma }$
, we denote by $\nabla _{\gamma }$![]() the gradient operator defined by
the gradient operator defined by
and so,
We would like to mention that $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() is a Hilbert space, when endowed with the scalar product given by
is a Hilbert space, when endowed with the scalar product given by
whose the corresponding norm is

By Sobolev inequality found in [Reference Monti33], we have

from where it follows that the embedding $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N}) \hookrightarrow L^{2_{\gamma }^{*}}(\mathbb {R}^{N})$![]() is continuous, then there is $\mathcal {C}>0$
is continuous, then there is $\mathcal {C}>0$![]() such that
such that
Since
the interpolation inequality on the Lebesgue space ensures that the embedding $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N}) \hookrightarrow L^{p}(\mathbb {R}^{N})$![]() is continuous for every $p\in [2, 2^{*}_{\gamma }]$
is continuous for every $p\in [2, 2^{*}_{\gamma }]$![]() . Thus, for each $p \in [2, 2^{*}_{\gamma }]$
. Thus, for each $p \in [2, 2^{*}_{\gamma }]$![]() there is $\mathcal {C}_p>0$
there is $\mathcal {C}_p>0$![]() such that
such that
Here, we would like point out that if $\Omega _1 \subset \mathbb {R}^{m}$![]() and $\Omega _2 \subset \mathbb {R}^{k}$
and $\Omega _2 \subset \mathbb {R}^{k}$![]() are smooth bounded domains, the embeddings (2.2) still hold by replacing $\mathbb {R}^{N}$
are smooth bounded domains, the embeddings (2.2) still hold by replacing $\mathbb {R}^{N}$![]() for any set of the form $\Omega =\mathbb {R}^{m} \times \Omega _2$
for any set of the form $\Omega =\mathbb {R}^{m} \times \Omega _2$![]() , $\Omega =\Omega _1 \times \mathbb {R}^{k}$
, $\Omega =\Omega _1 \times \mathbb {R}^{k}$![]() or $\Omega =\Omega _1 \times \Omega _2$
or $\Omega =\Omega _1 \times \Omega _2$![]() , that is,
, that is,
where
and

The proof of (2.3) follows by showing the existence of an extension operator that can be done as in [Reference Brézis11, § 9.2]. Moreover, when $\Omega =\Omega _1 \times \Omega _2 \subset \mathbb {R}^{N}=\mathbb {R}^{m} \times \mathbb {R}^{k}$![]() the embedding $\mathcal {H}_{\gamma }^{1,2}(\Omega ) \hookrightarrow L^{p}(\Omega )$
the embedding $\mathcal {H}_{\gamma }^{1,2}(\Omega ) \hookrightarrow L^{p}(\Omega )$![]() is compact for every $p\in [1, 2^{*}_{\gamma })$
is compact for every $p\in [1, 2^{*}_{\gamma })$![]() , which is a consequence of the Strauss's compactness lemma [Reference Berestycki and Lions6, theorem A.I].
, which is a consequence of the Strauss's compactness lemma [Reference Berestycki and Lions6, theorem A.I].
Setting the space
it is easy to see that condition (A1) implies that $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$![]() is Hilbert space with scalar product and norm given by
is Hilbert space with scalar product and norm given by
Moreover, (A1) also yields $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$![]() is embedded continuously into $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
is embedded continuously into $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() . Hence, by (2.2), $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$
. Hence, by (2.2), $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$![]() is embedded continuously in $L^{p}(\mathbb {R}^{N})$
is embedded continuously in $L^{p}(\mathbb {R}^{N})$![]() for every $p\in [2, 2^{*}_{\gamma }]$
for every $p\in [2, 2^{*}_{\gamma }]$![]() .
.
Another important space that will consider in the present paper is $\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() , which is the closure of $C_{0}^{\infty }(\mathbb {R}^{N})$
, which is the closure of $C_{0}^{\infty }(\mathbb {R}^{N})$![]() with relation to the norm
with relation to the norm

From (2.1) we derive that the embedding $\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N}) \hookrightarrow L^{2^{*}_{\gamma }}(\mathbb {R}^{N})$![]() is continuous, that is, there is $\mathcal {S}>0$
is continuous, that is, there is $\mathcal {S}>0$![]() such that
such that
2.1 Compactness results
In this section, we will prove some important compactness results that will be used in the proof of theorems 1.1, 1.2 and 1.3. Our first result is a version of a famous result due to [Reference Lions29, lemma I.1] for the space $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() .
.
Lemma 2.1 Let $r>0, 2\leqslant q < 2^{*}_{\gamma }$![]() and $(u_n) \subset \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
and $(u_n) \subset \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() be a bounded sequence with
be a bounded sequence with
where $B_r^{k}(y)=\{\xi \in \mathbb {R}^{k}\,:\,|\xi - y|< r\}.$![]() Then, $u_n\longrightarrow 0$
Then, $u_n\longrightarrow 0$![]() in $L^{p}(\mathbb {R}^{N})$
in $L^{p}(\mathbb {R}^{N})$![]() for $2< p<2^{*}_{\gamma }$
for $2< p<2^{*}_{\gamma }$![]() .
.
Proof. As a first step we consider
in such way that each point of $\mathbb {R}^{k}$![]() is contained in a finite quantity $l$
is contained in a finite quantity $l$![]() of balls of radius $r$
of balls of radius $r$![]() . Now, let $q< s<2^{*}_{\gamma }$
. Now, let $q< s<2^{*}_{\gamma }$![]() and $\alpha :={(s-q)2^{*}_{\gamma }}/{(2^{*}_{\gamma }-q)s}$
and $\alpha :={(s-q)2^{*}_{\gamma }}/{(2^{*}_{\gamma }-q)s}$![]() . Then, if $u\in \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
. Then, if $u\in \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() , the embedding (2.3) together with the interpolation inequality on the Lebesgue space says that
, the embedding (2.3) together with the interpolation inequality on the Lebesgue space says that

Choosing $\alpha =2/s$![]() , we obtain the inequality
, we obtain the inequality
which leads to

As $(u_n)$![]() is bounded sequence in $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
is bounded sequence in $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() the last inequality allows us to conclude that $u_n\to 0$
the last inequality allows us to conclude that $u_n\to 0$![]() in $L^{s}(\mathbb {R}^{N})$
in $L^{s}(\mathbb {R}^{N})$![]() . Now, applying again the interpolation inequality on the Lebesgue spaces we conclude that $u_n \to 0$
. Now, applying again the interpolation inequality on the Lebesgue spaces we conclude that $u_n \to 0$![]() in $L^{p}(\mathbb {R}^{N})$
in $L^{p}(\mathbb {R}^{N})$![]() for $2< p<2^{*}_{\gamma }$
for $2< p<2^{*}_{\gamma }$![]() .
.
Next, we will show some compactness results that are crucial in our approach. Have this in mind, we will fix the following spaces:

and
Lemma 2.2 Let $p\in (2,2^{*}_{\gamma })$![]() and $r>0$
and $r>0$![]() . Then, the embedding of $\mathcal {H}_{\gamma,rad_x}^{1,2}(\mathbb {R}^{m}\times B_r(0))$
. Then, the embedding of $\mathcal {H}_{\gamma,rad_x}^{1,2}(\mathbb {R}^{m}\times B_r(0))$![]() into $L^{p}(\mathbb {R}^{m}\times B_r(0))$
into $L^{p}(\mathbb {R}^{m}\times B_r(0))$![]() is compact.
is compact.
Proof. It is enough to show that if $(u_n)$![]() is a sequence in $\mathcal {H}_{\gamma,rad_x}^{1,2}(\mathbb {R}^{m}\times B_r(0))$
is a sequence in $\mathcal {H}_{\gamma,rad_x}^{1,2}(\mathbb {R}^{m}\times B_r(0))$![]() such that $u_n \rightharpoonup 0$
such that $u_n \rightharpoonup 0$![]() in $\mathcal {H}_{\gamma,rad_x}^{1,2}(\mathbb {R}^{m}\times B_r(0))$
in $\mathcal {H}_{\gamma,rad_x}^{1,2}(\mathbb {R}^{m}\times B_r(0))$![]() , then $u_n\to 0$
, then $u_n\to 0$![]() in $L^{p}(\mathbb {R}^{m}\times B_r(0))$
in $L^{p}(\mathbb {R}^{m}\times B_r(0))$![]() . Let $\delta >0$
. Let $\delta >0$![]() and $h \in C^{\infty }(\mathbb {R},[0,1])$
and $h \in C^{\infty }(\mathbb {R},[0,1])$![]() defined by
defined by
Notice that if $u\in \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{m}\times B_r(0))$![]() and
and
then $|v|^{2}\leqslant |u|^{2}$![]() ,
,
and
Hence,

Therefore, $v\in H^{1}(\mathbb {R}^{m}\times B_r(0))$![]() and
and
Thereby, setting $v_n(x,y)=h(|x|^{2})u_n(x,y)$![]() we have $(v_n)$
we have $(v_n)$![]() is a bounded sequence in $H_{rad,x}^{1}(\mathbb {R}^{m}\times B_r(0))$
is a bounded sequence in $H_{rad,x}^{1}(\mathbb {R}^{m}\times B_r(0))$![]() and $v_n \rightharpoonup 0$
and $v_n \rightharpoonup 0$![]() in $H_{rad,x}^{1}(\mathbb {R}^{m}\times B_r(0))$
in $H_{rad,x}^{1}(\mathbb {R}^{m}\times B_r(0))$![]() . By [Reference Lions28, lemme III.2],
. By [Reference Lions28, lemme III.2],
Since $v_n(x,y)=u_n(x,y)$![]() for $(x,y) \in [|x| \geqslant \delta ] \times B_r(0)$
for $(x,y) \in [|x| \geqslant \delta ] \times B_r(0)$![]() , we derive that
, we derive that
Furthermore, recalling that if $\Omega =B_\delta (0) \times B_r(0) \subset \mathbb {R}^{N}$![]() the embedding $\mathcal {H}_{\gamma }^{1,2}(\Omega )$
the embedding $\mathcal {H}_{\gamma }^{1,2}(\Omega )$![]() into $L^{p}(\Omega )$
into $L^{p}(\Omega )$![]() is compact for $1\leqslant p<2_{\gamma }^{*}$
is compact for $1\leqslant p<2_{\gamma }^{*}$![]() , then
, then
Accordingly to (2.4) and (2.5), $u_n\to 0$![]() in $L^{p}(\mathbb {R}^{m}\times B_r(0))$
in $L^{p}(\mathbb {R}^{m}\times B_r(0))$![]() with $p\in (2,2^{*}_{\gamma })$
with $p\in (2,2^{*}_{\gamma })$![]() .
.
Lemma 2.3 Let $p\in (2,2^{*}_{\gamma })$![]() . Then the embedding of $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}) \hookrightarrow L^{p}(\mathbb {R}^{N})$
. Then the embedding of $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}) \hookrightarrow L^{p}(\mathbb {R}^{N})$![]() is compact.
is compact.
Proof. In what follows, we set $q \in (2,2^{*}_{\gamma })$![]() and
and
From definition of $k(y,r,O(k))$![]() ,
,
A simple computation gives that for each $u \in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() ,
,

Arguing as in the last lemma, let $(u_n)$![]() be a sequence in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
be a sequence in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() such that $u_n \rightharpoonup 0$
such that $u_n \rightharpoonup 0$![]() in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() . As $(u_n)$
. As $(u_n)$![]() is a bounded sequence in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
is a bounded sequence in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , given $\epsilon >0$
, given $\epsilon >0$![]() , the definition of $k(y,r,O(k))$
, the definition of $k(y,r,O(k))$![]() ensures that there is $R>0$
ensures that there is $R>0$![]() such that
such that
On the other hand, lemma 2.2 yields
and so,
Now the lemma follows by employing lemma 2.1.
Corollary 2.4 If (A1) holds, then the embedding $\mathcal {H}^{a}_{\gamma,rad_{x,y}}(\mathbb {R}^{N}) \hookrightarrow L^{p}(\mathbb {R}^{N})$![]() is compact.
is compact.
Proof. The corollary is an immediate consequence of the last lemma, because we have the continuous embedding of $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$![]() into $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
into $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() .
.
Lemma 2.5 Let $(u_n) \subset \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() be a bounded sequence with $u_n \rightharpoonup 0$
be a bounded sequence with $u_n \rightharpoonup 0$![]() in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() . Then, for each $0< a< b<+\infty$
. Then, for each $0< a< b<+\infty$![]() and $p \in (2,2^{*}_{\gamma })$
and $p \in (2,2^{*}_{\gamma })$![]() ,
,
Proof. In the sequel, let us consider $g \in C^{\infty }(\mathbb {R},\mathbb {R})$![]() satisfying
satisfying
and
Using the function $g$![]() , we set the sequence $v_n(x,y)=g(u_n(x,y))$
, we set the sequence $v_n(x,y)=g(u_n(x,y))$![]() . A direct computation shows that $v_n \in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
. A direct computation shows that $v_n \in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() and that it is a bounded sequence in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
and that it is a bounded sequence in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() . Thereby, by lemma 2.3, $v_n \to 0$
. Thereby, by lemma 2.3, $v_n \to 0$![]() in $L^{p}(\mathbb {R}^{N})$
in $L^{p}(\mathbb {R}^{N})$![]() , that is,
, that is,
and so,
Using the fact that $g(t)=t$![]() for $a \leqslant |t| \leqslant b$
for $a \leqslant |t| \leqslant b$![]() , it follows that
, it follows that
showing the lemma.
Lemma 2.6 Assume that (A1) and (A4) hold. Then the embedding of $\mathcal {H}_{\gamma,rad_x}^{a}(\mathbb {R}^{N}) \hookrightarrow L^{p}(\mathbb {R}^{N})$![]() is compact for all $p\in [2,2^{*}_{\gamma })$
is compact for all $p\in [2,2^{*}_{\gamma })$![]() .
.
Proof. It suffices to show that for any sequence $(u_n) \subset \mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() with $u_n \rightharpoonup 0$
with $u_n \rightharpoonup 0$![]() in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() , we must have $u_n\to 0$
, we must have $u_n\to 0$![]() in $L^{p}(\mathbb {R}^{N})$
in $L^{p}(\mathbb {R}^{N})$![]() . By (A4), given $\varepsilon >0$
. By (A4), given $\varepsilon >0$![]() , we can take $R_{\varepsilon }>0$
, we can take $R_{\varepsilon }>0$![]() such that
such that
From this,
where $M=\sup _{n}||u_n||^{2}_a$![]() .
.
Notice that (A1) and (2.3) imply that the space $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{m}\times [|y|\geqslant R_{\varepsilon }])$![]() is continuously immersed in $L^{2^{*}_{\gamma }}(\mathbb {R}^{m}\times [|y|\geqslant R_{\varepsilon }])$
is continuously immersed in $L^{2^{*}_{\gamma }}(\mathbb {R}^{m}\times [|y|\geqslant R_{\varepsilon }])$![]() . Then the last inequality together with the interpolation inequality ensures that for each $p\in (2, 2^{*}_{\gamma })$
. Then the last inequality together with the interpolation inequality ensures that for each $p\in (2, 2^{*}_{\gamma })$![]() ,
,
where $\alpha ={(p-2)2^{*}_{\gamma }}/{(2^{*}_{\gamma }-2)p}$![]() . Once again by (A1) and lemma 2.2, it is easy to conclude that the embedding of $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{m}\times B_{R_{\varepsilon }}(0))$
. Once again by (A1) and lemma 2.2, it is easy to conclude that the embedding of $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{m}\times B_{R_{\varepsilon }}(0))$![]() into $L^{p}(\mathbb {R}^{m}\times B_{R_{\varepsilon }}(0))$
into $L^{p}(\mathbb {R}^{m}\times B_{R_{\varepsilon }}(0))$![]() is compact for all $p\in [2,2^{*}_{\gamma })$
is compact for all $p\in [2,2^{*}_{\gamma })$![]() , and so, $u_n\to 0$
, and so, $u_n\to 0$![]() in $L^{p}(\mathbb {R}^{m}\times B_{R_{\varepsilon }}(0))$
in $L^{p}(\mathbb {R}^{m}\times B_{R_{\varepsilon }}(0))$![]() . This proves the desired result.
. This proves the desired result.
3. Proof of theorem 1.1
From now on, since we intend to find nonnegative solutions, without loss of generality we assume that
The energy functional associated with problem $(P)_1$![]() is the functional $I:\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})\longrightarrow \mathbb {R}$
is the functional $I:\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})\longrightarrow \mathbb {R}$![]() given by
given by
The reader is invited to see that weak solutions of problem $(P)_1$![]() are critical points of $I$
are critical points of $I$![]() .
.
In order to overcome the loss of compactness involving the space $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() , we will restrict to functional $I$
, we will restrict to functional $I$![]() to the space $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
to the space $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , and in this sense, an important tool in our approach is the Principle of Symmetric Criticality due to Palais (see [Reference Willem38, theorem 1.28]) that will be used to obtain solutions on the whole space $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
, and in this sense, an important tool in our approach is the Principle of Symmetric Criticality due to Palais (see [Reference Willem38, theorem 1.28]) that will be used to obtain solutions on the whole space $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() .
.
Our first lemma establishes that $I$![]() satisfies the mountain pass geometry.
satisfies the mountain pass geometry.
Lemma 3.1 There exist positive numbers $\rho$![]() and $\alpha$
and $\alpha$![]() such that:
such that:
(i) $I(u)\geqslant \alpha$
 if $||u||_{\gamma }=\rho$
if $||u||_{\gamma }=\rho$ .
.(ii) There exists $v\in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
 such that $||v||_{\gamma }>\rho$
such that $||v||_{\gamma }>\rho$ and $I(v)< 0$
and $I(v)< 0$ .
.
Proof. (i) From $(f_1)$![]() and $(f_2)$
and $(f_2)$![]() , there is a constant $C>0$
, there is a constant $C>0$![]() such that
such that
Hence,
Since the embedding $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}) \hookrightarrow L^{q}(\mathbb {R}^{N})$![]() is continuous,
is continuous,
and so,
Now, if $||u||_{\gamma }=\rho$![]() is small enough, it follows that
is small enough, it follows that
(ii) By $(f_3)$![]() there is a nonnegative function $\varphi \in C_0^{\infty }(\mathbb {R}^{N}) \cap \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}) \setminus \{0\}$
there is a nonnegative function $\varphi \in C_0^{\infty }(\mathbb {R}^{N}) \cap \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}) \setminus \{0\}$![]() satisfying
satisfying
By using the function $\varphi$![]() above, for each $t>0$
above, for each $t>0$![]() we fix the function
we fix the function
A direct computation leads to

Now, the fact that $0\leqslant \gamma <1$![]() combines with (3.1) to give
combines with (3.1) to give
Therefore, the result follows by fixing $v=\varphi _t$![]() for $t$
for $t$![]() large enough.
large enough.
The last lemma permits to conclude that the mountain pass level given by
where
is well defined.
Since we are not assuming in this section the Ambrosetti–Rabinowitz condition, we will adapt for our problem the same approach explored in [Reference Jeanjean23], which was also used in [Reference Hirata, Ikoma and Tanaka22].
Hereafter, let us consider an auxiliary functional $\tilde {I}:\mathbb {R} \times \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}) \to \mathbb {R}$![]() defined by
defined by
that is,
It is easy to check that
In what follows, we equip the space $\mathbb {R} \times \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() with the standard product norm $\|(s,u)\|_{\mathbb {R} \times \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})}=(|s|^{2}+\|u\|_{\gamma }^{2})^{{1}/{2}}$
with the standard product norm $\|(s,u)\|_{\mathbb {R} \times \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})}=(|s|^{2}+\|u\|_{\gamma }^{2})^{{1}/{2}}$![]() .
.
Arguing of the same way as in lemma 3.1, it is easy to see that $\tilde {I}$![]() also satisfies the mountain pass geometry, more precisely, we have the result below:
also satisfies the mountain pass geometry, more precisely, we have the result below:
Lemma 3.2 There exist positive numbers $\rho$![]() and $\alpha$
and $\alpha$![]() such that:
such that:
(i) $\tilde {I}(s,u)\geqslant \alpha$
 if $\|(s,u)\|_{\mathbb {R} \times \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})}=\rho$
if $\|(s,u)\|_{\mathbb {R} \times \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})}=\rho$ .
.(ii) There exists $v\in \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
 such that $\|(0,v)\|_{\mathbb {R} \times \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})}=\|v\|>\rho$
such that $\|(0,v)\|_{\mathbb {R} \times \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})}=\|v\|>\rho$ and $\tilde {I}(0,v)=I(v)< 0$
and $\tilde {I}(0,v)=I(v)< 0$ .
.
From now on, we denote by $\tilde {d}$![]() , the mountain pass level associated with $\tilde {I}$
, the mountain pass level associated with $\tilde {I}$![]() given by
given by
where
From definition of $I$![]() and $\tilde {I}$
and $\tilde {I}$![]() , we derive the equality $d=\tilde {d}$
, we derive the equality $d=\tilde {d}$![]() , which permits to repeat the same arguments explored in the proof of [Reference Hirata, Ikoma and Tanaka22, propostion 4.2] (see also [Reference Jeanjean23, propostion 2.2]) to show the following proposition:
, which permits to repeat the same arguments explored in the proof of [Reference Hirata, Ikoma and Tanaka22, propostion 4.2] (see also [Reference Jeanjean23, propostion 2.2]) to show the following proposition:
Proposition 3.3 There is a sequence $\{(s_n,u_n)\} \subset \mathbb {R} \times \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() such that
such that
(a) $s_n \to 0$
 ;
;(b) $\tilde {I}(s_n,u_n) \to \tilde {d}$
 ;
;(c) $({\partial }/{\partial u})\tilde {I}(s_n,u_n)\to 0$
 strongly in $(\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}))^{*}$
strongly in $(\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}))^{*}$ ;
;(d) ${\partial }/{\partial s}\tilde {I}(s_n,u_n)\to 0$
 in $\mathbb {R}$
in $\mathbb {R}$ .
.
The next lemma establishes the boundedness of the sequence $(u_n) \subset \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() that was obtained in the last proposition.
that was obtained in the last proposition.
Lemma 3.4 The sequence $(u_n) \subset \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() is bounded.
is bounded.
Proof. From proposition 3.3,

The first and second equation of the previous system ensure that $(\|u_n\|_{\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})})$![]() is bounded. From $(f_1)-(f_2)$
is bounded. From $(f_1)-(f_2)$![]() , there is $C>0$
, there is $C>0$![]() such that
such that
Using this information in the last equation of the above system, we get
Now, employing (2.1) we arrive at
from where it follows that $(u_n)$![]() is a bounded sequence in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
is a bounded sequence in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() .
.
3.1 Proof of theorem 1.1
By proposition 3.3 there is $\{(s_n,u_n)\} \subset \mathbb {R} \times \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() satisfying
satisfying
(a) $s_n \to 0;$

(b) $\tilde {I}(s_n,u_n) \to \tilde {d};$

(c) $({\partial }/{\partial u})\tilde {I}(s_n,u_n)\to 0$
 strongly in $(\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}))^{*}$
strongly in $(\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}))^{*}$ ;
;(d) $({\partial }/{\partial s})\tilde {I}(s_n,u_n)\to 0$
 in $\mathbb {R}$
in $\mathbb {R}$ .
.
By lemma 3.4 the sequence $(u_n)$![]() is bounded in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
is bounded in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , then for some subsequence, still denoted by itself, there is $u_0 \in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
, then for some subsequence, still denoted by itself, there is $u_0 \in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() such that
such that
The item $(d)$![]() implies that $({\partial }/{\partial u})\tilde {I}(s_n,u_n)w\to 0$
implies that $({\partial }/{\partial u})\tilde {I}(s_n,u_n)w\to 0$![]() for all $w \in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
for all $w \in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , that is,
, that is,

Since $s_n \to 0$![]() , it is easy to check that the above equality leads to
, it is easy to check that the above equality leads to
showing that $I'(u_0)w=0$![]() for all $w \in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
for all $w \in \mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , that is, $u_0$
, that is, $u_0$![]() is a critical point of $I$
is a critical point of $I$![]() . We claim that $u_0 \not = 0$
. We claim that $u_0 \not = 0$![]() , because if $u_0=0$
, because if $u_0=0$![]() the lemma 2.3 gives that
the lemma 2.3 gives that
From $(f_1)-(f_2)$![]() , given $\epsilon >0$
, given $\epsilon >0$![]() , there is $C>0$
, there is $C>0$![]() such that
such that
As $(u_n)$![]() is bounded in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
is bounded in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , it follows that
, it follows that
where $M=\sup _{n \in \mathbb {N}} \int _{\mathbb {R}^{N}}|u_n|^{2}\,{\rm d}z$![]() . Now, using the limit (3.2) we derive that
. Now, using the limit (3.2) we derive that
As $\epsilon$![]() is arbitrary, the last limit yields
is arbitrary, the last limit yields
This limit combined together with the limit $({\partial }/{\partial u})\tilde {I}(s_n,u_n)u_n=o_n(1)$![]() allows to deduce that $u_n \to 0$
allows to deduce that $u_n \to 0$![]() in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() . Hence,
. Hence,
which is absurd, because $\tilde {I}(s_n,u_n) \to \tilde {d}>0$![]() . Thus $u_0$
. Thus $u_0$![]() is a nontrivial critical point of $I$
is a nontrivial critical point of $I$![]() in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() .
.
Next, we will show that $u_0$![]() is in fact a critical point of $I$
is in fact a critical point of $I$![]() in the space $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
in the space $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() . To see why, we will apply the Principle of Symmetric Criticality [Reference Palais35] (see also [Reference Willem38, theorem 1.28]). In what follows, let us denote by $G$
. To see why, we will apply the Principle of Symmetric Criticality [Reference Palais35] (see also [Reference Willem38, theorem 1.28]). In what follows, let us denote by $G$![]() the following subgroup of $O(N)$
the following subgroup of $O(N)$![]() :
:
and the action $G\times \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N}) \longrightarrow \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() is given by
is given by
which is isometric, that is, $\|gu\|_{\gamma }=\|u\|_{\gamma }$![]() . Furthermore, the functional $I$
. Furthermore, the functional $I$![]() is invariant under the action of $G$
is invariant under the action of $G$![]() , since for all $g\in G$
, since for all $g\in G$![]() and $u \in \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
and $u \in \mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() ,
,
From the above commentaries, it is easy to check that
Hence, by the Principle of Symmetric Criticality of Palais, $u_0$![]() is a nontrivial critical point of $I$
is a nontrivial critical point of $I$![]() in $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() , and consequently $u_0$
, and consequently $u_0$![]() is the required nontrivial solution of $(P)_1$
is the required nontrivial solution of $(P)_1$![]() . Finally, we can see that $u_0$
. Finally, we can see that $u_0$![]() is nonnegative, because it satisfies
is nonnegative, because it satisfies
Then, choosing the test function $\varphi =u_0^{-}:=\min \{u,0\}$![]() , we find that $||u_0^{-}||_{\gamma }=0$
, we find that $||u_0^{-}||_{\gamma }=0$![]() . This proves that $u_0$
. This proves that $u_0$![]() is nonnegative.
is nonnegative.
4. Proof of theorem 1.2
In what follows, as in the previous section, without loss of generality we assume that
The energy functional associated with problem $(P)_0$![]() is the functional $J:\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})\longrightarrow \mathbb {R}$
is the functional $J:\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})\longrightarrow \mathbb {R}$![]() given by
given by
The reader is invited to see that weak solutions of problem $(P)_0$![]() are critical points of $I$
are critical points of $I$![]() .
.
In order to overcome the loss of compactness involving the space $\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() , we will restrict to functional $J$
, we will restrict to functional $J$![]() to the space $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
to the space $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() . In this section, $\|\,\,\|$
. In this section, $\|\,\,\|$![]() denotes the norm $\|\,\,\|_{\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})}$
denotes the norm $\|\,\,\|_{\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})}$![]() .
.
Our first lemma shows that $J$![]() verifies the mountain pass geometry. However, we will omit its proof because the arguments are similar those used in the proof of lemma 3.1.
verifies the mountain pass geometry. However, we will omit its proof because the arguments are similar those used in the proof of lemma 3.1.
Lemma 4.1 There exist positive numbers $\rho$![]() and $\alpha$
and $\alpha$![]() such that:
such that:
(i) $J(u)\geqslant \alpha$
 if $||u||=\rho$
if $||u||=\rho$ .
.(ii) There exists $v\in \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
 such that $\|v\|>\rho$
such that $\|v\|>\rho$ and $J(v)< 0$
and $J(v)< 0$ .
.
The last lemma permits to conclude that the mountain pass level given by
where
is well defined.
Arguing as in § 3, we will consider an auxiliary functional $\tilde {J}:\mathbb {R} \times \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}) \to \mathbb {R}$![]() given by
given by
that is,
It is easy to prove that $\tilde {J}$![]() also satisfies the mountain pass geometry, more precisely we have the result below
also satisfies the mountain pass geometry, more precisely we have the result below
Lemma 4.2 There exist positive numbers $\rho$![]() and $\alpha$
and $\alpha$![]() such that:
such that:
(i) $\tilde {J}(s,u)\geqslant \alpha$
 if $\|(s,u)\|_{\mathbb {R} \times \mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})}=\rho$
if $\|(s,u)\|_{\mathbb {R} \times \mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})}=\rho$ .
.(ii) There exists $v\in \mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$
 such that $\|(0,v)\|_{\mathbb {R} \times \mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})}=\|v\|>\rho$
such that $\|(0,v)\|_{\mathbb {R} \times \mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})}=\|v\|>\rho$ and $\tilde {J}(0,v)=J(v)< 0$
and $\tilde {J}(0,v)=J(v)< 0$ .
.
From now on, we denote by $\tilde {d}_0$![]() , the mountain pass level associated with $\tilde {J}$
, the mountain pass level associated with $\tilde {J}$![]() given by
given by
where
From definition of $J$![]() and $\tilde {J}$
and $\tilde {J}$![]() , we also derive that equality $d_0=\tilde {d}_0$
, we also derive that equality $d_0=\tilde {d}_0$![]() , which permits to repeat the same arguments explored in the proof of [Reference Hirata, Ikoma and Tanaka22, propostion 4.2] (see [Reference Jeanjean23, propostion 2.2]) to get the following proposition
, which permits to repeat the same arguments explored in the proof of [Reference Hirata, Ikoma and Tanaka22, propostion 4.2] (see [Reference Jeanjean23, propostion 2.2]) to get the following proposition
Proposition 4.3 There is a sequence $\{(s_n,u_n)\} \subset \mathbb {R} \times \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() such that
such that
(a) $s_n \to 0;$

(b) $\tilde {J}(s_n,u_n) \to \tilde {d};$

(c) $({\partial }/{\partial u})\tilde {J}(s_n,u_n)\to 0$
 strongly in $(\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}))^{*}$
strongly in $(\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}))^{*}$ ;
;(d) $({\partial }/{\partial s})\tilde {J}(s_n,u_n)\to 0$
 in $\mathbb {R}$
in $\mathbb {R}$ .
.
The next lemma establishes the boundedness of the sequence $(u_n) \subset \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() that was obtained in the last proposition. Since the proof follows the same spirit of lemma 3.4 we also omit its proof.
that was obtained in the last proposition. Since the proof follows the same spirit of lemma 3.4 we also omit its proof.
Lemma 4.4 The sequence $(u_n) \subset \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() is bounded.
is bounded.
4.1 Proof of theorem 1.2
By proposition 4.3, there is $\{(s_n,u_n)\} \subset \mathbb {R} \times \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() satisfying
satisfying
(a) $s_n \to 0;$

(b) $\tilde {J}(s_n,u_n) \to \tilde {d};$

(c) $({\partial }/{\partial u})\tilde {J}(s_n,u_n)\to 0$
 strongly in $(\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}))^{*}$
strongly in $(\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N}))^{*}$ ;
;(d) $({\partial }/{\partial s})\tilde {J}(s_n,u_n)\to 0$
 in $\mathbb {R}$
in $\mathbb {R}$ .
.
By lemma 3.4 the sequence $(u_n)$![]() is bounded in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
is bounded in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , then for some subsequence, still denoted by itself, there is $u_0 \in \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
, then for some subsequence, still denoted by itself, there is $u_0 \in \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() such that
such that
The item (d) implies that $({\partial }/{\partial u})\tilde {J}(s_n,u_n)w\to 0$![]() for all $w \in \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
for all $w \in \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , that is,
, that is,
Since $s_n \to 0$![]() , a simple computation guarantees that $u_0$
, a simple computation guarantees that $u_0$![]() satisfies the equality below
satisfies the equality below
showing that $J'(u_0)w=0$![]() for all $w \in \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
for all $w \in \mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , that is, $u_0$
, that is, $u_0$![]() is a critical point of $J$
is a critical point of $J$![]() in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() . We claim that $u_0 \not = 0$
. We claim that $u_0 \not = 0$![]() , because if $u_0=0$
, because if $u_0=0$![]() , lemma 2.5 gives that
, lemma 2.5 gives that
for all $0< a< b<+\infty$![]() and $p \in (2,2^{*}_{\gamma })$
and $p \in (2,2^{*}_{\gamma })$![]() .
.
From $(f_4)-(f_5)$![]() , given $\epsilon >0$
, given $\epsilon >0$![]() and $p \in (2,2^{*}_{\gamma })$
and $p \in (2,2^{*}_{\gamma })$![]() , there are $C>0$
, there are $C>0$![]() and $a< b<+\infty$
and $a< b<+\infty$![]() such that
such that
As $(u_n)$![]() is bounded in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
is bounded in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() , it follows that
, it follows that
where $M=\sup _{n \in \mathbb {N}}\int _{\mathbb {R}^{N}}|u_n|^{2^{*}_{\gamma }}\,{\rm d}z$![]() . Now, using the limit (4.1) we see that
. Now, using the limit (4.1) we see that
Thereby, as $\epsilon$![]() is arbitrary, we can infer that
is arbitrary, we can infer that
This limit combined with the limit $({\partial }/{\partial u})\tilde {J}(s_n,u_n)u_n=o_n(1)$![]() ensures that $u_n \to 0$
ensures that $u_n \to 0$![]() in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() . Hence,
. Hence,
which is absurd, because $\tilde {J}(s_n,u_n) \to \tilde {d}_0>0$![]() . Therefore, $u_0$
. Therefore, $u_0$![]() is a nontrivial critical point of $J$
is a nontrivial critical point of $J$![]() in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$
in $\mathcal {D}_{\gamma,rad_{x,y}}^{1,2}(\mathbb {R}^{N})$![]() . As in § 3, we can again use the Principle of Symmetric Criticality to prove that $u_0$
. As in § 3, we can again use the Principle of Symmetric Criticality to prove that $u_0$![]() is a critical point of $J$
is a critical point of $J$![]() in $\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$
in $\mathcal {D}_{\gamma }^{1,2}(\mathbb {R}^{N})$![]() .
.
5. Proof of theorem 1.3
In this section, the energy functional associated with $(P)_a$![]() is given by
is given by
Unfortunately the space $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$![]() does not have good compactness embedding to help us to find a nontrivial critical point $u$
does not have good compactness embedding to help us to find a nontrivial critical point $u$![]() for $I$
for $I$![]() . Following the same approach explored in the previous sections, we will restrict $I$
. Following the same approach explored in the previous sections, we will restrict $I$![]() to the space $\mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$
to the space $\mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$![]() . Since $I$
. Since $I$![]() satisfies the mountain pass geometry in $\mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$
satisfies the mountain pass geometry in $\mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$![]() , there is $(u_n) \subset \mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$
, there is $(u_n) \subset \mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$![]() such that
such that
where
where
Using well-known arguments, the condition $(f_7)$![]() helps us to prove that $(u_n)$
helps us to prove that $(u_n)$![]() is bounded in $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$
is bounded in $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$![]() . Since $\mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$
. Since $\mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$![]() is reflexive, we can assume that there exists $u_0\in \mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$
is reflexive, we can assume that there exists $u_0\in \mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$![]() such that, up to subsequence,
such that, up to subsequence,
• $u_n \rightharpoonup u_0$
 in $\mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N}),$
in $\mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N}),$
• $u_n \rightarrow u_0$
 in $L_{loc}^{p}(\mathbb {R}^{N})\ \text {for all}\ p\in (2,2^{*}_{\gamma })$
in $L_{loc}^{p}(\mathbb {R}^{N})\ \text {for all}\ p\in (2,2^{*}_{\gamma })$ ,
,• $u_n \rightarrow u_0\ a. e.\ \textit {in}\ \mathbb {R}^{N}$
 .
.
As $I'(u_n)v = o_n(1)$![]() for all $v \in \mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$
for all $v \in \mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$![]() , the above convergences yield
, the above convergences yield
from where it follows that $u_0$![]() is a critical point of $I$
is a critical point of $I$![]() .
.
Lemma 5.1 Let $(u_n)\subset \mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$![]() be a sequence such that
be a sequence such that
Then, there exist $(y_n)\subset \mathbb {R}^{k}$![]() and $r, \beta >0$
and $r, \beta >0$![]() such that
such that
Proof. In what follows, we will argue by contradiction. If the lemma is not true, we can employ lemma 2.1 to get
From $(f_1)$![]() and $(f_2)$
and $(f_2)$![]() , for each $\varepsilon >0$
, for each $\varepsilon >0$![]() , there is a constant $C_{\varepsilon }>0$
, there is a constant $C_{\varepsilon }>0$![]() such that
such that
Hence,
and so,
leading to
As $I'(u_n)u_n = o_n(1)$![]() , we have
, we have
that is,
Therefore
which is a contradiction, because $I(u_n)\longrightarrow d_a>0$![]() .
.
5.1 Proof of theorem 1.3
In what follows, we will divide the proof into two cases:
Case 1: (A3) holds.
In this case, we can assume that the weak limit $u_0 \in \mathcal {H}^{a}_{\gamma,rad_{x}}(\mathbb {R}^{N})$![]() of the sequence given in (5.1) is nontrivial. Otherwise, by lemma 5.1, there exist $(y_n)\subset \mathbb {R}^{k}$
of the sequence given in (5.1) is nontrivial. Otherwise, by lemma 5.1, there exist $(y_n)\subset \mathbb {R}^{k}$![]() and $r, \beta >0$
and $r, \beta >0$![]() such that
such that
Increasing $r$![]() if necessary, we can suppose that $y_n \in \mathbb {Z}^{k}$
if necessary, we can suppose that $y_n \in \mathbb {Z}^{k}$![]() . Now, setting the sequence
. Now, setting the sequence
it follows that $(v_n)\subset \mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() . As we are supposing that (A3) occurs, a simple computation shows that
. As we are supposing that (A3) occurs, a simple computation shows that
Hence $(v_n)$![]() is bounded, and for a subsequence of $(v_n)$
is bounded, and for a subsequence of $(v_n)$![]() , still denoted by itself, there is $v\in \mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$
, still denoted by itself, there is $v\in \mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() such that $v_n \rightharpoonup v$
such that $v_n \rightharpoonup v$![]() in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() . By lemma 2.2 $v_n \rightarrow v$
. By lemma 2.2 $v_n \rightarrow v$![]() in $L^{p}(\mathbb {R}^{m}\times B_r(0))$
in $L^{p}(\mathbb {R}^{m}\times B_r(0))$![]() for all $p\in (2,2^{*}_{\gamma })$
for all $p\in (2,2^{*}_{\gamma })$![]() and $v_n(z) \rightarrow v(z)$
and $v_n(z) \rightarrow v(z)$![]() almost everywhere in $\mathbb {R}^{N}$
almost everywhere in $\mathbb {R}^{N}$![]() . Furthermore,
. Furthermore,
from where it follows that $I'(v)w=0$![]() for all $w\in \mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$
for all $w\in \mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() , showing that $v$
, showing that $v$![]() is a critical point for $I$
is a critical point for $I$![]() in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() . On the other hand, the equality
. On the other hand, the equality
together with the limit $v_n \rightarrow v$![]() in $L^{p}(\mathbb {R}^{m}\times B_r(0))$
in $L^{p}(\mathbb {R}^{m}\times B_r(0))$![]() (see lemma 2.2) gives
(see lemma 2.2) gives
showing that $v\neq 0$![]() .
.
Case 1: (A4) holds.
If (A4) holds, lemma 2.6 implies that
This limit combined with lemma 2.6 ensures that
Now, since $I'(u_n)\to 0$![]() and $u_n \rightharpoonup u_0$
and $u_n \rightharpoonup u_0$![]() in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() , we find that
, we find that

which shows that $u_n \to u_0$![]() in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() . From this,
. From this,
and so, $u_0$![]() is a nontrivial critical point for $I$
is a nontrivial critical point for $I$![]() in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma, rad_x}^{a}(\mathbb {R}^{N})$![]() .
.
Finally, in order to conclude the proof, we must show that $u$![]() is a critical point for $I$
is a critical point for $I$![]() in $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$
in $\mathcal {H}_{\gamma }^{a}(\mathbb {R}^{N})$![]() . As in§ 3 and 4, it is enough to apply the Principle of Symmetric Criticality. However, since the argument are the same we omit its proof.
. As in§ 3 and 4, it is enough to apply the Principle of Symmetric Criticality. However, since the argument are the same we omit its proof.
6. Proof of theorem 1.4
Suppose that $u\in \mathcal {H}^{a}(\mathbb {R}^{N})$![]() is a solution of $(P)_a$
is a solution of $(P)_a$![]() . For any $R>0$
. For any $R>0$![]() , $0< r\leqslant R/2$
, $0< r\leqslant R/2$![]() , let $\eta \in C^{\infty }(\mathbb {R}^{N})$
, let $\eta \in C^{\infty }(\mathbb {R}^{N})$![]() , $0\leqslant \eta \leqslant 1$
, $0\leqslant \eta \leqslant 1$![]() with
with

where $R_{\gamma }=\max \{1, R^{2\gamma }\}$![]() . For each $L>0$
. For each $L>0$![]() , let us define
, let us define
$v_L=\eta ^{2}uu_{L}^{2(\beta -1)}$![]() and $w_L=\eta uu_{L}^{\beta -1}$
and $w_L=\eta uu_{L}^{\beta -1}$![]() with $\beta >1$
with $\beta >1$![]() to be determined later on. Then
to be determined later on. Then
and choosing $v_L$![]() as test function, we obtain
as test function, we obtain

As
and $a(z)\geqslant a_0>0$![]() in $\mathbb {R}^{N}$
in $\mathbb {R}^{N}$![]() we derive that
we derive that

By conditions $(f_1)$![]() and $(f_2)$
and $(f_2)$![]() , $f(u)\leqslant ({a_0}/{2})u+Cu^{q-1}$
, $f(u)\leqslant ({a_0}/{2})u+Cu^{q-1}$![]() , and so,
, and so,

Using Young's inequality with $\varepsilon >0$![]() sufficiently small, we get
sufficiently small, we get

From (2.2),
which leads to

and thus

Then from (6.1)
Using Hölder inequality with exponents ${{2^{*}_{\gamma }}}/({q-2})$![]() and ${\alpha ^{*}}/{2}:={{2^{*}_{\gamma }}}/{2^{*}_{\gamma }-(q-2)}$
and ${\alpha ^{*}}/{2}:={{2^{*}_{\gamma }}}/{2^{*}_{\gamma }-(q-2)}$![]() ,
,

Since $u\in L^{2^{*}_{\gamma }}(\mathbb {R}^{N})$![]() , we choose $\beta ={{2^{*}_{\gamma }}}/{\alpha ^{*}}>1$
, we choose $\beta ={{2^{*}_{\gamma }}}/{\alpha ^{*}}>1$![]() so that $u\in L^{\alpha ^{*}\beta }(\mathbb {R}^{N})\cap L^{2\beta }(\mathbb {R}^{N})$
so that $u\in L^{\alpha ^{*}\beta }(\mathbb {R}^{N})\cap L^{2\beta }(\mathbb {R}^{N})$![]() , and so,
, and so,

Hence

By employing the Fatou's lemma in the variable $L$![]() we conclude that
we conclude that
Once again from inequality (6.2),

We now consider $t={(2^{*}_{\gamma })^{2}}/{\alpha ^{*}(q-2)}>1$![]() and $\beta ={2^{*}_{\gamma }(t-1)}/{2t}>1$
and $\beta ={2^{*}_{\gamma }(t-1)}/{2t}>1$![]() . Then by Hölder inequality with exponents $t/(t-1)$
. Then by Hölder inequality with exponents $t/(t-1)$![]() and $t$
and $t$![]() ,
,

It follows from (6.3) that

On the other hand,

This combined with (6.4) leads to

Hence, by Fatou's lemma
Setting $\chi :={2^{*}_{\gamma }(t-1)}/{2t}$![]() and $s:={2t}/({t-1})$
and $s:={2t}/({t-1})$![]() , the last inequality allows to conclude that
, the last inequality allows to conclude that
Letting $m\to \infty$![]() we obtain
we obtain
showing that $\lim _{|z|\to \infty }u(z)=0$![]() .
.
Finally, in order to show that $u\in L^{\infty }(\mathbb {R}^{N})$![]() , we need only show that the above inequality is true on balls of $\mathbb {R}^{N}$
, we need only show that the above inequality is true on balls of $\mathbb {R}^{N}$![]() , that is, there exist $R>0$
, that is, there exist $R>0$![]() and $\mathcal {C}>0$
and $\mathcal {C}>0$![]() such that
such that
The argument follows with some adjusts from the ideas found in [Reference Alves and Germano4, lemma 2.13].
Acknowledgments
This work was partially supported by Projeto Universal FAPESQ 3031/2021. C. O. Alves was partially supported by CNPq/Brazil 307045/2021-8.