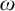1 Introduction
For a profinite group G, let
![]() ${\operatorname {Im}}(G)$
be the set of isomorphism classes of finite quotients of G. We say that G has the embedding property (EP) if for
${\operatorname {Im}}(G)$
be the set of isomorphism classes of finite quotients of G. We say that G has the embedding property (EP) if for
![]() $A,B\in {\operatorname {Im}}(G)$
and for every epimorphisms
$A,B\in {\operatorname {Im}}(G)$
and for every epimorphisms
![]() $\Pi :A\rightarrow B$
and
$\Pi :A\rightarrow B$
and
![]() $\varphi :G\rightarrow B$
, there is an epimorphism
$\varphi :G\rightarrow B$
, there is an epimorphism
![]() $\psi :G\rightarrow A$
such that
$\psi :G\rightarrow A$
such that
![]() $\Pi \circ \psi =\varphi $
.
$\Pi \circ \psi =\varphi $
.
In field arithmetic and model theory of fields, the embedding property for profinite groups appears surprisingly. Let
![]() $k^{ab}$
and
$k^{ab}$
and
![]() $k^{sol}$
be the maximal abelian extension and the maximal solvable extension of a number field k respectively. In [Reference Iwasaw11], Iwasawa showed that the Galois group
$k^{sol}$
be the maximal abelian extension and the maximal solvable extension of a number field k respectively. In [Reference Iwasaw11], Iwasawa showed that the Galois group
![]() $G(k^{sol}/k^{ab})$
has the embedding property. A Frobenius field is a PAC field whose absolute Galois group has the embedding property. Over all Frobenius fields whose absolute Galois groups have the same set of isomorphism classes of finite quotients and which contain a common subfield K, Fried, Haran, and Jarden in [Reference Fried, Haran and Jarden5] developed the theory of Galois stratification of K-constructible sets and proved an elimination of quantifiers of Galois formulas through Galois stratification. In [Reference Haran and Lubotzky7], Haran and Lubotzky gave a primitive recursive procedure to construct the universal embedding cover of a given finite group. Combined with the elimination of quantifiers of Galois formulas and the universal Frattini cover, they showed that the theory of perfect Frobenius fields is primitive recursive, and the theory of all Frobenius fields is decidable.
$G(k^{sol}/k^{ab})$
has the embedding property. A Frobenius field is a PAC field whose absolute Galois group has the embedding property. Over all Frobenius fields whose absolute Galois groups have the same set of isomorphism classes of finite quotients and which contain a common subfield K, Fried, Haran, and Jarden in [Reference Fried, Haran and Jarden5] developed the theory of Galois stratification of K-constructible sets and proved an elimination of quantifiers of Galois formulas through Galois stratification. In [Reference Haran and Lubotzky7], Haran and Lubotzky gave a primitive recursive procedure to construct the universal embedding cover of a given finite group. Combined with the elimination of quantifiers of Galois formulas and the universal Frattini cover, they showed that the theory of perfect Frobenius fields is primitive recursive, and the theory of all Frobenius fields is decidable.
Meanwhile, in model theory of PAC fields, one of most influential results is the work of Cherlin–van den Dries–Macintyre in [Reference Cherlin, van den Dries and Macintyre3]. In [Reference Cherlin, van den Dries and Macintyre3], they first introduced a notion of a complete system of a profinite group, which encodes the inverse system of finite quotients of the profinite group by its open normal subgroups, and so the category of complete systems whose morphisms are embeddings and the category of profinite groups whose morphisms are epimorphisms are equivalent by a contravariant functor. By the Elementary Equivalence Theorem for PAC fields (cf. [Reference Jarden and Kiehne8, Theorem 3.2]), the theory of a PAC field is determined by its basic algebraic properties, for example, characteristic, imperfection degree, and the relative algebraic closure of the prime field, and the theory of the complete system of the Galois group of the field. So, model theoretic properties of PAC fields are reduced to model theoretic properties of complete systems. Also, the complete system is the “right” object to study profinite groups model theoretically. Most of all, the class of complete systems is an elementary class, and for the Galois group
![]() $G(K)$
of a field K, its complete system is interpretable in the pair consisting of the algebraic closure of K and K. Note that an ultraproduct of profinite groups need not be a profinite group. In [Reference Chatzidakis1], Chatzidakis showed that the complete system of a profinite group having the embedding property is
$G(K)$
of a field K, its complete system is interpretable in the pair consisting of the algebraic closure of K and K. Note that an ultraproduct of profinite groups need not be a profinite group. In [Reference Chatzidakis1], Chatzidakis showed that the complete system of a profinite group having the embedding property is
![]() $\omega $
-stable. Using this with Chatzidakis’ independence theorem in [Reference Chatzidakis2, Theorem 3.1], Ramsey in [Reference Ramsey12, Theorem 3.9.31] showed that the theory of a Frobenius field is NSOP
$\omega $
-stable. Using this with Chatzidakis’ independence theorem in [Reference Chatzidakis2, Theorem 3.1], Ramsey in [Reference Ramsey12, Theorem 3.9.31] showed that the theory of a Frobenius field is NSOP
![]() $_1$
.
$_1$
.
In this article, we consider the embedding property in the category of sorted profinite groups. In [Reference Hoffmann and Lee10], we introduced a notion of sorted profinite groups to study the (Shelah-)Galois groups of first order structures. The Galois groups of first order structures are typical examples of sorted profinite groups (see Example 2.5). In [Reference Hoffmann and Lee10, Proposition 5.6], Hoffmann and the author developed the independence theorem for PAC structures, analogous to [Reference Chatzidakis2, Theorem 3.1], and we proved that for a PAC structure M, if the sorted complete system of the Galois group of M is
![]() $\omega $
-stable, then the theory of M is NSOP
$\omega $
-stable, then the theory of M is NSOP
![]() $_1$
. This leads us to try to find a class of sorted profinite groups having
$_1$
. This leads us to try to find a class of sorted profinite groups having
![]() $\omega $
-stable sorted complete systems. The possible candidates are the sorted profinite groups with “the embedding property.” So, we introduce a notion of the sorted embedding property (SEP) for a sorted profinite group, exactly analogous to the embedding property for profinite groups. Also, we need to find a notion of “embedding property” which is first order axiomatizable. For this, we introduce a weaker notion of the finitely sorted embedding property (FSEP) (see Definition 2.18). Fortunately, the two notions of the embedding properties for sorted profinite groups are equivalent (see Theorem 2.22).
$\omega $
-stable sorted complete systems. The possible candidates are the sorted profinite groups with “the embedding property.” So, we introduce a notion of the sorted embedding property (SEP) for a sorted profinite group, exactly analogous to the embedding property for profinite groups. Also, we need to find a notion of “embedding property” which is first order axiomatizable. For this, we introduce a weaker notion of the finitely sorted embedding property (FSEP) (see Definition 2.18). Fortunately, the two notions of the embedding properties for sorted profinite groups are equivalent (see Theorem 2.22).
We have two main results in this article. First, we show the existence and the uniqueness of the universal sorted embedding cover for a sorted profinite group (see Theorems 3.13 and 3.17). Second, we show that the theory of the complete system of a sorted profinite group having the sorted embedding property is
![]() $\omega $
-stable (see Theorem 4.15(2)).
$\omega $
-stable (see Theorem 4.15(2)).
In Section 2, we introduce the category of sorted profinite groups whose morphisms are always epimorphisms, and we see that the category of sorted profinite groups is closed under the inverse limit and the fibre product. And we recall the category of sorted complete systems, which is equivalent to the category of sorted profinite groups via natural contravariant functors. In Section 3, we prove the existence and uniqueness of a universal SEP-cover of given sorted profinite groups. In Section 4, we prove the theory of the sorted complete system of a sorted profinite group is
![]() $\omega $
-stable under the assumption that the set of sorts is countable, and we describe the forking independence there.
$\omega $
-stable under the assumption that the set of sorts is countable, and we describe the forking independence there.
2 Preliminaries
2.1 Sorted profinite group
For a profinite group G, we write
![]() ${\mathcal {N}}(G)$
for the set of open normal subgroups of G. Let
${\mathcal {N}}(G)$
for the set of open normal subgroups of G. Let
![]() ${\mathbb {N}}$
be the set of positive integers. Fix a set
${\mathbb {N}}$
be the set of positive integers. Fix a set
![]() ${\mathcal {J}}$
, called a set of sorts.
${\mathcal {J}}$
, called a set of sorts.
Notation 2.1.
-
(1) For
 $n\in {\mathbb {N}}$
, let
$n\in {\mathbb {N}}$
, let
 ${\mathcal {J}}^n$
be the set of n-tuples of elements in
${\mathcal {J}}^n$
be the set of n-tuples of elements in
 ${\mathcal {J}}$
, and let
${\mathcal {J}}$
, and let
 ${\mathcal {J}}^{<{\mathbb {N}}}:=\bigcup _{n\in {\mathbb {N}}} {\mathcal {J}}^n$
.
${\mathcal {J}}^{<{\mathbb {N}}}:=\bigcup _{n\in {\mathbb {N}}} {\mathcal {J}}^n$
. -
(2) For
 $J,J'\in {\mathcal {J}}^{<{\mathbb {N}}}$
, we write
$J,J'\in {\mathcal {J}}^{<{\mathbb {N}}}$
, we write
 $J\le J'$
if J is a subtuple of
$J\le J'$
if J is a subtuple of
 $J'$
, not necessarily consisting of successive elements.
$J'$
, not necessarily consisting of successive elements. -
(3) For
 $J,J'\in {\mathcal {J}}^{<{\mathbb {N}}}$
, we write
$J,J'\in {\mathcal {J}}^{<{\mathbb {N}}}$
, we write
 $J^{\frown } J'$
for the concatenation of J and
$J^{\frown } J'$
for the concatenation of J and
 $J'$
.
$J'$
. -
(4) For
 $J=(j_1,\ldots ,j_n)\in {\mathcal {J}}^n$
,
$J=(j_1,\ldots ,j_n)\in {\mathcal {J}}^n$
,
 $|J|:=n$
and
$|J|:=n$
and
 $\Vert J\Vert :=\{j_1,\ldots ,j_n\}$
.
$\Vert J\Vert :=\{j_1,\ldots ,j_n\}$
. -
(5) For
 $J=(j_1,\ldots ,j_n)\in {\mathcal {J}}^n$
and a permutation
$J=(j_1,\ldots ,j_n)\in {\mathcal {J}}^n$
and a permutation
 $\sigma \in \operatorname {Sym}(n)$
,
$\sigma \in \operatorname {Sym}(n)$
,
 $\sigma (J)=(j_{\sigma (1)},\ldots , j_{\sigma (n)})$
.
$\sigma (J)=(j_{\sigma (1)},\ldots , j_{\sigma (n)})$
. -
(6) For
 $J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,
$J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,
 $\sqrt {J}:=\{J'\in {\mathcal {J}}^{<{\mathbb {N}}}:\Vert J\Vert \subseteq \Vert J'\Vert \}$
.
$\sqrt {J}:=\{J'\in {\mathcal {J}}^{<{\mathbb {N}}}:\Vert J\Vert \subseteq \Vert J'\Vert \}$
.
Fix two functions:
-
•
 $J_{\subseteq }^*:{\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}\rightarrow {\mathcal {J}}^{<{\mathbb {N}}}$
; and
$J_{\subseteq }^*:{\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}\rightarrow {\mathcal {J}}^{<{\mathbb {N}}}$
; and -
•
 $J_{\cap }^*:{\mathcal {J}}^{<{\mathbb {N}}}\times {\mathcal {J}}^{<{\mathbb {N}}}\rightarrow {\mathcal {J}}^{<{\mathbb {N}}},(J,J')\mapsto J^{\frown }J'$
.
$J_{\cap }^*:{\mathcal {J}}^{<{\mathbb {N}}}\times {\mathcal {J}}^{<{\mathbb {N}}}\rightarrow {\mathcal {J}}^{<{\mathbb {N}}},(J,J')\mapsto J^{\frown }J'$
.
Note that the function
![]() $J_{\subseteq }^*$
can be arbitrary. The functions
$J_{\subseteq }^*$
can be arbitrary. The functions
![]() $J_{\subseteq }^*$
and
$J_{\subseteq }^*$
and
![]() $J_{\cap }^*$
are necessary to axiomatize the sorted complete system of a sorted profinite group in an appropriate first order language so that the sorted complete system satisfies the modular lattice axiom (cf. [Reference Hoffmann and Lee10, Definition 3.7]).
$J_{\cap }^*$
are necessary to axiomatize the sorted complete system of a sorted profinite group in an appropriate first order language so that the sorted complete system satisfies the modular lattice axiom (cf. [Reference Hoffmann and Lee10, Definition 3.7]).
Definition 2.2. For a profinite group G, we associate a non-empty subset
![]() $F(N)$
of
$F(N)$
of
![]() ${\mathcal {J}}^{<{\mathbb {N}}}$
for each
${\mathcal {J}}^{<{\mathbb {N}}}$
for each
![]() $N\in {\mathcal {N}}(G)$
and consider an indexed family
$N\in {\mathcal {N}}(G)$
and consider an indexed family
![]() $F:=\{F(N):N\in {\mathcal {N}}(G)\}$
. We say that the indexed family F is a sorting data of G if the following hold: For
$F:=\{F(N):N\in {\mathcal {N}}(G)\}$
. We say that the indexed family F is a sorting data of G if the following hold: For
![]() $N,N_1,N_2\in {\mathcal {N}}(G)$
:
$N,N_1,N_2\in {\mathcal {N}}(G)$
:
-
(1)
 $F(G)={\mathcal {J}}^{<{\mathbb {N}}}$
.
$F(G)={\mathcal {J}}^{<{\mathbb {N}}}$
. -
(2)
 $J\in F(N)\Leftrightarrow \sqrt {J}\subseteq F(N)$
.
$J\in F(N)\Leftrightarrow \sqrt {J}\subseteq F(N)$
. -
(3) Suppose
 $N_1\subseteq N_2$
and
$N_1\subseteq N_2$
and
 $[G:N_1]\le k$
. For
$[G:N_1]\le k$
. For
 $J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,
$J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,  $$ \begin{align*}J\in F(N_1)\Rightarrow J_{\subseteq}^*(k,J)\in F(N_2).\end{align*} $$
$$ \begin{align*}J\in F(N_1)\Rightarrow J_{\subseteq}^*(k,J)\in F(N_2).\end{align*} $$
-
(4) For
 $J_1\in F(N_1)$
and
$J_1\in F(N_1)$
and
 $J_2\in F(N_2)$
,
$J_2\in F(N_2)$
,
 $J_{\cap }^*(J_1,J_2)\in F(N_1\cap N_2)$
.
$J_{\cap }^*(J_1,J_2)\in F(N_1\cap N_2)$
.
We call the pair
![]() $(G,F)$
a sorted profinite group, and we say that the sorting data F comes from
$(G,F)$
a sorted profinite group, and we say that the sorting data F comes from
![]() ${\mathcal {J}}$
. For sorting data F,
${\mathcal {J}}$
. For sorting data F,
![]() $F'$
on G, we write
$F'$
on G, we write
![]() $F\subseteq F'$
if
$F\subseteq F'$
if
![]() $F(N)\subseteq F'(N)$
for any
$F(N)\subseteq F'(N)$
for any
![]() $N\in {\mathcal {N}}(G)$
. A sorting data F on G is called full if
$N\in {\mathcal {N}}(G)$
. A sorting data F on G is called full if
![]() $F(N)={\mathcal {J}}^{<{\mathbb {N}}}$
for all
$F(N)={\mathcal {J}}^{<{\mathbb {N}}}$
for all
![]() $N\in {\mathcal {N}}(G)$
.
$N\in {\mathcal {N}}(G)$
.
A typical example of a sorted profinite group comes from the Galois group of a first order structure by attaching additional data to each open normal subgroup of the Galois group, which recognize on which sorts a primitive element of a finite Galois extension of the structure live (cf. [Reference Hoffmann and Lee10, Section 2]). So, “sorted” in a sorted profinite group means that each open normal subgroup is sorted in a certain way.
Example 2.3. Fix a first order language
![]() ${\mathcal {L}}$
with a set
${\mathcal {L}}$
with a set
![]() ${\mathcal {J}}$
of all sorts. Let T be a complete
${\mathcal {J}}$
of all sorts. Let T be a complete
![]() ${\mathcal {L}}$
-theory eliminating quantifiers and imaginaries, and let
${\mathcal {L}}$
-theory eliminating quantifiers and imaginaries, and let
![]() $\mathfrak {C}\models T$
be a monster model. For each
$\mathfrak {C}\models T$
be a monster model. For each
![]() $J=(S_1,\ldots ,S_k)\in {\mathcal {J}}^{<{\mathbb {N}}}$
, write
$J=(S_1,\ldots ,S_k)\in {\mathcal {J}}^{<{\mathbb {N}}}$
, write
![]() $S_J$
for the sort of
$S_J$
for the sort of
![]() $S_1\times \cdots \times S_k$
.
$S_1\times \cdots \times S_k$
.
Let
![]() $K\subset \mathfrak {C}$
be a small definably closed substructure. Consider the Galois group of K,
$K\subset \mathfrak {C}$
be a small definably closed substructure. Consider the Galois group of K,
For each
![]() $N\in {\mathcal {N}}(G(K))$
, let
$N\in {\mathcal {N}}(G(K))$
, let
![]() ${\operatorname {acl}}(K)^N$
be the substructure consisting of elements in
${\operatorname {acl}}(K)^N$
be the substructure consisting of elements in
![]() ${\operatorname {acl}}(K)$
fixed pointwise by each
${\operatorname {acl}}(K)$
fixed pointwise by each
![]() $\sigma \in N$
, that is,
$\sigma \in N$
, that is,
Note that there is a finite tuple
![]() $a\in {\operatorname {acl}}(K)^N$
such that
$a\in {\operatorname {acl}}(K)^N$
such that
![]() ${\operatorname {acl}}(K)^N={\operatorname {dcl}}(a,K)$
and we call such an element a primitive element of
${\operatorname {acl}}(K)^N={\operatorname {dcl}}(a,K)$
and we call such an element a primitive element of
![]() ${\operatorname {acl}}(K)^N$
over K (cf. [Reference Hoffmann and Lee10, Fact 2.8]). An extension
${\operatorname {acl}}(K)^N$
over K (cf. [Reference Hoffmann and Lee10, Fact 2.8]). An extension
![]() $K\subseteq L \subseteq {\operatorname {acl}}(K)$
is called a Galois extension of K if
$K\subseteq L \subseteq {\operatorname {acl}}(K)$
is called a Galois extension of K if
![]() $L={\operatorname {dcl}}(L)$
and for each
$L={\operatorname {dcl}}(L)$
and for each
![]() $\sigma \in {\operatorname {Aut}}(\mathfrak {C}/K)$
,
$\sigma \in {\operatorname {Aut}}(\mathfrak {C}/K)$
,
![]() $\sigma [L]=L$
. For a Galois extension
$\sigma [L]=L$
. For a Galois extension
![]() $K\subseteq L$
, the Galois group of L over K is
$K\subseteq L$
, the Galois group of L over K is
Now, we define a natural sorting data F on
![]() $G(K)$
as follows: For
$G(K)$
as follows: For
![]() $N\in {\mathcal {N}}(G(K))$
with
$N\in {\mathcal {N}}(G(K))$
with
![]() $[G(K):N]=n$
and for
$[G(K):N]=n$
and for
![]() $J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,
$J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,
![]() $J\in F(N)$
if and only if there is a finite tuple a in
$J\in F(N)$
if and only if there is a finite tuple a in
![]() $(S_J({\operatorname {acl}}(K)))^n$
such that
$(S_J({\operatorname {acl}}(K)))^n$
such that
![]() ${\operatorname {dcl}}(K,a)={\operatorname {acl}}(K)^{N}$
. Note that there is a function
${\operatorname {dcl}}(K,a)={\operatorname {acl}}(K)^{N}$
. Note that there is a function
![]() $J_{\subseteq }^*:{\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}\rightarrow {\mathcal {J}}^{<{\mathbb {N}}}$
defined in [Reference Hoffmann and Lee10, Remark 3.1] such that for
$J_{\subseteq }^*:{\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}\rightarrow {\mathcal {J}}^{<{\mathbb {N}}}$
defined in [Reference Hoffmann and Lee10, Remark 3.1] such that for
![]() $N\subseteq N'\in {\mathcal {N}}(G(K))$
with
$N\subseteq N'\in {\mathcal {N}}(G(K))$
with
![]() $[G(K):N]\le k$
and for
$[G(K):N]\le k$
and for
![]() $J\in {\mathcal {J}}^{<{\mathbb {N}}}$
, if
$J\in {\mathcal {J}}^{<{\mathbb {N}}}$
, if
![]() $J\in F(N)$
, then
$J\in F(N)$
, then
![]() $J_{\subseteq }^*(k,J)\in F(N')$
. Similarly, for a Galois extension L of K, we define a natural sorting data F on
$J_{\subseteq }^*(k,J)\in F(N')$
. Similarly, for a Galois extension L of K, we define a natural sorting data F on
![]() $G(L/K)$
as follows: For
$G(L/K)$
as follows: For
![]() $N\in {\mathcal {N}}(G(L/K))$
with
$N\in {\mathcal {N}}(G(L/K))$
with
![]() $[G(L/K):N]=n$
and for
$[G(L/K):N]=n$
and for
![]() $J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,
$J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,
![]() $J\in F(N)$
if and only if there is a finite tuple a in
$J\in F(N)$
if and only if there is a finite tuple a in
![]() $(S_J(L))^n$
such that
$(S_J(L))^n$
such that
![]() ${\operatorname {dcl}}(K,a)=L^N$
.
${\operatorname {dcl}}(K,a)=L^N$
.
2.2 The category of sorted profinite groups
The category
![]() ${\operatorname {PG}}$
of profinite groups is a category consisting of the following:
${\operatorname {PG}}$
of profinite groups is a category consisting of the following:
-
•
 ${\operatorname {Ob}}$
: The objects of
${\operatorname {Ob}}$
: The objects of
 ${\operatorname {PG}}$
are profinite groups.
${\operatorname {PG}}$
are profinite groups. -
•
 ${\operatorname {Mor}}$
: Let
${\operatorname {Mor}}$
: Let
 $G_1$
and
$G_1$
and
 $G_2$
be profinite groups. A morphism from
$G_2$
be profinite groups. A morphism from
 $G_1$
to
$G_1$
to
 $G_2$
is a continuous homomorphism from
$G_2$
is a continuous homomorphism from
 $G_1$
to
$G_1$
to
 $G_2$
.
$G_2$
.
Next, we introduce the category of sorted profinite groups whose sorting data comes from
![]() ${\mathcal {J}}$
.
${\mathcal {J}}$
.
Definition 2.4. The category
![]() ${\operatorname {SPG}}_{{\mathcal {J}}}(J_{\subseteq }^*,J_{\cap }^*)$
of sorted profinite groups with sorting data from
${\operatorname {SPG}}_{{\mathcal {J}}}(J_{\subseteq }^*,J_{\cap }^*)$
of sorted profinite groups with sorting data from
![]() ${\mathcal {J}}$
consists of the following:
${\mathcal {J}}$
consists of the following:
-
•
 ${\operatorname {Ob}}$
: The objects are sorted profinite groups whose sorting data comes from
${\operatorname {Ob}}$
: The objects are sorted profinite groups whose sorting data comes from
 ${\mathcal {J}}$
; and
${\mathcal {J}}$
; and -
•
 ${\operatorname {Mor}}$
: Let
${\operatorname {Mor}}$
: Let
 $(G_1,F_1)$
and
$(G_1,F_1)$
and
 $(G_2, F_2)$
be in
$(G_2, F_2)$
be in
 ${\operatorname {Ob}}({\operatorname {SPG}}_{{\mathcal {J}}}(J_{\subseteq }^*,J_{\cap }^*))$
. A morphism f from
${\operatorname {Ob}}({\operatorname {SPG}}_{{\mathcal {J}}}(J_{\subseteq }^*,J_{\cap }^*))$
. A morphism f from
 $(G_1,F_1)$
to
$(G_1,F_1)$
to
 $(G_2,F_2)$
is an epimorphism from
$(G_2,F_2)$
is an epimorphism from
 $G_1$
to
$G_1$
to
 $G_2$
, that is, a surjective continuous homomorphism, satisfying that for
$G_2$
, that is, a surjective continuous homomorphism, satisfying that for
 $N\in {\mathcal {N}}(G_2)$
,
$N\in {\mathcal {N}}(G_2)$
,  $$ \begin{align*}F_2(N)\subseteq F_1(f^{-1}[N]).\end{align*} $$
$$ \begin{align*}F_2(N)\subseteq F_1(f^{-1}[N]).\end{align*} $$
For sorted profinite groups
![]() $(G,F)$
and
$(G,F)$
and
![]() $(G',F')$
, we say that an epimorphism
$(G',F')$
, we say that an epimorphism
![]() $\varphi :G'\rightarrow G$
is sorted with respect to
$\varphi :G'\rightarrow G$
is sorted with respect to
![]() $F'$
and F if the epimorphism
$F'$
and F if the epimorphism
![]() $\varphi $
induces a morphism from
$\varphi $
induces a morphism from
![]() $(G',F')$
to
$(G',F')$
to
![]() $(G,F)$
, that is, for each
$(G,F)$
, that is, for each
![]() $N\in {\mathcal {N}}(G)$
,
$N\in {\mathcal {N}}(G)$
,
If the sorting data F and
![]() $F'$
are clear from the context, we say
$F'$
are clear from the context, we say
![]() $\varphi $
is sorted instead of being sorted with respect to
$\varphi $
is sorted instead of being sorted with respect to
![]() $F'$
and F. If there is no confusion, we write
$F'$
and F. If there is no confusion, we write
![]() ${\operatorname {SPG}}$
for
${\operatorname {SPG}}$
for
![]() ${\operatorname {SPG}}_{{\mathcal {J}}}(J_{\subseteq }^*,J_{\cap }^*)$
.
${\operatorname {SPG}}_{{\mathcal {J}}}(J_{\subseteq }^*,J_{\cap }^*)$
.
The concept of a sorted epimorphism first appeared in [Reference Dobrowolski, Hoffmann and Lee4, Section 5] to study an ultraproduct of isomorphisms between Galois groups of first order structures. Note that in the category of sorted profinite groups, every morphism is surjective. To consider only epimorphisms between sorted profinite groups is natural in the view that the category of sorted profinite groups and the category of sorted complete systems are equivalent via a contravariant functor (cf. Section 2.4).
Example 2.5. Let us come back to Example 2.3 for a moment. For small definably closed substructures
![]() $K\subseteq K'\subset \mathfrak {C}$
, we say
$K\subseteq K'\subset \mathfrak {C}$
, we say
![]() $K'$
is a regular extension of K if
$K'$
is a regular extension of K if
![]() $K'\cap {\operatorname {acl}}(K)=K$
. Let
$K'\cap {\operatorname {acl}}(K)=K$
. Let
![]() $K\subset K' \subset \mathfrak {C}$
be small definably closed substructures such that
$K\subset K' \subset \mathfrak {C}$
be small definably closed substructures such that
![]() $K'$
is a regular extension of K. Then, the restriction map
$K'$
is a regular extension of K. Then, the restriction map
![]() ${\operatorname {res}}:G(K')\rightarrow G(K)$
induces a morphism from
${\operatorname {res}}:G(K')\rightarrow G(K)$
induces a morphism from
![]() $(G(K'),F')$
to
$(G(K'),F')$
to
![]() $(G(K),F)$
, where F and
$(G(K),F)$
, where F and
![]() $F'$
are the natural sorting data on
$F'$
are the natural sorting data on
![]() $G(K)$
and
$G(K)$
and
![]() $G(K')$
respectively. Namely, for each
$G(K')$
respectively. Namely, for each
![]() $N\in {\mathcal {N}}(G(K))$
and for a finite tuple a in
$N\in {\mathcal {N}}(G(K))$
and for a finite tuple a in
![]() ${\operatorname {acl}}(K)^N$
,
${\operatorname {acl}}(K)^N$
,
so that
![]() $F(N)\subseteq F'({\operatorname {res}}^{-1}[N])$
(cf. [Reference Hoffmann and Lee10, Fact 2.10]).
$F(N)\subseteq F'({\operatorname {res}}^{-1}[N])$
(cf. [Reference Hoffmann and Lee10, Fact 2.10]).
Our main goal in this subsection is to show that the category
![]() ${\operatorname {SPG}}$
is closed under taking the inverse limit and taking the fibre product. We first introduce some terminology.
${\operatorname {SPG}}$
is closed under taking the inverse limit and taking the fibre product. We first introduce some terminology.
Notation 2.6. Let I be an index set. For each
![]() $i\in I$
, let
$i\in I$
, let
![]() $F_i:=\{F_i(N)\subseteq {\mathcal {J}}^{<{\mathbb {N}}}:N\in {\mathcal {N}}(G)\}$
be an
$F_i:=\{F_i(N)\subseteq {\mathcal {J}}^{<{\mathbb {N}}}:N\in {\mathcal {N}}(G)\}$
be an
![]() ${\mathcal {N}}(G)$
-indexed set of subsets of
${\mathcal {N}}(G)$
-indexed set of subsets of
![]() ${\mathcal {J}}^{<{\mathbb {N}}}$
.
${\mathcal {J}}^{<{\mathbb {N}}}$
.
-
(1) Let
 $\bigcap _{i\in I}F_i$
be an
$\bigcap _{i\in I}F_i$
be an
 ${\mathcal {N}}(G)$
-indexed family given as follows: For each
${\mathcal {N}}(G)$
-indexed family given as follows: For each
 $N\in {\mathcal {N}}(G)$
,
$N\in {\mathcal {N}}(G)$
,
 $(\bigcap _{i\in I}F_i)(N):=\bigcap _{i\in I}F_i(N)$
.
$(\bigcap _{i\in I}F_i)(N):=\bigcap _{i\in I}F_i(N)$
. -
(2) Let
 $\bigcup _{i\in I}F_i$
be an
$\bigcup _{i\in I}F_i$
be an
 ${\mathcal {N}}(G)$
-indexed family given as follows: For each
${\mathcal {N}}(G)$
-indexed family given as follows: For each
 $N\in {\mathcal {N}}(G)$
,
$N\in {\mathcal {N}}(G)$
,
 $(\bigcup _{i\in I}F_i)(N):=\bigcup _{i\in I}F_i(N)$
.
$(\bigcup _{i\in I}F_i)(N):=\bigcup _{i\in I}F_i(N)$
.
Remark 2.7.
-
(1) For an indexed family
 $\hat F=\{\hat F(N)(\neq \emptyset ):N\in {\mathcal {N}}(G)\}$
, there is a unique minimal sorting data F on G such that for each
$\hat F=\{\hat F(N)(\neq \emptyset ):N\in {\mathcal {N}}(G)\}$
, there is a unique minimal sorting data F on G such that for each
 $N\in {\mathcal {N}}(G)$
,
$N\in {\mathcal {N}}(G)$
,
 $F(N)$
contains
$F(N)$
contains
 $\hat F(N)$
, which is called generated by
$\hat F(N)$
, which is called generated by
 $\hat {F}$
. Note that such a minimal one is given by taking intersections of all sorting data on G containing
$\hat {F}$
. Note that such a minimal one is given by taking intersections of all sorting data on G containing
 $\hat F$
. We call an indexed family
$\hat F$
. We call an indexed family
 $\hat F$
with
$\hat F$
with
 $\hat F(N)\neq \emptyset $
for each
$\hat F(N)\neq \emptyset $
for each
 $N\in {\mathcal {N}}(G)$
a pre-sorting data on G.
$N\in {\mathcal {N}}(G)$
a pre-sorting data on G. -
(2) Let
 $F_i=\{F_i(N):N\in {\mathcal {N}}(G)\}$
be a sorting data of a profinite group G for each
$F_i=\{F_i(N):N\in {\mathcal {N}}(G)\}$
be a sorting data of a profinite group G for each
 $i\in I$
. If
$i\in I$
. If
 $\bigcap _{i\in I}F_i(N)\neq \emptyset $
for each
$\bigcap _{i\in I}F_i(N)\neq \emptyset $
for each
 $N\in {\mathcal {N}}(G)$
, both of the pre-sorting data
$N\in {\mathcal {N}}(G)$
, both of the pre-sorting data
 $\bigcap F_i$
and
$\bigcap F_i$
and
 $\bigcup _{i\in I} F_i$
are sorting data on G. If I is finite, then
$\bigcup _{i\in I} F_i$
are sorting data on G. If I is finite, then
 $\bigcap _{i\in I}F_i(N)\neq \emptyset $
for each
$\bigcap _{i\in I}F_i(N)\neq \emptyset $
for each
 $N\in {\mathcal {N}}(G)$
so that
$N\in {\mathcal {N}}(G)$
so that
 $\bigcap _{i\in I} F_i$
is a sorting data on G. Namely, suppose
$\bigcap _{i\in I} F_i$
is a sorting data on G. Namely, suppose
 $I=\{i_1,\ldots ,i_n\}$
. Fix
$I=\{i_1,\ldots ,i_n\}$
. Fix
 $N\in {\mathcal {N}}(G)$
. For each
$N\in {\mathcal {N}}(G)$
. For each
 $i_j$
, choose
$i_j$
, choose
 $J_j\in F_{i_j}(N)$
. Then,
$J_j\in F_{i_j}(N)$
. Then,
 $J_1^{\frown }J_2^{\frown }\cdots ^{\frown }J_n$
is in
$J_1^{\frown }J_2^{\frown }\cdots ^{\frown }J_n$
is in
 $F_{i_1}(N)\cap \cdots \cap F_{i_n}(N)$
by Definition 2.2(2).
$F_{i_1}(N)\cap \cdots \cap F_{i_n}(N)$
by Definition 2.2(2).
Definition 2.8. Let
![]() $\varphi :G_1\rightarrow G_2$
be an epimorphism. Let
$\varphi :G_1\rightarrow G_2$
be an epimorphism. Let
![]() $F_1$
be a sorting data on
$F_1$
be a sorting data on
![]() $G_1$
. Consider a pre-sorting data
$G_1$
. Consider a pre-sorting data
![]() $\hat F_2$
on
$\hat F_2$
on
![]() $G_2$
given by
$G_2$
given by
![]() $\hat F_2(N_2):=F_1(f^{-1}[N_2])$
for
$\hat F_2(N_2):=F_1(f^{-1}[N_2])$
for
![]() $N_2\in {\mathcal {N}}(G_2)$
. Then, the pre-sorting data
$N_2\in {\mathcal {N}}(G_2)$
. Then, the pre-sorting data
![]() $\hat F_2$
is a sorting data, and we call this sorting data the push-forward sorting data of
$\hat F_2$
is a sorting data, and we call this sorting data the push-forward sorting data of
![]() $F_1$
along
$F_1$
along
![]() $\varphi $
, denoted by
$\varphi $
, denoted by
![]() $\varphi _*(F_1)$
.
$\varphi _*(F_1)$
.
Note that for any sorting data
![]() $F_2'$
on
$F_2'$
on
![]() $G_2$
,
$G_2$
,
![]() $\varphi :(G_1,F_1)\rightarrow (G_2,F_2')$
is sorted if and only if
$\varphi :(G_1,F_1)\rightarrow (G_2,F_2')$
is sorted if and only if
![]() $F_2'\subseteq \varphi _*(F_1)$
.
$F_2'\subseteq \varphi _*(F_1)$
.
Definition 2.9. Let G be a profinite group and let e be the identity of G.
-
(1) We say that a subset
 ${\mathcal {B}}\subseteq {\mathcal {N}}(G)$
is a base at e if for any
${\mathcal {B}}\subseteq {\mathcal {N}}(G)$
is a base at e if for any
 $N\in {\mathcal {N}}(G)$
, there is
$N\in {\mathcal {N}}(G)$
, there is
 $N'\in {\mathcal {B}}$
such that
$N'\in {\mathcal {B}}$
such that
 $N'\subseteq N$
.
$N'\subseteq N$
. -
(2) We say that a subset
 $X\subseteq {\mathcal {N}}(G)$
generates a base at e if the set
$X\subseteq {\mathcal {N}}(G)$
generates a base at e if the set
 ${\mathcal {B}}(X):=\{N_1\cap \cdots \cap N_k:N_i\in X\}$
forms a base at e, equivalently,
${\mathcal {B}}(X):=\{N_1\cap \cdots \cap N_k:N_i\in X\}$
forms a base at e, equivalently,
 $\bigcap X=\{e\}$
. Indeed, if X generates a base at e, then
$\bigcap X=\{e\}$
. Indeed, if X generates a base at e, then
 $\bigcap X=\{e\}$
because G is a Hausdorff space. Conversely, suppose
$\bigcap X=\{e\}$
because G is a Hausdorff space. Conversely, suppose
 $\bigcap X=\{e\}$
. Take
$\bigcap X=\{e\}$
. Take
 $N\in {\mathcal {N}}(G)$
arbitrary. Suppose
$N\in {\mathcal {N}}(G)$
arbitrary. Suppose
 $N_1\cap \cdots \cap N_k\not \subseteq N$
for any
$N_1\cap \cdots \cap N_k\not \subseteq N$
for any
 $N_1,\ldots ,N_k\in X$
. Then, by compactness, we have that
$N_1,\ldots ,N_k\in X$
. Then, by compactness, we have that
 $\bigcap X\cap G\setminus N\neq \emptyset $
and
$\bigcap X\cap G\setminus N\neq \emptyset $
and
 $e\not \in N$
, which is a contradiction. So, for some
$e\not \in N$
, which is a contradiction. So, for some
 $N_1,\ldots ,N_k\in X$
,
$N_1,\ldots ,N_k\in X$
,
 $N_1\cap \cdots \cap N_k\subseteq N$
.
$N_1\cap \cdots \cap N_k\subseteq N$
.
Remark 2.10. Let G be a profinite group.
-
(1) Let
 ${\mathcal {B}}\subseteq {\mathcal {N}}(G)$
be a base at e. For each
${\mathcal {B}}\subseteq {\mathcal {N}}(G)$
be a base at e. For each
 $N\in {\mathcal {B}}$
, choose
$N\in {\mathcal {B}}$
, choose
 $F_{{\mathcal {B}}}(N)(\neq \emptyset )\subseteq {\mathcal {J}}^{<{\mathbb {N}}}$
, and put
$F_{{\mathcal {B}}}(N)(\neq \emptyset )\subseteq {\mathcal {J}}^{<{\mathbb {N}}}$
, and put
 $F_{{\mathcal {B}}}:=\{F_{{\mathcal {B}}}(N):N\in {\mathcal {B}}\}$
, called a pre-sorting data on
$F_{{\mathcal {B}}}:=\{F_{{\mathcal {B}}}(N):N\in {\mathcal {B}}\}$
, called a pre-sorting data on
 ${\mathcal {B}}$
. Then, there is a unique minimal sorting data F such that for each
${\mathcal {B}}$
. Then, there is a unique minimal sorting data F such that for each
 $N\in {\mathcal {B}}$
,
$N\in {\mathcal {B}}$
,
 $F(N)$
contains
$F(N)$
contains
 $F_{{\mathcal {B}}}(N)$
. In this case, we say that F is generated by
$F_{{\mathcal {B}}}(N)$
. In this case, we say that F is generated by
 $F_{{\mathcal {B}}}$
.
$F_{{\mathcal {B}}}$
. -
(2) Let
 $X\subseteq {\mathcal {N}}(G)$
generate a base at e. For each
$X\subseteq {\mathcal {N}}(G)$
generate a base at e. For each
 $N\in X$
, choose
$N\in X$
, choose
 $F_X(N)(\neq \emptyset )\subseteq {\mathcal {J}}^{<{\mathbb {N}}}$
, and put
$F_X(N)(\neq \emptyset )\subseteq {\mathcal {J}}^{<{\mathbb {N}}}$
, and put
 $F_{X}:=\{F_X(N):N\in X\}$
, called a pre-sorting data on X. Then, there is a unique minimal sorting data F such that for each
$F_{X}:=\{F_X(N):N\in X\}$
, called a pre-sorting data on X. Then, there is a unique minimal sorting data F such that for each
 $N\in X$
,
$N\in X$
,
 $F(N)$
contains
$F(N)$
contains
 $F_X(N)$
. In this case, we say that F is generated by
$F_X(N)$
. In this case, we say that F is generated by
 $F_{X}$
.
$F_{X}$
.
Proof
![]() $(1)$
Let
$(1)$
Let
![]() ${\mathcal {B}}\subseteq {\mathcal {N}}(G)$
be a base at e and let
${\mathcal {B}}\subseteq {\mathcal {N}}(G)$
be a base at e and let
![]() $F_{{\mathcal {B}}}$
be a pre-sorting data on
$F_{{\mathcal {B}}}$
be a pre-sorting data on
![]() ${\mathcal {B}}$
. Define a pre-sorting data
${\mathcal {B}}$
. Define a pre-sorting data
![]() $\hat F$
on G given as follows: For
$\hat F$
on G given as follows: For
![]() $N\in {\mathcal {N}}(G)$
:
$N\in {\mathcal {N}}(G)$
:
-
• if
 $N\in {\mathcal {B}}$
, put
$N\in {\mathcal {B}}$
, put
 $\hat F(N):=F_{{\mathcal {B}}}(N)$
; and
$\hat F(N):=F_{{\mathcal {B}}}(N)$
; and -
• if
 $N\not \in {\mathcal {B}}$
, put
$N\not \in {\mathcal {B}}$
, put
 $\hat F(N):=\bigcup _{N'\subseteq N}\bigcup _{k\ge [G:N']}J_{\subseteq }^*[\{k\}\times F_{{\mathcal {B}}}(N') ]$
.
$\hat F(N):=\bigcup _{N'\subseteq N}\bigcup _{k\ge [G:N']}J_{\subseteq }^*[\{k\}\times F_{{\mathcal {B}}}(N') ]$
.
Let F be a sorting data of G generated by
![]() $\hat F$
, which exists by Remark 2.7. Then, the sorting data F is also generated by
$\hat F$
, which exists by Remark 2.7. Then, the sorting data F is also generated by
![]() $F_{{\mathcal {B}}}$
.
$F_{{\mathcal {B}}}$
.
![]() $(2)$
Let
$(2)$
Let
![]() $X\subseteq {\mathcal {N}}(G)$
generate a base at e. Put
$X\subseteq {\mathcal {N}}(G)$
generate a base at e. Put
![]() ${\mathcal {B}}:=\{N_1\cap \cdots \cap N_k:N_i\in X\}$
, which is a base at e. Let
${\mathcal {B}}:=\{N_1\cap \cdots \cap N_k:N_i\in X\}$
, which is a base at e. Let
![]() $F_X$
be a pre-sorting data on X. Define a pre-sorting data
$F_X$
be a pre-sorting data on X. Define a pre-sorting data
![]() $F_{{\mathcal {B}}}$
on
$F_{{\mathcal {B}}}$
on
![]() ${\mathcal {B}}$
given as follows: For
${\mathcal {B}}$
given as follows: For
![]() $N\in {\mathcal {B}}$
, put
$N\in {\mathcal {B}}$
, put
Let F be a sorting data of G generated by
![]() $F_{{\mathcal {B}}}$
, which exists by
$F_{{\mathcal {B}}}$
, which exists by
![]() $(1)$
. Then, the sorting data F is also generated by
$(1)$
. Then, the sorting data F is also generated by
![]() $F_{X}$
.
$F_{X}$
.
Now, we show that the category
![]() ${\operatorname {SPG}}$
is closed under taking the inverse limit.
${\operatorname {SPG}}$
is closed under taking the inverse limit.
Proposition 2.11. The category
![]() ${\operatorname {SPG}}$
is closed under taking the inverse limit.
${\operatorname {SPG}}$
is closed under taking the inverse limit.
Proof Consider an inverse system
![]() $((G_i,F_i),f_j^i:(G_i,F_i)\rightarrow (G_j,F_j))_{j\le i\in I}$
of sorted profinite groups indexed by a directed poset
$((G_i,F_i),f_j^i:(G_i,F_i)\rightarrow (G_j,F_j))_{j\le i\in I}$
of sorted profinite groups indexed by a directed poset
![]() $(I,\le )$
. Let G be the inverse limit of
$(I,\le )$
. Let G be the inverse limit of
![]() $G_i$
in the category of profinite groups, which is a profinite group. Then, for each
$G_i$
in the category of profinite groups, which is a profinite group. Then, for each
![]() $i\in I$
, there is an epimorphism
$i\in I$
, there is an epimorphism
![]() $f_i:G\rightarrow G_i$
such that for
$f_i:G\rightarrow G_i$
such that for
![]() $j\le i$
,
$j\le i$
,
![]() $f_j=f_j^i\circ f_i$
.
$f_j=f_j^i\circ f_i$
.
We consider a pre-sorting data
![]() $\hat F$
on G given as follows: Let
$\hat F$
on G given as follows: Let
![]() $N\in {\mathcal {N}}(G)$
. Put
$N\in {\mathcal {N}}(G)$
. Put
![]() ${I_N:=\{i\in I:N=f_i^{-1}[f_i[N]]\}}$
, equivalently,
${I_N:=\{i\in I:N=f_i^{-1}[f_i[N]]\}}$
, equivalently,
![]() $i\in I_N$
if and only if
$i\in I_N$
if and only if
![]() ${\operatorname {Ker}} f_i\subseteq N$
. Note that
${\operatorname {Ker}} f_i\subseteq N$
. Note that
![]() $I_N\neq \emptyset $
. Put
$I_N\neq \emptyset $
. Put
 $$ \begin{align*}\hat F(N):=\bigcup_{i\in I_N} F_i(f_i[N]).\end{align*} $$
$$ \begin{align*}\hat F(N):=\bigcup_{i\in I_N} F_i(f_i[N]).\end{align*} $$
Note that
![]() $F_j(f_j[N])\subseteq F_i(f_i[N])$
for
$F_j(f_j[N])\subseteq F_i(f_i[N])$
for
![]() $j\le i \in I_N$
.
$j\le i \in I_N$
.
Claim 2.12. The pre-sorting data
![]() $\hat F$
is a sorting data.
$\hat F$
is a sorting data.
Proof It is enough to show that
![]() $\hat F$
satisfies the conditions
$\hat F$
satisfies the conditions
![]() $(3)$
and
$(3)$
and
![]() $(4)$
in Definition 2.2. We first show that the condition
$(4)$
in Definition 2.2. We first show that the condition
![]() $(3)$
holds for
$(3)$
holds for
![]() $\hat F$
. Take
$\hat F$
. Take
![]() $N_1\subseteq N_2\in {\mathcal {N}}(G)$
and
$N_1\subseteq N_2\in {\mathcal {N}}(G)$
and
![]() $k\in {\mathbb {N}}$
with
$k\in {\mathbb {N}}$
with
![]() $[G:N_1]\le k$
. Take
$[G:N_1]\le k$
. Take
![]() $J\in {\mathcal {J}}^{<{\mathbb {N}}}$
with
$J\in {\mathcal {J}}^{<{\mathbb {N}}}$
with
![]() $J\in \hat F(N_1)$
. Then, by definition, there is
$J\in \hat F(N_1)$
. Then, by definition, there is
![]() $i\in I$
such that
$i\in I$
such that
![]() ${\operatorname {Ker}} f_i\subseteq N_1$
and
${\operatorname {Ker}} f_i\subseteq N_1$
and
![]() $J\in F_i(f_i[N_1])$
. We have that
$J\in F_i(f_i[N_1])$
. We have that
![]() $J_{\subseteq }^*(k,J)\in F_i(f_i[N_2])$
because
$J_{\subseteq }^*(k,J)\in F_i(f_i[N_2])$
because
![]() $F_i$
is a sorting data of
$F_i$
is a sorting data of
![]() $G_i$
and
$G_i$
and
![]() $f_i[N_1]\subseteq f_i[N_2]\in {\mathcal {N}}(G_i)$
. Since
$f_i[N_1]\subseteq f_i[N_2]\in {\mathcal {N}}(G_i)$
. Since
![]() ${\operatorname {Ker}} f_i\subseteq N_1\subseteq N_2$
, we have that
${\operatorname {Ker}} f_i\subseteq N_1\subseteq N_2$
, we have that
![]() $i\in I_{N_2}$
and
$i\in I_{N_2}$
and
![]() $J_{\subseteq }^*(k,J)\in F_i(f_i[N_2])\subseteq \hat F(N_2)$
.
$J_{\subseteq }^*(k,J)\in F_i(f_i[N_2])\subseteq \hat F(N_2)$
.
Next, we show that the condition
![]() $(4)$
holds. Take
$(4)$
holds. Take
![]() $J_1\in \hat F(N_1)$
and
$J_1\in \hat F(N_1)$
and
![]() $J_2\in \hat F(N_2)$
. Take
$J_2\in \hat F(N_2)$
. Take
![]() $i\in I$
such that:
$i\in I$
such that:
-
•
 ${\operatorname {Ker}} f_i\subseteq N_1\cap N_2$
; and
${\operatorname {Ker}} f_i\subseteq N_1\cap N_2$
; and -
•
 $J_1\in F_i(f_i[N_1])$
and
$J_1\in F_i(f_i[N_1])$
and
 $J_2\in F_i(f_i[N_2])$
.
$J_2\in F_i(f_i[N_2])$
.
We have that
![]() $f_i[N_1]\cap f_i[N_2]=f_i[N_1\cap N_2]$
because
$f_i[N_1]\cap f_i[N_2]=f_i[N_1\cap N_2]$
because
![]() ${\operatorname {Ker}} f_i\subseteq N_1\cap N_2$
. Also, since
${\operatorname {Ker}} f_i\subseteq N_1\cap N_2$
. Also, since
![]() $F_i$
is a sorting data of
$F_i$
is a sorting data of
![]() $G_i$
, we have that
$G_i$
, we have that
Claim 2.13. The sorted profinite group
![]() $(G,\hat F)$
satisfies the following universal property: Let
$(G,\hat F)$
satisfies the following universal property: Let
![]() $(G',F')$
be a sorted profinite group and let
$(G',F')$
be a sorted profinite group and let
![]() $g_i :(G',F')\rightarrow (G_i,F_i)$
be a morphism for each
$g_i :(G',F')\rightarrow (G_i,F_i)$
be a morphism for each
![]() $i\in I$
. Then, there is a morphism
$i\in I$
. Then, there is a morphism
![]() $g:(G',F')\rightarrow (G,F)$
such that for each i,
$g:(G',F')\rightarrow (G,F)$
such that for each i,
![]() $g_i=f_i\circ g$
.
$g_i=f_i\circ g$
.
Proof Since G is the inverse limit of
![]() $G_i$
in the category of profinite groups, there is a morphism
$G_i$
in the category of profinite groups, there is a morphism
![]() $g:G'\rightarrow G$
such that for each i,
$g:G'\rightarrow G$
such that for each i,
![]() $g_i=f_i\circ g$
. It is enough to show that g is a morphism in the category of
$g_i=f_i\circ g$
. It is enough to show that g is a morphism in the category of
![]() ${\operatorname {SPG}}$
, that is, for each
${\operatorname {SPG}}$
, that is, for each
![]() $N\in {\mathcal {N}}(G)$
,
$N\in {\mathcal {N}}(G)$
,
Take
![]() $J\in \hat F(N)$
. By definition, there is
$J\in \hat F(N)$
. By definition, there is
![]() $i\in I$
such that
$i\in I$
such that
![]() ${\operatorname {Ker}} f_i\subseteq N$
and
${\operatorname {Ker}} f_i\subseteq N$
and
![]() ${J\in F_i(f_i[N])}$
. Since
${J\in F_i(f_i[N])}$
. Since
![]() ${\operatorname {Ker}} f_i\subseteq N$
and
${\operatorname {Ker}} f_i\subseteq N$
and
![]() $g_i=f_i\circ g$
, we have that
$g_i=f_i\circ g$
, we have that
Since
![]() $g_i$
is a morphism in
$g_i$
is a morphism in
![]() ${\operatorname {SPG}}$
and
${\operatorname {SPG}}$
and
![]() $J\in F_i(f_i[N])$
, we have that
$J\in F_i(f_i[N])$
, we have that
By Claim 2.13, the sorted profinite group
![]() $(G,\hat F)$
is the inverse limit in the category
$(G,\hat F)$
is the inverse limit in the category
![]() ${\operatorname {SPG}}$
.
${\operatorname {SPG}}$
.
Next, we consider a notion of the fibre product in the category
![]() ${\operatorname {SPG}}$
.
${\operatorname {SPG}}$
.
Definition 2.14. Let
![]() $\Pi _1:(B_1,F_1)\rightarrow (A,F_A)$
and
$\Pi _1:(B_1,F_1)\rightarrow (A,F_A)$
and
![]() $\Pi _2:(B_2,F_2)\rightarrow (A,F_A)$
be morphisms of sorted profinite groups so that they are epimorphisms. The fibre product of
$\Pi _2:(B_2,F_2)\rightarrow (A,F_A)$
be morphisms of sorted profinite groups so that they are epimorphisms. The fibre product of
![]() $B_1$
and
$B_1$
and
![]() $B_2$
over A with respect to
$B_2$
over A with respect to
![]() $\Pi _1$
and
$\Pi _1$
and
![]() $\Pi _2$
is the following sorted profinite group
$\Pi _2$
is the following sorted profinite group
![]() $(B,F)$
:
$(B,F)$
:
-
•
 $B=B_1\times _A B_2=\{(b_1,b_2)\in B_1\times B_2:\Pi _1(b_1)=\Pi _2(b_2)\}$
, which is the fibre product in the category of profinite groups.
$B=B_1\times _A B_2=\{(b_1,b_2)\in B_1\times B_2:\Pi _1(b_1)=\Pi _2(b_2)\}$
, which is the fibre product in the category of profinite groups. -
• Let
 $p_1:B\rightarrow B_1$
and
$p_1:B\rightarrow B_1$
and
 $p_2:B\rightarrow B_2$
be the projection maps. Let
$p_2:B\rightarrow B_2$
be the projection maps. Let
 $X=\{p_1^{-1}[N_1]:N_1\in {\mathcal {N}}(B_1)\}\cup \{p_2^{-1}[N_2]:N_2\in {\mathcal {N}}(B_2)\}$
, which generates a base at e because
$X=\{p_1^{-1}[N_1]:N_1\in {\mathcal {N}}(B_1)\}\cup \{p_2^{-1}[N_2]:N_2\in {\mathcal {N}}(B_2)\}$
, which generates a base at e because
 $\bigcap X=\{(e_1,e_2)\}$
where
$\bigcap X=\{(e_1,e_2)\}$
where
 $e_1$
and
$e_1$
and
 $e_2$
are the identities of
$e_2$
are the identities of
 $B_1$
and
$B_1$
and
 $B_2$
respectively. Let
$B_2$
respectively. Let
 $F_X$
be a pre-sorting data on X given as follows: For
$F_X$
be a pre-sorting data on X given as follows: For
 $N_1\in {\mathcal {N}}(G_1)$
and
$N_1\in {\mathcal {N}}(G_1)$
and
 $N_2\in {\mathcal {N}}(G_2)$
, Let F be the sorting data generated by
$N_2\in {\mathcal {N}}(G_2)$
, Let F be the sorting data generated by $$ \begin{align*}F_X(p_1^{-1}[N_1])=F_1(N_1),\ F_X(p_2^{-1}[N_2])=F_2(N_2).\end{align*} $$
$$ \begin{align*}F_X(p_1^{-1}[N_1])=F_1(N_1),\ F_X(p_2^{-1}[N_2])=F_2(N_2).\end{align*} $$
 $F_X$
.
$F_X$
.
The sorting data F is the minimal one among sorting data
![]() $F'$
on B to make
$F'$
on B to make
![]() $p_1$
and
$p_1$
and
![]() $p_2$
morphisms in the category
$p_2$
morphisms in the category
![]() ${\operatorname {SPG}}$
, that is, the sorting data F makes the projection maps
${\operatorname {SPG}}$
, that is, the sorting data F makes the projection maps
![]() $p_1$
and
$p_1$
and
![]() $p_2$
sorted and for any such sorting data
$p_2$
sorted and for any such sorting data
![]() $F'$
,
$F'$
,
![]() $F\subseteq F'$
.
$F\subseteq F'$
.
In the category of profinite groups, the fibre product is characterized by the following properties:
Remark 2.15 [Reference Haran and Lubotzky7, Lemma 1.1].
Consider a commutative diagram of groups with epimorphisms in the category of sorted profinite groups:

and put
![]() $p=\Pi _1\circ p_1=\Pi _2\circ p_2$
. The following are equivalent:
$p=\Pi _1\circ p_1=\Pi _2\circ p_2$
. The following are equivalent:
-
(1) B is isomorphic to the fibre product of
 $B_1$
and
$B_1$
and
 $B_2$
over A.
$B_2$
over A. -
(2) B with
 $p_1$
and
$p_1$
and
 $p_2$
is a pullback of the pair
$p_2$
is a pullback of the pair
 $(\Pi _1,\Pi _2)$
, that is, for any morphisms
$(\Pi _1,\Pi _2)$
, that is, for any morphisms
 $\psi _i:C\rightarrow B_i$
for
$\psi _i:C\rightarrow B_i$
for
 $i=1,2$
with
$i=1,2$
with
 $\Pi _1\circ \psi _1=\Pi _2\circ \psi _2$
, there is a unique morphism
$\Pi _1\circ \psi _1=\Pi _2\circ \psi _2$
, there is a unique morphism
 $\psi :C\rightarrow B$
such that
$\psi :C\rightarrow B$
such that
 $p_i\circ \psi =\psi _i$
for
$p_i\circ \psi =\psi _i$
for
 $i=1,2$
.
$i=1,2$
. 
-
(3)
 ${\operatorname {Ker}} p_1\cap {\operatorname {Ker}} p_2=\{e\}$
, and A with
${\operatorname {Ker}} p_1\cap {\operatorname {Ker}} p_2=\{e\}$
, and A with
 $\Pi _1, \Pi _2$
is a pushout of the pair
$\Pi _1, \Pi _2$
is a pushout of the pair
 $(p_1,p_2)$
, that is, for any morphism
$(p_1,p_2)$
, that is, for any morphism
 $\varphi _i:B_i\rightarrow G$
for
$\varphi _i:B_i\rightarrow G$
for
 $i=1,2$
with
$i=1,2$
with
 $\varphi _1\circ p_1=\varphi _2\circ p_2$
, there is a unique morphism
$\varphi _1\circ p_1=\varphi _2\circ p_2$
, there is a unique morphism
 $\varphi :A\rightarrow G$
such that
$\varphi :A\rightarrow G$
such that
 $\varphi \circ \Pi _i=\varphi _i$
for
$\varphi \circ \Pi _i=\varphi _i$
for
 $i=1,2$
.
$i=1,2$
. 
-
(4)
 ${\operatorname {Ker}} p={\operatorname {Ker}} p_1 \times {\operatorname {Ker}} p_2$
.
${\operatorname {Ker}} p={\operatorname {Ker}} p_1 \times {\operatorname {Ker}} p_2$
.
Note the universal property of
![]() $(2)$
does not make sense in the category
$(2)$
does not make sense in the category
![]() ${\operatorname {SPG}}$
because the map
${\operatorname {SPG}}$
because the map
![]() $\psi $
need not be surjective even though both
$\psi $
need not be surjective even though both
![]() $\psi _1$
and
$\psi _1$
and
![]() $\psi _2$
are surjective.
$\psi _2$
are surjective.
We borrow a notion of a Cartesian diagram in the category of profinite groups from [Reference Haran and Lubotzky7, p. 185].
Definition 2.16. We say that a diagram of sorted profinite groups in the category
![]() ${\operatorname {SPG}}$
${\operatorname {SPG}}$

is called Cartesian if
![]() $(B,F)$
is isomorphic to the fibre product of
$(B,F)$
is isomorphic to the fibre product of
![]() $(B_1,F_1)$
and
$(B_1,F_1)$
and
![]() $(B_2,F_2)$
over
$(B_2,F_2)$
over
![]() $(A,F_A)$
.
$(A,F_A)$
.
We have the following analogue to [Reference Haran and Lubotzky7, Lemma 1.2].
Remark 2.17. Let
![]() $\psi _i:(C,F_C)\rightarrow (B_i,F_i)$
be a morphism for
$\psi _i:(C,F_C)\rightarrow (B_i,F_i)$
be a morphism for
![]() $i=1,2$
. Then there is a commutative diagram:
$i=1,2$
. Then there is a commutative diagram:

where the square is Cartesian and
![]() $\psi $
is a morphism.
$\psi $
is a morphism.
Proof By [Reference Haran and Lubotzky7, Lemma 1.2], there is a unique commutative diagram

such that B is the fibre product of
![]() $B_1$
and
$B_1$
and
![]() $B_2$
over A with an epimorphism
$B_2$
over A with an epimorphism
![]() $\psi $
in the category of profinite groups. Let
$\psi $
in the category of profinite groups. Let
![]() $F_A$
be an arbitrary sorting data on A such that the epimorphism
$F_A$
be an arbitrary sorting data on A such that the epimorphism
![]() $\Pi _i:(B_i,F_i)\rightarrow (A,F_A)$
is sorted for each
$\Pi _i:(B_i,F_i)\rightarrow (A,F_A)$
is sorted for each
![]() $i=1,2$
. Take the sorting data F such that
$i=1,2$
. Take the sorting data F such that
![]() $(B,F)$
is the fibre product of
$(B,F)$
is the fibre product of
![]() $(B_1,F_1)$
and
$(B_1,F_1)$
and
![]() $(B_2,F_2)$
over
$(B_2,F_2)$
over
![]() $(A,F_A)$
so that the square is Cartesian in the category
$(A,F_A)$
so that the square is Cartesian in the category
![]() ${\operatorname {SPG}}$
. Note that the sorting data F depends only on two morphisms
${\operatorname {SPG}}$
. Note that the sorting data F depends only on two morphisms
![]() $p_1$
and
$p_1$
and
![]() $p_2$
.
$p_2$
.
It remains to show that the surjective homomorphism
![]() $\psi :(C,F_C)\rightarrow (B,F)$
is sorted. Put
$\psi :(C,F_C)\rightarrow (B,F)$
is sorted. Put
![]() $X=\{p_1^{-1}[N_1]:N_1\in {\mathcal {N}}(B_1)\}\cup \{p_2^{-1}[N_2]:N_2\in {\mathcal {N}}(B_2)\}$
. Take
$X=\{p_1^{-1}[N_1]:N_1\in {\mathcal {N}}(B_1)\}\cup \{p_2^{-1}[N_2]:N_2\in {\mathcal {N}}(B_2)\}$
. Take
![]() $N_1\in {\mathcal {N}}(B_1)$
and
$N_1\in {\mathcal {N}}(B_1)$
and
![]() $J_1\in F_1(N_1)$
. Then, we have that
$J_1\in F_1(N_1)$
. Then, we have that
because
![]() $\psi _1=p_1\circ \psi $
. The same things holds for
$\psi _1=p_1\circ \psi $
. The same things holds for
![]() $N_2\in {\mathcal {N}}(B_2)$
and
$N_2\in {\mathcal {N}}(B_2)$
and
![]() $J_2\in F_2(N_2)$
. Thus, by the minimality of F, we have that
$J_2\in F_2(N_2)$
. Thus, by the minimality of F, we have that
![]() $F[N]\subseteq F_C(\psi ^{-1}[N])(=\psi _*(F_C)[N])$
for any
$F[N]\subseteq F_C(\psi ^{-1}[N])(=\psi _*(F_C)[N])$
for any
![]() $N\in X$
and so
$N\in X$
and so
![]() $F\subseteq \psi _*(F_C)$
. So, by Remark 2.8(1), the epimorphism
$F\subseteq \psi _*(F_C)$
. So, by Remark 2.8(1), the epimorphism
![]() $\psi :(C,F_C)\rightarrow (B,F)$
is sorted.
$\psi :(C,F_C)\rightarrow (B,F)$
is sorted.
2.3 Sorted embedding property
We introduce the sorted embedding property for sorted profinite groups, analogous to the embedding property for profinite groups in [Reference Haran and Lubotzky7] (or also called the Iwasawa property in [Reference Chatzidakis1]). We start with the definition of embedding condition from [Reference Haran and Lubotzky7, p. 185]. Fix a set
![]() ${\mathcal {J}}$
. Throughout this section, a sorted profinite group means a sorted profinite group in
${\mathcal {J}}$
. Throughout this section, a sorted profinite group means a sorted profinite group in
![]() ${\operatorname {SPG}}$
. Let
${\operatorname {SPG}}$
. Let
![]() $(G,F)$
be a sorted profinite group. For a pair
$(G,F)$
be a sorted profinite group. For a pair
![]() $((A,F_A),(B,F_B))$
of sorted profinite groups, the sorted embedding condition, denoted by
$((A,F_A),(B,F_B))$
of sorted profinite groups, the sorted embedding condition, denoted by
![]() ${\operatorname {Emb}}_{(G,F)}((A,F_A),(B,F_B))$
, is defined as follows: If
${\operatorname {Emb}}_{(G,F)}((A,F_A),(B,F_B))$
, is defined as follows: If
![]() $(A,F_A)$
is a quotient of
$(A,F_A)$
is a quotient of
![]() $(G,F)$
, then for every pair of morphisms
$(G,F)$
, then for every pair of morphisms
![]() $\Pi :(A,F_A)\rightarrow (B,F_B)$
and
$\Pi :(A,F_A)\rightarrow (B,F_B)$
and
![]() $\varphi :(G,F)\rightarrow (B,F_B)$
, there is a morphism
$\varphi :(G,F)\rightarrow (B,F_B)$
, there is a morphism
![]() $\psi :(G,F)\rightarrow (A,F_A)$
such that
$\psi :(G,F)\rightarrow (A,F_A)$
such that
![]() $\Pi \circ \psi =\varphi $
. Let
$\Pi \circ \psi =\varphi $
. Let
![]() ${\operatorname {SIm}}(G,F)$
be the set of isomorphism classes of sorted finite quotients of
${\operatorname {SIm}}(G,F)$
be the set of isomorphism classes of sorted finite quotients of
![]() $(G,F)$
.
$(G,F)$
.
Definition 2.18. We say that a sorted profinite group
![]() $(G,F)$
satisfies the sorted embedding property (SEP) if for all
$(G,F)$
satisfies the sorted embedding property (SEP) if for all
![]() $(A,F_A),(B,F_B)\in {\operatorname {SIm}}(G,F)$
, the condition
$(A,F_A),(B,F_B)\in {\operatorname {SIm}}(G,F)$
, the condition
![]() ${\operatorname {Emb}}_{(G,F)}((A,F_A),(B,F_B))$
holds.
${\operatorname {Emb}}_{(G,F)}((A,F_A),(B,F_B))$
holds.
Also, we introduce the finitely sorted embedding property (FSEP), which is weaker than SEP, but they will turn out to be equivalent in Theorem 2.22. The advantage of FSEP is that it can be first order axiomatizable in the language of sorted complete systems. We say that a sorting data F on a profinite group G is finitely generated if there are a subset X of
![]() ${\mathcal {N}}(G)$
generating a base at e and a pre-sorting data
${\mathcal {N}}(G)$
generating a base at e and a pre-sorting data
![]() $F_{X}$
such that:
$F_{X}$
such that:
-
•
 $F_X$
generates F; and
$F_X$
generates F; and -
•
 $F_X(N)$
is finite for each
$F_X(N)$
is finite for each
 $N\in X$
.
$N\in X$
.
In this case, we say that
![]() $(G,F)$
is finitely sorted. Let
$(G,F)$
is finitely sorted. Let
![]() ${\operatorname {FSIm}}(G,F)$
be the set of isomorphism classes of finitely sorted finite quotients of
${\operatorname {FSIm}}(G,F)$
be the set of isomorphism classes of finitely sorted finite quotients of
![]() $(G,F)$
. Clearly we have that
$(G,F)$
. Clearly we have that
![]() ${\operatorname {FSIm}}(G,F)\subset {\operatorname {SIm}}(G,F)$
.
${\operatorname {FSIm}}(G,F)\subset {\operatorname {SIm}}(G,F)$
.
Definition 2.19. We say that a sorted profinite group
![]() $(G,F)$
satisfies the finitely sorted embedding property (FSEP) if for all
$(G,F)$
satisfies the finitely sorted embedding property (FSEP) if for all
![]() $(A,F_A),(B,F_B)\in {\operatorname {FSIm}}(G,F)$
, the condition
$(A,F_A),(B,F_B)\in {\operatorname {FSIm}}(G,F)$
, the condition
![]() ${\operatorname {Emb}}_{(G,F)}((A,F_A),(B,F_B))$
holds.
${\operatorname {Emb}}_{(G,F)}((A,F_A),(B,F_B))$
holds.
Example 2.20.
-
(1) If G is a profinite group having the embedding property, then the sorted profinite group
 $(G,F)$
has SEP where F is the full sorting data on G. For example, the free profinite group has the embedding property.
$(G,F)$
has SEP where F is the full sorting data on G. For example, the free profinite group has the embedding property.In general, for any profinite group H, there is an epimorphism
 $p:G\rightarrow H$
such that G has the embedding property. In this case, we call such a G an embedding cover. We say an embedding cover G of H is universal if for any embedding cover
$p:G\rightarrow H$
such that G has the embedding property. In this case, we call such a G an embedding cover. We say an embedding cover G of H is universal if for any embedding cover
 $G'$
of H and any epimorphisms
$G'$
of H and any epimorphisms
 $p:G\rightarrow H$
and
$p:G\rightarrow H$
and
 $p':G'\rightarrow H$
, there is an epimorphism
$p':G'\rightarrow H$
, there is an epimorphism
 $q:G'\rightarrow G$
such that
$q:G'\rightarrow G$
such that
 $p'=p\circ q$
. Moreover, any profinite group has a unique universal embedding cover and any finite group has a unique finite universal embedding cover (see [Reference Chatzidakis1, Theorem 2.7] and [Reference Haran and Lubotzky7, Theorem 1.12]).
$p'=p\circ q$
. Moreover, any profinite group has a unique universal embedding cover and any finite group has a unique finite universal embedding cover (see [Reference Chatzidakis1, Theorem 2.7] and [Reference Haran and Lubotzky7, Theorem 1.12]). -
(2) Let
 ${\mathcal {J}}=\{s_1,s_2\}$
. Put
${\mathcal {J}}=\{s_1,s_2\}$
. Put
 $J_{\subseteq }^*:{\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}\rightarrow {\mathcal {J}}^{<{\mathbb {N}}}, (k,J)\mapsto J^{\frown }(s_1,s_2)$
. Let
$J_{\subseteq }^*:{\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}\rightarrow {\mathcal {J}}^{<{\mathbb {N}}}, (k,J)\mapsto J^{\frown }(s_1,s_2)$
. Let
 $G={\mathbb {Z}}/2{\mathbb {Z}}\times {\mathbb {Z}}/2{\mathbb {Z}}$
, which has the embedding property as a profinite group. Then
$G={\mathbb {Z}}/2{\mathbb {Z}}\times {\mathbb {Z}}/2{\mathbb {Z}}$
, which has the embedding property as a profinite group. Then  $$ \begin{align*}{\mathcal{N}}(G)=\{0, G,N_{(1,1)}, N_{(1,0)}, N_{(0,1)} \}\end{align*} $$
$$ \begin{align*}{\mathcal{N}}(G)=\{0, G,N_{(1,1)}, N_{(1,0)}, N_{(0,1)} \}\end{align*} $$
where
 $N_a$
is the subgroup of G generated by a for
$N_a$
is the subgroup of G generated by a for
 $a\in G$
. Define a sorting data F on G as follows:
$a\in G$
. Define a sorting data F on G as follows:-
•
 $F(G)={\mathcal {J}}^{<{\mathbb {N}}}$
;
$F(G)={\mathcal {J}}^{<{\mathbb {N}}}$
; -
•
 $F(N_{(1,0)})=F(N_{(0,1)})=F(N_{(1,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
;
$F(N_{(1,0)})=F(N_{(0,1)})=F(N_{(1,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
; -
•
 $F(0)=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1,s_2\in ||J||\}$
.
$F(0)=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1,s_2\in ||J||\}$
.
Then,
 $(G,F)$
has SEP.
$(G,F)$
has SEP. -
Now, we show that the weaker notion of FSEP is actually equivalent to the notion of SEP.
Lemma 2.21. Let
![]() $(G,F)$
be a sorted profinite group having FSEP and let
$(G,F)$
be a sorted profinite group having FSEP and let
![]() $N,N'\in {\mathcal {N}}(G)$
with
$N,N'\in {\mathcal {N}}(G)$
with
![]() $G/N\cong G/N'$
. Then,
$G/N\cong G/N'$
. Then,
![]() $F(N)=F(N')$
.
$F(N)=F(N')$
.
Proof Take
![]() $N,N'\in {\mathcal {N}}(G)$
with
$N,N'\in {\mathcal {N}}(G)$
with
![]() $\varphi :G/N'\cong G/N$
. For a contradiction, suppose
$\varphi :G/N'\cong G/N$
. For a contradiction, suppose
![]() $F(N)\neq F(N')$
. Without loss of generality, we may assume that there is
$F(N)\neq F(N')$
. Without loss of generality, we may assume that there is
![]() $J'\in F(N')\setminus F(N)$
. Let
$J'\in F(N')\setminus F(N)$
. Let
![]() $A:=G/N$
,
$A:=G/N$
,
![]() $A':=G/N'$
, and let
$A':=G/N'$
, and let
![]() $\pi :G\rightarrow A$
and
$\pi :G\rightarrow A$
and
![]() $\pi ':G\rightarrow A'$
be the projections from G to A and
$\pi ':G\rightarrow A'$
be the projections from G to A and
![]() $A'$
. For each
$A'$
. For each
![]() $\tilde N\in {\mathcal {N}}(A)$
, choose
$\tilde N\in {\mathcal {N}}(A)$
, choose
Consider a pre-sorting data on A and
![]() $A'$
given as follows: For
$A'$
given as follows: For
![]() $\tilde N\in {\mathcal {N}}(A)$
,
$\tilde N\in {\mathcal {N}}(A)$
,
 $$ \begin{align*} \hat F_A(\tilde N)&:=\{\tilde J\},\\ \hat F_{A'}(\varphi^{-1}[\tilde N])&:=\begin{cases} \{\tilde J\},&\varphi^{-1}[\tilde N]\neq \{e_{A'}\},\\ \{\tilde J,J'\},&\varphi^{-1}[\tilde N]= \{e_{A'}\}. \end{cases} \end{align*} $$
$$ \begin{align*} \hat F_A(\tilde N)&:=\{\tilde J\},\\ \hat F_{A'}(\varphi^{-1}[\tilde N])&:=\begin{cases} \{\tilde J\},&\varphi^{-1}[\tilde N]\neq \{e_{A'}\},\\ \{\tilde J,J'\},&\varphi^{-1}[\tilde N]= \{e_{A'}\}. \end{cases} \end{align*} $$
Let
![]() $F_A$
and
$F_A$
and
![]() $F_{A'}$
be the sorting data generated by
$F_{A'}$
be the sorting data generated by
![]() $\hat F_A$
and
$\hat F_A$
and
![]() $\hat F_{A'}$
respectively. Then, clearly
$\hat F_{A'}$
respectively. Then, clearly
![]() $(A,F_A),(A',F_{A'})\in {\operatorname {FSIm}}(G,F)$
. Since
$(A,F_A),(A',F_{A'})\in {\operatorname {FSIm}}(G,F)$
. Since
![]() $(G,F)$
has FSEP, we have the following diagram:
$(G,F)$
has FSEP, we have the following diagram:

for a morphism
![]() $\psi :(G,F)\rightarrow (A',F_{A'})$
. So, we have that
$\psi :(G,F)\rightarrow (A',F_{A'})$
. So, we have that
 $$ \begin{align*} J'\in F_{A'}(\{e_{A'}\})&\subseteq F(\psi^{-1}[\{e_{A'}\}])\\ &=F(\psi^{-1}[\{\varphi^{-1}(e_A)\}])\\ &=F(\pi^{-1}[\{e_A\}])\\ &=F(N), \end{align*} $$
$$ \begin{align*} J'\in F_{A'}(\{e_{A'}\})&\subseteq F(\psi^{-1}[\{e_{A'}\}])\\ &=F(\psi^{-1}[\{\varphi^{-1}(e_A)\}])\\ &=F(\pi^{-1}[\{e_A\}])\\ &=F(N), \end{align*} $$
which contradicts the assumption that
![]() $J'\notin F(N)$
.
$J'\notin F(N)$
.
Theorem 2.22. For a sorted profinite group
![]() $(G,F)$
, the following are equivalent:
$(G,F)$
, the following are equivalent:
-
(1)
 $(G,F)$
has SEP.
$(G,F)$
has SEP. -
(2)
 $(G,F)$
has FSEP.
$(G,F)$
has FSEP. -
(3) G has EP and for all
 $N,N'\in {\mathcal {N}}(G)$
with
$N,N'\in {\mathcal {N}}(G)$
with
 $G/N\cong G/N'$
,
$G/N\cong G/N'$
,
 $F(N)=F(N')$
.
$F(N)=F(N')$
.
Proof It is clear that
![]() $(1)\Rightarrow (2)$
by definition and
$(1)\Rightarrow (2)$
by definition and
![]() $(2)\Rightarrow (3)$
by Lemma 2.21. It is enough to show that
$(2)\Rightarrow (3)$
by Lemma 2.21. It is enough to show that
![]() $(3)\Rightarrow (1)$
.
$(3)\Rightarrow (1)$
.
Take
![]() $(A,F_A)$
and
$(A,F_A)$
and
![]() $(B,F_B)$
in
$(B,F_B)$
in
![]() ${\operatorname {SIm}}(G,F)$
, and take two morphisms
${\operatorname {SIm}}(G,F)$
, and take two morphisms
![]() $\pi _A:(G,F)\rightarrow (A,F_A)$
and
$\pi _A:(G,F)\rightarrow (A,F_A)$
and
![]() $\pi :(B,F_B)\rightarrow (A,F_A)$
arbitrary. Since G has EP, there is an epimorphism
$\pi :(B,F_B)\rightarrow (A,F_A)$
arbitrary. Since G has EP, there is an epimorphism
![]() $\pi _B':G\rightarrow B$
such that
$\pi _B':G\rightarrow B$
such that

It is enough to show that
![]() $\pi _B'$
is sorted, that is, for each
$\pi _B'$
is sorted, that is, for each
![]() $N_B\in {\mathcal {N}}(B)$
,
$N_B\in {\mathcal {N}}(B)$
,
Since
![]() $(B,F_B)\in {\operatorname {SIm}}(G,F)$
, there is a morphism
$(B,F_B)\in {\operatorname {SIm}}(G,F)$
, there is a morphism
![]() $\pi _B:(G,F)\rightarrow (B,F_B)$
. Take
$\pi _B:(G,F)\rightarrow (B,F_B)$
. Take
![]() ${N_B\in {\mathcal {N}}(B)}$
arbitrary. Then, we have
${N_B\in {\mathcal {N}}(B)}$
arbitrary. Then, we have
and
![]() $F_B(N_B)\subseteq F(\pi _B^{-1}[N_B])$
. So, by Lemma 2.21,
$F_B(N_B)\subseteq F(\pi _B^{-1}[N_B])$
. So, by Lemma 2.21,
2.4 Sorted complete system
We recall the notion of sorted complete system from [Reference Hoffmann and Lee10, Section 3.2]. The sorted complete system of a sorted profinite group is a first order structure to encode the inverse system of finite quotients of a sorted profinite group by its open normal subgroups as the complete system of a profinite group encodes the inverse system of finite quotients of the profinite group by its open normal subgroups.
For each sorted profinite group in the category
![]() ${\operatorname {SPG}}$
, we associate a dual object, called a sorted complete system. Consider the following first order language
${\operatorname {SPG}}$
, we associate a dual object, called a sorted complete system. Consider the following first order language
![]() ${\mathcal {L}}_{SCS}({\mathcal {J}})$
with the sorts
${\mathcal {L}}_{SCS}({\mathcal {J}})$
with the sorts
![]() $m(k,J)$
for each
$m(k,J)$
for each
![]() $(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
together with:
$(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
together with:
-
• a family of binary relations
 $\le _{k,k',J,J'}$
and
$\le _{k,k',J,J'}$
and
 $C_{k,k',J,J'}$
; and
$C_{k,k',J,J'}$
; and -
• a family of ternary relations
 $P_{k,J}$
.
$P_{k,J}$
.
For a sorted profinite group
![]() $(G,F)$
, the sorted complete system
$(G,F)$
, the sorted complete system
![]() ${\mathcal {S}}(G,F)$
is an
${\mathcal {S}}(G,F)$
is an
![]() ${\mathcal {L}}_{SCS}({\mathcal {J}})$
-structure given as follows:
${\mathcal {L}}_{SCS}({\mathcal {J}})$
-structure given as follows:
-
• For
 $(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,
$(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,  $$ \begin{align*}m(k,J):=\bigcup_{N\in{\mathcal{N}}(G),[G:N]\le k,J\in F(N)} G/N\times \{k\}.\end{align*} $$
$$ \begin{align*}m(k,J):=\bigcup_{N\in{\mathcal{N}}(G),[G:N]\le k,J\in F(N)} G/N\times \{k\}.\end{align*} $$
-
• For
 $(k,J),(k',J')\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,
$(k,J),(k',J')\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,  $$ \begin{align*}\le_{k,k',J,J'}:=\{\left((gN,k),(g'N',k')\right)\in m(k,J)\times m(k',J'):k\ge k',N\subseteq N'\}.\end{align*} $$
$$ \begin{align*}\le_{k,k',J,J'}:=\{\left((gN,k),(g'N',k')\right)\in m(k,J)\times m(k',J'):k\ge k',N\subseteq N'\}.\end{align*} $$
-
• For
 $(k,J),(k',J')\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,
$(k,J),(k',J')\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,  $$ \begin{align*}C_{k,k',J,J'}\kern1.2pt{:=}\kern1.2pt\{\left((gN,k),(g'N',k')\right)\kern1.3pt{\in}\kern1.3pt m(k,J)\kern1.3pt{\times}\kern1.3pt m(k',J'):k\kern1.3pt{\ge}\kern1.3pt k',gN\kern1.3pt{\subseteq}\kern1.3pt g'N'\}\kern-1pt.\end{align*} $$
$$ \begin{align*}C_{k,k',J,J'}\kern1.2pt{:=}\kern1.2pt\{\left((gN,k),(g'N',k')\right)\kern1.3pt{\in}\kern1.3pt m(k,J)\kern1.3pt{\times}\kern1.3pt m(k',J'):k\kern1.3pt{\ge}\kern1.3pt k',gN\kern1.3pt{\subseteq}\kern1.3pt g'N'\}\kern-1pt.\end{align*} $$
-
• For
 $(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,
$(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,  $$ \begin{align*}P_{k,J}=\{ \left((g_1N,k),(g_2N,k),(g_3N,k) \right)\in m(k,J)^3:g_3N=g_1g_2N\}.\end{align*} $$
$$ \begin{align*}P_{k,J}=\{ \left((g_1N,k),(g_2N,k),(g_3N,k) \right)\in m(k,J)^3:g_3N=g_1g_2N\}.\end{align*} $$
If there is no confusion, we omit the subscripts and write
![]() $\le $
,C, and P. We also write
$\le $
,C, and P. We also write
![]() $gN$
for
$gN$
for
![]() $(gN,k)$
. Sorted complete systems are axiomatized by an
$(gN,k)$
. Sorted complete systems are axiomatized by an
![]() ${\mathcal {L}}_{SCS}({\mathcal {J}})$
-theory,
${\mathcal {L}}_{SCS}({\mathcal {J}})$
-theory,
![]() $SCS$
in [Reference Hoffmann and Lee10, Definition 3.7].
$SCS$
in [Reference Hoffmann and Lee10, Definition 3.7].
Conversely, any model S of
![]() $SCS$
is a sorted complete system of a sorted profinite group, denoted by
$SCS$
is a sorted complete system of a sorted profinite group, denoted by
![]() $(G(S),F(S))$
. Let
$(G(S),F(S))$
. Let
![]() $\sim $
be the equivalence relation on S given as follows: For
$\sim $
be the equivalence relation on S given as follows: For
![]() $a,b\in S$
,
$a,b\in S$
,
For
![]() $a\in S$
, let
$a\in S$
, let
![]() $[a]$
be the
$[a]$
be the
![]() $\sim $
-class of a. Then, for each
$\sim $
-class of a. Then, for each
![]() $a\in m(k,J)$
,
$a\in m(k,J)$
,
![]() $[a]\cap m(k,J)$
forms a group whose group operation is induced from P. The profinite group
$[a]\cap m(k,J)$
forms a group whose group operation is induced from P. The profinite group
![]() $G(S)$
is the inverse limit of the group
$G(S)$
is the inverse limit of the group
![]() $[a]\cap m(k,J)$
with the transition maps induced from C. Note that for each
$[a]\cap m(k,J)$
with the transition maps induced from C. Note that for each
![]() $N\in {\mathcal {N}}(G(S))$
, there is
$N\in {\mathcal {N}}(G(S))$
, there is
![]() $a\in m(k,J)$
such that N is the kernel of the projection from
$a\in m(k,J)$
such that N is the kernel of the projection from
![]() $G(S)$
to
$G(S)$
to
![]() $[a]\cap m(k,J)$
. In this case, we denote N by
$[a]\cap m(k,J)$
. In this case, we denote N by
![]() $N_a$
. We now associate the sorting data
$N_a$
. We now associate the sorting data
![]() $F(S)$
on
$F(S)$
on
![]() $G(S)$
as follows: For
$G(S)$
as follows: For
![]() $N\in {\mathcal {N}}(G(S))$
and
$N\in {\mathcal {N}}(G(S))$
and
![]() $J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,
$J\in {\mathcal {J}}^{<{\mathbb {N}}}$
,
Then, the sorted complete system of
![]() $(G(S),F(S))$
is naturally isomorphic to S. We write
$(G(S),F(S))$
is naturally isomorphic to S. We write
![]() ${\mathcal {G}}(S)$
for
${\mathcal {G}}(S)$
for
![]() $(G(S),F(S))$
.
$(G(S),F(S))$
.
Moreover, the associations
![]() $\mathcal S$
and
$\mathcal S$
and
![]() ${\mathcal {G}}$
define contravariant functors to make the category
${\mathcal {G}}$
define contravariant functors to make the category
![]() ${\operatorname {SPG}}$
of sorted profinite groups and the category of sorted complete systems whose morphisms are
${\operatorname {SPG}}$
of sorted profinite groups and the category of sorted complete systems whose morphisms are
![]() ${\mathcal {L}}_{SCS}({\mathcal {J}})$
-embeddings equivalent. For more detailed information, see [Reference Hoffmann and Lee10, Section 3.2].
${\mathcal {L}}_{SCS}({\mathcal {J}})$
-embeddings equivalent. For more detailed information, see [Reference Hoffmann and Lee10, Section 3.2].
If necessary, we write
![]() $m(k,J)(S)$
for
$m(k,J)(S)$
for
![]() $m(k,J)$
to emphasize the sort
$m(k,J)$
to emphasize the sort
![]() $m(k,J)$
in a model S of
$m(k,J)$
in a model S of
![]() $SCS$
.
$SCS$
.
3 Universal SEP-cover
Since the category
![]() ${\operatorname {SPG}}$
is closed under taking the inverse limit and the fibre product, we can transfer many arguments for profinite groups in [Reference Haran and Lubotzky7, Section 1] and in [Reference Fried and Jarden6, Section 24.4] into the case of sorted profinite groups after modifying several notions properly. In this section, we aim to show that any sorted profinite group
${\operatorname {SPG}}$
is closed under taking the inverse limit and the fibre product, we can transfer many arguments for profinite groups in [Reference Haran and Lubotzky7, Section 1] and in [Reference Fried and Jarden6, Section 24.4] into the case of sorted profinite groups after modifying several notions properly. In this section, we aim to show that any sorted profinite group
![]() $(G,F)$
has a universal SEP-cover, generalizing [Reference Haran and Lubotzky7, Theorem 1.12] and [Reference Fried and Jarden6, Proposition 24.4.5].
$(G,F)$
has a universal SEP-cover, generalizing [Reference Haran and Lubotzky7, Theorem 1.12] and [Reference Fried and Jarden6, Proposition 24.4.5].
Definition 3.1. We say that a morphism
![]() $p:(H,F_H)\rightarrow (G,F)$
is a SEP-cover if
$p:(H,F_H)\rightarrow (G,F)$
is a SEP-cover if
![]() $(H,F_H)$
has SEP.
$(H,F_H)$
has SEP.
Definition 3.2. Let
![]() $(G,F)$
be a sorted profinite group. A universal SEP-cover
$(G,F)$
be a sorted profinite group. A universal SEP-cover
![]() $p:(H,F_H)\rightarrow (G,F)$
is a SEP-cover satisfying the following property: For any SEP-cover
$p:(H,F_H)\rightarrow (G,F)$
is a SEP-cover satisfying the following property: For any SEP-cover
![]() $r:(H',F_{H'})\rightarrow (G,F)$
, there is a morphism
$r:(H',F_{H'})\rightarrow (G,F)$
, there is a morphism
![]() $q:(H',F_{H'})\rightarrow (H,F_{H})$
such that
$q:(H',F_{H'})\rightarrow (H,F_{H})$
such that
![]() $p\circ q=r$
.
$p\circ q=r$
.
Remark 3.3. Let
![]() $p:(G',F')\rightarrow (G,F)$
be a universal SEP-cover of a sorted profinite group
$p:(G',F')\rightarrow (G,F)$
be a universal SEP-cover of a sorted profinite group
![]() $(G,F)$
. Then,
$(G,F)$
. Then,
![]() $p:G'\rightarrow G$
is the universal embedding cover of G in the category of profinite groups. Namely, let
$p:G'\rightarrow G$
is the universal embedding cover of G in the category of profinite groups. Namely, let
![]() $q:G"\rightarrow G$
be an embedding cover. Let
$q:G"\rightarrow G$
be an embedding cover. Let
![]() $F"$
be the full sorting data on
$F"$
be the full sorting data on
![]() $G"$
. Then,
$G"$
. Then,
![]() $q:(G",F")\rightarrow (G,F)$
is a SEP-cover. Since
$q:(G",F")\rightarrow (G,F)$
is a SEP-cover. Since
![]() $p:(G',F')\rightarrow (G,F)$
is the universal SEP-cover, there is a morphism
$p:(G',F')\rightarrow (G,F)$
is the universal SEP-cover, there is a morphism
![]() ${r:(G",F")\rightarrow (G',F')}$
such that
${r:(G",F")\rightarrow (G',F')}$
such that
![]() $q=p\circ r:(G",F")\rightarrow (G,F)$
, which implies
$q=p\circ r:(G",F")\rightarrow (G,F)$
, which implies
![]() ${q=p\circ r:G"\rightarrow G}$
. Thus,
${q=p\circ r:G"\rightarrow G}$
. Thus,
![]() $p:G'\rightarrow G$
is the universal embedding cover of G.
$p:G'\rightarrow G$
is the universal embedding cover of G.
Before showing that any sorted profinite group has a universal SEP-cover, we first introduce two posets
![]() ${\mathcal {P}}$
and
${\mathcal {P}}$
and
![]() ${\mathcal {H}}$
(see [Reference Haran and Lubotzky7, p. 188] or [Reference Fried and Jarden6, Section 24.4]). Let
${\mathcal {H}}$
(see [Reference Haran and Lubotzky7, p. 188] or [Reference Fried and Jarden6, Section 24.4]). Let
![]() $(G_1,F_1)$
and
$(G_1,F_1)$
and
![]() $(G_2,F_2)$
be sorted profinite groups. We consider the following class of pairs of morphisms with common images:
$(G_2,F_2)$
be sorted profinite groups. We consider the following class of pairs of morphisms with common images:
 $$ \begin{align*} {\mathcal{P}}&:={\mathcal{P}}((G_1,F_1),(G_2,F_2))\\ &=\{(\Pi_1,\Pi_2):\Pi_1:(G_1,F_1)\rightarrow (A,F_A),\Pi_2:(G_2,F_2)\rightarrow (A,F_A)\} \end{align*} $$
$$ \begin{align*} {\mathcal{P}}&:={\mathcal{P}}((G_1,F_1),(G_2,F_2))\\ &=\{(\Pi_1,\Pi_2):\Pi_1:(G_1,F_1)\rightarrow (A,F_A),\Pi_2:(G_2,F_2)\rightarrow (A,F_A)\} \end{align*} $$
and define a pre-order
![]() $\le $
on
$\le $
on
![]() ${\mathcal {P}}$
as follows: For
${\mathcal {P}}$
as follows: For
![]() $(\Pi _1,\Pi _2), (\Pi _1',\Pi _2')\in {\mathcal {P}}$
, we write
$(\Pi _1,\Pi _2), (\Pi _1',\Pi _2')\in {\mathcal {P}}$
, we write
![]() $(\Pi _1,\Pi _2)\le (\Pi _1',\Pi _2')$
if there is a morphism
$(\Pi _1,\Pi _2)\le (\Pi _1',\Pi _2')$
if there is a morphism
![]() $\Pi :(A',F_{A'})\rightarrow (A,F_A)$
such that the following diagram is commutative:
$\Pi :(A',F_{A'})\rightarrow (A,F_A)$
such that the following diagram is commutative:

We write
![]() $(\Pi _1,\Pi _2)\approx (\Pi _1',\Pi _2')$
if
$(\Pi _1,\Pi _2)\approx (\Pi _1',\Pi _2')$
if
![]() $(\Pi _1,\Pi _2)\le (\Pi _1',\Pi _2')$
and
$(\Pi _1,\Pi _2)\le (\Pi _1',\Pi _2')$
and
![]() $(\Pi _1,\Pi _2)\ge (\Pi _1',\Pi _2')$
. Then, the relation
$(\Pi _1,\Pi _2)\ge (\Pi _1',\Pi _2')$
. Then, the relation
![]() $\approx $
is an equivalence relation on
$\approx $
is an equivalence relation on
![]() ${\mathcal {P}}$
and
${\mathcal {P}}$
and
![]() $\le $
gives a partial order, still denoted by
$\le $
gives a partial order, still denoted by
![]() $\le $
, on the quotient set
$\le $
, on the quotient set
![]() ${\mathcal {P}}/\approx $
.
${\mathcal {P}}/\approx $
.
Remark 3.4.
![]() $(\Pi _1,\Pi _2)\approx (\Pi _1',\Pi _2')$
if and only if
$(\Pi _1,\Pi _2)\approx (\Pi _1',\Pi _2')$
if and only if
![]() $\Pi $
is an isomorphism.
$\Pi $
is an isomorphism.
Proof It is enough to show that the left-to-right implication holds. Suppose
![]() $(\Pi _1,\Pi _2)\approx (\Pi _1',\Pi _2')$
and
$(\Pi _1,\Pi _2)\approx (\Pi _1',\Pi _2')$
and
![]() $\Pi $
is not an isomorphism. First, note that
$\Pi $
is not an isomorphism. First, note that
![]() $\Pi $
is bijective. Let
$\Pi $
is bijective. Let
![]() $F:=\Pi _*(F_{A'})$
be the push-forward sorting data on A. Since
$F:=\Pi _*(F_{A'})$
be the push-forward sorting data on A. Since
![]() $\Pi $
is not an isomorphism,
$\Pi $
is not an isomorphism,
![]() $F_A\subset F$
. Put
$F_A\subset F$
. Put
![]() $\Pi _1":=\Pi \circ \Pi _1':(G_1,F_1)\rightarrow (A,F)$
and
$\Pi _1":=\Pi \circ \Pi _1':(G_1,F_1)\rightarrow (A,F)$
and
![]() $\Pi _2":=\Pi \circ \Pi _2'$
. Since
$\Pi _2":=\Pi \circ \Pi _2'$
. Since
![]() $\Pi :(A',F_{A'})\rightarrow (A,F)$
is an isomorphism,
$\Pi :(A',F_{A'})\rightarrow (A,F)$
is an isomorphism,
So, there is a morphism
![]() $\Pi ':(A,F_A)\rightarrow (A,F)$
such that
$\Pi ':(A,F_A)\rightarrow (A,F)$
such that
![]() $\Pi '\circ \Pi _1=\Pi _1$
and
$\Pi '\circ \Pi _1=\Pi _1$
and
![]() $\Pi '\circ \Pi _2=\Pi _2$
, which implies that
$\Pi '\circ \Pi _2=\Pi _2$
, which implies that
![]() $\Pi '$
is the identity map. Thus, we have that
$\Pi '$
is the identity map. Thus, we have that
![]() $F\subseteq F_A$
, a contradiction.
$F\subseteq F_A$
, a contradiction.
We introduce a dual notion to
![]() ${\mathcal {P}}$
. Let
${\mathcal {P}}$
. Let
![]() $(G_1,F_1)\times (G_2,F_2)$
be the fibre product of
$(G_1,F_1)\times (G_2,F_2)$
be the fibre product of
![]() $(G_1,F_1)$
and
$(G_1,F_1)$
and
![]() $(G_2,F_2)$
over the trivial group. Let
$(G_2,F_2)$
over the trivial group. Let
![]() $p_i:(G_1,F_1)\times (G_2,F_2)\rightarrow (G_i,F_i)$
for
$p_i:(G_1,F_1)\times (G_2,F_2)\rightarrow (G_i,F_i)$
for
![]() $i=1,2$
be the canonical projection. Put
$i=1,2$
be the canonical projection. Put
 $$ \begin{align*} {\mathcal{H}}&:={\mathcal{H}}((G_1,F_1),(G_2,F_2))\\ &=\{\left((H,F_H),\Pi_1,\Pi_2\right):H\le G_1\times G_2, p_i(H)=G_i, i=1,2\} \end{align*} $$
$$ \begin{align*} {\mathcal{H}}&:={\mathcal{H}}((G_1,F_1),(G_2,F_2))\\ &=\{\left((H,F_H),\Pi_1,\Pi_2\right):H\le G_1\times G_2, p_i(H)=G_i, i=1,2\} \end{align*} $$
such that:
-
•
 $p_{i}:(H,F_H)\rightarrow (G_i,F_i)$
is a morphism for
$p_{i}:(H,F_H)\rightarrow (G_i,F_i)$
is a morphism for
 $i=1,2$
.
$i=1,2$
. -
•
 $(\Pi _1,\Pi _2)\in {\mathcal {P}}$
with common an image
$(\Pi _1,\Pi _2)\in {\mathcal {P}}$
with common an image
 $(A,F_A)$
;
$(A,F_A)$
; -
• The following diagram is Cartesian:

By Remark 2.17,
![]() ${\mathcal {H}}$
is not empty. We define a pre-order
${\mathcal {H}}$
is not empty. We define a pre-order
![]() $\le '$
on
$\le '$
on
![]() ${\mathcal {H}}$
as follows:
${\mathcal {H}}$
as follows:
if:
-
•
 $(\Pi _1',\Pi _2')\le (\Pi _1,\Pi _2)$
;
$(\Pi _1',\Pi _2')\le (\Pi _1,\Pi _2)$
; -
•
 $H\subseteq H'$
.
$H\subseteq H'$
.
Note that
![]() $F_H=F_{H'}$
if
$F_H=F_{H'}$
if
![]() $H=H'$
because H and
$H=H'$
because H and
![]() $H'$
are fibre products. We write
$H'$
are fibre products. We write
![]() $\left ((H,F_H),\Pi _1,\Pi _2\right )\approx ' \left ((H',F_{H'}),\Pi _1',\Pi _2'\right )$
if
$\left ((H,F_H),\Pi _1,\Pi _2\right )\approx ' \left ((H',F_{H'}),\Pi _1',\Pi _2'\right )$
if
![]() $H=H'$
and
$H=H'$
and
![]() $(\Pi _1,\Pi _2)\approx (\Pi _1',\Pi _2')$
, that is,
$(\Pi _1,\Pi _2)\approx (\Pi _1',\Pi _2')$
, that is,
![]() $((H,F_H),\Pi _1,\Pi _2)\le ' ((H',F_{H'}),\Pi _1',\Pi _2')$
and
$((H,F_H),\Pi _1,\Pi _2)\le ' ((H',F_{H'}),\Pi _1',\Pi _2')$
and
![]() $((H',F_{H'}),\Pi _1',\Pi _2')\le ' ((H,F_H),\Pi _1,\Pi _2)$
. Then, the relation
$((H',F_{H'}),\Pi _1',\Pi _2')\le ' ((H,F_H),\Pi _1,\Pi _2)$
. Then, the relation
![]() $\approx '$
is an equivalence relation on
$\approx '$
is an equivalence relation on
![]() ${\mathcal {H}}$
and
${\mathcal {H}}$
and
![]() $\le '$
gives a partial order on the quotient set
$\le '$
gives a partial order on the quotient set
![]() ${\mathcal {H}}/\approx '$
.
${\mathcal {H}}/\approx '$
.
Now we define a map
![]() $T:{\mathcal {P}}\rightarrow {\mathcal {H}}$
given as follows: For
$T:{\mathcal {P}}\rightarrow {\mathcal {H}}$
given as follows: For
![]() $(\Pi _1,\Pi _2)\in {\mathcal {P}}$
with
$(\Pi _1,\Pi _2)\in {\mathcal {P}}$
with
![]() $(A,F_A)={\operatorname {Im}} \Pi _1={\operatorname {Im}} \Pi _2$
, let
$(A,F_A)={\operatorname {Im}} \Pi _1={\operatorname {Im}} \Pi _2$
, let
The map T induces a map from
![]() ${\mathcal {P}}/\approx $
to
${\mathcal {P}}/\approx $
to
![]() ${\mathcal {H}}/\approx '$
. By abusing notation, we denote
${\mathcal {H}}/\approx '$
. By abusing notation, we denote
![]() ${\mathcal {P}}/\approx $
,
${\mathcal {P}}/\approx $
,
![]() ${\mathcal {H}}/\approx '$
, and the induced map by
${\mathcal {H}}/\approx '$
, and the induced map by
![]() ${\mathcal {P}}$
,
${\mathcal {P}}$
,
![]() ${\mathcal {H}}$
, and T respectively. Note that the map T is an order-reversing injection by definition. Then, we have a result analogous to [Reference Haran and Lubotzky7, Lemma 1.7] using Remark 2.17.
${\mathcal {H}}$
, and T respectively. Note that the map T is an order-reversing injection by definition. Then, we have a result analogous to [Reference Haran and Lubotzky7, Lemma 1.7] using Remark 2.17.
Lemma 3.5 [Reference Haran and Lubotzky7, Lemma 1.7].
The map T induces an order-reversing bijection between two posets
![]() ${\mathcal {P}}/\approx $
and
${\mathcal {P}}/\approx $
and
![]() ${\mathcal {H}}/\approx '$
.
${\mathcal {H}}/\approx '$
.
Remark 3.6. Let
![]() $(G_1,F_1)$
and
$(G_1,F_1)$
and
![]() $(G_2,F_2)$
be sorted profinite groups. Let
$(G_2,F_2)$
be sorted profinite groups. Let
![]() $p_i:G_1\times G_2\rightarrow G_i$
be the canonical projection for
$p_i:G_1\times G_2\rightarrow G_i$
be the canonical projection for
![]() $i=1,2$
. Let
$i=1,2$
. Let
![]() $H'\le H\le G_1\times G_2$
such that
$H'\le H\le G_1\times G_2$
such that
![]() $p_i[H]=G_i$
and
$p_i[H]=G_i$
and
![]() $p_i[H']=G_i$
for
$p_i[H']=G_i$
for
![]() $i=1,2$
. For any
$i=1,2$
. For any
![]() $((H,F),\Pi _1,\Pi _2)\in {\mathcal {H}}$
, there is
$((H,F),\Pi _1,\Pi _2)\in {\mathcal {H}}$
, there is
![]() $(\Pi _1',\Pi _2')$
in
$(\Pi _1',\Pi _2')$
in
![]() ${\mathcal {P}}$
such that
${\mathcal {P}}$
such that
where
![]() $(H',F')$
is the fibre product of
$(H',F')$
is the fibre product of
![]() $(G_1,F_1)$
and
$(G_1,F_1)$
and
![]() $(G_2,F_2)$
along
$(G_2,F_2)$
along
![]() $\Pi _1'$
and
$\Pi _1'$
and
![]() $\Pi _2'$
.
$\Pi _2'$
.
Proof Since
![]() $H'\le H$
, by [Reference Haran and Lubotzky7, Lemma 1.7], there are epimorphisms
$H'\le H$
, by [Reference Haran and Lubotzky7, Lemma 1.7], there are epimorphisms
![]() $\Pi _1'$
and
$\Pi _1'$
and
![]() $\Pi _2'$
with
$\Pi _2'$
with
![]() ${\operatorname {Im}}(\Pi _1')={\operatorname {Im}}(\Pi _2')=:A'$
and an epimorphism
${\operatorname {Im}}(\Pi _1')={\operatorname {Im}}(\Pi _2')=:A'$
and an epimorphism
![]() $\Pi :A'\rightarrow A$
such that the following diagram is commutative:
$\Pi :A'\rightarrow A$
such that the following diagram is commutative:

where the square is Cartesian. Let
![]() $F_{A'}$
be a sorting data on
$F_{A'}$
be a sorting data on
![]() $A'$
such that all epimorphisms
$A'$
such that all epimorphisms
![]() $\Pi _1'$
,
$\Pi _1'$
,
![]() $\Pi _2'$
, and
$\Pi _2'$
, and
![]() $\Pi $
are sorted. Let
$\Pi $
are sorted. Let
![]() $F'$
be the sorting data on
$F'$
be the sorting data on
![]() $H'$
such that
$H'$
such that
![]() $(H',F')$
is the fibre product of
$(H',F')$
is the fibre product of
![]() $(G_1,F_1)$
and
$(G_1,F_1)$
and
![]() $(G_2,F_2)$
over
$(G_2,F_2)$
over
![]() $(A',F_{A'})$
. Then, by the choice of
$(A',F_{A'})$
. Then, by the choice of
![]() $F'$
and
$F'$
and
![]() $F_{A'}$
, we have that
$F_{A'}$
, we have that
Using Zorn’s Lemma with the inverse limit, we have the following result:
Lemma 3.7 [Reference Haran and Lubotzky7, Lemma 1.8].
For every
![]() $(\Pi _1,\Pi _2)\in {\mathcal {P}}$
, there is a maximal element
$(\Pi _1,\Pi _2)\in {\mathcal {P}}$
, there is a maximal element
![]() $(\Pi _1',\Pi _2')\in {\mathcal {P}}$
such that
$(\Pi _1',\Pi _2')\in {\mathcal {P}}$
such that
![]() $(\Pi _1,\Pi _2)\le (\Pi _1',\Pi _2')$
.
$(\Pi _1,\Pi _2)\le (\Pi _1',\Pi _2')$
.
We introduce a notion of the quasi SEP-cover for sorted profinite groups, analogous to the quasi-embedding cover of profinite groups in [Reference Haran and Lubotzky7, p. 189] or the I-cover in [Reference Fried and Jarden6, Definition 24.4.3].
Definition 3.8. A morphism
![]() $p:(H,F_H)\rightarrow (G,F)$
is called a quasi SEP-cover (q.s.c.) if for every SEP-cover
$p:(H,F_H)\rightarrow (G,F)$
is called a quasi SEP-cover (q.s.c.) if for every SEP-cover
![]() $\varphi :(E,F_E)\rightarrow (G,F)$
, there is a morphism
$\varphi :(E,F_E)\rightarrow (G,F)$
, there is a morphism
![]() $\psi :(E,F_E)\rightarrow (H,F_H)$
such that
$\psi :(E,F_E)\rightarrow (H,F_H)$
such that
![]() $p\circ \psi =\varphi $
.
$p\circ \psi =\varphi $
.
Remark 3.9. Let
![]() $(G,F)$
be a sorted profinite group.
$(G,F)$
be a sorted profinite group.
-
(1) For two morphisms
 $p:(H,F_H)\rightarrow (G,F)$
and
$p:(H,F_H)\rightarrow (G,F)$
and
 $\Pi :(G,F)\rightarrow (A,F_A)$
of sorted profinite groups, if both p and
$\Pi :(G,F)\rightarrow (A,F_A)$
of sorted profinite groups, if both p and
 $\Pi $
are q.s.c., then
$\Pi $
are q.s.c., then
 $\Pi \circ p$
is a q.s.c.
$\Pi \circ p$
is a q.s.c. -
(2) There is a cardinal
 $\kappa $
depending only on the cardinality of G such that for any q.s.c.
$\kappa $
depending only on the cardinality of G such that for any q.s.c.
 $p:(H,F_H)\rightarrow (G,F)$
, the cardinality of H is less than
$p:(H,F_H)\rightarrow (G,F)$
, the cardinality of H is less than
 $\kappa $
. Furthermore, if G is finite, we can take
$\kappa $
. Furthermore, if G is finite, we can take
 $\kappa $
as finite.
$\kappa $
as finite. -
(3) Let
 $p:(H,F_H)\rightarrow (G,F)$
be a q.s.c. which is a SEP-cover. Then, p is a universal SEP-cover.
$p:(H,F_H)\rightarrow (G,F)$
be a q.s.c. which is a SEP-cover. Then, p is a universal SEP-cover.
From the proof of [Reference Fried and Jarden6, Lemma 24.4.4], we have the following result.
Lemma 3.10. Let
![]() $(G,F)$
be a sorted profinite group and let
$(G,F)$
be a sorted profinite group and let
![]() $(B,F_B)\in {\operatorname {SIm}}(G,F)$
. Let
$(B,F_B)\in {\operatorname {SIm}}(G,F)$
. Let
![]() $(\Pi _1,\Pi _2)\in {\mathcal {P}}((B,F_B),(G,F))$
be a maximal element. Consider the following Cartesian diagram induced from
$(\Pi _1,\Pi _2)\in {\mathcal {P}}((B,F_B),(G,F))$
be a maximal element. Consider the following Cartesian diagram induced from
![]() $(\Pi _1,\Pi _2)$
:
$(\Pi _1,\Pi _2)$
:

Then,
![]() $p_2$
is a q.s.c.
$p_2$
is a q.s.c.
Proof Let
![]() $\psi _2:(G',F')\rightarrow (G,F)$
be a SEP-cover. Then,
$\psi _2:(G',F')\rightarrow (G,F)$
be a SEP-cover. Then,
![]() $(B,F_B)$
is in
$(B,F_B)$
is in
![]() ${\operatorname {SIm}}(G',F')$
. Since
${\operatorname {SIm}}(G',F')$
. Since
![]() $(G',F')$
has SEP, there is a morphism
$(G',F')$
has SEP, there is a morphism
![]() $\psi _1:(G',F')\rightarrow (B,F_B)$
such that
$\psi _1:(G',F')\rightarrow (B,F_B)$
such that
![]() $\Pi _1\circ \psi _1=\Pi _2 \circ \psi _2$
. Since H is a fibre product of B and G over A, by Remark 2.15(2), there is a homomorphism
$\Pi _1\circ \psi _1=\Pi _2 \circ \psi _2$
. Since H is a fibre product of B and G over A, by Remark 2.15(2), there is a homomorphism
![]() $\psi : G'\rightarrow H$
such that the following diagram is commutative:
$\psi : G'\rightarrow H$
such that the following diagram is commutative:

Since
![]() $\psi [G']\le H$
and
$\psi [G']\le H$
and
![]() $((H,F_H),\Pi _1,\Pi _2)$
is minimal, we have that
$((H,F_H),\Pi _1,\Pi _2)$
is minimal, we have that
![]() $\psi [G']=H$
so that
$\psi [G']=H$
so that
![]() $\psi $
is an epimorphism. Indeed, if
$\psi $
is an epimorphism. Indeed, if
![]() $\psi [G']\lneq H$
, then by Remark 3.6, there is
$\psi [G']\lneq H$
, then by Remark 3.6, there is
![]() $(\Pi _1',\Pi _2')$
in
$(\Pi _1',\Pi _2')$
in
![]() $\mathcal P$
such that
$\mathcal P$
such that
![]() $((\psi [G'],F_{\psi [G']}),\Pi _1',\Pi _2')\lneq ' ((H,F_H),\Pi _1,\Pi _2)$
, where
$((\psi [G'],F_{\psi [G']}),\Pi _1',\Pi _2')\lneq ' ((H,F_H),\Pi _1,\Pi _2)$
, where
![]() $(\psi [G'],F_{\psi [G']})$
is the fibre product of
$(\psi [G'],F_{\psi [G']})$
is the fibre product of
![]() $(B,F_B)$
and
$(B,F_B)$
and
![]() $(G,F)$
along
$(G,F)$
along
![]() $\Pi _1'$
and
$\Pi _1'$
and
![]() $\Pi _2'$
. This contradicts the minimality of
$\Pi _2'$
. This contradicts the minimality of
![]() $((H,F_H),\Pi _1,\Pi _2)$
.
$((H,F_H),\Pi _1,\Pi _2)$
.
We will show that
![]() $\psi $
is sorted. Since
$\psi $
is sorted. Since
![]() $(H,F_H)$
is the fibre product of
$(H,F_H)$
is the fibre product of
![]() $(B,F_B)$
and
$(B,F_B)$
and
![]() $(G,F)$
, the sorting data
$(G,F)$
, the sorting data
![]() $F_H$
is generated by the following pre-sorting data
$F_H$
is generated by the following pre-sorting data
![]() $F_X$
(see Definition 2.14):
$F_X$
(see Definition 2.14):
-
•
 $X=\{p_1^{-1}[N_1]:N_1\in {\mathcal {N}}(B)\}\cup \{p_2^{-1}[N_2]:N_2\in {\mathcal {N}}(G)\}$
.
$X=\{p_1^{-1}[N_1]:N_1\in {\mathcal {N}}(B)\}\cup \{p_2^{-1}[N_2]:N_2\in {\mathcal {N}}(G)\}$
. -
• For
 $N_1\in {\mathcal {N}}(B)$
and
$N_1\in {\mathcal {N}}(B)$
and
 $N_2\in {\mathcal {N}}(G)$
,
$N_2\in {\mathcal {N}}(G)$
,  $$ \begin{align*}F_X(p_1^{-1}[N_1])=F_1(N_1),\ F_X(p_2^{-1}[N_2])=F_2(N_2).\end{align*} $$
$$ \begin{align*}F_X(p_1^{-1}[N_1])=F_1(N_1),\ F_X(p_2^{-1}[N_2])=F_2(N_2).\end{align*} $$
Since
![]() $\psi _1$
and
$\psi _1$
and
![]() $\psi _2$
are sorted, for
$\psi _2$
are sorted, for
![]() $N_1\in {\mathcal {N}}(B)$
and
$N_1\in {\mathcal {N}}(B)$
and
![]() $N_2\in {\mathcal {N}}(G)$
,
$N_2\in {\mathcal {N}}(G)$
,
Since
![]() $\psi _1=p_1\circ \psi $
and
$\psi _1=p_1\circ \psi $
and
![]() $\psi _2=p_2\circ \psi $
, we have that for
$\psi _2=p_2\circ \psi $
, we have that for
![]() $N_1\in {\mathcal {N}}(B)$
and
$N_1\in {\mathcal {N}}(B)$
and
![]() $N_2\in {\mathcal {N}}(G)$
,
$N_2\in {\mathcal {N}}(G)$
,
 $$ \begin{align*} F'(\psi^{-1}[p_1^{-1}[N_1]])&=F'(\psi_1^{-1}[N_1])\\ &\supseteq F_1(N_1)\\ &=F_H(p_1^{-1}[N_1]), \end{align*} $$
$$ \begin{align*} F'(\psi^{-1}[p_1^{-1}[N_1]])&=F'(\psi_1^{-1}[N_1])\\ &\supseteq F_1(N_1)\\ &=F_H(p_1^{-1}[N_1]), \end{align*} $$
and
 $$ \begin{align*} F'(\psi^{-1}[p_2^{-1}[N_2]])&=F'(\psi_2^{-1}[N_2])\\ &\supseteq F_2(N_2)\\ &=F_H(p_2^{-1}[N_2]), \end{align*} $$
$$ \begin{align*} F'(\psi^{-1}[p_2^{-1}[N_2]])&=F'(\psi_2^{-1}[N_2])\\ &\supseteq F_2(N_2)\\ &=F_H(p_2^{-1}[N_2]), \end{align*} $$
which implies
![]() $\psi $
is sorted because
$\psi $
is sorted because
![]() $F_H$
is generated by
$F_H$
is generated by
![]() $F_X$
. Therefore, we have that
$F_X$
. Therefore, we have that
![]() $\psi _2=p_2\circ \psi $
for a morphism
$\psi _2=p_2\circ \psi $
for a morphism
![]() $\psi $
, and
$\psi $
, and
![]() $p_2$
is a q.s.c.
$p_2$
is a q.s.c.
We have the following characterization of sorted profinite groups not having SEP, analogous to [Reference Fried and Jarden6, Lemma 24.4.4].
Lemma 3.11. If a sorted profinite group
![]() $(G,F)$
does not have SEP, then, either:
$(G,F)$
does not have SEP, then, either:
-
(1) there exists a q.s.c.
 $p:(H,F_H)\rightarrow (G,F)$
with a non-trivial kernel, or
$p:(H,F_H)\rightarrow (G,F)$
with a non-trivial kernel, or -
(2) there is a q.s.c.
 ${\operatorname {id}}:(G,F')\rightarrow (G,F)$
with
${\operatorname {id}}:(G,F')\rightarrow (G,F)$
with
 $F\subset F'$
.
$F\subset F'$
.
Proof Suppose a sorted profinite group
![]() $(G,F)$
does not have SEP. So, there exist:
$(G,F)$
does not have SEP. So, there exist:
-
•
 $(A,F_A),(B,F_B)\in {\operatorname {SIm}}(G,F)$
; and
$(A,F_A),(B,F_B)\in {\operatorname {SIm}}(G,F)$
; and -
• morphisms
 $\pi _1:(B,F_B)\rightarrow (A,F_A)$
and
$\pi _1:(B,F_B)\rightarrow (A,F_A)$
and
 $\pi _2 :(G,F)\rightarrow (A,F_A)$
,
$\pi _2 :(G,F)\rightarrow (A,F_A)$
,
such that there is no morphism
![]() $p:(G,F)\rightarrow (B,F_B)$
with
$p:(G,F)\rightarrow (B,F_B)$
with
![]() $\pi _2=\pi _1\circ p$
. By Lemma 3.7, there is a maximal element
$\pi _2=\pi _1\circ p$
. By Lemma 3.7, there is a maximal element
![]() $(\Pi _1,\Pi _2)\in {\mathcal {P}}((B,F_B),(G,F))$
such that
$(\Pi _1,\Pi _2)\in {\mathcal {P}}((B,F_B),(G,F))$
such that
![]() $(\pi _1,\pi _2)\le (\Pi _1,\Pi _2)$
. Then, we have the following diagram:
$(\pi _1,\pi _2)\le (\Pi _1,\Pi _2)$
. Then, we have the following diagram:

where
![]() $(H,F_H)$
is the fibre product of
$(H,F_H)$
is the fibre product of
![]() $(B,F_B)$
and
$(B,F_B)$
and
![]() $(G,F)$
over
$(G,F)$
over
![]() $(A',F_{A'})$
.
$(A',F_{A'})$
.
Note that
![]() $p_2$
is a q.s.c. by Lemma 3.10. Suppose
$p_2$
is a q.s.c. by Lemma 3.10. Suppose
![]() $p_2$
is an isomorphism. Let
$p_2$
is an isomorphism. Let
![]() $p=p_1\circ p_2^{-1}$
. Then, we have that
$p=p_1\circ p_2^{-1}$
. Then, we have that
 $$ \begin{align*} \pi_1\circ p&=\pi_1\circ (p_1\circ p_2^{-1})\\ &=\left ((\pi\circ \Pi_1)\circ p_1\right) \circ p_2^{-1}\\ &=\pi\circ (\Pi_2\circ p_2)\circ p_2^{-1}\\ &=\pi\circ \Pi_2\\ &=\pi_2, \end{align*} $$
$$ \begin{align*} \pi_1\circ p&=\pi_1\circ (p_1\circ p_2^{-1})\\ &=\left ((\pi\circ \Pi_1)\circ p_1\right) \circ p_2^{-1}\\ &=\pi\circ (\Pi_2\circ p_2)\circ p_2^{-1}\\ &=\pi\circ \Pi_2\\ &=\pi_2, \end{align*} $$
which is a contradiction. So,
![]() $p_2$
is not an isomorphism. If
$p_2$
is not an isomorphism. If
![]() $p_2$
has a non-trivial kernel, then
$p_2$
has a non-trivial kernel, then
![]() $p_2$
is the desired q.s.c. Suppose
$p_2$
is the desired q.s.c. Suppose
![]() $p_2$
has trivial kernel. Consider a sorting data
$p_2$
has trivial kernel. Consider a sorting data
![]() $F'$
on G given as follows: For
$F'$
on G given as follows: For
![]() $N\in {\mathcal {N}}(G)$
,
$N\in {\mathcal {N}}(G)$
,
Then,
![]() $p_2 : (H,F_H)\rightarrow (G,F')$
is an isomorphism and a q.s.c. So, by Remark 3.9(1),
$p_2 : (H,F_H)\rightarrow (G,F')$
is an isomorphism and a q.s.c. So, by Remark 3.9(1),
![]() ${\operatorname {id}}=p_2^{-1}\circ p_2 :(G,F')\rightarrow (H,F_H)\rightarrow (G,F)$
is a q.s.c. with
${\operatorname {id}}=p_2^{-1}\circ p_2 :(G,F')\rightarrow (H,F_H)\rightarrow (G,F)$
is a q.s.c. with
![]() $F\subset F'$
.
$F\subset F'$
.
Motivated by the proof of [Reference Haran and Lubotzky7, Theorem 1.12], we provide the following lemma.
Lemma 3.12. For an ordinal
![]() $\alpha $
, consider an inverse system
$\alpha $
, consider an inverse system
![]() $((G_i,F_i))_i$
indexed by ordinals
$((G_i,F_i))_i$
indexed by ordinals
![]() $i< \alpha $
such that:
$i< \alpha $
such that:
-
• For each
 $i<j$
, the transition map
$i<j$
, the transition map
 $\pi _{j,i}$
is a q.s.c.
$\pi _{j,i}$
is a q.s.c. -
• For each limit ordinal
 $\beta $
,
$\beta $
,
 $(G_{\beta },F_{\beta })$
is the inverse limit of the inverse system
$(G_{\beta },F_{\beta })$
is the inverse limit of the inverse system
 $((G_i,F_i))_{i<\beta }$
with transition maps
$((G_i,F_i))_{i<\beta }$
with transition maps
 $\pi _{j,i}$
for
$\pi _{j,i}$
for
 $i<j<\beta $
.
$i<j<\beta $
. -
• For each limit ordinal
 $\beta $
and for
$\beta $
and for
 $i<\beta $
, the transition map
$i<\beta $
, the transition map
 $\pi _{\beta ,i}$
is the natural projection from
$\pi _{\beta ,i}$
is the natural projection from
 $(G_{\beta },F_{\beta })$
to
$(G_{\beta },F_{\beta })$
to
 $(G_i,F_i)$
coming from the inverse limit construction.
$(G_i,F_i)$
coming from the inverse limit construction.
Let
![]() $(G,F)$
be the inverse limit of
$(G,F)$
be the inverse limit of
![]() $((G_i,F_i))_{i<\alpha }$
and let
$((G_i,F_i))_{i<\alpha }$
and let
![]() $\pi _i:(G,F)\rightarrow (G_i,F_i)$
be the canonical projection for each
$\pi _i:(G,F)\rightarrow (G_i,F_i)$
be the canonical projection for each
![]() $i<\alpha $
. Then,
$i<\alpha $
. Then,
![]() $\pi _0$
is a q.s.c.
$\pi _0$
is a q.s.c.
Proof If
![]() $\alpha $
is a successor ordinal, that is,
$\alpha $
is a successor ordinal, that is,
![]() $\alpha =\alpha '+1$
, then
$\alpha =\alpha '+1$
, then
![]() $(G,F)=(G_{\alpha '},F_{\alpha '})$
and we are done. We assume that
$(G,F)=(G_{\alpha '},F_{\alpha '})$
and we are done. We assume that
![]() $\alpha $
is a limit ordinal. Let
$\alpha $
is a limit ordinal. Let
![]() $p:(G',F')\rightarrow (G_0,F_0)$
be a SEP-cover. To show that
$p:(G',F')\rightarrow (G_0,F_0)$
be a SEP-cover. To show that
![]() $\pi $
is a q.s.c., using transfinite induction, we will construct a sequence
$\pi $
is a q.s.c., using transfinite induction, we will construct a sequence
![]() $(p_i:(G',F')\rightarrow (G_i,F_i))_{i<\alpha }$
of morphisms such that for each
$(p_i:(G',F')\rightarrow (G_i,F_i))_{i<\alpha }$
of morphisms such that for each
![]() $0\le i<j<\alpha $
,
$0\le i<j<\alpha $
,
![]() $p_i=\pi _{j,i}\circ p_j$
. Put
$p_i=\pi _{j,i}\circ p_j$
. Put
![]() $p_0:=p$
. Suppose we have constructed
$p_0:=p$
. Suppose we have constructed
![]() $(p_i)_{i< \gamma }$
for some
$(p_i)_{i< \gamma }$
for some
![]() $\gamma <\alpha $
. If
$\gamma <\alpha $
. If
![]() $\gamma $
is a limit ordinal, there is a desired morphism
$\gamma $
is a limit ordinal, there is a desired morphism
![]() $p_{\gamma }:(G',F')\rightarrow (G_{\gamma },F_{\gamma })$
because
$p_{\gamma }:(G',F')\rightarrow (G_{\gamma },F_{\gamma })$
because
![]() $(G_{\gamma },F_{\gamma })$
is the inverse limit of
$(G_{\gamma },F_{\gamma })$
is the inverse limit of
![]() $(G_i,F_i)_{i<\gamma }$
. If
$(G_i,F_i)_{i<\gamma }$
. If
![]() $\gamma =\gamma '+1$
is a successor ordinal, there is a morphism
$\gamma =\gamma '+1$
is a successor ordinal, there is a morphism
![]() $r:(G',F')\rightarrow (G_{\gamma },F_{\gamma })$
such that
$r:(G',F')\rightarrow (G_{\gamma },F_{\gamma })$
such that
![]() $p_{\gamma '}=\pi _{\gamma ,\gamma '}\circ r$
because
$p_{\gamma '}=\pi _{\gamma ,\gamma '}\circ r$
because
![]() $\pi _{\gamma ,\gamma '}$
is a q.s.c. Put
$\pi _{\gamma ,\gamma '}$
is a q.s.c. Put
![]() $p_{\gamma }:=r$
, which is the desired one.
$p_{\gamma }:=r$
, which is the desired one.
Since
![]() $(G,F)$
is the inverse limit of
$(G,F)$
is the inverse limit of
![]() $(G_i,F_i)_{i<\beta }$
, there is
$(G_i,F_i)_{i<\beta }$
, there is
![]() $q:(G',F')\rightarrow (G,F)$
such that for each
$q:(G',F')\rightarrow (G,F)$
such that for each
![]() $i<\alpha $
,
$i<\alpha $
,
![]() $p_i=\pi _i\circ q$
. Thus, we have that
$p_i=\pi _i\circ q$
. Thus, we have that
![]() $p=\pi _0\circ q$
, and
$p=\pi _0\circ q$
, and
![]() $\pi _0$
is a q.s.c.
$\pi _0$
is a q.s.c.
Theorem 3.13. Let
![]() $(G,F)$
be a sorted profinite group. Then, there is a universal SEP-cover
$(G,F)$
be a sorted profinite group. Then, there is a universal SEP-cover
![]() $p:(H,F_H)\rightarrow (G,F)$
. Furthermore, if G is finitely generated, then p is the unique universal SEP-cover (up to isomorphism).
$p:(H,F_H)\rightarrow (G,F)$
. Furthermore, if G is finitely generated, then p is the unique universal SEP-cover (up to isomorphism).
Proof If
![]() $(G,F)$
has SEP, then
$(G,F)$
has SEP, then
![]() ${\operatorname {id}} : (G,F)\rightarrow (G,F)$
is a universal SEP-cover. Suppose
${\operatorname {id}} : (G,F)\rightarrow (G,F)$
is a universal SEP-cover. Suppose
![]() $(G,F)$
does not have SEP. Let
$(G,F)$
does not have SEP. Let
![]() $\kappa $
be a cardinal such that any q.s.c.
$\kappa $
be a cardinal such that any q.s.c.
![]() $(H,F_H)$
of
$(H,F_H)$
of
![]() $(G,F)$
has the cardinality less than
$(G,F)$
has the cardinality less than
![]() $\kappa $
and let
$\kappa $
and let
![]() $\aleph $
be a cardinal with
$\aleph $
be a cardinal with
![]() $2^{\kappa }<\aleph $
.
$2^{\kappa }<\aleph $
.
Using transfinite induction, we will construct an inverse system
![]() $((G_i,F_i))_i$
indexed by ordinals
$((G_i,F_i))_i$
indexed by ordinals
![]() $i\le \alpha $
for some ordinal
$i\le \alpha $
for some ordinal
![]() $\alpha <\aleph $
such that:
$\alpha <\aleph $
such that:
-
(1) For each
 $i<j$
, the transition map
$i<j$
, the transition map
 $\pi _{j,i}$
is a q.s.c. with a non-trivial kernel.
$\pi _{j,i}$
is a q.s.c. with a non-trivial kernel. -
(2) For each limit ordinal
 $\beta $
,
$\beta $
,
 $(G_{\beta },F_{\beta })$
is the inverse limit of the inverse system
$(G_{\beta },F_{\beta })$
is the inverse limit of the inverse system
 $((G_i,F_i))_{i<\beta }$
with transition maps
$((G_i,F_i))_{i<\beta }$
with transition maps
 $\pi _{i,j}$
for
$\pi _{i,j}$
for
 $i<j<\beta $
.
$i<j<\beta $
. -
(3) For each limit ordinal
 $\beta $
and for
$\beta $
and for
 $i<\beta $
, the transition map
$i<\beta $
, the transition map
 $\pi _{\beta ,i}$
is the natural projection from
$\pi _{\beta ,i}$
is the natural projection from
 $(G_{\beta },F_{\beta })$
to
$(G_{\beta },F_{\beta })$
to
 $(G_i,F_i)$
coming from the inverse limit construction.
$(G_i,F_i)$
coming from the inverse limit construction. -
(4) Any q.s.c. to
 $(G_{\alpha },F_{\alpha })$
has trivial kernel.
$(G_{\alpha },F_{\alpha })$
has trivial kernel.
Put
![]() $(G_0,F_0)=(G,F)$
. Suppose we have constructed an inverse system
$(G_0,F_0)=(G,F)$
. Suppose we have constructed an inverse system
![]() $((G_i,F_i))_{i<\beta }$
for an ordinal
$((G_i,F_i))_{i<\beta }$
for an ordinal
![]() $\beta $
, which satisfies
$\beta $
, which satisfies
![]() $(1)$
–
$(1)$
–
![]() $(3)$
.
$(3)$
.
Case.
![]() $\beta $
is a successor ordinal, that is,
$\beta $
is a successor ordinal, that is,
![]() $\beta =\beta '+1$
. If
$\beta =\beta '+1$
. If
![]() $(G_{\beta '},F_{\beta '})$
has SEP, then we stop the process. Suppose
$(G_{\beta '},F_{\beta '})$
has SEP, then we stop the process. Suppose
![]() $(G_{\beta '},F_{\beta '})$
does not have SEP. By Lemma 3.11, there is a q.s.c. to
$(G_{\beta '},F_{\beta '})$
does not have SEP. By Lemma 3.11, there is a q.s.c. to
![]() $(G_{\beta '},F_{\beta '})$
, which is not an isomorphism. If every q.s.c. to
$(G_{\beta '},F_{\beta '})$
, which is not an isomorphism. If every q.s.c. to
![]() $(G_{\beta '},F_{\beta '})$
is injective, we stop the process. So, we may assume that there is a q.s.c.
$(G_{\beta '},F_{\beta '})$
is injective, we stop the process. So, we may assume that there is a q.s.c.
![]() $p:(G',F')\rightarrow (G_{\beta '},F_{\beta '})$
with a non-trivial kernel. Put
$p:(G',F')\rightarrow (G_{\beta '},F_{\beta '})$
with a non-trivial kernel. Put
![]() $(G_{\beta },F_{\beta }):=(G',F')$
and
$(G_{\beta },F_{\beta }):=(G',F')$
and
![]() $\pi _{\beta ,\beta '}:=p$
. For each
$\pi _{\beta ,\beta '}:=p$
. For each
![]() $i<\beta $
, put
$i<\beta $
, put
![]() $\pi _{\beta ,i}:=\pi _{\beta ',i}\circ p$
. By Remark 3.9(1), each
$\pi _{\beta ,i}:=\pi _{\beta ',i}\circ p$
. By Remark 3.9(1), each
![]() $\pi _{\beta ,i}$
is a q.s.c.
$\pi _{\beta ,i}$
is a q.s.c.
Case.
![]() $\beta $
is a limit ordinal. Let
$\beta $
is a limit ordinal. Let
![]() $(G_{\beta },F_{\beta })$
be the inverse limit of
$(G_{\beta },F_{\beta })$
be the inverse limit of
![]() $((G_i,F_i))_{i<\beta }$
. For each
$((G_i,F_i))_{i<\beta }$
. For each
![]() $i<\beta $
, let
$i<\beta $
, let
![]() $\pi _{\beta ,i}$
be the natural projection map from
$\pi _{\beta ,i}$
be the natural projection map from
![]() $(G_{\beta },F_{\beta })$
to
$(G_{\beta },F_{\beta })$
to
![]() $(G_i,F_i)$
. By Lemma 3.12, each
$(G_i,F_i)$
. By Lemma 3.12, each
![]() $\pi _{\beta ,i}$
is a q.s.c.
$\pi _{\beta ,i}$
is a q.s.c.
For each
![]() $i<j$
,
$i<j$
,
![]() $\pi _{j,i}$
has a non-trivial kernel. Namely, suppose there are
$\pi _{j,i}$
has a non-trivial kernel. Namely, suppose there are
![]() $i<j$
such that
$i<j$
such that
![]() ${\operatorname {Ker}}(\pi _{j,i})$
is trivial. Since
${\operatorname {Ker}}(\pi _{j,i})$
is trivial. Since
![]() $\pi _{j,i}=\pi _{i+1,i}\circ \pi _{j,i+1}$
, where
$\pi _{j,i}=\pi _{i+1,i}\circ \pi _{j,i+1}$
, where
![]() $\pi _{k,k}={\operatorname {id}}$
for each k,
$\pi _{k,k}={\operatorname {id}}$
for each k,
![]() ${\operatorname {Ker}}(\pi _{i+1,i})$
is also trivial, which is a contradiction. In our construction,
${\operatorname {Ker}}(\pi _{i+1,i})$
is also trivial, which is a contradiction. In our construction,
![]() $\beta $
should be less than
$\beta $
should be less than
![]() $\aleph $
. Suppose
$\aleph $
. Suppose
![]() $\beta \ge \aleph $
. Without loss of generality, we may assume that
$\beta \ge \aleph $
. Without loss of generality, we may assume that
![]() $\beta $
is a successor ordinal and write
$\beta $
is a successor ordinal and write
![]() $\beta =\beta '+1$
. We have that
$\beta =\beta '+1$
. We have that
![]() $|G_{\beta '}|\le \kappa $
. So,
$|G_{\beta '}|\le \kappa $
. So,
![]() $|{\mathcal {N}}(G_{\beta '})|\le 2^{\kappa }$
. Since
$|{\mathcal {N}}(G_{\beta '})|\le 2^{\kappa }$
. Since
![]() $|\beta '|\ge \aleph>2^{\kappa }$
, by the pigeon hole principle, for some
$|\beta '|\ge \aleph>2^{\kappa }$
, by the pigeon hole principle, for some
![]() $i<j<\beta '$
,
$i<j<\beta '$
,
![]() ${\operatorname {Ker}}(\pi _{\beta ',j})={\operatorname {Ker}}(\pi _{\beta ',i})$
. Since
${\operatorname {Ker}}(\pi _{\beta ',j})={\operatorname {Ker}}(\pi _{\beta ',i})$
. Since
![]() $\pi _{\beta ',i}=\pi ^j_i\circ \pi _{\beta ',j}$
, we have that
$\pi _{\beta ',i}=\pi ^j_i\circ \pi _{\beta ',j}$
, we have that
![]() $\pi _{j,i}$
is injective, which is a contradiction.
$\pi _{j,i}$
is injective, which is a contradiction.
Now, take a maximal inverse system
![]() $((G_i,F_i))_{i<\beta }$
satisfying
$((G_i,F_i))_{i<\beta }$
satisfying
![]() $(1)$
–
$(1)$
–
![]() $(3)$
. Note that
$(3)$
. Note that
![]() $\beta $
is a successor ordinal and write
$\beta $
is a successor ordinal and write
![]() $\beta =\alpha +1$
. Then, for the q.s.c.
$\beta =\alpha +1$
. Then, for the q.s.c.
![]() $\pi _{\alpha ,0}:(G_{\alpha },F_{\alpha })\rightarrow (G,F)$
, we have that every q.s.c. to
$\pi _{\alpha ,0}:(G_{\alpha },F_{\alpha })\rightarrow (G,F)$
, we have that every q.s.c. to
![]() $(G_{\alpha },F_{\alpha })$
is injective and so
$(G_{\alpha },F_{\alpha })$
is injective and so
![]() $((G_i,F_i))_{i\le \alpha }$
is the desired one.
$((G_i,F_i))_{i\le \alpha }$
is the desired one.
Let
![]() $(E(G),E(F)):=(G_{\alpha },F_{\alpha })$
and let
$(E(G),E(F)):=(G_{\alpha },F_{\alpha })$
and let
![]() $\pi :=\pi _{\alpha ,0}$
. If
$\pi :=\pi _{\alpha ,0}$
. If
![]() $(E(G),E(F))$
has SEP, then
$(E(G),E(F))$
has SEP, then
![]() $\pi $
is a universal SEP-cover of
$\pi $
is a universal SEP-cover of
![]() $(G,F)$
. Suppose
$(G,F)$
. Suppose
![]() $(E(G),E(F))$
does not have SEP. By transfinite induction, we will construct a sequence
$(E(G),E(F))$
does not have SEP. By transfinite induction, we will construct a sequence
![]() $(E^i(F))_{i\le \gamma '}$
of sorting data on
$(E^i(F))_{i\le \gamma '}$
of sorting data on
![]() $E(G)$
such that for
$E(G)$
such that for
![]() $i<j\le \gamma '$
:
$i<j\le \gamma '$
:
-
•
 $E^i(F)\subset E^j(G)$
.
$E^i(F)\subset E^j(G)$
. -
•
 ${\operatorname {id}}_{j,i}:={\operatorname {id}}:(E(G),E^j(F))\rightarrow (E(G),E^i(F))$
is a q.s.c.
${\operatorname {id}}_{j,i}:={\operatorname {id}}:(E(G),E^j(F))\rightarrow (E(G),E^i(F))$
is a q.s.c. -
•
 $(E(G),E^{\gamma '}(F))$
has SEP.
$(E(G),E^{\gamma '}(F))$
has SEP.
Suppose we have constructed such a sequence
![]() $(E^i(F))_{i< \alpha '}$
for an ordinal
$(E^i(F))_{i< \alpha '}$
for an ordinal
![]() $\alpha '$
. If
$\alpha '$
. If
![]() $\alpha '$
is a limit ordinal, put
$\alpha '$
is a limit ordinal, put
![]() $E^{\alpha '}(F):=\bigcup _{i<\alpha '}E^i(F)$
. By Lemma 3.12, each
$E^{\alpha '}(F):=\bigcup _{i<\alpha '}E^i(F)$
. By Lemma 3.12, each
![]() ${\operatorname {id}}_{\alpha ',i}:(E(G),E^{\alpha '}(F))\rightarrow (E(G),E^i(F))$
is a q.s.c.
${\operatorname {id}}_{\alpha ',i}:(E(G),E^{\alpha '}(F))\rightarrow (E(G),E^i(F))$
is a q.s.c.
Suppose
![]() $\alpha '$
is a successor ordinal, that is,
$\alpha '$
is a successor ordinal, that is,
![]() $\alpha '=\alpha "+1$
. If
$\alpha '=\alpha "+1$
. If
![]() $(E(G),E^{\alpha "}(F))$
has SEP, put
$(E(G),E^{\alpha "}(F))$
has SEP, put
![]() $\gamma '=\alpha "$
and stop the process. If
$\gamma '=\alpha "$
and stop the process. If
![]() $(E(G),E^{\alpha "}(F))$
does not have SEP, then by Lemma 3.11(2), there is a sorting data
$(E(G),E^{\alpha "}(F))$
does not have SEP, then by Lemma 3.11(2), there is a sorting data
![]() $F'$
on
$F'$
on
![]() $E(G)$
such that
$E(G)$
such that
![]() $E^{\alpha "}(F)\subset F'$
and
$E^{\alpha "}(F)\subset F'$
and
![]() ${\operatorname {id}}:(E(G),F')\rightarrow (E(G),E^{\alpha "}(F))$
is a q.s.c, and put
${\operatorname {id}}:(E(G),F')\rightarrow (E(G),E^{\alpha "}(F))$
is a q.s.c, and put
![]() $E^{\alpha '}(F):=F'$
. Note that we cannot apply Lemma 3.11(1) in this case. Namely, if there is a q.s.c
$E^{\alpha '}(F):=F'$
. Note that we cannot apply Lemma 3.11(1) in this case. Namely, if there is a q.s.c
![]() $\pi :(G',F')\rightarrow (E(G),E^{\alpha "}(F))$
whose kernel is non-trivial, then we have a q.s.c.
$\pi :(G',F')\rightarrow (E(G),E^{\alpha "}(F))$
whose kernel is non-trivial, then we have a q.s.c.
![]() ${\operatorname {id}} \circ \pi :(G',F')\rightarrow (E(G),E^{\alpha "}(F))\rightarrow (G,F)$
whose kernel is non-trivial, which contradicts the choice of
${\operatorname {id}} \circ \pi :(G',F')\rightarrow (E(G),E^{\alpha "}(F))\rightarrow (G,F)$
whose kernel is non-trivial, which contradicts the choice of
![]() $(G,F)$
.
$(G,F)$
.
This transfinite inductive process should stop for some
![]() $\gamma '<|{\mathcal {J}}|^+$
so that
$\gamma '<|{\mathcal {J}}|^+$
so that
![]() $(E(G),E^{\gamma '}(F))$
has SEP. Thus, a q.s.c.
$(E(G),E^{\gamma '}(F))$
has SEP. Thus, a q.s.c.
is a SEP-cover so that the SEP-cover
![]() $\pi _{\alpha ,0}\circ {\operatorname {id}}_{\gamma ',0}$
is a universal SEP-cover.
$\pi _{\alpha ,0}\circ {\operatorname {id}}_{\gamma ',0}$
is a universal SEP-cover.
We now prove the furthermore part. Suppose G is finitely generated. Let
![]() $p_i:(G_i,F_i)\rightarrow (G,F)$
be a universal SEP-cover of
$p_i:(G_i,F_i)\rightarrow (G,F)$
be a universal SEP-cover of
![]() $(G,F)$
for
$(G,F)$
for
![]() $i=1,2$
. By Remark 3.3 and [Reference Haran and Lubotzky7, Theorem 1.12],
$i=1,2$
. By Remark 3.3 and [Reference Haran and Lubotzky7, Theorem 1.12],
![]() $G_1$
and
$G_1$
and
![]() $G_2$
are finitely generated. By universality, there are morphisms
$G_2$
are finitely generated. By universality, there are morphisms
![]() $q:(G_2,F_2)\rightarrow (G_1,F_1)$
and
$q:(G_2,F_2)\rightarrow (G_1,F_1)$
and
![]() $q':(G_1,F_1)\rightarrow (G_2,F_2)$
such that
$q':(G_1,F_1)\rightarrow (G_2,F_2)$
such that
![]() ${p_2=p_1\circ q}$
and
${p_2=p_1\circ q}$
and
![]() $p_1=p_2\circ q'$
. Since
$p_1=p_2\circ q'$
. Since
![]() $G_1$
and
$G_1$
and
![]() $G_2$
are finitely generated, by [Reference Ribes13, Proposition 7.6], both q and
$G_2$
are finitely generated, by [Reference Ribes13, Proposition 7.6], both q and
![]() $q'$
are bijective. We want to show that
$q'$
are bijective. We want to show that
![]() $q_*(F_2)=F_1$
. Since
$q_*(F_2)=F_1$
. Since
![]() $q:(G_2,F_2)\rightarrow (G_1,F_1)$
is a morphism,
$q:(G_2,F_2)\rightarrow (G_1,F_1)$
is a morphism,
![]() $F_1\subseteq q_*(F_2)$
. Also we have that
$F_1\subseteq q_*(F_2)$
. Also we have that
![]() $q:(G_2,F_2)\cong (G_1,q_*(F_2))$
and
$q:(G_2,F_2)\cong (G_1,q_*(F_2))$
and
![]() $p_2\circ q^{-1}:(G_1,q_*(F_2))\rightarrow (G,F)$
is a universal SEP-cover. So, there is a morphism
$p_2\circ q^{-1}:(G_1,q_*(F_2))\rightarrow (G,F)$
is a universal SEP-cover. So, there is a morphism
![]() $r:(G_1,F_1)\rightarrow (G_1,q_*(F_2))$
such that
$r:(G_1,F_1)\rightarrow (G_1,q_*(F_2))$
such that
![]() $p_1=(p_2\circ q^{-1})\circ r$
. Since
$p_1=(p_2\circ q^{-1})\circ r$
. Since
![]() $p_2=p_1\circ q$
, the morphism r should be the identity map on G and
$p_2=p_1\circ q$
, the morphism r should be the identity map on G and
![]() $q_*(F_2)\subseteq F_1$
. Thus,
$q_*(F_2)\subseteq F_1$
. Thus,
![]() $F_1=q_*(F_2)$
.
$F_1=q_*(F_2)$
.
Remark 3.14. Let
![]() $\mathcal C$
be a formation of finite groups, that is, a set of finite groups closed under taking quotients and fibre products (see [Reference Fried and Jarden6, Section 17.3]). Let
$\mathcal C$
be a formation of finite groups, that is, a set of finite groups closed under taking quotients and fibre products (see [Reference Fried and Jarden6, Section 17.3]). Let
![]() ${\operatorname {Pro}}\mathcal C$
be the set of pro-
${\operatorname {Pro}}\mathcal C$
be the set of pro-
![]() $\mathcal C$
groups, that is, the set of inverse limits of groups in
$\mathcal C$
groups, that is, the set of inverse limits of groups in
![]() $\mathcal C$
. Let
$\mathcal C$
. Let
![]() ${\operatorname {Pro}}\mathcal C_{{\mathcal {J}}}$
be the full subcategory of the category
${\operatorname {Pro}}\mathcal C_{{\mathcal {J}}}$
be the full subcategory of the category
![]() ${\operatorname {SPG}}$
whose objects are of the form
${\operatorname {SPG}}$
whose objects are of the form
![]() $(G,F)$
, called a sorted pro-
$(G,F)$
, called a sorted pro-
![]() $\mathcal C$
group, for a pro-
$\mathcal C$
group, for a pro-
![]() ${\mathcal {C}}$
group G. In [Reference Fried and Jarden6, Lemma 24.4.6], any pro-
${\mathcal {C}}$
group G. In [Reference Fried and Jarden6, Lemma 24.4.6], any pro-
![]() $\mathcal C$
group G has the universal embedding cover, which is also a pro-
$\mathcal C$
group G has the universal embedding cover, which is also a pro-
![]() $\mathcal C$
group. So, by Remark 3.3, any sorted pro-
$\mathcal C$
group. So, by Remark 3.3, any sorted pro-
![]() $\mathcal C$
group has a universal SEP-cover, which is also a pro-
$\mathcal C$
group has a universal SEP-cover, which is also a pro-
![]() $\mathcal C$
group.
$\mathcal C$
group.
Suppose
![]() $\mathcal C$
is closed under taking subgroups. For example, let
$\mathcal C$
is closed under taking subgroups. For example, let
![]() $\mathcal C$
be the set of abelian groups, nilpotent groups, solvable groups, or p-groups for a fixed prime p. By [Reference Fried and Jarden6, Lemma 17.3.1],
$\mathcal C$
be the set of abelian groups, nilpotent groups, solvable groups, or p-groups for a fixed prime p. By [Reference Fried and Jarden6, Lemma 17.3.1],
![]() ${\operatorname {Pro}}\mathcal C$
is closed under taking quotients, inverse limits, and fibre products. Since our proof works for any full subcategory of
${\operatorname {Pro}}\mathcal C$
is closed under taking quotients, inverse limits, and fibre products. Since our proof works for any full subcategory of
![]() ${\operatorname {SPG}}$
closed under taking quotients, inverse limits, and fibre products, we also deduce from the proof of Theorem 3.13 that any sorted pro-
${\operatorname {SPG}}$
closed under taking quotients, inverse limits, and fibre products, we also deduce from the proof of Theorem 3.13 that any sorted pro-
![]() $\mathcal C$
group has a universal SEP-cover, which is also a sorted pro-
$\mathcal C$
group has a universal SEP-cover, which is also a sorted pro-
![]() $\mathcal C$
group.
$\mathcal C$
group.
Example 3.15. We continue working with the notations in Example 2.20(2). Define a sorting data F on
![]() $G={\mathbb {Z}}_2\times {\mathbb {Z}}_2$
as follows:
$G={\mathbb {Z}}_2\times {\mathbb {Z}}_2$
as follows:
-
•
 $F(0)=F(G)=F(N_{(1,1)})={\mathcal {J}}^{<{\mathbb {N}}}$
.
$F(0)=F(G)=F(N_{(1,1)})={\mathcal {J}}^{<{\mathbb {N}}}$
. -
•
 $F(N_{(1,0)})=F(N_{(0,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
.
$F(N_{(1,0)})=F(N_{(0,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
.
Take
![]() $(\Pi _1,\Pi _2)\in {\mathcal {P}}((G,F),(G,F))$
such that:
$(\Pi _1,\Pi _2)\in {\mathcal {P}}((G,F),(G,F))$
such that:
-
•
 $A:=G$
,
$A:=G$
,
 $F_A$
is a sorting data on A given as follows:
$F_A$
is a sorting data on A given as follows:-
–
 $F_A(A)={\mathcal {J}}^{{\mathbb {N}}}$
.
$F_A(A)={\mathcal {J}}^{{\mathbb {N}}}$
. -
–
 $F_A(0)=F(N_{(1,0)})=F(N_{(0,1)})=F(N_{(1,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
.
$F_A(0)=F(N_{(1,0)})=F(N_{(0,1)})=F(N_{(1,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
.
-
-
•
 $\Pi _1,\Pi _2:(G,F)\rightarrow (A,F_A)$
given as follows:
$\Pi _1,\Pi _2:(G,F)\rightarrow (A,F_A)$
given as follows:-
–
 $\Pi _2={\operatorname {id}}$
.
$\Pi _2={\operatorname {id}}$
. -
–
 $\Pi _1:(1,1)\mapsto (1,0),(1,0)\mapsto (1,1), (0,1)\mapsto (0,1)$
.
$\Pi _1:(1,1)\mapsto (1,0),(1,0)\mapsto (1,1), (0,1)\mapsto (0,1)$
.
-
Then,
![]() $(\Pi _1,\Pi _2)$
is a non-trivial maximal element. The fibre product with respect to
$(\Pi _1,\Pi _2)$
is a non-trivial maximal element. The fibre product with respect to
![]() $(\Pi _1,\Pi _2)$
is
$(\Pi _1,\Pi _2)$
is
![]() $(G,E^0(F))$
where the sorting data
$(G,E^0(F))$
where the sorting data
![]() $E^0(F)$
is given as follows:
$E^0(F)$
is given as follows:
-
•
 $E^0(F)(0)=E^0(F)(G)=E^0(F)(N_{(1,0)})=E^0(F)(N_{(1,1)})={\mathcal {J}}^{<{\mathbb {N}}}$
.
$E^0(F)(0)=E^0(F)(G)=E^0(F)(N_{(1,0)})=E^0(F)(N_{(1,1)})={\mathcal {J}}^{<{\mathbb {N}}}$
. -
•
 $E^0(F)(N_{(0,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
.
$E^0(F)(N_{(0,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
.
Take
![]() $(\Pi _1',\Pi _2')\in {\mathcal {P}}((G,E^0(F)),(G,E^0(F)))$
such that:
$(\Pi _1',\Pi _2')\in {\mathcal {P}}((G,E^0(F)),(G,E^0(F)))$
such that:
-
•
 $A':=G$
,
$A':=G$
,
 $F_{A'}$
is a sorting data on
$F_{A'}$
is a sorting data on
 $A'$
given as follows:
$A'$
given as follows:-
–
 $F_{A'}(0)=F_{A'}(A')=F_{A'}(N_{(1,1)})={\mathcal {J}}^{<{\mathbb {N}}}$
.
$F_{A'}(0)=F_{A'}(A')=F_{A'}(N_{(1,1)})={\mathcal {J}}^{<{\mathbb {N}}}$
. -
–
 $F_{A'}(N_{(1,0)})=F_{A'}(N_{(0,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
.
$F_{A'}(N_{(1,0)})=F_{A'}(N_{(0,1)})=\{J\in {\mathcal {J}}^{<{\mathbb {N}}}:s_1\in ||J||\}$
.
-
-
•
 $\Pi _1',\Pi _2':(G,E^0(F))\rightarrow (A',F_{A'})$
given as follows:
$\Pi _1',\Pi _2':(G,E^0(F))\rightarrow (A',F_{A'})$
given as follows:-
–
 $\Pi _2'={\operatorname {id}}$
.
$\Pi _2'={\operatorname {id}}$
. -
–
 $\Pi _1':(1,1)\mapsto (0,1), (1,0)\mapsto (1,1), (0,1)\mapsto (1,0)$
.
$\Pi _1':(1,1)\mapsto (0,1), (1,0)\mapsto (1,1), (0,1)\mapsto (1,0)$
.
-
Then,
![]() $(\Pi _1',\Pi _2')$
is a non-trivial maximal element. The fibre product with respect to
$(\Pi _1',\Pi _2')$
is a non-trivial maximal element. The fibre product with respect to
![]() $(\Pi _1',\Pi _2')$
is
$(\Pi _1',\Pi _2')$
is
![]() $(G,E^1(F))$
where the sorting data
$(G,E^1(F))$
where the sorting data
![]() $E^1(F)$
is full. Thus, the identity map from
$E^1(F)$
is full. Thus, the identity map from
![]() $(G,E^1(F))$
to
$(G,E^1(F))$
to
![]() $(G,F)$
is a universal SEP-cover of
$(G,F)$
is a universal SEP-cover of
![]() $(G,F)$
.
$(G,F)$
.
Using the characterization of SEP in Theorem 2.22, we show that there is a unique (up to isomorphism) SEP-cover of a given sorted profinite group.
Fact 3.16 [Reference Chatzidakis1, Theorem 2.7].
A profinite group H has the unique universal embedding cover
![]() $E(H)$
and
$E(H)$
and
where
![]() $E(A)$
is the unique embedding cover of A.
$E(A)$
is the unique embedding cover of A.
Theorem 3.17. There is a unique (up to isomorphism) SEP-cover
![]() $(G,F)$
of a sorted profinite group
$(G,F)$
of a sorted profinite group
![]() $(H,F_H)$
.
$(H,F_H)$
.
Proof By Theorem 3.13, each
![]() $(A,F_A)\in {\operatorname {FSIm}}(H,F_H)$
has the unique SEP-cover
$(A,F_A)\in {\operatorname {FSIm}}(H,F_H)$
has the unique SEP-cover
![]() $(E(A),E(F_A))$
. Let
$(E(A),E(F_A))$
. Let
 $$ \begin{align*}\Gamma:=\bigcup_{(A,F)\in {\operatorname{FSIm}}(H,F_H)}{\operatorname{FSIm}}(E(A),E(F_A)).\end{align*} $$
$$ \begin{align*}\Gamma:=\bigcup_{(A,F)\in {\operatorname{FSIm}}(H,F_H)}{\operatorname{FSIm}}(E(A),E(F_A)).\end{align*} $$
Let G be the unique embedding cover of H. Note that
by Fact 3.16. Define a pre-sorting data
![]() $\hat F$
on G as follows: For each
$\hat F$
on G as follows: For each
![]() $N\in {\mathcal {N}}(G)$
,
$N\in {\mathcal {N}}(G)$
,
 $$ \begin{align*}\hat F(N):=\bigcup_{(A,F_A)\in \Gamma, A\cong G/N}F_A(\{e_A\}).\end{align*} $$
$$ \begin{align*}\hat F(N):=\bigcup_{(A,F_A)\in \Gamma, A\cong G/N}F_A(\{e_A\}).\end{align*} $$
Claim 3.18. The pre-sorting data
![]() $\hat F$
is a sorting data.
$\hat F$
is a sorting data.
Proof It is enough to show that
![]() $\hat F$
satisfies
$\hat F$
satisfies
![]() $(3)$
and
$(3)$
and
![]() $(4)$
in Definition 2.2, that is:
$(4)$
in Definition 2.2, that is:
-
(3) Suppose
 $N_1\subseteq N_2$
and
$N_1\subseteq N_2$
and
 $[G:N_1]\le k$
. For
$[G:N_1]\le k$
. For
 $J\in {\mathcal {J}}^{<\mathbb N}$
,
$J\in {\mathcal {J}}^{<\mathbb N}$
,  $$ \begin{align*}J\in \hat F(N_1)\Rightarrow J^*_{\subseteq}(k,J)\in \hat F(N_2).\end{align*} $$
$$ \begin{align*}J\in \hat F(N_1)\Rightarrow J^*_{\subseteq}(k,J)\in \hat F(N_2).\end{align*} $$
-
(4) For
 $J_1\in \hat F(N_1)$
and
$J_1\in \hat F(N_1)$
and
 $J_2\in \hat F(N_2)$
,
$J_2\in \hat F(N_2)$
,
 $J^*_{\cap }(J_1,J_2)\in \hat F(N_1\cap N_2)$
.
$J^*_{\cap }(J_1,J_2)\in \hat F(N_1\cap N_2)$
.
![]() $(3)$
Let
$(3)$
Let
![]() $N_1\subseteq N_2\in {\mathcal {N}}(G)$
with
$N_1\subseteq N_2\in {\mathcal {N}}(G)$
with
![]() $[G:N_1]\le k$
and let
$[G:N_1]\le k$
and let
![]() $J\in {\mathcal {J}}^{<\mathbb N}$
with
$J\in {\mathcal {J}}^{<\mathbb N}$
with
![]() $J\in \hat F(N_1)$
. By the choice of
$J\in \hat F(N_1)$
. By the choice of
![]() $\hat F$
, there are:
$\hat F$
, there are:
-
• the universal SEP-cover
 $(E(A),E(F_A))$
of some
$(E(A),E(F_A))$
of some
 $(A,F_A)\in {\operatorname {FSIm}}(H,F_H)$
; and
$(A,F_A)\in {\operatorname {FSIm}}(H,F_H)$
; and -
•
 $(G/N_1,F_1)\in {\operatorname {FSIm}}(E(A),E(F_A))$
such that
$(G/N_1,F_1)\in {\operatorname {FSIm}}(E(A),E(F_A))$
such that
 $J\in F_1(\{e_{G/N_1}\})$
.
$J\in F_1(\{e_{G/N_1}\})$
.
Then,
![]() $J^*_{\subseteq }(k,J)\in F_1(N_2/N_1)$
. Consider a push-forward sorting data
$J^*_{\subseteq }(k,J)\in F_1(N_2/N_1)$
. Consider a push-forward sorting data
![]() $F_2:=\pi _*(F_1)$
on
$F_2:=\pi _*(F_1)$
on
![]() $G/N_2$
where
$G/N_2$
where
![]() $\pi :G/N_1\rightarrow G/N_2$
is the projection. Then,
$\pi :G/N_1\rightarrow G/N_2$
is the projection. Then,
![]() $(G/N_2,F_2)\in {\operatorname {FSIm}}(E(A),E(F_A))$
and
$(G/N_2,F_2)\in {\operatorname {FSIm}}(E(A),E(F_A))$
and
![]() $J^*_{\subseteq }(k,J)\in F_2(\{e_{G/N_2}\})=F_1(N_2/N_1)\subseteq \hat F(N_2)$
.
$J^*_{\subseteq }(k,J)\in F_2(\{e_{G/N_2}\})=F_1(N_2/N_1)\subseteq \hat F(N_2)$
.
![]() $(4)$
Take
$(4)$
Take
![]() $N_1,N_2\in {\mathcal {N}}(G)$
,
$N_1,N_2\in {\mathcal {N}}(G)$
,
![]() $J_1\in \hat F(N_1)$
, and
$J_1\in \hat F(N_1)$
, and
![]() $J_2\in \hat F(N_2)$
arbitrary. By the choice of
$J_2\in \hat F(N_2)$
arbitrary. By the choice of
![]() $\hat F$
, for each
$\hat F$
, for each
![]() $i=1,2$
, there are:
$i=1,2$
, there are:
-
•
 $\bar N_i\in {\mathcal {N}}(H)$
;
$\bar N_i\in {\mathcal {N}}(H)$
; -
• the universal SEP-cover
 $(E(G/\bar N_i),E(\bar F_i))$
of some
$(E(G/\bar N_i),E(\bar F_i))$
of some
 $(G{/}\bar N_i,\bar F_i)\kern1.2pt{\in}\kern1.2pt {\operatorname {FSIm}}(H,F_H)$
; and
$(G{/}\bar N_i,\bar F_i)\kern1.2pt{\in}\kern1.2pt {\operatorname {FSIm}}(H,F_H)$
; and -
•
 $(G/N_i,F_i)\in {\operatorname {FSIm}}(E(G/\bar N_i),E(\bar F_i))$
such that
$(G/N_i,F_i)\in {\operatorname {FSIm}}(E(G/\bar N_i),E(\bar F_i))$
such that
 $J_i\in F_i(\{e_{G/N_i}\})$
.
$J_i\in F_i(\{e_{G/N_i}\})$
.
Let
![]() $\bar F_0$
be a sorting data on
$\bar F_0$
be a sorting data on
![]() $H/(\bar N_1\bar N_2)$
such that the projection from
$H/(\bar N_1\bar N_2)$
such that the projection from
![]() $H/\bar N_i$
to
$H/\bar N_i$
to
![]() $H/(\bar N_1\bar N_2))$
is sorted for
$H/(\bar N_1\bar N_2))$
is sorted for
![]() $i=1,2$
. Let
$i=1,2$
. Let
![]() $(H/(\bar N_1\cap \bar N_2),\bar F_3)$
be the fibre product of
$(H/(\bar N_1\cap \bar N_2),\bar F_3)$
be the fibre product of
![]() $(H/\bar N_1,\bar F_1)$
and
$(H/\bar N_1,\bar F_1)$
and
![]() $(H/\bar N_2,\bar F_2)$
over
$(H/\bar N_2,\bar F_2)$
over
![]() $(H/(\bar N_1\bar N_2), \bar F_0)$
. Let
$(H/(\bar N_1\bar N_2), \bar F_0)$
. Let
![]() $\left (E(H/(\bar N_1\cap \bar N_2)),E(\bar F_3)\right )$
be the unique SEP-cover of
$\left (E(H/(\bar N_1\cap \bar N_2)),E(\bar F_3)\right )$
be the unique SEP-cover of
![]() $(H/(\bar N_1\cap \bar N_2),\bar F_3)$
.
$(H/(\bar N_1\cap \bar N_2),\bar F_3)$
.
Let
![]() $F_0$
be a sorting data on
$F_0$
be a sorting data on
![]() $G/(N_1N_2)$
such that the projection from
$G/(N_1N_2)$
such that the projection from
![]() $G/N_i$
to
$G/N_i$
to
![]() $G/(N_1N_2)$
is sorted for
$G/(N_1N_2)$
is sorted for
![]() $i=1,2$
. Let
$i=1,2$
. Let
![]() $(G/(N_1\cap N_2),F_3)$
be the fibre product of
$(G/(N_1\cap N_2),F_3)$
be the fibre product of
![]() $(G/N_1,F_1)$
and
$(G/N_1,F_1)$
and
![]() $(G/N_2,F_2)$
over
$(G/N_2,F_2)$
over
![]() $(G/(N_1N_2),F_0)$
.
$(G/(N_1N_2),F_0)$
.
Then, there is a morphism from
![]() $(E(H/(\bar N_1\cap \bar N_2)),E(\bar F_3))$
to
$(E(H/(\bar N_1\cap \bar N_2)),E(\bar F_3))$
to
![]() $(E(H/\bar N_i),E(\bar F_i))$
and so there is a morphism from
$(E(H/\bar N_i),E(\bar F_i))$
and so there is a morphism from
![]() $(E(H/(\bar N_1\cap \bar N_2)),E(\bar F_3))$
to
$(E(H/(\bar N_1\cap \bar N_2)),E(\bar F_3))$
to
![]() $(G/N_i,F_i)$
for
$(G/N_i,F_i)$
for
![]() $i=1,2$
. Since
$i=1,2$
. Since
![]() $(G/(N_1\cap N_2),F_3)$
is the fibre product of
$(G/(N_1\cap N_2),F_3)$
is the fibre product of
![]() $(G/N_1,F_1)$
and
$(G/N_1,F_1)$
and
![]() $(G/N_2,F_2)$
over
$(G/N_2,F_2)$
over
![]() $(G/(N_1N_2),F_0)$
, there is a morphism from
$(G/(N_1N_2),F_0)$
, there is a morphism from
![]() $(E(H/(\bar N_1\cap \bar N_2)),E(\bar F_3))$
to
$(E(H/(\bar N_1\cap \bar N_2)),E(\bar F_3))$
to
![]() $(G/(N_1\cap N_2),F_3)$
. So, we have the following diagram:
$(G/(N_1\cap N_2),F_3)$
. So, we have the following diagram:

Therefore,
and so
By Theorem 2.22(3),
![]() $(G,\hat F)$
has SEP. Now we show that
$(G,\hat F)$
has SEP. Now we show that
![]() $(G,\hat F)$
is the unique universal SEP-cover of
$(G,\hat F)$
is the unique universal SEP-cover of
![]() $(H,F_H)$
.
$(H,F_H)$
.
Claim 3.19. For any SEP-cover
![]() $(G',F')$
of
$(G',F')$
of
![]() $(H,F_H)$
, any epimorphism
$(H,F_H)$
, any epimorphism
![]() ${\varphi :G'\rightarrow G}$
is sorted, that is, for each
${\varphi :G'\rightarrow G}$
is sorted, that is, for each
![]() $N\in {\mathcal {N}}(G)$
,
$N\in {\mathcal {N}}(G)$
,
Proof Let
![]() $(G',F')$
be a SEP-cover of
$(G',F')$
be a SEP-cover of
![]() $(H,F_H)$
and let
$(H,F_H)$
and let
![]() $\varphi $
be an epimorphism from
$\varphi $
be an epimorphism from
![]() $G'$
to G. Take
$G'$
to G. Take
![]() $N\in {\mathcal {N}}(G)$
and
$N\in {\mathcal {N}}(G)$
and
![]() $J\in \hat F(N)$
arbitrary. We show that
$J\in \hat F(N)$
arbitrary. We show that
![]() $J\in F'(\varphi ^{-1}[N])$
. There is a sorting data F on
$J\in F'(\varphi ^{-1}[N])$
. There is a sorting data F on
![]() $G/N$
with
$G/N$
with
![]() $(G/N,F)\in \Gamma $
such that
$(G/N,F)\in \Gamma $
such that
![]() $J\in F(\{e_{G/N}\})$
. Since
$J\in F(\{e_{G/N}\})$
. Since
![]() $(G/N,F)\in \Gamma \subseteq {\operatorname {FSIm}}(G',F')$
and
$(G/N,F)\in \Gamma \subseteq {\operatorname {FSIm}}(G',F')$
and
![]() $G'/\varphi ^{-1}[N]\cong G/N$
, by Lemma 2.21,
$G'/\varphi ^{-1}[N]\cong G/N$
, by Lemma 2.21,
![]() ${J\in F(\{e_{G/N}\})\subseteq F'(\varphi ^{-1}[N])}$
.
${J\in F(\{e_{G/N}\})\subseteq F'(\varphi ^{-1}[N])}$
.
Let
![]() $(G',F')$
be a SEP-cover of
$(G',F')$
be a SEP-cover of
![]() $(H,F_H)$
. Since
$(H,F_H)$
. Since
![]() $G'$
is an embedding cover of H and G is the universal embedding cover of H, there is an epimorphism from
$G'$
is an embedding cover of H and G is the universal embedding cover of H, there is an epimorphism from
![]() $\varphi :G'\rightarrow G$
. By Claim 3.19, the epimorphism
$\varphi :G'\rightarrow G$
. By Claim 3.19, the epimorphism
![]() $\varphi $
is sorted. This implies that
$\varphi $
is sorted. This implies that
![]() $(G,F)$
is a universal SEP-cover of
$(G,F)$
is a universal SEP-cover of
![]() $(H,F_H)$
.
$(H,F_H)$
.
It remains to show that
![]() $(G, \hat F)$
is unique up to isomorphism. Let
$(G, \hat F)$
is unique up to isomorphism. Let
![]() $(G',F')$
be a universal SEP-cover of
$(G',F')$
be a universal SEP-cover of
![]() $(H,F_H)$
. Since
$(H,F_H)$
. Since
![]() $G'$
is a universal embedding cover of H, there is an isomorphism
$G'$
is a universal embedding cover of H, there is an isomorphism
![]() $\varphi :G'\rightarrow G$
. We show that the isomorphism
$\varphi :G'\rightarrow G$
. We show that the isomorphism
![]() $\varphi :G'\rightarrow G$
induces an isomorphism
$\varphi :G'\rightarrow G$
induces an isomorphism
![]() $\varphi :(G',F')\rightarrow (G,\hat F)$
. It is enough to show that for any
$\varphi :(G',F')\rightarrow (G,\hat F)$
. It is enough to show that for any
![]() $N\in {\mathcal {N}}(G)$
,
$N\in {\mathcal {N}}(G)$
,
Take
![]() $N\in {\mathcal {N}}(G)$
arbitrary. By Claim 3.19,
$N\in {\mathcal {N}}(G)$
arbitrary. By Claim 3.19,
![]() $\varphi $
is sorted so that
$\varphi $
is sorted so that
![]() $\hat F(N)\subseteq F'(\varphi ^{-1}[N])$
. Since
$\hat F(N)\subseteq F'(\varphi ^{-1}[N])$
. Since
![]() $(G',F')$
is a universal SEP-cover of
$(G',F')$
is a universal SEP-cover of
![]() $(H,F_H)$
, there is a morphism
$(H,F_H)$
, there is a morphism
![]() $\psi :(G,\hat F)\rightarrow (G',F')$
. So, we have that:
$\psi :(G,\hat F)\rightarrow (G',F')$
. So, we have that:
-
•
 $G/N\cong G'/\varphi ^{-1}[N]\cong G/\psi ^{-1}[\varphi ^{-1}[N]]$
; and
$G/N\cong G'/\varphi ^{-1}[N]\cong G/\psi ^{-1}[\varphi ^{-1}[N]]$
; and -
•
 $\hat F(N)\subseteq F'(\varphi ^{-1}[N])\subseteq \hat F(\psi ^{-1}[\varphi ^{-1}[N]])$
.
$\hat F(N)\subseteq F'(\varphi ^{-1}[N])\subseteq \hat F(\psi ^{-1}[\varphi ^{-1}[N]])$
.
Since
![]() $G/N\cong G/\psi ^{-1}[\varphi ^{-1}[N]]$
, by Lemma 2.21,
$G/N\cong G/\psi ^{-1}[\varphi ^{-1}[N]]$
, by Lemma 2.21,
and so
![]() $\hat F(N)= F'(\varphi ^{-1}[N])= \hat F(\psi ^{-1}[\varphi ^{-1}[N]])$
.
$\hat F(N)= F'(\varphi ^{-1}[N])= \hat F(\psi ^{-1}[\varphi ^{-1}[N]])$
.
4 Model theory of sorted profinite groups having SEP
In [Reference Chatzidakis1, Theorem 2.4], Chatzidakis showed that the theory of a complete system of a profinite group having embedding property is
![]() $\omega $
-stable and gave a description of forking independence. In this section, our main goal is to generalize this phenomenon into the sorted profinite group having SEP when
$\omega $
-stable and gave a description of forking independence. In this section, our main goal is to generalize this phenomenon into the sorted profinite group having SEP when
![]() ${\mathcal {J}}$
is countable.
${\mathcal {J}}$
is countable.
Definition 4.1.
-
(1) A subsystem of S is a substructure of S which is a model of
 $SCS$
.
$SCS$
. -
(2) Let
 $X\subseteq S$
. There is the smallest subsystem
$X\subseteq S$
. There is the smallest subsystem
 $S_X$
containing X. In this case, we say that
$S_X$
containing X. In this case, we say that
 $S_X$
is generated by X.
$S_X$
is generated by X. -
(3.) We say that a subsystem
 $S'$
is finitely generated if there is a finite subset
$S'$
is finitely generated if there is a finite subset
 $X'$
such that
$X'$
such that
 $S'=S_{X'}$
.
$S'=S_{X'}$
.
Definition 4.2.
-
(1) Let X be a subset of S. We say that X is locally full if for each
 $x\in X$
and for each
$x\in X$
and for each
 $(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,
$(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,  $$ \begin{align*}[x]\cap m(k,J)\subseteq X.\end{align*} $$
$$ \begin{align*}[x]\cap m(k,J)\subseteq X.\end{align*} $$
-
(2) A subset X of S is called relatively dense if for each
 $s\in S_X$
, there is
$s\in S_X$
, there is
 $x\in X$
such that
$x\in X$
such that
 $x\le s$
.
$x\le s$
. -
(3) A subset X of S is called a presystem if it is locally full and relatively dense.
Note that any subsystem is already locally full because for a complete system S and for
![]() $s\in S$
and
$s\in S$
and
![]() $(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,
$(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
,
Remark 4.3.
-
(1) Let
 $S'$
be a subsystem generated by X. Then, we can take X as a presystem. Also, if X is finite, we can take X as a finite presystem.
$S'$
be a subsystem generated by X. Then, we can take X as a presystem. Also, if X is finite, we can take X as a finite presystem. -
(2) If X is a presystem, then
 $S_X\subseteq {\operatorname {dcl}}(X)$
. More precisely, for each
$S_X\subseteq {\operatorname {dcl}}(X)$
. More precisely, for each
 $x\in S_X\cap m(k,J)$
, there is
$x\in S_X\cap m(k,J)$
, there is
 $a_x\in X$
such that x is the unique element in S satisfying
$a_x\in X$
such that x is the unique element in S satisfying
 $S_X\models C(a_x,x)\wedge x\in m(k,J)$
. So, any embedding from
$S_X\models C(a_x,x)\wedge x\in m(k,J)$
. So, any embedding from
 $S_X$
to S is uniquely determined by the image of X.
$S_X$
to S is uniquely determined by the image of X. -
(3) Let
 $S'$
be a finitely generated sorted complete system. By (1), there is a finite presystem X generating X. Without loss of generality, we may assume that
$S'$
be a finitely generated sorted complete system. By (1), there is a finite presystem X generating X. Without loss of generality, we may assume that
 $m(k,J)\cap S'\subseteq X$
for
$m(k,J)\cap S'\subseteq X$
for
 $(k,J)\in \mathbb N\times \mathcal J^{<\mathbb N}$
with
$(k,J)\in \mathbb N\times \mathcal J^{<\mathbb N}$
with
 $X\cap m(k,J)\neq \emptyset $
. We call such a presystem nice.
$X\cap m(k,J)\neq \emptyset $
. We call such a presystem nice. -
(4) Let
 $S'$
be a finitely generated sorted complete system and let X be a nice finite presystem generating
$S'$
be a finitely generated sorted complete system and let X be a nice finite presystem generating
 $S'$
. Note that for any
$S'$
. Note that for any
 ${\mathcal {L}}_{SCS}({\mathcal {J}})$
-embedding
${\mathcal {L}}_{SCS}({\mathcal {J}})$
-embedding
 $\Phi :S'\rightarrow S'$
, the restriction
$\Phi :S'\rightarrow S'$
, the restriction
 $\Phi |_X$
is an automorphism of X. Conversely, any automorphism of X determines a unique automorphism of
$\Phi |_X$
is an automorphism of X. Conversely, any automorphism of X determines a unique automorphism of
 $S'$
by (2). Also, there is a formula
$S'$
by (2). Also, there is a formula
 $\theta _X(\bar x)$
such that
$\theta _X(\bar x)$
such that
 $SCS\models \theta _X(\bar x)\rightarrow {\operatorname {qftp}}(X)$
.
$SCS\models \theta _X(\bar x)\rightarrow {\operatorname {qftp}}(X)$
.
Recall that
![]() $S/\sim $
forms a distributive lattice, denoted by
$S/\sim $
forms a distributive lattice, denoted by
![]() $(S/\sim ,\vee ,\wedge )$
induced from the partial order
$(S/\sim ,\vee ,\wedge )$
induced from the partial order
![]() $\le $
on S (cf. [Reference Hoffmann and Lee10, Section 3.2]).
$\le $
on S (cf. [Reference Hoffmann and Lee10, Section 3.2]).
Definition 4.4. Let A and B be subsets of S.
-
(1)
 $\min A:=\{a\in A:\forall b\in A(a\le b) \}$
.
$\min A:=\{a\in A:\forall b\in A(a\le b) \}$
. -
(2)
 $A\vee B:=\min \{c\in S:[c]=[a]\vee [b],\ a\in A,\ b\in B\}$
.
$A\vee B:=\min \{c\in S:[c]=[a]\vee [b],\ a\in A,\ b\in B\}$
. -
(3) We write
 $A\le B$
if
$A\le B$
if
 $a\le b$
for every
$a\le b$
for every
 $a\in A$
and
$a\in A$
and
 $b\in B$
.
$b\in B$
. -
(4) We write
 $A\sim B$
if
$A\sim B$
if
 $a\sim b$
for every
$a\sim b$
for every
 $a\in A$
and
$a\in A$
and
 $b\in B$
.
$b\in B$
.
For
![]() $a\in S$
, we write
$a\in S$
, we write
![]() $a\vee A$
for
$a\vee A$
for
![]() $\{a\}\vee A$
. Note that if
$\{a\}\vee A$
. Note that if
![]() $A\vee B\neq \emptyset $
, then
$A\vee B\neq \emptyset $
, then
![]() $A\vee B=[a]$
for any
$A\vee B=[a]$
for any
![]() $a\in A\vee B$
.
$a\in A\vee B$
.
Remark 4.5.
-
(1) Let
 $S_1$
and
$S_1$
and
 $S_2$
be subsystems of S, and let
$S_2$
be subsystems of S, and let
 $S_0=S_1\cap S_2$
. Suppose
$S_0=S_1\cap S_2$
. Suppose
 $S_1$
is finitely generated. Then,
$S_1$
is finitely generated. Then,
 $\min S_1\neq \emptyset $
,
$\min S_1\neq \emptyset $
,
 $S_1\vee S_2\neq \emptyset $
, and
$S_1\vee S_2\neq \emptyset $
, and
 $\min S_0\sim S_1\vee S_2$
.
$\min S_0\sim S_1\vee S_2$
. -
(2) Let
 $S'$
be a sorted complete system with
$S'$
be a sorted complete system with
 $\min S'\neq \emptyset $
. Choose
$\min S'\neq \emptyset $
. Choose
 $a\in \min S'\cap m(k,J)\neq \emptyset $
. Then,
$a\in \min S'\cap m(k,J)\neq \emptyset $
. Then,
 $G(S')\cong [a]\cap m(k,J)$
and for each
$G(S')\cong [a]\cap m(k,J)$
and for each
 $x\in m(k',J')$
, there is a unique
$x\in m(k',J')$
, there is a unique
 $a_x\in [a]\cap m(k,J)$
such that x is the unique element in
$a_x\in [a]\cap m(k,J)$
such that x is the unique element in
 $S'$
satisfying
$S'$
satisfying
 $S'\models C(a_x,x)\wedge x\in m(k',J')$
.
$S'\models C(a_x,x)\wedge x\in m(k',J')$
. -
(3) A sorted complete system
 $S'$
is finitely generated if and only if
$S'$
is finitely generated if and only if
 $\mathcal G(S')$
is a finitely sorted finite group.
$\mathcal G(S')$
is a finitely sorted finite group.
Proof
![]() $(1)$
Suppose
$(1)$
Suppose
![]() $S_1$
is finitely generated by a finite subset X of
$S_1$
is finitely generated by a finite subset X of
![]() $S_1$
. We may assume that X is relatively dense. Since X is finite,
$S_1$
. We may assume that X is relatively dense. Since X is finite,
![]() $\min X\neq \emptyset $
, and since X is relatively dense,
$\min X\neq \emptyset $
, and since X is relatively dense,
![]() $\min X\subset \min S_1$
.
$\min X\subset \min S_1$
.
Next, we show that
![]() $S_1\vee S_2$
is not empty. Choose
$S_1\vee S_2$
is not empty. Choose
![]() $a_0\in \min S_1$
arbitrary. Then, there are only finitely many elements in the set
$a_0\in \min S_1$
arbitrary. Then, there are only finitely many elements in the set
![]() $\{x\in S:a_0\le x\}$
up to
$\{x\in S:a_0\le x\}$
up to
![]() $\sim $
and so there is a minimal element
$\sim $
and so there is a minimal element
![]() $c\in S$
such that
$c\in S$
such that
![]() $[c]=[a_0]\vee [b]$
for some
$[c]=[a_0]\vee [b]$
for some
![]() $b\in S_2$
. So,
$b\in S_2$
. So,
![]() $c\in S_1\vee S_2$
and
$c\in S_1\vee S_2$
and
![]() $S_1\vee S_2\neq \emptyset $
.
$S_1\vee S_2\neq \emptyset $
.
For all
![]() $a_0\in \min S_1$
and for all
$a_0\in \min S_1$
and for all
![]() $c\in S_1\vee S_2$
,
$c\in S_1\vee S_2$
,
![]() $a_0\le c$
, and so there are only finitely many elements in
$a_0\le c$
, and so there are only finitely many elements in
![]() $S_1\vee S_2$
up to
$S_1\vee S_2$
up to
![]() $\sim $
. Thus,
$\sim $
. Thus,
![]() $S_1\vee S_2\neq \emptyset $
.
$S_1\vee S_2\neq \emptyset $
.
It remains to show that
![]() $\min S_0\sim S_1\vee S_2$
. Since
$\min S_0\sim S_1\vee S_2$
. Since
![]() $S_0\subset S_1$
, we have that
$S_0\subset S_1$
, we have that
![]() $\min S_0\neq \emptyset $
. Take
$\min S_0\neq \emptyset $
. Take
![]() $c\in S_1\vee S_2$
and
$c\in S_1\vee S_2$
and
![]() $d\in \min S_0$
arbitrary. Since
$d\in \min S_0$
arbitrary. Since
![]() $d\in S_1\cap S_2$
, it is clear that
$d\in S_1\cap S_2$
, it is clear that
![]() $c\le d$
. Take
$c\le d$
. Take
![]() $a\in S_1\cap m(k,J)$
and
$a\in S_1\cap m(k,J)$
and
![]() $b\in S_2\cap m(k',J')$
such that
$b\in S_2\cap m(k',J')$
such that
![]() $[c]=[a]\vee [b]$
and
$[c]=[a]\vee [b]$
and
![]() $c\in m(kk',J_{\subset }^*(k,J))$
. Since
$c\in m(kk',J_{\subset }^*(k,J))$
. Since
![]() $S_1$
and
$S_1$
and
![]() $S_2$
are subsystems, there is
$S_2$
are subsystems, there is
![]() $c'\in S_1\cap S_2\cap m(kk',J_{\subset }^*(k,J)^{\frown } JJ')$
such that
$c'\in S_1\cap S_2\cap m(kk',J_{\subset }^*(k,J)^{\frown } JJ')$
such that
![]() $c'\sim c$
. Thus,
$c'\sim c$
. Thus,
![]() $d\le c'\le c$
.
$d\le c'\le c$
.
![]() $(2)$
Fix
$(2)$
Fix
![]() $a\in \min S'\cap m(k,J)$
for some
$a\in \min S'\cap m(k,J)$
for some
![]() $(k,J)\in \mathbb N\times {\mathcal {J}}^{<\mathbb N}$
. Since
$(k,J)\in \mathbb N\times {\mathcal {J}}^{<\mathbb N}$
. Since
![]() $G(S)$
is the inverse limit of the group
$G(S)$
is the inverse limit of the group
![]() $[x]\cap m(k',J')$
of
$[x]\cap m(k',J')$
of
![]() $x\in m(k',J')$
with the transition maps induced from C,
$x\in m(k',J')$
with the transition maps induced from C,
![]() $G(S)\cong [a]\cap m(k,J)$
. Also for each
$G(S)\cong [a]\cap m(k,J)$
. Also for each
![]() $b\in S$
, after putting
$b\in S$
, after putting
![]() $C(b,S):=\{x\in S:S\models C(b,x)\}$
,
$C(b,S):=\{x\in S:S\models C(b,x)\}$
,
![]() $C(b,S)\cap m(k',J')$
is linearly ordered by
$C(b,S)\cap m(k',J')$
is linearly ordered by
![]() $\le $
for each
$\le $
for each
![]() $(k',J')\in \mathbb N\times {\mathcal {J}}^{<\mathbb N}$
. Thus, for each
$(k',J')\in \mathbb N\times {\mathcal {J}}^{<\mathbb N}$
. Thus, for each
![]() $x\in m(k',J')$
, there is a unique
$x\in m(k',J')$
, there is a unique
![]() $a_x\in [a]\cap m(k,J)$
such that x is the unique element in S satisfying
$a_x\in [a]\cap m(k,J)$
such that x is the unique element in S satisfying
![]() $S\models C(a_x,x)\wedge x\in m(k',J')$
.
$S\models C(a_x,x)\wedge x\in m(k',J')$
.
![]() $(3)$
Let
$(3)$
Let
![]() $S'\models SCS$
. Suppose
$S'\models SCS$
. Suppose
![]() $S'$
is finitely generated by a finite presystem X of
$S'$
is finitely generated by a finite presystem X of
![]() $S'$
. Since X is relatively dense in
$S'$
. Since X is relatively dense in
![]() $S'$
and X is finite,
$S'$
and X is finite,
![]() $\min X\neq \emptyset $
and
$\min X\neq \emptyset $
and
![]() $\min X\sim \min S'$
. By Remark 4.5(2),
$\min X\sim \min S'$
. By Remark 4.5(2),
![]() $G(S')$
is finite. Also, since
$G(S')$
is finite. Also, since
![]() $S'=S_{X'}$
and
$S'=S_{X'}$
and
![]() $X'$
is finite,
$X'$
is finite,
![]() $F(S')$
is finitely generated. So,
$F(S')$
is finitely generated. So,
![]() ${\mathcal {G}}(S')=(G(S'),F(S'))$
is finitely sorted.
${\mathcal {G}}(S')=(G(S'),F(S'))$
is finitely sorted.
Conversely, suppose
![]() $\mathcal G(S')=(G(S'),F(S'))$
is a finitely sorted finite group. Since
$\mathcal G(S')=(G(S'),F(S'))$
is a finitely sorted finite group. Since
![]() ${\mathcal {N}}(G(S'))$
is finite, we may assume that
${\mathcal {N}}(G(S'))$
is finite, we may assume that
![]() $F(S')$
is generated by a pre-sorting data
$F(S')$
is generated by a pre-sorting data
![]() $\hat F$
such that for each
$\hat F$
such that for each
![]() $N\in {\mathcal {N}}(G(S'))$
,
$N\in {\mathcal {N}}(G(S'))$
,
![]() $\hat F(N)$
is finite. Define a presystem X as follows: For
$\hat F(N)$
is finite. Define a presystem X as follows: For
![]() $(k,J)\in \mathbb N\times {\mathcal {J}}^{<\mathbb N}$
,
$(k,J)\in \mathbb N\times {\mathcal {J}}^{<\mathbb N}$
,
 $$ \begin{align*}X\cap m(k,J)=\bigcup_{N\in {\mathcal{N}}(G(s')),[G(S'):N]\le k, J\in \hat F(N)}G(S')/N\times \{k\},\end{align*} $$
$$ \begin{align*}X\cap m(k,J)=\bigcup_{N\in {\mathcal{N}}(G(s')),[G(S'):N]\le k, J\in \hat F(N)}G(S')/N\times \{k\},\end{align*} $$
which is finite because
![]() $\hat F(N)$
is finite for each
$\hat F(N)$
is finite for each
![]() $N\in {\mathcal {N}}(G(S'))$
. Then, the finite presystem X generates
$N\in {\mathcal {N}}(G(S'))$
. Then, the finite presystem X generates
![]() $S'$
.
$S'$
.
From the fibre product in Definition 2.14, we have the following lemma.
Lemma 4.6. Let
![]() $S_0$
,
$S_0$
,
![]() $S_1$
, and
$S_1$
, and
![]() $S_2$
be subsystems of S and let
$S_2$
be subsystems of S and let
![]() $S_3=S_{S_1\cup S_2}$
. Suppose that
$S_3=S_{S_1\cup S_2}$
. Suppose that
![]() $\min S_1\neq \emptyset $
and
$\min S_1\neq \emptyset $
and
![]() $S_0\subset S_1\cap S_2$
. Consider the following inclusions:
$S_0\subset S_1\cap S_2$
. Consider the following inclusions:
-
•
 $\iota _{S_0,S_1}:S_0\rightarrow S_1$
,
$\iota _{S_0,S_1}:S_0\rightarrow S_1$
,
 $\iota _{S_0,S_2}:S_0\rightarrow S_2$
.
$\iota _{S_0,S_2}:S_0\rightarrow S_2$
. -
•
 $\iota _{S_1,S_3}:S_{1}\rightarrow S_{3}$
.
$\iota _{S_1,S_3}:S_{1}\rightarrow S_{3}$
. -
•
 $\iota _{S_2,S_3}:S_{2}\rightarrow S_{3}$
.
$\iota _{S_2,S_3}:S_{2}\rightarrow S_{3}$
.
-
(1) Suppose
 $S_1\vee S_2\sim \min S_0$
. Then,
$S_1\vee S_2\sim \min S_0$
. Then,
 $S_3$
is the co-fibre product of
$S_3$
is the co-fibre product of
 $S_1$
and
$S_1$
and
 $S_2$
over
$S_2$
over
 $S_0$
, that is, we have the following Cartesian diagram:
$S_0$
, that is, we have the following Cartesian diagram: 
-
(2) Let
 $S_1'$
be a subsystem of S such that
$S_1'$
be a subsystem of S such that
 $S_0\subset S_1'$
and
$S_0\subset S_1'$
and
 $S_1'\vee S_2\sim \min S_0$
. Suppose there is an isomorphism
$S_1'\vee S_2\sim \min S_0$
. Suppose there is an isomorphism
 $f:S_{1}\rightarrow S_{1}'$
making the following diagram commute:
$f:S_{1}\rightarrow S_{1}'$
making the following diagram commute: 
Then, there is an isomorphism from
 $g:S_3\rightarrow S_3'$
extending
$g:S_3\rightarrow S_3'$
extending
 $f\cup {\operatorname {id}}_{S_2}$
, where
$f\cup {\operatorname {id}}_{S_2}$
, where
 $S_3'=S_{S_1'\cup S_2}$
.
$S_3'=S_{S_1'\cup S_2}$
.
Thus, if one of
![]() $S_1$
and
$S_1$
and
![]() $S_2$
is finitely generated, the subsystem
$S_2$
is finitely generated, the subsystem
![]() $S_{S_1\cup S_2}$
is a co-fibre product of
$S_{S_1\cup S_2}$
is a co-fibre product of
![]() $S_1$
and
$S_1$
and
![]() $S_2$
over
$S_2$
over
![]() $S_1\cap S_2$
$S_1\cap S_2$
Proof For subsystems
![]() $S'\subseteq S" \subseteq S$
, we write
$S'\subseteq S" \subseteq S$
, we write
![]() $\iota _{S',S"}$
for the inclusion from
$\iota _{S',S"}$
for the inclusion from
![]() $S'$
to
$S'$
to
![]() $S"$
. Let
$S"$
. Let
![]() $S_0$
,
$S_0$
,
![]() $S_1$
, and
$S_1$
, and
![]() $S_2$
be subsystems of S and let
$S_2$
be subsystems of S and let
![]() $S_3:=S_{S_1\cup S_2}$
. Suppose
$S_3:=S_{S_1\cup S_2}$
. Suppose
![]() $\min S_1\neq \emptyset $
,
$\min S_1\neq \emptyset $
,
![]() $S_0\subset S_1\cap S_2$
, and
$S_0\subset S_1\cap S_2$
, and
![]() $S_1\vee S_2\sim \min S_0$
.
$S_1\vee S_2\sim \min S_0$
.
![]() $(1)$
Put
$(1)$
Put
![]() $G:=G(S)$
. Let
$G:=G(S)$
. Let
![]() $N_i:={\operatorname {Ker}}({\mathcal {G}}(\iota _{S_i,S}))$
so that we can identify
$N_i:={\operatorname {Ker}}({\mathcal {G}}(\iota _{S_i,S}))$
so that we can identify
![]() $G(S_i)=G/N_i$
for
$G(S_i)=G/N_i$
for
![]() $i=0,1,2,3$
. Let
$i=0,1,2,3$
. Let
and let
![]() $F_X$
be a pre-sorting data on X given as follows: For
$F_X$
be a pre-sorting data on X given as follows: For
![]() $N_1\in {\mathcal {N}}(G(S_1))$
and
$N_1\in {\mathcal {N}}(G(S_1))$
and
![]() $N_2\in {\mathcal {N}}(G(S_2))$
,
$N_2\in {\mathcal {N}}(G(S_2))$
,
We show that the diagram

is Cartesian, that is:
-
•
 $G(S_3)$
is the fibre product of
$G(S_3)$
is the fibre product of
 $G(S_1)$
and
$G(S_1)$
and
 $G(S_2)$
over
$G(S_2)$
over
 $G(S_0)$
; and
$G(S_0)$
; and -
•
 $F(S_3)$
is generated by a pre-sorting data
$F(S_3)$
is generated by a pre-sorting data
 $F_X$
.
$F_X$
.
Since
![]() $S_3=S_{S_1\cup S_2}$
and
$S_3=S_{S_1\cup S_2}$
and
![]() $S_1\vee S_2\sim \min S_0$
, we have
$S_1\vee S_2\sim \min S_0$
, we have
![]() $N_3=N_1\cap N_2$
and
$N_3=N_1\cap N_2$
and
![]() $N_0=N_1N_2$
. Recall that
$N_0=N_1N_2$
. Recall that
![]() $G/(N_1\cap N_2)$
is the fibre product of
$G/(N_1\cap N_2)$
is the fibre product of
![]() $G/N_1$
and
$G/N_1$
and
![]() $G/N_2$
over
$G/N_2$
over
![]() $G/N_1N_2$
and so
$G/N_1N_2$
and so
![]() $G(S_3)$
is the fibre product of
$G(S_3)$
is the fibre product of
![]() $G(S_1)$
and
$G(S_1)$
and
![]() $G(S_2)$
over
$G(S_2)$
over
![]() $G(S_0)$
. Since
$G(S_0)$
. Since
![]() $S_3$
is the smallest subsystem containing
$S_3$
is the smallest subsystem containing
![]() $S_1$
and
$S_1$
and
![]() $S_2$
, the sorting data
$S_2$
, the sorting data
![]() $F(S_3)$
is generated by the pre-sorting data
$F(S_3)$
is generated by the pre-sorting data
![]() $F_X$
.
$F_X$
.
![]() $(2)$
Let
$(2)$
Let
![]() $S_1'$
be a subsystem of S such that
$S_1'$
be a subsystem of S such that
![]() $S_0\subset S_1'$
and
$S_0\subset S_1'$
and
![]() $S_1'\vee S_2\sim \min S_0$
. Suppose there is an isomorphism
$S_1'\vee S_2\sim \min S_0$
. Suppose there is an isomorphism
![]() $f:S_1\rightarrow S_1'$
such that
$f:S_1\rightarrow S_1'$
such that
![]() $\iota _{S_0,S_1'}=f\circ \iota _{S_0,S_1}$
.
$\iota _{S_0,S_1'}=f\circ \iota _{S_0,S_1}$
.
Let
and let
![]() $F_{X'}$
be a pre-sorting data on
$F_{X'}$
be a pre-sorting data on
![]() $X'$
given as follows: For
$X'$
given as follows: For
![]() $N_1'\in {\mathcal {N}}(G(S_1'))$
and
$N_1'\in {\mathcal {N}}(G(S_1'))$
and
![]() $N_2\in {\mathcal {N}}(G(S_2))$
,
$N_2\in {\mathcal {N}}(G(S_2))$
,
Then, the sorting data
![]() $F(S_3')$
is generated by
$F(S_3')$
is generated by
![]() $F_{X'}$
.
$F_{X'}$
.
We first identify
 $$ \begin{align*} G(S_3)\cong G(S_1)\times_{G(S_0)}G(S_2)&,\ x\mapsto ({\mathcal{G}}(\iota_{S_1,S_3}(x),{\mathcal{G}}(\iota_{S_2,S_3})(x)),\\ G(S_3')\cong G(S_1')\times_{G(S_0)}G(S_2)&,\ x'\mapsto ({\mathcal{G}}(\iota_{S_1',S_3'}(x'),{\mathcal{G}}(\iota_{S_2,S_3'})(x')), \end{align*} $$
$$ \begin{align*} G(S_3)\cong G(S_1)\times_{G(S_0)}G(S_2)&,\ x\mapsto ({\mathcal{G}}(\iota_{S_1,S_3}(x),{\mathcal{G}}(\iota_{S_2,S_3})(x)),\\ G(S_3')\cong G(S_1')\times_{G(S_0)}G(S_2)&,\ x'\mapsto ({\mathcal{G}}(\iota_{S_1',S_3'}(x'),{\mathcal{G}}(\iota_{S_2,S_3'})(x')), \end{align*} $$
where
 $$ \begin{align*} G(S_1)\times_{G(S_0)}G(S_2)&:=\{(a,b)\in G(S_1)\times G(S_2):{\mathcal{G}}(\iota_{S_0,S_1})(a)={\mathcal{G}}(\iota_{S_0,S_2})(b)\},\\ G(S_1')\times_{G(S_0)}G(S_2)&:=\{(a',b)\in G(S_1')\times G(S_2):{\mathcal{G}}(\iota_{S_0,S_1'})(a')={\mathcal{G}}(\iota_{S_0,S_2})(b)\}, \end{align*} $$
$$ \begin{align*} G(S_1)\times_{G(S_0)}G(S_2)&:=\{(a,b)\in G(S_1)\times G(S_2):{\mathcal{G}}(\iota_{S_0,S_1})(a)={\mathcal{G}}(\iota_{S_0,S_2})(b)\},\\ G(S_1')\times_{G(S_0)}G(S_2)&:=\{(a',b)\in G(S_1')\times G(S_2):{\mathcal{G}}(\iota_{S_0,S_1'})(a')={\mathcal{G}}(\iota_{S_0,S_2})(b)\}, \end{align*} $$
and so we have an isomorphism
Since for each
![]() $N_1'\in {\mathcal {N}}(G(S_1'))$
,
$N_1'\in {\mathcal {N}}(G(S_1'))$
,
and so the isomorphism
![]() ${\mathcal {G}}(f)\times {\mathcal {G}}(\iota _{S_0,S_2}):G(S_3')\rightarrow G(S_3)$
induces an isomorphism from
${\mathcal {G}}(f)\times {\mathcal {G}}(\iota _{S_0,S_2}):G(S_3')\rightarrow G(S_3)$
induces an isomorphism from
![]() ${\mathcal {G}}(S_3')$
to
${\mathcal {G}}(S_3')$
to
![]() ${\mathcal {G}}(S_3)$
. Then, the isomorphism
${\mathcal {G}}(S_3)$
. Then, the isomorphism
![]() $\mathcal S({\mathcal {G}}(f)\times {\mathcal {G}}(\iota _{S_0,S_2})):S_3\rightarrow S_3'$
is the desired one.
$\mathcal S({\mathcal {G}}(f)\times {\mathcal {G}}(\iota _{S_0,S_2})):S_3\rightarrow S_3'$
is the desired one.
Remark 4.7. For any subsystems
![]() $S_1$
and
$S_1$
and
![]() $S_2$
of S, the subsystem
$S_2$
of S, the subsystem
![]() $S_{S_1\cup S_2}$
is a co-fibre product of
$S_{S_1\cup S_2}$
is a co-fibre product of
![]() $S_1$
and
$S_1$
and
![]() $S_2$
over
$S_2$
over
![]() $S_0$
, where
$S_0$
, where
![]() $S_0$
is the subsystem generated by
$S_0$
is the subsystem generated by
![]() $(S_1\vee S_2)\cap S_1\cap S_2$
.
$(S_1\vee S_2)\cap S_1\cap S_2$
.
Next, we introduce a notion of the co-sorted embedding property, which is a dual notion of SEP for sorted profinite groups. Let S be a sorted complete system.
Definition 4.8. We say that S has co-sorted embedding property (co-SEP) if for any finitely generated subsystems
![]() $S_1,S_2\subseteq S$
, and for any embedding
$S_1,S_2\subseteq S$
, and for any embedding
![]() $\Pi :S_2\rightarrow S_1$
and any embedding
$\Pi :S_2\rightarrow S_1$
and any embedding
![]() $\Phi :S_2\rightarrow S_2$
, there is an embedding
$\Phi :S_2\rightarrow S_2$
, there is an embedding
![]() $\Psi :S_1\rightarrow S$
such that
$\Psi :S_1\rightarrow S$
such that

where
![]() $\iota $
is the inclusion.
$\iota $
is the inclusion.
Remark 4.9. By Remark 4.3, co-SEP is first order axiomatizable in the language
![]() ${\mathcal {L}}_{SCS}({\mathcal {J}})$
. Denote the theory of sorted complete systems having co-SEP by
${\mathcal {L}}_{SCS}({\mathcal {J}})$
. Denote the theory of sorted complete systems having co-SEP by
![]() $SCS_{SEP}$
.
$SCS_{SEP}$
.
Proof For each finitely generated sorted complete systems
![]() $S_1$
and
$S_1$
and
![]() $S_2$
and for an embedding
$S_2$
and for an embedding
![]() $\Pi :S_2\rightarrow S_1$
, fix nice finite presystems
$\Pi :S_2\rightarrow S_1$
, fix nice finite presystems
![]() $X_1$
and
$X_1$
and
![]() $X_2$
generating
$X_2$
generating
![]() $S_1$
and
$S_1$
and
![]() $S_2$
respectively such that
$S_2$
respectively such that
![]() $\Pi [X_2]\subseteq X_1$
. Consider a sentence
$\Pi [X_2]\subseteq X_1$
. Consider a sentence
![]() $\varphi _{S_1,S_2,\Pi }$
given by
$\varphi _{S_1,S_2,\Pi }$
given by
 $$ \begin{align*}\forall \bar x_1\bar x_2\left(\theta_{X_1}(\bar x_1)\wedge\theta_{X_2}(\bar X_2)\rightarrow \bigwedge_{\Phi}\exists \bar x_3\!\left(\theta_{X_1}(\bar x_3)\wedge \bar x_2\subseteq \bar x_3\wedge\bigvee_{\Psi}(\Psi\circ \Pi)(\bar x_2)=\Phi(\bar x_2) \!\right) \!\right)\!,\end{align*} $$
$$ \begin{align*}\forall \bar x_1\bar x_2\left(\theta_{X_1}(\bar x_1)\wedge\theta_{X_2}(\bar X_2)\rightarrow \bigwedge_{\Phi}\exists \bar x_3\!\left(\theta_{X_1}(\bar x_3)\wedge \bar x_2\subseteq \bar x_3\wedge\bigvee_{\Psi}(\Psi\circ \Pi)(\bar x_2)=\Phi(\bar x_2) \!\right) \!\right)\!,\end{align*} $$
where
![]() $\Phi :\bar x_2\rightarrow \bar x_2$
, and
$\Phi :\bar x_2\rightarrow \bar x_2$
, and
![]() $\Psi :\bar x_1\rightarrow \bar x_3$
are
$\Psi :\bar x_1\rightarrow \bar x_3$
are
![]() ${\mathcal {L}}_{SCS}({\mathcal {J}})$
-embeddings. Then, the theory of sorted complete systems having co-SEP is given by
${\mathcal {L}}_{SCS}({\mathcal {J}})$
-embeddings. Then, the theory of sorted complete systems having co-SEP is given by
Since the category
![]() ${\operatorname {SPG}}$
of sorted profinite groups and the category of sorted complete systems are equivalent by contravariant functors, we have the following relationship between SEP and co-SEP.
${\operatorname {SPG}}$
of sorted profinite groups and the category of sorted complete systems are equivalent by contravariant functors, we have the following relationship between SEP and co-SEP.
Remark 4.10. Let
![]() $(G,F)$
be a sorted profinite group and let S be the sorted complete system of
$(G,F)$
be a sorted profinite group and let S be the sorted complete system of
![]() $(G,F)$
. By Theorem 2.22, we have that
$(G,F)$
. By Theorem 2.22, we have that
![]() $(G,F)$
has SEP if and only if
$(G,F)$
has SEP if and only if
![]() $(G,F)$
has FSEP if and only if S has co-SEP.
$(G,F)$
has FSEP if and only if S has co-SEP.
For a sorted complete system S, let
![]() ${\operatorname {coFSIm}}(S)$
be the set of isomorphism classes of finitely generated subsystems of S. We have the following analogue to [Reference Chatzidakis1, Theorem 2.2].
${\operatorname {coFSIm}}(S)$
be the set of isomorphism classes of finitely generated subsystems of S. We have the following analogue to [Reference Chatzidakis1, Theorem 2.2].
Lemma 4.11. Let
![]() $S_1$
and
$S_1$
and
![]() $S_2$
be sorted complete systems having co-SEP. Suppose
$S_2$
be sorted complete systems having co-SEP. Suppose
![]() $|S_1|=|S_2|=\aleph _0$
and
$|S_1|=|S_2|=\aleph _0$
and
![]() ${\operatorname {coFSIm}}(S_1)={\operatorname {coFSIm}}(S_2)$
. Then,
${\operatorname {coFSIm}}(S_1)={\operatorname {coFSIm}}(S_2)$
. Then,
![]() $S_1\cong S_2$
.
$S_1\cong S_2$
.
Proof We follow the proof scheme of back-and-forth argument in the proof of [Reference Chatzidakis1, Theorem 2.2]. List
![]() $S_1=\{\alpha _0,\alpha _1,\ldots \}$
and
$S_1=\{\alpha _0,\alpha _1,\ldots \}$
and
![]() $S_2=\{\beta _0,\beta _1,\ldots \}$
.
$S_2=\{\beta _0,\beta _1,\ldots \}$
.
Inductively, we will construct an increasing sequence of isomorphisms
![]() ${f_i:S_{1,i}\rightarrow S_{2,i}}$
between finitely generated subsystems
${f_i:S_{1,i}\rightarrow S_{2,i}}$
between finitely generated subsystems
![]() $S_{1,i}$
and
$S_{1,i}$
and
![]() $S_{2,i}$
of
$S_{2,i}$
of
![]() $S_1$
and
$S_1$
and
![]() $S_2$
respectively, such that for
$S_2$
respectively, such that for
![]() $i\in \omega $
,
$i\in \omega $
,
![]() $\alpha _i\in S_{1,i}$
if i is even, and
$\alpha _i\in S_{1,i}$
if i is even, and
![]() $\beta _i\in S_{2,i}$
if i is odd. For each
$\beta _i\in S_{2,i}$
if i is odd. For each
![]() $l=1,2$
, let
$l=1,2$
, let
![]() $S_{l,-1}$
be the trivial subsystem of
$S_{l,-1}$
be the trivial subsystem of
![]() $S_l$
such that
$S_l$
such that
![]() $m(k,J)(S_{l,-1})$
consists of the maximal element with respect to the partial order
$m(k,J)(S_{l,-1})$
consists of the maximal element with respect to the partial order
![]() $\le $
on
$\le $
on
![]() $S_l$
for each
$S_l$
for each
![]() $(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
. Let
$(k,J)\in {\mathbb {N}}\times {\mathcal {J}}^{<{\mathbb {N}}}$
. Let
![]() $f_{-1}:S_{1,-1}\rightarrow S_{2,-1}$
be the canonical isomorphism. Suppose that we have constructed
$f_{-1}:S_{1,-1}\rightarrow S_{2,-1}$
be the canonical isomorphism. Suppose that we have constructed
![]() $f_0,\ldots ,f_i$
. Without loss of generality, we may assume that i is odd. Suppose
$f_0,\ldots ,f_i$
. Without loss of generality, we may assume that i is odd. Suppose
![]() $S_{1,i}$
is generated by a finite presystem
$S_{1,i}$
is generated by a finite presystem
![]() $X_i$
of
$X_i$
of
![]() $S_1$
.
$S_1$
.
If
![]() $\alpha _{i+1}\in S_{1,i}$
, put
$\alpha _{i+1}\in S_{1,i}$
, put
![]() $S_{1,i+1}:=S_{1,i}$
and put
$S_{1,i+1}:=S_{1,i}$
and put
![]() $f_{i+1}:=f_i$
. If
$f_{i+1}:=f_i$
. If
![]() $\alpha _{i+1}\notin S_{1,i}$
, let
$\alpha _{i+1}\notin S_{1,i}$
, let
![]() $S_{1,i+1}$
be the subsystem generated by the finite subset
$S_{1,i+1}$
be the subsystem generated by the finite subset
![]() $X_i\cup \left ([\alpha _{i+1}]\cap m(k,J)\right )$
where
$X_i\cup \left ([\alpha _{i+1}]\cap m(k,J)\right )$
where
![]() $\alpha _{i+1}\in m(k,J)$
. Since
$\alpha _{i+1}\in m(k,J)$
. Since
![]() ${\operatorname {coFSIm}}(S_1)={\operatorname {coFSIm}}(S_2)$
, there is a subsystem
${\operatorname {coFSIm}}(S_1)={\operatorname {coFSIm}}(S_2)$
, there is a subsystem
![]() $\bar S_{2,i+1}$
of
$\bar S_{2,i+1}$
of
![]() $S_2$
which is isomorphic to
$S_2$
which is isomorphic to
![]() $S_{1,i+1}$
. Let
$S_{1,i+1}$
. Let
![]() $\bar \psi :S_{1,i+1}\rightarrow \bar S_{2,i+1}$
be an isomorphism. Note that
$\bar \psi :S_{1,i+1}\rightarrow \bar S_{2,i+1}$
be an isomorphism. Note that
![]() $\bar S_{2,i+1}$
is also finitely generated because
$\bar S_{2,i+1}$
is also finitely generated because
![]() $S_{1,i+1}$
is finitely generated. Since
$S_{1,i+1}$
is finitely generated. Since
![]() $S_2$
has co-SEP, there is an embedding
$S_2$
has co-SEP, there is an embedding
![]() $\psi :\bar S_{2,i+1}\rightarrow S_2$
to make the following diagram commute:
$\psi :\bar S_{2,i+1}\rightarrow S_2$
to make the following diagram commute:

Put
![]() $S_{2,i+1}:=\psi \circ \bar \psi [S_{1,i+1}]$
and put
$S_{2,i+1}:=\psi \circ \bar \psi [S_{1,i+1}]$
and put
![]() $f_{i+1}=\psi \circ \bar \psi $
. Note that
$f_{i+1}=\psi \circ \bar \psi $
. Note that
![]() $f_{i+1}$
extends
$f_{i+1}$
extends
![]() $f_i$
because of the following diagram:
$f_i$
because of the following diagram:

4.1
 $\omega $
-stability of sorted complete system with co-SEP
$\omega $
-stability of sorted complete system with co-SEP
From now on, we assume that
![]() ${\mathcal {J}}$
is countable.
${\mathcal {J}}$
is countable.
Remark 4.12. Let
![]() $S'$
be a finitely generated sorted complete system and let X be a nice finite presystem generating
$S'$
be a finitely generated sorted complete system and let X be a nice finite presystem generating
![]() $S'$
. Then, by Remark 4.3(4),
$S'$
. Then, by Remark 4.3(4),
![]() $S'\in {\operatorname {coFSIm}}(S)$
if and only if
$S'\in {\operatorname {coFSIm}}(S)$
if and only if
![]() $S\models \exists \bar x\theta _X(\bar x)$
.
$S\models \exists \bar x\theta _X(\bar x)$
.
As a corollary of Lemma 4.11, we have the following result analogous to [Reference Chatzidakis1, Theorem 2.3].
Theorem 4.13. Suppose that S has co-SEP. For each finitely generated sorted complete system
![]() $S'$
, fix a nice finite presystem
$S'$
, fix a nice finite presystem
![]() $X_{S'}$
generating
$X_{S'}$
generating
![]() $S'$
. Then,
$S'$
. Then,
![]() $Th(S)$
is axiomatized by
$Th(S)$
is axiomatized by
 $$ \begin{align*} &SCS_{SEP}\\ \cup&\{\exists \bar x \theta_{X_{S'}}(\bar x):S'\in {\operatorname{coFSIm}}(S) \}\\ \cup&\{\neg \exists \bar x\theta_{X_{S'}}(\bar x):S'\notin {\operatorname{coFSIm}}(S)\}. \end{align*} $$
$$ \begin{align*} &SCS_{SEP}\\ \cup&\{\exists \bar x \theta_{X_{S'}}(\bar x):S'\in {\operatorname{coFSIm}}(S) \}\\ \cup&\{\neg \exists \bar x\theta_{X_{S'}}(\bar x):S'\notin {\operatorname{coFSIm}}(S)\}. \end{align*} $$
Proof Let
![]() $S_1$
and
$S_1$
and
![]() $S_2$
be sorted complete systems. Suppose
$S_2$
be sorted complete systems. Suppose
 $$ \begin{align*} S_1,S_2\models &SCS_{SEP}\\ \cup&\{\exists \bar x \theta_{X_{S'}}(\bar x):S'\in {\operatorname{coFSIm}}(S) \}\\ \cup&\{\neg \exists \bar x\theta_{X_{S'}}(\bar x):S'\notin {\operatorname{coFSIm}}(S)\}. \end{align*} $$
$$ \begin{align*} S_1,S_2\models &SCS_{SEP}\\ \cup&\{\exists \bar x \theta_{X_{S'}}(\bar x):S'\in {\operatorname{coFSIm}}(S) \}\\ \cup&\{\neg \exists \bar x\theta_{X_{S'}}(\bar x):S'\notin {\operatorname{coFSIm}}(S)\}. \end{align*} $$
We show that
![]() $S_1\equiv S_2$
. By Remark 4.12,
$S_1\equiv S_2$
. By Remark 4.12,
![]() ${\operatorname {coFSIm}}(S_1)={\operatorname {coFSIm}}(S_2)$
and by Löwenheim–Skolem, we may assume that
${\operatorname {coFSIm}}(S_1)={\operatorname {coFSIm}}(S_2)$
and by Löwenheim–Skolem, we may assume that
![]() $S_1$
and
$S_1$
and
![]() $S_2$
are countable. Then, by Lemma 4.11,
$S_2$
are countable. Then, by Lemma 4.11,
![]() $S_1\equiv S_2$
.
$S_1\equiv S_2$
.
Next, we describe compete types of sorted complete systems with co-SEP, which is analogous to [Reference Chatzidakis1, Theorem 2.4].
Definition 4.14. For
![]() $a\in m(k,J)$
and for a subsystem
$a\in m(k,J)$
and for a subsystem
![]() $S'$
of S, we write
$S'$
of S, we write
![]() $a\vee S':=\{c\in m(kk',J_{\subseteq }^*)(k,J):[c]=[a]\vee [b], b\in \min S'\cap m(k',J')\}$
.
$a\vee S':=\{c\in m(kk',J_{\subseteq }^*)(k,J):[c]=[a]\vee [b], b\in \min S'\cap m(k',J')\}$
.
Note that
![]() $a\vee S'$
is locally full, and
$a\vee S'$
is locally full, and
![]() $c_1\sim c_2$
for any
$c_1\sim c_2$
for any
![]() $c_1,c_2\in a\vee S'$
so that
$c_1,c_2\in a\vee S'$
so that
![]() $a\vee S'$
is relatively dense.
$a\vee S'$
is relatively dense.
Theorem 4.15. Let
![]() $S\models SCS_{SEP}$
.
$S\models SCS_{SEP}$
.
-
(1) Let A be a subsystem of S and let
 $a,b\in m(k,J)$
. Then,
$a,b\in m(k,J)$
. Then,
 ${\operatorname {tp}}(a/A)={\operatorname {tp}}(b/A)$
if and only if
${\operatorname {tp}}(a/A)={\operatorname {tp}}(b/A)$
if and only if
 $a\vee A=b\vee A$
and there is an isomorphism
$a\vee A=b\vee A$
and there is an isomorphism
 $f:S_{(a\vee A)\cup \{a\}}\rightarrow S_{(b\vee A)\cup \{b\}}$
such that:
$f:S_{(a\vee A)\cup \{a\}}\rightarrow S_{(b\vee A)\cup \{b\}}$
such that:-
•
 $f\restriction _{S_{a\vee A}}={\operatorname {id}}_{S_{a\vee A}}$
.
$f\restriction _{S_{a\vee A}}={\operatorname {id}}_{S_{a\vee A}}$
. -
•
 $f(a)=b$
.
$f(a)=b$
.
-
-
(2)
 ${\operatorname {Th}}(S)$
is
${\operatorname {Th}}(S)$
is
 $\omega $
-stable.
$\omega $
-stable.
Proof We follow the proof scheme of [Reference Chatzidakis1, Theorem 2.4].
![]() $(1)$
Suppose
$(1)$
Suppose
![]() ${\operatorname {tp}}(a/A)={\operatorname {tp}}(b/A)$
. Without loss of generality, we may assume that S is saturated. Then, there is an automorphism f of S over A sending a to b. By the choice of f, we have that
${\operatorname {tp}}(a/A)={\operatorname {tp}}(b/A)$
. Without loss of generality, we may assume that S is saturated. Then, there is an automorphism f of S over A sending a to b. By the choice of f, we have that
![]() $a\vee A=b\vee A$
. Also, the restriction of f on
$a\vee A=b\vee A$
. Also, the restriction of f on
![]() $S_{(a\vee A)\cup \{a\}}$
is the desired one.
$S_{(a\vee A)\cup \{a\}}$
is the desired one.
Conversely, suppose
![]() $a\vee A=b\vee A$
and there is an isomorphism
$a\vee A=b\vee A$
and there is an isomorphism
![]() $f:S_{(a\vee A)\cup \{a\}}\rightarrow S_{(b\vee A)\cup \{b\}}$
over
$f:S_{(a\vee A)\cup \{a\}}\rightarrow S_{(b\vee A)\cup \{b\}}$
over
![]() $a\vee A$
sending a to b. Take a locally full, relatively dense, and finite subset X of A so that X contains an element
$a\vee A$
sending a to b. Take a locally full, relatively dense, and finite subset X of A so that X contains an element
![]() $x_0\in \min A$
. Take a locally full finite subset Y of
$x_0\in \min A$
. Take a locally full finite subset Y of
![]() $a\vee A$
. By the choice of
$a\vee A$
. By the choice of
![]() $x_0$
and Y, we have that:
$x_0$
and Y, we have that:
-
•
 $S_{X\cup Y}\vee S_{Y\cup \{a\}}\sim \{x_0\}\vee \{a\}\sim S_{a\vee A}\sim S_Y$
.
$S_{X\cup Y}\vee S_{Y\cup \{a\}}\sim \{x_0\}\vee \{a\}\sim S_{a\vee A}\sim S_Y$
. -
•
 $S_{X\cup Y}\vee S_{Y\cup \{b\}}\sim \{x_0\}\vee \{b\}\sim S_{b\vee A}\sim S_Y$
.
$S_{X\cup Y}\vee S_{Y\cup \{b\}}\sim \{x_0\}\vee \{b\}\sim S_{b\vee A}\sim S_Y$
.
And the restriction
![]() $f\restriction _{S_{Y\cup \{a\}}}:S_{Y\cup \{a\}}\rightarrow S_{Y\cup \{b\}}$
is an isomorphism over
$f\restriction _{S_{Y\cup \{a\}}}:S_{Y\cup \{a\}}\rightarrow S_{Y\cup \{b\}}$
is an isomorphism over
![]() $S_Y$
with
$S_Y$
with
![]() $f([a]\cap m(k,J))=[b]\cap m(k,J)$
so that we have the following diagram:
$f([a]\cap m(k,J))=[b]\cap m(k,J)$
so that we have the following diagram:

By Lemma 4.6, there is an isomorphism
![]() $g:S_{X\cup Y\cup \{a\}}\rightarrow S_{X\cup Y\cup \{b\}}$
over
$g:S_{X\cup Y\cup \{a\}}\rightarrow S_{X\cup Y\cup \{b\}}$
over
![]() $S_{X\cup Y}$
. Take a countable elementary substructure
$S_{X\cup Y}$
. Take a countable elementary substructure
![]() $S'$
of S containing
$S'$
of S containing
![]() $X,Y,a,b$
so that
$X,Y,a,b$
so that
![]() $S_{X\cup Y\cup \{a\}}$
and
$S_{X\cup Y\cup \{a\}}$
and
![]() $S_{X\cup Y\cup \{b\}}$
are subsystems of
$S_{X\cup Y\cup \{b\}}$
are subsystems of
![]() $S'$
. By the back-and-forth argument with co-SEP, we extend the isomorphism g into an automorphism of
$S'$
. By the back-and-forth argument with co-SEP, we extend the isomorphism g into an automorphism of
![]() $S'$
and we have that
$S'$
and we have that
![]() ${\operatorname {tp}}_{S'}(a/S_X)={\operatorname {tp}}_{S'}(b/S_X)$
where
${\operatorname {tp}}_{S'}(a/S_X)={\operatorname {tp}}_{S'}(b/S_X)$
where
![]() ${\operatorname {tp}}_{S'}(a/S_X)$
is the complete type of a over
${\operatorname {tp}}_{S'}(a/S_X)$
is the complete type of a over
![]() $S_X$
in
$S_X$
in
![]() $S'$
. Since
$S'$
. Since
![]() $S'$
is an elementary substructure of S, we have that
$S'$
is an elementary substructure of S, we have that
![]() ${\operatorname {tp}}(a/S_X)={\operatorname {tp}}(b/S_X)$
, and so by compactness,
${\operatorname {tp}}(a/S_X)={\operatorname {tp}}(b/S_X)$
, and so by compactness,
![]() ${\operatorname {tp}}(a/A)={\operatorname {tp}}(b/A)$
.
${\operatorname {tp}}(a/A)={\operatorname {tp}}(b/A)$
.
![]() $(2)$
As noted in the proof of [Reference Chatzidakis1, Theorem 2.4], it is enough to show that for a countable subset A of S, there are only countably many unary types over A.
$(2)$
As noted in the proof of [Reference Chatzidakis1, Theorem 2.4], it is enough to show that for a countable subset A of S, there are only countably many unary types over A.
Let A be a countable subset of S. Without loss of generality, we may assume that A is a subsystem of S and S is
![]() $\aleph _1$
-saturated. By
$\aleph _1$
-saturated. By
![]() $(1)$
, for each
$(1)$
, for each
![]() $a\in m(k,J)$
,
$a\in m(k,J)$
,
![]() ${\operatorname {tp}}(a/A)$
is determined by the isomorphism classes of
${\operatorname {tp}}(a/A)$
is determined by the isomorphism classes of
![]() $S_{a\vee A\cup \{a\}}$
over
$S_{a\vee A\cup \{a\}}$
over
![]() $S_{a\vee A}$
. Since A is countable and
$S_{a\vee A}$
. Since A is countable and
![]() ${\mathcal {J}}$
is countable, there are only countably many possibilities of
${\mathcal {J}}$
is countable, there are only countably many possibilities of
![]() $a\vee A$
for each
$a\vee A$
for each
![]() $a\in m(k,J)$
. Also,
$a\in m(k,J)$
. Also,
![]() $a\vee A\cup ([a]\cap m(k,J))$
is locally full and dense in
$a\vee A\cup ([a]\cap m(k,J))$
is locally full and dense in
![]() $S_{a\vee A\cup \{a\}}$
because
$S_{a\vee A\cup \{a\}}$
because
![]() $a\le s$
for all
$a\le s$
for all
![]() $s\in S_{a\vee A\cup \{a\}}$
. Thus, the isomorphism from
$s\in S_{a\vee A\cup \{a\}}$
. Thus, the isomorphism from
![]() $S_{a\vee A\cup \{a\}}$
is determined by the image of
$S_{a\vee A\cup \{a\}}$
is determined by the image of
![]() $a\vee A\cup ([a]\cap m(k,J))$
. Thus, for each
$a\vee A\cup ([a]\cap m(k,J))$
. Thus, for each
![]() $a\in m(k,J)$
, the number of the isomorphism classes of
$a\in m(k,J)$
, the number of the isomorphism classes of
![]() $S_{a\vee A\cup \{a\}}$
over
$S_{a\vee A\cup \{a\}}$
over
![]() $a\vee A$
is bounded by the number of isomorphism classes of
$a\vee A$
is bounded by the number of isomorphism classes of
![]() $[a]$
, which is finite. Therefore, there are only countably many types
$[a]$
, which is finite. Therefore, there are only countably many types
![]() ${\operatorname {tp}}(a/A)$
for each
${\operatorname {tp}}(a/A)$
for each
![]() $a\in m(k,J)$
.
$a\in m(k,J)$
.
4.2 Description of forking
In this subsection, we aim to describe forking independence and U-rank in sorted complete systems having co-SEP. We basically follow the proof scheme in [Reference Chatzidakis1, Section 4]. We fix a complete extension T of
![]() $SCS_{SEP}$
and assume that
$SCS_{SEP}$
and assume that
![]() $S\models T$
.
$S\models T$
.
Definition 4.16 [Reference Chatzidakis1, Definition 1.10].
Let
![]() $a\le b\in S$
. The length of a over b, denoted by
$a\le b\in S$
. The length of a over b, denoted by
![]() $L(a/b)$
is the largest integer n such that there exists a chain
$L(a/b)$
is the largest integer n such that there exists a chain
Lemma 4.17 [Reference Chatzidakis1, Lemma 4.1].
Let
![]() $a<b\in S$
and let
$a<b\in S$
and let
![]() $A\subset S$
. Suppose
$A\subset S$
. Suppose
![]() $b\in a\vee A$
. Then,
$b\in a\vee A$
. Then,
Proof If
![]() $b\in \min A$
, then it holds trivially. So, we assume that
$b\in \min A$
, then it holds trivially. So, we assume that
![]() $\{b\}>\min A$
. It is enough to show the left-to-right implication holds. Suppose
$\{b\}>\min A$
. It is enough to show the left-to-right implication holds. Suppose
![]() $a\in {\operatorname {acl}}(A)$
. We use induction on
$a\in {\operatorname {acl}}(A)$
. We use induction on
![]() $L(a/b)$
. Suppose
$L(a/b)$
. Suppose
![]() $L(a/b)=1$
. Without loss of generality, we may assume that S is
$L(a/b)=1$
. Without loss of generality, we may assume that S is
![]() $(\aleph _0+|A|)^+$
-saturated.
$(\aleph _0+|A|)^+$
-saturated.
Suppose
![]() $a\in {\operatorname {acl}}(A)\setminus {\operatorname {acl}}(b)$
. Then,
$a\in {\operatorname {acl}}(A)\setminus {\operatorname {acl}}(b)$
. Then,
![]() ${\operatorname {tp}}(a/{\operatorname {acl}}(b))$
has
${\operatorname {tp}}(a/{\operatorname {acl}}(b))$
has
![]() $(\aleph _0+|A|)^+$
-many realizations in S and so there are infinitely many realizations
$(\aleph _0+|A|)^+$
-many realizations in S and so there are infinitely many realizations
![]() $a_0,a_1,\ldots $
of
$a_0,a_1,\ldots $
of
![]() ${\operatorname {tp}}(a/{\operatorname {acl}}(b))$
outside of A. Since
${\operatorname {tp}}(a/{\operatorname {acl}}(b))$
outside of A. Since
![]() $a_i\models {\operatorname {tp}}(a/{\operatorname {acl}}(b))$
, we have that
$a_i\models {\operatorname {tp}}(a/{\operatorname {acl}}(b))$
, we have that
![]() $L(a_i/b)=1$
. Note that
$L(a_i/b)=1$
. Note that
![]() $\{a_i\},\min A<\{b\}$
and
$\{a_i\},\min A<\{b\}$
and
![]() $L(a_i/b)=1$
for each
$L(a_i/b)=1$
for each
![]() $i=0,1,\ldots $
. So, for each
$i=0,1,\ldots $
. So, for each
![]() $i=0,1,\ldots $
,
$i=0,1,\ldots $
,
![]() $a_i\vee A\le \{b\}$
. If
$a_i\vee A\le \{b\}$
. If
![]() $a_i\vee A<\{b\}$
, then
$a_i\vee A<\{b\}$
, then
![]() $b\notin a\vee A$
, which contradicts the assumption that
$b\notin a\vee A$
, which contradicts the assumption that
![]() $b\in a\vee A$
. So,
$b\in a\vee A$
. So,
![]() $b\in a_i\vee A$
and
$b\in a_i\vee A$
and
![]() $a\vee A=a_i\vee A=[b]$
for all i. Because
$a\vee A=a_i\vee A=[b]$
for all i. Because
![]() $b\le x$
for all
$b\le x$
for all
![]() $x\in S_{a\vee A}$
,
$x\in S_{a\vee A}$
,
![]() $S_{a\vee A}\subseteq {\operatorname {acl}}(b)$
. Since
$S_{a\vee A}\subseteq {\operatorname {acl}}(b)$
. Since
![]() $a_i\models {\operatorname {tp}}(a/{\operatorname {acl}}(b))$
,
$a_i\models {\operatorname {tp}}(a/{\operatorname {acl}}(b))$
,
![]() $a_i\models {\operatorname {tp}}(a/S_{a\vee A})={\operatorname {tp}}(a/S_{a_i\vee A})$
. By Theorem 4.15(1), there is an
$a_i\models {\operatorname {tp}}(a/S_{a\vee A})={\operatorname {tp}}(a/S_{a_i\vee A})$
. By Theorem 4.15(1), there is an
![]() ${\mathcal {L}}_{SCS}({\mathcal {J}})$
-isomorphism from
${\mathcal {L}}_{SCS}({\mathcal {J}})$
-isomorphism from
![]() $S_{(a_i\vee A)\cup \{a_i\}}$
to
$S_{(a_i\vee A)\cup \{a_i\}}$
to
![]() $S_{(a\vee A)\cup \{a\}}$
sending
$S_{(a\vee A)\cup \{a\}}$
sending
![]() $a_i$
to a and fixing
$a_i$
to a and fixing
![]() $S_{a\vee A}=S_{a_i\vee A}$
pointwise. In summary, we have that:
$S_{a\vee A}=S_{a_i\vee A}$
pointwise. In summary, we have that:
-
•
 $a_i\vee A=a\vee A$
; and
$a_i\vee A=a\vee A$
; and -
• there is an
 ${\mathcal {L}}_{SCS}({\mathcal {J}})$
-isomorphism
${\mathcal {L}}_{SCS}({\mathcal {J}})$
-isomorphism
 $S_{a_i\vee A\cup \{a_i\}}$
to
$S_{a_i\vee A\cup \{a_i\}}$
to
 $S_{a\vee A\cup \{a\}}$
sending
$S_{a\vee A\cup \{a\}}$
sending
 $a_i$
to a and fixing
$a_i$
to a and fixing
 $S_{a\vee A}=S_{a_i\vee A}$
pointwise.
$S_{a\vee A}=S_{a_i\vee A}$
pointwise.
Therefore, by Theorem 4.15(1),
![]() $a_i\models {\operatorname {tp}}(a/A)$
. Since
$a_i\models {\operatorname {tp}}(a/A)$
. Since
![]() $a_i$
’s are distinct,
$a_i$
’s are distinct,
![]() $a\not \in {\operatorname {acl}}(A)$
, a contradiction.
$a\not \in {\operatorname {acl}}(A)$
, a contradiction.
Suppose
![]() $L(a/b)=n\ge 2$
. Take
$L(a/b)=n\ge 2$
. Take
![]() $a=a_0<a_1<\cdots <a_n=b$
. Note that each
$a=a_0<a_1<\cdots <a_n=b$
. Note that each
![]() $a_i$
is in
$a_i$
is in
![]() ${\operatorname {acl}}(A)$
because
${\operatorname {acl}}(A)$
because
![]() $a\in {\operatorname {acl}}(A)$
. Since
$a\in {\operatorname {acl}}(A)$
. Since
![]() $\{b\}>\min A$
, we have that
$\{b\}>\min A$
, we have that
![]() $a_{i+1}\in a_i\vee S_{A\cup \{a_{i+1}\}}$
for each i. Since
$a_{i+1}\in a_i\vee S_{A\cup \{a_{i+1}\}}$
for each i. Since
![]() $L(a_i/a_{i+1})=1$
, by induction,
$L(a_i/a_{i+1})=1$
, by induction,
![]() $a_i\in {\operatorname {acl}}(a_{i+1})$
for each i. Thus,
$a_i\in {\operatorname {acl}}(a_{i+1})$
for each i. Thus,
![]() $a=a_0\in {\operatorname {acl}}(a_n)={\operatorname {acl}}(b)$
.
$a=a_0\in {\operatorname {acl}}(a_n)={\operatorname {acl}}(b)$
.
We describe forking independence, analogous to [Reference Chatzidakis1, Proposition 4.1].
Proposition 4.18. Let
![]() $A\subseteq B$
be substructures of S and let
$A\subseteq B$
be substructures of S and let
![]() $a\in S$
. Then,
$a\in S$
. Then,
Proof Without loss of generality, we may assume that A and B are algebraically closed. Since
![]() $\min A\ge \min B$
, we have that
$\min A\ge \min B$
, we have that
![]() $a\vee A\ge a\vee B$
. Since
$a\vee A\ge a\vee B$
. Since
![]() $\{a\}, \min B\le a\vee B$
, we have that
$\{a\}, \min B\le a\vee B$
, we have that
![]() $a\vee B\subseteq {\operatorname {acl}}(a)\cap {\operatorname {acl}}(B)$
. So, if
$a\vee B\subseteq {\operatorname {acl}}(a)\cap {\operatorname {acl}}(B)$
. So, if
![]() $a\vee B\not \subseteq {\operatorname {acl}}(A)$
, then
$a\vee B\not \subseteq {\operatorname {acl}}(A)$
, then
![]() $B{\mathop { \not \smile \hskip -0.9em ^| \ }}_A a$
. Namely, if
$B{\mathop { \not \smile \hskip -0.9em ^| \ }}_A a$
. Namely, if
![]() $B{\mathop {\smile \hskip -0.9em ^| \ }}_A a$
, then
$B{\mathop {\smile \hskip -0.9em ^| \ }}_A a$
, then
![]() ${\operatorname {acl}}(B){\mathop {\smile \hskip -0.9em ^| \ }}_{{\operatorname {acl}}(A)}{\operatorname {acl}}(a)$
and so
${\operatorname {acl}}(B){\mathop {\smile \hskip -0.9em ^| \ }}_{{\operatorname {acl}}(A)}{\operatorname {acl}}(a)$
and so
![]() $a\vee B{\mathop {\smile \hskip -0.9em ^| \ }}_{{\operatorname {acl}}(A)}a\vee B$
by monotonicity of non-forking and the fact that
$a\vee B{\mathop {\smile \hskip -0.9em ^| \ }}_{{\operatorname {acl}}(A)}a\vee B$
by monotonicity of non-forking and the fact that
![]() $a\vee B\subseteq {\operatorname {acl}}(B)\cap {\operatorname {acl}}(a)$
, which implies
$a\vee B\subseteq {\operatorname {acl}}(B)\cap {\operatorname {acl}}(a)$
, which implies
![]() $a\vee B\subseteq {\operatorname {acl}}(A)$
, a contradiction. Suppose
$a\vee B\subseteq {\operatorname {acl}}(A)$
, a contradiction. Suppose
![]() $a\vee B\subseteq {\operatorname {acl}}(A)=A$
so that
$a\vee B\subseteq {\operatorname {acl}}(A)=A$
so that
![]() $a\vee B=a\vee A$
and
$a\vee B=a\vee A$
and
![]() $S_{a\vee B}\subseteq A$
. Consider the following partial type over B,
$S_{a\vee B}\subseteq A$
. Consider the following partial type over B,
By Theorem 4.15(1), the partial type
![]() $\Sigma $
is consistent and
$\Sigma $
is consistent and
![]() $\Sigma \models {\operatorname {tp}}(a/B)$
. Since
$\Sigma \models {\operatorname {tp}}(a/B)$
. Since
![]() $a\vee B=a\vee A\subseteq A$
, the type
$a\vee B=a\vee A\subseteq A$
, the type
![]() ${\operatorname {tp}}(a/B)$
is definable over A and it does not fork over A. The second equivalence comes from Lemma 4.17.
${\operatorname {tp}}(a/B)$
is definable over A and it does not fork over A. The second equivalence comes from Lemma 4.17.
By the same proof of [Reference Chatzidakis1, Theorem 4.2], we have the following description of U-rank.
Theorem 4.19. Let
![]() $a\in S$
and
$a\in S$
and
![]() $A\subset S$
. Let
$A\subset S$
. Let
![]() $n=L(a/b)$
for some (equivalently, any)
$n=L(a/b)$
for some (equivalently, any)
![]() $b\in a\vee S_A$
. Choose a sequence
$b\in a\vee S_A$
. Choose a sequence
![]() $a=a_0<a_1<\cdots <a_n=b$
. Then, the U-rank of
$a=a_0<a_1<\cdots <a_n=b$
. Then, the U-rank of
![]() ${\operatorname {tp}}(a/A)$
is the number of indices
${\operatorname {tp}}(a/A)$
is the number of indices
![]() $i<n$
such that
$i<n$
such that
![]() $a_i\not \in {\operatorname {acl}}(a_{i+1})$
.
$a_i\not \in {\operatorname {acl}}(a_{i+1})$
.
We end our paper with the following question.
Question 4.20. We know by [Reference Waterhouse14, Theorem 2] that any profinite group is the Galois group of some field extension, that is, for a given profinite group G, there is a Galois extension L of K whose Galois group is G. More generally, Hoffmann in [Reference Hoffmann9, Corollary 3.3] showed that given a stable theory T eliminating quantifiers and imaginaries, any profinite group is the Galois group of a Galois extension in a monster model, that is, for a given profinite group G, there is a Galois extension
![]() $K\subseteq L\subset \mathfrak {C}$
with
$K\subseteq L\subset \mathfrak {C}$
with
![]() $G(L/K)\cong G$
where
$G(L/K)\cong G$
where
![]() $\mathfrak {C}$
is a monster model of T.
$\mathfrak {C}$
is a monster model of T.
We ask whether the following generalisation holds. Fix a language
![]() ${\mathcal {L}}$
with a set
${\mathcal {L}}$
with a set
![]() ${\mathcal {J}}$
of all sorts with the functions
${\mathcal {J}}$
of all sorts with the functions
![]() $J^*_{\cap }$
and
$J^*_{\cap }$
and
![]() $J^*_{\subseteq }$
given in Example 2.3. Is there a stable
$J^*_{\subseteq }$
given in Example 2.3. Is there a stable
![]() ${\mathcal {L}}$
-theory eliminating quantifiers and imaginaries such that for a monster model
${\mathcal {L}}$
-theory eliminating quantifiers and imaginaries such that for a monster model
![]() $\mathfrak {C}$
of T, any sorted profinite group
$\mathfrak {C}$
of T, any sorted profinite group
![]() $(G,F)$
is a Galois group of a Galois extension
$(G,F)$
is a Galois group of a Galois extension
![]() $K\subseteq L\subset \mathfrak {C}$
, that is, for the Galois group
$K\subseteq L\subset \mathfrak {C}$
, that is, for the Galois group
![]() $(G(L/K),F_{G(L/K)})$
defined in Example 2.3, there is a isomorphism
$(G(L/K),F_{G(L/K)})$
defined in Example 2.3, there is a isomorphism
![]() $\varphi :(G,F)\rightarrow (G(L/K),F_{G(L/K)})$
such that
$\varphi :(G,F)\rightarrow (G(L/K),F_{G(L/K)})$
such that
![]() $F_{G(L/K)}=\varphi _*(F)$
?
$F_{G(L/K)}=\varphi _*(F)$
?
Acknowledgments
The author thanks Dan Haran for his kind answers on several questions on [Reference Fried, Haran and Jarden5] and his recommending a reference from [Reference Fried and Jarden6]. Also, the author thanks the anonymous referee for careful reading and all the comments and suggestions.
Funding
This research was supported by Changwon National University in 2023–2024. The author is supported by KAIST Advanced Institute for Science-X fellowship.