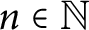1 Introduction
The volume of a measurable set
![]() $M\subset \mathbb {R}^n$
, i.e., its n-dimensional Lebesgue measure, is denoted by
$M\subset \mathbb {R}^n$
, i.e., its n-dimensional Lebesgue measure, is denoted by
![]() $\mathrm {vol}(M)$
and, in particular, we write
$\mathrm {vol}(M)$
and, in particular, we write
![]() $\kappa _n:=\mathrm {vol}(B_n)$
for the volume of the n-dimensional Euclidean unit ball
$\kappa _n:=\mathrm {vol}(B_n)$
for the volume of the n-dimensional Euclidean unit ball
![]() $B_n$
.
$B_n$
.
For two convex bodies (i.e., nonempty compact and convex sets)
![]() $K,E\subset \mathbb {R}^n$
and a non-negative real number
$K,E\subset \mathbb {R}^n$
and a non-negative real number
![]() $\lambda $
, the volume of the Minkowski sum
$\lambda $
, the volume of the Minkowski sum
![]() $K+\lambda E$
is a polynomial of degree at most n in
$K+\lambda E$
is a polynomial of degree at most n in
![]() $\lambda $
, and it is written as
$\lambda $
, and it is written as
 $$ \begin{align} \mathrm{vol}(K+\lambda E)=\sum_{i=0}^n\binom{n}{i}\mathrm{W}_i(K;E)\lambda^i. \end{align} $$
$$ \begin{align} \mathrm{vol}(K+\lambda E)=\sum_{i=0}^n\binom{n}{i}\mathrm{W}_i(K;E)\lambda^i. \end{align} $$
This expression is called the Steiner formula of K and E. The coefficients
![]() $\mathrm {W}_i(K;E)$
are the relative quermassintegrals of K w.r.t. E, and they are a special case of the more general mixed volumes, for which we refer to [Reference Shephard17, Section 5.1]. In particular,
$\mathrm {W}_i(K;E)$
are the relative quermassintegrals of K w.r.t. E, and they are a special case of the more general mixed volumes, for which we refer to [Reference Shephard17, Section 5.1]. In particular,
![]() $\mathrm {W}_0(K;E)=\mathrm {vol}(K)$
and
$\mathrm {W}_0(K;E)=\mathrm {vol}(K)$
and
![]() $\mathrm {W}_n(K;E)=\mathrm {vol}(E)$
.
$\mathrm {W}_n(K;E)=\mathrm {vol}(E)$
.
If we regard the right-hand side of (1.1) as a formal polynomial in a complex variable
![]() $z\in \mathbb {C}$
, the study of its roots has been investigated in several papers [Reference Green and Osher4, Reference Henk and Hernández Cifre6–Reference Hernández Cifre and Tárraga9, Reference Katsnelson11, Reference Lutwak12]: topology of the cone of roots, monotonicity with respect to the dimension, stability, and so on. We emphasize that most of these results are based on the characterization of the relative quermassintegrals via the well-known Aleksandrov–Fenchel inequalities
$z\in \mathbb {C}$
, the study of its roots has been investigated in several papers [Reference Green and Osher4, Reference Henk and Hernández Cifre6–Reference Hernández Cifre and Tárraga9, Reference Katsnelson11, Reference Lutwak12]: topology of the cone of roots, monotonicity with respect to the dimension, stability, and so on. We emphasize that most of these results are based on the characterization of the relative quermassintegrals via the well-known Aleksandrov–Fenchel inequalities
(see, e.g., [Reference Shephard17, (7.66)]). This characterization problem was solved in [Reference Shephard18] and [Reference Henk, Hernández Cifre and Saorín8]: any given set of
![]() $n+1$
non-negative real numbers
$n+1$
non-negative real numbers
![]() $\mathrm {W}_0,\dots ,\mathrm {W}_n\geq 0$
satisfying the inequalities
$\mathrm {W}_0,\dots ,\mathrm {W}_n\geq 0$
satisfying the inequalities
![]() $\mathrm {W}_i^2\geq \mathrm {W}_{i-1}\mathrm {W}_{i+1}$
,
$\mathrm {W}_i^2\geq \mathrm {W}_{i-1}\mathrm {W}_{i+1}$
,
![]() $1\leq i\leq n-1$
, arises as the set of relative quermassintegrals of two convex bodies. Such a tuple
$1\leq i\leq n-1$
, arises as the set of relative quermassintegrals of two convex bodies. Such a tuple
![]() $(\mathrm {W}_0,\dots ,\mathrm {W}_n)$
is called log-concave. Therefore, Steiner polynomials are precisely those ones whose coefficients, up to the combinatorial numbers, form a log-concave tuple.
$(\mathrm {W}_0,\dots ,\mathrm {W}_n)$
is called log-concave. Therefore, Steiner polynomials are precisely those ones whose coefficients, up to the combinatorial numbers, form a log-concave tuple.
As a natural counterpart to the above issue, we consider the family of those nth degree polynomials whose coefficients (up to the combinatorial numbers) form a log-convex sequence. From now on,
![]() $n\geq 2$
.
$n\geq 2$
.
Definition 1.1 We say that a polynomial has log-convex coefficients if it is of the form
 $$ \begin{align*} f_{\boldsymbol{\omega}}(z)=\sum_{i=0}^n\binom{n}{i}\omega_iz^i \end{align*} $$
$$ \begin{align*} f_{\boldsymbol{\omega}}(z)=\sum_{i=0}^n\binom{n}{i}\omega_iz^i \end{align*} $$
with
![]() $\omega _i\geq 0$
and
$\omega _i\geq 0$
and
![]() ${\boldsymbol {\omega }}=(\omega _0,\omega _1,\dots ,\omega _n)$
a log-convex tuple, i.e., satisfying
${\boldsymbol {\omega }}=(\omega _0,\omega _1,\dots ,\omega _n)$
a log-convex tuple, i.e., satisfying
![]() $\omega _i^2\leq \omega _{i-1}\omega _{i+1}$
for all
$\omega _i^2\leq \omega _{i-1}\omega _{i+1}$
for all
![]() $1\leq i\leq n-1$
.
$1\leq i\leq n-1$
.
As in the case of the Steiner polynomials, we are interested in investigating the structure and behavior of the set of roots of these log-convex coefficients polynomials.
In order to state our main results, we note first that if
![]() ${\boldsymbol {\omega }}=(\omega _0,\omega _1,\dots ,\omega _n)$
is a log-convex tuple, then
${\boldsymbol {\omega }}=(\omega _0,\omega _1,\dots ,\omega _n)$
is a log-convex tuple, then
-
• either
 $\omega _i>0$
for all
$\omega _i>0$
for all
 $0\leq i\leq n$
$0\leq i\leq n$
-
• or
 $\omega _1=\dots =\omega _{n-1}=0$
and
$\omega _1=\dots =\omega _{n-1}=0$
and
 $\omega _0,\omega _n\geq 0$
.
$\omega _0,\omega _n\geq 0$
.
Indeed, if
![]() $\omega _i=0$
for some
$\omega _i=0$
for some
![]() $i\in \{0,\dots ,n\}$
, then
$i\in \{0,\dots ,n\}$
, then
![]() $\omega _{i+1}=0$
if
$\omega _{i+1}=0$
if
![]() $i\leq n-2$
because
$i\leq n-2$
because
![]() $\omega _{i+1}^2\leq \omega _i\omega _{i+2}=0$
, and
$\omega _{i+1}^2\leq \omega _i\omega _{i+2}=0$
, and
![]() $\omega _{i-1}=0$
if
$\omega _{i-1}=0$
if
![]() $i\geq 2$
(now
$i\geq 2$
(now
![]() $\omega _{i-1}^2\leq \omega _{i-2}\omega _i=0$
). Thus, we can distinguish two families of log-convex finite sequences: let
$\omega _{i-1}^2\leq \omega _{i-2}\omega _i=0$
). Thus, we can distinguish two families of log-convex finite sequences: let
 $$ \begin{align*} \mathcal{L}^n & =\bigl\{{\boldsymbol{\omega}}=(\omega_0,\dots,\omega_n)\;\text{log-convex}: \omega_i\geq 0,\, i=0,\dots,n,\;{\boldsymbol{\omega}}\neq(0,\dots,0)\bigr\},\\ {\mathcal{L}}^n_{>0} & =\bigl\{{\boldsymbol{\omega}}=(\omega_0,\dots,\omega_n)\in{\mathcal{L}}^n:\omega_i>0,\, i=0,\dots,n\bigr\}. \end{align*} $$
$$ \begin{align*} \mathcal{L}^n & =\bigl\{{\boldsymbol{\omega}}=(\omega_0,\dots,\omega_n)\;\text{log-convex}: \omega_i\geq 0,\, i=0,\dots,n,\;{\boldsymbol{\omega}}\neq(0,\dots,0)\bigr\},\\ {\mathcal{L}}^n_{>0} & =\bigl\{{\boldsymbol{\omega}}=(\omega_0,\dots,\omega_n)\in{\mathcal{L}}^n:\omega_i>0,\, i=0,\dots,n\bigr\}. \end{align*} $$
Clearly,
![]() ${\mathcal {L}}^n$
can be expressed as the disjoint union
${\mathcal {L}}^n$
can be expressed as the disjoint union

From now on, we will write
![]() $\mathop{\mathrm {Re}}\nolimits (z)$
,
$\mathop{\mathrm {Re}}\nolimits (z)$
,
![]() $\mathop{\mathrm {Im}}\nolimits (z)$
,
$\mathop{\mathrm {Im}}\nolimits (z)$
,
![]() $|z|$
and
$|z|$
and
![]() $\bar {z}$
to represent the real and imaginary parts, the modulus and the complex conjugate of
$\bar {z}$
to represent the real and imaginary parts, the modulus and the complex conjugate of
![]() $z\in \mathbb {C}$
, respectively. Let
$z\in \mathbb {C}$
, respectively. Let
![]() $\mathbb {C}^+=\{z\in \mathbb {C}:\mathop{\mathrm {Im}}\nolimits (z)\geq 0\}$
, and for
$\mathbb {C}^+=\{z\in \mathbb {C}:\mathop{\mathrm {Im}}\nolimits (z)\geq 0\}$
, and for
![]() $n\geq 2$
let
$n\geq 2$
let
 $$ \begin{align*} {\mathcal{R}}_{\mathcal{L}}(n) & =\bigl\{z\in\mathbb{C}^+:f_{\boldsymbol{\omega}}(z)=0 \text{ for some }{\boldsymbol{\omega}}\in{\mathcal{L}}^n\bigr\} \;\text{ and}\\ {\mathcal{R}}_{{\mathcal{L}}_{>0}}(n) & =\bigl\{z\in\mathbb{C}^+:f_{\boldsymbol{\omega}}(z)=0 \text{ for some } {\boldsymbol{\omega}}\in{\mathcal{L}}^n_{>0}\bigr\}. \end{align*} $$
$$ \begin{align*} {\mathcal{R}}_{\mathcal{L}}(n) & =\bigl\{z\in\mathbb{C}^+:f_{\boldsymbol{\omega}}(z)=0 \text{ for some }{\boldsymbol{\omega}}\in{\mathcal{L}}^n\bigr\} \;\text{ and}\\ {\mathcal{R}}_{{\mathcal{L}}_{>0}}(n) & =\bigl\{z\in\mathbb{C}^+:f_{\boldsymbol{\omega}}(z)=0 \text{ for some } {\boldsymbol{\omega}}\in{\mathcal{L}}^n_{>0}\bigr\}. \end{align*} $$
It is known (see [Reference Hernández Cifre and Saorín8]) that the set of roots of all log-concave coefficients (i.e., Steiner) polynomials in
![]() $\mathbb {C}^+$
is a closed convex cone containing the non-positive real axis
$\mathbb {C}^+$
is a closed convex cone containing the non-positive real axis
![]() $\mathbb {R}_{\leq 0}$
. In this paper, we show that log-convex coefficients polynomials share these properties.
$\mathbb {R}_{\leq 0}$
. In this paper, we show that log-convex coefficients polynomials share these properties.
Theorem 1.1
![]() ${\mathcal {R}}_{\mathcal {L}}(n)$
is a convex cone containing the non-positive real axis
${\mathcal {R}}_{\mathcal {L}}(n)$
is a convex cone containing the non-positive real axis
![]() $\mathbb {R}_{\leq 0}$
.
$\mathbb {R}_{\leq 0}$
.
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
is a convex cone containing the negative real axis
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
is a convex cone containing the negative real axis
![]() $\mathbb {R}_{<0}$
.
$\mathbb {R}_{<0}$
.
As usual in the literature, we represent by
![]() $\operatorname {\mathrm {int}} M$
,
$\operatorname {\mathrm {int}} M$
,
![]() $\mathop{\mathrm {cl}}\nolimits M$
,
$\mathop{\mathrm {cl}}\nolimits M$
,
![]() $\mathop{\mathrm {bd}}\nolimits M$
, and
$\mathop{\mathrm {bd}}\nolimits M$
, and
![]() $\mathop{\mathrm {conv}}\nolimits M$
, the interior, closure, boundary, and convex hull of M, respectively. We also stress that, from now on, any topological issue concerning subsets of
$\mathop{\mathrm {conv}}\nolimits M$
, the interior, closure, boundary, and convex hull of M, respectively. We also stress that, from now on, any topological issue concerning subsets of
![]() $\mathbb {C}$
must be understood with respect to the standard topology.
$\mathbb {C}$
must be understood with respect to the standard topology.
Theorem 1.2 The cone
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
is closed. Moreover,
${\mathcal {R}}_{{\mathcal {L}}}(n)$
is closed. Moreover,
![]() $\mathop{\mathrm {cl}}\nolimits {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)={\mathcal {R}}_{{\mathcal {L}}}(n)$
and
$\mathop{\mathrm {cl}}\nolimits {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)={\mathcal {R}}_{{\mathcal {L}}}(n)$
and
![]() $\operatorname {\mathrm {int}}{\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)=\operatorname {\mathrm {int}}{\mathcal {R}}_{{\mathcal {L}}}(n)$
.
$\operatorname {\mathrm {int}}{\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)=\operatorname {\mathrm {int}}{\mathcal {R}}_{{\mathcal {L}}}(n)$
.
These theorems will play a key role in order to get the precise description of the cones
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
and
${\mathcal {R}}_{{\mathcal {L}}}(n)$
and
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
. When
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
. When
![]() $n=2$
, it can be directly obtained from results in [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1]:
$n=2$
, it can be directly obtained from results in [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1]:
(see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Proof of Proposition 4.2]). Here, for arbitrary
![]() $n\in \mathbb {N}$
,
$n\in \mathbb {N}$
,
![]() $n\geq 3$
, we describe the cones
$n\geq 3$
, we describe the cones
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
and
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
and
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
, and show that they are determined by the nth roots of
${\mathcal {R}}_{{\mathcal {L}}}(n)$
, and show that they are determined by the nth roots of
![]() $-1$
:
$-1$
:
Theorem 1.3 Let
![]() $n\geq 3$
. Then
$n\geq 3$
. Then
 $$ \begin{align*} {\mathcal{R}}_{{\mathcal{L}}_{>0}}(n) & =\bigl\{a+b\,\mathrm{i}\in\mathbb{C}^+:b>\tan(\pi/n)a\bigr\}\quad\text{and}\\ {\mathcal{R}}_{{\mathcal{L}}}(n) & =\bigl\{a+b\,\mathrm{i}\in\mathbb{C}^+:b\geq\tan(\pi/n)a\bigr\}. \end{align*} $$
$$ \begin{align*} {\mathcal{R}}_{{\mathcal{L}}_{>0}}(n) & =\bigl\{a+b\,\mathrm{i}\in\mathbb{C}^+:b>\tan(\pi/n)a\bigr\}\quad\text{and}\\ {\mathcal{R}}_{{\mathcal{L}}}(n) & =\bigl\{a+b\,\mathrm{i}\in\mathbb{C}^+:b\geq\tan(\pi/n)a\bigr\}. \end{align*} $$
A prominent subset of this family of polynomials is the one consisting of the well-known dual Steiner polynomials (see Section 2 for its explicit definition), which have been also studied thoroughgoingly in the last years. So, many properties of this general family of log-convex coefficients polynomials will apply to the dual Steiner ones.
The paper is organized as follows: Section 2 is devoted to a brief introduction on the dual Steiner polynomials and its connection with our general family of polynomials; the main results that we obtain in this setting are also presented. Next, in the brief Section 3, we collect the classical properties on polynomials that will be needed in the proofs of our results. In Section 4, we study the structure of the set of roots of all log-convex coefficients polynomials, showing, among others, that it is a closed convex cone in the upper half-plane (Theorems 1.1 and 1.2), monotonic with respect to n, which covers its interior when n tends to infinity. We also give its precise description for all
![]() $n\in \mathbb {N}$
,
$n\in \mathbb {N}$
,
![]() $n\geq 3$
(Theorem 1.3), and derive some consequences for dual Steiner polynomials. Finally, in Section 5, we get bounds for the roots of these polynomials in terms of the coefficients. Here, we also obtain a characterization of the Euclidean ball as the only star body such that all the roots of its dual Steiner polynomial have equal real part.
$n\geq 3$
(Theorem 1.3), and derive some consequences for dual Steiner polynomials. Finally, in Section 5, we get bounds for the roots of these polynomials in terms of the coefficients. Here, we also obtain a characterization of the Euclidean ball as the only star body such that all the roots of its dual Steiner polynomial have equal real part.
2 A brief tour on dual Steiner polynomials
An outstanding extension of the classical Brunn–Minkowski theory is obtained by replacing convex bodies and the classical Minkowski addition, by another family of sets and a different additive operation: the dual Brunn–Minkowski theory (see, e.g., [Reference Shephard17, Section 9.3]), introduced by Lutwak in [Reference Lutwak13, Reference Marden14], and based on the radial addition
![]() $x\widetilde {+}y$
for
$x\widetilde {+}y$
for
![]() $x,y\in \mathbb {R}^n$
, where
$x,y\in \mathbb {R}^n$
, where
 $$ \begin{align*} x\widetilde{+}y=\left\{\begin{array}{ll} x+y, & \;\text{ if}\ x,y\ \text{are linearly dependent,}\\ 0, & \;\text{ otherwise.} \end{array}\right. \end{align*} $$
$$ \begin{align*} x\widetilde{+}y=\left\{\begin{array}{ll} x+y, & \;\text{ if}\ x,y\ \text{are linearly dependent,}\\ 0, & \;\text{ otherwise.} \end{array}\right. \end{align*} $$
In general, the radial sum
![]() $K\widetilde {+}E=\{x\widetilde {+}y:x\in K,\,y\in E\}$
of two convex bodies
$K\widetilde {+}E=\{x\widetilde {+}y:x\in K,\,y\in E\}$
of two convex bodies
![]() $K,E$
is not a convex set, but the radial sum of two star bodies is again a star body. In order to define star bodies, we call a nonempty set
$K,E$
is not a convex set, but the radial sum of two star bodies is again a star body. In order to define star bodies, we call a nonempty set
![]() $S\subset \mathbb {R}^n $
starshaped (with respect to the origin) if the segment
$S\subset \mathbb {R}^n $
starshaped (with respect to the origin) if the segment
![]() $[0,x]\subset S$
for all
$[0,x]\subset S$
for all
![]() $x\in S$
. For a compact starshaped set K its radial function
$x\in S$
. For a compact starshaped set K its radial function
![]() $\rho _K:\mathbb {S}^{n-1}\longrightarrow \mathbb {R}_{\geq 0}$
is defined by
$\rho _K:\mathbb {S}^{n-1}\longrightarrow \mathbb {R}_{\geq 0}$
is defined by
![]() $\rho _K(u)=\max \{\rho \geq 0:\rho u\in K\}$
, where, as usual,
$\rho _K(u)=\max \{\rho \geq 0:\rho u\in K\}$
, where, as usual,
![]() $\mathbb {S}^{n-1}$
represents the (
$\mathbb {S}^{n-1}$
represents the (
![]() $n-1$
)-dimensional sphere. If this function is positive and continuous then K is called a star body. In particular, any star body has nonempty interior and any convex body containing the origin in its interior is a star body. We denote by
$n-1$
)-dimensional sphere. If this function is positive and continuous then K is called a star body. In particular, any star body has nonempty interior and any convex body containing the origin in its interior is a star body. We denote by
![]() $\mathcal {S}^n_0$
the set of all star bodies in
$\mathcal {S}^n_0$
the set of all star bodies in
![]() $\mathbb {R}^n$
.
$\mathbb {R}^n$
.
It is easy to see that, for
![]() $K,E\in \mathcal {S}^n_0$
and
$K,E\in \mathcal {S}^n_0$
and
![]() $\lambda \geq 0$
, the volume of the radial sum
$\lambda \geq 0$
, the volume of the radial sum
![]() $K\widetilde {+}\lambda E=\left \{x\widetilde {+}\lambda y:x\in K,\,y\in E\right \}$
is also expressed as a polynomial of degree n in
$K\widetilde {+}\lambda E=\left \{x\widetilde {+}\lambda y:x\in K,\,y\in E\right \}$
is also expressed as a polynomial of degree n in
![]() $\lambda $
(see, e.g., [Reference Shephard17, p. 508]), the so-called (relative) dual Steiner formula, which is written as
$\lambda $
(see, e.g., [Reference Shephard17, p. 508]), the so-called (relative) dual Steiner formula, which is written as
 $$ \begin{align} \mathrm{vol}\bigl(K\widetilde{+}\lambda E\bigr) =\sum_{i=0}^n\binom{n}{i}\widetilde{\mathrm{W}}_i(K;E)\lambda^i. \end{align} $$
$$ \begin{align} \mathrm{vol}\bigl(K\widetilde{+}\lambda E\bigr) =\sum_{i=0}^n\binom{n}{i}\widetilde{\mathrm{W}}_i(K;E)\lambda^i. \end{align} $$
The coefficients
![]() $\widetilde {\mathrm {W}}_i(K;E)$
are the (relative) dual quermassintegrals of K and E, and they are special cases of the dual mixed volumes, which were introduced by Lutwak in [Reference Lutwak13] (see also [Reference Shephard17, Section 9.3]). Since star bodies have nonempty interior, it is easy to see that
$\widetilde {\mathrm {W}}_i(K;E)$
are the (relative) dual quermassintegrals of K and E, and they are special cases of the dual mixed volumes, which were introduced by Lutwak in [Reference Lutwak13] (see also [Reference Shephard17, Section 9.3]). Since star bodies have nonempty interior, it is easy to see that
![]() $\widetilde {\mathrm {W}}_i(K;E)>0$
for all
$\widetilde {\mathrm {W}}_i(K;E)>0$
for all
![]() $i=0,\dots ,n$
. Dual quermassintegrals also satisfy that
$i=0,\dots ,n$
. Dual quermassintegrals also satisfy that
![]() $\widetilde {\mathrm {W}}_0(K;E)=\mathrm {vol}(K)$
and
$\widetilde {\mathrm {W}}_0(K;E)=\mathrm {vol}(K)$
and
![]() $\widetilde {\mathrm {W}}_n(K;E)=\mathrm {vol}(E)$
, and furthermore, they are homogeneous of degree
$\widetilde {\mathrm {W}}_n(K;E)=\mathrm {vol}(E)$
, and furthermore, they are homogeneous of degree
![]() $n-i$
(respectively, degree i) in the first (respectively, second) argument. When
$n-i$
(respectively, degree i) in the first (respectively, second) argument. When
![]() $E=B_n$
, we write for short
$E=B_n$
, we write for short
![]() $\widetilde {\mathrm {W}}_i(K)=\widetilde {\mathrm {W}}_i(K;B_n)$
.
$\widetilde {\mathrm {W}}_i(K)=\widetilde {\mathrm {W}}_i(K;B_n)$
.
It is well-known that for
![]() $K,E\in \mathcal {S}^n_0$
,
$K,E\in \mathcal {S}^n_0$
,
which are the “dual” counterpart to the classical Aleksandrov–Fenchel inequalities (see, e.g., [Reference Shephard17, (9.40)]). Equality holds in (2.2) if and only if K and E are dilates.
Regarding again the right-hand side of (2.1) as a formal polynomial in a complex variable
![]() $z\in \mathbb {C}$
, which we denote by
$z\in \mathbb {C}$
, which we denote by
 $$ \begin{align*} \widetilde{f}_{K;E}(z)=\sum_{i=0}^n\binom{n}{i}\widetilde{\mathrm{W}}_i(K;E)z^i, \end{align*} $$
$$ \begin{align*} \widetilde{f}_{K;E}(z)=\sum_{i=0}^n\binom{n}{i}\widetilde{\mathrm{W}}_i(K;E)z^i, \end{align*} $$
structural properties of the set of roots of dual Steiner polynomials are investigated in [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1]. Most of these results are based on a characterization of those tuples
![]() $(\omega _0,\dots ,\omega _n)$
of real numbers for which there exist
$(\omega _0,\dots ,\omega _n)$
of real numbers for which there exist
![]() $K,E\in \mathcal {S}^n_0$
with
$K,E\in \mathcal {S}^n_0$
with
 $\widetilde {\mathrm {W}}_j(K;E)=\omega _j$
,
$\widetilde {\mathrm {W}}_j(K;E)=\omega _j$
,
![]() $j=0,\dots ,n$
, which is also proved in [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1]. This characterization is, however, much more involved than the one describing the classical quermassintegrals (see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Theorems 1.1 and 2.2]): now, the dual Aleksandrov–Fenchel inequalities are, in general, not enough in order to characterize dual quermassintegrals.
$j=0,\dots ,n$
, which is also proved in [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1]. This characterization is, however, much more involved than the one describing the classical quermassintegrals (see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Theorems 1.1 and 2.2]): now, the dual Aleksandrov–Fenchel inequalities are, in general, not enough in order to characterize dual quermassintegrals.
At this point we make a key observation. It arises from [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Lemma 2.2] that one of the star bodies in the above mentioned characterization is always an Euclidean ball. Therefore, and without loss of generality, we will always take the relative star body
![]() $E=B_n$
. Furthermore, those results in which the dual quermassintegrals characterization is not used are equally valid for an arbitrary
$E=B_n$
. Furthermore, those results in which the dual quermassintegrals characterization is not used are equally valid for an arbitrary
![]() $E\in \mathcal {S}^n_0$
without additional considerations, and so, for the sake of simplicity, we will always work with the dual quermassintegrals
$E\in \mathcal {S}^n_0$
without additional considerations, and so, for the sake of simplicity, we will always work with the dual quermassintegrals
![]() $\widetilde {\mathrm {W}}_i(K)$
.
$\widetilde {\mathrm {W}}_i(K)$
.
Thus, we write
 $$ \begin{align*} \widetilde{\mathcal{R}}(n)=\bigl\{z\in\mathbb{C}^+: \widetilde{f}_{K;B_n}(z)=0 \text{ for some }K\in\mathcal{S}^n_0\bigr\} \end{align*} $$
$$ \begin{align*} \widetilde{\mathcal{R}}(n)=\bigl\{z\in\mathbb{C}^+: \widetilde{f}_{K;B_n}(z)=0 \text{ for some }K\in\mathcal{S}^n_0\bigr\} \end{align*} $$
to represent the set of roots of all dual Steiner polynomials in the upper half-plane, which is known to be a convex cone containing the negative real axis
![]() $\mathbb {R}_{<0}$
(see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Theorem 1.3]). Since the dual quermassintegrals fulfill
$\mathbb {R}_{<0}$
(see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Theorem 1.3]). Since the dual quermassintegrals fulfill
![]() $\widetilde {\mathrm {W}}_i(K)>0$
for all
$\widetilde {\mathrm {W}}_i(K)>0$
for all
![]() $i=0,\dots ,n$
and every
$i=0,\dots ,n$
and every
![]() $K\in \mathcal {S}^n_0$
, and they satisfy the dual Aleksandrov–Fenchel inequalities (2.2), dual Steiner polynomials are particular cases of log-convex coefficients polynomials, and thus
$K\in \mathcal {S}^n_0$
, and they satisfy the dual Aleksandrov–Fenchel inequalities (2.2), dual Steiner polynomials are particular cases of log-convex coefficients polynomials, and thus
 $$ \begin{align} \widetilde{\mathcal{R}}(n)\subset{\mathcal{R}}_{{\mathcal{L}}_{>0}}(n). \end{align} $$
$$ \begin{align} \widetilde{\mathcal{R}}(n)\subset{\mathcal{R}}_{{\mathcal{L}}_{>0}}(n). \end{align} $$
But since the dual Aleksandrov–Fenchel inequalities do not characterize, in general, dual quermassintegrals, the inclusion (2.3) may be strict. However, both cones are known to coincide when
![]() $n=2$
(see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Proposition 4.2]):
$n=2$
(see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Proposition 4.2]):
 $$ \begin{align*} \widetilde{\mathcal{R}}(2)={\mathcal{R}}_{{\mathcal{L}}_{>0}}(2)=\left\{z\in\mathbb{C}^+:\mathop{\mathrm{Re}}\nolimits(z)<0\right\}. \end{align*} $$
$$ \begin{align*} \widetilde{\mathcal{R}}(2)={\mathcal{R}}_{{\mathcal{L}}_{>0}}(2)=\left\{z\in\mathbb{C}^+:\mathop{\mathrm{Re}}\nolimits(z)<0\right\}. \end{align*} $$
Singular cases turn out to be dimensions
![]() $n=2,3$
, where the dual Aleksandrov–Fenchel inequalities do characterize dual quermassintegrals (see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Proof of Proposition 4.2]) and [Reference Jetter10, Corollary 3.1], respectively). We collect both results in the following theorem:
$n=2,3$
, where the dual Aleksandrov–Fenchel inequalities do characterize dual quermassintegrals (see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Proof of Proposition 4.2]) and [Reference Jetter10, Corollary 3.1], respectively). We collect both results in the following theorem:
Theorem 2.1 Given
![]() $\omega _0,\omega _1>0$
, there exists a star body
$\omega _0,\omega _1>0$
, there exists a star body
![]() $K\in \mathcal {S}^2_0$
such that
$K\in \mathcal {S}^2_0$
such that
![]() $\widetilde {\mathrm {W}}_i(K)=\omega _i$
,
$\widetilde {\mathrm {W}}_i(K)=\omega _i$
,
![]() $i=0,1$
, if and only if either they verify the strict dual Aleksandrov–Fenchel inequality
$i=0,1$
, if and only if either they verify the strict dual Aleksandrov–Fenchel inequality
![]() $\omega _1^2<\omega _0\omega _2$
, or
$\omega _1^2<\omega _0\omega _2$
, or
![]() $\omega _i=\lambda ^{2-i}\kappa _2$
for some
$\omega _i=\lambda ^{2-i}\kappa _2$
for some
![]() $\lambda>0$
and
$\lambda>0$
and
![]() $i=0,1$
, and in this case
$i=0,1$
, and in this case
![]() $K=\lambda B_2$
.
$K=\lambda B_2$
.
Given
![]() $\omega _0,\omega _1,\omega _2>0$
, there exists a star body
$\omega _0,\omega _1,\omega _2>0$
, there exists a star body
![]() $K\in \mathcal {S}^3_0$
such that
$K\in \mathcal {S}^3_0$
such that
![]() $\widetilde {\mathrm {W}}_i(K)=\omega _i$
,
$\widetilde {\mathrm {W}}_i(K)=\omega _i$
,
![]() $i=0,1,2$
, if and only if either they verify the strict dual Aleksandrov–Fenchel inequalities
$i=0,1,2$
, if and only if either they verify the strict dual Aleksandrov–Fenchel inequalities
![]() $\omega _1^2<\omega _0\omega _2$
and
$\omega _1^2<\omega _0\omega _2$
and
![]() $\omega _2^2<\kappa _3\omega _1$
, or
$\omega _2^2<\kappa _3\omega _1$
, or
![]() $\omega _i=\lambda ^{3-i}\kappa _3$
for some
$\omega _i=\lambda ^{3-i}\kappa _3$
for some
![]() $\lambda>0$
and
$\lambda>0$
and
![]() $i=0,1,2$
, and in this case
$i=0,1,2$
, and in this case
![]() $K=\lambda B_3$
.
$K=\lambda B_3$
.
Remark 2.1 In dimension
![]() $n=4$
it is easy to see that the dual Aleksandrov–Fenchel inequalities do not characterize the dual quermassintegrals. In fact, taking
$n=4$
it is easy to see that the dual Aleksandrov–Fenchel inequalities do not characterize the dual quermassintegrals. In fact, taking
![]() $\omega _0=(4\pi ^2-6)/(\pi ^2-2)$
,
$\omega _0=(4\pi ^2-6)/(\pi ^2-2)$
,
![]() $\omega _1=2$
and
$\omega _1=2$
and
![]() $\omega _2=\omega _3=1$
, one gets
$\omega _2=\omega _3=1$
, one gets
 $$ \begin{align*} \omega_1^2-\omega_0\omega_2=\frac{-2}{\pi^2-2},\quad \omega_2^2-\omega_3\omega_1=-1,\quad \omega_3^2-\omega_2\kappa_4=1-\frac{\pi^2}{2}. \end{align*} $$
$$ \begin{align*} \omega_1^2-\omega_0\omega_2=\frac{-2}{\pi^2-2},\quad \omega_2^2-\omega_3\omega_1=-1,\quad \omega_3^2-\omega_2\kappa_4=1-\frac{\pi^2}{2}. \end{align*} $$
However, using the characterization given in [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Theorem 2.2] via some properties of particular Hankel matrices, one can see that the above numbers are not dual quermassintegrals of any star body: indeed, it is a straightforward computation to check that the Hankel matrix
 $$ \begin{align*} \left(\begin{array}{ccc} \kappa_4 & \omega_3 & \omega_2\\ \omega_3 & \omega_2 & \omega_1\\ \omega_2 & \omega_1 & \omega_0 \end{array}\right) \end{align*} $$
$$ \begin{align*} \left(\begin{array}{ccc} \kappa_4 & \omega_3 & \omega_2\\ \omega_3 & \omega_2 & \omega_1\\ \omega_2 & \omega_1 & \omega_0 \end{array}\right) \end{align*} $$
is not positive definite because its determinant vanishes.
Accordingly, the results satisfied by second/third-degree log-convex coefficients polynomials will have a more or less direct translation for dual Steiner polynomials of planar/three-dimensional star bodies, not so when
![]() $n\geq 4$
. An example of this fact arises in the following consequence of Theorem 1.3:
$n\geq 4$
. An example of this fact arises in the following consequence of Theorem 1.3:
Corollary 2.1
 $\widetilde {\mathcal {R}}(3)=\left \{a+b\,\mathrm {i}\in \mathbb {C}^+:b>\sqrt {3}\,a\right \}$
.
$\widetilde {\mathcal {R}}(3)=\left \{a+b\,\mathrm {i}\in \mathbb {C}^+:b>\sqrt {3}\,a\right \}$
.
Its proof can be found in Section 4. Another of our main results for dual Steiner polynomials, which cannot be derived from results for log-convex coefficients polynomials, is the following characterization of the Euclidean ball. Its proof will be collected in Section 5.2:
Theorem 2.2 Let
![]() $K\in \mathcal {S}^n_0$
for
$K\in \mathcal {S}^n_0$
for
![]() $n\geq 3$
, let
$n\geq 3$
, let
![]() $\gamma _i$
,
$\gamma _i$
,
![]() $i=1,\dots ,n$
, be the roots of
$i=1,\dots ,n$
, be the roots of
 $\widetilde {f}_{K;B_n}(z)$
and let
$\widetilde {f}_{K;B_n}(z)$
and let
![]() $a>0$
. Then
$a>0$
. Then
![]() $\mathop{\mathrm {Re}}\nolimits (\gamma _i)=-a$
for all
$\mathop{\mathrm {Re}}\nolimits (\gamma _i)=-a$
for all
![]() $i=1,\dots ,n$
if and only if
$i=1,\dots ,n$
if and only if
![]() $K=aB_n$
.
$K=aB_n$
.
3 Background on polynomials
Since many of our results are strongly based on specific properties which are satisfied by the roots of polynomials, in order to make the reading of the manuscript easier, we devote this brief section to those results on polynomials that will be needed in the subsequent proofs.
An important well-known result establishes that the roots of a (complex) polynomial are continuous functions of its coefficients:
Theorem 3.1 [Reference Milovanović, Mitrinović and Rassias15, Theorem (1,4)]
Let
 $$ \begin{align*} f(z) & =a_0+a_1z+\dots+a_nz^n=a_n\prod_{i=1}^r(z-z_i)^{m_i},\qquad a_n\neq 0,\\ F(z) & =(a_0+\varepsilon_0)+(a_1+\varepsilon_1)z +\dots+(a_{n-1}+\varepsilon_{n-1})z^{n-1}+a_nz^n \end{align*} $$
$$ \begin{align*} f(z) & =a_0+a_1z+\dots+a_nz^n=a_n\prod_{i=1}^r(z-z_i)^{m_i},\qquad a_n\neq 0,\\ F(z) & =(a_0+\varepsilon_0)+(a_1+\varepsilon_1)z +\dots+(a_{n-1}+\varepsilon_{n-1})z^{n-1}+a_nz^n \end{align*} $$
be complex polynomials, and let
![]() $0<r_k<\min |z_k-z_i|$
for
$0<r_k<\min |z_k-z_i|$
for
![]() $i=1,\dots ,k-1,k+1,\dots ,r$
and all
$i=1,\dots ,k-1,k+1,\dots ,r$
and all
![]() $k=1,\dots ,r$
. Then there exists
$k=1,\dots ,r$
. Then there exists
![]() $\varepsilon>0$
such that, if
$\varepsilon>0$
such that, if
![]() $|\varepsilon _i|\leq \varepsilon $
for
$|\varepsilon _i|\leq \varepsilon $
for
![]() $i=0,\dots ,n-1$
, then
$i=0,\dots ,n-1$
, then
![]() $F(z)$
has precisely
$F(z)$
has precisely
![]() $m_k$
roots in the disk
$m_k$
roots in the disk
 $\bigl \{z\in \mathbb {C}:|z-z_k|<r_k\bigr \}$
,
$\bigl \{z\in \mathbb {C}:|z-z_k|<r_k\bigr \}$
,
![]() $k=1,\dots ,r$
.
$k=1,\dots ,r$
.
The following results provide bounds for the modulus of the roots of a polynomial:
Proposition 3.1 [Reference Milovanović, Mitrinović and Rassias15, p. 137, Exercise 2]
Let
![]() $f(z)=a_0+a_1z+\dots +a_nz^n$
be a real polynomial with
$f(z)=a_0+a_1z+\dots +a_nz^n$
be a real polynomial with
![]() $a_i>0$
,
$a_i>0$
,
![]() $i=0,\dots ,n$
. Then its roots lie in the ring
$i=0,\dots ,n$
. Then its roots lie in the ring
 $$ \begin{align*} \min_{0\leq i\leq n-1}\frac{a_i}{a_{i+1}}\leq|z|\leq\max_{0\leq i\leq n-1} \frac{a_i}{a_{i+1}}. \end{align*} $$
$$ \begin{align*} \min_{0\leq i\leq n-1}\frac{a_i}{a_{i+1}}\leq|z|\leq\max_{0\leq i\leq n-1} \frac{a_i}{a_{i+1}}. \end{align*} $$
Proposition 3.2 [Reference Milovanović, Mitrinović and Rassias15, p. 126, Exercise 7]
Let
![]() $g(z)=z^n+b_1z^{n-1}+\dots +b_n$
be a complex polynomial with roots
$g(z)=z^n+b_1z^{n-1}+\dots +b_n$
be a complex polynomial with roots
![]() $z_1,\dots ,z_n$
, and let
$z_1,\dots ,z_n$
, and let
![]() $M=\max _{i=1,\dots ,n}|z_i|$
. Then
$M=\max _{i=1,\dots ,n}|z_i|$
. Then
 $$ \begin{align*} M\geq\dfrac{1}{n}\sum_{i=1}^n{{\left|\frac{b_i}{\binom{n}{i}}\right|}^{1/i}}. \end{align*} $$
$$ \begin{align*} M\geq\dfrac{1}{n}\sum_{i=1}^n{{\left|\frac{b_i}{\binom{n}{i}}\right|}^{1/i}}. \end{align*} $$
Theorem 3.2 [Reference Milovanović, Mitrinović and Rassias15, Theorem (33,3)]
Let
![]() $r\in \{1,\dots ,n-1\}$
. A complex polynomial of the form
$r\in \{1,\dots ,n-1\}$
. A complex polynomial of the form
![]() $g(z)=1+b_rz^r+\dots +b_nz^n$
with
$g(z)=1+b_rz^r+\dots +b_nz^n$
with
![]() $b_r\neq 0$
has at least r roots in the disk
$b_r\neq 0$
has at least r roots in the disk
 $\left \{z\in \mathbb {C}:|z|\leq \bigl (\binom {n}{r}/|b_r|\bigr )^{1/r}\right \}$
.
$\left \{z\in \mathbb {C}:|z|\leq \bigl (\binom {n}{r}/|b_r|\bigr )^{1/r}\right \}$
.
An important tool will be the well-known Lucas theorem on the location of the roots of the derivative (critical points) of a polynomial (see, e.g., [Reference Milovanović, Mitrinović and Rassias15, Theorem (6,1)]):
Theorem 3.3 (Lucas’ theorem)
All the critical points of a
![]() $($
nonconstant
$($
nonconstant
![]() $)$
complex polynomial
$)$
complex polynomial
![]() $f(z)$
lie in the convex hull C of the set of roots of
$f(z)$
lie in the convex hull C of the set of roots of
![]() $f(z)$
. Moreover, if the roots of
$f(z)$
. Moreover, if the roots of
![]() $f(z)$
are not collinear, then no critical point of
$f(z)$
are not collinear, then no critical point of
![]() $f(z)$
lies on the boundary of C unless it is a multiple root of
$f(z)$
lies on the boundary of C unless it is a multiple root of
![]() $f(z)$
.
$f(z)$
.
Next property provides with a relation between the imaginary parts of the roots of the derivative of a polynomial and of the polynomial itself.
Theorem 3.4 [Reference Schneider16, Theorem 1.4.1]
Let the complex polynomial
![]() $f(z)$
of degree
$f(z)$
of degree
![]() $n>1$
have the roots
$n>1$
have the roots
![]() $z_1,\dots ,z_n$
, and let
$z_1,\dots ,z_n$
, and let
![]() $w_1,\dots ,w_{n-1}$
be those of
$w_1,\dots ,w_{n-1}$
be those of
![]() $f'(z)$
. Then
$f'(z)$
. Then
 $$ \begin{align*} \frac{1}{n-1}\sum_{i=1}^{n-1}\bigl|\mathop{\mathrm{Im}}\nolimits(w_i)\bigr|\leq\frac{1}{n}\sum_{i=1}^n\bigl|\mathop{\mathrm{Im}}\nolimits(z_i)\bigr|. \end{align*} $$
$$ \begin{align*} \frac{1}{n-1}\sum_{i=1}^{n-1}\bigl|\mathop{\mathrm{Im}}\nolimits(w_i)\bigr|\leq\frac{1}{n}\sum_{i=1}^n\bigl|\mathop{\mathrm{Im}}\nolimits(z_i)\bigr|. \end{align*} $$
The last result, known as the Davenport–Pólya theorem, deals with the convolution of two log-convex sequences.
Theorem 3.5 (Davenport–Pólya’s theorem [Reference Davenport and Pólya3])
Let
![]() $(a_i)_{i=0}^n$
and
$(a_i)_{i=0}^n$
and
![]() $(b_i)_{i=0}^n$
be log-convex sequences of positive numbers. Then the sequence
$(b_i)_{i=0}^n$
be log-convex sequences of positive numbers. Then the sequence
![]() $(v_i)_{i=0}^n$
given by
$(v_i)_{i=0}^n$
given by
 $$ \begin{align*} v_i=a_0b_i+\binom{i}{1}a_1b_{i-1}+\binom{i}{2}a_2b_{i-2}+\dots+a_ib_0 \end{align*} $$
$$ \begin{align*} v_i=a_0b_i+\binom{i}{1}a_1b_{i-1}+\binom{i}{2}a_2b_{i-2}+\dots+a_ib_0 \end{align*} $$
is also log-convex.
4 The sets of roots of log-convex coefficients polynomials
In this section, we prove our main results regarding the structure of the set of roots of log-convex coefficients polynomials. We start with Theorem 1.1, for which we need the following auxiliary result:
Lemma 4.1 Let
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^n ($
respectively,
${\boldsymbol {\omega }}\in {\mathcal {L}}^n ($
respectively,
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0})$
. Then:
${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0})$
. Then:
-
(i) for every
 $\lambda>0$
, there exists
$\lambda>0$
, there exists
 ${\boldsymbol {\omega }}'\in {\mathcal {L}}^n ($
respectively,
${\boldsymbol {\omega }}'\in {\mathcal {L}}^n ($
respectively,
 ${\boldsymbol {\omega }}'\in {\mathcal {L}}^n_{>0})$
such that
${\boldsymbol {\omega }}'\in {\mathcal {L}}^n_{>0})$
such that
 $f_{\boldsymbol {\omega }}(\lambda z)=f_{{\boldsymbol {\omega }}'}(z)$
and
$f_{\boldsymbol {\omega }}(\lambda z)=f_{{\boldsymbol {\omega }}'}(z)$
and -
(ii) for all
 $a>0$
, there exists
$a>0$
, there exists
 ${\boldsymbol {\omega }}'\in {\mathcal {L}}^n ($
respectively,
${\boldsymbol {\omega }}'\in {\mathcal {L}}^n ($
respectively,
 ${\boldsymbol {\omega }}'\in {\mathcal {L}}^n_{>0})$
such that
${\boldsymbol {\omega }}'\in {\mathcal {L}}^n_{>0})$
such that
 $f_{\boldsymbol {\omega }}(z+a)=f_{{\boldsymbol {\omega }}'}(z)$
.
$f_{\boldsymbol {\omega }}(z+a)=f_{{\boldsymbol {\omega }}'}(z)$
.
Proof Let
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n$
. To see (i), it suffices to consider the tuple
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n$
. To see (i), it suffices to consider the tuple
![]() ${\boldsymbol {\omega }}'=(\omega _0,\lambda \omega _1,\dots ,\lambda ^n\omega _n)$
, and the result follows.
${\boldsymbol {\omega }}'=(\omega _0,\lambda \omega _1,\dots ,\lambda ^n\omega _n)$
, and the result follows.
To prove (ii), we have to distinguish whether
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0}$
or
${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0}$
or
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^n\backslash {\mathcal {L}}^n_{>0}$
. First we assume that
${\boldsymbol {\omega }}\in {\mathcal {L}}^n\backslash {\mathcal {L}}^n_{>0}$
. First we assume that
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0}$
. Taking into account that also the reversed tuple
${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0}$
. Taking into account that also the reversed tuple
![]() $(\omega _n,\omega _{n-1},\dots ,\omega _0)\in {\mathcal {L}}^n_{>0}$
and that
$(\omega _n,\omega _{n-1},\dots ,\omega _0)\in {\mathcal {L}}^n_{>0}$
and that
![]() $(1,a,a^2,\dots ,a^n)\in {\mathcal {L}}^n_{>0}$
, we construct the new tuple
$(1,a,a^2,\dots ,a^n)\in {\mathcal {L}}^n_{>0}$
, we construct the new tuple
 $$ \begin{align*} \upsilon_i=\sum_{k=0}^i\binom{i}{k}a^k\omega_{n-i+k},\quad 0\leq i\leq n, \end{align*} $$
$$ \begin{align*} \upsilon_i=\sum_{k=0}^i\binom{i}{k}a^k\omega_{n-i+k},\quad 0\leq i\leq n, \end{align*} $$
given by their binomial convolution, which is also a log-convex sequence as an application of Davenport–Pólya’s theorem (see Theorem 3.5). So,
![]() ${\boldsymbol {\omega }}'=(\upsilon _n,\dots ,\upsilon _0)\in {\mathcal {L}}^n_{>0}$
, and since
${\boldsymbol {\omega }}'=(\upsilon _n,\dots ,\upsilon _0)\in {\mathcal {L}}^n_{>0}$
, and since
 $\binom {n}{i}\binom {n-i}{k}=\binom {n}{i+k}\binom {i+k}{i}$
, we get
$\binom {n}{i}\binom {n-i}{k}=\binom {n}{i+k}\binom {i+k}{i}$
, we get
 $$ \begin{align*} f_{{\boldsymbol{\omega}}'}(z) & =\sum_{i=0}^n\binom{n}{i}\upsilon_{n-i}z^i =\sum_{i=0}^n\binom{n}{i}\left(\sum_{k=0}^{n-i}\binom{n-i}{k}a^k\omega_{i+k}\right)z^i\\ & =\sum_{i=0}^n\left(\sum_{k=0}^{n-i}\binom{n}{i+k}\binom{i+k}{i}a^k\omega_{i+k}\right)z^i\\ & =\sum_{i=0}^n\left(\sum_{l=i}^n\binom{n}{l}\binom{l}{i}a^{l-i}\omega_l\right)z^i =\sum_{j=0}^n\binom{n}{j}\omega_j\left(\sum_{r=0}^j\binom{j}{r}a^{j-r}z^r\right)\\ & =\sum_{j=0}^n\binom{n}{j}\omega_j(z+a)^j=f_{\boldsymbol{\omega}}(z+a). \end{align*} $$
$$ \begin{align*} f_{{\boldsymbol{\omega}}'}(z) & =\sum_{i=0}^n\binom{n}{i}\upsilon_{n-i}z^i =\sum_{i=0}^n\binom{n}{i}\left(\sum_{k=0}^{n-i}\binom{n-i}{k}a^k\omega_{i+k}\right)z^i\\ & =\sum_{i=0}^n\left(\sum_{k=0}^{n-i}\binom{n}{i+k}\binom{i+k}{i}a^k\omega_{i+k}\right)z^i\\ & =\sum_{i=0}^n\left(\sum_{l=i}^n\binom{n}{l}\binom{l}{i}a^{l-i}\omega_l\right)z^i =\sum_{j=0}^n\binom{n}{j}\omega_j\left(\sum_{r=0}^j\binom{j}{r}a^{j-r}z^r\right)\\ & =\sum_{j=0}^n\binom{n}{j}\omega_j(z+a)^j=f_{\boldsymbol{\omega}}(z+a). \end{align*} $$
Finally, we assume that
![]() ${\boldsymbol {\omega }}=(\omega _0,0,\dots ,0,\omega _n)$
with
${\boldsymbol {\omega }}=(\omega _0,0,\dots ,0,\omega _n)$
with
![]() $\omega _0,\omega _n\geq 0$
,
$\omega _0,\omega _n\geq 0$
,
![]() ${\boldsymbol {\omega }}\neq (0,\dots ,0)$
(see (1.2)). If
${\boldsymbol {\omega }}\neq (0,\dots ,0)$
(see (1.2)). If
![]() $\omega _n=0$
, the result is trivial, and so we assume that
$\omega _n=0$
, the result is trivial, and so we assume that
![]() $\omega _n>0$
. Then
$\omega _n>0$
. Then
![]() $f_{\boldsymbol {\omega }}(z)=\omega _0+\omega _nz^n$
and taking
$f_{\boldsymbol {\omega }}(z)=\omega _0+\omega _nz^n$
and taking
we have
 $$ \begin{align*} f_{{\boldsymbol{\omega}}'}(z)=\left(\sum_{i=0}^n\binom{n}{i}a^{n-i}z^i\right)\omega_n+\omega_0 =\omega_n(z+a)^n+\omega_0=f_{\boldsymbol{\omega}}(z+a).\\[-40pt] \end{align*} $$
$$ \begin{align*} f_{{\boldsymbol{\omega}}'}(z)=\left(\sum_{i=0}^n\binom{n}{i}a^{n-i}z^i\right)\omega_n+\omega_0 =\omega_n(z+a)^n+\omega_0=f_{\boldsymbol{\omega}}(z+a).\\[-40pt] \end{align*} $$
▪
Now, we are in a position to prove Theorem 1.1.
Proof of Theorem 1.1
Let
![]() $\gamma \in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
. Then there exists
$\gamma \in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
. Then there exists
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0}$
such that
${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0}$
such that
![]() $f_{\boldsymbol {\omega }}(\gamma )=0$
, and Lemma 4.1 (ii) ensures that, for all
$f_{\boldsymbol {\omega }}(\gamma )=0$
, and Lemma 4.1 (ii) ensures that, for all
![]() $a>0$
,
$a>0$
,
![]() $\gamma -a$
is a root of
$\gamma -a$
is a root of
![]() $f_{\boldsymbol {\omega }}(z+a)=f_{{\boldsymbol {\omega }}'}(z)$
for some
$f_{\boldsymbol {\omega }}(z+a)=f_{{\boldsymbol {\omega }}'}(z)$
for some
![]() ${\boldsymbol {\omega }}'\in {\mathcal {L}}^n_{>0}$
. Therefore,
${\boldsymbol {\omega }}'\in {\mathcal {L}}^n_{>0}$
. Therefore,
![]() $\gamma -a\in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
.
$\gamma -a\in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
.
Furthermore, by Lemma 4.1 (i), we know that, for any
![]() $\lambda>0$
,
$\lambda>0$
,
![]() $\lambda \gamma $
is a root of the polynomial
$\lambda \gamma $
is a root of the polynomial
![]() $f_{\boldsymbol {\omega }}(z/\lambda )=f_{{\boldsymbol {\omega }}''}(z)$
for some
$f_{\boldsymbol {\omega }}(z/\lambda )=f_{{\boldsymbol {\omega }}''}(z)$
for some
![]() ${\boldsymbol {\omega }}''\in {\mathcal {L}}^n_{>0}$
, and hence,
${\boldsymbol {\omega }}''\in {\mathcal {L}}^n_{>0}$
, and hence,
![]() $\lambda \gamma \in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
. These two properties imply that
$\lambda \gamma \in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
. These two properties imply that
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
is a convex cone. The proof concludes by noting that the tuple
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
is a convex cone. The proof concludes by noting that the tuple
![]() $(1,1,\dots ,1)\in {\mathcal {L}}^n_{>0}$
, and so
$(1,1,\dots ,1)\in {\mathcal {L}}^n_{>0}$
, and so
![]() $-1\in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
; therefore,
$-1\in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
; therefore,
![]() $\mathbb {R}_{<0}\subset {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
.
$\mathbb {R}_{<0}\subset {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
.
In the case of
![]() ${\mathcal {L}}^n$
, the argument is analogous. We just have to observe that now the tuple
${\mathcal {L}}^n$
, the argument is analogous. We just have to observe that now the tuple
![]() $(0,\dots ,0,\omega _n)\in {\mathcal {L}}^n$
for
$(0,\dots ,0,\omega _n)\in {\mathcal {L}}^n$
for
![]() $\omega _n>0$
, and thus
$\omega _n>0$
, and thus
![]() $0\in {\mathcal {R}}_{{\mathcal {L}}}(n)$
.▪
$0\in {\mathcal {R}}_{{\mathcal {L}}}(n)$
.▪
Remark 4.1 At this point, we would like to stress the pertinence of working with polynomials of the form
 $f_{\boldsymbol {\omega }}(z)=\sum _{i=0}^n\binom {n}{i}\omega _iz^i$
instead of just considering
$f_{\boldsymbol {\omega }}(z)=\sum _{i=0}^n\binom {n}{i}\omega _iz^i$
instead of just considering
![]() $\sum _{i=0}^n\omega _iz^i$
for
$\sum _{i=0}^n\omega _iz^i$
for
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0}$
(or
${\boldsymbol {\omega }}\in {\mathcal {L}}^n_{>0}$
(or
![]() ${\mathcal {L}}^n$
). The combinatorial numbers play a key role if one aims to have analogous properties to the ones of the Steiner polynomials (e.g., convexity or monotonicity). Indeed, it is an easy computation to check that the set of roots of all the second-degree polynomials
${\mathcal {L}}^n$
). The combinatorial numbers play a key role if one aims to have analogous properties to the ones of the Steiner polynomials (e.g., convexity or monotonicity). Indeed, it is an easy computation to check that the set of roots of all the second-degree polynomials
![]() $\omega _0+\omega _1z+\omega _2z^2$
, with
$\omega _0+\omega _1z+\omega _2z^2$
, with
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^2_{>0}$
, is
${\boldsymbol {\omega }}\in {\mathcal {L}}^2_{>0}$
, is
 $\left \{a+b\,\mathrm {i}\in \mathbb {C}^+:-b\leq \sqrt {3}\,a<0\right \}$
, and hence convex; however, for the third-degree polynomials
$\left \{a+b\,\mathrm {i}\in \mathbb {C}^+:-b\leq \sqrt {3}\,a<0\right \}$
, and hence convex; however, for the third-degree polynomials
![]() $\sum _{i=0}^3\omega _iz^i$
,
$\sum _{i=0}^3\omega _iz^i$
,
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^3_{>0}$
, the set of roots is contained in the union
${\boldsymbol {\omega }}\in {\mathcal {L}}^3_{>0}$
, the set of roots is contained in the union
 $\left \{a+b\mathrm {i}\in \mathbb {C}^+:b>\sqrt {3}\,|a|\right \}\cup \mathbb {R}_{<0}$
, and hence, since it trivially contains the set
$\left \{a+b\mathrm {i}\in \mathbb {C}^+:b>\sqrt {3}\,|a|\right \}\cup \mathbb {R}_{<0}$
, and hence, since it trivially contains the set
![]() $\mathbb {R}_{<0}\cup \{b\mathrm {i}:b>0\}$
, the convexity, for instance, is lost. Of course, further research can be developed for them.
$\mathbb {R}_{<0}\cup \{b\mathrm {i}:b>0\}$
, the convexity, for instance, is lost. Of course, further research can be developed for them.
Next, we investigate the topological properties of the cones
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
and
${\mathcal {R}}_{{\mathcal {L}}}(n)$
and
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
: we prove Theorem 1.2.
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
: we prove Theorem 1.2.
Proof of Theorem 1.2
Let
![]() $\gamma \in \mathop{\mathrm {bd}}\nolimits {\mathcal {R}}_{{\mathcal {L}}}(n)$
, and let
$\gamma \in \mathop{\mathrm {bd}}\nolimits {\mathcal {R}}_{{\mathcal {L}}}(n)$
, and let
![]() $(\gamma _m)_{m\in \mathbb {N}}\subset {\mathcal {R}}_{{\mathcal {L}}}(n)$
be a sequence such that
$(\gamma _m)_{m\in \mathbb {N}}\subset {\mathcal {R}}_{{\mathcal {L}}}(n)$
be a sequence such that
![]() $\lim _{m\to \infty }\gamma _m=\gamma $
. Then, for every
$\lim _{m\to \infty }\gamma _m=\gamma $
. Then, for every
![]() $m\in \mathbb {N}$
, there exists
$m\in \mathbb {N}$
, there exists
![]() ${\boldsymbol {\omega }}^m=(\omega _0^m,\dots ,\omega _n^m)\in {\mathcal {L}}^n$
such that
${\boldsymbol {\omega }}^m=(\omega _0^m,\dots ,\omega _n^m)\in {\mathcal {L}}^n$
such that
![]() $f_{{\boldsymbol {\omega }}^m}(\gamma _m)=0$
.
$f_{{\boldsymbol {\omega }}^m}(\gamma _m)=0$
.
Since
![]() $f_{{\boldsymbol {\omega }}^m}(1)>0$
because
$f_{{\boldsymbol {\omega }}^m}(1)>0$
because
![]() ${\boldsymbol {\omega }}^m\neq (0,\dots ,0)$
, without loss of generality we may assume that
${\boldsymbol {\omega }}^m\neq (0,\dots ,0)$
, without loss of generality we may assume that
![]() $f_{{\boldsymbol {\omega }}^m}(1)=1$
; otherwise one might take the tuple
$f_{{\boldsymbol {\omega }}^m}(1)=1$
; otherwise one might take the tuple
![]() ${\boldsymbol {\omega }}^m/f_{{\boldsymbol {\omega }}^m}(1)\in {\mathcal {L}}^n$
. Thus, from
${\boldsymbol {\omega }}^m/f_{{\boldsymbol {\omega }}^m}(1)\in {\mathcal {L}}^n$
. Thus, from
 $f_{{\boldsymbol {\omega }}^m}(1)=\sum _{i=0}^n\binom {n}{i}\omega _i^m$
, we get
$f_{{\boldsymbol {\omega }}^m}(1)=\sum _{i=0}^n\binom {n}{i}\omega _i^m$
, we get
![]() $\omega _i^m\in [0,1]$
for every
$\omega _i^m\in [0,1]$
for every
![]() $i=0,\dots ,n$
and all
$i=0,\dots ,n$
and all
![]() $m\in \mathbb {N}$
. Thus, a subsequence of each sequence
$m\in \mathbb {N}$
. Thus, a subsequence of each sequence
![]() $(\omega _i^m)_{m\in \mathbb {N}}$
converges to a point
$(\omega _i^m)_{m\in \mathbb {N}}$
converges to a point
![]() $\omega _i$
,
$\omega _i$
,
![]() $0\leq i\leq n$
, and without loss of generality we assume that
$0\leq i\leq n$
, and without loss of generality we assume that
![]() $\lim _{m\to \infty }\omega _i^m=\omega _i$
for all
$\lim _{m\to \infty }\omega _i^m=\omega _i$
for all
![]() $i=0,\dots ,n$
.
$i=0,\dots ,n$
.
Moreover, since
![]() $(\omega _i^m)^2\leq \omega _{i-1}^m\omega _{i+1}^m$
for all
$(\omega _i^m)^2\leq \omega _{i-1}^m\omega _{i+1}^m$
for all
![]() $m\in \mathbb {N}$
, the same inequality holds for the limit values, i.e.,
$m\in \mathbb {N}$
, the same inequality holds for the limit values, i.e.,
![]() $(\omega _i)^2\leq \omega _{i-1}\omega _{i+1}$
,
$(\omega _i)^2\leq \omega _{i-1}\omega _{i+1}$
,
![]() $1\leq i\leq n-1$
. We also note that
$1\leq i\leq n-1$
. We also note that
![]() ${\boldsymbol {\omega }}\neq (0,\dots ,0)$
because
${\boldsymbol {\omega }}\neq (0,\dots ,0)$
because
![]() $f_{{\boldsymbol {\omega }}^m}(1)=1$
for all
$f_{{\boldsymbol {\omega }}^m}(1)=1$
for all
![]() $m\in \mathbb {N}$
; hence
$m\in \mathbb {N}$
; hence
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n$
. Finally, since
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n$
. Finally, since
![]() $f_{{\boldsymbol {\omega }}^m}(\gamma _m)=0$
for every
$f_{{\boldsymbol {\omega }}^m}(\gamma _m)=0$
for every
![]() $m\in \mathbb {N}$
, also
$m\in \mathbb {N}$
, also
![]() $f_{\boldsymbol {\omega }}(\gamma )=0$
, and so
$f_{\boldsymbol {\omega }}(\gamma )=0$
, and so
![]() $\gamma \in {\mathcal {R}}_{{\mathcal {L}}}(n)$
.
$\gamma \in {\mathcal {R}}_{{\mathcal {L}}}(n)$
.
Next, we show the identities of the statement. First, we observe that the set difference
 ${\mathcal {R}}_{{\mathcal {L}}}(n)\backslash {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)\subset \bigl \{z\in \mathbb {C}^+:z^n+a=0\;\text { for some }a\geq 0\bigr \}$
. Moreover, the set in the right-hand side is the (finite) union of those rays determined by the nth roots of
${\mathcal {R}}_{{\mathcal {L}}}(n)\backslash {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)\subset \bigl \{z\in \mathbb {C}^+:z^n+a=0\;\text { for some }a\geq 0\bigr \}$
. Moreover, the set in the right-hand side is the (finite) union of those rays determined by the nth roots of
![]() $-1$
which are contained in the upper half-plane
$-1$
which are contained in the upper half-plane
![]() $\mathbb {C}^+$
. Then, taking into account that both
$\mathbb {C}^+$
. Then, taking into account that both
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
and
${\mathcal {R}}_{{\mathcal {L}}}(n)$
and
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
are convex cones (Theorem 1.1) and that they only differ, at most, in the above finite union of rays, we may conclude that
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
are convex cones (Theorem 1.1) and that they only differ, at most, in the above finite union of rays, we may conclude that
![]() $\operatorname {\mathrm {int}}{\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)=\operatorname {\mathrm {int}}{\mathcal {R}}_{{\mathcal {L}}}(n)$
and
$\operatorname {\mathrm {int}}{\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)=\operatorname {\mathrm {int}}{\mathcal {R}}_{{\mathcal {L}}}(n)$
and
![]() $\mathop{\mathrm {cl}}\nolimits {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)=\mathop{\mathrm {cl}}\nolimits {\mathcal {R}}_{{\mathcal {L}}}(n)={\mathcal {R}}_{{\mathcal {L}}}(n)$
.▪
$\mathop{\mathrm {cl}}\nolimits {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)=\mathop{\mathrm {cl}}\nolimits {\mathcal {R}}_{{\mathcal {L}}}(n)={\mathcal {R}}_{{\mathcal {L}}}(n)$
.▪
Using the previous results, in the next subsection, we will determine
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
and
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
and
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
.
${\mathcal {R}}_{{\mathcal {L}}}(n)$
.
4.1 Describing the cones
 ${\mathcal {R}}_{{\mathcal {L}}}(n)$
and
${\mathcal {R}}_{{\mathcal {L}}}(n)$
and
 ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
Before the proof of Theorem 1.3, we need several lemmas and additional notation.
First, we introduce and study particular polynomials that will be crucial in the proof of our main result. The classical De Moivre formula states that, for any
![]() $x\in \mathbb {R}$
and
$x\in \mathbb {R}$
and
![]() $m\in \mathbb {Z}$
,
$m\in \mathbb {Z}$
,
 $\bigl (\cos (x)+\sin (x)\,\mathrm {i}\bigr )^m=\cos (mx)+\sin (mx)\,\mathrm {i}$
(see, e.g., [Reference Conway2, p. 5]). Then, for any
$\bigl (\cos (x)+\sin (x)\,\mathrm {i}\bigr )^m=\cos (mx)+\sin (mx)\,\mathrm {i}$
(see, e.g., [Reference Conway2, p. 5]). Then, for any
![]() $x\in (0,\pi /m]$
,
$x\in (0,\pi /m]$
,
![]() $m\in \mathbb {N}$
with
$m\in \mathbb {N}$
with
![]() $m\geq 3$
, we have
$m\geq 3$
, we have
 $$ \begin{align*} 0 & \leq\mathop{\mathrm{Im}}\nolimits\bigl(\cos(mx)+\sin(mx)\,\mathrm{i}\bigr) =\mathop{\mathrm{Im}}\nolimits\Bigl(\bigl(\cos(x)+\sin(x)\,\mathrm{i}\bigr)^m\Bigr)\\ & =\sum_{\substack{i=1\\i\text{ odd}}}^m\binom{m}{i}(-1)^{(i-1)/2} \cos^{m-i}(x)\sin^i(x), \end{align*} $$
$$ \begin{align*} 0 & \leq\mathop{\mathrm{Im}}\nolimits\bigl(\cos(mx)+\sin(mx)\,\mathrm{i}\bigr) =\mathop{\mathrm{Im}}\nolimits\Bigl(\bigl(\cos(x)+\sin(x)\,\mathrm{i}\bigr)^m\Bigr)\\ & =\sum_{\substack{i=1\\i\text{ odd}}}^m\binom{m}{i}(-1)^{(i-1)/2} \cos^{m-i}(x)\sin^i(x), \end{align*} $$
and dividing by
![]() $\cos ^m(x)>0$
we get
$\cos ^m(x)>0$
we get
 $$ \begin{align*} \sum_{\substack{i=1\\i\text{ odd}}}^m\binom{m}{i}(-1)^{(i-1)/2}\tan^i(x)\geq 0. \end{align*} $$
$$ \begin{align*} \sum_{\substack{i=1\\i\text{ odd}}}^m\binom{m}{i}(-1)^{(i-1)/2}\tan^i(x)\geq 0. \end{align*} $$
Thus, for any
![]() $m\in \mathbb {N}$
, the formal polynomial in a real variable
$m\in \mathbb {N}$
, the formal polynomial in a real variable
![]() $t\in \mathbb {R}$
$t\in \mathbb {R}$
 $$ \begin{align*} p_m(t):=\sum_{\substack{i=0\\i\text{ even}}}^{m-1}\binom{m}{i+1}(-1)^{i/2}t^i \end{align*} $$
$$ \begin{align*} p_m(t):=\sum_{\substack{i=0\\i\text{ even}}}^{m-1}\binom{m}{i+1}(-1)^{i/2}t^i \end{align*} $$
satisfies the following basic but crucial property:
Lemma 4.2 Let
![]() $m\in \mathbb {N}$
. Then
$m\in \mathbb {N}$
. Then
![]() $p_m(t)\geq 0$
for all
$p_m(t)\geq 0$
for all
 $t\in \bigl [0,\tan (\pi /m)\bigr ]$
, and equality holds, for
$t\in \bigl [0,\tan (\pi /m)\bigr ]$
, and equality holds, for
![]() $m\geq 3$
, if and only if
$m\geq 3$
, if and only if
![]() $t=\tan (\pi /m)$
.
$t=\tan (\pi /m)$
.
Next lemma establishes an important relation for these polynomials that will be needed later.
Lemma 4.3 Let
![]() $m\in \mathbb {N}$
. For all
$m\in \mathbb {N}$
. For all
![]() $t\in \mathbb {R}$
,
$t\in \mathbb {R}$
,
Proof From the definition of
![]() $p_k(t)$
, for
$p_k(t)$
, for
![]() $k=m,m+1,m+2$
, we have
$k=m,m+1,m+2$
, we have
 $$ \begin{align*} (1+t^2)p_m(t) & =\sum_{\substack{i=0\\i\text{ even}}}^{m-1} \binom{m}{i+1}(-1)^{i/2}t^i +\sum_{\substack{i=0\\i\text{ even}}}^{m-1}\binom{m}{i+1}(-1)^{i/2}t^{i+2}\\ & =\sum_{\substack{i=0\\i\text{ even}}}^{m-1}\binom{m}{i+1}(-1)^{i/2}t^i +\sum_{\substack{i=2\\i\text{ even}}}^{m+1}\binom{m}{i-1}(-1)^{i/2-1}t^i\\ & =m+\sum_{\substack{i=2\\i\text{ even}}}^{m-1}\left[\binom{m}{i+1}-\binom{m}{i-1}\right](-1)^{i/2}t^i+S_1, \end{align*} $$
$$ \begin{align*} (1+t^2)p_m(t) & =\sum_{\substack{i=0\\i\text{ even}}}^{m-1} \binom{m}{i+1}(-1)^{i/2}t^i +\sum_{\substack{i=0\\i\text{ even}}}^{m-1}\binom{m}{i+1}(-1)^{i/2}t^{i+2}\\ & =\sum_{\substack{i=0\\i\text{ even}}}^{m-1}\binom{m}{i+1}(-1)^{i/2}t^i +\sum_{\substack{i=2\\i\text{ even}}}^{m+1}\binom{m}{i-1}(-1)^{i/2-1}t^i\\ & =m+\sum_{\substack{i=2\\i\text{ even}}}^{m-1}\left[\binom{m}{i+1}-\binom{m}{i-1}\right](-1)^{i/2}t^i+S_1, \end{align*} $$
where
 $$ \begin{align*} S_1=\left\{\begin{array}{ll} m(-1)^{m/2-1}t^m & \text{if}\ m\ \text{is even,}\\ (-1)^{(m-1)/2}t^{m+1} & \text{if}\ m\ \text{is odd,} \end{array}\right. \end{align*} $$
$$ \begin{align*} S_1=\left\{\begin{array}{ll} m(-1)^{m/2-1}t^m & \text{if}\ m\ \text{is even,}\\ (-1)^{(m-1)/2}t^{m+1} & \text{if}\ m\ \text{is odd,} \end{array}\right. \end{align*} $$
and
 $$ \begin{align*} 2p_{m+1}(t)-p_{m+2}(t) & =2\sum_{\substack{i=0\\i\text{ even}}}^m \binom{m+1}{i+1}(-1)^{i/2}t^i -\sum_{\substack{i=0\\i\text{ even}}}^{m+1}\binom{m+2}{i+1}(-1)^{i/2}t^i\\ & =\sum_{\substack{i=0\\i\text{ even}}}^m\left[2\binom{m+1}{i+1}-\binom{m+2}{i+1}\right](-1)^{i/2}t^i+S_2, \end{align*} $$
$$ \begin{align*} 2p_{m+1}(t)-p_{m+2}(t) & =2\sum_{\substack{i=0\\i\text{ even}}}^m \binom{m+1}{i+1}(-1)^{i/2}t^i -\sum_{\substack{i=0\\i\text{ even}}}^{m+1}\binom{m+2}{i+1}(-1)^{i/2}t^i\\ & =\sum_{\substack{i=0\\i\text{ even}}}^m\left[2\binom{m+1}{i+1}-\binom{m+2}{i+1}\right](-1)^{i/2}t^i+S_2, \end{align*} $$
where
 $$ \begin{align*} S_2=\left\{\begin{array}{ll} 0 & \text{if}\ m\ \text{is even,}\\ (-1)^{(m-1)/2}t^{m+1} & \text{if}\ m\ \text{is odd.} \end{array}\right. \end{align*} $$
$$ \begin{align*} S_2=\left\{\begin{array}{ll} 0 & \text{if}\ m\ \text{is even,}\\ (-1)^{(m-1)/2}t^{m+1} & \text{if}\ m\ \text{is odd.} \end{array}\right. \end{align*} $$
Clearly, the constant terms m and
 $2\binom {m+1}{1}-\binom {m+2}{1}$
coincide in the two above polynomials. Also the leading coefficients in both cases, m even and odd, are equal:
$2\binom {m+1}{1}-\binom {m+2}{1}$
coincide in the two above polynomials. Also the leading coefficients in both cases, m even and odd, are equal:
![]() $m(-1)^{m/2-1}$
and
$m(-1)^{m/2-1}$
and
![]() $(-1)^{(m-1)/2}$
, respectively. Furthermore, since
$(-1)^{(m-1)/2}$
, respectively. Furthermore, since
 $$ \begin{align*} \binom{m}{i+1}-\binom{m}{i-1}=\frac{(m+1)!\,(m-2i)}{(m-i+1)!(i+1)!}=2\binom{m+1}{i+1}-\binom{m+2}{i+1}, \end{align*} $$
$$ \begin{align*} \binom{m}{i+1}-\binom{m}{i-1}=\frac{(m+1)!\,(m-2i)}{(m-i+1)!(i+1)!}=2\binom{m+1}{i+1}-\binom{m+2}{i+1}, \end{align*} $$
for all
![]() $i=2,\dots ,m-1$
, we get the required identity.▪
$i=2,\dots ,m-1$
, we get the required identity.▪
Next, we define a (finite) sequence of functions
 $c_k\!:\!\left [0,\tan \bigl (\frac {\pi }{k+3}\bigr )\right ]\!\longrightarrow \mathbb {R}_{>0}$
,
$c_k\!:\!\left [0,\tan \bigl (\frac {\pi }{k+3}\bigr )\right ]\!\longrightarrow \mathbb {R}_{>0}$
,
![]() $k=1,\dots ,n-3$
, recursively by
$k=1,\dots ,n-3$
, recursively by
 $$ \begin{align*} c_k(t)=\left\{ \begin{array}{ll} \frac{1+t^2}{2} & \;\text{if }k=1,\\ \frac{2c_1(t)}{2-c_{k-1}(t)} & \;\text{if }k=2,\dots,n-3. \end{array} \right. \end{align*} $$
$$ \begin{align*} c_k(t)=\left\{ \begin{array}{ll} \frac{1+t^2}{2} & \;\text{if }k=1,\\ \frac{2c_1(t)}{2-c_{k-1}(t)} & \;\text{if }k=2,\dots,n-3. \end{array} \right. \end{align*} $$
In order to assure that they are well-defined, we have to see that
![]() $c_k(t)\neq 2$
for all
$c_k(t)\neq 2$
for all
![]() $k=1,\dots ,n-3$
in its domain. This will be a direct consequence of the following lemma, which will be needed in the proof of Theorem 1.3.
$k=1,\dots ,n-3$
in its domain. This will be a direct consequence of the following lemma, which will be needed in the proof of Theorem 1.3.
Lemma 4.4 For every
![]() $k=1,\dots ,n-3$
we have
$k=1,\dots ,n-3$
we have
 $$ \begin{align*} c_k(t)\leq\frac{3-t^2}{2}\quad\text{ for all }\;\; 0\leq t\leq\tan\left(\frac{\pi}{k+3}\right). \end{align*} $$
$$ \begin{align*} c_k(t)\leq\frac{3-t^2}{2}\quad\text{ for all }\;\; 0\leq t\leq\tan\left(\frac{\pi}{k+3}\right). \end{align*} $$
Equality holds if and only if
 $t=\tan \big (\pi /(k+3)\big )$
. In particular,
$t=\tan \big (\pi /(k+3)\big )$
. In particular,
![]() $c_k(t)<2$
for
$c_k(t)<2$
for
 $0\leq t\leq \tan \big (\pi /(k+3)\big )$
and all
$0\leq t\leq \tan \big (\pi /(k+3)\big )$
and all
![]() $k=1,\dots ,n-3$
.
$k=1,\dots ,n-3$
.
Proof We notice that it is enough to show that the functions
![]() $c_k(t)$
can be expressed as
$c_k(t)$
can be expressed as
 $$ \begin{align} c_k(t)=\frac{3-t^2}{2}-\frac{p_{k+3}(t)}{2p_{k+1}(t)},\quad k=1,\dots,n-3, \end{align} $$
$$ \begin{align} c_k(t)=\frac{3-t^2}{2}-\frac{p_{k+3}(t)}{2p_{k+1}(t)},\quad k=1,\dots,n-3, \end{align} $$
for all
 $0\leq t\leq \tan \bigl (\pi /(k+3)\bigr )$
. Indeed, since
$0\leq t\leq \tan \bigl (\pi /(k+3)\bigr )$
. Indeed, since
![]() $p_{k+3}(t)\geq 0$
for all
$p_{k+3}(t)\geq 0$
for all
 $0\leq t\leq \tan \bigl (\pi /(k+3)\bigr )$
with equality if and only if
$0\leq t\leq \tan \bigl (\pi /(k+3)\bigr )$
with equality if and only if
 $t=\tan \bigl (\pi /(k+3)\bigr )$
(see Lemma 4.2) and, in this range,
$t=\tan \bigl (\pi /(k+3)\bigr )$
(see Lemma 4.2) and, in this range,
![]() $p_{k+1}(t)>0$
because
$p_{k+1}(t)>0$
because
 $\bigl (\tan (\pi /m)\bigr )_{m=2}^{\infty }$
is a decreasing sequence and so
$\bigl (\tan (\pi /m)\bigr )_{m=2}^{\infty }$
is a decreasing sequence and so
 $\tan \bigl (\pi /(k+3)\bigr )<\tan \bigl (\pi /(k+1)\bigr )$
, then the result follows. As usual, we are using the convention
$\tan \bigl (\pi /(k+3)\bigr )<\tan \bigl (\pi /(k+1)\bigr )$
, then the result follows. As usual, we are using the convention
![]() $\tan (\pi /2)=\infty $
.
$\tan (\pi /2)=\infty $
.
We prove (4.1) by induction on k. Clearly,
 $$ \begin{align*} \frac{3-t^2}{2}-\frac{p_4(t)}{2p_2(t)}=\frac{3-t^2}{2}-\frac{4-4t^2}{4}=\frac{1+t^2}{2}=c_1(t). \end{align*} $$
$$ \begin{align*} \frac{3-t^2}{2}-\frac{p_4(t)}{2p_2(t)}=\frac{3-t^2}{2}-\frac{4-4t^2}{4}=\frac{1+t^2}{2}=c_1(t). \end{align*} $$
So, let
![]() $k>1$
and we assume that (4.1) holds for
$k>1$
and we assume that (4.1) holds for
![]() $k-1$
. Then,
$k-1$
. Then,
 $$ \begin{align*} 2-c_{k-1}(t)=2-\frac{3-t^2}{2}+\frac{p_{k+2}(t)}{2p_k(t)}=\frac{1+t^2}{2}+\frac{p_{k+2}(t)}{2p_k(t)}, \end{align*} $$
$$ \begin{align*} 2-c_{k-1}(t)=2-\frac{3-t^2}{2}+\frac{p_{k+2}(t)}{2p_k(t)}=\frac{1+t^2}{2}+\frac{p_{k+2}(t)}{2p_k(t)}, \end{align*} $$
and using Lemma 4.3, we get
 $$ \begin{align*} c_k(t) & =\frac{2c_1(t)}{2-c_{k-1}(t)} =\frac{2(1+t^2)p_k(t)}{(1+t^2)p_k(t)+p_{k+2}(t)} =(1+t^2)\frac{p_k(t)}{p_{k+1}(t)}. \end{align*} $$
$$ \begin{align*} c_k(t) & =\frac{2c_1(t)}{2-c_{k-1}(t)} =\frac{2(1+t^2)p_k(t)}{(1+t^2)p_k(t)+p_{k+2}(t)} =(1+t^2)\frac{p_k(t)}{p_{k+1}(t)}. \end{align*} $$
The identity (4.1) is now obtained using again Lemma 4.3 twice:
 $$ \begin{align*} c_k(t) & =(1+t^2)\frac{p_k(t)}{p_{k+1}(t)}=\frac{4p_{k+1}(t)-2p_{k+2}(t)}{2p_{k+1}(t)}\\ & =\frac{4p_{k+1}(t)-p_{k+3}(t)-(1+t^2)p_{k+1}(t)}{2p_{k+1}(t)}=\frac{(3-t^2)p_{k+1}(t)-p_{k+3}(t)}{2p_{k+1}(t)}. \end{align*} $$
$$ \begin{align*} c_k(t) & =(1+t^2)\frac{p_k(t)}{p_{k+1}(t)}=\frac{4p_{k+1}(t)-2p_{k+2}(t)}{2p_{k+1}(t)}\\ & =\frac{4p_{k+1}(t)-p_{k+3}(t)-(1+t^2)p_{k+1}(t)}{2p_{k+1}(t)}=\frac{(3-t^2)p_{k+1}(t)-p_{k+3}(t)}{2p_{k+1}(t)}. \end{align*} $$
This concludes the proof.▪
Now, we are in a position to prove our main result.
Proof of Theorem 1.3
We state the result for
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
; the description of the cones
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
; the description of the cones
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
is achieved just noticing that
${\mathcal {R}}_{{\mathcal {L}}}(n)$
is achieved just noticing that
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)=\mathop{\mathrm {cl}}\nolimits {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
(see Theorem 1.2).
${\mathcal {R}}_{{\mathcal {L}}}(n)=\mathop{\mathrm {cl}}\nolimits {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
(see Theorem 1.2).
First, we observe that since
 $$ \begin{align*} 1+\tan\left(\frac{\pi}{n}\right)\mathrm{i}\in\bigl\{z\in\mathbb{C}^+:z^n+a=0\bigr\}\subset{\mathcal{R}}_{{\mathcal{L}}}(n), \end{align*} $$
$$ \begin{align*} 1+\tan\left(\frac{\pi}{n}\right)\mathrm{i}\in\bigl\{z\in\mathbb{C}^+:z^n+a=0\bigr\}\subset{\mathcal{R}}_{{\mathcal{L}}}(n), \end{align*} $$
where
![]() $a=\cos ^{-n}(\pi /n)$
, and
$a=\cos ^{-n}(\pi /n)$
, and
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
is a convex cone containing
${\mathcal {R}}_{{\mathcal {L}}}(n)$
is a convex cone containing
![]() $\mathbb {R}_{\leq 0}$
(Theorem 1.1), we have from Theorem 1.2 that
$\mathbb {R}_{\leq 0}$
(Theorem 1.1), we have from Theorem 1.2 that
 $$ \begin{align*} \left\{a+b\,\mathrm{i}\in\mathbb{C}^+:b>\tan\left(\frac{\pi}{n}\right)a\right\} & \subset\operatorname{\mathrm{int}}{\mathcal{R}}_{{\mathcal{L}}}(n)\cup\mathbb{R}_{<0}\\ & =\operatorname{\mathrm{int}}{\mathcal{R}}_{{\mathcal{L}}_{>0}}(n)\cup\mathbb{R}_{<0}\subset{\mathcal{R}}_{{\mathcal{L}}_{>0}}(n). \end{align*} $$
$$ \begin{align*} \left\{a+b\,\mathrm{i}\in\mathbb{C}^+:b>\tan\left(\frac{\pi}{n}\right)a\right\} & \subset\operatorname{\mathrm{int}}{\mathcal{R}}_{{\mathcal{L}}}(n)\cup\mathbb{R}_{<0}\\ & =\operatorname{\mathrm{int}}{\mathcal{R}}_{{\mathcal{L}}_{>0}}(n)\cup\mathbb{R}_{<0}\subset{\mathcal{R}}_{{\mathcal{L}}_{>0}}(n). \end{align*} $$
So, we have to prove the reverse inclusion. Let
![]() $n\in \mathbb {N}$
with
$n\in \mathbb {N}$
with
![]() $n\geq 3$
, and we take
$n\geq 3$
, and we take
![]() $a+b\,\mathrm {i}\in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
. We may suppose that
$a+b\,\mathrm {i}\in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
. We may suppose that
![]() $a>0$
, otherwise the inequality is satisfied, and hence, using Lemma 4.1 (i), we assume without loss of generality that
$a>0$
, otherwise the inequality is satisfied, and hence, using Lemma 4.1 (i), we assume without loss of generality that
![]() $a=1$
. Let
$a=1$
. Let
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
be such that
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
be such that
![]() $f_{\boldsymbol {\omega }}(1+b\,\mathrm {i})=0$
.
$f_{\boldsymbol {\omega }}(1+b\,\mathrm {i})=0$
.
From
 $f_{\boldsymbol {\omega }}(z)=\omega _n(z^2-2z+1+b^2)\left (z^{n-2}+\sum _{i=0}^{n-3}a_iz^i\right )$
we obtain the following identities: if
$f_{\boldsymbol {\omega }}(z)=\omega _n(z^2-2z+1+b^2)\left (z^{n-2}+\sum _{i=0}^{n-3}a_iz^i\right )$
we obtain the following identities: if
![]() $n=3$
, we get
$n=3$
, we get
 $$ \begin{align*} \frac{\omega_0}{\omega_3}=a_0(1+b^2),\qquad 3\,\frac{\omega_1}{\omega_3}=1+b^2-2a_0,\qquad 3\,\frac{\omega_2}{\omega_3}=a_0-2, \end{align*} $$
$$ \begin{align*} \frac{\omega_0}{\omega_3}=a_0(1+b^2),\qquad 3\,\frac{\omega_1}{\omega_3}=1+b^2-2a_0,\qquad 3\,\frac{\omega_2}{\omega_3}=a_0-2, \end{align*} $$
whereas for
![]() $n\geq 4$
, we have
$n\geq 4$
, we have
 $$ \begin{align*} \frac{\omega_0}{\omega_n} & =a_0(1+b^2), \qquad n\,\frac{\omega_1}{\omega_n}=a_1(1+b^2)-2a_0,\\ \binom{n}{i}\,\frac{\omega_i}{\omega_n} & =a_i(1+b^2)-2a_{i-1}+a_{i-2} \quad\text{ for }\; i=2,\dots,n-3,\\ \binom{n}{2}\,\frac{\omega_{n-2}}{\omega_n} & =1+b^2-2a_{n-3}+a_{n-4}\quad\text{ and }\quad n\,\frac{\omega_{n-1}}{\omega_n}=a_{n-3}-2. \end{align*} $$
$$ \begin{align*} \frac{\omega_0}{\omega_n} & =a_0(1+b^2), \qquad n\,\frac{\omega_1}{\omega_n}=a_1(1+b^2)-2a_0,\\ \binom{n}{i}\,\frac{\omega_i}{\omega_n} & =a_i(1+b^2)-2a_{i-1}+a_{i-2} \quad\text{ for }\; i=2,\dots,n-3,\\ \binom{n}{2}\,\frac{\omega_{n-2}}{\omega_n} & =1+b^2-2a_{n-3}+a_{n-4}\quad\text{ and }\quad n\,\frac{\omega_{n-1}}{\omega_n}=a_{n-3}-2. \end{align*} $$
Since
![]() $\omega _i>0$
for all
$\omega _i>0$
for all
![]() $i=0,\dots ,n$
, we have
$i=0,\dots ,n$
, we have
![]() $a_0>0$
and
$a_0>0$
and
 $$ \begin{align} \begin{array}{ll} \text{(i)}\; a_1(1+b^2)>2a_0, & \text{(ii)}\; 1+b^2+a_{n-4}>2a_{n-3},\\ \text{(iii)}\; a_i(1+b^2)+a_{i-2}>2a_{i-1} & \text{ for }\; i=2,\dots,n-3,\\ \text{(iv)}\; a_{n-3}>2. & \end{array} \end{align} $$
$$ \begin{align} \begin{array}{ll} \text{(i)}\; a_1(1+b^2)>2a_0, & \text{(ii)}\; 1+b^2+a_{n-4}>2a_{n-3},\\ \text{(iii)}\; a_i(1+b^2)+a_{i-2}>2a_{i-1} & \text{ for }\; i=2,\dots,n-3,\\ \text{(iv)}\; a_{n-3}>2. & \end{array} \end{align} $$
Note that for
![]() $n=3$
, there are no inequalities (ii) and (iii), being
$n=3$
, there are no inequalities (ii) and (iii), being
![]() $a_1=1$
; for
$a_1=1$
; for
![]() $n=4$
, there is no inequality (iii). In both cases, the conclusion in this argument will be obtained directly.
$n=4$
, there is no inequality (iii). In both cases, the conclusion in this argument will be obtained directly.
We assume now that
![]() $b=\tan (\pi /n)$
, and we will get a contradiction. From (ii) and (iv) in (4.2) we immediately get that
$b=\tan (\pi /n)$
, and we will get a contradiction. From (ii) and (iv) in (4.2) we immediately get that
 $$ \begin{align} 3-\tan^2\left(\frac{\pi}{n}\right)<a_{n-4}. \end{align} $$
$$ \begin{align} 3-\tan^2\left(\frac{\pi}{n}\right)<a_{n-4}. \end{align} $$
Next, we consider the recursive (finite) sequence of numbers
 $$ \begin{align*} c_k=\left\{ \begin{array}{ll} \frac{1+\tan^2\left(\frac{\pi}{n}\right)}{2} & \;\text{for }k=1,\\ \frac{2c_1}{2-c_{k-1}} & \;\text{for }k=2,\dots,n-2, \end{array} \right. \end{align*} $$
$$ \begin{align*} c_k=\left\{ \begin{array}{ll} \frac{1+\tan^2\left(\frac{\pi}{n}\right)}{2} & \;\text{for }k=1,\\ \frac{2c_1}{2-c_{k-1}} & \;\text{for }k=2,\dots,n-2, \end{array} \right. \end{align*} $$
which is well-defined by Lemma 4.4: note, on the one hand, that
 $\tan (\pi /n)\leq \tan \bigl (\pi /(k+3)\bigr )$
for all
$\tan (\pi /n)\leq \tan \bigl (\pi /(k+3)\bigr )$
for all
![]() $k=1,\dots ,n-3$
and, on the other hand, that
$k=1,\dots ,n-3$
and, on the other hand, that
![]() $c_{n-3}<2$
, which ensures that
$c_{n-3}<2$
, which ensures that
![]() $c_{n-2}$
can be defined. Using (4.2)(i), we get
$c_{n-2}$
can be defined. Using (4.2)(i), we get
![]() $a_0<c_1a_1$
, and together with (iii) for
$a_0<c_1a_1$
, and together with (iii) for
![]() $i=2$
, we obtain
$i=2$
, we obtain
 $$ \begin{align*} a_1<c_1a_2+\frac{a_0}{2}<c_1a_2+\frac{c_1a_1}{2},\quad\text{ i.e., }\quad a_1<\frac{2c_1}{2-c_1}a_2=c_2a_2 \end{align*} $$
$$ \begin{align*} a_1<c_1a_2+\frac{a_0}{2}<c_1a_2+\frac{c_1a_1}{2},\quad\text{ i.e., }\quad a_1<\frac{2c_1}{2-c_1}a_2=c_2a_2 \end{align*} $$
because
![]() $c_1<2$
(see Lemma 4.4). An inductive procedure yields, using (iii) for any
$c_1<2$
(see Lemma 4.4). An inductive procedure yields, using (iii) for any
![]() $2\leq i\leq n-3$
,
$2\leq i\leq n-3$
,
 $$ \begin{align*} a_{i-1}<c_1a_i+\frac{a_{i-2}}{2}<c_1a_i+\frac{c_{i-1}a_{i-1}}{2}, \end{align*} $$
$$ \begin{align*} a_{i-1}<c_1a_i+\frac{a_{i-2}}{2}<c_1a_i+\frac{c_{i-1}a_{i-1}}{2}, \end{align*} $$
and so we get
because
![]() $c_{i-1}<2$
(see Lemma 4.4). Finally, the above relation for
$c_{i-1}<2$
(see Lemma 4.4). Finally, the above relation for
![]() $i=n-3$
together with (4.2)(ii) gives
$i=n-3$
together with (4.2)(ii) gives
 $$ \begin{align*} a_{n-4}<c_{n-3}a_{n-3}<c_{n-3}\left(c_1+\frac{a_{n-4}}{2}\right), \end{align*} $$
$$ \begin{align*} a_{n-4}<c_{n-3}a_{n-3}<c_{n-3}\left(c_1+\frac{a_{n-4}}{2}\right), \end{align*} $$
and hence, since
![]() $c_{n-3}<2$
(see Lemma 4.4), we have
$c_{n-3}<2$
(see Lemma 4.4), we have
 $$ \begin{align*} a_{n-4}<\frac{2c_1}{2-c_{n-3}}c_{n-3}=c_{n-2}c_{n-3}. \end{align*} $$
$$ \begin{align*} a_{n-4}<\frac{2c_1}{2-c_{n-3}}c_{n-3}=c_{n-2}c_{n-3}. \end{align*} $$
From the equality case of Lemma 4.4, we get
 $$ \begin{align*} c_{n-3}=\frac{3-\tan^2\left(\frac{\pi}{n}\right)}{2}\quad\text{ and }\quad c_{n-2} =\frac{2c_1}{2-c_{n-3}} =\frac{1+\tan^2\left(\frac{\pi}{n}\right)}{2-\frac{3-\tan^2\left(\frac{\pi}{n}\right)}{2}}=2, \end{align*} $$
$$ \begin{align*} c_{n-3}=\frac{3-\tan^2\left(\frac{\pi}{n}\right)}{2}\quad\text{ and }\quad c_{n-2} =\frac{2c_1}{2-c_{n-3}} =\frac{1+\tan^2\left(\frac{\pi}{n}\right)}{2-\frac{3-\tan^2\left(\frac{\pi}{n}\right)}{2}}=2, \end{align*} $$
and so
 $$ \begin{align*} a_{n-4}<c_{n-2}c_{n-3}=3-\tan^2\left(\frac{\pi}{n}\right), \end{align*} $$
$$ \begin{align*} a_{n-4}<c_{n-2}c_{n-3}=3-\tan^2\left(\frac{\pi}{n}\right), \end{align*} $$
which contradicts (4.3). Therefore, since
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
is a convex cone containing
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
is a convex cone containing
![]() $\mathbb {R}_{<0}$
, it must be
$\mathbb {R}_{<0}$
, it must be
![]() $b>\tan (\pi /n)$
, which shows the reverse inclusion
$b>\tan (\pi /n)$
, which shows the reverse inclusion
 ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)\subset \bigl \{1+b\,\mathrm {i}\in \mathbb {C}^+:b>\tan (\pi /n)\bigr \}$
. This concludes the proof.▪
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)\subset \bigl \{1+b\,\mathrm {i}\in \mathbb {C}^+:b>\tan (\pi /n)\bigr \}$
. This concludes the proof.▪
Note that, surprisingly, the proof of the inclusion
 ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)\subset \bigl \{a+b\,\mathrm {i}\in \mathbb {C}^+:b>\tan (\pi /n)a\bigr \}$
in Theorem 1.3 does not make use of the log-convexity property of the tuple
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)\subset \bigl \{a+b\,\mathrm {i}\in \mathbb {C}^+:b>\tan (\pi /n)a\bigr \}$
in Theorem 1.3 does not make use of the log-convexity property of the tuple
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)$
: just the positivity
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)$
: just the positivity
![]() $\omega _i>0$
, for all
$\omega _i>0$
, for all
![]() $i=0,\dots ,n$
, is needed. This shows the following property:
$i=0,\dots ,n$
, is needed. This shows the following property:
Corollary 4.1 There exists no nth degree real polynomial
![]() $a_0+a_1z+\dots +a_nz^n$
with
$a_0+a_1z+\dots +a_nz^n$
with
![]() $a_i>0$
for all
$a_i>0$
for all
![]() $i=0,\dots ,n$
, having
$i=0,\dots ,n$
, having
![]() $1+\tan (\pi /n)\,\mathrm {i}$
as a root.
$1+\tan (\pi /n)\,\mathrm {i}$
as a root.
As a direct consequence of Theorem 1.3, we get that the cones
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
and
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
and
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
are strictly monotonic with respect to n, and also that they cover the whole upper half-plane
${\mathcal {R}}_{{\mathcal {L}}}(n)$
are strictly monotonic with respect to n, and also that they cover the whole upper half-plane
![]() $\mathbb {C}^+$
, except
$\mathbb {C}^+$
, except
![]() $\mathbb {R}_{\geq 0}$
, when n tends to infinity. For simplicity, we state the result just for
$\mathbb {R}_{\geq 0}$
, when n tends to infinity. For simplicity, we state the result just for
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
; the case of
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
; the case of
![]() ${\mathcal {R}}_{{\mathcal {L}}}(n)$
is analogous.
${\mathcal {R}}_{{\mathcal {L}}}(n)$
is analogous.
Corollary 4.2
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)\varsubsetneq {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n+1)$
. Moreover, for all
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)\varsubsetneq {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n+1)$
. Moreover, for all
![]() $\gamma \in \mathbb {C}^+\backslash \mathbb {R}_{\geq 0}$
, there exists
$\gamma \in \mathbb {C}^+\backslash \mathbb {R}_{\geq 0}$
, there exists
![]() $n_0\in \mathbb {N}$
such that
$n_0\in \mathbb {N}$
such that
![]() $\gamma \in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
for all
$\gamma \in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
for all
![]() $n\geq n_0$
.
$n\geq n_0$
.
Proof The first assertion is obvious from the description of
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
given in Theorem 1.3. Finally, for a given
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
given in Theorem 1.3. Finally, for a given
![]() $\gamma \in \mathbb {C}^+\backslash \mathbb {R}_{\geq 0}$
, since
$\gamma \in \mathbb {C}^+\backslash \mathbb {R}_{\geq 0}$
, since
![]() $\lim _{n\to \infty }\tan (\pi /n)=0$
then there exists
$\lim _{n\to \infty }\tan (\pi /n)=0$
then there exists
![]() $n_0\in \mathbb {N}$
such that
$n_0\in \mathbb {N}$
such that
 $\gamma \in \bigl \{a+b\,\mathrm {i}\in \mathbb {C}^+:b>\tan (\pi /n)a\bigr \}={\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
for all
$\gamma \in \bigl \{a+b\,\mathrm {i}\in \mathbb {C}^+:b>\tan (\pi /n)a\bigr \}={\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
for all
![]() $n\geq n_0$
. This concludes the proof.▪
$n\geq n_0$
. This concludes the proof.▪
As another consequence of Theorem 1.3, we can prove Corollary 2.1. Indeed, since the dual Aleksandrov–Fenchel inequalities characterize the triples
![]() $(\omega _0,\omega _1,\omega _2)$
of positive numbers that can be the dual quermassintegrals
$(\omega _0,\omega _1,\omega _2)$
of positive numbers that can be the dual quermassintegrals
![]() $\widetilde {\mathrm {W}}_i(K)$
of some star body
$\widetilde {\mathrm {W}}_i(K)$
of some star body
![]() $K\in \mathcal {S}^3_0$
(see Theorem 2.1), with a slight extra effort we easily get that
$K\in \mathcal {S}^3_0$
(see Theorem 2.1), with a slight extra effort we easily get that
 $\widetilde {\mathcal {R}}(3)={\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
:
$\widetilde {\mathcal {R}}(3)={\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
:
Proof of Corollary 2.1
The inclusion
 $\widetilde {\mathcal {R}}(3)\subset {\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
is clear. In order to prove the reverse inclusion, let
$\widetilde {\mathcal {R}}(3)\subset {\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
is clear. In order to prove the reverse inclusion, let
![]() $\gamma \in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
, which we suppose not to be real, otherwise the assertion holds. Then there exists
$\gamma \in {\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
, which we suppose not to be real, otherwise the assertion holds. Then there exists
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^3_{>0}$
such that
${\boldsymbol {\omega }}\in {\mathcal {L}}^3_{>0}$
such that
![]() $f_{\boldsymbol {\omega }}(\gamma )=0$
. Note that we may assume, without loss of generality, that
$f_{\boldsymbol {\omega }}(\gamma )=0$
. Note that we may assume, without loss of generality, that
![]() ${\boldsymbol {\omega }}=(\omega _0,\omega _1,\omega _2,\kappa _3)$
. If
${\boldsymbol {\omega }}=(\omega _0,\omega _1,\omega _2,\kappa _3)$
. If
then Theorem 2.1 ensures that
![]() $f_{\boldsymbol {\omega }}(z)$
is a dual Steiner polynomial of some star body, and therefore
$f_{\boldsymbol {\omega }}(z)$
is a dual Steiner polynomial of some star body, and therefore
 $\gamma \in \widetilde {\mathcal {R}}(3)$
.
$\gamma \in \widetilde {\mathcal {R}}(3)$
.
If, on the contrary, some of the above inequalities is an equality, we consider the
![]() $4$
-tuple
$4$
-tuple
 $$ \begin{align*} {\boldsymbol{\omega}}'=(\omega_0',\omega_1',\omega_2',\kappa_3):= \frac{\kappa_3}{\kappa_3+\varepsilon}\bigl(\omega_0+\varepsilon,\omega_1,\omega_2,\kappa_3+\varepsilon\bigr) \end{align*} $$
$$ \begin{align*} {\boldsymbol{\omega}}'=(\omega_0',\omega_1',\omega_2',\kappa_3):= \frac{\kappa_3}{\kappa_3+\varepsilon}\bigl(\omega_0+\varepsilon,\omega_1,\omega_2,\kappa_3+\varepsilon\bigr) \end{align*} $$
for fixed
![]() $\varepsilon>0$
. Clearly
$\varepsilon>0$
. Clearly
![]() ${\boldsymbol {\omega }}'\in {\mathcal {L}}^3_{>0}$
, and it is straightforward that the inequalities
${\boldsymbol {\omega }}'\in {\mathcal {L}}^3_{>0}$
, and it is straightforward that the inequalities
![]() $(\omega _1')^2<\omega _0'\omega _2'$
and
$(\omega _1')^2<\omega _0'\omega _2'$
and
![]() $(\omega _2')^2<\omega _1'\kappa _3$
hold. Hence, by Theorem 2.1, there exists a star body
$(\omega _2')^2<\omega _1'\kappa _3$
hold. Hence, by Theorem 2.1, there exists a star body
![]() $K\in \mathcal {S}^3_0$
such that
$K\in \mathcal {S}^3_0$
such that
 $f_{{\boldsymbol {\omega }}'}(z)=\widetilde {f}_{K;B_3}(z)$
is a dual Steiner polynomial.
$f_{{\boldsymbol {\omega }}'}(z)=\widetilde {f}_{K;B_3}(z)$
is a dual Steiner polynomial.
Let
![]() $\delta>0$
be fixed. Since the roots of a polynomial are continuous functions of the coefficients of the polynomial (see Theorem 3.1), if
$\delta>0$
be fixed. Since the roots of a polynomial are continuous functions of the coefficients of the polynomial (see Theorem 3.1), if
![]() $\varepsilon>0$
is small enough, then there exists
$\varepsilon>0$
is small enough, then there exists
![]() $\gamma '\in \mathbb {C}^+$
with
$\gamma '\in \mathbb {C}^+$
with
![]() $f_{{\boldsymbol {\omega }}'}(\gamma ')=0$
, i.e.,
$f_{{\boldsymbol {\omega }}'}(\gamma ')=0$
, i.e.,
 $\gamma '\in \widetilde {\mathcal {R}}(3)$
, such that
$\gamma '\in \widetilde {\mathcal {R}}(3)$
, such that
![]() $|\gamma -\gamma '|<\delta $
. This shows that
$|\gamma -\gamma '|<\delta $
. This shows that
 $\gamma \in \mathop{\mathrm {cl}}\nolimits \widetilde {\mathcal {R}}(3)$
, i.e., that
$\gamma \in \mathop{\mathrm {cl}}\nolimits \widetilde {\mathcal {R}}(3)$
, i.e., that
 ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)\subset \mathop{\mathrm {cl}}\nolimits \widetilde {\mathcal {R}}(3)$
. Since both
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)\subset \mathop{\mathrm {cl}}\nolimits \widetilde {\mathcal {R}}(3)$
. Since both
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
and
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
and
 $\widetilde {\mathcal {R}}(3)$
are convex cones, the proof is then concluded from the inclusion
$\widetilde {\mathcal {R}}(3)$
are convex cones, the proof is then concluded from the inclusion
 $\widetilde {\mathcal {R}}(3)\subset {\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
jointly with the fact that the upper ray of the boundary of
$\widetilde {\mathcal {R}}(3)\subset {\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
jointly with the fact that the upper ray of the boundary of
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
, i.e.,
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)$
, i.e.,
![]() $\mathop{\mathrm {bd}}\nolimits {\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)\backslash \mathbb {R}_{<0}$
, is not included therein.▪
$\mathop{\mathrm {bd}}\nolimits {\mathcal {R}}_{{\mathcal {L}}_{>0}}(3)\backslash \mathbb {R}_{<0}$
, is not included therein.▪
Unfortunately, since the dual Aleksandrov–Fenchel inequalities do not characterize dual quermassintegrals (cf. Remark 2.1), Theorem 1.3 does not provide us with a description for
 $\widetilde {\mathcal {R}}(4)$
.
$\widetilde {\mathcal {R}}(4)$
.
4.2 Further properties of the cones of roots
An immediate outcome of Theorem 1.3 is the fact that, when
![]() $n\geq 3$
, a pure imaginary complex root always exists in
$n\geq 3$
, a pure imaginary complex root always exists in
![]() ${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
, since it is a convex cone. Indeed, for
${\mathcal {R}}_{{\mathcal {L}}_{>0}}(n)$
, since it is a convex cone. Indeed, for
![]() $n=3$
, there are log-convex coefficients polynomials all whose complex roots are pure imaginary. However, when
$n=3$
, there are log-convex coefficients polynomials all whose complex roots are pure imaginary. However, when
![]() $n\geq 4$
, not all the roots can be of that type. More precisely, we have:
$n\geq 4$
, not all the roots can be of that type. More precisely, we have:
Theorem 4.1 For
![]() $n\geq 4$
,
$n\geq 4$
,
![]() $n\neq 5$
, there does not exist
$n\neq 5$
, there does not exist
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^n$
such that all the roots of
${\boldsymbol {\omega }}\in {\mathcal {L}}^n$
such that all the roots of
![]() $f_{\boldsymbol {\omega }}(z)$
are imaginary pure complex numbers
$f_{\boldsymbol {\omega }}(z)$
are imaginary pure complex numbers
![]() $($
excluding the real root always existing for odd degree
$($
excluding the real root always existing for odd degree
![]() $)$
. When
$)$
. When
![]() $n=5$
, the only
$n=5$
, the only
![]() $6$
-tuples satisfying the above condition are
$6$
-tuples satisfying the above condition are
 ${\boldsymbol {\omega }}=\lambda \bigl (c^5,c^4/5,c^3/5,c^2/5,c/5,1\bigr )\in {\mathcal {L}}^5$
for
${\boldsymbol {\omega }}=\lambda \bigl (c^5,c^4/5,c^3/5,c^2/5,c/5,1\bigr )\in {\mathcal {L}}^5$
for
![]() $\lambda>0$
and
$\lambda>0$
and
![]() $c>0$
, and in this case
$c>0$
, and in this case
 $f_{\boldsymbol {\omega }}(z)=\lambda (z+c)\left (z^2+c^2\right )^2$
.
$f_{\boldsymbol {\omega }}(z)=\lambda (z+c)\left (z^2+c^2\right )^2$
.
In order to prove the theorem, we need the following auxiliary result:
Lemma 4.5 For any
![]() $n\geq 5$
, let
$n\geq 5$
, let
![]() $x_1,\dots ,x_r>0$
,
$x_1,\dots ,x_r>0$
,
![]() $r\geq 2$
, be positive real numbers, such that
$r\geq 2$
, be positive real numbers, such that
 $$ \begin{align*} x_1+\dots+x_r\leq\frac{3}{2}\,\frac{n-1}{n-2}. \end{align*} $$
$$ \begin{align*} x_1+\dots+x_r\leq\frac{3}{2}\,\frac{n-1}{n-2}. \end{align*} $$
Then
 $$ \begin{align} \frac{1}{x_1}+\dots+\frac{1}{x_r}\geq\frac{3}{2}\,\frac{n-1}{n-2}, \end{align} $$
$$ \begin{align} \frac{1}{x_1}+\dots+\frac{1}{x_r}\geq\frac{3}{2}\,\frac{n-1}{n-2}, \end{align} $$
and equality holds if and only if
![]() $n=5$
,
$n=5$
,
![]() $r=2$
and
$r=2$
and
![]() $x_1=x_2=1$
.
$x_1=x_2=1$
.
Proof For the sake of simplicity, we write
 $a_n:=3(n-1)/\bigl (2(n-2)\bigr )$
. Clearly
$a_n:=3(n-1)/\bigl (2(n-2)\bigr )$
. Clearly
![]() $a_5=2$
, and since
$a_5=2$
, and since
![]() $a_n$
is a decreasing sequence in n, it can be easily seen that
$a_n$
is a decreasing sequence in n, it can be easily seen that
![]() $a_n<2$
for all
$a_n<2$
for all
![]() $n>5$
, and so
$n>5$
, and so
![]() $a_n\leq 4/a_n$
with equality if and only if
$a_n\leq 4/a_n$
with equality if and only if
![]() $n=5$
.
$n=5$
.
We assume that
![]() $\sum _{i=1}^rx_i\leq a_n$
, and first we show the result for
$\sum _{i=1}^rx_i\leq a_n$
, and first we show the result for
![]() $r=2$
. Let
$r=2$
. Let
![]() $m:=x_1+x_2\leq a_n$
. Since
$m:=x_1+x_2\leq a_n$
. Since
![]() $m-x_1=x_2>0$
, inequality (4.4) can be expressed as
$m-x_1=x_2>0$
, inequality (4.4) can be expressed as
![]() $m\geq a_nx_1(m-x_1)$
, or equivalently,
$m\geq a_nx_1(m-x_1)$
, or equivalently,
Using that
![]() $a_n\leq 4/a_n$
, the discriminant of the second-degree polynomial (in
$a_n\leq 4/a_n$
, the discriminant of the second-degree polynomial (in
![]() $x_1$
) in the left-hand side satisfies that
$x_1$
) in the left-hand side satisfies that
 $$ \begin{align*} {\textrm{D}}=a_nm(a_nm-4)\leq a_nm\left(\frac{4}{a_n}a_n-4\right)=0. \end{align*} $$
$$ \begin{align*} {\textrm{D}}=a_nm(a_nm-4)\leq a_nm\left(\frac{4}{a_n}a_n-4\right)=0. \end{align*} $$
Therefore,
![]() $a_nx_1^2-a_nmx_1+m\geq 0$
, and thus (4.4) holds. Note, moreover, that if
$a_nx_1^2-a_nmx_1+m\geq 0$
, and thus (4.4) holds. Note, moreover, that if
![]() $n>5$
then
$n>5$
then
![]() ${\textrm {D}}<0$
and (4.4) holds strictly.
${\textrm {D}}<0$
and (4.4) holds strictly.
Now, we assume that
![]() $r>2$
and consider the positive numbers
$r>2$
and consider the positive numbers
 $$ \begin{align*} x:=\sum_{i=1}^{r-1}x_i\quad\text{ and }\quad y=x_r, \end{align*} $$
$$ \begin{align*} x:=\sum_{i=1}^{r-1}x_i\quad\text{ and }\quad y=x_r, \end{align*} $$
which satisfy
![]() $x+y\leq a_n$
. We observe that since
$x+y\leq a_n$
. We observe that since
![]() $r>2$
and
$r>2$
and
![]() $1/x$
is a convex function, then
$1/x$
is a convex function, then
 $$ \begin{align} \frac{1}{\sum_{i=1}^{r-1}x_i}<\frac{1}{\frac{1}{r-1}\sum_{i=1}^{r-1}x_i}\leq\sum_{i=1}^{r-1}\frac{1}{r-1}\frac{1}{x_i} <\sum_{i=1}^{r-1}\frac{1}{x_i}. \end{align} $$
$$ \begin{align} \frac{1}{\sum_{i=1}^{r-1}x_i}<\frac{1}{\frac{1}{r-1}\sum_{i=1}^{r-1}x_i}\leq\sum_{i=1}^{r-1}\frac{1}{r-1}\frac{1}{x_i} <\sum_{i=1}^{r-1}\frac{1}{x_i}. \end{align} $$
If we assume that
![]() $\sum _{i=1}^r1/x_i\leq a_n$
holds, (4.6) would yield
$\sum _{i=1}^r1/x_i\leq a_n$
holds, (4.6) would yield
 $$ \begin{align*} a_n\geq\left(\sum_{i=1}^{r-1}\frac{1}{x_i}\right)+\frac{1}{x_r}>\frac{1}{\sum_{i=1}^{r-1}x_i}+\frac{1}{x_r}=\frac{1}{x}+\frac{1}{y}, \end{align*} $$
$$ \begin{align*} a_n\geq\left(\sum_{i=1}^{r-1}\frac{1}{x_i}\right)+\frac{1}{x_r}>\frac{1}{\sum_{i=1}^{r-1}x_i}+\frac{1}{x_r}=\frac{1}{x}+\frac{1}{y}, \end{align*} $$
in contradiction to the case
![]() $r=2$
previously proved. This shows that for
$r=2$
previously proved. This shows that for
![]() $r>2$
, inequality (4.4) holds strictly.
$r>2$
, inequality (4.4) holds strictly.
For the equality case, we assume that (4.4) holds with equality. We already know that then, necessarily,
![]() $r=2$
, otherwise the inequality (4.4) would be strict. Therefore, the equality in (4.4) is equivalent to the identity
$r=2$
, otherwise the inequality (4.4) would be strict. Therefore, the equality in (4.4) is equivalent to the identity
![]() $a_nx_1^2-a_nmx_1+m=0$
(cf. (4.5)). But in this case, they must be
$a_nx_1^2-a_nmx_1+m=0$
(cf. (4.5)). But in this case, they must be
![]() $n=5$
and
$n=5$
and
![]() ${\textrm {D}}=0$
, otherwise (4.5) would hold strictly. Finally, notice that
${\textrm {D}}=0$
, otherwise (4.5) would hold strictly. Finally, notice that
![]() ${\textrm {D}}=0$
(when
${\textrm {D}}=0$
(when
![]() $n=5$
) occurs if and only if
$n=5$
) occurs if and only if
![]() $m=2$
. Thus,
$m=2$
. Thus,
if and only if
![]() $x_1=1$
and so
$x_1=1$
and so
![]() $x_2=1$
.▪
$x_2=1$
.▪
Now, we are in a position to show Theorem 4.1.
Proof of Theorem 4.1
First, we note that if
![]() ${\boldsymbol {\omega }}=(\omega _0,0,\dots ,0,\omega _n)\in {\mathcal {L}}^n\backslash {\mathcal {L}}^n_{>0}$
then
${\boldsymbol {\omega }}=(\omega _0,0,\dots ,0,\omega _n)\in {\mathcal {L}}^n\backslash {\mathcal {L}}^n_{>0}$
then
![]() $f_{\boldsymbol {\omega }}(z)=\omega _0+\omega _nz^n$
, all whose roots cannot be imaginary pure complex numbers (aside from the real root if n is odd) for any values of
$f_{\boldsymbol {\omega }}(z)=\omega _0+\omega _nz^n$
, all whose roots cannot be imaginary pure complex numbers (aside from the real root if n is odd) for any values of
![]() $\omega _0,\omega _n\in \mathbb {R}_{\geq 0}$
. So, we will consider only tuples in
$\omega _0,\omega _n\in \mathbb {R}_{\geq 0}$
. So, we will consider only tuples in
![]() ${\mathcal {L}}^n_{>0}$
.
${\mathcal {L}}^n_{>0}$
.
Let n be even, and we assume there exists
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
such that all the roots of
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
such that all the roots of
![]() $f_{\boldsymbol {\omega }}(z)$
are
$f_{\boldsymbol {\omega }}(z)$
are
![]() $\{\pm b_j\,\mathrm {i}, j=1,\dots ,n/2\}$
, with
$\{\pm b_j\,\mathrm {i}, j=1,\dots ,n/2\}$
, with
![]() $b_j\in \mathbb {R}_{>0}$
. Then
$b_j\in \mathbb {R}_{>0}$
. Then
 $$ \begin{align*} f_{\boldsymbol{\omega}}(z)=\omega_n\prod_{j=1}^{n/2}(z^2+b_j^2), \end{align*} $$
$$ \begin{align*} f_{\boldsymbol{\omega}}(z)=\omega_n\prod_{j=1}^{n/2}(z^2+b_j^2), \end{align*} $$
which would imply, in particular, that
![]() $\omega _{2i+1}=0$
for all
$\omega _{2i+1}=0$
for all
![]() $i=0,\dots ,(n-2)/2$
. Thus
$i=0,\dots ,(n-2)/2$
. Thus
![]() $\omega _i=0$
for every
$\omega _i=0$
for every
![]() $i=1,\dots ,n-1$
, and so
$i=1,\dots ,n-1$
, and so
![]() $f_{\boldsymbol {\omega }}(z)$
would be of the form
$f_{\boldsymbol {\omega }}(z)$
would be of the form
![]() $f_{\boldsymbol {\omega }}(z)=\omega _n(z^n+a)$
, a contradiction.
$f_{\boldsymbol {\omega }}(z)=\omega _n(z^n+a)$
, a contradiction.
Now let n be odd. Let
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
be such that the roots of
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
be such that the roots of
![]() $f_{\boldsymbol {\omega }}(z)$
are
$f_{\boldsymbol {\omega }}(z)$
are
 $\bigl \{-c,\pm b_j\,\mathrm {i},j=1,\dots ,(n-1)/2\bigr \}$
, with
$\bigl \{-c,\pm b_j\,\mathrm {i},j=1,\dots ,(n-1)/2\bigr \}$
, with
![]() $c,b_j\in \mathbb {R}_{>0}$
. From
$c,b_j\in \mathbb {R}_{>0}$
. From
 $$ \begin{align*} f_{\boldsymbol{\omega}}(z)=\omega_n(z+c)\prod_{j=1}^{(n-1)/2}(z^2+b_j^2), \end{align*} $$
$$ \begin{align*} f_{\boldsymbol{\omega}}(z)=\omega_n(z+c)\prod_{j=1}^{(n-1)/2}(z^2+b_j^2), \end{align*} $$
we get
 $$ \begin{align*} c\sum_{j=1}^{(n-1)/2}b_j^2 & =\binom{n}{3}\frac{\omega_{n-3}}{\omega_n}, \qquad \sum_{j=1}^{(n-1)/2}b_j^2=\binom{n}{2}\frac{\omega_{n-2}}{\omega_n}, \qquad c=n\frac{\omega_{n-1}}{\omega_n},\\ \prod_{j=1}^{(n-1)/2}b_j^2 & =n\frac{\omega_1}{\omega_n}, \quad\; c\sum_{j=1}^{(n-1)/2}\prod_{i\neq j}b_i^2=\binom{n}{2}\frac{\omega_2}{\omega_n}, \quad\;\sum_{j=1}^{(n-1)/2} \prod_{i\neq j}b_i^2 =\binom{n}{3}\frac{\omega_3}{\omega_n}. \end{align*} $$
$$ \begin{align*} c\sum_{j=1}^{(n-1)/2}b_j^2 & =\binom{n}{3}\frac{\omega_{n-3}}{\omega_n}, \qquad \sum_{j=1}^{(n-1)/2}b_j^2=\binom{n}{2}\frac{\omega_{n-2}}{\omega_n}, \qquad c=n\frac{\omega_{n-1}}{\omega_n},\\ \prod_{j=1}^{(n-1)/2}b_j^2 & =n\frac{\omega_1}{\omega_n}, \quad\; c\sum_{j=1}^{(n-1)/2}\prod_{i\neq j}b_i^2=\binom{n}{2}\frac{\omega_2}{\omega_n}, \quad\;\sum_{j=1}^{(n-1)/2} \prod_{i\neq j}b_i^2 =\binom{n}{3}\frac{\omega_3}{\omega_n}. \end{align*} $$
Since
![]() ${\boldsymbol {\omega }}$
is log-convex, it must be, in particular,
${\boldsymbol {\omega }}$
is log-convex, it must be, in particular,
![]() $\omega _{n-2}^2\leq \omega _{n-3}\omega _{n-1}$
and
$\omega _{n-2}^2\leq \omega _{n-3}\omega _{n-1}$
and
![]() $\omega _2^2\leq \omega _1\omega _3$
, which are equivalent to the relations
$\omega _2^2\leq \omega _1\omega _3$
, which are equivalent to the relations
 $$ \begin{align*} \frac{2}{n-1}\,\frac{1}{c^2}\!\sum_{j=1}^{(n-1)/2}b_j^2\leq\frac{3}{n-2} \quad\text{and}\quad \frac{2}{n-1}\,c^2\!\sum_{j=1}^{(n-1)/2}\prod_{i\neq j}b_i^2 \leq\frac{3}{n-2}\prod_{j=1}^{(n-1)/2}b_j^2, \end{align*} $$
$$ \begin{align*} \frac{2}{n-1}\,\frac{1}{c^2}\!\sum_{j=1}^{(n-1)/2}b_j^2\leq\frac{3}{n-2} \quad\text{and}\quad \frac{2}{n-1}\,c^2\!\sum_{j=1}^{(n-1)/2}\prod_{i\neq j}b_i^2 \leq\frac{3}{n-2}\prod_{j=1}^{(n-1)/2}b_j^2, \end{align*} $$
i.e.,
 $$ \begin{align} \sum_{j=1}^{(n-1)/2}\frac{b_j^2}{c^2}\leq\frac{3}{2}\,\frac{n-1}{n-2} \quad\text{ and } \quad\sum_{j=1}^{(n-1)/2}\frac{c^2}{b_j^2}\leq\frac{3}{2}\,\frac{n-1}{n-2}, \end{align} $$
$$ \begin{align} \sum_{j=1}^{(n-1)/2}\frac{b_j^2}{c^2}\leq\frac{3}{2}\,\frac{n-1}{n-2} \quad\text{ and } \quad\sum_{j=1}^{(n-1)/2}\frac{c^2}{b_j^2}\leq\frac{3}{2}\,\frac{n-1}{n-2}, \end{align} $$
respectively. Lemma 4.5 ensures that, when
![]() $n>5$
, the above two inequalities cannot hold simultaneously, which gives the desired contradiction.
$n>5$
, the above two inequalities cannot hold simultaneously, which gives the desired contradiction.
If
![]() $n=5$
, then (4.7) becomes
$n=5$
, then (4.7) becomes
 $$ \begin{align*} \frac{b_1^2}{c^2}+\frac{b_2^2}{c^2}\leq 2\quad\text{ and } \quad \frac{c^2}{b_1^2}+\frac{c^2}{b_2^2}\leq 2, \end{align*} $$
$$ \begin{align*} \frac{b_1^2}{c^2}+\frac{b_2^2}{c^2}\leq 2\quad\text{ and } \quad \frac{c^2}{b_1^2}+\frac{c^2}{b_2^2}\leq 2, \end{align*} $$
and Lemma 4.5 ensures that both inequalities hold simultaneously if and only if
![]() $b_1=b_2=c$
. Therefore,
$b_1=b_2=c$
. Therefore,
 $f_{\boldsymbol {\omega }}(z)=\omega _5(z+c)\left (z^2+c^2\right )^2$
, which concludes the proof.▪
$f_{\boldsymbol {\omega }}(z)=\omega _5(z+c)\left (z^2+c^2\right )^2$
, which concludes the proof.▪
For dual Steiner polynomials, the property provided by Theorem 4.1 is slightly more restrictive.
Corollary 4.3 For
![]() $n\geq 4$
, there does not exist a star body
$n\geq 4$
, there does not exist a star body
![]() $K\in \mathcal {S}^n_0$
such that all the roots of
$K\in \mathcal {S}^n_0$
such that all the roots of
 $\widetilde {f}_{K;B_n}(z)$
are imaginary pure complex numbers
$\widetilde {f}_{K;B_n}(z)$
are imaginary pure complex numbers
![]() $($
excluding the real root always existing in odd dimension
$($
excluding the real root always existing in odd dimension
![]() $)$
.
$)$
.
Proof Since all dual Steiner polynomials are log-convex coefficients polynomials, Theorem 4.1 ensures that when
![]() $n\geq 4$
,
$n\geq 4$
,
![]() $n\neq 5$
, there does not exist
$n\neq 5$
, there does not exist
![]() $K\in \mathcal {S}^n_0$
such that all the roots of
$K\in \mathcal {S}^n_0$
such that all the roots of
 $\widetilde {f}_{K;B_n}(z)$
are imaginary pure complex numbers.
$\widetilde {f}_{K;B_n}(z)$
are imaginary pure complex numbers.
So, we set
![]() $n=5$
. Theorem 4.1 ensures that the only possible log-convex coefficients polynomial, all whose roots are pure complex numbers (excluding the existing real root), is of the form
$n=5$
. Theorem 4.1 ensures that the only possible log-convex coefficients polynomial, all whose roots are pure complex numbers (excluding the existing real root), is of the form
 $\lambda (z+c)\bigl (z^2+c^2\bigr )^2$
, for
$\lambda (z+c)\bigl (z^2+c^2\bigr )^2$
, for
![]() $\lambda ,c>0$
. However, it cannot be a dual Steiner polynomial for any star body
$\lambda ,c>0$
. However, it cannot be a dual Steiner polynomial for any star body
![]() $K\in \mathcal {S}^5_0$
. Indeed, if this was the case, it should be
$K\in \mathcal {S}^5_0$
. Indeed, if this was the case, it should be
 $$ \begin{align*} \widetilde{\mathrm{W}}_0(K)=\lambda c^5,\quad \widetilde{\mathrm{W}}_i(K)=\lambda\dfrac{c^{5-i}}{5} \;\text{ for}\ i=1,\dots,4,\quad \widetilde{\mathrm{W}}_5(K)=\lambda \end{align*} $$
$$ \begin{align*} \widetilde{\mathrm{W}}_0(K)=\lambda c^5,\quad \widetilde{\mathrm{W}}_i(K)=\lambda\dfrac{c^{5-i}}{5} \;\text{ for}\ i=1,\dots,4,\quad \widetilde{\mathrm{W}}_5(K)=\lambda \end{align*} $$
for some
![]() $K\in \mathcal {S}^5_0$
, which would verify the dual Aleksandrov–Fenchel inequalities (2.2). But since, for instance,
$K\in \mathcal {S}^5_0$
, which would verify the dual Aleksandrov–Fenchel inequalities (2.2). But since, for instance,
 $$ \begin{align*} \widetilde{\mathrm{W}}_2(K)^2-\widetilde{\mathrm{W}}_1(K)\widetilde{\mathrm{W}}_3(K)=\dfrac{c^6}{25}\lambda^2 -\dfrac{c^4}{5}\lambda\dfrac{c^2}{5}\lambda=0, \end{align*} $$
$$ \begin{align*} \widetilde{\mathrm{W}}_2(K)^2-\widetilde{\mathrm{W}}_1(K)\widetilde{\mathrm{W}}_3(K)=\dfrac{c^6}{25}\lambda^2 -\dfrac{c^4}{5}\lambda\dfrac{c^2}{5}\lambda=0, \end{align*} $$
the equality case in (2.2) would imply that K is a suitable dilation of
![]() $B_5$
, and so, all dual Aleksandrov–Fenchel inequalities should hold with equality. This is however not the case, because
$B_5$
, and so, all dual Aleksandrov–Fenchel inequalities should hold with equality. This is however not the case, because
![]() $\widetilde {\mathrm {W}}_4(K)^2-\widetilde {\mathrm {W}}_3(K)\widetilde {\mathrm {W}}_5(K)=-4\lambda ^2c^2/25<0$
. It concludes the proof.▪
$\widetilde {\mathrm {W}}_4(K)^2-\widetilde {\mathrm {W}}_3(K)\widetilde {\mathrm {W}}_5(K)=-4\lambda ^2c^2/25<0$
. It concludes the proof.▪
With respect to real roots, a comparable result to Theorem 4.1 can be obtained: not all the roots can be real numbers, unless they coincide. For completeness, we include its proof, following the argument of [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Proposition 4.4], where it was shown that in the case of the more restrictive family of dual Steiner polynomials, not all their roots can be real unless they all are equal. For the proof we need the following notation: for complex numbers
![]() $z_1,\dots ,z_r\in \mathbb {C}$
let
$z_1,\dots ,z_r\in \mathbb {C}$
let
 $$ \begin{align*} {\textrm{s}}_{i}\!\left(z_1,\dots,z_r\right)=\sum_{\substack{J\subset\{1,\dots,r\}\\\#J=i}} \prod_{j\in J}z_j \end{align*} $$
$$ \begin{align*} {\textrm{s}}_{i}\!\left(z_1,\dots,z_r\right)=\sum_{\substack{J\subset\{1,\dots,r\}\\\#J=i}} \prod_{j\in J}z_j \end{align*} $$
denote the ith elementary symmetric function of
![]() $z_1,\dots ,z_r$
,
$z_1,\dots ,z_r$
,
![]() $i=1,\dots ,r$
, with
$i=1,\dots ,r$
, with
![]() ${\textrm {s}}_{0}\!\left (z_1,\dots ,z_r\right )=1$
.
${\textrm {s}}_{0}\!\left (z_1,\dots ,z_r\right )=1$
.
Proposition 4.1 Let
![]() ${\boldsymbol {\omega }}\in {\mathcal {L}}^n$
. If
${\boldsymbol {\omega }}\in {\mathcal {L}}^n$
. If
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
, then all the roots of
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
, then all the roots of
![]() $f_{\boldsymbol {\omega }}(z)$
are real if and only if
$f_{\boldsymbol {\omega }}(z)$
are real if and only if
![]() ${\boldsymbol {\omega }}=\omega _n(a^n,a^{n-1},\dots ,a,1)$
for some
${\boldsymbol {\omega }}=\omega _n(a^n,a^{n-1},\dots ,a,1)$
for some
![]() $a>0$
, i.e., if and only if
$a>0$
, i.e., if and only if
![]() $f_{\boldsymbol {\omega }}(z)=\omega _n(z+a)^n$
; therefore, all the roots are equal.
$f_{\boldsymbol {\omega }}(z)=\omega _n(z+a)^n$
; therefore, all the roots are equal.
If
![]() ${\boldsymbol {\omega }}=(\omega _0,0,\dots ,0,\omega _n)\in {\mathcal {L}}^n$
, then all the roots are real if and only if
${\boldsymbol {\omega }}=(\omega _0,0,\dots ,0,\omega _n)\in {\mathcal {L}}^n$
, then all the roots are real if and only if
![]() $\omega _0=0$
, i.e., if and only if all the roots are equal
$\omega _0=0$
, i.e., if and only if all the roots are equal
![]() $0$
.
$0$
.
Proof If
![]() ${\boldsymbol {\omega }}=(\omega _0,0,\dots ,0,\omega _n)\in {\mathcal {L}}^n$
, then
${\boldsymbol {\omega }}=(\omega _0,0,\dots ,0,\omega _n)\in {\mathcal {L}}^n$
, then
![]() $f_{\boldsymbol {\omega }}(z)=\omega _0+\omega _nz^n$
, and the thesis follows trivially.
$f_{\boldsymbol {\omega }}(z)=\omega _0+\omega _nz^n$
, and the thesis follows trivially.
So we assume that
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
, and let
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
, and let
![]() $\gamma _1,\dots ,\gamma _n\in \mathbb {R}_{<0}$
be the roots of
$\gamma _1,\dots ,\gamma _n\in \mathbb {R}_{<0}$
be the roots of
![]() $f_{\boldsymbol {\omega }}(z)$
. Then, its coefficients can be expressed in terms of the elementary symmetric functions of the roots, namely,
$f_{\boldsymbol {\omega }}(z)$
. Then, its coefficients can be expressed in terms of the elementary symmetric functions of the roots, namely,
 $$ \begin{align*} {\textrm{s}}_{i}\!\left(\gamma_1,\dots,\gamma_n\right)=(-1)^i\binom{n}{i}\dfrac{\omega_{n-i}}{\omega_n},\quad i=0,\dots,n. \end{align*} $$
$$ \begin{align*} {\textrm{s}}_{i}\!\left(\gamma_1,\dots,\gamma_n\right)=(-1)^i\binom{n}{i}\dfrac{\omega_{n-i}}{\omega_n},\quad i=0,\dots,n. \end{align*} $$
We know that the elementary symmetric functions satisfy the Newton inequalities (see, e.g., [Reference Hardy, Littlewood and Pólya5, Theorem 51]), i.e.,
 $$ \begin{align*} \left(\frac{{\textrm{s}}_{i}\!\left(\gamma_1,\dots,\gamma_n\right)}{\binom{n}{i}}\right)^2\geq \frac{{\textrm{s}}_{i-1}\!\left(\gamma_1,\dots,\gamma_n\right)}{\binom{n}{i-1}}\frac{{\textrm{s}}_{i+1}\!\left(\gamma_1,\dots,\gamma_n\right)}{\binom{n}{i+1}},\quad i=1,\dots,n-1, \end{align*} $$
$$ \begin{align*} \left(\frac{{\textrm{s}}_{i}\!\left(\gamma_1,\dots,\gamma_n\right)}{\binom{n}{i}}\right)^2\geq \frac{{\textrm{s}}_{i-1}\!\left(\gamma_1,\dots,\gamma_n\right)}{\binom{n}{i-1}}\frac{{\textrm{s}}_{i+1}\!\left(\gamma_1,\dots,\gamma_n\right)}{\binom{n}{i+1}},\quad i=1,\dots,n-1, \end{align*} $$
and thus we get
![]() $\omega _{n-i}^2\geq \omega _{n-i+1}\omega _{n-i-1}$
, for all
$\omega _{n-i}^2\geq \omega _{n-i+1}\omega _{n-i-1}$
, for all
![]() $i=1,\dots ,n-1$
. Since
$i=1,\dots ,n-1$
. Since
![]() ${\boldsymbol {\omega }}$
is a log-convex tuple, we must have the equalities
${\boldsymbol {\omega }}$
is a log-convex tuple, we must have the equalities
![]() $\omega _{n-i}^2=\omega _{n-i+1}\omega _{n-i-1}$
for every
$\omega _{n-i}^2=\omega _{n-i+1}\omega _{n-i-1}$
for every
![]() $i=1,\dots ,n-1$
, and hence, setting
$i=1,\dots ,n-1$
, and hence, setting
![]() $a=\omega _{n-1}/\omega _n$
, we have that
$a=\omega _{n-1}/\omega _n$
, we have that
![]() $\omega _{n-i}=a^i\omega _n$
for all
$\omega _{n-i}=a^i\omega _n$
for all
![]() $i=0,\dots ,n$
. Therefore,
$i=0,\dots ,n$
. Therefore,
![]() $f_{\boldsymbol {\omega }}(z)=\omega _n(z+a)^n$
, as required.▪
$f_{\boldsymbol {\omega }}(z)=\omega _n(z+a)^n$
, as required.▪
5 Bounds for the roots of log-convex coefficients and dual Steiner polynomials
In this section, we investigate additional properties for the roots of dual Steiner polynomials, providing, among others, bounds for them and a new characterization of the Euclidean ball. Again, some of these results will be obtained as consequences of the corresponding ones for log-convex coefficients polynomials. We start with some bounds for the moduli of the roots of these general polynomials.
Proposition 5.1 Let
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
and let
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
and let
![]() $\gamma _i$
,
$\gamma _i$
,
![]() $i=1,\dots ,n$
, be the roots of the polynomial
$i=1,\dots ,n$
, be the roots of the polynomial
![]() $f_{\boldsymbol {\omega }}(z)$
. Then,
$f_{\boldsymbol {\omega }}(z)$
. Then,
-
(i) The roots are bounded by
 $$ \begin{align*} \frac{\omega_{n-1}}{n\omega_n}\leq|\gamma_i|\leq\frac{n\omega_0}{\omega_1}. \end{align*} $$
$$ \begin{align*} \frac{\omega_{n-1}}{n\omega_n}\leq|\gamma_i|\leq\frac{n\omega_0}{\omega_1}. \end{align*} $$
-
(ii) Moreover,
 $$ \begin{align*} \frac{\omega_{n-1}}{\omega_n}\leq\max_{1\leq i\leq n}|\gamma_i|\quad\text{ and }\quad \min_{1\leq i\leq n}|\gamma_i|\leq\frac{\omega_0}{\omega_1}. \end{align*} $$
$$ \begin{align*} \frac{\omega_{n-1}}{\omega_n}\leq\max_{1\leq i\leq n}|\gamma_i|\quad\text{ and }\quad \min_{1\leq i\leq n}|\gamma_i|\leq\frac{\omega_0}{\omega_1}. \end{align*} $$
Proof In order to prove (i), we follow the ideas of [Reference Henk and Hernández Cifre6, Proposition 2.1]. Using Proposition 3.1, we just have to find the minimum and maximum of the quotient
 $\binom {n}{i}\omega _i/\bigl (\binom {n}{i+1}\omega _{i+1}\bigr )$
,
$\binom {n}{i}\omega _i/\bigl (\binom {n}{i+1}\omega _{i+1}\bigr )$
,
![]() $i=0,\dots ,n-1$
. The log-convexity of the tuple
$i=0,\dots ,n-1$
. The log-convexity of the tuple
![]() $(\omega _0,\dots ,\omega _n)$
, and since
$(\omega _0,\dots ,\omega _n)$
, and since
![]() $\omega _i>0$
for all
$\omega _i>0$
for all
![]() $i=0,\dots ,n$
, implies that
$i=0,\dots ,n$
, implies that
 $$ \begin{align} \frac{\omega_0}{\omega_1}\geq\frac{\omega_1}{\omega_2}\geq\dots\geq\frac{\omega_{n-1}}{\omega_n}, \end{align} $$
$$ \begin{align} \frac{\omega_0}{\omega_1}\geq\frac{\omega_1}{\omega_2}\geq\dots\geq\frac{\omega_{n-1}}{\omega_n}, \end{align} $$
whereas
 $\binom {n}{i}/\binom {n}{i+1}$
is increasing. So we get
$\binom {n}{i}/\binom {n}{i+1}$
is increasing. So we get
 $$ \begin{align*} \frac{\omega_{n-1}}{n\omega_n}\leq\frac{\binom{n}{i}\omega_i}{\binom{n}{i+1}\omega_{i+1}} \leq\frac{n\omega_0}{\omega_1} \end{align*} $$
$$ \begin{align*} \frac{\omega_{n-1}}{n\omega_n}\leq\frac{\binom{n}{i}\omega_i}{\binom{n}{i+1}\omega_{i+1}} \leq\frac{n\omega_0}{\omega_1} \end{align*} $$
for
![]() $i=0,\dots ,n-1$
.
$i=0,\dots ,n-1$
.
Next, we use Proposition 3.2: for
![]() $f_{\boldsymbol {\omega }}(z)$
it is
$f_{\boldsymbol {\omega }}(z)$
it is
 $b_i=\binom {n}{n-i}\omega _{n-i}/\omega _n$
, and hence the biggest (with regard to modulus) root of
$b_i=\binom {n}{n-i}\omega _{n-i}/\omega _n$
, and hence the biggest (with regard to modulus) root of
![]() $f_{\boldsymbol {\omega }}(z)$
satisfies
$f_{\boldsymbol {\omega }}(z)$
satisfies
 $$ \begin{align*} \max_{1\leq i\leq n}|\gamma_i|\geq \frac{1}{n}\sum_{i=1}^n\left(\frac{\omega_{n-i}}{\omega_n}\right)^{1/i}. \end{align*} $$
$$ \begin{align*} \max_{1\leq i\leq n}|\gamma_i|\geq \frac{1}{n}\sum_{i=1}^n\left(\frac{\omega_{n-i}}{\omega_n}\right)^{1/i}. \end{align*} $$
Now, from (5.1) and taking geometric means, we get that
 $$ \begin{align*} \left(\frac{\omega_{n-i}}{\omega_{n-i+1}}\frac{\omega_{n-i+1}}{\omega_{n-i+2}} \dots\frac{\omega_{n-2}}{\omega_{n-1}}\right)^{1/(i-1)}\geq\frac{\omega_{n-1}}{\omega_n},\quad i=1,\dots,n, \end{align*} $$
$$ \begin{align*} \left(\frac{\omega_{n-i}}{\omega_{n-i+1}}\frac{\omega_{n-i+1}}{\omega_{n-i+2}} \dots\frac{\omega_{n-2}}{\omega_{n-1}}\right)^{1/(i-1)}\geq\frac{\omega_{n-1}}{\omega_n},\quad i=1,\dots,n, \end{align*} $$
and thus
![]() $\omega _{n-i}\geq \omega _{n-1}^i/\omega _n^{i-1}$
for all
$\omega _{n-i}\geq \omega _{n-1}^i/\omega _n^{i-1}$
for all
![]() $i=1,\dots ,n$
. Therefore,
$i=1,\dots ,n$
. Therefore,
 $$ \begin{align*} \max_{1\leq i\leq n}|\gamma_i|\geq \frac{1}{n}\sum_{i=1}^n\frac{1}{\omega_n^{1/i}}\frac{\omega_{n-1}}{\omega_n^{(i-1)/i}}=\frac{\omega_{n-1}}{\omega_n}. \end{align*} $$
$$ \begin{align*} \max_{1\leq i\leq n}|\gamma_i|\geq \frac{1}{n}\sum_{i=1}^n\frac{1}{\omega_n^{1/i}}\frac{\omega_{n-1}}{\omega_n^{(i-1)/i}}=\frac{\omega_{n-1}}{\omega_n}. \end{align*} $$
Finally, to show the second bound in (ii), we note, on the one hand, that
![]() $\gamma _i$
are also roots of the polynomial
$\gamma _i$
are also roots of the polynomial
![]() $f_{{\boldsymbol {\omega }}'}(z)$
for
$f_{{\boldsymbol {\omega }}'}(z)$
for
![]() ${\boldsymbol {\omega }}'=(1,\omega _1/\omega _0,\dots ,\omega _n/\omega _0)\in {\mathcal {L}}^n_{>0}$
. On the other hand, Theorem 3.2 for
${\boldsymbol {\omega }}'=(1,\omega _1/\omega _0,\dots ,\omega _n/\omega _0)\in {\mathcal {L}}^n_{>0}$
. On the other hand, Theorem 3.2 for
![]() $r=1$
ensures that
$r=1$
ensures that
 $$ \begin{align*} \min_{1\leq i\leq n}|\gamma_i|\leq\frac{\omega_0}{\omega_1}. \end{align*} $$
$$ \begin{align*} \min_{1\leq i\leq n}|\gamma_i|\leq\frac{\omega_0}{\omega_1}. \end{align*} $$
This concludes the proof.▪
Next, we get bounds for the real and the imaginary parts of the roots.
Proposition 5.2 Let
![]() ${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
and let
${\boldsymbol {\omega }}=(\omega _0,\dots ,\omega _n)\in {\mathcal {L}}^n_{>0}$
and let
![]() $\gamma _i$
,
$\gamma _i$
,
![]() $i=1,\dots ,n$
, be the roots of the polynomial
$i=1,\dots ,n$
, be the roots of the polynomial
![]() $f_{\boldsymbol {\omega }}(z)$
. Then, the following properties hold:
$f_{\boldsymbol {\omega }}(z)$
. Then, the following properties hold:
-
(i) There exists
 $i_0\in \{1,\dots ,n\}$
such that
$i_0\in \{1,\dots ,n\}$
such that
 $\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _{i_0})\bigr |\leq \omega _0/\omega _1$
.
$\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _{i_0})\bigr |\leq \omega _0/\omega _1$
. -
(ii)
 $\sum _{i=0}^n\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _i)\bigr |\geq n\omega _{n-1}/\omega _n$
, and equality holds if
$\sum _{i=0}^n\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _i)\bigr |\geq n\omega _{n-1}/\omega _n$
, and equality holds if
 $\mathop{\mathrm {Re}}\nolimits (\gamma _i)\leq 0$
for all
$\mathop{\mathrm {Re}}\nolimits (\gamma _i)\leq 0$
for all
 $i=1,\dots ,n$
.
$i=1,\dots ,n$
. -
(iii)
 $\max _{1\leq i\leq n}\bigl |\mathop{\mathrm {Im}}\nolimits (\gamma _i)\bigr |\geq (1/\omega _n)\sqrt {\omega _n\omega _{n-2}-\omega _{n-1}^2}$
.
$\max _{1\leq i\leq n}\bigl |\mathop{\mathrm {Im}}\nolimits (\gamma _i)\bigr |\geq (1/\omega _n)\sqrt {\omega _n\omega _{n-2}-\omega _{n-1}^2}$
.
Proof Since
![]() $f_{\boldsymbol {\omega }}(z)=\omega _n\prod _{i=1}^n(z-\gamma _i)$
we have
$f_{\boldsymbol {\omega }}(z)=\omega _n\prod _{i=1}^n(z-\gamma _i)$
we have
 $$ \begin{align*} (-1)^n\omega_0=\omega_n\prod_{i=1}^n\gamma_i\quad\text{ and }\quad (-1)^{n-1}n\omega_1=\omega_n\sum_{i=1}^n\prod_{j\neq i}\gamma_j. \end{align*} $$
$$ \begin{align*} (-1)^n\omega_0=\omega_n\prod_{i=1}^n\gamma_i\quad\text{ and }\quad (-1)^{n-1}n\omega_1=\omega_n\sum_{i=1}^n\prod_{j\neq i}\gamma_j. \end{align*} $$
Thus, we get
 $$ \begin{align*} -n\dfrac{\omega_1}{\omega_0}=\sum_{i=1}^n\dfrac{1}{\gamma_i}=\sum_{i=1}^n\mathop{\mathrm{Re}}\nolimits\left(\dfrac{1}{\gamma_i}\right). \end{align*} $$
$$ \begin{align*} -n\dfrac{\omega_1}{\omega_0}=\sum_{i=1}^n\dfrac{1}{\gamma_i}=\sum_{i=1}^n\mathop{\mathrm{Re}}\nolimits\left(\dfrac{1}{\gamma_i}\right). \end{align*} $$
Therefore, there exists a root
![]() $\gamma _{i_0}$
such that
$\gamma _{i_0}$
such that
![]() $\mathop{\mathrm {Re}}\nolimits (1/\gamma _{i_0})\leq -\omega _1/\omega _0$
, i.e., such that
$\mathop{\mathrm {Re}}\nolimits (1/\gamma _{i_0})\leq -\omega _1/\omega _0$
, i.e., such that
 $\bigl |\mathop{\mathrm {Re}}\nolimits (1/\gamma _{i_0})\bigr |\geq \omega _1/\omega _0$
, and hence
$\bigl |\mathop{\mathrm {Re}}\nolimits (1/\gamma _{i_0})\bigr |\geq \omega _1/\omega _0$
, and hence
 $\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _{i_0})\bigr |\leq \omega _0/\omega _1$
. It proves (i).
$\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _{i_0})\bigr |\leq \omega _0/\omega _1$
. It proves (i).
Next, since
 $$ \begin{align*} \omega_n\sum_{i=1}^n\gamma_i=-n\omega_{n-1}, \end{align*} $$
$$ \begin{align*} \omega_n\sum_{i=1}^n\gamma_i=-n\omega_{n-1}, \end{align*} $$
we have
 $$ \begin{align*} n\frac{\omega_{n-1}}{\omega_n}=\left|\sum_{i=1}^n\gamma_i\right|=\left|\sum_{i=1}^n\mathop{\mathrm{Re}}\nolimits(\gamma_i)\right|\leq \sum_{i=1}^n\bigl|\mathop{\mathrm{Re}}\nolimits(\gamma_i)\bigr|. \end{align*} $$
$$ \begin{align*} n\frac{\omega_{n-1}}{\omega_n}=\left|\sum_{i=1}^n\gamma_i\right|=\left|\sum_{i=1}^n\mathop{\mathrm{Re}}\nolimits(\gamma_i)\right|\leq \sum_{i=1}^n\bigl|\mathop{\mathrm{Re}}\nolimits(\gamma_i)\bigr|. \end{align*} $$
Furthermore, if all the roots have non-positive real part, we even have
 $$ \begin{align*} \sum_{i=1}^n\bigl|\mathop{\mathrm{Re}}\nolimits(\gamma_i)\bigr|=\left|\sum_{i=1}^n\mathop{\mathrm{Re}}\nolimits(\gamma_i)\right|, \end{align*} $$
$$ \begin{align*} \sum_{i=1}^n\bigl|\mathop{\mathrm{Re}}\nolimits(\gamma_i)\bigr|=\left|\sum_{i=1}^n\mathop{\mathrm{Re}}\nolimits(\gamma_i)\right|, \end{align*} $$
which shows (ii).
Finally, we prove (iii). Denoting by
 $\gamma _i^{\tiny{(j)}}$
,
$\gamma _i^{\tiny{(j)}}$
,
![]() $j=1,\dots ,n-2$
and
$j=1,\dots ,n-2$
and
![]() $i=1,\dots ,n-j$
, the roots of the jth derivative
$i=1,\dots ,n-j$
, the roots of the jth derivative
 $f_{\boldsymbol {\omega }}^{\tiny{(j)}}(z)$
, Theorem 3.4 yields
$f_{\boldsymbol {\omega }}^{\tiny{(j)}}(z)$
, Theorem 3.4 yields
 $$ \begin{align*} \sum_{i=1}^n\bigl|\mathop{\mathrm{Im}}\nolimits(\gamma_i)\bigr| & \geq \frac{n}{n-1}\sum_{i=1}^{n-1}\left|\mathop{\mathrm{Im}}\nolimits\bigl(\gamma_i^{\tiny{(1)}}\bigr)\right| \geq\frac{n}{n-1}\frac{n-1}{n-2}\sum_{i=1}^{n-2}\left|\mathop{\mathrm{Im}}\nolimits\bigl(\gamma_i^{\tiny{(2)}}\bigr)\right|\\ & \geq\frac{n}{n-1}\frac{n-1}{n-2}\dots\frac{3}{2} \sum_{i=1}^2\left|\mathop{\mathrm{Im}}\nolimits\bigl(\gamma_i^{\tiny{(n-2)}}\bigr)\right| =\frac{n}{2}\sum_{i=1}^2\left|\mathop{\mathrm{Im}}\nolimits\bigl(\gamma_i^{\tiny{(n-2)}}\bigr)\right|. \end{align*} $$
$$ \begin{align*} \sum_{i=1}^n\bigl|\mathop{\mathrm{Im}}\nolimits(\gamma_i)\bigr| & \geq \frac{n}{n-1}\sum_{i=1}^{n-1}\left|\mathop{\mathrm{Im}}\nolimits\bigl(\gamma_i^{\tiny{(1)}}\bigr)\right| \geq\frac{n}{n-1}\frac{n-1}{n-2}\sum_{i=1}^{n-2}\left|\mathop{\mathrm{Im}}\nolimits\bigl(\gamma_i^{\tiny{(2)}}\bigr)\right|\\ & \geq\frac{n}{n-1}\frac{n-1}{n-2}\dots\frac{3}{2} \sum_{i=1}^2\left|\mathop{\mathrm{Im}}\nolimits\bigl(\gamma_i^{\tiny{(n-2)}}\bigr)\right| =\frac{n}{2}\sum_{i=1}^2\left|\mathop{\mathrm{Im}}\nolimits\bigl(\gamma_i^{\tiny{(n-2)}}\bigr)\right|. \end{align*} $$
Since
 $f_{\boldsymbol {\omega }}^{\tiny{(n-2)}}(z)=(n!/2)\bigl (\omega _{n-2}+2\omega _{n-1}z+\omega _nz^2\bigr )$
, the roots of
$f_{\boldsymbol {\omega }}^{\tiny{(n-2)}}(z)=(n!/2)\bigl (\omega _{n-2}+2\omega _{n-1}z+\omega _nz^2\bigr )$
, the roots of
 $f_{\boldsymbol {\omega }}^{\tiny{(n-2)}}(z)$
are given by
$f_{\boldsymbol {\omega }}^{\tiny{(n-2)}}(z)$
are given by
 $$ \begin{align*} \gamma_1^{\tiny{(n-2)}},\gamma_2^{\tiny{(n-2)}} =\frac{-\omega_{n-1}}{\omega_n}\pm\frac{\sqrt{\omega_n\omega_{n-2}-\omega_{n-1}^2}}{\omega_n}\,\mathrm{i}, \end{align*} $$
$$ \begin{align*} \gamma_1^{\tiny{(n-2)}},\gamma_2^{\tiny{(n-2)}} =\frac{-\omega_{n-1}}{\omega_n}\pm\frac{\sqrt{\omega_n\omega_{n-2}-\omega_{n-1}^2}}{\omega_n}\,\mathrm{i}, \end{align*} $$
and then we get
 $$ \begin{align*} \sum_{i=1}^n\bigl|\mathop{\mathrm{Im}}\nolimits(\gamma_i)\bigr|\geq n\frac{\sqrt{\omega_n\omega_{n-2}-\omega_{n-1}^2}}{\omega_n}. \end{align*} $$
$$ \begin{align*} \sum_{i=1}^n\bigl|\mathop{\mathrm{Im}}\nolimits(\gamma_i)\bigr|\geq n\frac{\sqrt{\omega_n\omega_{n-2}-\omega_{n-1}^2}}{\omega_n}. \end{align*} $$
Therefore,
 $$ \begin{align*} n\max_{1\leq i\leq n}\bigl|\mathop{\mathrm{Im}}\nolimits(\gamma_i)\bigr| \geq\sum_{i=1}^n\bigl|\mathop{\mathrm{Im}}\nolimits(\gamma_i)\bigr|\geq n\frac{\sqrt{\omega_n\omega_{n-2}-\omega_{n-1}^2}}{\omega_n}.\\[-40pt] \end{align*} $$
$$ \begin{align*} n\max_{1\leq i\leq n}\bigl|\mathop{\mathrm{Im}}\nolimits(\gamma_i)\bigr| \geq\sum_{i=1}^n\bigl|\mathop{\mathrm{Im}}\nolimits(\gamma_i)\bigr|\geq n\frac{\sqrt{\omega_n\omega_{n-2}-\omega_{n-1}^2}}{\omega_n}.\\[-40pt] \end{align*} $$
▪
5.1 Bounds for the roots of dual Steiner polynomials
Using Propositions 5.1 and 5.2, we directly get bounds for the moduli and real parts of the roots of dual Steiner polynomials depending on the dual quermassintegrals. We are also interested in obtaining bounds in terms of additional functionals related to star bodies.
We define the inner and outer radii,
![]() $\mathrm {r}(K)$
and
$\mathrm {r}(K)$
and
![]() $\mathrm {R}(K)$
, of a star body K as
$\mathrm {R}(K)$
, of a star body K as
and we will use the inequalities
for
![]() $i=0,\dots ,n-1$
: since
$i=0,\dots ,n-1$
: since
![]() $\mathrm {r}(K)B_n\subset K$
and
$\mathrm {r}(K)B_n\subset K$
and
![]() $K\subset \mathrm {R}(K)B_n$
, the above inequalities are a direct consequence of the monotonicity of the dual mixed volumes. We stress that the functionals
$K\subset \mathrm {R}(K)B_n$
, the above inequalities are a direct consequence of the monotonicity of the dual mixed volumes. We stress that the functionals
![]() $\mathrm {r}(K)$
and
$\mathrm {r}(K)$
and
![]() $\mathrm {R}(K)$
are different from the classical inradius and circumradius, because here the balls are taken to be centered at the origin; indeed, since dual quermassintegrals are not translation invariant, inequalities (5.2) would be in general not true for the classical inradius and circumradius.
$\mathrm {R}(K)$
are different from the classical inradius and circumradius, because here the balls are taken to be centered at the origin; indeed, since dual quermassintegrals are not translation invariant, inequalities (5.2) would be in general not true for the classical inradius and circumradius.
We start considering the two-dimensional case. By the dual Aleksandrov–Fenchel inequalities (2.2), the roots of the polynomial
 $\widetilde {f}_{K;B_2}(z)$
, namely,
$\widetilde {f}_{K;B_2}(z)$
, namely,
 $$ \begin{align*} \gamma_i=-\frac{\widetilde{\mathrm{W}}_1(K)}{\pi}\pm\frac{\sqrt{\pi\mathrm{vol}(K)-\widetilde{\mathrm{W}}_1(K)^2}}{\pi}\,\mathrm{i},\quad i=1,2, \end{align*} $$
$$ \begin{align*} \gamma_i=-\frac{\widetilde{\mathrm{W}}_1(K)}{\pi}\pm\frac{\sqrt{\pi\mathrm{vol}(K)-\widetilde{\mathrm{W}}_1(K)^2}}{\pi}\,\mathrm{i},\quad i=1,2, \end{align*} $$
are always nonreal complex numbers (unless
![]() $K=\lambda B_2$
), with modulus
$K=\lambda B_2$
), with modulus
 $|\gamma _i|=\sqrt {\mathrm {vol}(K)/\pi }$
; hence, using (5.2), we get the bounds
$|\gamma _i|=\sqrt {\mathrm {vol}(K)/\pi }$
; hence, using (5.2), we get the bounds
In arbitrary dimension, we can get the following bounds for the moduli and real parts of the roots. They are obtained as direct consequences of Propositions 5.1 and 5.2, and using (5.2):
Proposition 5.3 Let
![]() $K\in \mathcal {S}^n_0$
and let
$K\in \mathcal {S}^n_0$
and let
![]() $\gamma _i$
,
$\gamma _i$
,
![]() $i=1,\dots ,n$
, be the roots of the dual Steiner polynomial
$i=1,\dots ,n$
, be the roots of the dual Steiner polynomial
 $\widetilde {f}_{K;B_n}(z)$
. Then the following properties hold:
$\widetilde {f}_{K;B_n}(z)$
. Then the following properties hold:
-
(i) The roots are bounded by
Furthermore, $$ \begin{align*} \frac{\mathrm{r}(K)}{n}\leq|\gamma_i|\leq n\mathrm{R}(K). \end{align*} $$
$$ \begin{align*} \frac{\mathrm{r}(K)}{n}\leq|\gamma_i|\leq n\mathrm{R}(K). \end{align*} $$
 $$ \begin{align*} \mathrm{r}(K)\leq\max_{1\leq i\leq n}|\gamma_i|\quad\text{ and }\quad \min_{1\leq i\leq n}|\gamma_i|\leq\mathrm{R}(K). \end{align*} $$
$$ \begin{align*} \mathrm{r}(K)\leq\max_{1\leq i\leq n}|\gamma_i|\quad\text{ and }\quad \min_{1\leq i\leq n}|\gamma_i|\leq\mathrm{R}(K). \end{align*} $$
-
(ii) There exists
 $i_0\in \{1,\dots ,n\}$
such that
$i_0\in \{1,\dots ,n\}$
such that
 $\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _{i_0})\bigr |\leq \mathrm {R}(K)$
.
$\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _{i_0})\bigr |\leq \mathrm {R}(K)$
. -
(iii) Moreover, if
 $\mathop{\mathrm {Re}}\nolimits (\gamma _i)\leq 0$
for all
$\mathop{\mathrm {Re}}\nolimits (\gamma _i)\leq 0$
for all
 $i=1,\dots ,n$
then
$i=1,\dots ,n$
then
 $\sum _{i=0}^n\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _i)\bigr |\leq n\mathrm {R}(K)$
.
$\sum _{i=0}^n\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _i)\bigr |\leq n\mathrm {R}(K)$
. -
(iv)
 $\sum _{i=0}^n\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _i)\bigr |\geq n\mathrm {r}(K)$
.
$\sum _{i=0}^n\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _i)\bigr |\geq n\mathrm {r}(K)$
.
Remark 5.1 Following the argument of the proof of (i) in Proposition 5.2, we can also write the inequality
 $$ \begin{align*} -n\frac{1}{\mathrm{r}(K)}\leq-n\dfrac{\widetilde{\mathrm{W}}_1(K)}{\mathrm{vol}(K)}=\sum_{i=1}^n\mathop{\mathrm{Re}}\nolimits\left(\dfrac{1}{\gamma_i}\right). \end{align*} $$
$$ \begin{align*} -n\frac{1}{\mathrm{r}(K)}\leq-n\dfrac{\widetilde{\mathrm{W}}_1(K)}{\mathrm{vol}(K)}=\sum_{i=1}^n\mathop{\mathrm{Re}}\nolimits\left(\dfrac{1}{\gamma_i}\right). \end{align*} $$
Thus, one may conclude the existence of a root
![]() $\gamma _j$
with
$\gamma _j$
with
![]() $\mathop{\mathrm {Re}}\nolimits (1/\gamma _j)\geq -1/\mathrm {r}(K)$
. This would provide a bound for the real part of the root
$\mathop{\mathrm {Re}}\nolimits (1/\gamma _j)\geq -1/\mathrm {r}(K)$
. This would provide a bound for the real part of the root
![]() $\widetilde {\gamma }_j=1/\gamma _j$
of the dual Steiner polynomial
$\widetilde {\gamma }_j=1/\gamma _j$
of the dual Steiner polynomial
 $\widetilde {f}_{B_n;K}(z)$
. Indeed, since
$\widetilde {f}_{B_n;K}(z)$
. Indeed, since
![]() $\widetilde {\mathrm {W}}_i(K)=\widetilde {\mathrm {W}}_{n-i}(B_n;K)$
we have
$\widetilde {\mathrm {W}}_i(K)=\widetilde {\mathrm {W}}_{n-i}(B_n;K)$
we have
 $\widetilde {f}_{K;B_n}(z)=z^n\widetilde {f}_{B_n;K}(1/z)$
, and thus, the roots of
$\widetilde {f}_{K;B_n}(z)=z^n\widetilde {f}_{B_n;K}(1/z)$
, and thus, the roots of
 $\widetilde {f}_{B_n;K}(z)$
are precisely
$\widetilde {f}_{B_n;K}(z)$
are precisely
![]() $1/\gamma _i$
,
$1/\gamma _i$
,
![]() $i=1,\dots ,n$
.
$i=1,\dots ,n$
.
5.2 A characterization of the ball via the roots of the dual Steiner polynomials
As we have seen, for any dual Steiner polynomial there always exists a root
![]() $\gamma $
with real part lying in the interval
$\gamma $
with real part lying in the interval
 $\bigl [-\mathrm {R}(K),\mathrm {R}(K)\bigr ]$
(cf. Proposition 5.3 (ii)), and indeed, there are star bodies such that all the real parts of the roots of
$\bigl [-\mathrm {R}(K),\mathrm {R}(K)\bigr ]$
(cf. Proposition 5.3 (ii)), and indeed, there are star bodies such that all the real parts of the roots of
 $\widetilde {f}_{K;B_n}(z)$
lie in the above interval, as the following example shows.
$\widetilde {f}_{K;B_n}(z)$
lie in the above interval, as the following example shows.
Example 5.1 We consider the positive numbers
![]() $(2,1,1)$
. Since they satisfy the dual Aleksandrov–Fenchel inequalities, Theorem 2.1 ensures the existence of
$(2,1,1)$
. Since they satisfy the dual Aleksandrov–Fenchel inequalities, Theorem 2.1 ensures the existence of
![]() $K\in \mathcal {S}^3_0$
such that
$K\in \mathcal {S}^3_0$
such that
![]() $\mathrm {vol}(K)=2$
and
$\mathrm {vol}(K)=2$
and
![]() $\widetilde {\mathrm {W}}_1(K)=\widetilde {\mathrm {W}}_2(K)=1$
. Then, by numerical computations, one can check that the real parts of the roots of the dual Steiner polynomial
$\widetilde {\mathrm {W}}_1(K)=\widetilde {\mathrm {W}}_2(K)=1$
. Then, by numerical computations, one can check that the real parts of the roots of the dual Steiner polynomial
 $\widetilde {f}_{K;B_3}(z)=2+3z+3z^2+(4\pi /3)z^3$
lie in the interval
$\widetilde {f}_{K;B_3}(z)=2+3z+3z^2+(4\pi /3)z^3$
lie in the interval
 $$ \begin{align*} (-2,2)=\left(-\frac{\mathrm{vol}(K)}{\widetilde{\mathrm{W}}_1(K)},\frac{\mathrm{vol}(K)}{\widetilde{\mathrm{W}}_1(K)}\right)\subset\bigl(-\mathrm{R}(K),\mathrm{R}(K)\bigr), \end{align*} $$
$$ \begin{align*} (-2,2)=\left(-\frac{\mathrm{vol}(K)}{\widetilde{\mathrm{W}}_1(K)},\frac{\mathrm{vol}(K)}{\widetilde{\mathrm{W}}_1(K)}\right)\subset\bigl(-\mathrm{R}(K),\mathrm{R}(K)\bigr), \end{align*} $$
where the last inclusion follows from (5.2).
However, the interval
 $\bigl (-\mathrm {R}(K),\mathrm {R}(K)\bigr )$
cannot be reduced to the one determined by the inner radius, as Corollary 5.1 shows. This will be an easy consequence of the characterization of the Euclidean ball given in Theorem 2.2, which we prove next.
$\bigl (-\mathrm {R}(K),\mathrm {R}(K)\bigr )$
cannot be reduced to the one determined by the inner radius, as Corollary 5.1 shows. This will be an easy consequence of the characterization of the Euclidean ball given in Theorem 2.2, which we prove next.
Proof of Theorem 2.2
If
![]() $K=aB_n$
, then
$K=aB_n$
, then
 $\widetilde {f}_{K;B_n}(z)=\kappa _n(z+a)^n$
, and hence it has an n-fold real root, namely,
$\widetilde {f}_{K;B_n}(z)=\kappa _n(z+a)^n$
, and hence it has an n-fold real root, namely,
![]() $\gamma _1=\dots =\gamma _n=-a$
. So we assume that
$\gamma _1=\dots =\gamma _n=-a$
. So we assume that
![]() $\mathop{\mathrm {Re}}\nolimits (\gamma _i)=-a$
for
$\mathop{\mathrm {Re}}\nolimits (\gamma _i)=-a$
for
![]() $i=1,\dots ,n$
, and we prove the assertion by induction on the dimension.
$i=1,\dots ,n$
, and we prove the assertion by induction on the dimension.
Let
![]() $n=3$
, and we suppose that K is not a ball. Let
$n=3$
, and we suppose that K is not a ball. Let
![]() $\gamma _1=-a$
,
$\gamma _1=-a$
,
![]() $\gamma _2=-a+b\mathrm {i}$
and
$\gamma _2=-a+b\mathrm {i}$
and
![]() $\gamma _3=-a-b\mathrm {i}$
, where
$\gamma _3=-a-b\mathrm {i}$
, where
![]() $b>0$
because
$b>0$
because
![]() $K\neq aB_3$
. Then
$K\neq aB_3$
. Then
 $\widetilde {f}_{K;B_3}(z)=\kappa _3\bigl (z^3+3az^2+(3a^2+b^2)z+a(a^2+b^2)\bigr )$
, and hence
$\widetilde {f}_{K;B_3}(z)=\kappa _3\bigl (z^3+3az^2+(3a^2+b^2)z+a(a^2+b^2)\bigr )$
, and hence
 $$ \begin{align} \widetilde{\mathrm{W}}_2(K)=\kappa_3a,\quad \widetilde{\mathrm{W}}_1(K)=\frac{\kappa_3}{3}(3a^2+b^2),\quad \mathrm{vol}(K)=\kappa_3a(a^2+b^2). \end{align} $$
$$ \begin{align} \widetilde{\mathrm{W}}_2(K)=\kappa_3a,\quad \widetilde{\mathrm{W}}_1(K)=\frac{\kappa_3}{3}(3a^2+b^2),\quad \mathrm{vol}(K)=\kappa_3a(a^2+b^2). \end{align} $$
Since K is not a ball, the dual Aleksandrov–Fenchel inequalities must hold strictly; in particular one has
![]() $\widetilde {\mathrm {W}}_1(K)^2<\mathrm {vol}(K)\widetilde {\mathrm {W}}_2(K)$
, or equivalently, using (5.3), we have
$\widetilde {\mathrm {W}}_1(K)^2<\mathrm {vol}(K)\widetilde {\mathrm {W}}_2(K)$
, or equivalently, using (5.3), we have
 $$ \begin{align*} \frac{\kappa_3^2}{9}b^2(b^2-3a^2)<0. \end{align*} $$
$$ \begin{align*} \frac{\kappa_3^2}{9}b^2(b^2-3a^2)<0. \end{align*} $$
Since
![]() $b\neq 0$
, the above inequality holds if and only if
$b\neq 0$
, the above inequality holds if and only if
![]() $b<\sqrt {3}\,a$
. But this is a contradiction, because, as we have proved in Corollary 2.1, if
$b<\sqrt {3}\,a$
. But this is a contradiction, because, as we have proved in Corollary 2.1, if
![]() $-a+b\mathrm {i}$
is a root of a three-dimensional dual Steiner polynomial then
$-a+b\mathrm {i}$
is a root of a three-dimensional dual Steiner polynomial then
![]() $b>\sqrt {3}\,a$
. Therefore,
$b>\sqrt {3}\,a$
. Therefore,
![]() $K=aB_3$
, which concludes the proof in the case
$K=aB_3$
, which concludes the proof in the case
![]() $n=3$
.
$n=3$
.
Now let
![]() $n>3$
, and we assume that the assertion is true in dimension
$n>3$
, and we assume that the assertion is true in dimension
![]() $n-1$
. Again, we suppose that K is not a ball. It is known that the derivative of
$n-1$
. Again, we suppose that K is not a ball. It is known that the derivative of
 $\widetilde {f}_{K;B_n}(z)$
is also a dual Steiner polynomial (see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Proposition 4.1]). Hence, there exists
$\widetilde {f}_{K;B_n}(z)$
is also a dual Steiner polynomial (see [Reference Alonso-Gutiérrez, Henk and Hernández Cifre1, Proposition 4.1]). Hence, there exists
![]() $K'\in \mathcal {S}^{n-1}_0$
such that
$K'\in \mathcal {S}^{n-1}_0$
such that
 $$ \begin{align*} \widetilde{f}_{K;B_n}^{\;\prime}(z) & =\sum_{i=1}^n\binom{n}{i}i\widetilde{\mathrm{W}}_i(K)z^{i-1} =\sum_{i=0}^{n-1}\binom{n-1}{i}n\widetilde{\mathrm{W}}_{i+1}(K)z^i\\ & =\frac{n\kappa_n}{\kappa_{n-1}}\sum_{i=0}^{n-1}\binom{n-1}{i}\widetilde{\mathrm{W}}_i(K')z^i =\frac{n\kappa_n}{\kappa_{n-1}}\widetilde{f}_{K';B_{n-1}}(z), \end{align*} $$
$$ \begin{align*} \widetilde{f}_{K;B_n}^{\;\prime}(z) & =\sum_{i=1}^n\binom{n}{i}i\widetilde{\mathrm{W}}_i(K)z^{i-1} =\sum_{i=0}^{n-1}\binom{n-1}{i}n\widetilde{\mathrm{W}}_{i+1}(K)z^i\\ & =\frac{n\kappa_n}{\kappa_{n-1}}\sum_{i=0}^{n-1}\binom{n-1}{i}\widetilde{\mathrm{W}}_i(K')z^i =\frac{n\kappa_n}{\kappa_{n-1}}\widetilde{f}_{K';B_{n-1}}(z), \end{align*} $$
where
![]() $\widetilde {\mathrm {W}}_i(K')=(\kappa _{n-1}/\kappa _n)\widetilde {\mathrm {W}}_{i+1}(K)$
,
$\widetilde {\mathrm {W}}_i(K')=(\kappa _{n-1}/\kappa _n)\widetilde {\mathrm {W}}_{i+1}(K)$
,
![]() $i=0,\dots ,n-1$
. On the one hand, since K is not a ball, the dual Aleksandrov–Fenchel inequalities (2.2) hold strictly for the dual quermassintegrals
$i=0,\dots ,n-1$
. On the one hand, since K is not a ball, the dual Aleksandrov–Fenchel inequalities (2.2) hold strictly for the dual quermassintegrals
![]() $\widetilde {\mathrm {W}}_i(K)$
, and therefore, the same occurs for
$\widetilde {\mathrm {W}}_i(K)$
, and therefore, the same occurs for
![]() $\widetilde {\mathrm {W}}_i(K')$
; so,
$\widetilde {\mathrm {W}}_i(K')$
; so,
![]() $K'$
is not an (
$K'$
is not an (
![]() $n-1$
)-dimensional ball.
$n-1$
)-dimensional ball.
On the other hand, denoting by
![]() $\gamma _i'$
,
$\gamma _i'$
,
![]() $i=1,\dots ,n-1$
, the roots of
$i=1,\dots ,n-1$
, the roots of
 $\widetilde {f}_{K;B_n}^{\;\prime }(z)$
, Lucas’ theorem (see Theorem 3.3) ensures that
$\widetilde {f}_{K;B_n}^{\;\prime }(z)$
, Lucas’ theorem (see Theorem 3.3) ensures that
and hence
![]() $\mathop{\mathrm {Re}}\nolimits (\gamma _i')=-a$
for all
$\mathop{\mathrm {Re}}\nolimits (\gamma _i')=-a$
for all
![]() $i=1,\dots ,n-1$
. Since we are assuming that the assertion is true in dimension
$i=1,\dots ,n-1$
. Since we are assuming that the assertion is true in dimension
![]() $n-1$
, we get the desired contradiction. This concludes the proof.▪
$n-1$
, we get the desired contradiction. This concludes the proof.▪
Corollary 5.1 Let
![]() $K\in \mathcal {S}^n_0$
and let
$K\in \mathcal {S}^n_0$
and let
![]() $\gamma _i$
,
$\gamma _i$
,
![]() $i=1,\dots ,n$
, be the roots of the dual Steiner polynomial
$i=1,\dots ,n$
, be the roots of the dual Steiner polynomial
 $\widetilde {f}_{K;B_n}(z)$
. If
$\widetilde {f}_{K;B_n}(z)$
. If
 $\mathop{\mathrm {Re}}\nolimits (\gamma _i)\in \bigl [-\mathrm {r}(K),\mathrm {r}(K)\bigr )$
for all
$\mathop{\mathrm {Re}}\nolimits (\gamma _i)\in \bigl [-\mathrm {r}(K),\mathrm {r}(K)\bigr )$
for all
![]() $i=1,\dots ,n$
, then
$i=1,\dots ,n$
, then
![]() $\gamma _i=-\mathrm {r}(K)$
for all
$\gamma _i=-\mathrm {r}(K)$
for all
![]() $i=1,\dots ,n$
, and hence
$i=1,\dots ,n$
, and hence
![]() $K=\mathrm {r}(K)B_n$
.
$K=\mathrm {r}(K)B_n$
.
Proof We assume there exists
![]() $j\in \{1,\dots ,n\}$
such that
$j\in \{1,\dots ,n\}$
such that
![]() $\mathop{\mathrm {Re}}\nolimits (\gamma _j)>-\mathrm {r}(K)$
. Then
$\mathop{\mathrm {Re}}\nolimits (\gamma _j)>-\mathrm {r}(K)$
. Then
 $\sum _{i=1}^n\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _i)\bigr |<n\mathrm {r}(K)$
, which is not possible (see Proposition 5.3 (iv)). Therefore
$\sum _{i=1}^n\bigl |\mathop{\mathrm {Re}}\nolimits (\gamma _i)\bigr |<n\mathrm {r}(K)$
, which is not possible (see Proposition 5.3 (iv)). Therefore
![]() $\mathop{\mathrm {Re}}\nolimits (\gamma _i)=-\mathrm {r}(K)$
for all
$\mathop{\mathrm {Re}}\nolimits (\gamma _i)=-\mathrm {r}(K)$
for all
![]() $i=1,\dots ,n$
and Theorem 2.2 gives the result.▪
$i=1,\dots ,n$
and Theorem 2.2 gives the result.▪
Acknowledgement
The authors would like to strongly thank the anonymous referees for the very valuable comments and helpful suggestions; their observations allowed us to considerably improve the article.