No CrossRef data available.
Published online by Cambridge University Press: 31 January 2023
We clarify and refine the definition of a reciprocal random field on an undirected graph, with the reciprocal chain as a special case, by introducing four new properties: the factorizing, global, local, and pairwise reciprocal properties, in decreasing order of strength, with respect to a set of nodes 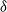 $\delta$. They reduce to the better-known Markov properties if
$\delta$. They reduce to the better-known Markov properties if 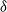 $\delta$ is the empty set, or, with the exception of the local property, if
$\delta$ is the empty set, or, with the exception of the local property, if 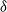 $\delta$ is a complete set. Conditions for each reciprocal property to imply the next stronger property are derived, and it is shown that, conditionally on the values at a set of nodes
$\delta$ is a complete set. Conditions for each reciprocal property to imply the next stronger property are derived, and it is shown that, conditionally on the values at a set of nodes 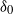 $\delta_0$, all four properties are preserved for the subgraph induced by the remaining nodes, with respect to the node set
$\delta_0$, all four properties are preserved for the subgraph induced by the remaining nodes, with respect to the node set 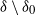 $\delta\setminus\delta_0$. We note that many of the above results are new even for reciprocal chains.
$\delta\setminus\delta_0$. We note that many of the above results are new even for reciprocal chains.