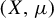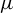1 Introduction
The study of ergodic theorems is an important bridge between functional analysis and probability theory. Originally proved by Birkhoff [Reference Birkhoff4] in 1931, the Birkhoff ergodic theorem has become the fundamental theorem in the study of measure-preserving transformations of a measure space. The subadditive ergodic theorem, obtained by Kingman [Reference Kingman10] in 1968, is an important extension of this fundamental result, which has found many applications. One important application is the Furstenberg–Kesten theorem [Reference Furstenberg and Kesten6], on the structure of multiplicative cocycles from a measure-preserving transformation T of a measure space
![]() $(X, \mu )$
, with values in
$(X, \mu )$
, with values in
![]() $GL(d, {\mathbb {R}})$
. The Furstenberg–Kesten theorem has been extended and refined by the well-known Oseledeč ergodic theorem on the products of randomly chosen matrices [Reference Oseledets11].
$GL(d, {\mathbb {R}})$
. The Furstenberg–Kesten theorem has been extended and refined by the well-known Oseledeč ergodic theorem on the products of randomly chosen matrices [Reference Oseledets11].
A statement of Kingman’s theorem is as follows.
Theorem 1. (The sub-additive ergodic theorem)
Let
![]() $(X,{\mathcal {B}},\mu )$
be a probability space, and
$(X,{\mathcal {B}},\mu )$
be a probability space, and
![]() $T:X\to X$
an invertible and measure-preserving transformation. Let
$T:X\to X$
an invertible and measure-preserving transformation. Let
![]() $f_{n}\in L^{1}$
be a sequence of functions satisfying the subadditivity condition:
$f_{n}\in L^{1}$
be a sequence of functions satisfying the subadditivity condition:
![]() $f_{m+n}(x)\leq f_{n}(x)+f_{m}(T^{n}x)$
for almost all
$f_{m+n}(x)\leq f_{n}(x)+f_{m}(T^{n}x)$
for almost all
![]() $x\in X$
. Then
$x\in X$
. Then
exists
![]() $\mu $
-almost everywhere (a.e). Furthermore,
$\mu $
-almost everywhere (a.e). Furthermore,
![]() $f(x)$
is a T-invariant measurable function over
$f(x)$
is a T-invariant measurable function over
![]() $(X,{\mathcal {B}},\mu )$
.
$(X,{\mathcal {B}},\mu )$
.
There have been several proofs of this theorem since Kingman’s original version. See [Reference Aaronson1] for a survey of these. Most of them have made the assumption that the measure
![]() $\mu $
is invariant under the transformation T. However, [Reference Silva and Thieullen14, Theorem 3.4] is a version of the subadditive ergodic theorem under the assumption that T is a Markovian transformation of
$\mu $
is invariant under the transformation T. However, [Reference Silva and Thieullen14, Theorem 3.4] is a version of the subadditive ergodic theorem under the assumption that T is a Markovian transformation of
![]() $(X, \mathcal {B}, \mu )$
.
$(X, \mathcal {B}, \mu )$
.
Note that the theorem generalizes the following result in elementary analysis, which we recover in the case where the
![]() $f_{n}$
are all constant functions.
$f_{n}$
are all constant functions.
Lemma 1. If (
![]() $f_{n}$
) is a subadditive sequence then
$f_{n}$
) is a subadditive sequence then
The aim of this paper is to use [Reference Silva and Thieullen14, Theorem 3.4] to extend the Furstenberg–Kesten theorem and the Oceledeč theorem to the setting of non-singular transformations. The key idea is to define subadditive sequences by
where
![]() $\omega _n(x)= ({d{\kern-1pt}\mu \circ T^n})/{d{\kern-1pt}\mu }$
is the Radon–Nikodým derivative.
$\omega _n(x)= ({d{\kern-1pt}\mu \circ T^n})/{d{\kern-1pt}\mu }$
is the Radon–Nikodým derivative.
The non-singular version theorem of [Reference Silva and Thieullen14] allows us to conclude that
 $$ \begin{align*} \lim_{n\to\infty} \frac{f_{n}(x)}{\sum_{i=0}^{n-1}\omega_{i}(x)}=f_{\ast}(x)< \infty \end{align*} $$
$$ \begin{align*} \lim_{n\to\infty} \frac{f_{n}(x)}{\sum_{i=0}^{n-1}\omega_{i}(x)}=f_{\ast}(x)< \infty \end{align*} $$
exists
![]() $\mu $
-a.e.
$\mu $
-a.e.
After some preliminary remarks and definitions in §2, we review [Reference Silva and Thieullen14, Theorem 3.4] in §3. In §4 we state and prove non-singular versions of the Furstenberg–Kesten theorem (Theorem 6) and the Oseledeč ergodic theorem (Theorem 7).
We expect these results to lead to new applications of these theorems in the non-singular setting. One key application of the Oseledeč theorem in the measure-preserving case is the calculation of Lyapunov exponents for random dynamical systems; see [Reference Arnold2]. In future work, we will extend this construction to non-singular random dynamical systems.
2 Preliminaries
The dynamical system
![]() $(X,{\mathcal {B}},\mu , T)$
is said to be non-singular if the map
$(X,{\mathcal {B}},\mu , T)$
is said to be non-singular if the map
![]() $T:X\to X$
is a non-singular transformation of
$T:X\to X$
is a non-singular transformation of
![]() $(X,\mu )$
, that is, for any
$(X,\mu )$
, that is, for any
![]() $N\in {\mathcal {B}}$
,
$N\in {\mathcal {B}}$
,
![]() $\mu (T N)=0$
if and only if
$\mu (T N)=0$
if and only if
![]() $\mu (N)=0$
. Recall that the system is measure-preserving if
$\mu (N)=0$
. Recall that the system is measure-preserving if
![]() $\mu (A)=\mu (T A)$
for all
$\mu (A)=\mu (T A)$
for all
![]() $A\in {\mathcal {B}}$
. By the Poincaré recurrence lemma, measure-preserving transformations are conservative.
$A\in {\mathcal {B}}$
. By the Poincaré recurrence lemma, measure-preserving transformations are conservative.
The structure of non-singular transformations is given by the Hopf decomposition theorem, a proof of which can be found in [Reference Aaronson1].
Theorem 2. (Hopf decomposition)
Let T be a non-singular transformation. There exist disjoint invariant sets
![]() $C,D\in {\mathcal {B}}$
such that
$C,D\in {\mathcal {B}}$
such that
![]() $X=C\sqcup D$
, T restricted to C is conservative, and
$X=C\sqcup D$
, T restricted to C is conservative, and
![]() $D=\bigsqcup _{n=-\infty }^{\infty } T^{n}W$
, where W is a wandering set. If
$D=\bigsqcup _{n=-\infty }^{\infty } T^{n}W$
, where W is a wandering set. If
![]() $f\in L^{1}(X,\mu )$
,
$f\in L^{1}(X,\mu )$
,
![]() $f>0$
, then
$f>0$
, then
![]() $C=\{x:\sum _{i=1}^{n-1}f(T^{i}x)\omega _{i}(x)=\infty \ {a.e.}\}$
and
$C=\{x:\sum _{i=1}^{n-1}f(T^{i}x)\omega _{i}(x)=\infty \ {a.e.}\}$
and
![]() $D=\{ x:\sum _{i=1}^{n-1}f(T^{i}x)\omega _{i}(x)<\infty \ {a.e.}\}$
$D=\{ x:\sum _{i=1}^{n-1}f(T^{i}x)\omega _{i}(x)<\infty \ {a.e.}\}$
The set C is called the conservative part of T. If the non-singular transformation is invertible, so that T and its inverse
![]() $T^{-1}$
are measurable, then we have both
$T^{-1}$
are measurable, then we have both
![]() $\mu \circ T^{-1}\sim \mu $
and
$\mu \circ T^{-1}\sim \mu $
and
![]() $\mu \circ T\sim \mu $
. However, we do not assume further the transformation is invertible.
$\mu \circ T\sim \mu $
. However, we do not assume further the transformation is invertible.
We will denote the Radon–Nikodým derivative
![]() ${d(\mu \circ T^{i})}/{d{\kern-1pt}\mu }$
by
${d(\mu \circ T^{i})}/{d{\kern-1pt}\mu }$
by
![]() $\omega _{i}$
. Note that the Radon–Nikodým derivatives must satisfy the cocycle identity
$\omega _{i}$
. Note that the Radon–Nikodým derivatives must satisfy the cocycle identity
for almost every x and for every
![]() $i,j \in {\mathbb {Z}}$
. Clearly, T is measure-preserving if and only if
$i,j \in {\mathbb {Z}}$
. Clearly, T is measure-preserving if and only if
![]() $\omega _{i}(x)=1$
for almost every x for all i.
$\omega _{i}(x)=1$
for almost every x for all i.
It follows that for every
![]() $f\in L^{1}(X,\mu )$
$f\in L^{1}(X,\mu )$
If
![]() $f_{n}=\sum _{i=0}^{n-1}f(T^{i}x)\omega _{i}(x),n\geq 1$
, where
$f_{n}=\sum _{i=0}^{n-1}f(T^{i}x)\omega _{i}(x),n\geq 1$
, where
![]() $\omega _{0}(x)=1$
, it is easy to show that
$\omega _{0}(x)=1$
, it is easy to show that
![]() $f_{m+n}(x)=f_{n}(x)+\omega _{n}(x)f_{m}(T^{n}x)$
. The Hurewicz ergodic theorem [Reference Hurewicz7] is a generalization of the Birkhoff ergodic theorem to the setting of non-singular conservative transformations.
$f_{m+n}(x)=f_{n}(x)+\omega _{n}(x)f_{m}(T^{n}x)$
. The Hurewicz ergodic theorem [Reference Hurewicz7] is a generalization of the Birkhoff ergodic theorem to the setting of non-singular conservative transformations.
Theorem 3. (Hurewicz ergodic theorem)
Let
![]() $(X,{\mathcal {B}},\mu )$
be a probability space, and
$(X,{\mathcal {B}},\mu )$
be a probability space, and
![]() $T:X\to X$
a non-singular conservative transformation. If
$T:X\to X$
a non-singular conservative transformation. If
![]() $f\in L^{1}(\mu )$
, then
$f\in L^{1}(\mu )$
, then
 $$ \begin{align*} \lim_{n\to\infty} \frac{\sum_{i=0}^{n-1}f(T^{i}x)\omega_{i}(x)}{\sum_{i=0}^{n-1}\omega_{i}(x)}=f_{\ast}(x) \end{align*} $$
$$ \begin{align*} \lim_{n\to\infty} \frac{\sum_{i=0}^{n-1}f(T^{i}x)\omega_{i}(x)}{\sum_{i=0}^{n-1}\omega_{i}(x)}=f_{\ast}(x) \end{align*} $$
exists
![]() $\mu $
-a.e. Furthermore,
$\mu $
-a.e. Furthermore,
![]() $f_{\ast }(x)$
is T- invariant and
$f_{\ast }(x)$
is T- invariant and
Note that if T is measure-preserving, the left-hand side becomes
 $$ \begin{align*} \lim _{n\to \infty } ({1}/{n})\sum _{i=0}^{n-1} f(T^{i}x), \end{align*} $$
$$ \begin{align*} \lim _{n\to \infty } ({1}/{n})\sum _{i=0}^{n-1} f(T^{i}x), \end{align*} $$
and so we recover the Birkhoff theorem.
3 Non-singular Kingman theorem
Thus, let T be a conservative non-singular transformation of a measure space
![]() $(X, {\mathcal {B}}, \mu )$
, and denote by
$(X, {\mathcal {B}}, \mu )$
, and denote by
![]() $\omega _i$
the Radon–Nikodým derivative
$\omega _i$
the Radon–Nikodým derivative
![]() $({d{\kern-1pt}\mu \circ T^{i}})/{ d{\kern-1pt}\mu }$
.
$({d{\kern-1pt}\mu \circ T^{i}})/{ d{\kern-1pt}\mu }$
.
Definition 1. We say that
![]() $\{f_n \}$
in
$\{f_n \}$
in
![]() $L^1(X, \mu )$
is a subadditive sequence for T if, for all integers m and n,
$L^1(X, \mu )$
is a subadditive sequence for T if, for all integers m and n,
It is easy to see that if f is integrable, then
 $$ \begin{align*} f_{n}(x) = \sum_{i=0}^{n-1}f(T^{i}x)\omega_{i}(x). \end{align*} $$
$$ \begin{align*} f_{n}(x) = \sum_{i=0}^{n-1}f(T^{i}x)\omega_{i}(x). \end{align*} $$
is subadditive.
Similarly, we say that
![]() $\{f_n \}$
in
$\{f_n \}$
in
![]() $L^1(X, \mu )$
is superadditive for T if, for all integers m and n,
$L^1(X, \mu )$
is superadditive for T if, for all integers m and n,
Observe that
![]() $f_{n}$
is a superadditive sequence if and only if
$f_{n}$
is a superadditive sequence if and only if
![]() $-f_{n}$
is a subadditive sequence.
$-f_{n}$
is a subadditive sequence.
We now state the non-singular Kingman theorem,
Theorem 4. (Non-singular Kingman ergodic theorem)
Let
![]() $(X,{\mathcal {B}},\mu )$
be a probability space, and
$(X,{\mathcal {B}},\mu )$
be a probability space, and
![]() $T:X\to X$
a non-singular conservative transformation. Let
$T:X\to X$
a non-singular conservative transformation. Let
![]() $f_{n}\in L^{1}$
be a sequence of functions satisfying the subadditivity relation
$f_{n}\in L^{1}$
be a sequence of functions satisfying the subadditivity relation
![]() $f_{m+n}(x)\leq f_{n}(x)+\omega _{n}(x)f_{m}(T^{n}x)$
for almost all
$f_{m+n}(x)\leq f_{n}(x)+\omega _{n}(x)f_{m}(T^{n}x)$
for almost all
![]() $x\in X$
. Then
$x\in X$
. Then
 $$ \begin{align*} \lim_{n\to\infty} \frac{f_{n}(x)}{\sum_{i=0}^{n-1}\omega_{i}(x)}=f_{\ast}(x)< \infty \end{align*} $$
$$ \begin{align*} \lim_{n\to\infty} \frac{f_{n}(x)}{\sum_{i=0}^{n-1}\omega_{i}(x)}=f_{\ast}(x)< \infty \end{align*} $$
exists
![]() $\mu $
-a.e. Furthermore,
$\mu $
-a.e. Furthermore,
![]() $f_{\ast }(x)$
is T-invariant and
$f_{\ast }(x)$
is T-invariant and
This theorem follows easily from the following fact, which follows from the maximal ergodic theorem:
 $$ \begin{align*} \int_{X}\limsup_{n\to\infty}\frac{f_{n}}{\sum_{i=0}^{n-1}\omega_{i}(x)}\,d{\kern-1pt}\mu\leq \int L \,d{\kern-1pt}\mu \leq \int_{X}\liminf_{n\to\infty} \frac{f_{n}}{\sum_{i=0}^{n-1}\omega_{i}(x)}\,d{\kern-1pt}\mu \end{align*} $$
$$ \begin{align*} \int_{X}\limsup_{n\to\infty}\frac{f_{n}}{\sum_{i=0}^{n-1}\omega_{i}(x)}\,d{\kern-1pt}\mu\leq \int L \,d{\kern-1pt}\mu \leq \int_{X}\liminf_{n\to\infty} \frac{f_{n}}{\sum_{i=0}^{n-1}\omega_{i}(x)}\,d{\kern-1pt}\mu \end{align*} $$
where L is
 $$ \begin{align*}\lim_{n\to\infty}\frac{f_{n}}{\sum_{i=0}^{n-1}\omega_{i}(x)}.\end{align*} $$
$$ \begin{align*}\lim_{n\to\infty}\frac{f_{n}}{\sum_{i=0}^{n-1}\omega_{i}(x)}.\end{align*} $$
Proof. Theorem 3.4 of [Reference Silva and Thieullen14] states that if
![]() $\{f_n\}$
is subadditive and
$\{f_n\}$
is subadditive and
![]() $\{g_n\}$
is superadditive, the limit
$\{g_n\}$
is superadditive, the limit
 $$ \begin{align*} \lim_{n\to \infty} \frac{f_n}{g_n} = \frac{\lim_{n \to \infty}(1/n) \mathbb{E}_{h\mu} [ f_n / h |\mathcal{I}]}{\lim_{n \to \infty}(1/n) \mathbb{E}_{h\mu} [ g_n / h |\mathcal{I}]} \end{align*} $$
$$ \begin{align*} \lim_{n\to \infty} \frac{f_n}{g_n} = \frac{\lim_{n \to \infty}(1/n) \mathbb{E}_{h\mu} [ f_n / h |\mathcal{I}]}{\lim_{n \to \infty}(1/n) \mathbb{E}_{h\mu} [ g_n / h |\mathcal{I}]} \end{align*} $$
for any positive
![]() $\mu $
-integrable function h (where
$\mu $
-integrable function h (where
![]() $\mathcal {I}$
is the invariant
$\mathcal {I}$
is the invariant
![]() $\sigma $
-algebra). In our case, we may take
$\sigma $
-algebra). In our case, we may take
![]() $h=1$
, since X is a probability space, and noting that
$h=1$
, since X is a probability space, and noting that
![]() $g_n(x) = \sum _{i=0}^{n-1}\omega _{i}(x)$
is a superadditive sequence of non-negative functions, the result follows.
$g_n(x) = \sum _{i=0}^{n-1}\omega _{i}(x)$
is a superadditive sequence of non-negative functions, the result follows.
We would like to thank the referee for pointing out this elegant proof of the theorem. Silva and Thieullen’s proof of their Theorem 3.4 uses a maximal function argument: their Lemma 2.4 extends the maximal ergodic theorem to subadditive sequences. Using it, we obtain the following maximal function estimate for non-singular actions.
Theorem 5. Suppose that
![]() $(f_{n})$
is a subadditive sequence of functions satisfying the integrability condition
$(f_{n})$
is a subadditive sequence of functions satisfying the integrability condition
![]() $f_{1}^{+}\in L^{1}$
, and that
$f_{1}^{+}\in L^{1}$
, and that
![]() $p\geq 1$
an integer. Define
$p\geq 1$
an integer. Define
and
Suppose further that for all integers
![]() $n\geq p$
and for almost every
$n\geq p$
and for almost every
![]() $x\in X$
we have
$x\in X$
we have
 $$ \begin{align*}f_{n}\leq \sum^{n-1}_{i=0}f_{1}\circ T^{i}\chi_{A}\circ T^{i}\omega_{i}+\sum^{n-1}_{i=n-p}\|f_{1}\circ T^{i}\|\omega_{i}\end{align*} $$
$$ \begin{align*}f_{n}\leq \sum^{n-1}_{i=0}f_{1}\circ T^{i}\chi_{A}\circ T^{i}\omega_{i}+\sum^{n-1}_{i=n-p}\|f_{1}\circ T^{i}\|\omega_{i}\end{align*} $$
and
 $$ \begin{align*}f_{n}^{+}\leq \sum^{n-1}_{i=0}f_{1}\circ T^{i}\chi_{B}\circ T^{i}\omega_{i}+\sum^{n-1}_{i=n-p}\|f_{1}\circ T^{i}\|\omega_{i}.\end{align*} $$
$$ \begin{align*}f_{n}^{+}\leq \sum^{n-1}_{i=0}f_{1}\circ T^{i}\chi_{B}\circ T^{i}\omega_{i}+\sum^{n-1}_{i=n-p}\|f_{1}\circ T^{i}\|\omega_{i}.\end{align*} $$
Then
 $$ \begin{align*}\inf_{n\geq 1}\bigg(\frac{1}{n}\bigg)\int f_{n} \,d{\kern-1pt}\mu=\lim_{n\to\infty}\bigg(\frac{1}{n}\bigg)\int f_{n}\,d{\kern-1pt}\mu\leq\int_{A}f_{1}\,d{\kern-1pt}\mu,\end{align*} $$
$$ \begin{align*}\inf_{n\geq 1}\bigg(\frac{1}{n}\bigg)\int f_{n} \,d{\kern-1pt}\mu=\lim_{n\to\infty}\bigg(\frac{1}{n}\bigg)\int f_{n}\,d{\kern-1pt}\mu\leq\int_{A}f_{1}\,d{\kern-1pt}\mu,\end{align*} $$
 $$ \begin{align*}0\leq \lim_{n\to\infty}\bigg(\frac{1}{n}\bigg)\int f_{n}^{+}\,d{\kern-1pt}\mu\leq\int_{B}f_{1}\,d{\kern-1pt}\mu .\end{align*} $$
$$ \begin{align*}0\leq \lim_{n\to\infty}\bigg(\frac{1}{n}\bigg)\int f_{n}^{+}\,d{\kern-1pt}\mu\leq\int_{B}f_{1}\,d{\kern-1pt}\mu .\end{align*} $$
Many proofs of the Kingman theorem deduce it from a maximal inequality; see [Reference Jones8, Reference Katznelson and Weiss9]. There are other types of proofs [Reference Avila and Bochi3, Reference Steel15] which do not rely on a maximal inequality. One can also follow these approaches, replacing the quantity
![]() $1/n$
with
$1/n$
with
![]() ${1}/{\Omega _n(x)}$
, where
${1}/{\Omega _n(x)}$
, where
![]() $\Omega _n(x)=\sum _{i=0}^{n-1}\omega _{i}(x)$
, to find an alternative proof of Theorem 4.
$\Omega _n(x)=\sum _{i=0}^{n-1}\omega _{i}(x)$
, to find an alternative proof of Theorem 4.
Note that in the measure-preserving case, we have
![]() $\Omega _n(x) = n$
, which gives us back the standard Kingman theorem, Theorem 1: our theorem shows how to replace the quantity
$\Omega _n(x) = n$
, which gives us back the standard Kingman theorem, Theorem 1: our theorem shows how to replace the quantity
![]() $1/n$
with
$1/n$
with
![]() ${1}/{\Omega _n(x)}$
, which is the key to proving the multiplicative ergodic theorem below.
${1}/{\Omega _n(x)}$
, which is the key to proving the multiplicative ergodic theorem below.
In the case where the measure
![]() $\mu $
is non-singular and has critical dimension
$\mu $
is non-singular and has critical dimension
![]() $\alpha \in [0,1]$
(see [Reference Dooley and Mortiss5]), we have that
$\alpha \in [0,1]$
(see [Reference Dooley and Mortiss5]), we have that
![]() ${\Omega _n(x)}/{n^\alpha }$
is non-zero a.e., and the conclusion of Theorem 6 is equivalent to
${\Omega _n(x)}/{n^\alpha }$
is non-zero a.e., and the conclusion of Theorem 6 is equivalent to
![]() $\lim _{n\to \infty } ({f_{n}(x)}/{n^\alpha })=f(x)< \infty $
.
$\lim _{n\to \infty } ({f_{n}(x)}/{n^\alpha })=f(x)< \infty $
.
4 The multiplicative ergodic theorem
We now introduce the notion of cocycles with values in
![]() $GL(d)$
of a non-singular transformation T of
$GL(d)$
of a non-singular transformation T of
![]() $(X, \mathcal {B}, \mu )$
; see [Reference Schmidt13]. A cocycle with respect to the action of T is a function
$(X, \mathcal {B}, \mu )$
; see [Reference Schmidt13]. A cocycle with respect to the action of T is a function
![]() $\Phi : {\mathbb {N}} \times X \to GL(d)$
satisfying
$\Phi : {\mathbb {N}} \times X \to GL(d)$
satisfying
![]() $\Phi (n+m,x)=\Phi (n,x)\Phi (m,T^{n-1}x) $
.
$\Phi (n+m,x)=\Phi (n,x)\Phi (m,T^{n-1}x) $
.
Cocycles can be generated by choosing a (random)
![]() $d\times d$
matrix,
$d\times d$
matrix,
![]() $A(x)$
, for each
$A(x)$
, for each
![]() $x\in X$
, and defining
$x\in X$
, and defining
It is easy to see that this formula defines a cocycle. We will say that
![]() $A(x)$
is the generator of
$A(x)$
is the generator of
![]() $\Phi $
.
$\Phi $
.
The operator norm of a square matrix A of dimension d is defined as
 $$ \begin{align*}\|A\|=\sup\bigg \{\frac{\|Av\|}{\|v\|}:v\in {\mathbb{R}}^{d}\backslash\{0\} \bigg\}.\end{align*} $$
$$ \begin{align*}\|A\|=\sup\bigg \{\frac{\|Av\|}{\|v\|}:v\in {\mathbb{R}}^{d}\backslash\{0\} \bigg\}.\end{align*} $$
It follows directly from the definition that the norm of the product of two matrices is less than or equal to the product of the norms of those matrices. Thus
If T is measure-preserving, the Furstenberg–Kesten theorem is an application of the Kingman subadditive ergodic theorem, applied to the subadditive sequence:
In the non-singular case, we define a (non-singular) subadditive sequence by
 $$ \begin{align*}\log\|\Phi(n,x)\|\leq \sum_{i=0}^{n-1} \omega_{i} \log \|A(T^{i}x)\|.\end{align*} $$
$$ \begin{align*}\log\|\Phi(n,x)\|\leq \sum_{i=0}^{n-1} \omega_{i} \log \|A(T^{i}x)\|.\end{align*} $$
We define singular values and exterior powers before we introduce the theorem.
Definition 2. (Exterior power)
Let V be a vector space with dimension r. For
![]() $1<k<r$
, the k-fold exterior power of V is
$1<k<r$
, the k-fold exterior power of V is
![]() $\wedge ^{k}V$
, which is the vector space of alternating k-linear forms on the dual space. The k-fold exterior power of a matrix A is
$\wedge ^{k}V$
, which is the vector space of alternating k-linear forms on the dual space. The k-fold exterior power of a matrix A is
![]() $\wedge ^{k}A$
, which has the following properties:
$\wedge ^{k}A$
, which has the following properties:
-
(i)
 $ (AB)^{\wedge k}=A^{\wedge k}B^{\wedge k}$
;
$ (AB)^{\wedge k}=A^{\wedge k}B^{\wedge k}$
; -
(ii)
 $(A^{\wedge k})^{-1}=(A^{-1})^{\wedge k}$
;
$(A^{\wedge k})^{-1}=(A^{-1})^{\wedge k}$
; -
(iii)
 $(cA)^{\wedge k}=c^{\wedge k }A^{\wedge k}$
, where
$(cA)^{\wedge k}=c^{\wedge k }A^{\wedge k}$
, where
 $c \in {\mathbb {R}}$
.
$c \in {\mathbb {R}}$
.
The singular valued decomposition of exterior powers is
![]() $\wedge ^{k}A= \wedge ^{k}V\wedge ^{k}D\wedge ^{k}U$
, where
$\wedge ^{k}A= \wedge ^{k}V\wedge ^{k}D\wedge ^{k}U$
, where
![]() $\wedge ^{k}D$
is a diagonal matrix with entries
$\wedge ^{k}D$
is a diagonal matrix with entries
![]() $\{\delta _{i_{1}} \delta _{i_{2}} \cdots \delta _{i_{k}} ,1\leq i_{1}\leq \cdots \leq i_{k} \leq r\}$
. The largest singular value is
$\{\delta _{i_{1}} \delta _{i_{2}} \cdots \delta _{i_{k}} ,1\leq i_{1}\leq \cdots \leq i_{k} \leq r\}$
. The largest singular value is
![]() $\delta _{r-k+1} \cdots \delta _{r}$
, and the smallest value is
$\delta _{r-k+1} \cdots \delta _{r}$
, and the smallest value is
![]() $\delta _{1} \cdots \delta _{k}$
. The norm of
$\delta _{1} \cdots \delta _{k}$
. The norm of
![]() $\wedge ^{k}A $
is the largest singular value.
$\wedge ^{k}A $
is the largest singular value.
Theorem 6. (Non-singular Furstenberg–Kesten theorem)
Let
![]() $\Phi $
be a linear cocycle with one side in discrete time over the non-singular dynamical system
$\Phi $
be a linear cocycle with one side in discrete time over the non-singular dynamical system
![]() $(\Omega ,\mathcal {F},\mu ,T)$
. Assume that the generator
$(\Omega ,\mathcal {F},\mu ,T)$
. Assume that the generator
![]() $A:X \to Gl(d,{\mathbb {R}})$
of
$A:X \to Gl(d,{\mathbb {R}})$
of
![]() $\Phi $
satisfies
$\Phi $
satisfies
Then the following statements hold.
![]() $(1)$
For each
$(1)$
For each
![]() $k=1,\ldots ,d$
, the sequence
$k=1,\ldots ,d$
, the sequence
is subadditive and
![]() $f_{1}^{k+}\in L^{1}(X,\mathcal {F},\mu )$
. That is,
$f_{1}^{k+}\in L^{1}(X,\mathcal {F},\mu )$
. That is,
![]() $(2)$
There is an invariant set
$(2)$
There is an invariant set
![]() $\bar {\Omega }$
of full measure and measurable functions
$\bar {\Omega }$
of full measure and measurable functions
![]() $\gamma ^{k}:X \to {\mathbb {R}}$
with
$\gamma ^{k}:X \to {\mathbb {R}}$
with
![]() $\gamma ^{k+}\in L^{1}(X,\mathcal {F},\mu )$
:
$\gamma ^{k+}\in L^{1}(X,\mathcal {F},\mu )$
:
 $$ \begin{align*}\gamma^{k}(x)=\lim_{n\to\infty} \frac{\log\lVert\land^{k}\Phi(n,x)\rVert}{\sum_{i=0}^{n-1}\omega_{i}(x)}\end{align*} $$
$$ \begin{align*}\gamma^{k}(x)=\lim_{n\to\infty} \frac{\log\lVert\land^{k}\Phi(n,x)\rVert}{\sum_{i=0}^{n-1}\omega_{i}(x)}\end{align*} $$
and
Let
![]() $\Lambda _{k}$
be the function defined by
$\Lambda _{k}$
be the function defined by
![]() $\Lambda _{k}=\gamma ^{k+1}-\gamma ^{k},$
and let
$\Lambda _{k}=\gamma ^{k+1}-\gamma ^{k},$
and let
![]() $\delta _k$
be the corresponding singular value of
$\delta _k$
be the corresponding singular value of
![]() $\Phi (n,x)$
. Then
$\Phi (n,x)$
. Then
 $$ \begin{align*}\Lambda_{k}=\lim_{n\to\infty}\frac{\log \delta_{k}(\Phi(n,x))}{\sum_{i=0}^{n-1}\omega_{i}(x)}.\end{align*} $$
$$ \begin{align*}\Lambda_{k}=\lim_{n\to\infty}\frac{\log \delta_{k}(\Phi(n,x))}{\sum_{i=0}^{n-1}\omega_{i}(x)}.\end{align*} $$
Proof. Note that
![]() $A(x)=\Phi (1,x)$
. For all k,
$A(x)=\Phi (1,x)$
. For all k,
is a subadditive sequence, and
Hence subadditivity of
![]() $ f_{n}^{k}(x)$
follows. By Theorem 4, we have
$ f_{n}^{k}(x)$
follows. By Theorem 4, we have
 $$ \begin{align*}\gamma^{k}(x)=\lim_{n\to\infty} \frac{\log\lVert\land^{k}\Phi(n,x)\rVert}{\sum_{i=0}^{n-1}\omega_{i}(x)}.\end{align*} $$
$$ \begin{align*}\gamma^{k}(x)=\lim_{n\to\infty} \frac{\log\lVert\land^{k}\Phi(n,x)\rVert}{\sum_{i=0}^{n-1}\omega_{i}(x)}.\end{align*} $$
Since
![]() $\lVert \land ^{k+l}\Phi (n,x)\rVert \leq \lVert \land ^{k}\Phi (n,x)\rVert \lVert \land ^{l}\Phi (n,x)\rVert $
,
$\lVert \land ^{k+l}\Phi (n,x)\rVert \leq \lVert \land ^{k}\Phi (n,x)\rVert \lVert \land ^{l}\Phi (n,x)\rVert $
,
![]() $\gamma ^{k}$
is a subadditive sequence. For
$\gamma ^{k}$
is a subadditive sequence. For
![]() $k=1,\ldots ,d$
,
$k=1,\ldots ,d$
,
 $$ \begin{align*}\log\lVert\land^{k}\Phi(n,x)\rVert=\sum_{i=1}^{k}\log\delta_{i}(\Phi (n,x))\end{align*} $$
$$ \begin{align*}\log\lVert\land^{k}\Phi(n,x)\rVert=\sum_{i=1}^{k}\log\delta_{i}(\Phi (n,x))\end{align*} $$
where
![]() $\delta _{i}$
is the corresponding singular value of
$\delta _{i}$
is the corresponding singular value of
![]() $\Phi (n,x)$
.
$\Phi (n,x)$
.
We now consider the behaviour of
![]() $ \|\Phi (n,x)v\|$
for
$ \|\Phi (n,x)v\|$
for
![]() $v \in {\mathbb {R}}^{d}$
as
$v \in {\mathbb {R}}^{d}$
as
![]() $n\to \infty $
. If
$n\to \infty $
. If
![]() $A \in M_d({\mathbb {R}})$
with transpose
$A \in M_d({\mathbb {R}})$
with transpose
![]() $A^*$
, both
$A^*$
, both
![]() $A^{*}A$
and
$A^{*}A$
and
![]() $A A^{*}$
are symmetric and positive semidefinite. Any positive semidefinite and symmetric matrix S may be written in the form
$A A^{*}$
are symmetric and positive semidefinite. Any positive semidefinite and symmetric matrix S may be written in the form
where D is diagonal with non-negative entries in non-deceasing order and C is orthogonal.
The polar decomposition of a matrix A is
where
![]() $C^{\prime },C^{\prime \prime }$
are orthogonal matrices. Applying the polar decomposition to
$C^{\prime },C^{\prime \prime }$
are orthogonal matrices. Applying the polar decomposition to
![]() $\Phi (n,x)$
in the theorem, we obtain
$\Phi (n,x)$
in the theorem, we obtain
for some orthogonal matrix
![]() $C^{\prime \prime }_{n}$
. Since orthogonal matrices are isometries, we have
$C^{\prime \prime }_{n}$
. Since orthogonal matrices are isometries, we have
![]() $\|C^{\prime \prime }_{n} v\|=\|v\|$
. Thus
$\|C^{\prime \prime }_{n} v\|=\|v\|$
. Thus
Returning to the symmetric matrix
![]() $\Phi (n,x)^{*}\Phi (n,x)$
, we know that
$\Phi (n,x)^{*}\Phi (n,x)$
, we know that
![]() $\Phi (n,x)^{*}\Phi (n,x)=C_{n}^{*}D_{n}C_{n}$
, and
$\Phi (n,x)^{*}\Phi (n,x)=C_{n}^{*}D_{n}C_{n}$
, and
![]() $\Phi (n,x)=L_{n}(D_{n})^{1/2}C_{n}$
, and hence
$\Phi (n,x)=L_{n}(D_{n})^{1/2}C_{n}$
, and hence
If the limit exists, then there are an orthogonal matrix
![]() $C=\lim C_{n}$
and a diagonal matrix
$C=\lim C_{n}$
and a diagonal matrix
![]() $D=\lim D_{n}^{{1}/{2n}}$
. By Theorem 5, we see that
$D=\lim D_{n}^{{1}/{2n}}$
. By Theorem 5, we see that
![]() $\lim _{n\to \infty } ({\log \lVert \land ^{k}\Phi (n,x)\rVert }/{\sum _{i=0}^{n-1}\omega _{i}(x)})$
converges for all k to
$\lim _{n\to \infty } ({\log \lVert \land ^{k}\Phi (n,x)\rVert }/{\sum _{i=0}^{n-1}\omega _{i}(x)})$
converges for all k to
![]() $-\infty $
or a finite limit. Hence
$-\infty $
or a finite limit. Hence
![]() $\lim _{n\to \infty }({ \log \delta _{i} (\Phi (n,x))}/{\sum _{i=0}^{n-1}\omega _{i}(x)})$
converges to a finite limit for every i. Now we can assume that
$\lim _{n\to \infty }({ \log \delta _{i} (\Phi (n,x))}/{\sum _{i=0}^{n-1}\omega _{i}(x)})$
converges to a finite limit for every i. Now we can assume that
![]() $D_{n}^{{1}/{2n}} $
converges as
$D_{n}^{{1}/{2n}} $
converges as
![]() $n \to \infty $
.
$n \to \infty $
.
By the monotonicity of
![]() $\Lambda ^{i}$
,
$\Lambda ^{i}$
,
There is a unique partition I, given by
such that
![]() $ \Lambda ^{i_{q}}= \Lambda ^{i_{q+1}-1} < \Lambda ^{i_{q+1}}$
. This partition splits
$ \Lambda ^{i_{q}}= \Lambda ^{i_{q+1}-1} < \Lambda ^{i_{q+1}}$
. This partition splits
![]() $\{1,2,\ldots ,r\}$
into finitely many intervals
$\{1,2,\ldots ,r\}$
into finitely many intervals
![]() $[i_{q},i_{q+1}-1]$
. If
$[i_{q},i_{q+1}-1]$
. If
![]() $ \Lambda ^{i}= \Lambda ^{j}$
, then they belong to same interval. Let
$ \Lambda ^{i}= \Lambda ^{j}$
, then they belong to same interval. Let
![]() $\Sigma (I,q)(x)$
be a vector subspace of
$\Sigma (I,q)(x)$
be a vector subspace of
![]() ${\mathbb {R}}^{r}$
; it is a union of the zero vector 0 and the set of all eigenvectors corresponding to eigenvalues smaller than or equal to
${\mathbb {R}}^{r}$
; it is a union of the zero vector 0 and the set of all eigenvectors corresponding to eigenvalues smaller than or equal to
![]() $\Lambda ^{i_{q+1}-1}$
. It is easily seen that
$\Lambda ^{i_{q+1}-1}$
. It is easily seen that
![]() $\Sigma (I,0)(x)$
is
$\Sigma (I,0)(x)$
is
![]() $\{0\}$
and
$\{0\}$
and
![]() $\Sigma (I,p)(x)$
is
$\Sigma (I,p)(x)$
is
![]() ${\mathbb {R}}^{r}$
. We see that
${\mathbb {R}}^{r}$
. We see that
![]() $C_{n}^{-1}e_{i}$
is an eigenvector of
$C_{n}^{-1}e_{i}$
is an eigenvector of
![]() $(\Phi (n,x)^{*}\Phi (n,x))^{1/2}$
with eigenvalue
$(\Phi (n,x)^{*}\Phi (n,x))^{1/2}$
with eigenvalue
![]() $\delta _{i}^{2}$
. We know that
$\delta _{i}^{2}$
. We know that
![]() $D_{n}^{{1}/{2n}}$
converges, but the question is whether the vector space spanned by
$D_{n}^{{1}/{2n}}$
converges, but the question is whether the vector space spanned by
![]() $C_{n}^{-1}e_{i}$
converges. We will formulate a one-sided multiplicative ergodic theorem which is based on Raghunathan’s version [Reference Raghunathan12].
$C_{n}^{-1}e_{i}$
converges. We will formulate a one-sided multiplicative ergodic theorem which is based on Raghunathan’s version [Reference Raghunathan12].
Theorem 7. (Non-singular Oseledeč theorem)
Let (
![]() $X,S,m$
) be a probability space. Suppose that T is a non-singular transformation and
$X,S,m$
) be a probability space. Suppose that T is a non-singular transformation and
![]() $u:Z\times X\to M(r,{\mathbb {R}})$
is a measurable cocycle over T such that
$u:Z\times X\to M(r,{\mathbb {R}})$
is a measurable cocycle over T such that
![]() $\log ^{+}\|\Phi (1, \dot )\| \in L^{1}(X,S,m)$
. We set
$\log ^{+}\|\Phi (1, \dot )\| \in L^{1}(X,S,m)$
. We set
 $$ \begin{align*}B=\bigg\{(x,v)\in X \times {\mathbb{R}}^{r}: \frac{\log \|\Phi(n,x)v\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}\,\, \mathrm{tends\ to\ a \ finite \ limit \ or\ }-\infty\bigg\}\end{align*} $$
$$ \begin{align*}B=\bigg\{(x,v)\in X \times {\mathbb{R}}^{r}: \frac{\log \|\Phi(n,x)v\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}\,\, \mathrm{tends\ to\ a \ finite \ limit \ or\ }-\infty\bigg\}\end{align*} $$
and
Then there is a subset Y of
![]() $X^{\prime }$
which has full measure and a sequence of functions
$X^{\prime }$
which has full measure and a sequence of functions
![]() $ \Lambda ^{1}(x) \leq \cdots \leq \Lambda ^{r}(x)$
(taking values in
$ \Lambda ^{1}(x) \leq \cdots \leq \Lambda ^{r}(x)$
(taking values in
![]() ${\mathbb {R}}\cup -\infty $
) such that the following statements hold.
${\mathbb {R}}\cup -\infty $
) such that the following statements hold.
-
(i) Let
 $I=\{1=i_{1}<i_{2}<\cdots <i_{p}<i_{p+1}=r+1\}$
be
$I=\{1=i_{1}<i_{2}<\cdots <i_{p}<i_{p+1}=r+1\}$
be
 $n+1$
tuples of integers. Define
$n+1$
tuples of integers. Define
 $Y(I)=\{x\in X^{\prime }|\Lambda ^{i}(x)=\Lambda ^{j}(x)$
for
$Y(I)=\{x\in X^{\prime }|\Lambda ^{i}(x)=\Lambda ^{j}(x)$
for
 $ i_{q}\leq i,j<i_{q+1}$
and
$ i_{q}\leq i,j<i_{q+1}$
and
 $\Lambda ^{i_{q}}(x)<\Lambda ^{i_{q+1}}(x)$
for all q with
$\Lambda ^{i_{q}}(x)<\Lambda ^{i_{q+1}}(x)$
for all q with
 $1<q<p\}$
. Then for all
$1<q<p\}$
. Then for all
 $x\in Y(I),1<q<p$
, is a vector subspace of
$x\in Y(I),1<q<p$
, is a vector subspace of $$ \begin{align*} \Sigma(I,q)(x)=\bigg\{v\in {\mathbb{R}}^{r}| \lim_{n\to \infty}\frac{\log \|\Phi(n,x)v\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}\leq \Lambda^{i_q}(x)\bigg\} \end{align*} $$
$$ \begin{align*} \Sigma(I,q)(x)=\bigg\{v\in {\mathbb{R}}^{r}| \lim_{n\to \infty}\frac{\log \|\Phi(n,x)v\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}\leq \Lambda^{i_q}(x)\bigg\} \end{align*} $$
 ${\mathbb {R}}^{r}$
with dimension
${\mathbb {R}}^{r}$
with dimension
 $i^{q+1}-1$
.
$i^{q+1}-1$
.
-
(ii) If
 $\Sigma (I,0)=\{0\}$
then for any vector
$\Sigma (I,0)=\{0\}$
then for any vector $$ \begin{align*} \lim_{n\to \infty}\frac{\log \|\Phi(n,x)v\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}= \Lambda^{i_q}(x) \end{align*} $$
$$ \begin{align*} \lim_{n\to \infty}\frac{\log \|\Phi(n,x)v\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}= \Lambda^{i_q}(x) \end{align*} $$
 $v \in \Sigma (I,q)(x) -\Sigma (I,q-1)(x)$
.
$v \in \Sigma (I,q)(x) -\Sigma (I,q-1)(x)$
.
-
(iii) For
 $x\in Y$
, the sequence converges to a matrix
$x\in Y$
, the sequence converges to a matrix $$ \begin{align*} A(n,x)=(\Phi(n,x)^{*}\Phi(n,x))^{{1}/{2n}} \end{align*} $$
$$ \begin{align*} A(n,x)=(\Phi(n,x)^{*}\Phi(n,x))^{{1}/{2n}} \end{align*} $$
 $A(x) \in M(r,{\mathbb {R}})$
. The eigenspace of
$A(x) \in M(r,{\mathbb {R}})$
. The eigenspace of
 $A(x)$
is the orthogonal complement of
$A(x)$
is the orthogonal complement of
 $\Sigma (I,q)(x)$
in
$\Sigma (I,q)(x)$
in
 $\Sigma (I,q+1)(x)$
corresponding to the eigenvalue
$\Sigma (I,q+1)(x)$
corresponding to the eigenvalue
 $\exp \Lambda ^{i_{q+1}}$
.
$\exp \Lambda ^{i_{q+1}}$
.
Lemma 2. Suppose that
![]() $ \log ^{+}\| \Phi (1,\dot )\|$
is a measurable function and T a non-singular transformation. There is a set
$ \log ^{+}\| \Phi (1,\dot )\|$
is a measurable function and T a non-singular transformation. There is a set
![]() $Y\subset X$
of full measure such that for every
$Y\subset X$
of full measure such that for every
![]() $x\in Y$
, the following statements hold.
$x\in Y$
, the following statements hold.
-
(i) The sequence
 $S_{n}={\sum _{0\leq q<n}\log ^{+}\| \Phi (1,T^{q}(x))\|}/{\sum _{i=0}^{n-1}\omega _{i}(x)}$
converges to a limit a.e.
$S_{n}={\sum _{0\leq q<n}\log ^{+}\| \Phi (1,T^{q}(x))\|}/{\sum _{i=0}^{n-1}\omega _{i}(x)}$
converges to a limit a.e. -
(ii)
 $\lim _{n\to \infty }\| \Phi (1,T^{n}(x))\|=0.$
$\lim _{n\to \infty }\| \Phi (1,T^{n}(x))\|=0.$
Proof.
-
(i) This follows directly from the Hurewicz ergodic theorem.
-
(ii) By (i), the sequence
 $S_{n}$
converges to a limit, Since
$S_{n}$
converges to a limit, Since $$ \begin{align*} S_{n}&=\frac{\sum_{0\leq q<n}\log ^{+}\| \Phi(1,T^{q}(x))\|}{\sum_{i=0}^{n-1}\omega_{i}(x)},\\[3pt] S_{n-1}&=\frac{\sum_{0\leq q<n-1}\log ^{+}\| \Phi(1,T^{q}(x))\|}{\sum_{i=0}^{n-2}\omega_{i}(x)},\\[3pt] S_{n}&=\frac{\sum_{i=0}^{n-2}\omega_{i}(x)}{\sum_{i=0}^{n-1}\omega_{i}(x)\sum_{i=0}^{n-2}\omega_{i}(x)}\sum_{0\leq q<n-1}\log ^{+}\| \Phi(1,T^{q}(x))\| \\[3pt] &\quad +\frac{\log ^{+}\| \Phi(1,T^{n}(x))\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}. \end{align*} $$
$$ \begin{align*} S_{n}&=\frac{\sum_{0\leq q<n}\log ^{+}\| \Phi(1,T^{q}(x))\|}{\sum_{i=0}^{n-1}\omega_{i}(x)},\\[3pt] S_{n-1}&=\frac{\sum_{0\leq q<n-1}\log ^{+}\| \Phi(1,T^{q}(x))\|}{\sum_{i=0}^{n-2}\omega_{i}(x)},\\[3pt] S_{n}&=\frac{\sum_{i=0}^{n-2}\omega_{i}(x)}{\sum_{i=0}^{n-1}\omega_{i}(x)\sum_{i=0}^{n-2}\omega_{i}(x)}\sum_{0\leq q<n-1}\log ^{+}\| \Phi(1,T^{q}(x))\| \\[3pt] &\quad +\frac{\log ^{+}\| \Phi(1,T^{n}(x))\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}. \end{align*} $$
 $n\to \infty $
and
$n\to \infty $
and
 $|S_{n}-S_{n-1}|\to 0$
, we can conclude that for all
$|S_{n}-S_{n-1}|\to 0$
, we can conclude that for all $$ \begin{align*}\frac{\log ^{+}\| \Phi(1,T^{n}(x))\|}{\sum_{i=0}^{n-1}\omega_{i}(x)} \to 0\end{align*} $$
$$ \begin{align*}\frac{\log ^{+}\| \Phi(1,T^{n}(x))\|}{\sum_{i=0}^{n-1}\omega_{i}(x)} \to 0\end{align*} $$
 $x\in Y$
.
$x\in Y$
.
Now, given
![]() $\varepsilon>0$
, there exists
$\varepsilon>0$
, there exists
![]() $N(\varepsilon ,x)$
such that for all
$N(\varepsilon ,x)$
such that for all
![]() $n>N$
,
$n>N$
,
 $$ \begin{align*} \| \Phi(1,T^{n}(x))\|< \exp \sum_{i=0}^{n-1}\omega_{i}(x)\varepsilon. \end{align*} $$
$$ \begin{align*} \| \Phi(1,T^{n}(x))\|< \exp \sum_{i=0}^{n-1}\omega_{i}(x)\varepsilon. \end{align*} $$
![]() $\| \Phi (n,x)\|$
satisfies the cocycle identity:
$\| \Phi (n,x)\|$
satisfies the cocycle identity:
![]() $\Phi (n+1,x)= \Phi (1,T^{n}(x)) \Phi (n,x)$
.
$\Phi (n+1,x)= \Phi (1,T^{n}(x)) \Phi (n,x)$
.
For a unit vector
![]() $v \in \Sigma (I,q,n)(x)$
,
$v \in \Sigma (I,q,n)(x)$
,
 $$ \begin{align*}\|\Phi(n+1,x)v\|\leq \exp\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)(\Lambda ^{i_{q}}+2\varepsilon)\bigg).\end{align*} $$
$$ \begin{align*}\|\Phi(n+1,x)v\|\leq \exp\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)(\Lambda ^{i_{q}}+2\varepsilon)\bigg).\end{align*} $$
Choose a unit vector
![]() $v \in \Sigma (I,q,n)(x)$
, and let
$v \in \Sigma (I,q,n)(x)$
, and let
![]() $v^{\prime } \in \Sigma (I,q,n+1)(x)$
be the orthogonal projection of v on
$v^{\prime } \in \Sigma (I,q,n+1)(x)$
be the orthogonal projection of v on
![]() $\Sigma (I,q,n+1)(x)$
. The orthogonal complement of
$\Sigma (I,q,n+1)(x)$
. The orthogonal complement of
![]() $v^{\prime }$
in
$v^{\prime }$
in
![]() $\Sigma (I,q,n+1)(x)$
has the form
$\Sigma (I,q,n+1)(x)$
has the form
![]() $C_{n+1}^{-1}\sum _{i\geq _{q+1}-1}b_{i}e_{i}$
.
$C_{n+1}^{-1}\sum _{i\geq _{q+1}-1}b_{i}e_{i}$
.
Lemma 3. Given
![]() $\varepsilon>0$
, there exists
$\varepsilon>0$
, there exists
![]() $N(\varepsilon ,x), x\in Y$
, with the following property. There is a unit vector
$N(\varepsilon ,x), x\in Y$
, with the following property. There is a unit vector
![]() $v \in \Sigma (I,q,n)(x)$
, for some number
$v \in \Sigma (I,q,n)(x)$
, for some number
![]() $b_{i}\in {\mathbb {R}}$
and
$b_{i}\in {\mathbb {R}}$
and
![]() $v^{\prime } \in \Sigma (I,q,n+1)(x)$
, such that
$v^{\prime } \in \Sigma (I,q,n+1)(x)$
, such that
 $$ \begin{align*} v=v^{\prime}+C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}. \end{align*} $$
$$ \begin{align*} v=v^{\prime}+C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}. \end{align*} $$
Then
![]() $|b_{i}|<$
$|b_{i}|<$
![]() $\exp \{-\sum _{i=0}^{n-1}\omega _{i}(x)(\Lambda ^{i}-\Lambda ^{i_{q}}-\varepsilon )\}$
for
$\exp \{-\sum _{i=0}^{n-1}\omega _{i}(x)(\Lambda ^{i}-\Lambda ^{i_{q}}-\varepsilon )\}$
for
![]() $n\geq N$
.
$n\geq N$
.
Proof. We have
![]() $\|\Phi (n+1,x)v\|\leq \exp \sum _{i=0}^{n-1}\omega _{i}(x)(\Lambda ^{i_{q}}+2\varepsilon )$
by Lemma 2. Notice that
$\|\Phi (n+1,x)v\|\leq \exp \sum _{i=0}^{n-1}\omega _{i}(x)(\Lambda ^{i_{q}}+2\varepsilon )$
by Lemma 2. Notice that
 $$ \begin{align*} \|\Phi(n+1,x)v\|\geq \bigg\|\sum_{i\geq i_{q+1}}|b_{i}|\Phi(n+1,x)C_{n+1}^{-1}e_{i}\bigg\|. \end{align*} $$
$$ \begin{align*} \|\Phi(n+1,x)v\|\geq \bigg\|\sum_{i\geq i_{q+1}}|b_{i}|\Phi(n+1,x)C_{n+1}^{-1}e_{i}\bigg\|. \end{align*} $$
Now
![]() $\|b_{i}\Phi (n+1,x)C_{n+1}^{-1}e_{i}\|\leq \|\sum _{i\geq i_{q+1}}b_{i}\Phi (n+1,x)C_{n+1}^{-1}e_{i}\|$
as
$\|b_{i}\Phi (n+1,x)C_{n+1}^{-1}e_{i}\|\leq \|\sum _{i\geq i_{q+1}}b_{i}\Phi (n+1,x)C_{n+1}^{-1}e_{i}\|$
as
![]() $\Lambda ^{i}$
is non-decreasing. Hence
$\Lambda ^{i}$
is non-decreasing. Hence
Now
![]() $\|\Phi (n+1,x)C_{n+1}^{-1}e_{i}\|$
is the ith eigenvalue, since
$\|\Phi (n+1,x)C_{n+1}^{-1}e_{i}\|$
is the ith eigenvalue, since
Let
![]() $\Lambda ^{i}$
be the limit of
$\Lambda ^{i}$
be the limit of
![]() ${\log \delta _{i} (\Phi _{n+1}(x))}/{\sum _{i=0}^{n}\omega _{i}(x)}$
. It follows from the above that
${\log \delta _{i} (\Phi _{n+1}(x))}/{\sum _{i=0}^{n}\omega _{i}(x)}$
. It follows from the above that
 $$ \begin{align*} \|\Phi(n+1,x)v\|\geq|b_{i}| \exp\bigg(\bigg(\sum_{i=0}^{n}\omega_{i}(x)\bigg)(\Lambda^{i}-\varepsilon)\bigg). \end{align*} $$
$$ \begin{align*} \|\Phi(n+1,x)v\|\geq|b_{i}| \exp\bigg(\bigg(\sum_{i=0}^{n}\omega_{i}(x)\bigg)(\Lambda^{i}-\varepsilon)\bigg). \end{align*} $$
Thus
![]() $\Lambda ^{i}$
is in a bounded interval which is greater than
$\Lambda ^{i}$
is in a bounded interval which is greater than
![]() $\Lambda ^{i_{q}}$
,
$\Lambda ^{i_{q}}$
,
![]() $0<\varepsilon <1$
. For large n, we may assume
$0<\varepsilon <1$
. For large n, we may assume
 $$ \begin{align*} \exp\bigg(\bigg(\sum_{i=0}^{n}\omega_{i}(x)\bigg)(\Lambda^{i}-\varepsilon)\bigg)\geq \exp\bigg(\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i}-2\varepsilon)\bigg). \end{align*} $$
$$ \begin{align*} \exp\bigg(\bigg(\sum_{i=0}^{n}\omega_{i}(x)\bigg)(\Lambda^{i}-\varepsilon)\bigg)\geq \exp\bigg(\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i}-2\varepsilon)\bigg). \end{align*} $$
Thus
 $$ \begin{align*} |b_{i}|\leq \exp -\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i}-4\varepsilon-\Lambda ^{i_{q}}) \end{align*} $$
$$ \begin{align*} |b_{i}|\leq \exp -\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i}-4\varepsilon-\Lambda ^{i_{q}}) \end{align*} $$
which completes the proof of the lemma.
Lemma 3 shows that a vector in
![]() $\Sigma (I,q,n)(x)$
can be combined with the projection on
$\Sigma (I,q,n)(x)$
can be combined with the projection on
![]() $\Sigma (I,q,n+1)(x)$
and the orthogonal complement of
$\Sigma (I,q,n+1)(x)$
and the orthogonal complement of
![]() $\Sigma (I,q,n+1)(x)$
, that is,
$\Sigma (I,q,n+1)(x)$
, that is,
 $$ \begin{align*} C_{n}^{-1}\sum_{i=1}^{i_{q+1}-1}Ke_{i}=C_{n+1}^{-1}\sum_{j=1}^{r}b_{j}e_{j} \quad\mbox{and}\quad v_{n}=v_{m+1}^{\prime}+w_{m+1}. \end{align*} $$
$$ \begin{align*} C_{n}^{-1}\sum_{i=1}^{i_{q+1}-1}Ke_{i}=C_{n+1}^{-1}\sum_{j=1}^{r}b_{j}e_{j} \quad\mbox{and}\quad v_{n}=v_{m+1}^{\prime}+w_{m+1}. \end{align*} $$
Now
![]() $v_{m+1}^{\prime }$
is the orthogonal projection of
$v_{m+1}^{\prime }$
is the orthogonal projection of
![]() $v_{n}$
onto
$v_{n}$
onto
![]() $\Sigma (I,q,n+1)(x)$
and the norm of
$\Sigma (I,q,n+1)(x)$
and the norm of
![]() $v_{m+1}^{\prime }$
is given by
$v_{m+1}^{\prime }$
is given by
 $$ \begin{align*} \|v_{m+1}^{\prime}\|=\bigg\|v_{n}-C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|. \end{align*} $$
$$ \begin{align*} \|v_{m+1}^{\prime}\|=\bigg\|v_{n}-C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|. \end{align*} $$
Since
![]() $v_{n}$
is a unit vector, we have the following upper and lower bounds for
$v_{n}$
is a unit vector, we have the following upper and lower bounds for
![]() $\|v_{m+1}^{\prime }\|$
:
$\|v_{m+1}^{\prime }\|$
:
 $$ \begin{align*} 1-\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|\leq \|v_{m+1}^{\prime}\|\leq 1+\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|. \end{align*} $$
$$ \begin{align*} 1-\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|\leq \|v_{m+1}^{\prime}\|\leq 1+\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|. \end{align*} $$
We want to show that
![]() $\|v_{n+1}-v_{n}\|\leq 2r \exp \{-(\sum _{i=0}^{n-1})\omega _{i}(x)(\Lambda ^{i_{q+1}}-\Lambda ^{i_{q}}-\varepsilon )\}$
.
$\|v_{n+1}-v_{n}\|\leq 2r \exp \{-(\sum _{i=0}^{n-1})\omega _{i}(x)(\Lambda ^{i_{q+1}}-\Lambda ^{i_{q}}-\varepsilon )\}$
.
Lemma 4. If
![]() $v_{n+1} \in \Sigma (I,q,n+1)(x)$
is a unit vector and
$v_{n+1} \in \Sigma (I,q,n+1)(x)$
is a unit vector and
![]() $v_{n} \in \Sigma (I,q,n)(x)$
satisfies the conditions of Lemma 3, then
$v_{n} \in \Sigma (I,q,n)(x)$
satisfies the conditions of Lemma 3, then
 $$ \begin{align*} \|v_{n+1}-v_{n}\|\leq 2r \exp \bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\}. \end{align*} $$
$$ \begin{align*} \|v_{n+1}-v_{n}\|\leq 2r \exp \bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\}. \end{align*} $$
Proof.
and
It follows that
 $$ \begin{align*}\|v_{n}-v_{n+1}^{\prime}\|=\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|.\end{align*} $$
$$ \begin{align*}\|v_{n}-v_{n+1}^{\prime}\|=\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|.\end{align*} $$
On the other hand, let
![]() $v_{n+1}^{\prime }=av_{n+1}$
, and we have
$v_{n+1}^{\prime }=av_{n+1}$
, and we have
![]() $1-\|C_{n+1}^{-1}\sum _{i\geq i_{q+1}}b_{i}e_{i}\|\leq \|v_{m+1}^{\prime }\|\leq 1+\|C_{n+1}^{-1}\sum _{i\geq i_{q+1}}b_{i}e_{i}\|$
. Thus
$1-\|C_{n+1}^{-1}\sum _{i\geq i_{q+1}}b_{i}e_{i}\|\leq \|v_{m+1}^{\prime }\|\leq 1+\|C_{n+1}^{-1}\sum _{i\geq i_{q+1}}b_{i}e_{i}\|$
. Thus
 $$ \begin{align*} 1-\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|\leq a\leq 1+\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|. \end{align*} $$
$$ \begin{align*} 1-\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|\leq a\leq 1+\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|. \end{align*} $$
Now
![]() $\|v_{n+1}^{\prime }-v_{n+1}\| =\|(a-1)v_{n+1}\| $
, which is smaller than
$\|v_{n+1}^{\prime }-v_{n+1}\| =\|(a-1)v_{n+1}\| $
, which is smaller than
![]() $\|C_{n+1}^{-1}\sum _{i\geq i_{q+1}}b_{i}e_{i}\|$
. We thus have
$\|C_{n+1}^{-1}\sum _{i\geq i_{q+1}}b_{i}e_{i}\|$
. We thus have
 $$ \begin{align*} \|v_{n+1}-v_{n}\|\leq 2 \bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|. \end{align*} $$
$$ \begin{align*} \|v_{n+1}-v_{n}\|\leq 2 \bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\|. \end{align*} $$
It follows that
 $$ \begin{align*}\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\| &\leq \sum_{i\geq i_{q+1}}\|b_{i}e_{i}\| \leq r|b_{i_{q+1}}| \\[3pt] &\leq r \exp \bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\}.\end{align*} $$
$$ \begin{align*}\bigg\|C_{n+1}^{-1}\sum_{i\geq i_{q+1}}b_{i}e_{i}\bigg\| &\leq \sum_{i\geq i_{q+1}}\|b_{i}e_{i}\| \leq r|b_{i_{q+1}}| \\[3pt] &\leq r \exp \bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\}.\end{align*} $$
This completes the proof.
We will show that
![]() $\|v_{n+k}-v_{n+l}\| $
is a Cauchy sequence. In fact, the sequence
$\|v_{n+k}-v_{n+l}\| $
is a Cauchy sequence. In fact, the sequence
![]() $\sum _{i=l}^{\infty } r \exp \{-(n+i)(\Lambda ^{i_{q+i}}-\Lambda ^{i_{q}}-\varepsilon )\}$
is the sum of a geometric series. Thus
$\sum _{i=l}^{\infty } r \exp \{-(n+i)(\Lambda ^{i_{q+i}}-\Lambda ^{i_{q}}-\varepsilon )\}$
is the sum of a geometric series. Thus
 $$ \begin{align*} &\|v_{n+k}-v_{n+l}\|\\[3pt] &\quad\leq \sum_{i=l}^{\infty}\|v_{n+k}-v_{n+l}\| \\[3pt] &\quad=2r \frac{1}{1-\exp \{-(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)}\exp \bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)+l\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\}\\[3pt] &\quad=C \exp\bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)+l\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\}. \end{align*} $$
$$ \begin{align*} &\|v_{n+k}-v_{n+l}\|\\[3pt] &\quad\leq \sum_{i=l}^{\infty}\|v_{n+k}-v_{n+l}\| \\[3pt] &\quad=2r \frac{1}{1-\exp \{-(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)}\exp \bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)+l\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\}\\[3pt] &\quad=C \exp\bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)+l\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\}. \end{align*} $$
This shows that
![]() $v_{n}$
is a Cauchy sequence, as claimed.
$v_{n}$
is a Cauchy sequence, as claimed.
Lemma 5. Let
![]() $\{v^{1}_{n},v^{2}_{n},\ldots ,v^{i}_{n}\}$
be a collection of vectors which is a basis for
$\{v^{1}_{n},v^{2}_{n},\ldots ,v^{i}_{n}\}$
be a collection of vectors which is a basis for
![]() $\Sigma (I,q,n)(x)$
, where
$\Sigma (I,q,n)(x)$
, where
![]() $ 0\leq i<i_{q+1}$
. The sequence
$ 0\leq i<i_{q+1}$
. The sequence
![]() $\{\Sigma (I,q,n)(x)\}$
has limit
$\{\Sigma (I,q,n)(x)\}$
has limit
![]() $\Sigma (I,q)(x)$
. Furthermore,
$\Sigma (I,q)(x)$
. Furthermore,
 $$ \begin{align*}\|v_{n+k}-v_{n+l}\| \leq \sum_{i=l}^{k}\|v_{n+k}-v_{n+l}\| \leq \sum_{i=l}^{\infty}\|v_{n+k}-v_{n+l}\|.\end{align*} $$
$$ \begin{align*}\|v_{n+k}-v_{n+l}\| \leq \sum_{i=l}^{k}\|v_{n+k}-v_{n+l}\| \leq \sum_{i=l}^{\infty}\|v_{n+k}-v_{n+l}\|.\end{align*} $$
Proof. By Lemma 4, we see easily that
 $$ \begin{align*} \|v_{n+k}^{i}-v_{n+l}^{i}\|\leq C \exp\bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)+\max(k,l)\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\} \end{align*} $$
$$ \begin{align*} \|v_{n+k}^{i}-v_{n+l}^{i}\|\leq C \exp\bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)+\max(k,l)\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\} \end{align*} $$
for
![]() $i_q \leq i < i_{q+1}$
. Moreover,
$i_q \leq i < i_{q+1}$
. Moreover,
 $$ \begin{align*} \|v_{n}^{i}-v^{i}\|\leq C \exp\bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\} \end{align*} $$
$$ \begin{align*} \|v_{n}^{i}-v^{i}\|\leq C \exp\bigg\{-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i_{q+1}}-\Lambda^{i_{q}}-\varepsilon)\bigg\} \end{align*} $$
where
![]() $v^{i}$
is the limit of
$v^{i}$
is the limit of
![]() $v_{n}^{i}$
. The sequence
$v_{n}^{i}$
. The sequence
![]() $\{v_{n}^{i}\}$
converges to a linearly independent set of vectors as
$\{v_{n}^{i}\}$
converges to a linearly independent set of vectors as
![]() $n\to \infty $
.
$n\to \infty $
.
![]() $\Sigma (I,q,n)(x)$
is the space spanned by
$\Sigma (I,q,n)(x)$
is the space spanned by
![]() $\{v^{1}_{n},v^{2}_{n},\ldots ,v^{i}_{n}\}$
. Thus
$\{v^{1}_{n},v^{2}_{n},\ldots ,v^{i}_{n}\}$
. Thus
![]() $ \Sigma (I,q,n)(x) \to \Sigma (I,q)(x)$
as
$ \Sigma (I,q,n)(x) \to \Sigma (I,q)(x)$
as
![]() $n\to \infty $
.
$n\to \infty $
.
Writing the matrix
![]() $B=C_{n}C^{-1}_{n+k}$
, a vector in the space
$B=C_{n}C^{-1}_{n+k}$
, a vector in the space
![]() $\Sigma (I,q,n)(x)$
has the form
$\Sigma (I,q,n)(x)$
has the form
![]() $C_{n}^{-1}e_{i}$
which we can split into an orthogonal projection in
$C_{n}^{-1}e_{i}$
which we can split into an orthogonal projection in
![]() $\Sigma (I,q,n+1)(x)$
and its orthogonal complement in
$\Sigma (I,q,n+1)(x)$
and its orthogonal complement in
![]() $\Sigma (I,q,n+1)(x)^{\perp }$
. By Lemma 3,
$\Sigma (I,q,n+1)(x)^{\perp }$
. By Lemma 3,
![]() $C_{n}^{-1}e_{i}=C_{n+1}^{-1}\sum _{j=1}^{r}b_{j}e_{j}$
. Hence
$C_{n}^{-1}e_{i}=C_{n+1}^{-1}\sum _{j=1}^{r}b_{j}e_{j}$
. Hence
![]() $|C_{n+1}C_{n}^{-1}|=|b_{j}|$
as
$|C_{n+1}C_{n}^{-1}|=|b_{j}|$
as
![]() $e_{i}$
is the standard basis. The inverse of
$e_{i}$
is the standard basis. The inverse of
![]() $C_{n+1}C_{n}^{-1}$
is
$C_{n+1}C_{n}^{-1}$
is
![]() $C_{n}C_{n+1}^{-1}$
. Thus we have a similar decomposition of a vector in
$C_{n}C_{n+1}^{-1}$
. Thus we have a similar decomposition of a vector in
![]() $\Sigma (I,q,n+1)(x)$
, namely,
$\Sigma (I,q,n+1)(x)$
, namely,
 $$ \begin{align*} v=v^{\prime}+C_{n}^{-1}\sum_{i\geq_{q+1}}b_{i*}e_{i}, \end{align*} $$
$$ \begin{align*} v=v^{\prime}+C_{n}^{-1}\sum_{i\geq_{q+1}}b_{i*}e_{i}, \end{align*} $$
where
![]() $v^{\prime } \in \Sigma (I,q,n)(x)$
and
$v^{\prime } \in \Sigma (I,q,n)(x)$
and
![]() $C_{n}^{-1}\sum _{i\geq _{q+1}}b_{i*}e_{i} \in \Sigma (I,q,n)(x)^{\perp } $
. We set
$C_{n}^{-1}\sum _{i\geq _{q+1}}b_{i*}e_{i} \in \Sigma (I,q,n)(x)^{\perp } $
. We set
![]() $a_{i}= \exp (\Lambda ^{i}-i\varepsilon )$
, and note that
$a_{i}= \exp (\Lambda ^{i}-i\varepsilon )$
, and note that
![]() $|C_{n}C_{n+1}^{-1}|=|b_{i*}|$
. It then follows that
$|C_{n}C_{n+1}^{-1}|=|b_{i*}|$
. It then follows that
 $$ \begin{align*} |b_{i*}|\leq C \exp-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i}-\Lambda ^{i_{q}}-r\varepsilon), \end{align*} $$
$$ \begin{align*} |b_{i*}|\leq C \exp-\bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i}-\Lambda ^{i_{q}}-r\varepsilon), \end{align*} $$
since we have a cycle of length r.
Lemma 6. If
![]() $v^{i} \in \Sigma (I,q)(x)$
, then
$v^{i} \in \Sigma (I,q)(x)$
, then
![]() ${\log \|\Phi (n,x)(v_{n}^{i})\|}/{\Omega (x)}\leq \lambda ^{i_q}(x)$
.
${\log \|\Phi (n,x)(v_{n}^{i})\|}/{\Omega (x)}\leq \lambda ^{i_q}(x)$
.
Proof. Firstly, we can see that
If
![]() $i<i_{q+1}$
, we have
$i<i_{q+1}$
, we have
![]() $\limsup ({\log \|\Phi (n,x)(v_{n}^{i})\|}/{\Omega (x)})\leq \Lambda ^{i_{q}}$
.
$\limsup ({\log \|\Phi (n,x)(v_{n}^{i})\|}/{\Omega (x)})\leq \Lambda ^{i_{q}}$
.
On the other hand,
![]() $ v^{i}-v_{n}^{i}=w+C_{n}^{-1}\sum _{i\geq _{q+1}}b_{i*}e_{i}$
. For
$ v^{i}-v_{n}^{i}=w+C_{n}^{-1}\sum _{i\geq _{q+1}}b_{i*}e_{i}$
. For
![]() $w\in \Sigma (I,q,n)(x)$
,
$w\in \Sigma (I,q,n)(x)$
,
![]() $\limsup ({\log }$
$\limsup ({\log }$
![]() ${\|\Phi (n,x)(w)\|}/{\Omega (x)})\leq \Lambda ^{i_{q}}$
. Hence
${\|\Phi (n,x)(w)\|}/{\Omega (x)})\leq \Lambda ^{i_{q}}$
. Hence
 $$ \begin{align*} &\limsup\frac{\log \|\Phi(n,x)C_{n}^{-1}\sum_{i\geq i_{q+1}}b_{i*}e_{i}\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}\\[3pt] &\quad\leq \limsup\frac{\log\{ C \exp-(\Omega(x))(\Lambda^{i}-\Lambda ^{i_{q}}-r\epsilon)\times \delta_{i} (\Phi(n,x))\}}{\Omega(x)}\\[3pt] &\quad=-\Lambda^{i}+\Lambda ^{i_{q}}+r\epsilon +\Lambda^{i}=\Lambda ^{i_{q}}+r\epsilon \end{align*} $$
$$ \begin{align*} &\limsup\frac{\log \|\Phi(n,x)C_{n}^{-1}\sum_{i\geq i_{q+1}}b_{i*}e_{i}\|}{\sum_{i=0}^{n-1}\omega_{i}(x)}\\[3pt] &\quad\leq \limsup\frac{\log\{ C \exp-(\Omega(x))(\Lambda^{i}-\Lambda ^{i_{q}}-r\epsilon)\times \delta_{i} (\Phi(n,x))\}}{\Omega(x)}\\[3pt] &\quad=-\Lambda^{i}+\Lambda ^{i_{q}}+r\epsilon +\Lambda^{i}=\Lambda ^{i_{q}}+r\epsilon \end{align*} $$
for
![]() $i\geq i_{q+1}$
.
$i\geq i_{q+1}$
.
The triangle inequality implies that
 $$ \begin{align*}\|\Phi(n,x)(v^{i})\|\leq \|\Phi(n,x)(v_{n}^{i})\|+\|\Phi(n,x)(v^{i}-v_{n}^{i})\| \leq 2 \exp \bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda ^{i_{q}}+\varepsilon), \end{align*} $$
$$ \begin{align*}\|\Phi(n,x)(v^{i})\|\leq \|\Phi(n,x)(v_{n}^{i})\|+\|\Phi(n,x)(v^{i}-v_{n}^{i})\| \leq 2 \exp \bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda ^{i_{q}}+\varepsilon), \end{align*} $$
and thus
![]() $\limsup ({\log \|\Phi (n,x)(v^{i})\|}/{\sum _{i=0}^{n-1}\omega _{i}(x)})\leq \Lambda ^{i_{q}}$
.
$\limsup ({\log \|\Phi (n,x)(v^{i})\|}/{\sum _{i=0}^{n-1}\omega _{i}(x)})\leq \Lambda ^{i_{q}}$
.
Lemma 7. If the vector
![]() $v^{i}$
is not in
$v^{i}$
is not in
![]() $\Sigma (I,q-1)(x)$
, for large n, the projection
$\Sigma (I,q-1)(x)$
, for large n, the projection
![]() $v^{i\prime }$
belongs to
$v^{i\prime }$
belongs to
![]() $\Sigma (I,q-1,n)(x)$
with
$\Sigma (I,q-1,n)(x)$
with
![]() $\|v^{i\prime }\|>c>0$
. Then
$\|v^{i\prime }\|>c>0$
. Then
 $$ \begin{align*} \liminf\frac{\log\|\Phi(n,x)(v^{i})\|}{\sum_{i=0}^{n-1}\omega_{i}(x)} \geq \Lambda^{i_q}(x). \end{align*} $$
$$ \begin{align*} \liminf\frac{\log\|\Phi(n,x)(v^{i})\|}{\sum_{i=0}^{n-1}\omega_{i}(x)} \geq \Lambda^{i_q}(x). \end{align*} $$
Proof. This proof is quite straightforward. We take a unit vector
![]() $v^{i}$
which is not in
$v^{i}$
which is not in
![]() $\Sigma (I,q-1)(x)$
. There is a
$\Sigma (I,q-1)(x)$
. There is a
![]() $\delta \in V$
such that
$\delta \in V$
such that
![]() $v^{i}+\delta \in \Sigma (I,q-1)(x) $
. When n is large enough, the vector
$v^{i}+\delta \in \Sigma (I,q-1)(x) $
. When n is large enough, the vector
![]() $v^{i}_{n}$
has projection
$v^{i}_{n}$
has projection
![]() $v^{i\prime }$
in
$v^{i\prime }$
in
![]() $\Sigma (I,q-1,n)(x)$
and orthogonal complement
$\Sigma (I,q-1,n)(x)$
and orthogonal complement
![]() ${v^i}'' \in \Sigma (I,q-1,n)(x)^{\perp } $
. We take the difference
${v^i}'' \in \Sigma (I,q-1,n)(x)^{\perp } $
. We take the difference
![]() $\|v^{i}-v^{i\prime }\|\geq {\delta }/{2}$
, obtaining
$\|v^{i}-v^{i\prime }\|\geq {\delta }/{2}$
, obtaining
 $$ \begin{align*} \|\Phi(n,x)(v^{i})\|& \approx \|\Phi(n,x)v^{i}_{n}\|\\[3pt] &\geq \|\Phi(n,x)(v^{i}-v^{i\prime})\|\\[3pt] &\geq \frac{\delta}{2}\exp \bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i_q}(x)-\varepsilon).\\[-3.8pc] \end{align*} $$
$$ \begin{align*} \|\Phi(n,x)(v^{i})\|& \approx \|\Phi(n,x)v^{i}_{n}\|\\[3pt] &\geq \|\Phi(n,x)(v^{i}-v^{i\prime})\|\\[3pt] &\geq \frac{\delta}{2}\exp \bigg(\sum_{i=0}^{n-1}\omega_{i}(x)\bigg)(\Lambda^{i_q}(x)-\varepsilon).\\[-3.8pc] \end{align*} $$
Combining Lemmas 6 and 7, we can conclude that
 $$ \begin{align*} \lim ({\log \|\Phi (n,x)(v^{i})\|}/ \sum _{i=0}^{n-1} \omega _{i}(x)) =\Lambda ^{i_q}(x),\end{align*} $$
$$ \begin{align*} \lim ({\log \|\Phi (n,x)(v^{i})\|}/ \sum _{i=0}^{n-1} \omega _{i}(x)) =\Lambda ^{i_q}(x),\end{align*} $$
for
![]() $v_{i} \in \Sigma (I,q)(x)\setminus \Sigma (I,q-1)(x)$
. We now see that the eigenspace is
$v_{i} \in \Sigma (I,q)(x)\setminus \Sigma (I,q-1)(x)$
. We now see that the eigenspace is
![]() $C_{n}^{-1}e_{i} \to C^{-1}e_{i}$
and the eigenvalue is
$C_{n}^{-1}e_{i} \to C^{-1}e_{i}$
and the eigenvalue is
![]() $\Lambda ^{k}(x)=\lim _{n\to \infty }({ \log \delta _{k} (\Phi (n,x))}/ {\sum _{i=0}^{n-1}\omega _{i}(x)})$
, so that the limit matrix
$\Lambda ^{k}(x)=\lim _{n\to \infty }({ \log \delta _{k} (\Phi (n,x))}/ {\sum _{i=0}^{n-1}\omega _{i}(x)})$
, so that the limit matrix
![]() $A(x) =\lim _{n\to \infty } (\Phi (n,x)^{*}\Phi (n,x))^{{1}/{2n}}$
exists.
$A(x) =\lim _{n\to \infty } (\Phi (n,x)^{*}\Phi (n,x))^{{1}/{2n}}$
exists.
Acknowledgements
We have been influenced by Raghunathan’s elegant proof of the Oseledeč theorem [Reference Raghunathan12]. We would like to thank Anthony Quas for drawing our attention to this paper, and for useful discussions. We would also like to thank the referee for several useful remarks.