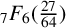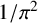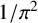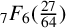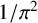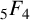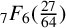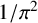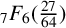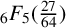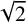1 Introduction
The study and application of the acceleration of convergence forms a major part of the discipline of numerical analysis. In this article, we offer new applications of a series acceleration method we had previously formulated in [Reference Campbell3]. We apply this method to obtain series evaluations related to a remarkable formula due to Guillera [Reference Guillera18].
The
$\Gamma $
-function defined by
$\Gamma (x) = \int _{0}^{\infty } u^{x-1} e^{-u} \, du$
for
$\Re (x)> 0$
has many remarkable properties [Reference Rainville24, Sections 17 and 20], such as the reflection formula
$$ \begin{align} \Gamma(x) \Gamma(1-x) = \frac{\pi}{\sin(\pi x)}. \end{align} $$
The Pochhammer symbol may be defined so that
$ (x)_{n} = {\Gamma (x+n)}/{\Gamma (x)}$
, and it is common to use the notation
$$ \begin{align*} \left[ \begin{matrix} \alpha, \beta, \ldots, \gamma \\ A, B, \ldots, C \end{matrix} \right]_{k} = \frac{ (\alpha)_{k} (\beta)_{k} \cdots (\gamma)_{k} }{ (A)_{k} (B)_{k} \cdots (C)_{k}}. \end{align*} $$
A generalised hypergeometric series [Reference Bailey1] may be defined by
$$ \begin{align*} {}_{p}F_{q} \left[ \begin{matrix} a_{1}, a_{2}, \ldots, a_{p} \\ b_{1}, b_{2}, \ldots, b_{q} \end{matrix} \ \Bigg| \ x \right] = \sum_{k = 0}^{\infty} \left[ \begin{matrix} a_{1}, a_{2}, \ldots, a_{p} \\ b_{1}, b_{2}, \ldots, b_{q} \end{matrix} \right]_{k} \frac{x^{k}}{k!}. \end{align*} $$
In 2011, Guillera applied the Wilf–Zeilberger (WZ) method [Reference Petkovšek, Wilf and Zeilberger23] to prove the hypergeometric formulas
$$ \begin{align} \frac{48}{\pi^2} = \sum_{k = 0}^{\infty} \bigg( \frac{27}{64} \bigg)^{k} \left[ \begin{matrix} \frac{1}{2}, \frac{1}{2}, \frac{1}{2}, \frac{1}{3}, \frac{2}{3} \\ 1, 1, 1, 1, 1 \end{matrix} \right]_{k} ( 74 k^2 + 27 k + 3 ) \end{align} $$
and
$$ \begin{align} \frac{16 \pi^2}{3} = \sum_{k = 0}^{\infty} \bigg( \frac{27}{64} \bigg)^{k} \left[ \begin{matrix} 1, 1, 1, \frac{5}{6}, \frac{7}{6} \\ \frac{3}{2}, \frac{3}{2}, \frac{3}{2}, \frac{3}{2}, \frac{3}{2} \end{matrix} \right]_{k} ( 74 k^2 + 101 k + 35 ). \end{align} $$
These formulas are the main sources of inspiration behind the hypergeometric formulas introduced in this article.
Subsequent to Guillera introducing (1.2) and (1.3) [Reference Guillera18], Chu and Zhang [Reference Chu and Zhang9], in 2014, provided alternative proofs, via an acceleration formula based on Dougall’s
${}_{5}F_{4}$
-identity, for the series of convergence rate
$\frac {27}{64}$
shown in (1.2) and (1.3). Afterwards, in 2018, Guillera [Reference Guillera19] offered yet another proof of (1.2), by applying a recursive argument using Dougall’s sum together with the WZ method. The convergence rate of
$\frac {27}{64}$
of Guillera’s series in (1.2) and (1.3) was a subject of emphasis in Chen and Chu’s number-theoretic work in [Reference Chen and Chu4], and q-analogues of (1.2) and (1.3) were the main results of the 2021 research contribution from Chen and Chu [Reference Chen and Chu4]. The many research publications concerning Guillera’s formula in (1.2) [Reference Chen and Chu4, Reference Chu and Zhang9, Reference Guillera18, Reference Guillera19] motivate the development of techniques for evaluating new, high-order
${}_{p}F_{q}$
-series of convergence rate
$\frac {27}{64}$
and this is the main purpose of our article.
It is easily seen that (1.2) may be expressed with a
${}_{7}F_{6}( \frac {27}{64} )$
-series. The interest in the hypergeometric formulas in (1.2) and (1.3) noted above raises the question as to how we may obtain similar results for fundamental mathematical constants apart from
$\pi ^{\pm 2}$
with new rational
${}_{p}F_{q}( \frac {27}{64} )$
-series. In this article, we apply a WZ-based method we had given in [Reference Campbell3], to prove new
${}_{7}F_{6}( \frac {27}{64} )$
- and
${}_{6}F_{5}( \frac {27}{64} )$
-series for the value
$\sqrt {2}$
(sometimes referred to as Pythagoras’s constant [Reference Weisstein27]). Our new formulas for this constant closely resemble (1.2) and (1.3) and also bear a resemblance to the identities
$$ \begin{align*} \frac{105 \sqrt{2}}{4} = \sum_{k = 0}^{\infty} \bigg( \frac{-4}{27} \bigg)^{k} \left[ \begin{matrix} \frac{1}{2}, \frac{1}{4}, \frac{3}{4}, \frac{1}{6}, \frac{5}{6} \\ 1, \frac{11}{12}, \frac{13}{12}, \frac{17}{12}, \frac{19}{12} \end{matrix} \right]_{k} ( 1488 k^3 + 1640 k^2 + 517 k + 40 ), \end{align*} $$
$$ \begin{align*} \frac{45 \sqrt{2}}{4} = \sum_{k = 0}^{\infty} \bigg( \frac{4}{27} \bigg)^{k} \left[ \begin{matrix} \frac{1}{4}, \frac{1}{4}, \frac{3}{4}, \frac{3}{4}, \frac{3}{4} \\ 1, \frac{7}{8}, \frac{11}{8}, \frac{13}{12}, \frac{17}{12} \end{matrix} \right]_{k} ( 736 k^3 + 644 k^2 + 169 k + 12 ) \end{align*} $$
and
$$ \begin{align*} \frac{21 \sqrt{2}}{4} = \sum_{k = 0}^{\infty} \bigg( \frac{16}{27} \bigg)^{k} \left[ \begin{matrix} \frac{1}{2}, \frac{3}{4}, \frac{1}{8}, \frac{5}{8} \\ 1, \frac{5}{4}, \frac{11}{12}, \frac{19}{12} \end{matrix} \right]_{k} ( 44 k^2 + 37 k + 6 ) \end{align*} $$
given in [Reference Chu and Zhang9]. The
${}_{4}F_{3}( {2}/{27} )$
-identity
$$ \begin{align*} \frac{3 \sqrt{2}}{4} = \sum_{k = 0}^{\infty} \bigg( \frac{2}{27} \bigg)^{k} \left[ \begin{matrix} \frac{1}{2}, \frac{1}{2}, \frac{1}{2} \\ \frac{5}{6}, 1, \frac{7}{6} \end{matrix} \right]_{k} (5 k+1) \end{align*} $$
of Ramanujan type [Reference Chu6] was proved using the modified Abel lemma on summation by parts by Chu in [Reference Chu6] and was highlighted as a main result in [Reference Chu6]. This provides further motivation for the interest in Theorems 3.1 and 3.2 below.
2 Background
As in [Reference Ekhad12], the Ekhad computer system of Zeilberger produced many so-called ‘strange’ finite hypergeometric identities, through a systematised computer search for new finite summation identities for hypergeometric expressions. We have discovered experimentally that WZ pairs indicated in [Reference Ekhad12] may be applied, in conjunction with the WZ-based acceleration method from [Reference Campbell3], to obtain new
${}_{p}F_{q}( \frac {27}{64} )$
-series resembling Guillera’s series in (1.2). It seems that our main results, which are highlighted in Theorems 3.1 and 3.2 below, are new and that there are no equivalent results given in any past literature citing [Reference Ekhad12], including [Reference Beukers and Forsgøard2, Reference Chu5, Reference Chu7, Reference Chu and Kılıç8, Reference Ebisu10, Reference Ebisu11, Reference Mao and Tauraso20, Reference Oerlemans22].
The WZ-based series acceleration method in [Reference Campbell3] was applied, using WZ pairs corresponding to finite Catalan sum identities given by Chu and Kılıç [Reference Chu and Kılıç8], to prove new and very fast convergent series for fundamental constants such as Apéry’s constant
$\zeta (3) = 1 + {1}/{2^3} + {1}/{3^3} + \cdots $
, and many of the series introduced in [Reference Campbell3] were later included in the online reference work of the Wolfram Research company [Reference Marichev, Sondow and Weisstein21, Reference Weisstein25, Reference Weisstein26], including Campbell’s formula
$$ \begin{align} \frac{\pi^2}{4} = \sum_{n=1}^{\infty} \frac{16^{n} (n+1) (3n+1) }{n(2n+1)^2 \binom{2n}{n}^3}, \end{align} $$
which is of convergence rate
$\tfrac 14$
, and Campbell’s formula
$$ \begin{align*} -448 \zeta (3)-128 = \sum_{n=1}^{\infty} \frac{(-2^{12})^n (7168 n^5-1664 n^4-1328 n^3 + 212 n^2+49 n-9)}{n^4 (2 n-1) (3 n+1) (4 n+1) \binom{2 n}{n} \binom{3 n}{n} \binom{4 n}{2 n}^3}, \end{align*} $$
which is of convergence rate
$\frac {1}{27}$
. We briefly recall some preliminaries on WZ pairs, and we briefly review the acceleration method employed in [Reference Campbell3], which is related to WZ-based techniques given in the work of Guillera [Reference Guillera13–Reference Guillera19].
Bivariate hypergeometric functions
$F(n, k)$
and
$G(n, k)$
are said to form a WZ pair if they satisfy the discrete difference equation
$$ \begin{align} F(n+1, k) - F(n, k) = G(n, k+1) - G(n, k), \end{align} $$
with
$\lim _{k \to \infty } G(n, k) = 0$
and
$G(n, 0) = 0$
[Reference Petkovšek, Wilf and Zeilberger23]. We also typically work under the assumption that
$F(n, k)$
, as a discrete function for integers n and k, vanishes everywhere outside a finite interval for k if n is fixed. Although WZ pairs may be thought of as having been chiefly designed for the purposes of proving conjectured evaluations for finite sums of the form
$\sum _{k} F(n, k)$
by making use of the telescoping of the left-hand side of (2.2) upon the application of
$\sum _{k}$
, the difference equation in (2.2) is extremely versatile in terms of identities for WZ pairs that we may derive using (2.2) and through the use of telescoping arguments. For example, Guillera has often made use of identities obtained by summing both sides of (2.2) with respect to n, as opposed to k, and Guillera’s applications of the WZ identity
$$ \begin{align} \sum_{n=0}^{\infty} G(n, k) - \sum_{n=0}^{\infty} G(n, k+1) = F(0, k) - \lim_{n \to \infty} F(n, k) \end{align} $$
have led us to consider the following approach, as in [Reference Campbell3].
For a WZ pair
$(F, G)$
such that
$\lim _{a \to \infty } F(a, r) = 0$
for all r, the WZ identity in (2.3) gives
$$ \begin{align*} -F(0, r) = \sum_{n=0}^{\infty} (G(n,r+1) - G(n,r)). \end{align*} $$
Following [Reference Campbell3], we set the variable r to be b, and then
$b+1$
, and then
$b+2$
, and so forth, and then we add the resultant identities, so that a telescoping phenomenon gives
$$ \begin{align} -\sum_{n=0}^{m} F(0, b+n) = \sum_{n=0}^{\infty} (G(n, b+m+1) - G(n, b)). \end{align} $$
We then argue, if possible (depending on possible issues concerning convergence or the interchange of limiting operations), as in [Reference Campbell3], how the sum
$ \sum _{n=0}^{\infty } G(n, b+m+1) $
may be simplified, so as to obtain an equality such as
$$ \begin{align} -\sum_{n=0}^{\infty} F(0, b+n) = \text{constant} - \sum_{n=0}^{\infty} G(n, b). \end{align} $$
This approach was used in [Reference Campbell3] to prove fast converging formulas as in (2.1). If both of the series in (2.5) are convergent, then (2.5) may be regarded as an acceleration formula, in view of the applications of (2.5) from [Reference Campbell3], including the series of convergence rate
$\frac {1}{27}$
for Apéry’s constant that we have reproduced above. To successfully apply (2.5) as a series acceleration identity, we need to use a WZ pair
$(F, G)$
satisfying the conditions we have indicated and such that the above issues would not apply. Given a WZ pair
$(F, G)$
, it seems that only in exceptional cases, (2.5) may be applied for accelerating series, which undelines the remarkable nature of Theorems 3.1 and 3.2 below.
3 New
${}_{p}F_{q}( \frac {27}{64} )$
-series
Our proof of Theorem 3.1 below involves the first WZ pair introduced in [Reference Ekhad12]. This first WZ pair corresponds to the following identity introduced in [Reference Ekhad12]:
$$ \begin{align} {}_{2}F_{1}\!\!\left[ \begin{matrix} -n, -4n - \frac{1}{2} \\ -3n \end{matrix} \ \Bigg| \ -1 \right] = \bigg( \frac{64}{27} \bigg)^{n} \left[ \begin{matrix} \frac{3}{8}, \frac{5}{8} \\ \frac{1}{3}, \frac{2}{3} \end{matrix} \right]_{n}. \end{align} $$
Theorem 3.1. The closed-form evaluation
$$ \begin{align*} 3 \sqrt{2} = \sum _{n = 0}^{\infty} \bigg(\frac{1}{2}\bigg)^{6 n} \frac{ \big(\frac{1}{2}\big)_{3 n} \big(\frac{1}{2}\big)_{4 n} }{ \big(\frac{3}{8}\big)_n \big(\frac{1}{2}\big)_n \big(\frac{5}{8}\big)_n (1)_{4 n} } \frac{ 592 n^2-154 n+3 }{ (6 n-1) (8 n-1) } \end{align*} $$
holds.
Proof. We write the left-hand side of the Ekhad–Zeilberger identity (3.1) as a finite sum, according to the Pochhammer identity which shows that
$(-n)_{k} $
vanishes for
$k> n \in \mathbb {N}_{0}$
. Dividing the summand of the resultant sum by the right-hand side of (3.1), gives an expression equivalent to
$$ \begin{align*} \frac{(-1)^k \big(\frac{27}{64}\big)^n \big(\frac{1}{3}\big)_n \big(\frac{2}{3}\big)_n \big(-4 n - \frac{1}{2}\big)_k (-n)_k}{k! \big(\frac{3}{8}\big)_n \big(\frac{5}{8}\big)_n (-3 n)_k}. \end{align*} $$
To compute the above expression for noninteger values, we apply the reflection formula in (1.1) so as to obtain the hypergeometric function
$$ \begin{align*} F(n, k) = \frac{\pi 2^{-6 n} \sec \big(\frac{\pi }{8}\big) \Gamma \big(4 n+\frac{3}{2}\big) \Gamma (3 n-k+1)}{\Gamma (k+1) \Gamma \big(n+\frac{3}{8}\big) \Gamma \big(n+\frac{5}{8}\big) \Gamma (n-k+1) \Gamma \big(4 n-k+\frac{3}{2}\big)}. \end{align*} $$
So, we may apply the WZ method to obtain the WZ proof certificate given by the following verbatim output [Reference Ekhad12]:
Letting
$R(n, k)$
denote the rational function given by this output, we write
$ G(n, k) = R(n,k) F(n,k)$
, which shows that the WZ difference equation in (2.2) holds for the pair
$(F, G)$
of bivariate mappings we have defined. Applying the summation operator
$\sum _{k=0}^{m} \cdot $
to both sides of this difference equation, the left-hand side telescopes under the application of this operator, giving
$$ \begin{align*} F(m+1,k)-F(0,k) = \sum _{n=0}^m (G(n,k+1)-G(n,k)). \end{align*} $$
So,
$$ \begin{align*} -F(0, k) = \sum_{n=0}^{\infty} (G(n, k+1) - G(n, k)). \end{align*} $$
Mimicking a telescoping argument from [Reference Campbell3], this can be used to show that (2.4) holds for the WZ pair
$(F, G)$
involved in our current proof. Writing
$$ \begin{align} \sum_{n=0}^{m} - F(0, b+n) + \sum_{n=0}^{\infty} G(n, b) = \sum_{n=0}^{\infty} G(n, b+m+1), \end{align} $$
we claim that we obtain
$-\sqrt {2}$
after letting
$m \to \infty $
and
$b \to 0$
. It is easily seen that the left-hand side of (3.2) is convergent as
$m \to \infty $
, which shows that the value of
$\sum _{n=0}^{\infty } G(n, b + m + 1)$
does not depend on m. Since
$G(n, 0)$
vanishes, and since
$$ \begin{align*} -F(0, 0 + n) = -\frac{\pi ^{3/2} \sec \big(\frac{\pi }{8}\big)}{2 \Gamma \big(\frac{3}{8}\big) \Gamma \big(\frac{5}{8}\big) \Gamma \big(\frac{3}{2}-n\big) \Gamma (n+1)}, \end{align*} $$
we may easily check that
$$ \begin{align*} -\sqrt{2} = \sum _{n=0}^{\infty } -F(0,0+n). \end{align*} $$
So, this gives us a proof of the identity
$$ \begin{align*} \sum _{n=0}^\infty -F(0,b+n) = -\sqrt{2}-\sum _{n=0}^{\infty} G(n,b). \end{align*} $$
Equivalently,
$$ \begin{align*} -\sqrt{2} + \frac{\sqrt{\pi }}{2 \Gamma \big(\frac{3-2 b}{2} \big) \Gamma (b+1)} \, {}_{2}F_{1}\!\!\left[ \begin{matrix} 1, b - \frac{1}{2} \\ b + 1 \end{matrix} \ \bigg| \ -1 \right] = \sum_{n=0}^{\infty} G(n, b). \end{align*} $$
Setting
$b = \tfrac 12$
and applying an index shift, we obtain an equivalent formulation of the desired result.
The series highlighted in Theorem 3.1, which may be rewritten as a
${}_{6}F_{5}( \frac {27}{64} )$
- series, is very nontrivial in the sense that state-of-the-art Computer Algebra Systems are not able to provide any closed form or simplification for this high-order
${}_{p}F_{q}$
-series.
After (3.1), the next out of the forty strange identities generated by Ekhad in [Reference Ekhad12] is
$$ \begin{align} {}_{2}F_{1}\!\!\left[ \begin{matrix} -n, -4n - \frac{5}{2} \\ -3n - 1 \end{matrix} \ \Bigg| \ -1 \right] = \bigg( \frac{64}{27} \bigg)^{n} \left[ \begin{matrix} \frac{7}{8}, \frac{9}{8} \\ \frac{2}{3}, \frac{4}{3} \end{matrix} \right]_{n}. \end{align} $$
Using the WZ certificate associated with (3.3), as given in [Reference Ekhad12], and by mimicking our proof given in Section 3, we may prove the following companion to Theorem 3.1. We may check that the series in Theorem 3.2 may be written as a
${}_{7}F_{6}( \frac {27}{64} )$
-series, giving a natural companion to Guillera’s
${}_{7}F_{6}( \frac {27}{64} )$
-series in (1.2).
Theorem 3.2. The closed-form evaluation
$$ \begin{align*} -16 \sqrt{2} = \sum _{n = 0}^{\infty} \bigg(\frac{1}{2}\bigg)^{6 n} \frac{\big(\frac{1}{2}\big)_{3 n} \big(\frac{1}{2}\big)_{4 n}}{\big(\frac{1}{8}\big)_n \big(\frac{1}{2}\big)_n \big(\frac{7}{8}\big)_n (1)_{4 n}} \frac{1184 n^3+876 n^2+216 n+29}{(2 n+1) (4 n+1) (6 n-1)} \end{align*} $$
holds.
We may obtain many similar results using variants of the finite hypergeometric identities in (3.1) and (3.3).
