The Equally Tempered Scale

In an equally tempered scale, the size of each step equals the largest common factor of all its constituent intervals. The semitone and quarter-tone scales both belong to this category of scales. Moreover, both are octave-repeating, so that in both semitonal and quarter-tonal contexts, all pitches separated by one or several octaves belong to the same pitch class and, consequently, bear the same name. To devise the semitone scale, one begins with the interval between the second and third harmonics: the perfect fifth (see Figure 1).

Figure 1: Selection of the second and third harmonics within the harmonic series.

Its root belongs to the pitch class of the fundamental. By cycling this interval until returning to that pitch class, one obtains the circle of fifths (see Figure 2).

Figure 2: Circle of fifths.

The circle of fifths generates the semitone scale when its 12 constituent pitch classes are organised in an ascending pattern of the narrowest possible equal intervals (see Figure 3).

Figure 3: Semitone scale.

This is known as the cyclic principle, according to which a scale results from the cycling of an interval other than the unison once the return to the original pitch class has occurred.

The quarter-tone scale can be devised following the same principle. The interval between the eighth and eleventh harmonics is that of the major fourth (see Figure 4).

Figure 4: Selection of the eighth and eleventh harmonics within the harmonic series.

Like the perfect fifth, its root belongs to the pitch class of the fundamental. By cycling this interval until returning to that pitch class, one obtains the circle of major fourths (see Figure 5).

Figure 5: Circle of major fourths.

The circle of major fourths generates the quarter-tone scale when its 24 constituent pitch classes are organised in an ascending pattern of the narrowest possible equal intervals (see Figure 6).

Figure 6: Quarter-tone scale.

The White-Key Scale

Anyone who looks at a piano keyboard will notice that the semitone scale is arranged in two rows: the white keys and the black keys. The former corresponds to the diatonic scale and the latter to the pentatonic one. To derive the diatonic scale, one begins by adding a perfect fifth, the interval at the origin of the semitone scale, above a given pitch and below its octave. This yields the interval of the tonos (ancient Greek: ‘tone’) (see Figure 7).

Figure 7: Tonos.

When this tone passes through the two perfect fourths it separates, the diatonic scale is formed, composed of two identical tetrachords (see Figure 8).

Figure 8: Diatonic scale.

The first degree of the first tetrachord is the tonic and that of the second the dominant (see Figure 9).

Figure 9: Tonic and dominant in the diatonic system.

The diatonic scale contains seven degrees. This equals half of the pitch classes contained within the semitone scale from which it is derived plus one, while its complementary black-key scale, the pentatonic, contains five degrees, half of the pitch classes minus one. Together, these two numbers of degrees form a pair of twin primes. The interval used cyclically to generate the semitone scale and its inverse correspond to these numbers when expressed in integer notation: [0, 7] modulo 12 represents the perfect fifth and [0, 5] modulo 12 the perfect fourth.

A white-key scale can be derived from the quarter-tone scale in the same way. When a major fourth, the interval at the origin of the quarter-tone scale, is added above a given pitch and below its octave, the interval of the hemitonos (ancient Greek: ‘semitone’) appears (see Figure 10).

Figure 10: Hemitonos.

When this semitone passes through the two major fourths it separates, the diahemitonicFootnote ¹ scale, as I term it, is formed, composed of two identical heptachords (see Figure 11).

Figure 11: Diahemitonic scale.

The first degree of the first heptachord is therefore the tonic and that of the second the dominant (see Figure 12).

Figure 12: Tonic and dominant in the diahemitonic system.

The diahemitonic scale contains 13 degrees. This equals half of the pitch classes contained within the quarter-tone scale from which it is derived plus one, while its complementary black-key scale, which I term the hendecatonic,Footnote ² contains 11 degrees, half of the pitch classes minus one. Together, these two numbers of degrees form the pair of twin primes which directly follows that formed by the diatonic–pentatonic couple within the sequence of pairs of twin primes. The interval used cyclically to generate the quarter-tone scale and its inverse correspond to these numbers when expressed in integer notation: [0, 13] modulo 24 represents the minor fifth and [0, 11] modulo 24 the major fourth, which parallels the above. Wyschnegradsky described the diahemitonic scale as ‘diatonicised chromaticism’, the quarter-tonal analogue of the diatonic scale.Footnote ³ The mathematician, philosopher and musicologist Franck Jedrzejewski also derived this scale (starting from the thirteenth pitch class onwards) when running an algorithm conceived to identify the white keys within any equal division of the octave (see Table 1).Footnote ⁴

Table 1: White-key scales derived algorithmically from the semi- to sixth-tone divisions of the octave

The Mode

The mode is the configuration of the white-key scale once the tonic is defined. The mood of the music is dependent upon the chosen mode and some modes do indeed appear to sound brighter or darker than others, according to their intervallic pattern. Those with wide intervals may be said to sound bright and those with narrow intervals dark. I shall explain the logic following which the diatonic modes have been hierarchised from the brightest to the darkest. When the diatonic scale is left in its original state, it is configured as the C diatonic mode (see Figure 13).

Figure 13: C diatonic mode.

When inverted, it is configured as the E diatonic mode (see Figure 14).

Figure 14: E diatonic mode.

By virtue of their reciprocal inversion, the former must be located at the same distance from the brightest level in the hierarchy of the diatonic modes as the latter is located from the darkest. Equidistantly from these two modes lies a symmetric one, the D diatonic mode (see Figure 15).

Figure 15: D diatonic mode.

By virtue of its symmetry, this mode must be located at the midpoint of the hierarchy of the diatonic modes. When following the circle of fifths clockwise, one establishes this hierarchy with one interval reduced by a semitone at each darker level (see Figure 16).

Figure 16: Hierarchy of the diatonic modes.

The two pitch classes involved in this intervallic reduction are separated by the interval of the tritone and the diatonic modes of which they are the tonics are located at the two ends of the hierarchy of the diatonic modes.

A hierarchy of the diahemitonic modes, from the brightest to the darkest, can be established following the same logic. When the diahemitonic scale is left in its original state, it is configured as the C diahemitonic mode (see Figure 17).

Figure 17: C diahemitonic mode.

When inverted, it is configured as the F diahemitonic mode (see Figure 18).

Figure 18: F diahemitonic mode.

By virtue of their reciprocal inversion, the former must be located at the same distance from the brightest level in the hierarchy of the diahemitonic modes as the latter is located from the darkest. Equidistantly from these two modes lies a symmetric one, the A-quarter-tone-flat diahemitonic mode (see Figure 19).

Figure 19: A-quarter-tone-flat diahemitonic mode.

By virtue of its symmetry, this mode must be located at the midpoint of the hierarchy of the diahemitonic modes. When following the circle of major fourths anticlockwise, one establishes this hierarchy with one interval reduced by a quarter-tone at each darker level (see Figure 20).

Figure 20: Hierarchy of the diahemitonic modes.

The two pitch classes involved in this intervallic reduction are separated by the interval of the tritone and the diahemitonic modes of which they are the tonics are located at the two ends of the hierarchy of the diahemitonic modes, which parallels the above.

The Chord

The chord is a set of pitched notes sounded simultaneously. A variety of chord types exist, of which two are judged to be perfect: the major and the minor. The former originates in the harmonic series and the latter in the subharmonic series.Footnote ⁵ The fifth harmonic lies between the octave-equivalents of the second and third harmonics which form the basis of the diatonic system, enabling a ray to be traced (see Figure 21).

Figure 21: Selection of the fourth to sixth harmonic within the harmonic series.

By tracing it, one obtains the major triad (see Figure 22).

Figure 22: Major triad.

This is known as the radial principle, according to which a perfect chord results from the tracing of a ray between two harmonics or subharmonics whose frequency ratio corresponds to the interval used cyclically to generate a given scale.Footnote ⁶ Once the major triad is detected within the diatonic scale, two interlaced patterns appear (see Figure 23).

Figure 23: Major triad within the diatonic scale.

This enables the derivation of the triadic scale (see Figure 24).

Figure 24: Triadic scale.

The minor triad results from the inversion of the major triad, making it its subharmonic counterpart (see Figure 25).

Figure 25: Minor triad.

Thus, in the C diatonic mode, for example, the first-, fourth- and fifth-degree triads are major, the second-, third- and sixth-degree triads are minor, and the seventh-degree triad is neither major nor minor but diminished.

Diahemitonic chords can be built following the same principle. The eighth and eleventh harmonics form the basis of the diahemitonic system and between them lie the ninth and tenth harmonics, enabling a ray to be traced (see Figure 26).

Figure 26: Selection of the eighth to eleventh harmonic within the harmonic series.

By tracing it, one obtains what I term the major tetrad (see Figure 27).

Figure 27: Major tetrad.

Once the major tetrad is detected within the diahemitonic scale, two interlaced patterns, analogous to those within the diatonic scale, appear (see Figure 28).

Figure 28: Major tetrad within the diahemitonic scale.

This enables the derivation of what I term the tetradic scale (see Figure 29).

Figure 29: Tetradic scale.

What I term the minor tetrad results from the inversion of the major tetrad, making it its subharmonic counterpart (see Figure 30).

Figure 30: Minor tetrad.

Thus, in the C diahemitonic mode, for example, the first-, second-, eighth- and ninth-degree tetrads are major, the fifth-, sixth-, twelfth- and thirteenth-degree tetrads are minor, the third-, fourth-, tenth- and eleventh-degree tetrads are neither major nor minor but what I term neutral, and the seventh-degree tetrad is neither major nor minor but augmented.

The Modal Harmonic Cadence

The modal harmonic cadence is the fall from one chord to another at the end of a phrase, punctuating the musical discourse. The two voices of this clausula vera (Latin: ‘true close’) resolve onto the octave by conjunct contrary motion. A third voice is often added, resolving conjunctly upwards onto the fifth, downwards onto the third or splitting to resolve onto both by conjunct contrary motion. In this case, the diatonic modal harmonic cadence may be defined as consisting of the progression of the last-degree triad in first inversion towards the first-degree triad in root position (see Table 2).

Table 2: Diatonic modal harmonic cadences

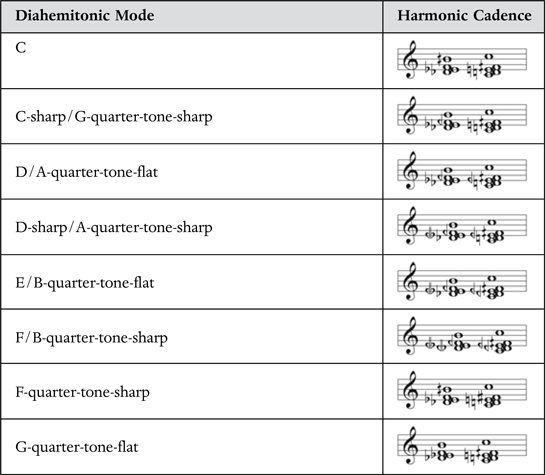
Analogously, the diahemitonic modal harmonic cadence consists of the progression of the last-degree tetrad in first inversion towards the first-degree tetrad in root position (see Table 3).

Table 3: Diahemitonic modal harmonic cadences

The Metre

A metre is a regular cycle of beats, the first of which is strong and those which follow weak. Diatonic modal music employs duple and triple metres, referred to as tempus imperfectum (Latin: ‘imperfect time’) and tempus perfectum (Latin: ‘perfect time’) respectively. This may be justified by the following: the 3:2 frequency ratio corresponds to the interval used cyclically to generate the semitone scale, from which the diatonic scale is derived. As the second harmonic oscillates twice as fast as the fundamental and the third three times as fast, converting these harmonic oscillations into metres results in the second harmonic becoming a duple metre and the third a triple one. The proportion which enables the metric modulation from the one into the other without altering the total duration of the bar is referred to as proportio sesquialtera (Latin: ‘sesquialtera proportion’).

Here I shall apply the reasoning followed above to quarter-tonally analogous numbers. The 11:8 frequency ratio corresponds to the interval used cyclically to generate the quarter-tone scale, from which the diahemitonic scale is derived. As the eighth harmonic oscillates eight times as fast as the fundamental and the eleventh 11 times as fast, converting these harmonic oscillations into metres results in the eighth harmonic becoming an octuple metre and the eleventh an undecuple one. Accordingly, diahemitonic modal music must employ octuple and undecuple metres as respective analogues of the imperfect and perfect times. I neologise the proportion which enables the metric modulation from the one into the other without altering the total duration of the bar as the octansdesesquialteraFootnote ⁸ proportion.

The Subdivision of Beats

Beat subdivision is the operation by which the beat is split into equal parts and is qualified according to its arity. Diatonic modal music employs binary and ternary beat subdivisions, referred to as prolatio minor (Latin: ‘minor prolation’) and prolatio major (Latin: ‘major prolation’) respectively. This may be justified by the following, which will echo the foregoing section: the 3:2 frequency ratio corresponds to the interval used cyclically to generate the semitone scale, from which the diatonic scale is derived. As the second harmonic oscillates twice as fast as the fundamental and the third three times as fast, converting these harmonic oscillations into beat subdivisions results in the second harmonic becoming a binary beat subdivision and the third a ternary one. What consists in the permutation of a two-beat ternary rhythm into a three-beat binary one is referred to as hemiolia (ancient Greek: ‘hemiola’).

As in the earlier section, here I shall apply the reasoning previously followed to quarter-tonally analogous numbers. The 11:8 frequency ratio corresponds to the interval used cyclically to generate the quarter-tone scale, from which the diahemitonic scale is derived. As the eighth harmonic oscillates eight times as fast as the fundamental and the eleventh 11 times as fast, converting these harmonic oscillations into beat subdivisions results in the eighth harmonic becoming an octonary beat subdivision and the eleventh an undenary one. Accordingly, diahemitonic modal music must employ octonary and undenary beat subdivisions as respective analogues of the minor and major prolations. I term the permutation of an eight-beat undenary rhythm into an 11-beat octonary one triogdoola.Footnote ⁹

The Rhythmic Mode and the Ordo

A rhythmic mode is a rhythmic pattern whose ordo is formed by its expression in a phrase. This ordo indicates the number of its consecutive statements before a rest. The rhythmic modes employed in diatonic modal music are in major prolation and are usually believed to have originated in the metres used in ancient Graeco-Latin poetry. However, I suggest an alternative derivation. The second and third harmonics are those at the basis of the diatonic system. If the harmonic series is studied as a rhythmic source, then their selection within it will be equivalent to that of a duplet and a triplet respectively (see Figure 31).

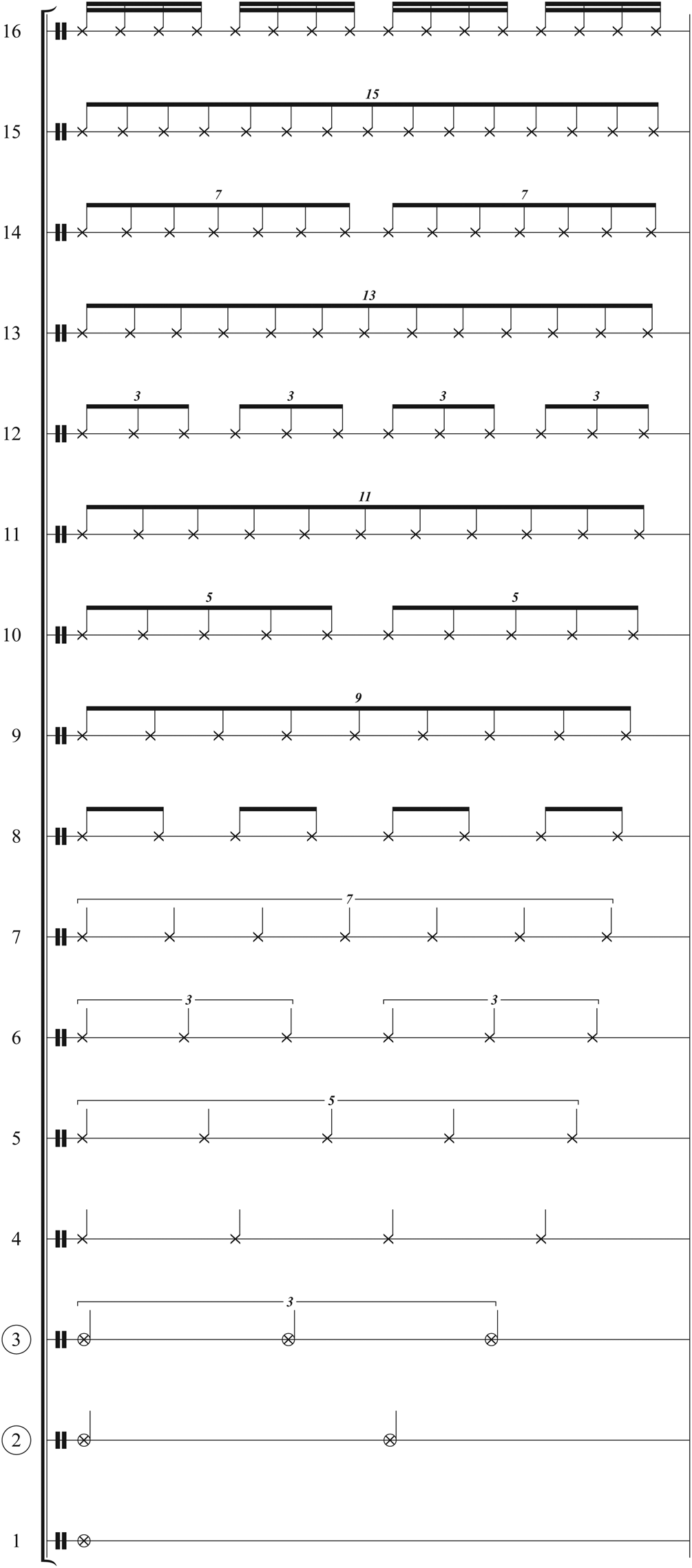
Figure 31: Selection of the second and third harmonics within the rhythm-converted harmonic series.

Their superposition results in two symmetric ternary beat subdivisions (see Figure 32).

Figure 32: Triplet against a duplet.

These ternary rhythms are the first two rhythmic modes employed in diatonic modal music (see Figure 33).

Figure 33: First two ternary rhythmic modes.

When expressed in phrases, they form ordines which indicate the number of their consecutive statements before a rest. Each ordo begins and ends with a note of the same value. A perfect ordo starts on the beat (see Table 4).

Table 4: Perfect ordines of ternary rhythmic modes

An imperfect ordo starts on a weak part of the beat (see Table 5).

Table 5: Imperfect ordines of ternary rhythmic modes

The other ternary rhythmic modes consist of the alternation of a one-beat note value and one of the first two ternary rhythmic modes, the succession of one-beat note values and the fusion of the first two ternary rhythmic modes, resulting in a triplet. Those belonging to the ante- and penultimate categories of rhythmic modes are ultra mensuram (Latin: ‘beyond measure’) in that their duration exceeds one beat, causing a modification of the rule of the ordo: given the composite nature of the rhythmic modes belonging to the former, their perfect ordines begin and end on the strong beat with their first rhythmic cell and their imperfect ones on a weak beat with their second. Similarly, given the exclusive composition of one-beat note values of the rhythmic mode belonging to the latter, its perfect ordo begins and ends on the strong beat and its imperfect one on a weak beat.

Undenary rhythmic modes can be devised in the same way. The eighth and eleventh harmonics are those at the basis of the diahemitonic system. If the harmonic series is studied as a rhythmic source, then their selection within it will be equivalent to that of an octuplet and an undecuplet respectively (see Figure 34).

Figure 34: Selection of the eighth and eleventh harmonics within the rhythm-converted harmonic series.

Their superposition results in two symmetric groups, each of four undenary beat subdivisions (see Figure 35).

Figure 35: Undecuplet against an octuplet.

Following the earlier examples, these undenary rhythms must be the first eight rhythmic modes employable in diahemitonic modal music (see Figure 36).

Figure 36: First eight undenary rhythmic modes.

Table 6 shows the perfect ordines they form when expressed in phrases which start on the beat.

Table 6: Perfect ordines of undenary rhythmic modes

Table 7 shows the imperfect ordines they form when expressed in phrases which start on a weak part of the beat.

Table 7: Imperfect ordines of undenary rhythmic modes

Analogously, the other undenary rhythmic modes consist of the alternation of a one-beat note value and one of the first eight undenary rhythmic modes, the succession of one-beat note values and the fusion of the first eight undenary rhythmic modes, resulting in an undecuplet. Those belonging to the ante- and penultimate categories of rhythmic modes are beyond measure in that their duration exceeds one beat, causing the same modification of the rule of the ordo which we saw earlier in the beyond-measure ternary rhythmic modes.

The Rhythm in Minor Prolation

While the rhythm in major prolation is either configured as a rhythmic mode or is exempt from modal configuration, that in minor prolation is never configured modally. The binary rhythm is usually understood as the product of splitting the beat into two halves. However, I suggest an alternative origin based upon the hemiola, the permutation of a two-beat ternary rhythm into a three-beat binary one. A two-beat ternary rhythm is in major prolation and, by permuting it into a three-beat binary rhythm, the hemiola makes it a rhythm in minor prolation. Since the juxtaposition of the first two ternary rhythmic modes results in a two-beat ternary rhythm, this rhythm can be read hemiolically (see Figure 37).

Figure 37: Hemiola.

By reading it in this way, one obtains the binary rhythm (see Figure 38).

Figure 38: Binary rhythm.

An octonary rhythm can be found in the same way by using the triogdoola, the permutation of an eight-beat undenary rhythm into an 11-beat octonary one. An eight-beat undenary rhythm is in major prolation and, by permuting it into an 11-beat octonary rhythm, the triogdoola makes it a rhythm in minor prolation. Since the juxtaposition of the first eight undenary rhythmic modes results in an eight-beat undenary rhythm, this rhythm can be read triogdoolically (see Figure 39).

Figure 39: Triogdoola.

By reading it in this way, one obtains the octonary rhythm in its first seven kinds (see Figure 40).

Figure 40: First seven kinds of the octonary rhythm.

The fusion of those whose smallest common value is equal to a quarter of a beat results in a quadruplet and that of those whose smallest common value is equal to an eighth of a beat in an octuplet. These two tuplets are added as the eighth and ninth kinds of the octonary rhythm.

The Appoggiatura

The appoggiatura is an ornamental note which delays the sounding of the main note and resolves onto it by conjunct motion. Although most embellishments are rhythmically unquantified, the appoggiatura has a fixed length. The diatonic appoggiatura conventionally has a length of one beat in duple metre, being half of the bar, leaving one beat to the main note (see Figure 41).Footnote ¹⁰

Figure 41: Diatonic appoggiatura in duple metre.

This proportion corresponds to the octave ratio delimiting the frame of the semitone scale and mirrors that of the median pair of note values of the hemiola (see Figure 37). The diatonic appoggiatura conventionally has a length of two beats in triple metre, being two thirds of the bar, leaving one beat to the main note (see Figure 42).Footnote ¹¹

Figure 42: Diatonic appoggiatura in triple metre.

This proportion corresponds to the perfect-fifth ratio at the origin of the semitone scale and mirrors that of the pair of note values of the first ternary rhythmic mode (see Figure 43).

Figure 43: Pair of note values of the first ternary rhythmic mode.

Quarter-tonally analogous proportions can be derived from the median pair of note values of the triogdoola and the pair of note values of the first undenary rhythmic mode, thus establishing the length of the diahemitonic appoggiatura. The median pair of note values of the triogdoola is represented numerically as 4 + 4, whose proportion corresponds to the octave ratio delimiting the frame of the quarter-tone scale (see Figure 44).

Figure 44: Median pair of note values of the triogdoola.

Thus, the diahemitonic appoggiatura must have a length of four beats in octuple metre, being half of the bar, leaving four beats to the main note (see Figure 45).

Figure 45: Diahemitonic appoggiatura in octuple metre.

The pair of note values of the first undenary rhythmic mode is represented numerically as 8 + 3, whose proportion corresponds to the major-fourth ratio at the origin of the quarter-tone scale (see Figure 46).

Figure 46: Pair of note values of the first undenary rhythmic mode.

Thus, the diahemitonic appoggiatura must have a length of eight beats in undecuple metre, being eight elevenths of the bar, leaving three beats to the main note (see Figure 47).

Figure 47: Diahemitonic appoggiatura in undecuple metre.