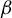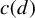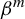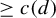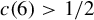Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Miyanohara, Eiji
2024.
A note on non-periodic greedy expansions in Salem base.
Research in Number Theory,
Vol. 10,
Issue. 1,
Hichri, Hachem
and
Smyth, Chris
2024.
The distribution of non-Parry Perron numbers and their conjugates.
Research in Number Theory,
Vol. 10,
Issue. 4,