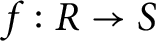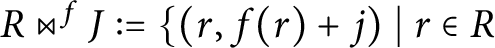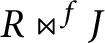1 Introduction
Throughout, let R and S be two commutative rings with unity, let J be a non-zero proper ideal of S and
![]() $f:R\to S$
be a ring homomorphism. D’Anna et al. in [Reference D’Anna, Finocchiaro and Fontana9, Reference D’Anna, Finocchiaro and Fontana10] have introduced the following subring
$f:R\to S$
be a ring homomorphism. D’Anna et al. in [Reference D’Anna, Finocchiaro and Fontana9, Reference D’Anna, Finocchiaro and Fontana10] have introduced the following subring
 $$ \begin{align*}R\bowtie^fJ:=\{(r,f(r)+j)\mid r\in R\text{ and }j\in J\},\end{align*} $$
$$ \begin{align*}R\bowtie^fJ:=\{(r,f(r)+j)\mid r\in R\text{ and }j\in J\},\end{align*} $$
of
![]() $R\times S$
, called the amalgamated algebra (or amalgamation) of R with S along J with respect to f. This construction generalizes the amalgamated duplication of a ring along an ideal (introduced and studied in [Reference D’Anna and Fontana12]). Moreover, several classical constructions such as Nagata’s idealization (cf. [Reference Nagata18, p. 2]), the
$R\times S$
, called the amalgamated algebra (or amalgamation) of R with S along J with respect to f. This construction generalizes the amalgamated duplication of a ring along an ideal (introduced and studied in [Reference D’Anna and Fontana12]). Moreover, several classical constructions such as Nagata’s idealization (cf. [Reference Nagata18, p. 2]), the
![]() $R + XS[X]$
and the
$R + XS[X]$
and the
![]() constructions can be studied as particular cases of this new construction (see [Reference D’Anna, Finocchiaro and Fontana9, Example 2.5 and Remark 2.8]). Amalgamation, in turn, can be realized as a pullback. The construction has proved its worth providing numerous examples and counterexamples in commutative ring theory [Reference Azimi, Sahandi and Shirmihammadi6–Reference Azimi, Sahandi and Shirmohammadi8, Reference D’Anna, Finocchiaro and Fontana11, Reference Finocchiaro13, Reference Sahandi, Shirmohammadi and Sohrabi20].
constructions can be studied as particular cases of this new construction (see [Reference D’Anna, Finocchiaro and Fontana9, Example 2.5 and Remark 2.8]). Amalgamation, in turn, can be realized as a pullback. The construction has proved its worth providing numerous examples and counterexamples in commutative ring theory [Reference Azimi, Sahandi and Shirmihammadi6–Reference Azimi, Sahandi and Shirmohammadi8, Reference D’Anna, Finocchiaro and Fontana11, Reference Finocchiaro13, Reference Sahandi, Shirmohammadi and Sohrabi20].
Recall that a ring R is Hilbert if each proper prime ideal of R is an intersection of maximal ideals of R. Thus, in view of Cohen’s theorem, it is natural to ask if a Hilbert ring which all its maximal ideals are finitely generated is necessarily Noetherian. There are counterexamples for this question, called unruly Hilbert rings. Gilmer and Heinzer, in 1976, constructed an unruly Hilbert ring [Reference Gilmer and Heinzer14]. Then, in [Reference Anderson, Dobbs and Fontana4, Reference Mott and Zafrullah17], the authors used the
![]() $R + XS[X]$
construction and pullbacks to provide a family of unruly Hilbert rings. In this paper we characterize when
$R + XS[X]$
construction and pullbacks to provide a family of unruly Hilbert rings. In this paper we characterize when
![]() $R\bowtie ^f J$
is a Hilbert ring (Theorem 3.3) and, as an application, we give examples of unruly Hilbert rings.
$R\bowtie ^f J$
is a Hilbert ring (Theorem 3.3) and, as an application, we give examples of unruly Hilbert rings.
The simplest examples of Hilbert rings include the polynomial rings over a field and the ring of integers. These rings have the property that all the maximal ideals are of the same height. Therefore, it is interesting to find Hilbert rings with maximal ideals of different heights. Roberts in [Reference Roberts19] and Heinzer in [Reference Heinzer15] constructed interesting examples of this kind. In this paper, we provide an easy way to construct such rings.
The outline of the paper is as follows. In Section 2, we fix our notation and give some elementary results on which we base our approach. In Section 3, we prove our main theorem, which provides a characterization of Hilbert property on the amalgamations (Theorem 3.3). Our attempt results in examples of Hilbert rings with maximal ideals of different heights and unruly Hilbert rings. These examples presented in Section 4.
2 Preliminaries
Let us first fix some notation which we shall use. For a commutative ring A, the set of nilpotent elements, prime ideals, and maximal ideals of A will be denoted by
![]() $\operatorname {Nil} (A)$
,
$\operatorname {Nil} (A)$
,
![]() $\operatorname {Spec} (A)$
, and
$\operatorname {Spec} (A)$
, and
![]() $\operatorname {Max} (A)$
, respectively.
$\operatorname {Max} (A)$
, respectively.
![]() $\operatorname {V}(I)$
denotes the set of prime ideals of A containing I. For a multiplicatively closed subset T of A, we use the notation
$\operatorname {V}(I)$
denotes the set of prime ideals of A containing I. For a multiplicatively closed subset T of A, we use the notation
![]() $T^{-1}A$
to denote the ring of fractions of A with respect to T. In the sequel, we will use the following remark without explicit mention.
$T^{-1}A$
to denote the ring of fractions of A with respect to T. In the sequel, we will use the following remark without explicit mention.
Remark 2.1 [Reference D’Anna, Finocchiaro and Fontana10, Proposition 2.6]
For
![]() $\frak {p}\in \operatorname {Spec}(R)$
and
$\frak {p}\in \operatorname {Spec}(R)$
and
![]() $\frak {q}\in \operatorname {Spec}(S)\backslash \operatorname {V}(J)$
, set
$\frak {q}\in \operatorname {Spec}(S)\backslash \operatorname {V}(J)$
, set
 $$ \begin{align*} \frak{p}^{\prime_f}:= & \frak{p}\bowtie^fJ:=\{(p,f(p)+j)\mid p\in \frak{p}, j\in J\}, \\[1ex] \overline{\frak{q}}^f:= & \{(r,f(r)+j)\mid r\in R, j\in J, f(r)+j\in \frak{q}\}. \end{align*} $$
$$ \begin{align*} \frak{p}^{\prime_f}:= & \frak{p}\bowtie^fJ:=\{(p,f(p)+j)\mid p\in \frak{p}, j\in J\}, \\[1ex] \overline{\frak{q}}^f:= & \{(r,f(r)+j)\mid r\in R, j\in J, f(r)+j\in \frak{q}\}. \end{align*} $$
Then, the following statements hold.
-
•
 $\operatorname {Spec}(R\bowtie ^fJ)=\{\frak {p}^{\prime _f}\mid \frak {p}\in \operatorname {Spec}(R)\}\cup \{\overline {\frak {q}}^f\mid \frak {q}\in \operatorname {Spec}(S)\backslash V(J)\}$
.
$\operatorname {Spec}(R\bowtie ^fJ)=\{\frak {p}^{\prime _f}\mid \frak {p}\in \operatorname {Spec}(R)\}\cup \{\overline {\frak {q}}^f\mid \frak {q}\in \operatorname {Spec}(S)\backslash V(J)\}$
. -
•
 $\operatorname {Max}(R\bowtie ^fJ)=\{\frak {p}^{\prime _f}\mid \frak {p}\in \operatorname {Max}(R)\}\cup \{\overline {\frak {q}}^f\mid \frak {q}\in \operatorname {Max}(S)\backslash V(J)\}$
.
$\operatorname {Max}(R\bowtie ^fJ)=\{\frak {p}^{\prime _f}\mid \frak {p}\in \operatorname {Max}(R)\}\cup \{\overline {\frak {q}}^f\mid \frak {q}\in \operatorname {Max}(S)\backslash V(J)\}$
.
We need the following lemmas in the proof of our main result.
Lemma 2.2 Let
![]() $\frak {p} ,\frak {p}_1, \frak {p}_2\in \operatorname {Spec}(R)$
and
$\frak {p} ,\frak {p}_1, \frak {p}_2\in \operatorname {Spec}(R)$
and
![]() $\frak {q}, \frak {q}_1 ,\frak {q}_2 \in \operatorname {Spec}(S)\backslash \operatorname {V}(J)$
. Then
$\frak {q}, \frak {q}_1 ,\frak {q}_2 \in \operatorname {Spec}(S)\backslash \operatorname {V}(J)$
. Then
-
(1)
 $\frak {p}_1\subseteq \frak {p}_2$
if and only if
$\frak {p}_1\subseteq \frak {p}_2$
if and only if
 $\ \frak {p}_1^{\prime _f}\subseteq \frak {p}_2^{\prime _f}$
.
$\ \frak {p}_1^{\prime _f}\subseteq \frak {p}_2^{\prime _f}$
. -
(2)
 $\frak {q}_1\subseteq \frak {q}_2$
if and only if
$\frak {q}_1\subseteq \frak {q}_2$
if and only if
 $\ \overline {\frak {q}_1}^f\subseteq \overline {\frak {q}_2}^f$
.
$\ \overline {\frak {q}_1}^f\subseteq \overline {\frak {q}_2}^f$
. -
(3)
 $ \overline {\frak {q}}^f \subseteq \frak {p}^{\prime _f}$
if and only if
$ \overline {\frak {q}}^f \subseteq \frak {p}^{\prime _f}$
if and only if
 $f^{-1}(\frak {q} + J) \subseteq \frak {p}$
.
$f^{-1}(\frak {q} + J) \subseteq \frak {p}$
. -
(4)
 $\frak {p}^{\prime _f}\nsubseteq \overline {\frak {q}}^f$
.
$\frak {p}^{\prime _f}\nsubseteq \overline {\frak {q}}^f$
.
Proof (1)–(3) are from [Reference Azimi5, Lemmas 2.2 and 2.3]. To see (4), pick
![]() $j \in J\backslash \frak {q}$
. Then
$j \in J\backslash \frak {q}$
. Then
 $(0,j)\in \frak {p}^{\prime _f}\backslash \overline {\frak {q}}^f$
.▪
$(0,j)\in \frak {p}^{\prime _f}\backslash \overline {\frak {q}}^f$
.▪
Lemma 2.3 Let
![]() $\frak {p},\frak {p}_\alpha \in \operatorname {Spec}(R)$
and
$\frak {p},\frak {p}_\alpha \in \operatorname {Spec}(R)$
and
![]() $\frak {q},\frak {q}_\beta \in \operatorname {Spec}(S)\backslash \operatorname {V}(J)$
;
$\frak {q},\frak {q}_\beta \in \operatorname {Spec}(S)\backslash \operatorname {V}(J)$
;
![]() $\alpha \in \Lambda $
,
$\alpha \in \Lambda $
,
![]() $\beta \in \Delta $
. Then
$\beta \in \Delta $
. Then
-
(1)
 $\frak {p}=\cap _{\alpha \in \Lambda } \frak {p}_\alpha $
if and only if
$\frak {p}=\cap _{\alpha \in \Lambda } \frak {p}_\alpha $
if and only if
 $\frak {p}^{\prime _f}=\cap _{\alpha \in \Lambda } \frak {p}_\alpha ^{\prime _f}$
.
$\frak {p}^{\prime _f}=\cap _{\alpha \in \Lambda } \frak {p}_\alpha ^{\prime _f}$
. -
(2)
 $\frak {q}=\cap _{\beta \in \Delta } \frak {q}_\beta $
if and only if
$\frak {q}=\cap _{\beta \in \Delta } \frak {q}_\beta $
if and only if
 $\overline {\frak {q}}^f=\cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f$
.
$\overline {\frak {q}}^f=\cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f$
.
Proof The proof of (1) follows immediately from definition of
![]() $\frak {p}^{\prime _f}$
in Remark 2.1. The proof of (2) is similar to the proof of [Reference Azimi5, Lemma 2.2], but we include a proof for the convenience of the reader. Note that, by [Reference Azimi5, Lemma 2.2],
$\frak {p}^{\prime _f}$
in Remark 2.1. The proof of (2) is similar to the proof of [Reference Azimi5, Lemma 2.2], but we include a proof for the convenience of the reader. Note that, by [Reference Azimi5, Lemma 2.2],
![]() $\frak {q}\subseteq \cap _{\beta \in \Delta } \frak {q}_\beta $
if and only if
$\frak {q}\subseteq \cap _{\beta \in \Delta } \frak {q}_\beta $
if and only if
 $\overline {\frak {q}}^f\subseteq \cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f$
. It is also clear that if
$\overline {\frak {q}}^f\subseteq \cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f$
. It is also clear that if
![]() $\cap _{\beta \in \Delta } \frak {q}_\beta \subseteq \frak {q}$
, then
$\cap _{\beta \in \Delta } \frak {q}_\beta \subseteq \frak {q}$
, then
 $\cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f \subseteq \overline {\frak {q}}^f$
. Now let
$\cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f \subseteq \overline {\frak {q}}^f$
. Now let
 $\cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f \subseteq \overline {\frak {q}}^f$
, and pick
$\cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f \subseteq \overline {\frak {q}}^f$
, and pick
![]() $y\in \cap _{\beta \in \Delta } \frak {q}_\beta $
. Let
$y\in \cap _{\beta \in \Delta } \frak {q}_\beta $
. Let
![]() $v \in J \backslash \frak {q}$
. Then
$v \in J \backslash \frak {q}$
. Then
 $(0,yv)\in \cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f$
, which implies
$(0,yv)\in \cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f$
, which implies
![]() $yv\in \frak {q}$
by assumption and so
$yv\in \frak {q}$
by assumption and so
![]() $y\in \frak {q}$
.▪
$y\in \frak {q}$
.▪
In order to construct examples of the title, we are required to know when maximal ideals of amalgamations are finitely generated. Here, we collect some elementary properties of this concept.
Lemma 2.4 Let
![]() $\frak {m} \in \operatorname {Spec}(R)$
and
$\frak {m} \in \operatorname {Spec}(R)$
and
![]() $\frak {n} \in \operatorname {Spec}(S)\backslash \operatorname {V}(J)$
. Then
$\frak {n} \in \operatorname {Spec}(S)\backslash \operatorname {V}(J)$
. Then
-
(1) If
 $\frak {m}$
and J are finitely generated, then
$\frak {m}$
and J are finitely generated, then
 $\frak {m}^{\prime _f}$
is finitely generated.
$\frak {m}^{\prime _f}$
is finitely generated. -
(2) If
 $\frak {m} =\langle x \rangle $
is nonzero principal and J is divisible by
$\frak {m} =\langle x \rangle $
is nonzero principal and J is divisible by
 $f(x)$
$f(x)$
 $($
i.e., for any
$($
i.e., for any
 $i\in J$
, there exists
$i\in J$
, there exists
 $j\in J$
such that
$j\in J$
such that
 $i=f(x)j)$
, then
$i=f(x)j)$
, then
 $\frak {m}^{\prime _f}$
is principal.
$\frak {m}^{\prime _f}$
is principal. -
(3) If
 $\frak {m}$
is finitely generated and
$\frak {m}$
is finitely generated and
 $f(\frak {m})S=S$
, then
$f(\frak {m})S=S$
, then
 $\frak {m}^{\prime _f}$
is finitely generated.
$\frak {m}^{\prime _f}$
is finitely generated. -
(4) Assume that f is injective,
 $f^{-1}(J)=0$
, and
$f^{-1}(J)=0$
, and
 $\frak {n}$
is generated by an element of
$\frak {n}$
is generated by an element of
 $f(R)+J=S$
. Then
$f(R)+J=S$
. Then
 $\overline {\frak {n}}^f$
is principal.
$\overline {\frak {n}}^f$
is principal.
Proof For the proof of (1) see the beginning of the proof of [Reference Azimi, Sahandi and Shirmihammadi6, Theorem 4.1]. To prove (2), Let
![]() $\frak {m} =\langle x \rangle $
and pick
$\frak {m} =\langle x \rangle $
and pick
![]() $(r,f(r)+i)\in \frak {m}^{\prime _f}$
. We want to prove that
$(r,f(r)+i)\in \frak {m}^{\prime _f}$
. We want to prove that
![]() $\frak {m}^{\prime _f}= \langle (x,f(x)) \rangle $
. By assumption,
$\frak {m}^{\prime _f}= \langle (x,f(x)) \rangle $
. By assumption,
![]() $r=xa$
and
$r=xa$
and
![]() $i=f(x)j$
, for some
$i=f(x)j$
, for some
![]() $a\in R$
and
$a\in R$
and
![]() $j\in J$
. Then
$j\in J$
. Then
![]() $(r,f(r)+i)= (x,f(x)) (a,f(a)+j)$
, as claimed. (3) is an immediate consequence of [Reference D’Anna, Finocchiaro and Fontana11, Proposition 3.1(3)]. (4): Let
$(r,f(r)+i)= (x,f(x)) (a,f(a)+j)$
, as claimed. (3) is an immediate consequence of [Reference D’Anna, Finocchiaro and Fontana11, Proposition 3.1(3)]. (4): Let
![]() $\frak {n} =\langle f(r)+i \rangle $
. We wish to show that
$\frak {n} =\langle f(r)+i \rangle $
. We wish to show that
 $\overline {\frak {n}}^f= \langle (r,f(r)+i) \rangle $
. To this end, let
$\overline {\frak {n}}^f= \langle (r,f(r)+i) \rangle $
. To this end, let
 $(x,f(x)+j)\in \overline {\frak {n}}^f$
. One can write
$(x,f(x)+j)\in \overline {\frak {n}}^f$
. One can write
![]() $f(x)+j= (f(r)+i)(f(s)+k)$
, for some
$f(x)+j= (f(r)+i)(f(s)+k)$
, for some
![]() $f(s)+k \in f(R)+J$
. Then
$f(s)+k \in f(R)+J$
. Then
![]() $f(x)-f(r)f(s)\in J$
, which implies
$f(x)-f(r)f(s)\in J$
, which implies
![]() $x=rs$
. Hence
$x=rs$
. Hence
![]() $(x,f(x)+j)= (r,f(r)+i)(s,f(s)+k)$
.▪
$(x,f(x)+j)= (r,f(r)+i)(s,f(s)+k)$
.▪
3 Main result
In this section, we investigate when the amalgamated algebra
![]() $R\bowtie ^f J$
is a Hilbert ring. Then we present several corollaries that recover or generalize previous works. Let us first introduce the concept of Hilbert condition for an arbitrary subset of
$R\bowtie ^f J$
is a Hilbert ring. Then we present several corollaries that recover or generalize previous works. Let us first introduce the concept of Hilbert condition for an arbitrary subset of
![]() $\operatorname {Spec} (R)$
.
$\operatorname {Spec} (R)$
.
Definition 3.1 Let
![]() $X \subseteq \operatorname {Spec} (R)$
. We call X Hilbert if each proper prime ideal
$X \subseteq \operatorname {Spec} (R)$
. We call X Hilbert if each proper prime ideal
![]() $P\in X$
is an intersection of elements of
$P\in X$
is an intersection of elements of
![]() $\operatorname {Max} (R) \cap X$
. We simply call R Hilbert if
$\operatorname {Max} (R) \cap X$
. We simply call R Hilbert if
![]() $\operatorname {Spec} (R)$
is Hilbert.
$\operatorname {Spec} (R)$
is Hilbert.
Note that R is Hilbert in the usual sense, if it is Hilbert in the sense of above definition. For
![]() $X \subseteq \operatorname {Spec} (R)$
, when we write that “
$X \subseteq \operatorname {Spec} (R)$
, when we write that “
![]() $\widehat {X}$
is Hilbert,” it is to be understood that each
$\widehat {X}$
is Hilbert,” it is to be understood that each
![]() $P\in X$
is an intersection of elements of
$P\in X$
is an intersection of elements of
![]() $\operatorname {Max} (R)$
(all maximal ideals of the ring not only those who are in X). We shall need the following well-known fact (see, e.g., [Reference Kaplansky16, Theorem 31]).
$\operatorname {Max} (R)$
(all maximal ideals of the ring not only those who are in X). We shall need the following well-known fact (see, e.g., [Reference Kaplansky16, Theorem 31]).
Lemma 3.2 For a ring R and indeterminates
![]() $X_1,\ldots ,X_n$
over R, the following conditions are equivalent
$X_1,\ldots ,X_n$
over R, the following conditions are equivalent
![]() $:$
$:$
-
(1) R is a Hilbert ring.
-
(2)
 $R/I$
is a Hilbert ring for each proper ideal I of R.
$R/I$
is a Hilbert ring for each proper ideal I of R. -
(3)
 $R[X_1,\ldots ,X_n]$
is a Hilbert ring.
$R[X_1,\ldots ,X_n]$
is a Hilbert ring.
The following theorem is the main result of this section.
Theorem 3.3 The following statements are equivalent
![]() $:$
$:$
-
(1)
 $R\bowtie ^f J$
is a Hilbert ring.
$R\bowtie ^f J$
is a Hilbert ring. -
(2) R and
 $\operatorname {Spec} (S)\backslash \operatorname {V}(J)$
are Hilbert.
$\operatorname {Spec} (S)\backslash \operatorname {V}(J)$
are Hilbert. -
(3) R and
 $\widehat {\operatorname {Spec} (S)\backslash \operatorname {V}(J)}$
are Hilbert.
$\widehat {\operatorname {Spec} (S)\backslash \operatorname {V}(J)}$
are Hilbert.
Proof (1)
![]() $\Rightarrow $
(2) Assume that
$\Rightarrow $
(2) Assume that
![]() $R\bowtie ^f J$
is a Hilbert ring. Then, by Lemma 3.2, R is also a Hilbert ring since it is a homomorphic image of
$R\bowtie ^f J$
is a Hilbert ring. Then, by Lemma 3.2, R is also a Hilbert ring since it is a homomorphic image of
![]() $R\bowtie ^f J$
. Next, let
$R\bowtie ^f J$
. Next, let
![]() $Q\in \operatorname {Spec} (S)\backslash \operatorname {V}(J)$
. Then
$Q\in \operatorname {Spec} (S)\backslash \operatorname {V}(J)$
. Then
 $\overline {Q}^f$
is a prime ideal of the Hilbert ring
$\overline {Q}^f$
is a prime ideal of the Hilbert ring
![]() $R\bowtie ^f J$
, and so
$R\bowtie ^f J$
, and so
 $\overline {Q}^f=(\cap _{\alpha \in \Lambda } \frak {p}_\alpha ^{\prime _f})\cap (\cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f)$
, for suitable families
$\overline {Q}^f=(\cap _{\alpha \in \Lambda } \frak {p}_\alpha ^{\prime _f})\cap (\cap _{\beta \in \Delta } \overline {\frak {q}_\beta }^f)$
, for suitable families
![]() $\frak {p}_\alpha \in \operatorname {Max} (R)$
and
$\frak {p}_\alpha \in \operatorname {Max} (R)$
and
![]() $\frak {q}_\beta \in \operatorname {Max} (S)\backslash \operatorname {V}(J)$
. The proof is completed by showing that
$\frak {q}_\beta \in \operatorname {Max} (S)\backslash \operatorname {V}(J)$
. The proof is completed by showing that
![]() $Q=\cap _{\beta \in \Delta } \frak {q}_\beta $
. From Lemma 2.2, we have
$Q=\cap _{\beta \in \Delta } \frak {q}_\beta $
. From Lemma 2.2, we have
![]() $Q\subseteq \cap _{\beta \in \Delta } \frak {q}_\beta $
. To see the converse inclusion, let
$Q\subseteq \cap _{\beta \in \Delta } \frak {q}_\beta $
. To see the converse inclusion, let
![]() $x\in \cap _{\beta \in \Delta } \frak {q}_\beta $
and pick
$x\in \cap _{\beta \in \Delta } \frak {q}_\beta $
and pick
![]() $v\in J\backslash Q$
. Hence, for all
$v\in J\backslash Q$
. Hence, for all
![]() $\beta \in \Delta $
we have
$\beta \in \Delta $
we have
![]() $xv\in \frak {q}_\beta \cap J$
, hence that
$xv\in \frak {q}_\beta \cap J$
, hence that
 $(0,xv)\in \overline {\frak {q}_\beta }^f$
. Note that for all
$(0,xv)\in \overline {\frak {q}_\beta }^f$
. Note that for all
![]() $\frak {p} \in \operatorname {Spec} (R)$
, we always have
$\frak {p} \in \operatorname {Spec} (R)$
, we always have
![]() $(0,xv)\in \frak {p}^{\prime _f}$
. It follows that
$(0,xv)\in \frak {p}^{\prime _f}$
. It follows that
 $(0,xv)\in \overline {Q}^f$
, which implies
$(0,xv)\in \overline {Q}^f$
, which implies
![]() $xv\in Q$
and so
$xv\in Q$
and so
![]() $x\in Q$
.
$x\in Q$
.
(2)
![]() $\Rightarrow $
(3) is trivial.
$\Rightarrow $
(3) is trivial.
(3)
![]() $\Rightarrow $
(1) Assume that R and
$\Rightarrow $
(1) Assume that R and
 $\widehat {\operatorname {Spec} (S)\backslash \operatorname {V}(J)}$
are Hilbert, and let
$\widehat {\operatorname {Spec} (S)\backslash \operatorname {V}(J)}$
are Hilbert, and let
![]() $\mathcal {P} \in \operatorname {Spec} (R\bowtie ^f J)$
. If
$\mathcal {P} \in \operatorname {Spec} (R\bowtie ^f J)$
. If
![]() $\mathcal {P} = \frak {p}^{\prime _f}$
, for some
$\mathcal {P} = \frak {p}^{\prime _f}$
, for some
![]() $\frak {p} \in \operatorname {Spec} (R)$
, then
$\frak {p} \in \operatorname {Spec} (R)$
, then
![]() $\frak {p}$
is an intersection of maximal ideals, and hence the same is true for
$\frak {p}$
is an intersection of maximal ideals, and hence the same is true for
![]() $\frak {p}^{\prime _f}$
, by Lemma 2.3. Next, let
$\frak {p}^{\prime _f}$
, by Lemma 2.3. Next, let
 $\mathcal {P} = \overline {\frak {q}}^f$
, for some
$\mathcal {P} = \overline {\frak {q}}^f$
, for some
![]() $\frak {q} \in \operatorname {Spec} (S)\backslash \operatorname {V}(J)$
. By the Hilbert assumption on
$\frak {q} \in \operatorname {Spec} (S)\backslash \operatorname {V}(J)$
. By the Hilbert assumption on
 $\widehat {\operatorname {Spec} (S)\backslash \operatorname {V}(J)}$
, we have
$\widehat {\operatorname {Spec} (S)\backslash \operatorname {V}(J)}$
, we have
![]() $q=(\cap _{\alpha \in \Lambda } \frak {m}_\alpha )\cap (\cap _{\beta \in \Delta } \frak {n}_\beta )$
, for suitable families
$q=(\cap _{\alpha \in \Lambda } \frak {m}_\alpha )\cap (\cap _{\beta \in \Delta } \frak {n}_\beta )$
, for suitable families
![]() $\frak {m}_\alpha \in \operatorname {Max} (S)\cap \operatorname {V} (J)$
and
$\frak {m}_\alpha \in \operatorname {Max} (S)\cap \operatorname {V} (J)$
and
![]() $\frak {n}_\beta \in \operatorname {Max} (S)\backslash \operatorname {V} (J)$
. Since R is a Hilbert ring, for any
$\frak {n}_\beta \in \operatorname {Max} (S)\backslash \operatorname {V} (J)$
. Since R is a Hilbert ring, for any
![]() $\alpha \in \Lambda $
we have
$\alpha \in \Lambda $
we have
![]() $f^{-1}(\frak {m}_\alpha )=\cap _{\gamma \in \Gamma } M_{\alpha \gamma }$
for a suitable families
$f^{-1}(\frak {m}_\alpha )=\cap _{\gamma \in \Gamma } M_{\alpha \gamma }$
for a suitable families
![]() $M_{\alpha \gamma }\in \operatorname {Max} (R)$
, which implies
$M_{\alpha \gamma }\in \operatorname {Max} (R)$
, which implies
 $\left ( f^{-1}(\frak {m}_\alpha )\right )^{\prime _f}=\cap _{\gamma \in \Gamma } \left ( M_{\alpha \gamma }\right )^{\prime _f}$
(Lemma 2.3). Thus, if we prove that
$\left ( f^{-1}(\frak {m}_\alpha )\right )^{\prime _f}=\cap _{\gamma \in \Gamma } \left ( M_{\alpha \gamma }\right )^{\prime _f}$
(Lemma 2.3). Thus, if we prove that
 $\overline {q}^f=(\cap _{\alpha \in \Lambda } \left ( f^{-1}(\frak {m}_\alpha )\right )^{\prime _f})\cap (\cap _{\beta \in \Delta } \overline {\frak {n}_\beta }^f)$
, the assertion follows. This we do.
$\overline {q}^f=(\cap _{\alpha \in \Lambda } \left ( f^{-1}(\frak {m}_\alpha )\right )^{\prime _f})\cap (\cap _{\beta \in \Delta } \overline {\frak {n}_\beta }^f)$
, the assertion follows. This we do.
It follows from Lemma 2.2(3) that
 $\overline {q}^f\subseteq \cap _{\alpha \in \Lambda } \left ( f^{-1}(\frak {m}_\alpha )\right )^{\prime _f}$
and from Lemma 2.2(2) that
$\overline {q}^f\subseteq \cap _{\alpha \in \Lambda } \left ( f^{-1}(\frak {m}_\alpha )\right )^{\prime _f}$
and from Lemma 2.2(2) that
 $\overline {q}^f\subseteq \cap _{\beta \in \Delta } \overline {\frak {n}_\beta }^f$
. To see the converse inclusion, let
$\overline {q}^f\subseteq \cap _{\beta \in \Delta } \overline {\frak {n}_\beta }^f$
. To see the converse inclusion, let
 $(r,f(r)+i)\in (\cap _{\alpha \in \Lambda } \left ( f^{-1}(\frak {m}_\alpha )\right )^{\prime _f})\cap (\cap _{\beta \in \Delta } \overline {\frak {n}_\beta }^f)$
. Then, for all
$(r,f(r)+i)\in (\cap _{\alpha \in \Lambda } \left ( f^{-1}(\frak {m}_\alpha )\right )^{\prime _f})\cap (\cap _{\beta \in \Delta } \overline {\frak {n}_\beta }^f)$
. Then, for all
![]() $\alpha \in \Lambda $
and
$\alpha \in \Lambda $
and
![]() $\beta \in \Delta $
, we have
$\beta \in \Delta $
, we have
![]() $r\in f^{-1}(\frak {m}_\alpha )$
and
$r\in f^{-1}(\frak {m}_\alpha )$
and
![]() $f(r)+i \in \frak {n}_\beta $
. Hence
$f(r)+i \in \frak {n}_\beta $
. Hence
![]() $f(r)+i \in (\cap _{\alpha \in \Lambda } \frak {m}_\alpha )\cap (\cap _{\beta \in \Delta } \frak {n}_\beta )=\frak {q}$
, which implies
$f(r)+i \in (\cap _{\alpha \in \Lambda } \frak {m}_\alpha )\cap (\cap _{\beta \in \Delta } \frak {n}_\beta )=\frak {q}$
, which implies
 $(r,f(r)+i)\in \overline {\frak {q}}^f$
.▪
$(r,f(r)+i)\in \overline {\frak {q}}^f$
.▪
Recall that if
![]() $f:=id_R$
is the identity homomorphism on R, and I is an ideal of R, then
$f:=id_R$
is the identity homomorphism on R, and I is an ideal of R, then
![]() $R\bowtie I:=R\bowtie ^{id_R} I$
is called the amalgamated duplication of R along I.
$R\bowtie I:=R\bowtie ^{id_R} I$
is called the amalgamated duplication of R along I.
Corollary 3.4 Let I be an ideal of R. Then
![]() $R\bowtie I$
is a Hilbert ring if and only if so is R.
$R\bowtie I$
is a Hilbert ring if and only if so is R.
In the following, we observe what happens if we let J be too small or too big.
Corollary 3.5 The following hold
![]() $:$
$:$
-
(1) Let
 $J\subseteq \operatorname {Nil} (S)$
. Then
$J\subseteq \operatorname {Nil} (S)$
. Then
 $R\bowtie ^f J$
is Hilbert if and only if so is R.
$R\bowtie ^f J$
is Hilbert if and only if so is R. -
(2) Let J be a maximal ideal of S. Then
 $R\bowtie ^f J$
is a Hilbert ring if and only if so are R and S.
$R\bowtie ^f J$
is a Hilbert ring if and only if so are R and S.
Proof In the first case
![]() $\operatorname {Spec} (S)\backslash \operatorname {V}(J)=\phi $
, and in the second case
$\operatorname {Spec} (S)\backslash \operatorname {V}(J)=\phi $
, and in the second case
![]() $\operatorname {Spec} (S)\backslash \operatorname {V}(J)=\operatorname {Spec} (S)\backslash \{J\}$
. The assertion follows from Theorem 3.3.▪
$\operatorname {Spec} (S)\backslash \operatorname {V}(J)=\operatorname {Spec} (S)\backslash \{J\}$
. The assertion follows from Theorem 3.3.▪
Let M (respectively,
![]() $N=(M_i)_{i=1}^n$
) be an R-module (respectively, a family of R-modules). Then
$N=(M_i)_{i=1}^n$
) be an R-module (respectively, a family of R-modules). Then
![]() $R\ltimes M$
(respectively,
$R\ltimes M$
(respectively,
![]() $R\ltimes _nN$
) denotes the trivial extension of R by M (respectively, the n-trivial extension of R by N). It should be noted that both constructions are special cases of amalgamation with
$R\ltimes _nN$
) denotes the trivial extension of R by M (respectively, the n-trivial extension of R by N). It should be noted that both constructions are special cases of amalgamation with
![]() $J^n=0$
(for definition and more details, see [Reference Anderson, Bennis, Fahid and Shaiea2, Reference Azimi, Sahandi and Shirmihammadi7, Reference D’Anna, Finocchiaro and Fontana9]). Hence the next result follows from the first part of Corollary 3.5.
$J^n=0$
(for definition and more details, see [Reference Anderson, Bennis, Fahid and Shaiea2, Reference Azimi, Sahandi and Shirmihammadi7, Reference D’Anna, Finocchiaro and Fontana9]). Hence the next result follows from the first part of Corollary 3.5.
Corollary 3.6 Let M be an R-module and
![]() $N=(M_i)_{i=1}^n$
be a family of R-modules. Then the following hold
$N=(M_i)_{i=1}^n$
be a family of R-modules. Then the following hold
![]() $:$
$:$
-
(1)
 $R\ltimes M$
is a Hilbert ring if and only if so is R.
$R\ltimes M$
is a Hilbert ring if and only if so is R. -
(2)
 $R\ltimes _n N$
is a Hilbert ring if and only if so is R.
$R\ltimes _n N$
is a Hilbert ring if and only if so is R.
Corollary 3.7 [Reference Anderson, Dobbs and Fontana4, Corollary 6]
Let M be a maximal ideal of a ring T and let D be a subring of T such that
![]() $M\cap D=(0)$
. Then
$M\cap D=(0)$
. Then
![]() $D + M$
is a Hilbert ring if and only if D and T are Hilbert rings.
$D + M$
is a Hilbert ring if and only if D and T are Hilbert rings.
Proof
![]() $D+M\cong D\bowtie ^\iota M$
, where
$D+M\cong D\bowtie ^\iota M$
, where
![]() $\iota : D\hookrightarrow T$
is the natural embedding. The result now follows from Corollary 3.5(2).▪
$\iota : D\hookrightarrow T$
is the natural embedding. The result now follows from Corollary 3.5(2).▪
Let
![]() $\alpha :A\to C , \beta : B \to C$
be ring homomorphisms. The subring
$\alpha :A\to C , \beta : B \to C$
be ring homomorphisms. The subring
![]() $D := \alpha \times _C \beta := \{ (a,b) \in A \times B \mid \alpha (a) = \beta (b) \}$
of
$D := \alpha \times _C \beta := \{ (a,b) \in A \times B \mid \alpha (a) = \beta (b) \}$
of
![]() $A \times B$
is called the pullback of
$A \times B$
is called the pullback of
![]() $\alpha $
and
$\alpha $
and
![]() $\beta $
. Note that the amalgamation can be studied in the framework of pullback constructions. In fact, if
$\beta $
. Note that the amalgamation can be studied in the framework of pullback constructions. In fact, if
![]() $\pi : S \to S/J$
is the canonical projection and
$\pi : S \to S/J$
is the canonical projection and
 $\check {f}= \pi \circ f$
, then
$\check {f}= \pi \circ f$
, then
 $R\bowtie ^f J = \check {f} \times _{S/J} \pi $
.
$R\bowtie ^f J = \check {f} \times _{S/J} \pi $
.
Assuming A is a subring of C and
![]() $\beta $
is surjective, Anderson et al. prove that D and C are Hilbert rings if and only if A and B are Hilbert rings [Reference Anderson, Dobbs and Fontana4, Theorem 3]. The following Proposition removes the assumption that A is a subring of C, in the case of amalgamations.
$\beta $
is surjective, Anderson et al. prove that D and C are Hilbert rings if and only if A and B are Hilbert rings [Reference Anderson, Dobbs and Fontana4, Theorem 3]. The following Proposition removes the assumption that A is a subring of C, in the case of amalgamations.
Proposition 3.8
![]() $R\bowtie ^f J$
and
$R\bowtie ^f J$
and
![]() $S/J$
are Hilbert rings if and only if so are R and S.
$S/J$
are Hilbert rings if and only if so are R and S.
Proof Assume that
![]() $R\bowtie ^f J$
and
$R\bowtie ^f J$
and
![]() $S/J$
are Hilbert rings. Then, by (the proof of) Theorem 3.3, R is Hilbert and any
$S/J$
are Hilbert rings. Then, by (the proof of) Theorem 3.3, R is Hilbert and any
![]() $Q\in \operatorname {Spec} (S)\backslash \operatorname {V}(J)$
is an intersection of maximal ideals of S. On the other hand, if
$Q\in \operatorname {Spec} (S)\backslash \operatorname {V}(J)$
is an intersection of maximal ideals of S. On the other hand, if
![]() $Q\in \operatorname {Spec} (S)\cap \operatorname {V}(J)$
, then
$Q\in \operatorname {Spec} (S)\cap \operatorname {V}(J)$
, then
![]() $Q/J$
is an intersection of maximal ideals of
$Q/J$
is an intersection of maximal ideals of
![]() $S/J$
and so Q is an intersection of maximal ideals of S, as desired. The converse is clear by Theorem 3.3 and Lemma 3.2.▪
$S/J$
and so Q is an intersection of maximal ideals of S, as desired. The converse is clear by Theorem 3.3 and Lemma 3.2.▪
In [Reference Mott and Zafrullah17, Theorem 5], assuming that R is a Hilbert domain contained in the field S, the authors prove that
![]() $R+XS[X]$
is a Hilbert domain. Then [Reference Anderson, Dobbs and Fontana4, Corollary 4] generalizes this result as follows. Let
$R+XS[X]$
is a Hilbert domain. Then [Reference Anderson, Dobbs and Fontana4, Corollary 4] generalizes this result as follows. Let
![]() $X_1,\ldots ,X_n$
be finitely many indeterminates over a ring E, and let D be a subring of E. Then
$X_1,\ldots ,X_n$
be finitely many indeterminates over a ring E, and let D be a subring of E. Then
![]() $D+(X_1,\ldots ,X_n) E[X_1,\ldots ,X_n]$
is a Hilbert ring if and only if D and E are Hilbert rings. We proceed with a slight generalization of these results.
$D+(X_1,\ldots ,X_n) E[X_1,\ldots ,X_n]$
is a Hilbert ring if and only if D and E are Hilbert rings. We proceed with a slight generalization of these results.
Corollary 3.9 Let
![]() $\textbf{X}:= \{X_1,\ldots ,X_n\}$
be a finite set of indeterminates over S. Then
$\textbf{X}:= \{X_1,\ldots ,X_n\}$
be a finite set of indeterminates over S. Then
![]() $f(R)+\textbf{X}S[\textbf{X}]$
is a Hilbert ring if and only if so are R and S. In particular, if R is a subring of S, then
$f(R)+\textbf{X}S[\textbf{X}]$
is a Hilbert ring if and only if so are R and S. In particular, if R is a subring of S, then
![]() $R + \textbf{X}S[\textbf{X}]$
is a Hilbert ring if and only if so are R and S.
$R + \textbf{X}S[\textbf{X}]$
is a Hilbert ring if and only if so are R and S.
Proof Let
![]() $\varphi $
be the composition homomorphism
$\varphi $
be the composition homomorphism
 $\varphi : R \xrightarrow {f} S \hookrightarrow S[\textbf{X}],$
and let
$\varphi : R \xrightarrow {f} S \hookrightarrow S[\textbf{X}],$
and let
![]() $J:=\textbf{X}S[\textbf{X}]$
. Then, by [Reference D’Anna, Finocchiaro and Fontana9, Proposition 5.1(3)],
$J:=\textbf{X}S[\textbf{X}]$
. Then, by [Reference D’Anna, Finocchiaro and Fontana9, Proposition 5.1(3)],
![]() $R \bowtie ^{\varphi } J \cong f(R) + \textbf{X}S[\textbf{X}]$
. Therefore, by Proposition 3.8 and and Lemma 3.2,
$R \bowtie ^{\varphi } J \cong f(R) + \textbf{X}S[\textbf{X}]$
. Therefore, by Proposition 3.8 and and Lemma 3.2,
![]() $f(R)+\textbf{X}S[\textbf{X}]$
and S are Hilbert rings if and only if so are R and S. Observe that S is a homomorphic image of
$f(R)+\textbf{X}S[\textbf{X}]$
and S are Hilbert rings if and only if so are R and S. Observe that S is a homomorphic image of
![]() $f(R)+\textbf{X}S[\textbf{X}]$
(via evaluation at n-tuple
$f(R)+\textbf{X}S[\textbf{X}]$
(via evaluation at n-tuple
![]() $1$
) and the result follows.▪
$1$
) and the result follows.▪
Let R be an integral domain and T be a multiplicatively closed subset of R. In [Reference Anderson, Anderson and Zafrullah1, Theorem 4.1], under certain conditions, the authors characterize when
![]() $R+XR_T[X]$
is a Hilbert ring and conjectured that the conditions on T are not necessary. This conjecture is proved in [Reference Anderson, Dobbs and Fontana4, Corollary 5] in a more general case; only assuming that each element of T is a nonzerodivisor. As an applications of Corollary 3.9, we now drop this assumption too. In the following,
$R+XR_T[X]$
is a Hilbert ring and conjectured that the conditions on T are not necessary. This conjecture is proved in [Reference Anderson, Dobbs and Fontana4, Corollary 5] in a more general case; only assuming that each element of T is a nonzerodivisor. As an applications of Corollary 3.9, we now drop this assumption too. In the following,
![]() $g:R \to R_T$
stands for the canonical homomorphism.
$g:R \to R_T$
stands for the canonical homomorphism.
Corollary 3.10 Let
![]() $\textbf{X}:= \{X_1,\ldots ,X_n\}$
be a finite set of indeterminates over R, and T be a multiplicatively closed subset of R. Then
$\textbf{X}:= \{X_1,\ldots ,X_n\}$
be a finite set of indeterminates over R, and T be a multiplicatively closed subset of R. Then
![]() $g (R)+\textbf{X}R_T[\textbf{X}]$
is a Hilbert ring if and only if R and
$g (R)+\textbf{X}R_T[\textbf{X}]$
is a Hilbert ring if and only if R and
![]() $R_T$
are Hilbert rings. In particular, if each element of T is a nonzerodivisor, then
$R_T$
are Hilbert rings. In particular, if each element of T is a nonzerodivisor, then
![]() $R+\textbf{X}R_T[\textbf{X}]$
is a Hilbert ring if and only if R and
$R+\textbf{X}R_T[\textbf{X}]$
is a Hilbert ring if and only if R and
![]() $R_T$
are Hilbert rings.
$R_T$
are Hilbert rings.
4 Examples
In this section, we give examples of the rings of the title. We first present unruly Hilbert rings using previously known rings of this kind [Reference Anderson, Dobbs and Fontana4, Reference Gilmer and Heinzer14, Reference Mott and Zafrullah17]. Then we construct new original examples. Finally, we construct a Hilbert ring with maximal ideals of different heights.
Example 4.1 Let R be an unruly Hilbert ring and M be a finitely generated R-module. By Corollary 3.6,
![]() $R\ltimes M$
is Hilbert, while [Reference Anderson and Winders3, Theorem 4.8] shows that it is not Noetherian. It follows from Lemma 2.4(1) that each maximal ideal of
$R\ltimes M$
is Hilbert, while [Reference Anderson and Winders3, Theorem 4.8] shows that it is not Noetherian. It follows from Lemma 2.4(1) that each maximal ideal of
![]() $R\ltimes M$
is finitely generated. Therefore
$R\ltimes M$
is finitely generated. Therefore
![]() $R\ltimes M$
is an unruly Hilbert ring.
$R\ltimes M$
is an unruly Hilbert ring.
Example 4.2 Let R be an unruly Hilbert ring with
![]() $0 \neq a \in \operatorname {Nil} (R)$
. Then
$0 \neq a \in \operatorname {Nil} (R)$
. Then
![]() $R\bowtie \langle a\rangle $
is an unruly Hilbert ring, by [Reference D’Anna, Finocchiaro and Fontana9, Proposition 5.6], Theorem 3.3, and Lemma 2.4(1).
$R\bowtie \langle a\rangle $
is an unruly Hilbert ring, by [Reference D’Anna, Finocchiaro and Fontana9, Proposition 5.6], Theorem 3.3, and Lemma 2.4(1).
To construct unruly Hilbert rings, one should consider the following questions:
-
• When is
 $R\bowtie ^f J$
a Noetherian ring?
$R\bowtie ^f J$
a Noetherian ring? -
• When is
 $R\bowtie ^f J$
a Hilbert ring?
$R\bowtie ^f J$
a Hilbert ring? -
• When are all maximal ideals of
 $R\bowtie ^f J$
finitely generated?
$R\bowtie ^f J$
finitely generated?
The first question is completely answered in [Reference D’Anna, Finocchiaro and Fontana9, Proposition 5.6] and the second one in Theorem 3.3 of this paper. The third question has a partial answer in Lemma 2.4. Before we proceed with new examples, it is worth to notice that the key for further examples via amalgamation is deepening of the answers for third question. In view of Theorem 3.3 and [Reference D’Anna, Finocchiaro and Fontana9, Proposition 5.6], one needs to answer the third question when R is Noetherian and
![]() $f(R)+J$
is not. The next example is a special case of [Reference Mott and Zafrullah17, Corollary 7].
$f(R)+J$
is not. The next example is a special case of [Reference Mott and Zafrullah17, Corollary 7].
Example 4.3
![]() $\mathbb {Z}+ X\mathbb {Q}[X]$
is an unruly Hilbert domain. Indeed, if we set
$\mathbb {Z}+ X\mathbb {Q}[X]$
is an unruly Hilbert domain. Indeed, if we set
![]() $R=\mathbb {Z}$
,
$R=\mathbb {Z}$
,
![]() $S=\mathbb {Q}[X]$
,
$S=\mathbb {Q}[X]$
,
![]() $J=X\mathbb {Q}[X]$
, and f be the natural inclusion, then
$J=X\mathbb {Q}[X]$
, and f be the natural inclusion, then
![]() $R\bowtie ^f J \cong \mathbb {Z}+ X\mathbb {Q}[X]$
. Note that, by Corollary 3.5 (or Corollary 3.10),
$R\bowtie ^f J \cong \mathbb {Z}+ X\mathbb {Q}[X]$
. Note that, by Corollary 3.5 (or Corollary 3.10),
![]() $\mathbb {Z}+ X\mathbb {Q}[X]$
is a Hilbert domain, while it follows from [Reference D’Anna, Finocchiaro and Fontana9, Corollary 5.9] that it is not Noetherian. Finally, Lemma 2.4(2),(4) implies that each maximal ideal of
$\mathbb {Z}+ X\mathbb {Q}[X]$
is a Hilbert domain, while it follows from [Reference D’Anna, Finocchiaro and Fontana9, Corollary 5.9] that it is not Noetherian. Finally, Lemma 2.4(2),(4) implies that each maximal ideal of
![]() $\mathbb {Z}+ X\mathbb {Q}[X]$
is finitely generated (In fact, the maximal ideals are principal since
$\mathbb {Z}+ X\mathbb {Q}[X]$
is finitely generated (In fact, the maximal ideals are principal since
![]() $\mathbb {Z}+ X\mathbb {Q}[X]$
is a Bézout domain). Therefore,
$\mathbb {Z}+ X\mathbb {Q}[X]$
is a Bézout domain). Therefore,
![]() $R\bowtie ^f J$
is an unruly Hilbert domain.
$R\bowtie ^f J$
is an unruly Hilbert domain.
References [Reference Heinzer15, Reference Roberts19] are devoted to construct examples of Hilbert rings with maximal ideals of different heights (1970s). Theorem 3.3 provides a very easy example with this property. The following example is one of the main results of this paper.
Example 4.4 Let k be a field and
![]() $X,Y$
be indeterminates over k. Let
$X,Y$
be indeterminates over k. Let
![]() $R=k[X,Y]$
,
$R=k[X,Y]$
,
![]() $S=k[X]$
,
$S=k[X]$
,
![]() $J=\langle X^2\rangle $
, and f be the natural surjection. Take
$J=\langle X^2\rangle $
, and f be the natural surjection. Take
![]() $\frak {m} = \langle X,Y\rangle $
and
$\frak {m} = \langle X,Y\rangle $
and
![]() $\frak {n}=\langle X-1\rangle $
. Then, by Theorem 3.3,
$\frak {n}=\langle X-1\rangle $
. Then, by Theorem 3.3,
![]() $R\bowtie ^f J$
is a Hilbert ring (of dimension
$R\bowtie ^f J$
is a Hilbert ring (of dimension
![]() $2$
, by [Reference D’Anna, Finocchiaro and Fontana10, Proposition 4.1]). It is easy to see that
$2$
, by [Reference D’Anna, Finocchiaro and Fontana10, Proposition 4.1]). It is easy to see that
 $\operatorname {\textrm{ht}} (\overline {\frak {n}}^f)=1$
. Indeed, by Lemma 2.2,
$\operatorname {\textrm{ht}} (\overline {\frak {n}}^f)=1$
. Indeed, by Lemma 2.2,
 $\operatorname {\textrm{ht}} (\overline {\frak {n}}^f)\leq 1$
and we have the sequence
$\operatorname {\textrm{ht}} (\overline {\frak {n}}^f)\leq 1$
and we have the sequence
 $\overline {0}^f \subsetneq \overline {\frak {n}}^f$
of prime ideals which implies
$\overline {0}^f \subsetneq \overline {\frak {n}}^f$
of prime ideals which implies
 $\operatorname {\textrm{ht}} (\overline {\frak {n}}^f)\geq 1$
, as desired. Another use of Lemma 2.2 shows that
$\operatorname {\textrm{ht}} (\overline {\frak {n}}^f)\geq 1$
, as desired. Another use of Lemma 2.2 shows that
![]() $\operatorname {\textrm{ht}} (\frak {m}^{\prime _f})=2$
, since
$\operatorname {\textrm{ht}} (\frak {m}^{\prime _f})=2$
, since
![]() $0^{\prime _f}\subsetneq \langle X\rangle ^{\prime _f} \subsetneq \frak {m}^{\prime _f}$
is a sequence of prime ideals of length
$0^{\prime _f}\subsetneq \langle X\rangle ^{\prime _f} \subsetneq \frak {m}^{\prime _f}$
is a sequence of prime ideals of length
![]() $2$
.
$2$
.
Acknowledgment
The author is grateful to the referee for his/her careful reading of the paper and valuable suggestions.