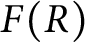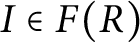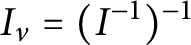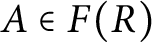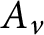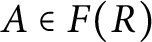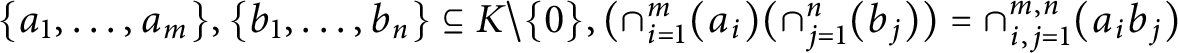1 Introduction
Let R be an integral domain with quotient field K, and let
![]() $F(R)$
be the set of nonzero fractional ideals of R. Call R a dually compact domain (DCD) if, for each set,
$F(R)$
be the set of nonzero fractional ideals of R. Call R a dually compact domain (DCD) if, for each set,
![]() $\{a_{\alpha }\}_{\alpha \in I} \subseteq K\backslash \{0\}$
with
$\{a_{\alpha }\}_{\alpha \in I} \subseteq K\backslash \{0\}$
with
![]() $\cap a_{\alpha }R\neq (0)$
, there is a finite set of elements
$\cap a_{\alpha }R\neq (0)$
, there is a finite set of elements
![]() $\{x_{1},\ldots ,x_{r}\}\subseteq K\backslash \{0\}$
such that
$\{x_{1},\ldots ,x_{r}\}\subseteq K\backslash \{0\}$
such that
![]() $\cap a_{\alpha }R=\cap _{i=1}^{r}x_{i}R$
, or equivalently, for each
$\cap a_{\alpha }R=\cap _{i=1}^{r}x_{i}R$
, or equivalently, for each
![]() $ I\in F(R)$
, the ideal
$ I\in F(R)$
, the ideal
![]() $I_{v}=(I^{-1})^{-1}$
is a finite intersection of principal fractional ideals of R. We characterize DCDs (in Section 3) and show that the class of DCDs properly contains various classes of integral domains of import, such as Noetherian, Mori, and Krull domains, in Section 4. In addition, we show that a pre-Schreier DCD is a GCD domain with the property that, for each
$I_{v}=(I^{-1})^{-1}$
is a finite intersection of principal fractional ideals of R. We characterize DCDs (in Section 3) and show that the class of DCDs properly contains various classes of integral domains of import, such as Noetherian, Mori, and Krull domains, in Section 4. In addition, we show that a pre-Schreier DCD is a GCD domain with the property that, for each
![]() $A\in F(R)$
, the ideal
$A\in F(R)$
, the ideal
![]() $A_{v}$
is principal. (Here, R is pre-Schreier if, for all
$A_{v}$
is principal. (Here, R is pre-Schreier if, for all
![]() $x,y,z\in R\backslash \{0\}$
,
$x,y,z\in R\backslash \{0\}$
,
![]() $x|yz \Rightarrow x=rs$
, with
$x|yz \Rightarrow x=rs$
, with
![]() $r,s\in R$
such that
$r,s\in R$
such that
![]() $r|y$
and
$r|y$
and
![]() $s|z$
.) We show that a domain R is a G-Dedekind domain (i.e., has the property that
$s|z$
.) We show that a domain R is a G-Dedekind domain (i.e., has the property that
![]() $A_{v}$
is invertible for each
$A_{v}$
is invertible for each
![]() $A\in F(R)$
) if and only if R is a DCD satisfying the property
$A\in F(R)$
) if and only if R is a DCD satisfying the property
![]() $\ast :$
for all pairs of subsets
$\ast :$
for all pairs of subsets
 $ \{a_{1},\ldots ,a_{m}\},\{b_{1},\ldots ,b_{n}\}\subseteq K\backslash \{0\}, (\cap _{i=1}^{m}(a_{i})(\cap _{j=1}^{n}(b_{j}))=\cap _{i,j=1}^{m,n}(a_{i}b_{j})$
from [Reference Zafrullah26]. We discuss in Section 2 what names for G-Dedekind domains and related notions should be appropriate. We also make some observations about how the DCDs behave under localizations and polynomial ring extensions.
$ \{a_{1},\ldots ,a_{m}\},\{b_{1},\ldots ,b_{n}\}\subseteq K\backslash \{0\}, (\cap _{i=1}^{m}(a_{i})(\cap _{j=1}^{n}(b_{j}))=\cap _{i,j=1}^{m,n}(a_{i}b_{j})$
from [Reference Zafrullah26]. We discuss in Section 2 what names for G-Dedekind domains and related notions should be appropriate. We also make some observations about how the DCDs behave under localizations and polynomial ring extensions.
In [Reference Zafrullah25], this author studied integral domains R with the property that
![]() $A_{v}$
is invertible for every nonzero ideal A, and called these domains “generalized Dedekind domains” or G-Dedekind domains. Later, using a new form of the abovementioned
$A_{v}$
is invertible for every nonzero ideal A, and called these domains “generalized Dedekind domains” or G-Dedekind domains. Later, using a new form of the abovementioned
![]() $\ast $
-property, Anderson and Kang [Reference Anderson and Kang6] published a much improved version of [Reference Zafrullah25]. Calling the G-Dedekind domains “Pseudo-Dedekind” domains, they showed that R is a Pseudo-Dedekind domain if and only if, for all sets
$\ast $
-property, Anderson and Kang [Reference Anderson and Kang6] published a much improved version of [Reference Zafrullah25]. Calling the G-Dedekind domains “Pseudo-Dedekind” domains, they showed that R is a Pseudo-Dedekind domain if and only if, for all sets
![]() $\{a_{\alpha }\}_{\alpha \in I}, \{b_{\beta }\}_{\beta \in J} \subseteq K\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}, \{b_{\beta }\}_{\beta \in J} \subseteq K\backslash \{0\}$
, we have
![]() $(\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
, where
$(\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
, where
![]() $\alpha $
ranges over I and
$\alpha $
ranges over I and
![]() $\beta $
over
$\beta $
over
![]() $J.$
Call a set of elements
$J.$
Call a set of elements
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
allowable if
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
allowable if
![]() $\cap (a_{\alpha })\neq (0).$
In this article, we show, among things already indicated, that, for a given star operation
$\cap (a_{\alpha })\neq (0).$
In this article, we show, among things already indicated, that, for a given star operation
![]() $\star $
,
$\star $
,
![]() $ A^{\star }$
is invertible for any ideal A of R if and only if, for any allowable set of elements
$ A^{\star }$
is invertible for any ideal A of R if and only if, for any allowable set of elements
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
and for every nonzero ideal A, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
and for every nonzero ideal A, we have
![]() $A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
. As a consequence, we show that R is a Pseudo-Dedekind/G-Dedekind domain if and only if, for each nonzero ideal A of R and for each allowable set
$A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
. As a consequence, we show that R is a Pseudo-Dedekind/G-Dedekind domain if and only if, for each nonzero ideal A of R and for each allowable set
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
![]() $A_{v}(\cap (a_{\alpha }))=\cap A_{v}(a_{\alpha })$
. We show that R is a DCD if and only if, for any allowable set
$A_{v}(\cap (a_{\alpha }))=\cap A_{v}(a_{\alpha })$
. We show that R is a DCD if and only if, for any allowable set
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, there is a set
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, there is a set
![]() $\{b_{1},b_{2},\ldots ,b_{n}\}\subseteq K\backslash \{0\}$
such that
$\{b_{1},b_{2},\ldots ,b_{n}\}\subseteq K\backslash \{0\}$
such that
![]() $\cap _{\alpha \in I}(a_{\alpha })=(b_{1},b_{2},\ldots ,b_{n})_{v}$
. We also show that R is a G-Dedekind/Pseudo-Dedekind domain if and only if R is a DCD with the abovementioned property
$\cap _{\alpha \in I}(a_{\alpha })=(b_{1},b_{2},\ldots ,b_{n})_{v}$
. We also show that R is a G-Dedekind/Pseudo-Dedekind domain if and only if R is a DCD with the abovementioned property
![]() $\ast $
. That is, the DCDs were essentially at work behind the scenes in the results in [Reference Zafrullah25] and subsequently in [Reference Anderson and Kang6].
$\ast $
. That is, the DCDs were essentially at work behind the scenes in the results in [Reference Zafrullah25] and subsequently in [Reference Anderson and Kang6].
We use star operations, in order to approach the subject from a more general standpoint. Included below is a brief introduction to star operations. The reader may consult [Reference Gilmer14], [Reference Elliott10], or [Reference Halter-Koch15] for more information on star operations. For our purposes, we include below some information that may be helpful in reading this article.
Let R be an integral domain with quotient field K, and let
![]() $F(R)$
be the set of nonzero fractional ideals of R. A star operation is a function
$F(R)$
be the set of nonzero fractional ideals of R. A star operation is a function
![]() $ A\mapsto A^{\star }$
on
$ A\mapsto A^{\star }$
on
![]() $F(R)$
with the following properties:
$F(R)$
with the following properties:
If
![]() $A,B\in F(R)$
and
$A,B\in F(R)$
and
![]() $a\in K\backslash \{0\}$
, then
$a\in K\backslash \{0\}$
, then
-
(i)
 $(a)^{\star }=(a)$
and
$(a)^{\star }=(a)$
and
 $(aA)^{\star }=aA^{\star }$
.
$(aA)^{\star }=aA^{\star }$
. -
(ii)
 $A\subseteq A^{\star }$
, and if
$A\subseteq A^{\star }$
, and if
 $A\subseteq B$
, then
$A\subseteq B$
, then
 $A^{\star }\subseteq B^{\star }$
.
$A^{\star }\subseteq B^{\star }$
. -
(iii)
 $(A^{\star })^{\star }=A^{\star }$
.
$(A^{\star })^{\star }=A^{\star }$
.
We may call
![]() $A^{\star }$
the
$A^{\star }$
the
![]() $\star $
-image (or
$\star $
-image (or
![]() $\star $
-envelope) of A. An ideal A is said to be a
$\star $
-envelope) of A. An ideal A is said to be a
![]() $\star $
-ideal if
$\star $
-ideal if
![]() $ A^{\star }=A$
. Thus,
$ A^{\star }=A$
. Thus,
![]() $A^{\star }$
is a
$A^{\star }$
is a
![]() $\star $
-ideal (by (iii)). Moreover, (by (i)), every principal fractional ideal, including
$\star $
-ideal (by (iii)). Moreover, (by (i)), every principal fractional ideal, including
![]() $R=(1)$
, is a
$R=(1)$
, is a
![]() $\star $
- ideal for any star operation
$\star $
- ideal for any star operation
![]() $\star $
.
$\star $
.
For all
![]() $A,B\in F(R)$
and for each star operation
$A,B\in F(R)$
and for each star operation
![]() $\star $
, we can show that
$\star $
, we can show that
![]() $(AB)^{\star }=(A^{\star }B)^{\star }=(A^{\star }B^{\star })^{\star }$
. These equations define what is called
$(AB)^{\star }=(A^{\star }B)^{\star }=(A^{\star }B^{\star })^{\star }$
. These equations define what is called
![]() $\star $
-multiplication (or
$\star $
-multiplication (or
![]() $\star $
-product).Associated with each star operation
$\star $
-product).Associated with each star operation
![]() $\star $
is a star operation
$\star $
is a star operation
![]() $\star _{f}$
defined by
$\star _{f}$
defined by
![]() $A^{\star _{f}}=\bigcup \{J^{\star }| 0\neq J$
is a finitely generated subideal of
$A^{\star _{f}}=\bigcup \{J^{\star }| 0\neq J$
is a finitely generated subideal of
![]() $A\}$
, for each
$A\}$
, for each
![]() $A\in F(D)$
. We say that a star operation
$A\in F(D)$
. We say that a star operation
![]() $\star $
is of finite type or of finite character if
$\star $
is of finite type or of finite character if
![]() $\star =\star _{f}$
, i.e.,
$\star =\star _{f}$
, i.e.,
![]() $A^{\star }=A^{\star _{f}}$
for each
$A^{\star }=A^{\star _{f}}$
for each
![]() $A\in F(R)$
.
$A\in F(R)$
.
Define
![]() $A^{-1}=\{x\in K|xA\subseteq R\}$
, for
$A^{-1}=\{x\in K|xA\subseteq R\}$
, for
![]() $A\in F(R)$
. Thus,
$A\in F(R)$
. Thus,
 $A^{-1}=\cap _{a\in A\backslash \{0\}}(\frac {1}{a})$
. Furthermore, define
$A^{-1}=\cap _{a\in A\backslash \{0\}}(\frac {1}{a})$
. Furthermore, define
![]() $A_{v}=(A^{-1})^{-1}$
and
$A_{v}=(A^{-1})^{-1}$
and
![]() $A_{t}=A_{v_{f}}=\bigcup \{J_{v}| 0\neq J$
is a finitely generated subideal of
$A_{t}=A_{v_{f}}=\bigcup \{J_{v}| 0\neq J$
is a finitely generated subideal of
![]() $A\}$
. By the definition,
$A\}$
. By the definition,
![]() $A_{t}=A_{v}$
for each finitely generated nonzero ideal of
$A_{t}=A_{v}$
for each finitely generated nonzero ideal of
![]() $R.$
The functions
$R.$
The functions
![]() $A\mapsto A_{v}$
and
$A\mapsto A_{v}$
and
![]() $A\mapsto A_{t}$
on
$A\mapsto A_{t}$
on
![]() $F(R)$
are more familiar examples of star operations defined on an integral domain. A fractional ideal
$F(R)$
are more familiar examples of star operations defined on an integral domain. A fractional ideal
![]() $A\in F(R)$
is
$A\in F(R)$
is
![]() $\star $
-invertible if
$\star $
-invertible if
![]() $(AA^{-1})^{\star }=R.$
An invertible ideal is a
$(AA^{-1})^{\star }=R.$
An invertible ideal is a
![]() $\star $
-invertible
$\star $
-invertible
![]() $ \star $
-ideal for each
$ \star $
-ideal for each
![]() $\star $
-operation
$\star $
-operation
![]() $\star $
, and so is a v-ideal. A
$\star $
, and so is a v-ideal. A
![]() $ v$
-ideal is better known as a divisorial ideal, and using the definition, it can be shown that
$ v$
-ideal is better known as a divisorial ideal, and using the definition, it can be shown that
 $A_{v}=\cap _{\substack { x\in K\backslash \{0\} \\ A\subseteq xR}}xR$
. The identity function d on
$A_{v}=\cap _{\substack { x\in K\backslash \{0\} \\ A\subseteq xR}}xR$
. The identity function d on
![]() $F(R)$
, defined by
$F(R)$
, defined by
![]() $ A\mapsto A$
, is another example of a star operation. Indeed, a “d-invertible” ideal is the usual invertible ideal. There are of course many more star operations that can be defined on an integral domain R. But for any star operation
$ A\mapsto A$
, is another example of a star operation. Indeed, a “d-invertible” ideal is the usual invertible ideal. There are of course many more star operations that can be defined on an integral domain R. But for any star operation
![]() $\star $
and for any
$\star $
and for any
![]() $A\in F(R), A^{\star }\subseteq A_{v}$
. Some other useful relations are: For any
$A\in F(R), A^{\star }\subseteq A_{v}$
. Some other useful relations are: For any
![]() $A\in F(R), (A^{-1})^{\star }= A^{-1}=(A^{\star })^{-1}$
, and so
$A\in F(R), (A^{-1})^{\star }= A^{-1}=(A^{\star })^{-1}$
, and so
![]() $(A_{v})^{\star }=A_{v}=(A^{\star })_{v}$
. Using the definition of the t-operation, one can show that an ideal that is maximal w.r.t. being a proper integral t-ideal is a prime ideal of R, each nonzero ideal A of R with
$(A_{v})^{\star }=A_{v}=(A^{\star })_{v}$
. Using the definition of the t-operation, one can show that an ideal that is maximal w.r.t. being a proper integral t-ideal is a prime ideal of R, each nonzero ideal A of R with
![]() $A_{t}\neq R$
is contained in a maximal t-ideal of R, and
$A_{t}\neq R$
is contained in a maximal t-ideal of R, and
![]() $R=\cap R_{M}$
, where M ranges over maximal t-ideals of
$R=\cap R_{M}$
, where M ranges over maximal t-ideals of
![]() $R.$
The set of maximal t-ideals of R is denoted by t-
$R.$
The set of maximal t-ideals of R is denoted by t-
![]() $Max(R)$
. For more on v- and t-operations, the reader may consult Sections 32 and 34 of Gilmer [Reference Gilmer14], or the other two books cited above. This is the barest minimum of description to get us started, and we shall expand on it when need arises. Our terminology comes from [Reference Gilmer14]. Of course, we have called a subset
$Max(R)$
. For more on v- and t-operations, the reader may consult Sections 32 and 34 of Gilmer [Reference Gilmer14], or the other two books cited above. This is the barest minimum of description to get us started, and we shall expand on it when need arises. Our terminology comes from [Reference Gilmer14]. Of course, we have called a subset
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
allowable
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
allowable
![]() $\cap (a_{\alpha })\neq (0)$
(a) to save on space and (b) because the characteristic property of a G-Dedekind/Pseudo-Dedekind domain is that, for all
$\cap (a_{\alpha })\neq (0)$
(a) to save on space and (b) because the characteristic property of a G-Dedekind/Pseudo-Dedekind domain is that, for all
![]() $A,B\in F(D)$
, we have
$A,B\in F(D)$
, we have
![]() $ (AB)^{-1}=A^{-1}B^{-1} $
, and this does not require any set
$ (AB)^{-1}=A^{-1}B^{-1} $
, and this does not require any set
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
with
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
with
![]() $\cap (a_{\alpha })=(0)$
. The reader may expect such purpose-oriented terminology in the sequel as well. In Section 2, we fix a name for G-/Pseudo-Dedekind domains, indicating reasons why we should, and in Section 3, we show that R is a G-/Pseudo-Dedekind domain if and only if R is a dually compact (DC)
$\cap (a_{\alpha })=(0)$
. The reader may expect such purpose-oriented terminology in the sequel as well. In Section 2, we fix a name for G-/Pseudo-Dedekind domains, indicating reasons why we should, and in Section 3, we show that R is a G-/Pseudo-Dedekind domain if and only if R is a dually compact (DC)
![]() $\ast $
-domain. Finally, in Section 4, we touch on some related questions and indicate how DCDs behave under some extensions such as quotient ring formation or, in case of integrally closed DCDs, polynomial ring formation.
$\ast $
-domain. Finally, in Section 4, we touch on some related questions and indicate how DCDs behave under some extensions such as quotient ring formation or, in case of integrally closed DCDs, polynomial ring formation.
2 What is in a name?
Popescu [Reference Popescu20] introduced the notion of a generalized Dedekind domain via localizing systems. Nowadays, the following equivalent definition is usually given: An integral domain is a generalized Dedekind domain if it is a strongly discrete Prüfer domain (i.e.,
![]() $P\neq P^{2}$
for every prime ideal P) and every (prime) ideal I has
$P\neq P^{2}$
for every prime ideal P) and every (prime) ideal I has
![]() $\surd I=\surd (a_{1},\ldots ,a_{n})$
for some
$\surd I=\surd (a_{1},\ldots ,a_{n})$
for some
![]() $a_{1},\ldots ,a_{n}\in I$
(or equivalently, every principal ideal has only finitely many minimal prime ideals). Unbeknownst to this author, [Reference Popescu20] was already out. I personally do not think there is anything pseudo about the G-Dedekind domains. On the other hand, some serious studies related to G-Dedekind prime rings, introduced by Akalan [Reference Akalan1], are being carried out. This indicates that there is need for a name close to G-Dedekind domains. The aim of this section is to fix a suitable name for the domains that are given two different names, one not quite appropriate and the other overshadowed by a previously adopted name. Of course, as a result, we end up with one more notion a more suitable name for a so-called
$a_{1},\ldots ,a_{n}\in I$
(or equivalently, every principal ideal has only finitely many minimal prime ideals). Unbeknownst to this author, [Reference Popescu20] was already out. I personally do not think there is anything pseudo about the G-Dedekind domains. On the other hand, some serious studies related to G-Dedekind prime rings, introduced by Akalan [Reference Akalan1], are being carried out. This indicates that there is need for a name close to G-Dedekind domains. The aim of this section is to fix a suitable name for the domains that are given two different names, one not quite appropriate and the other overshadowed by a previously adopted name. Of course, as a result, we end up with one more notion a more suitable name for a so-called
![]() $ \pi $
-domain.
$ \pi $
-domain.
The following lemma essentially comes from [Reference Anderson2], yet we use it to give a more general view of what started as G-Dedekind domains or Pseudo-Dedekind domains. Of course, our statement is different and more streamlined.
Lemma 2.1 Let
![]() $\star $
be a star operation defined on an integral domain R, and let
$\star $
be a star operation defined on an integral domain R, and let
![]() $A\in F(R)$
. Then
$A\in F(R)$
. Then
![]() $A^{\star }$
is invertible if and only if, for any allowable set of elements
$A^{\star }$
is invertible if and only if, for any allowable set of elements
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
![]() $A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
.
$A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
.
The proof of the above lemma has been used as part of the proof of the following theorem.
Theorem 2.2 Let
![]() $\star $
be a star operation defined on an integral domain R. Then
$\star $
be a star operation defined on an integral domain R. Then
![]() $A^{\star }$
is invertible for every nonzero fractional ideal A of R if and only if, for any allowable set of elements
$A^{\star }$
is invertible for every nonzero fractional ideal A of R if and only if, for any allowable set of elements
![]() $ \{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
and for every nonzero ideal A, we have
$ \{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
and for every nonzero ideal A, we have
![]() $A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
.
$A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
.
Proof Let A be any nonzero ideal of R, and suppose that, for every allowable set
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
![]() $ A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
. Then
$ A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
. Then
 $R\supseteq A^{\star }A^{-1} = A^{\star }(\cap _{a_{\beta }\in A\backslash \{0\}}( \frac {1}{a_{\beta }})) =\cap _{a_{\beta }\in A\backslash \{0\}}A^{\star }( \frac {1}{a_{\beta }})$
by the condition. Since for each of
$R\supseteq A^{\star }A^{-1} = A^{\star }(\cap _{a_{\beta }\in A\backslash \{0\}}( \frac {1}{a_{\beta }})) =\cap _{a_{\beta }\in A\backslash \{0\}}A^{\star }( \frac {1}{a_{\beta }})$
by the condition. Since for each of
![]() $a_{\beta }\in A\backslash \{0\}$
we have
$a_{\beta }\in A\backslash \{0\}$
we have
 $A^{\star }(\frac {1}{a_{\beta }})\supseteq R$
and so
$A^{\star }(\frac {1}{a_{\beta }})\supseteq R$
and so
 $R\supseteq (A^{\star }A^{-1}= A^{\star }(\cap _{a_{\beta }\in A\backslash \{0\}}(\frac {1}{a_{\beta }}))=\cap _{a_{\beta }\in A\backslash \{0\}}A^{\star }(\frac {1}{a_{\beta }})\supseteq R$
, showing that
$R\supseteq (A^{\star }A^{-1}= A^{\star }(\cap _{a_{\beta }\in A\backslash \{0\}}(\frac {1}{a_{\beta }}))=\cap _{a_{\beta }\in A\backslash \{0\}}A^{\star }(\frac {1}{a_{\beta }})\supseteq R$
, showing that
 $R=A^{\star }(\cap _{a_{\beta }\in A\backslash \{0\}}(\frac {1}{a_{\beta }}))=A^{\star }A^{-1}$
. Thus, as A was chosen arbitrarily, the condition implies that, for every nonzero ideal A, we have that
$R=A^{\star }(\cap _{a_{\beta }\in A\backslash \{0\}}(\frac {1}{a_{\beta }}))=A^{\star }A^{-1}$
. Thus, as A was chosen arbitrarily, the condition implies that, for every nonzero ideal A, we have that
![]() $A^{\star }$
is invertible. Conversely, suppose that A is a nonzero ideal such that
$A^{\star }$
is invertible. Conversely, suppose that A is a nonzero ideal such that
![]() $A^{\star }$
is invertible. Then, by an exercise on page 80 of [Reference Gilmer14], we have, for any allowable set,
$A^{\star }$
is invertible. Then, by an exercise on page 80 of [Reference Gilmer14], we have, for any allowable set,
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
,
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
,
![]() $ A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }(a_{\alpha })$
. Thus, for every nonzero ideal A, the ideal
$ A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }(a_{\alpha })$
. Thus, for every nonzero ideal A, the ideal
![]() $A^{\star }$
being invertible implies that, for every nonzero ideal A and for every allowable set
$A^{\star }$
being invertible implies that, for every nonzero ideal A and for every allowable set
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
![]() $A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
.▪
$A^{\star }(\cap (a_{\alpha }))=\cap A^{\star }a_{\alpha }$
.▪
Corollary 1 For
![]() $\star =d$
, R is a Dedekind domain if and only if, for each nonzero ideal A of R and for each allowable set
$\star =d$
, R is a Dedekind domain if and only if, for each nonzero ideal A of R and for each allowable set
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
![]() $A(\cap (a_{\alpha }))=\cap A(a_{\alpha })$
.
$A(\cap (a_{\alpha }))=\cap A(a_{\alpha })$
.
Proof Indeed, it is well known that R is a Dedekind domain if and only if every nonzero ideal of R is invertible and Theorem 2.2 provides the, general, necessary and sufficient conditions for every nonzero ideal to be
![]() $ \star $
-invertible, when
$ \star $
-invertible, when
![]() $\star =d.$
▪
$\star =d.$
▪
Let us recall that an ideal I is t-invertible if I is v-invertible and
![]() $I^{-1}$
is a v-ideal of finite type, that an ideal I that is invertible is
$I^{-1}$
is a v-ideal of finite type, that an ideal I that is invertible is
![]() $\star $
-invertible for every star operation
$\star $
-invertible for every star operation
![]() $\star $
[Reference Zafrullah, Glaz and Chapman28], and that an integral domain R is a Krull domain if and only if every nonzero ideal of R is t-invertible [Reference Jaffard19]. Now, if
$\star $
[Reference Zafrullah, Glaz and Chapman28], and that an integral domain R is a Krull domain if and only if every nonzero ideal of R is t-invertible [Reference Jaffard19]. Now, if
![]() $I_{t}$
is invertible, then
$I_{t}$
is invertible, then
![]() $I_{t}$
and hence I are t-invertible. Thus, if, for each ideal I,
$I_{t}$
and hence I are t-invertible. Thus, if, for each ideal I,
![]() $I_{t}$
is invertible in a domain R, then R is at least a Krull domain. According to Theorem 1.10 of [Reference Zafrullah25], if
$I_{t}$
is invertible in a domain R, then R is at least a Krull domain. According to Theorem 1.10 of [Reference Zafrullah25], if
![]() $I_{t}$
is invertible for every nonzero ideal of R, then R is a locally factorial Krull domain. Such domains are often called
$I_{t}$
is invertible for every nonzero ideal of R, then R is a locally factorial Krull domain. Such domains are often called
![]() $\pi $
-domains for some reason. Now, Theorem 2.2 characterizes
$\pi $
-domains for some reason. Now, Theorem 2.2 characterizes
![]() $\pi $
-domains for
$\pi $
-domains for
![]() $\star =t$
as follows.
$\star =t$
as follows.
Corollary 2 A domain R is a
![]() $\pi $
-domain if and only if, for each nonzero ideal A of R and for each allowable set
$\pi $
-domain if and only if, for each nonzero ideal A of R and for each allowable set
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\},$
we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\},$
we have
![]() $A_{t}(\cap (a_{\alpha }))=\cap A_{t}(a_{\alpha })$
.
$A_{t}(\cap (a_{\alpha }))=\cap A_{t}(a_{\alpha })$
.
Proof Indeed, R is a
![]() $\pi $
-domain if and only if
$\pi $
-domain if and only if
![]() $A_{t}$
is invertible for every nonzero ideal A of R (Theorem 1.10 of [Reference Zafrullah25]) and Theorem 2.2 provides the, general, necessary and sufficient conditions for every nonzero ideal to be
$A_{t}$
is invertible for every nonzero ideal A of R (Theorem 1.10 of [Reference Zafrullah25]) and Theorem 2.2 provides the, general, necessary and sufficient conditions for every nonzero ideal to be
![]() $\star $
-invertible, when
$\star $
-invertible, when
![]() $\star =t$
.▪
$\star =t$
.▪
Of course, by saying that an ideal A of R is v-invertible, we mean that
![]() $ (AA^{-1})_{v}=R.$
Similar to earlier comments, we note that
$ (AA^{-1})_{v}=R.$
Similar to earlier comments, we note that
![]() $A_{v}$
being invertible entails A being v-invertible. We also note that domains R with
$A_{v}$
being invertible entails A being v-invertible. We also note that domains R with
![]() $A_{v}$
invertible for each nonzero ideal A are the G-Dedekind/Pseudo-Dedekind domains. So, for
$A_{v}$
invertible for each nonzero ideal A are the G-Dedekind/Pseudo-Dedekind domains. So, for
![]() $\star =v$
, Theorem 2.2 provides the following characterization of G-Dedekind/Pseudo-Dedekind domains.
$\star =v$
, Theorem 2.2 provides the following characterization of G-Dedekind/Pseudo-Dedekind domains.
Corollary 3 A domain R is a G-Dedekind/Pseudo-Dedekind domain if and only if, for each nonzero ideal A of R and for each allowable set
![]() $ \{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
$ \{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have
![]() $ A_{v}(\cap (a_{\alpha }))=\cap A_{v}(a_{\alpha })$
.
$ A_{v}(\cap (a_{\alpha }))=\cap A_{v}(a_{\alpha })$
.
The proof is the same as the one provided by Theorem 2.2 replacing
![]() $\star $
by
$\star $
by
![]() $v$
.
$v$
.
Remark 2.3 Looking at Theorem 2.2 and Corollaries 1–3, we may call R a
![]() $ \star $
-Dedekind domain if
$ \star $
-Dedekind domain if
![]() $A^{\star }$
is invertible for each
$A^{\star }$
is invertible for each
![]() $A\in F(R)$
and note that in a
$A\in F(R)$
and note that in a
![]() $\star $
-Dedekind domain, we have
$\star $
-Dedekind domain, we have
![]() $A^{\star }=A_{v}$
for all
$A^{\star }=A_{v}$
for all
![]() $A\in F(R)$
. This is because an invertible ideal is divisorial. Thus, Corollary 2 gives for
$A\in F(R)$
. This is because an invertible ideal is divisorial. Thus, Corollary 2 gives for
![]() $\star =t$
a t-Dedekind domain, and Corollary 3 gives the name of a v-Dedekind domain to the G-Dedekind domain of [Reference Zafrullah25] and the Pseudo-Dedekind domain of [Reference Anderson and Kang6]. But there is a slight problem with this naming system; Elliott in [Reference Elliott10] calls a Krull domain a t-Dedekind domain. So, perhaps,
$\star =t$
a t-Dedekind domain, and Corollary 3 gives the name of a v-Dedekind domain to the G-Dedekind domain of [Reference Zafrullah25] and the Pseudo-Dedekind domain of [Reference Anderson and Kang6]. But there is a slight problem with this naming system; Elliott in [Reference Elliott10] calls a Krull domain a t-Dedekind domain. So, perhaps,
![]() $\star $
-G-Dedekind may be the general name with the note that a d-G-Dedekind domain is the usual Dedekind domain and a t-G-Dedekind domain is a locally factorial Krull domain, while the v-G-Dedekind domain is the usual Pseudo-Dedekind domain, or the old G-Dedekind domain. Of course, as
$\star $
-G-Dedekind may be the general name with the note that a d-G-Dedekind domain is the usual Dedekind domain and a t-G-Dedekind domain is a locally factorial Krull domain, while the v-G-Dedekind domain is the usual Pseudo-Dedekind domain, or the old G-Dedekind domain. Of course, as
![]() $\star =v$
in a
$\star =v$
in a
![]() $\star $
-G-Dedekind domain, each
$\star $
-G-Dedekind domain, each
![]() $\star $
-G-Dedekind domain has the properties listed in [Reference Zafrullah25] for G-Dedekind domains and are shared by
$\star $
-G-Dedekind domain has the properties listed in [Reference Zafrullah25] for G-Dedekind domains and are shared by
![]() $ \star $
-G-Dedekind domains, or in [Reference Anderson and Kang6] for Pseudo-Dedekind domains. Thus, if
$ \star $
-G-Dedekind domains, or in [Reference Anderson and Kang6] for Pseudo-Dedekind domains. Thus, if
![]() $\star $
is of finite character, then
$\star $
is of finite character, then
![]() $\star =\star _{f}=v_{f}=t$
. That is, if R is a
$\star =\star _{f}=v_{f}=t$
. That is, if R is a
![]() $\star $
-G-Dedekind domain and
$\star $
-G-Dedekind domain and
![]() $\star $
is of finite character, then R is a t-G-Dedekind domain. Recall that an integral domain R with quotient field K is completely integrally closed if whenever
$\star $
is of finite character, then R is a t-G-Dedekind domain. Recall that an integral domain R with quotient field K is completely integrally closed if whenever
![]() $rx^{n}\in R$
for
$rx^{n}\in R$
for
![]() $x\in K$
,
$x\in K$
,
![]() $0\neq r\in R$
, and every integer
$0\neq r\in R$
, and every integer
![]() $ n\geq 1$
, then
$ n\geq 1$
, then
![]() $x\in R$
. Equivalently, R is completely integrally closed if and only if
$x\in R$
. Equivalently, R is completely integrally closed if and only if
![]() $(AA^{-1})_{v}=R$
for every
$(AA^{-1})_{v}=R$
for every
![]() $A\in F(R)$
[Reference Gilmer14]. If, for a star operation
$A\in F(R)$
[Reference Gilmer14]. If, for a star operation
![]() $\star $
defined on
$\star $
defined on
![]() $R, A$
is
$R, A$
is
![]() $\star $
invertible for each
$\star $
invertible for each
![]() $ A\in F(R)$
following [Reference Anderson, Anderson, Fontana and Zafrullah4], we may call R a
$ A\in F(R)$
following [Reference Anderson, Anderson, Fontana and Zafrullah4], we may call R a
![]() $\star $
-CICD. Thus, as noted in [Reference Zafrullah25], a
$\star $
-CICD. Thus, as noted in [Reference Zafrullah25], a
![]() $\star $
-G-Dedekind domain is a completely integrally closed domain (CICD), for each star operation
$\star $
-G-Dedekind domain is a completely integrally closed domain (CICD), for each star operation
![]() $\star $
that it is defined for. There is a star operation called the w-operation, defined in terms of the t-operation as
$\star $
that it is defined for. There is a star operation called the w-operation, defined in terms of the t-operation as
![]() $A\mapsto A_{w}=\cap _{M\in t\text {-} Max(R)}AR_{M}$
(see [Reference Anderson and Cook5] and the references therein). As indicated in [Reference Anderson and Cook5], this operation is of finite character. Thus, in view of earlier comments in this remark, a w-G-Dedekind domain is a t-G-Dedekind domain. (While the w-operation has been around for some time, Wang and McCasland adopted it in [Reference Wang and McCasland23].)
$A\mapsto A_{w}=\cap _{M\in t\text {-} Max(R)}AR_{M}$
(see [Reference Anderson and Cook5] and the references therein). As indicated in [Reference Anderson and Cook5], this operation is of finite character. Thus, in view of earlier comments in this remark, a w-G-Dedekind domain is a t-G-Dedekind domain. (While the w-operation has been around for some time, Wang and McCasland adopted it in [Reference Wang and McCasland23].)
3 Dually compact domains
Cohn [Reference Cohn9] called an element
![]() $x\in R$
primal if, for all
$x\in R$
primal if, for all
![]() $y,z\in R,x|yz$
implies that
$y,z\in R,x|yz$
implies that
![]() $x=rs$
where
$x=rs$
where
![]() $r|y$
and
$r|y$
and
![]() $s|z.$
A domain all of whose nonzero elements are primal was called a pre-Schreier domain in [Reference Zafrullah26]; this was a break from Cohn who called R a Schreier domain if R was integrally closed with all elements primal. Based on a study of the group of divisibility of a pre-Schreier domain, this author extracted, in [Reference Zafrullah26], what he called the
$s|z.$
A domain all of whose nonzero elements are primal was called a pre-Schreier domain in [Reference Zafrullah26]; this was a break from Cohn who called R a Schreier domain if R was integrally closed with all elements primal. Based on a study of the group of divisibility of a pre-Schreier domain, this author extracted, in [Reference Zafrullah26], what he called the
![]() $\ast $
property, saying: R is a
$\ast $
property, saying: R is a
![]() $\ast $
domain if, for all pairs of subsets
$\ast $
domain if, for all pairs of subsets
![]() $\{a_{1},\ldots ,a_{m}\},\{b_{1},\ldots ,b_{n}\}\subseteq K\backslash \{0\}$
,
$\{a_{1},\ldots ,a_{m}\},\{b_{1},\ldots ,b_{n}\}\subseteq K\backslash \{0\}$
,
 $(\cap _{i=1}^{m}(a_{i}))(\cap _{j=1}^{n}(b_{j}))=\cap _{i,j=1}^{m,n}(a_{i}b_{j})$
.
$(\cap _{i=1}^{m}(a_{i}))(\cap _{j=1}^{n}(b_{j}))=\cap _{i,j=1}^{m,n}(a_{i}b_{j})$
.
We now look at the facts working behind the Anderson–Kang/Zafrullah results. For this, let us call a domain R DC if, for any allowable subset
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\},$
there is a set
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\},$
there is a set
![]() $\{x_{1},x_{2},\ldots ,x_{n}\}\subseteq K\backslash \{0\}$
such that
$\{x_{1},x_{2},\ldots ,x_{n}\}\subseteq K\backslash \{0\}$
such that
![]() $\cap _{\alpha \in I}(a_{\alpha })=(x_{1})\cap (x_{2})\cap \cdots \cap (x_{n})$
. Let us also note that a fractional ideal A being divisorial ideal (or v -ideal) of finite type means that there are elements
$\cap _{\alpha \in I}(a_{\alpha })=(x_{1})\cap (x_{2})\cap \cdots \cap (x_{n})$
. Let us also note that a fractional ideal A being divisorial ideal (or v -ideal) of finite type means that there are elements
![]() $s_{1},\ldots ,s_{r}\in K\backslash \{0\}$
such that
$s_{1},\ldots ,s_{r}\in K\backslash \{0\}$
such that
![]() $A=(s_{1},\ldots ,s_{r})_{v}$
.
$A=(s_{1},\ldots ,s_{r})_{v}$
.
Theorem 3.1 The following are equivalent for an integral domain R that is different from its quotient field
![]() $K.$
$K.$
-
(1) R is DC.
-
(2)
 $A_{v}$
is a v-ideal of finite type for every
$A_{v}$
is a v-ideal of finite type for every
 $A\in F(R)$
.
$A\in F(R)$
. -
(3)
 $A^{-1}$
is of finite type for each
$A^{-1}$
is of finite type for each
 $A\in F(R)$
.
$A\in F(R)$
. -
(4)
 $A^{-1}$
is of finite type for each nonzero integral ideal A of R.
$A^{-1}$
is of finite type for each nonzero integral ideal A of R. -
(5)
 $A_{v}$
is of finite type for each nonzero integral ideal A of R.
$A_{v}$
is of finite type for each nonzero integral ideal A of R. -
(6) Every divisorial ideal of R is expressible as a finite intersection of principal fractional ideals.
-
(7) For a star operation
 $\star $
and for each
$\star $
and for each
 $A\in F(R)$
, the ideal
$A\in F(R)$
, the ideal
 $ A^{\star }$
is a v-ideal of finite type.
$ A^{\star }$
is a v-ideal of finite type. -
(8) For a star operation
 $\star $
and for each
$\star $
and for each
 $A\in F(R)$
, the ideal
$A\in F(R)$
, the ideal
 $ A^{\star }$
is a finite intersection of principal ideals from
$ A^{\star }$
is a finite intersection of principal ideals from
 $F(R)$
.
$F(R)$
.
Proof (1)
![]() $\Rightarrow $
(2). Suppose that R is DC, and suppose that A is a nonzero fractional ideal generated by
$\Rightarrow $
(2). Suppose that R is DC, and suppose that A is a nonzero fractional ideal generated by
![]() $\{c_{\gamma }\}_{\gamma \in J}$
. Then
$\{c_{\gamma }\}_{\gamma \in J}$
. Then
 $A^{-1}=\cap _{\gamma }(\frac {1}{c_{\gamma }})\neq (0)$
. So, by the DC property, there is a finite set
$A^{-1}=\cap _{\gamma }(\frac {1}{c_{\gamma }})\neq (0)$
. So, by the DC property, there is a finite set
![]() $\{x_{1},x_{2},\ldots ,x_{n}\}\subseteq K\backslash \{0\}$
such that
$\{x_{1},x_{2},\ldots ,x_{n}\}\subseteq K\backslash \{0\}$
such that
 $\cap _{\gamma }(\frac {1}{c_{\gamma }})=\cap _{i=1}^{n}(x_{i})=(x_{1}^{-1},\ldots ,x_{n}^{-1})^{-1}$
. Now,
$\cap _{\gamma }(\frac {1}{c_{\gamma }})=\cap _{i=1}^{n}(x_{i})=(x_{1}^{-1},\ldots ,x_{n}^{-1})^{-1}$
. Now,
![]() $ A^{-1}=(x_{1}^{-1},\ldots ,x_{n}^{-1})^{-1}$
implies that
$ A^{-1}=(x_{1}^{-1},\ldots ,x_{n}^{-1})^{-1}$
implies that
![]() $ A_{v}=(x_{1}^{-1},\ldots ,x_{n}^{-1})_{v}$
.
$ A_{v}=(x_{1}^{-1},\ldots ,x_{n}^{-1})_{v}$
.
(2)
![]() $\Rightarrow $
(1). Suppose that, for each
$\Rightarrow $
(1). Suppose that, for each
![]() $A\in F(R)$
, there are
$A\in F(R)$
, there are
![]() $ x_{1},x_{2},\ldots ,x_{n}\in K\backslash \{0\}$
such that
$ x_{1},x_{2},\ldots ,x_{n}\in K\backslash \{0\}$
such that
![]() $ A_{v}=(x_{1},x_{2},\ldots ,x_{n})_{v}$
. Then, since
$ A_{v}=(x_{1},x_{2},\ldots ,x_{n})_{v}$
. Then, since
![]() $A^{-1}$
is a divisorial ideal, as
$A^{-1}$
is a divisorial ideal, as
![]() $(A^{-1})_{v}=A^{-1}$
, we have
$(A^{-1})_{v}=A^{-1}$
, we have
![]() $A^{-1}=(y_{1},\ldots ,y_{r})_{v}$
. Or, assuming that all the
$A^{-1}=(y_{1},\ldots ,y_{r})_{v}$
. Or, assuming that all the
![]() $y_{i}$
are nonzero,
$y_{i}$
are nonzero,
 $A_{v}=\cap (\frac {1}{y_{i}})$
for each
$A_{v}=\cap (\frac {1}{y_{i}})$
for each
![]() $A\in F(R)$
. Thus, if, for each
$A\in F(R)$
. Thus, if, for each
![]() $A\in F(R)$
,
$A\in F(R)$
,
![]() $A_{v}$
is of finite type, then, for each
$A_{v}$
is of finite type, then, for each
![]() $A\in F(R)$
, we can find some
$A\in F(R)$
, we can find some
![]() $b_{i}\in K\backslash \{0\}$
such that
$b_{i}\in K\backslash \{0\}$
such that
![]() $A_{v}=\cap _{i=1}^{r}(b_{i}).$
Now, let
$A_{v}=\cap _{i=1}^{r}(b_{i}).$
Now, let
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
be allowable, and let
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
be allowable, and let
![]() $A=\cap (a_{\alpha }).$
Since
$A=\cap (a_{\alpha }).$
Since
![]() $\cap (a_{\alpha })\neq (0)$
, A is a divisorial ideal by [Reference Gilmer14] and hence of finite type, and so, by (2), for some
$\cap (a_{\alpha })\neq (0)$
, A is a divisorial ideal by [Reference Gilmer14] and hence of finite type, and so, by (2), for some
![]() $x_{1},\ldots ,x_{n}\in K\backslash \{0\}$
, we have
$x_{1},\ldots ,x_{n}\in K\backslash \{0\}$
, we have
![]() $A=\cap (a_{\alpha })=\cap _{i=1}^{n}(x_{i})$
.
$A=\cap (a_{\alpha })=\cap _{i=1}^{n}(x_{i})$
.
Next, (2)
![]() $\Leftrightarrow $
(5) and (3)
$\Leftrightarrow $
(5) and (3)
![]() $\Leftrightarrow $
(4) because every fractional ideal A of R is of the form
$\Leftrightarrow $
(4) because every fractional ideal A of R is of the form
![]() $\frac {B}{d}$
where B is an integral ideal. (2)
$\frac {B}{d}$
where B is an integral ideal. (2)
![]() $\Rightarrow $
(3) because
$\Rightarrow $
(3) because
![]() $A^{-1}=(A^{-1})_{v}$
and (3)
$A^{-1}=(A^{-1})_{v}$
and (3)
![]() $\Rightarrow $
(2) because
$\Rightarrow $
(2) because
![]() $A_{v}=(A^{-1})^{-1}$
.
$A_{v}=(A^{-1})^{-1}$
.
(1)
![]() $\Rightarrow $
(6). A nonzero ideal A is divisorial if and only if
$\Rightarrow $
(6). A nonzero ideal A is divisorial if and only if
 $ A=\cap _{\substack { A\subseteq x_{\alpha }R \\ x_{\alpha }\in K\backslash \{0\}}}x_{\alpha }R$
. By the DC condition, there are
$ A=\cap _{\substack { A\subseteq x_{\alpha }R \\ x_{\alpha }\in K\backslash \{0\}}}x_{\alpha }R$
. By the DC condition, there are
![]() $x_{1},\dots ,x_{n}$
in
$x_{1},\dots ,x_{n}$
in
![]() $ K\backslash \{0\}$
such that
$ K\backslash \{0\}$
such that
 $A=\cap _{\substack { A\subseteq x_{\alpha }R \\ x_{\alpha }\in K\backslash \{0\}}}x_{\alpha }R=\cap _{i=1}^{n}x_{i}R$
,
$A=\cap _{\substack { A\subseteq x_{\alpha }R \\ x_{\alpha }\in K\backslash \{0\}}}x_{\alpha }R=\cap _{i=1}^{n}x_{i}R$
,
(6)
![]() $\Rightarrow $
(1). Let for
$\Rightarrow $
(1). Let for
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
,
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
,
![]() $\cap (a_{\alpha })\neq (0)$
. Note that
$\cap (a_{\alpha })\neq (0)$
. Note that
![]() $\cap (a_{\alpha })$
is divisorial. So, by (6), there are elements
$\cap (a_{\alpha })$
is divisorial. So, by (6), there are elements
![]() $x_{1},\dots ,x_{r}\in K\backslash \{0\}$
such that
$x_{1},\dots ,x_{r}\in K\backslash \{0\}$
such that
![]() $\cap (a_{\alpha })=\cap _{i=1}^{r}(x_{i})$
. Finally, each of (7) and (8) holds if and only if
$\cap (a_{\alpha })=\cap _{i=1}^{r}(x_{i})$
. Finally, each of (7) and (8) holds if and only if
![]() $A^{\star }=A_{v}$
for all
$A^{\star }=A_{v}$
for all
![]() $ A\in F(R)$
because in both cases
$ A\in F(R)$
because in both cases
![]() $A^{\star }=B$
where B is divisorial and so
$A^{\star }=B$
where B is divisorial and so
![]() $A_{v}=B=A^{\star }$
. Observing that, we have (7)
$A_{v}=B=A^{\star }$
. Observing that, we have (7)
![]() $\Leftrightarrow $
(5) and (8)
$\Leftrightarrow $
(5) and (8)
![]() $\Leftrightarrow $
(6).▪
$\Leftrightarrow $
(6).▪
Remark 3.2
-
(1) There are a number of integral domains that fit the description of DCDs. Noetherian domains do fit nicely, as do the so-called Mori domains. Recall that R is a Mori domain if R satisfies ascending chain conditions on divisorial ideals. It is well known that R is a Mori domain if and only if, for each
 $A\in F(R)$
, there is a finitely generated fractional ideal
$A\in F(R)$
, there is a finitely generated fractional ideal
 $ B\subseteq A$
such that
$ B\subseteq A$
such that
 $A_{v}=B_{v}$
. Indeed, a Krull domain is a DCD, being a Mori domain as indicated in Fossum [Reference Fossum13]. On the other hand, there are DCDs, such as the ring of entire functions in which
$A_{v}=B_{v}$
. Indeed, a Krull domain is a DCD, being a Mori domain as indicated in Fossum [Reference Fossum13]. On the other hand, there are DCDs, such as the ring of entire functions in which
 $A_{v}$
is principal for each
$A_{v}$
is principal for each
 $A\in F(R)$
and the ring of entire functions is neither a Mori domain, nor a Krull domain.
$A\in F(R)$
and the ring of entire functions is neither a Mori domain, nor a Krull domain. -
(2) If the star operation
 $\star $
defined on R is such that
$\star $
defined on R is such that
 $A^{\star }$
is a v-ideal of finite type for each
$A^{\star }$
is a v-ideal of finite type for each
 $A\in F(R)$
, we can call R a
$A\in F(R)$
, we can call R a
 $\star $
-DCD.
$\star $
-DCD. -
(3) It may be somewhat hard to see, for some, that any t-invertible t -ideal, and hence any invertible ideal, is a finite intersection of principal fractional ideals. Let me note for the record that if A is a t -invertible t-ideal, then
 $A^{-1}$
is of finite type. Say, for some
$A^{-1}$
is of finite type. Say, for some
 $ B=\{b_{1},\ldots ,b_{n}\}$
, we have
$ B=\{b_{1},\ldots ,b_{n}\}$
, we have
 $A^{-1}=B_{v}.$
But then,
$A^{-1}=B_{v}.$
But then,
 $ A=A_{v}=(B_{v})^{-1}=\cap _{i=1}^{n}(\frac {1}{b_{i}})$
.
$ A=A_{v}=(B_{v})^{-1}=\cap _{i=1}^{n}(\frac {1}{b_{i}})$
.
Indeed, as in a DCD, the inverse of every nonzero fractional ideal is of finite type, and all we need for the v-G-Dedekind property to hold is the
![]() $ \ast $
-property.
$ \ast $
-property.
Theorem 3.3 Let R be a DCD. Then R is a v-G-Dedekind domain if and only if R is a
![]() $\ast $
-domain.
$\ast $
-domain.
Proof It is easy to see, from the treatment of it in [Reference Anderson and Kang6], that a v -G-Dedekind domain is a
![]() $\ast $
-domain. (This fact was also mentioned in [Reference Zafrullah25].) But, of course, we need to show that a v-G-Dedekind domain is DC. This follows from the fact that, in a v-G-Dedekind domain
$\ast $
-domain. (This fact was also mentioned in [Reference Zafrullah25].) But, of course, we need to show that a v-G-Dedekind domain is DC. This follows from the fact that, in a v-G-Dedekind domain
![]() $R, A_{v}$
is invertible for each
$R, A_{v}$
is invertible for each
![]() $A\in F(R)$
and, as shown in Remark 3.2, an intersection of finitely many principal fractional ideals. For the converse, we show that DC plus the
$A\in F(R)$
and, as shown in Remark 3.2, an intersection of finitely many principal fractional ideals. For the converse, we show that DC plus the
![]() $\ast $
-property imply the v -G-Dedekind property. For this, consider for allowable sets
$\ast $
-property imply the v -G-Dedekind property. For this, consider for allowable sets
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq K\backslash \{0\}$
the product
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq K\backslash \{0\}$
the product
![]() $P=(\cap (a_{\alpha }))(\cap (b_{\beta }))$
. By the DC property of R, we can find
$P=(\cap (a_{\alpha }))(\cap (b_{\beta }))$
. By the DC property of R, we can find
![]() $\{x_{1},\ldots ,x_{m}\}, \{y_{1},\ldots ,y_{n}\}\subseteq K\backslash \{0\}$
such that
$\{x_{1},\ldots ,x_{m}\}, \{y_{1},\ldots ,y_{n}\}\subseteq K\backslash \{0\}$
such that
![]() $\cap (a_{\alpha })=\cap _{i=1}^{m}(x_{i})$
and
$\cap (a_{\alpha })=\cap _{i=1}^{m}(x_{i})$
and
 $\cap (b_{\beta })=(\cap _{j=1}^{n}(y_{j})$
. Thus, using DC plus
$\cap (b_{\beta })=(\cap _{j=1}^{n}(y_{j})$
. Thus, using DC plus
![]() $\ast $
,
$\ast $
,
 $P=(\cap (a_{\alpha }))(\cap (b_{\beta }))=(\cap (x_{i}))(\cap (y_{j}))=\cap _{i,j=1}^{m,n}(x_{i}y_{j})=\cap _{i}x_{i}(\cap (y_{j}))=\cap x_{i}(\cap (b_{\beta }))$
(using
$P=(\cap (a_{\alpha }))(\cap (b_{\beta }))=(\cap (x_{i}))(\cap (y_{j}))=\cap _{i,j=1}^{m,n}(x_{i}y_{j})=\cap _{i}x_{i}(\cap (y_{j}))=\cap x_{i}(\cap (b_{\beta }))$
(using
![]() $\cap (y_{j})=\cap (b_{\beta }))$
. This gives
$\cap (y_{j})=\cap (b_{\beta }))$
. This gives
![]() $P=\cap _{i}x_{i}(\cap (b_{\beta }))=\cap _{i,\beta }(x_{i}b_{\beta })=\cap _{\beta }b_{\beta }(\cap (x_{i}))=\cap _{\beta }b_{\beta }(\cap (a_{\alpha }))=\cap _{\alpha ,\beta }(a_{\alpha }b_{\beta }) $
.▪
$P=\cap _{i}x_{i}(\cap (b_{\beta }))=\cap _{i,\beta }(x_{i}b_{\beta })=\cap _{\beta }b_{\beta }(\cap (x_{i}))=\cap _{\beta }b_{\beta }(\cap (a_{\alpha }))=\cap _{\alpha ,\beta }(a_{\alpha }b_{\beta }) $
.▪
Recall that an integral domain R is called v-coherent if every finite intersection of v-ideals of finite type is a v-ideal of finite type, equivalently, if
![]() $A^{-1}$
is a v-ideal of finite for all finitely generated
$A^{-1}$
is a v-ideal of finite for all finitely generated
![]() $A\in F(R)$
[Reference Fontana and Gabelli11]. Also that R is a generalized greatest common divisor (GGCD) domain if
$A\in F(R)$
[Reference Fontana and Gabelli11]. Also that R is a generalized greatest common divisor (GGCD) domain if
![]() $aR\cap bR$
is invertible for all
$aR\cap bR$
is invertible for all
![]() $a,b\in R\backslash \{0\}$
[Reference Anderson and Anderson3]. According to Corollary 1.7 of [Reference Zafrullah25], a v-coherent domain R is a GGCD domain if and only if R is a
$a,b\in R\backslash \{0\}$
[Reference Anderson and Anderson3]. According to Corollary 1.7 of [Reference Zafrullah25], a v-coherent domain R is a GGCD domain if and only if R is a
![]() $\ast $
-domain. Now, a DCD R is slightly more than a v-coherent domain.
$\ast $
-domain. Now, a DCD R is slightly more than a v-coherent domain.
Corollary 4 Suppose that for a star operation
![]() $\star $
,
$\star $
,
![]() $A^{\star }$
is a v-ideal of finite type for each
$A^{\star }$
is a v-ideal of finite type for each
![]() $A\in F(R)$
. Then R is a
$A\in F(R)$
. Then R is a
![]() $\star $
-G-Dedekind domain if and only if R is a
$\star $
-G-Dedekind domain if and only if R is a
![]() $\ast $
-domain. Consequently, a Mori (and hence a Krull) domain R is a locally factorial Krull domain if and only if R is a
$\ast $
-domain. Consequently, a Mori (and hence a Krull) domain R is a locally factorial Krull domain if and only if R is a
![]() $\ast $
-domain.
$\ast $
-domain.
The proof can be easily constructed from the preceding comments, and so is left to the reader.
Considered in [Reference Anderson and Kang6] was also the notion of a pseudo principal ideal domain (pseudo PID) or, in our terminology, v-G-PID by requiring that
![]() $ A_{v}$
is principal, for each nonzero ideal A of
$ A_{v}$
is principal, for each nonzero ideal A of
![]() $R.$
This notion appeared in Bourbaki too, as pointed out in [Reference Anderson and Kang6]. Using the DC approach, one can prove the following result.
$R.$
This notion appeared in Bourbaki too, as pointed out in [Reference Anderson and Kang6]. Using the DC approach, one can prove the following result.
Theorem 3.4 A domain R is a v-G-PID if and only if R is a DC Schreier domain.
Proof Suppose that R is a DC Schreier domain. Now, a Schreier domain is a
![]() $\ast $
-domain too [Reference Zafrullah26, Corollary 1.7]. So, a DCD that is a Schreier domain is at least a v-G-Dedekind domain, by Theorem 3.3. But then,
$\ast $
-domain too [Reference Zafrullah26, Corollary 1.7]. So, a DCD that is a Schreier domain is at least a v-G-Dedekind domain, by Theorem 3.3. But then,
![]() $ A_{v}$
is invertible for each nonzero ideal A of R, and in a Schreier domain, every invertible ideal is principal [Reference Zafrullah26, Theorem 3.6]. Thus,
$ A_{v}$
is invertible for each nonzero ideal A of R, and in a Schreier domain, every invertible ideal is principal [Reference Zafrullah26, Theorem 3.6]. Thus,
![]() $ A_{v}$
is principal for each nonzero ideal A of R, and R is a v -G-PID. Conversely, note that a v-G-PID is DC and is at least a greatest common divisor (GCD) domain, and a GCD domain is Schreier [Reference Cohn9]. Thus, a v-G-PID R is a DC Schreier domain.▪
$ A_{v}$
is principal for each nonzero ideal A of R, and R is a v -G-PID. Conversely, note that a v-G-PID is DC and is at least a greatest common divisor (GCD) domain, and a GCD domain is Schreier [Reference Cohn9]. Thus, a v-G-PID R is a DC Schreier domain.▪
Theorem 3.5 Let
![]() $\star $
be a star operation defined on R such that for each
$\star $
be a star operation defined on R such that for each
![]() $A\in F(R), A^{\star }$
is a v-ideal of finite type. Then the following are equivalent.
$A\in F(R), A^{\star }$
is a v-ideal of finite type. Then the following are equivalent.
-
(1) R is a
 $\star $
-G-PID.
$\star $
-G-PID. -
(2) R is a Schreier domain.
-
(3) R is a
 $\star $
-G-Dedekind domain with
$\star $
-G-Dedekind domain with
 $Pic(R)=(0)$
.
$Pic(R)=(0)$
.
Proof (1)
![]() $\Leftrightarrow $
(2). A
$\Leftrightarrow $
(2). A
![]() $\star $
-G-PID, by definition, is a GCD domain and so a Schreier domain. Conversely, “for each
$\star $
-G-PID, by definition, is a GCD domain and so a Schreier domain. Conversely, “for each
![]() $A\in F(R)$
,
$A\in F(R)$
,
![]() $A^{\star }$
is a v-ideal of finite type” makes each
$A^{\star }$
is a v-ideal of finite type” makes each
![]() $A^{\star }\in F(R)$
a v-ideal of finite type. But then, for each
$A^{\star }\in F(R)$
a v-ideal of finite type. But then, for each
![]() $A\in F(D)$
,
$A\in F(D)$
,
![]() $A^{\star }$
is a finite intersection of principal fractional ideals and of finite type and by Theorem 3.6 of [Reference Zafrullah26] principal because R is Schreier.
$A^{\star }$
is a finite intersection of principal fractional ideals and of finite type and by Theorem 3.6 of [Reference Zafrullah26] principal because R is Schreier.
(1)
![]() $\Leftrightarrow $
(3).
$\Leftrightarrow $
(3).
![]() $\star $
-GPID is
$\star $
-GPID is
![]() $\star $
-G-Dedekind with every invertible ideal principal which is exactly (3).
$\star $
-G-Dedekind with every invertible ideal principal which is exactly (3).
For the converse, note that (3) implies that R is at least a GCD domain and a GCD domain is Schreier. That is, (2) holds, and (2) is equivalent to (1).▪
4 Related stuff
We end this article with some interesting characterizations of the
![]() $\star $
-GPIDs and a discussion of related material.
$\star $
-GPIDs and a discussion of related material.
Recall that a Riesz group is a directed group that satisfies the Riesz interpolation property: Given that
![]() $ x_{1},x_{2},\ldots ,x_{m};y_{1},y_{2},\ldots ,y_{n}\in G$
such that
$ x_{1},x_{2},\ldots ,x_{m};y_{1},y_{2},\ldots ,y_{n}\in G$
such that
![]() $x_{i}\leq y_{j}$
for all
$x_{i}\leq y_{j}$
for all
![]() $i\in \lbrack 1,m],j\in \lbrack 1,n]$
, there is
$i\in \lbrack 1,m],j\in \lbrack 1,n]$
, there is
![]() $z\in G$
such that
$z\in G$
such that
![]() $ x_{i}\leq z\leq y_{j}$
for all
$ x_{i}\leq z\leq y_{j}$
for all
![]() $(i,j)\in \lbrack 1,m]\times \lbrack 1,n])$
. It was shown in [Reference Zafrullah26] that the Riesz interpolation property translates in the commutative ring theory set up to: For all
$(i,j)\in \lbrack 1,m]\times \lbrack 1,n])$
. It was shown in [Reference Zafrullah26] that the Riesz interpolation property translates in the commutative ring theory set up to: For all
![]() $ x_{1},x_{2},\ldots ,x_{m};y_{1},y_{2},\ldots ,y_{n}\in K\backslash \{0\}$
with
$ x_{1},x_{2},\ldots ,x_{m};y_{1},y_{2},\ldots ,y_{n}\in K\backslash \{0\}$
with
![]() $ x_{1},x_{2},\ldots ,x_{m}\in \cap y_{i}R$
, there is a
$ x_{1},x_{2},\ldots ,x_{m}\in \cap y_{i}R$
, there is a
![]() $z\in \cap y_{i}R$
such that
$z\in \cap y_{i}R$
such that
![]() $(x_{1},\ldots ,x_{m})\subseteq zR\subseteq \cap y_{i}R$
. So, a pre-Schreier domain is actually a pre-Riesz domain. We have no interest in changing existing names; we only want to add a new name. Call R a super Riesz domain if, for any divisorial ideal A of R and for any set
$(x_{1},\ldots ,x_{m})\subseteq zR\subseteq \cap y_{i}R$
. So, a pre-Schreier domain is actually a pre-Riesz domain. We have no interest in changing existing names; we only want to add a new name. Call R a super Riesz domain if, for any divisorial ideal A of R and for any set
![]() $\{x_{\alpha }\}$
of elements contained in
$\{x_{\alpha }\}$
of elements contained in
![]() $A,$
with
$A,$
with
![]() $\cap (x_{\alpha })\neq (0),$
there is a
$\cap (x_{\alpha })\neq (0),$
there is a
![]() $d\in A$
such that
$d\in A$
such that
![]() $(x_{\alpha })\subseteq (d).$
Because a divisorial ideal is expressible as an intersection of principal fractional ideals, a super Riesz domain can be easily seen to be pre-Schreier. Furthermore, let us call a product
$(x_{\alpha })\subseteq (d).$
Because a divisorial ideal is expressible as an intersection of principal fractional ideals, a super Riesz domain can be easily seen to be pre-Schreier. Furthermore, let us call a product
![]() $AB$
of ideals
$AB$
of ideals
![]() $A,B$
subtle if, for each
$A,B$
subtle if, for each
![]() $x\in AB$
, we have
$x\in AB$
, we have
![]() $x=ab$
where
$x=ab$
where
![]() $a\in A$
and
$a\in A$
and
![]() $b\in B.$
The authors of [Reference Anderson and Kang6] also touched on the following question: Let R be an integral domain that satisfies
$b\in B.$
The authors of [Reference Anderson and Kang6] also touched on the following question: Let R be an integral domain that satisfies
![]() $(\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
for all subsets
$(\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
for all subsets
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
. Is R Pseudo-Dedekind?
$\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
. Is R Pseudo-Dedekind?
We try to give a partial answer below.
Proposition 1 Let R be an integral domain. Then the following are equivalent.
-
(1) For all allowable
 $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq K\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq K\backslash \{0\}$
, we have
 $ (\cap (a_{\alpha })) (\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
.
$ (\cap (a_{\alpha })) (\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
. -
(2) For all allowable
 $\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
, we have
 $ (\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
, and for all allowable sets
$ (\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
, and for all allowable sets
 $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have a
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we have a
 $d\in R\backslash \{0\}$
such that
$d\in R\backslash \{0\}$
such that
 $d(\cap (a_{\alpha }))$
is expressible as an intersection of principal integral ideals.
$d(\cap (a_{\alpha }))$
is expressible as an intersection of principal integral ideals. -
(3) R is a DCD that satisfies: For all allowable
 $\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
, we have
 $(\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
.
$(\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta })$
.
Proof (1)
![]() $\Rightarrow $
(2). Obvious because the first part follows directly and (1) means R is DC and so for each allowable set
$\Rightarrow $
(2). Obvious because the first part follows directly and (1) means R is DC and so for each allowable set
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we can find
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we can find
![]() $x_{1},\ldots ,x_{n}$
such that
$x_{1},\ldots ,x_{n}$
such that
![]() $ \cap (a_{\alpha })=\cap _{i=1}^{n}(x_{i})$
. But, for the right-hand side, we can find a nonzero d such that
$ \cap (a_{\alpha })=\cap _{i=1}^{n}(x_{i})$
. But, for the right-hand side, we can find a nonzero d such that
![]() $dx_{i}$
are all integral.
$dx_{i}$
are all integral.
(2)
![]() $\Rightarrow $
(1). Consider
$\Rightarrow $
(1). Consider
![]() $(\cap (a_{\alpha }))(\cap (b_{\beta }))$
for all allowable
$(\cap (a_{\alpha }))(\cap (b_{\beta }))$
for all allowable
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq K\backslash \{0\}$
. Since
$\{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq K\backslash \{0\}$
. Since
![]() $ \cap (a_{\alpha }),\cap (b_{\beta })$
are nonzero fractional ideals by the given property of R, we have, for some
$ \cap (a_{\alpha }),\cap (b_{\beta })$
are nonzero fractional ideals by the given property of R, we have, for some
![]() $r,s\in R$
,
$r,s\in R$
,
![]() $r(\cap (a_{\alpha }))=\cap (x_{\gamma })$
and
$r(\cap (a_{\alpha }))=\cap (x_{\gamma })$
and
![]() $s(\cap (b_{\beta }))= \cap (y_{\delta })$
, where
$s(\cap (b_{\beta }))= \cap (y_{\delta })$
, where
![]() $x_{\gamma }$
and
$x_{\gamma }$
and
![]() $y_{\delta }$
are in
$y_{\delta }$
are in
![]() $R,$
by (2). But then,
$R,$
by (2). But then,
![]() $(\cap (x_{\gamma }))(\cap (y_{\delta }))=\cap (x_{\gamma }y_{\delta })=P$
. Now,
$(\cap (x_{\gamma }))(\cap (y_{\delta }))=\cap (x_{\gamma }y_{\delta })=P$
. Now,
![]() $ P=\cap (x_{\gamma }y_{\delta }) =\cap _{\gamma }x_{\gamma }(\cap (y_{\delta }))$
(substituting for
$ P=\cap (x_{\gamma }y_{\delta }) =\cap _{\gamma }x_{\gamma }(\cap (y_{\delta }))$
(substituting for
![]() $\cap (y_{\delta })$
)
$\cap (y_{\delta })$
)
![]() $=\cap _{\gamma }x_{\gamma }(\cap (sb_{\beta }))=\cap _{\gamma ,\beta }(x_{\gamma }sb_{\beta })=\cap _{\beta }sb_{\beta }(\cap (x_{\gamma }))$
and substituting for
$=\cap _{\gamma }x_{\gamma }(\cap (sb_{\beta }))=\cap _{\gamma ,\beta }(x_{\gamma }sb_{\beta })=\cap _{\beta }sb_{\beta }(\cap (x_{\gamma }))$
and substituting for
![]() $\cap (x_{\gamma })$
) we get
$\cap (x_{\gamma })$
) we get
![]() $P=\cap _{\beta }sb_{\beta }(\cap (ra_{\alpha }))=\cap (ra_{\alpha }sb_{\beta })=rs(\cap (a_{\alpha }b_{\beta }))$
. But, on the other hand,
$P=\cap _{\beta }sb_{\beta }(\cap (ra_{\alpha }))=\cap (ra_{\alpha }sb_{\beta })=rs(\cap (a_{\alpha }b_{\beta }))$
. But, on the other hand,
![]() $P=(\cap (x_{\gamma }))(\cap (y_{\delta }))=(r(\cap (a_{\alpha })))(s(\cap (b_{\beta })))$
. Thus,
$P=(\cap (x_{\gamma }))(\cap (y_{\delta }))=(r(\cap (a_{\alpha })))(s(\cap (b_{\beta })))$
. Thus,
![]() $rs(\cap (a_{\alpha }))(\cap (b_{\beta }))=rs(\cap (a_{\alpha }b_{\beta })).$
Canceling
$rs(\cap (a_{\alpha }))(\cap (b_{\beta }))=rs(\cap (a_{\alpha }b_{\beta })).$
Canceling
![]() $rs$
from both sides, we get the desired equality.
$rs$
from both sides, we get the desired equality.
(1)
![]() $\Rightarrow $
(3). Obvious, in light of (1)
$\Rightarrow $
(3). Obvious, in light of (1)
![]() $\Rightarrow $
(2).
$\Rightarrow $
(2).
(3)
![]() $\Rightarrow $
(2). Note that because R is DC for each allowable set
$\Rightarrow $
(2). Note that because R is DC for each allowable set
![]() $ \{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we can find
$ \{a_{\alpha }\}_{\alpha \in I}\subseteq K\backslash \{0\}$
, we can find
![]() $ x_{1},\ldots ,x_{n}$
such that
$ x_{1},\ldots ,x_{n}$
such that
![]() $(\cap (a_{\alpha }))=(\cap _{i=1}^{n}(x_{i}))$
and so a
$(\cap (a_{\alpha }))=(\cap _{i=1}^{n}(x_{i}))$
and so a
![]() $d\in R\backslash \{0\}$
such that
$d\in R\backslash \{0\}$
such that
![]() $dx_{i}\in R.$
But then,
$dx_{i}\in R.$
But then,
![]() $d(\cap (a_{\alpha }))=\cap _{i-1}^{n}dx_{i}$
an intersection of principal fractional ideals.▪
$d(\cap (a_{\alpha }))=\cap _{i-1}^{n}dx_{i}$
an intersection of principal fractional ideals.▪
Recall from [Reference Zafrullah26], again, that R is a pre-Schreier domain if and only if, for all
![]() $\{a_{1},\ldots ,a_{m}\},\{b_{1},\ldots ,b_{n}\}\subseteq R\backslash \{0\},a_{i}b_{j}|x$
implies
$\{a_{1},\ldots ,a_{m}\},\{b_{1},\ldots ,b_{n}\}\subseteq R\backslash \{0\},a_{i}b_{j}|x$
implies
![]() $x=rs$
where
$x=rs$
where
![]() $a_{i}|r$
and
$a_{i}|r$
and
![]() $b_{j}|s.$
Since this property sprang in the context of pre-Schreier domains, we can call a domain R super pre-Schreier if
$b_{j}|s.$
Since this property sprang in the context of pre-Schreier domains, we can call a domain R super pre-Schreier if
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
such that
$\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
such that
![]() $a_{\alpha }b_{\beta }|x$
, then
$a_{\alpha }b_{\beta }|x$
, then
![]() $x=rs$
such that
$x=rs$
such that
![]() $a_{\alpha }|r$
and
$a_{\alpha }|r$
and
![]() $b_{\beta }|s,$
and ask if a super pre-Schreier domain must be a v-GPID. The reason for this question is provided by the following proposition.
$b_{\beta }|s,$
and ask if a super pre-Schreier domain must be a v-GPID. The reason for this question is provided by the following proposition.
Proposition 2 An integral domain R is super pre-Schreier if and only if, for all allowable
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
, we have
![]() $(\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta }) $
such that for each
$(\cap (a_{\alpha }))(\cap (b_{\beta }))=\cap (a_{\alpha }b_{\beta }) $
such that for each
![]() $x\in (\cap (a_{\alpha }))(\cap (b_{\beta }))$
,
$x\in (\cap (a_{\alpha }))(\cap (b_{\beta }))$
,
![]() $ x=rs $
where
$ x=rs $
where
![]() $r\in \cap (a_{\alpha })$
and
$r\in \cap (a_{\alpha })$
and
![]() $s\in \cap (b_{\beta })$
.
$s\in \cap (b_{\beta })$
.
Proof Suppose that R is super pre-Schreier. Then
![]() $(\cap (a_{\alpha }))(\cap (b_{\beta }))\subseteq (\cap (a_{\alpha }b_{\beta }))$
holds, always. So let
$(\cap (a_{\alpha }))(\cap (b_{\beta }))\subseteq (\cap (a_{\alpha }b_{\beta }))$
holds, always. So let
![]() $x\in \cap (a_{\alpha }b_{\beta }).$
This means
$x\in \cap (a_{\alpha }b_{\beta }).$
This means
![]() $a_{\alpha }b_{\beta }|x.$
But super pre-Schreier property requires that
$a_{\alpha }b_{\beta }|x.$
But super pre-Schreier property requires that
![]() $x=rs$
where
$x=rs$
where
![]() $r\in \cap (a_{\alpha })$
and
$r\in \cap (a_{\alpha })$
and
![]() $s\in \cap (b_{\beta })$
and that puts
$s\in \cap (b_{\beta })$
and that puts
![]() $x\in (\cap (a_{\alpha }))(\cap (b_{\beta }))$
and the other requirement is met. The converse is a similar translation.▪
$x\in (\cap (a_{\alpha }))(\cap (b_{\beta }))$
and the other requirement is met. The converse is a similar translation.▪
Call the product
![]() $IJ$
of two nonzero integral ideals
$IJ$
of two nonzero integral ideals
![]() $I,J$
of R subtle if each
$I,J$
of R subtle if each
![]() $d\in IJ\backslash \{0\}$
can be written as
$d\in IJ\backslash \{0\}$
can be written as
![]() $d=rs$
where
$d=rs$
where
![]() $r\in I$
and
$r\in I$
and
![]() $ s\in J.$
It was shown in [Reference Zafrullah26] that R is pre-Schreier if and only if R has the property
$ s\in J.$
It was shown in [Reference Zafrullah26] that R is pre-Schreier if and only if R has the property
![]() $\ast $
and for each pair of subsets
$\ast $
and for each pair of subsets
![]() $ \{a_{1},\ldots ,a_{m}\}, \{b_{1},\ldots ,b_{n}\}\subseteq R\backslash \{0\}$
the product
$ \{a_{1},\ldots ,a_{m}\}, \{b_{1},\ldots ,b_{n}\}\subseteq R\backslash \{0\}$
the product
![]() $(\cap _{i=1}^{n}(a_{i}))\left ( \cap _{i=1}^{n}(a_{i})\right ) $
is subtle. (Following an earlier version of [Reference Zafrullah26], Anderson and Dobbs [Reference Anderson and Dobbs8] studied domains products of whose ideals were all subtle.)
$(\cap _{i=1}^{n}(a_{i}))\left ( \cap _{i=1}^{n}(a_{i})\right ) $
is subtle. (Following an earlier version of [Reference Zafrullah26], Anderson and Dobbs [Reference Anderson and Dobbs8] studied domains products of whose ideals were all subtle.)
Corollary 5 The following are equivalent for an integral domain
![]() $R.$
(1) For all allowable
$R.$
(1) For all allowable
![]() $\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
, we have
$\{a_{\alpha }\}_{\alpha \in I}\subseteq R\backslash \{0\}, \{b_{\beta }\}_{\beta \in J}\subseteq R\backslash \{0\}$
, we have
![]() $ (\cap (a_{\alpha }))(\cap (b_{\beta }))=(\cap (a_{\alpha }b_{\beta }))$
such that for each
$ (\cap (a_{\alpha }))(\cap (b_{\beta }))=(\cap (a_{\alpha }b_{\beta }))$
such that for each
![]() $x\in (\cap (a_{\alpha }))(\cap (b_{\beta }))$
,
$x\in (\cap (a_{\alpha }))(\cap (b_{\beta }))$
,
![]() $x=rs$
where
$x=rs$
where
![]() $ r\in \cap (a_{\alpha })$
and
$ r\in \cap (a_{\alpha })$
and
![]() $s\in \cap (b_{\beta }),$
(2) for every pair of nonzero ideals
$s\in \cap (b_{\beta }),$
(2) for every pair of nonzero ideals
![]() $A,B$
, we have
$A,B$
, we have
![]() $(AB)_{v}=A_{v}B_{v}$
, and for every nonzero integral ideal A of R, there is a
$(AB)_{v}=A_{v}B_{v}$
, and for every nonzero integral ideal A of R, there is a
![]() $d\in R\backslash \{0\}$
with
$d\in R\backslash \{0\}$
with
![]() $dA_{v}$
an intersection of principal integral ideals and the product is subtle, and (3) R is a super pre-Schreier domain.
$dA_{v}$
an intersection of principal integral ideals and the product is subtle, and (3) R is a super pre-Schreier domain.
Finally, a word about DCDs. Indeed, a DCD can be characterized by:
![]() $A_{v}$
is a v-ideal of finite type for each
$A_{v}$
is a v-ideal of finite type for each
![]() $A\in F(R)$
. The first thing that comes to mind as a general property is that
$A\in F(R)$
. The first thing that comes to mind as a general property is that
![]() $A^{-1}$
is of finite type for each
$A^{-1}$
is of finite type for each
![]() $ A\in F(R)$
. This leads to the following result. But we need to recall some terminology. R is
$ A\in F(R)$
. This leads to the following result. But we need to recall some terminology. R is
![]() $\star $
-Prüfer if, for each finitely generated
$\star $
-Prüfer if, for each finitely generated
![]() $A\in F(R)$
, A is
$A\in F(R)$
, A is
![]() $\star $
-invertible [Reference Anderson, Anderson, Fontana and Zafrullah4]. Since, for
$\star $
-invertible [Reference Anderson, Anderson, Fontana and Zafrullah4]. Since, for
![]() $A\in F(R), A$
being
$A\in F(R), A$
being
![]() $ \star $
-invertible implies
$ \star $
-invertible implies
![]() $A^{\star }=A_{v}$
. So, for each finitely generated nonzero ideal J in a
$A^{\star }=A_{v}$
. So, for each finitely generated nonzero ideal J in a
![]() $\star $
-Prüfer domain R we have
$\star $
-Prüfer domain R we have
![]() $ J^{\star }=J_{v}.$
Furthermore, as
$ J^{\star }=J_{v}.$
Furthermore, as
![]() $(JJ^{-1})^{\star }=R$
implies
$(JJ^{-1})^{\star }=R$
implies
![]() $((JJ^{-1})^{\star })_{v}=(JJ^{-1})_{v}=R$
, a
$((JJ^{-1})^{\star })_{v}=(JJ^{-1})_{v}=R$
, a
![]() $\star $
-Prüfer domain is a v-domain and
$\star $
-Prüfer domain is a v-domain and
![]() $ \star _{f}=t.$
If
$ \star _{f}=t.$
If
![]() $\star $
is of finite character, then
$\star $
is of finite character, then
![]() $A^{-1}$
is a v -ideal of finite type for each finitely generated
$A^{-1}$
is a v -ideal of finite type for each finitely generated
![]() $A\in F(R)$
and so, if
$A\in F(R)$
and so, if
![]() $ \star $
is of finite type, a
$ \star $
is of finite type, a
![]() $\star $
-Prüfer domain is a Prüfer
$\star $
-Prüfer domain is a Prüfer
![]() $ \star $
-multiplication domain and,
$ \star $
-multiplication domain and,
![]() $\star =t$
.
$\star =t$
.
![]() $\star $
-Prüfer domains, for a finite character
$\star $
-Prüfer domains, for a finite character
![]() $\star $
-operation
$\star $
-operation
![]() $\star $
, were studied in [Reference Houston, Malik and Mott17] where they were called
$\star $
, were studied in [Reference Houston, Malik and Mott17] where they were called
![]() $\star $
-multiplication domains. They were later called Prüfer
$\star $
-multiplication domains. They were later called Prüfer
![]() $\star $
-multiplication domains (P
$\star $
-multiplication domains (P
![]() $\star $
MDs). An in-depth study of these domains can be found in [Reference Fontana, Jara and Santos12], along with an introduction to semistar operations. These days this concept is defined by:
$\star $
MDs). An in-depth study of these domains can be found in [Reference Fontana, Jara and Santos12], along with an introduction to semistar operations. These days this concept is defined by:
![]() $ R$
is a P
$ R$
is a P
![]() $\star $
MDs, for a star operation
$\star $
MDs, for a star operation
![]() $\star $
, if, for each finitely generated
$\star $
, if, for each finitely generated
![]() $A\in F(R)$
, A is
$A\in F(R)$
, A is
![]() $\star _{f}$
-invertible. If R is a P
$\star _{f}$
-invertible. If R is a P
![]() $\star $
MD for a finite character star operation, then
$\star $
MD for a finite character star operation, then
![]() $\star =t$
over R. On the other hand, if R is a P
$\star =t$
over R. On the other hand, if R is a P
![]() $\star $
MD for a “general” star-operation
$\star $
MD for a “general” star-operation
![]() $\star ,$
then
$\star ,$
then
![]() $\star _{f}=t$
.
$\star _{f}=t$
.
Proposition 3 Let R be a DCD, and let
![]() $\star $
be a star operation defined on R. If A is
$\star $
be a star operation defined on R. If A is
![]() $\star $
-invertible, for
$\star $
-invertible, for
![]() $A\in F(R)$
, then
$A\in F(R)$
, then
![]() $ A^{\star }$
is
$ A^{\star }$
is
![]() $\star _{f}$
-invertible. Consequently a DCD R is a P
$\star _{f}$
-invertible. Consequently a DCD R is a P
![]() $\star $
MD if and only if R is a
$\star $
MD if and only if R is a
![]() $\star $
-Prüfer domain.
$\star $
-Prüfer domain.
Proof Note that A being
![]() $\star _{f}$
-invertible means that
$\star _{f}$
-invertible means that
![]() $A^{-1}$
is a v -ideal of finite type. But
$A^{-1}$
is a v -ideal of finite type. But
![]() $A^{-1}$
is a v-ideal of finite type in a DCD. Thus, a DC
$A^{-1}$
is a v-ideal of finite type in a DCD. Thus, a DC
![]() $\star $
-Prüfer domain is a P
$\star $
-Prüfer domain is a P
![]() $\star $
MD. The converse is obvious in the case of a DCD.▪
$\star $
MD. The converse is obvious in the case of a DCD.▪
Remark 4.1 It may be noted that there is a marked difference between “A is
![]() $\star _{f}$
-invertible” and “A
$\star _{f}$
-invertible” and “A
![]() ${}^{\star }$
is
${}^{\star }$
is
![]() $\star _{f}$
-invertible.” That is if you require that every
$\star _{f}$
-invertible.” That is if you require that every
![]() $A\in F(R)$
is
$A\in F(R)$
is
![]() $\star _{f}$
-invertible, you will end up with a Krull domain, for
$\star _{f}$
-invertible, you will end up with a Krull domain, for
![]() $\star _{f}$
-invertible is t-invertible and every
$\star _{f}$
-invertible is t-invertible and every
![]() $A\in F(R)$
being t-invertible implies that R is Krull [Reference Jaffard19, p. 82]. On the other hand, if you require that, for each
$A\in F(R)$
being t-invertible implies that R is Krull [Reference Jaffard19, p. 82]. On the other hand, if you require that, for each
![]() $A\in F(R)$
, the ideal
$A\in F(R)$
, the ideal
![]() $A^{\star }$
is
$A^{\star }$
is
![]() $\star _{f}$
-invertible, you get a PVMD that acts and behaves very much like
$\star _{f}$
-invertible, you get a PVMD that acts and behaves very much like
![]() $\star $
-G-Dedekind domains. These domains were studied under the name of pre-Krull domains in [Reference Zafrullah27], just for
$\star $
-G-Dedekind domains. These domains were studied under the name of pre-Krull domains in [Reference Zafrullah27], just for
![]() $\star =v$
and later, under the name of
$\star =v$
and later, under the name of
![]() $ (t,v)$
-Dedekind domains, in [Reference Anderson, Anderson, Fontana and Zafrullah4], essentially based on the line taken in [Reference Zafrullah27], describing the
$ (t,v)$
-Dedekind domains, in [Reference Anderson, Anderson, Fontana and Zafrullah4], essentially based on the line taken in [Reference Zafrullah27], describing the
![]() $(t,v)$
-Dedekind domains as domains in which
$(t,v)$
-Dedekind domains as domains in which
![]() $(AB)^{-1}=(A^{-1}B^{-1})^{t},$
or as domains in which
$(AB)^{-1}=(A^{-1}B^{-1})^{t},$
or as domains in which
![]() $A_{v}$
is t -invertible for each
$A_{v}$
is t -invertible for each
![]() $A\in F(R)$
. The closest to that [Reference Anderson, Anderson, Fontana and Zafrullah4] came to was in its Theorem 1.14. These and similar concepts were also studied by Halter-Koch under “mixed invertibility.” Hopefully, with the introduction of DC property, the situation will become clearer.
$A\in F(R)$
. The closest to that [Reference Anderson, Anderson, Fontana and Zafrullah4] came to was in its Theorem 1.14. These and similar concepts were also studied by Halter-Koch under “mixed invertibility.” Hopefully, with the introduction of DC property, the situation will become clearer.
For now, we have the following corollary.
Corollary 6 The following are equivalent for an integral domain R, with a star operation
![]() $\star $
defined on it.
$\star $
defined on it.
-
(1)
 $A^{\star }$
is
$A^{\star }$
is
 $\star _{f}$
-invertible for each
$\star _{f}$
-invertible for each
 $A\in F(R)$
.
$A\in F(R)$
. -
(2) In R, we have A is
 $\star $
-invertible and
$\star $
-invertible and
 $(AB)^{\star }=(A^{\star }B^{\star })^{\star _{f}}$
, for all
$(AB)^{\star }=(A^{\star }B^{\star })^{\star _{f}}$
, for all
 $A,B\in F(R)$
.
$A,B\in F(R)$
. -
(3) R is a
 $\star $
-DC
$\star $
-DC
 $\star $
-Prüfer domain.
$\star $
-Prüfer domain. -
(4) R is a
 $\star $
-CICD and
$\star $
-CICD and
 $(AB)^{\star }=(A^{\star }B^{\star })^{\star _{f}},$
for all
$(AB)^{\star }=(A^{\star }B^{\star })^{\star _{f}},$
for all
 $A,B\in F(R)$
.
$A,B\in F(R)$
.
Proof (1)
![]() $\Rightarrow $
(2). Let
$\Rightarrow $
(2). Let
![]() $A^{\star }$
be
$A^{\star }$
be
![]() $\star _{f}$
-invertible, for all
$\star _{f}$
-invertible, for all
![]() $A\in F(R).$
Then
$A\in F(R).$
Then
![]() $A^{\star }$
and hence A are
$A^{\star }$
and hence A are
![]() $\star $
-invertible, forcing
$\star $
-invertible, forcing
![]() $A^{\star }=A_{v}$
[Reference Zafrullah, Glaz and Chapman28], for all
$A^{\star }=A_{v}$
[Reference Zafrullah, Glaz and Chapman28], for all
![]() $A\in F(R).$
Thus,
$A\in F(R).$
Thus,
![]() $\star =v$
over
$\star =v$
over
![]() $ R $
. Moreover, as
$ R $
. Moreover, as
![]() $\star _{f}$
is of finite character,
$\star _{f}$
is of finite character,
![]() $A^{\star }$
is a
$A^{\star }$
is a
![]() $ \star $
-ideal of finite type, because
$ \star $
-ideal of finite type, because
![]() $A^{\star }$
is
$A^{\star }$
is
![]() $\star _{f}$
-invertible
$\star _{f}$
-invertible
![]() $.$
Now, consider for
$.$
Now, consider for
![]() $A,B\in R$
the product
$A,B\in R$
the product
![]() $(AB)^{\star }$
. Indeed,
$(AB)^{\star }$
. Indeed,
![]() $ (AB)^{\star }=(A^{\star }B^{\star })^{\star }$
. Because each of
$ (AB)^{\star }=(A^{\star }B^{\star })^{\star }$
. Because each of
![]() $A^{\star },$
$A^{\star },$
![]() $B^{\star }$
is of finite type,
$B^{\star }$
is of finite type,
![]() $(AB)^{\star }=(A^{\star }B^{\star })^{\star _{f}}.$
$(AB)^{\star }=(A^{\star }B^{\star })^{\star _{f}}.$
(2)
![]() $\Rightarrow $
(1).
$\Rightarrow $
(1).
![]() $R=(AA^{-1})^{\star }=(A^{\star }A^{-1})^{\star _{f}} $
, by (2).
$R=(AA^{-1})^{\star }=(A^{\star }A^{-1})^{\star _{f}} $
, by (2).
(2)
![]() $\Rightarrow $
(3). By (2), A is
$\Rightarrow $
(3). By (2), A is
![]() $\star $
-invertible for every
$\star $
-invertible for every
![]() $A\in F(R)$
we conclude that R is a
$A\in F(R)$
we conclude that R is a
![]() $\star $
-CICD and hence
$\star $
-CICD and hence
![]() $\star $
-Prüfer. Since by (2) we have
$\star $
-Prüfer. Since by (2) we have
![]() $(AB)^{\star }=(A^{\star }B^{\star })^{\star _{f}}$
, we conclude that
$(AB)^{\star }=(A^{\star }B^{\star })^{\star _{f}}$
, we conclude that
![]() $R=(AA^{-1})^{\star }=(A^{\star }A^{-1})^{\star _{f}}$
which established that
$R=(AA^{-1})^{\star }=(A^{\star }A^{-1})^{\star _{f}}$
which established that
![]() $A^{\star }$
is a
$A^{\star }$
is a
![]() $\star $
-ideal of finite type. But, as
$\star $
-ideal of finite type. But, as
![]() $ \star =v$
, we conclude that R is
$ \star =v$
, we conclude that R is
![]() $\star $
-DC.
$\star $
-DC.
(3)
![]() $\Rightarrow $
(1). Follows from Proposition 3.
$\Rightarrow $
(1). Follows from Proposition 3.
Finally, (4) and (2) are restatements of each other.▪
The domain characterized by Corollary 6 is a completely integrally closed PVMD, that is a Dedekind domain for
![]() $\star =d$
, a Krull domain for
$\star =d$
, a Krull domain for
![]() $\star $
of finite character, or for
$\star $
of finite character, or for
![]() $\star =t,$
and a pre-Krull domain of [Reference Zafrullah27] for a star operation
$\star =t,$
and a pre-Krull domain of [Reference Zafrullah27] for a star operation
![]() $\star $
that is not of finite type, or
$\star $
that is not of finite type, or
![]() $\star =v$
. The following proposition is a reason why I tend to call these domains
$\star =v$
. The following proposition is a reason why I tend to call these domains
![]() $(\star ,v)$
-G-Dedekind domains. For this, let us recall that R is
$(\star ,v)$
-G-Dedekind domains. For this, let us recall that R is
![]() $\star $
-DC if, for each
$\star $
-DC if, for each
![]() $A\in F(R)$
, we have that
$A\in F(R)$
, we have that
![]() $A^{\star }$
is a v-ideal of finite type.
$A^{\star }$
is a v-ideal of finite type.
Proposition 4 A
![]() $(\star ,v)$
-G-Dedekind domain R is a
$(\star ,v)$
-G-Dedekind domain R is a
![]() $\star $
-G-Dedekind domain if and only if R is a
$\star $
-G-Dedekind domain if and only if R is a
![]() $\ast $
-domain.
$\ast $
-domain.
Proof A
![]() $(\star ,v)$
-G-Dedekind domain R is
$(\star ,v)$
-G-Dedekind domain R is
![]() $\star $
-DC, with
$\star $
-DC, with
![]() $\star =v$
, and so DC, whereas a DC
$\star =v$
, and so DC, whereas a DC
![]() $\ast $
-domain is a indeed a
$\ast $
-domain is a indeed a
![]() $\star $
-G-Dedekind domain, by Theorem 3.3. Conversely, a
$\star $
-G-Dedekind domain, by Theorem 3.3. Conversely, a
![]() $\star $
-G-Dedekind domain is a
$\star $
-G-Dedekind domain is a
![]() $\ast $
-domain and DC. This means that it is
$\ast $
-domain and DC. This means that it is
![]() $\star $
-DC, and as every
$\star $
-DC, and as every
![]() $\star $
-ideal in a
$\star $
-ideal in a
![]() $\star $
-G-Dedekind domain is invertible, we conclude that a
$\star $
-G-Dedekind domain is invertible, we conclude that a
![]() $ \star $
-G-Dedekind domain is a
$ \star $
-G-Dedekind domain is a
![]() $\ast $
-domain that is a
$\ast $
-domain that is a
![]() $(\star ,v)$
-G-Dedekind domain for every finite type star operation
$(\star ,v)$
-G-Dedekind domain for every finite type star operation
![]() $\star $
.▪
$\star $
.▪
It may be noted that although
![]() $(\star ,v)$
-G-Dedekind domains have been studied before, Corollary 6 and Proposition 4 provide a better view and highlight the connection of v-G-Dedekind domains with their generalization, the
$(\star ,v)$
-G-Dedekind domains have been studied before, Corollary 6 and Proposition 4 provide a better view and highlight the connection of v-G-Dedekind domains with their generalization, the
![]() $(\star ,v)$
-G-Dedekind domains. Now let’s take a look at some general properties of DCDs.
$(\star ,v)$
-G-Dedekind domains. Now let’s take a look at some general properties of DCDs.
It was indicated in [Reference Zafrullah25], using the example of the ring of entire functions, a v-G-Dedekind domain does not behave well under localizations. Using the same example, we can conclude that a DCD does not behave well under localization. But let us be clear about that example. Let
![]() $\mathcal {E}$
denote the ring of entire functions. Using the fact that
$\mathcal {E}$
denote the ring of entire functions. Using the fact that
![]() $\mathcal {E}$
is a Bezout domain and that an element s of
$\mathcal {E}$
is a Bezout domain and that an element s of
![]() $\mathcal {E}$
that is not divisible by any principal (height one) prime (i.e., one that does not have a zero) is a unit, one can show that
$\mathcal {E}$
that is not divisible by any principal (height one) prime (i.e., one that does not have a zero) is a unit, one can show that
![]() $\mathcal {E=\cap E}_{(p)}$
where p ranges over principal height one primes. In fact, we have the following lemma.
$\mathcal {E=\cap E}_{(p)}$
where p ranges over principal height one primes. In fact, we have the following lemma.
Lemma 4.2 Let R be a GCD domain such that an element
![]() $s\in R\backslash \{0\}$
is a nonunit if and only if s is divisible by at least one principal prime of
$s\in R\backslash \{0\}$
is a nonunit if and only if s is divisible by at least one principal prime of
![]() $R.$
Then
$R.$
Then
![]() $R=\cap R_{(p)}$
where p ranges over principal height one primes of
$R=\cap R_{(p)}$
where p ranges over principal height one primes of
![]() $R.$
Such a ring is completely integrally closed.
$R.$
Such a ring is completely integrally closed.
Proof Obviously, if R has no principal height, one primes then R is a field and
![]() $ R$
is trivially an intersection of localizations at its height one primes. So let us assume that R does have a set
$ R$
is trivially an intersection of localizations at its height one primes. So let us assume that R does have a set
![]() $\wp $
of principal height one primes and set
$\wp $
of principal height one primes and set
![]() $S=\cap _{p\in \wp }R_{(p)}.$
We already have
$S=\cap _{p\in \wp }R_{(p)}.$
We already have
![]() $R\subseteq \cap R_{(p)}.$
If there is
$R\subseteq \cap R_{(p)}.$
If there is
![]() $x\in S\backslash R,$
then
$x\in S\backslash R,$
then
![]() $x=r/s$
, and we can assume that
$x=r/s$
, and we can assume that
![]() $GCD(r,s)=1.$
Now, as
$GCD(r,s)=1.$
Now, as
![]() $pR_{(p)}$
is of height one and R is a GCD domain,
$pR_{(p)}$
is of height one and R is a GCD domain,
![]() $R_{(p)}$
is a discrete rank one valuation domain. Next,
$R_{(p)}$
is a discrete rank one valuation domain. Next,
![]() $r/s \in R_{(p)}$
for
$r/s \in R_{(p)}$
for
![]() $p\in \wp $
forces s to be a unit in
$p\in \wp $
forces s to be a unit in
![]() $R_{(p)}$
meaning s is not divisible by
$R_{(p)}$
meaning s is not divisible by
![]() $p.$
But then,
$p.$
But then,
![]() $x=r/s$
where s is not divisible by any of the
$x=r/s$
where s is not divisible by any of the
![]() $p\in \wp .$
This forces s to be a unit, by the rule. But then, x is an associate of
$p\in \wp .$
This forces s to be a unit, by the rule. But then, x is an associate of
![]() $r\in R.$
Thus,
$r\in R.$
Thus,
![]() $R=\cap R_{(p)}.$
Finally, each
$R=\cap R_{(p)}.$
Finally, each
![]() $R_{(p)}$
for each
$R_{(p)}$
for each
![]() $p\in \wp $
is a discrete rank one valuation domain and hence completely integrally closed, and an intersection of CICDs is completely integrally closed.▪
$p\in \wp $
is a discrete rank one valuation domain and hence completely integrally closed, and an intersection of CICDs is completely integrally closed.▪
Thus, we see the reason why one concludes that
![]() $\mathcal {E}$
is completely integrally closed. Now,
$\mathcal {E}$
is completely integrally closed. Now,
![]() $\mathcal {E}$
is Bezout and hence a PVMD such that every nonzero ideal of
$\mathcal {E}$
is Bezout and hence a PVMD such that every nonzero ideal of
![]() $\mathcal {E}$
is a t-ideal. Following [Reference Houston16], let P be a nonzero nonmaximal prime t-ideal of a PVMD R and set
$\mathcal {E}$
is a t-ideal. Following [Reference Houston16], let P be a nonzero nonmaximal prime t-ideal of a PVMD R and set
![]() $ S=\cap R_{M}$
where M ranges over all the maximal t-ideals which do not contain
$ S=\cap R_{M}$
where M ranges over all the maximal t-ideals which do not contain
![]() $P.$
Then
$P.$
Then
![]() $P^{-1}=R_{P}\cap S$
[Reference Houston16, Proposition 1.1]. (Houston had this to say about mentioning [Reference Houston16, Proposition 1.1]: You are applying it to a Prüfer domain, so Theorem 3.2 of the Huckaba–Papick paper [Reference Huckaba and Papick18], on which my paper is based, probably should take precedence. I would go even further. You can give a very simple proof as follows: Let P be a prime ideal of height
$P^{-1}=R_{P}\cap S$
[Reference Houston16, Proposition 1.1]. (Houston had this to say about mentioning [Reference Houston16, Proposition 1.1]: You are applying it to a Prüfer domain, so Theorem 3.2 of the Huckaba–Papick paper [Reference Huckaba and Papick18], on which my paper is based, probably should take precedence. I would go even further. You can give a very simple proof as follows: Let P be a prime ideal of height
![]() $>1$
[maximal or not]. Let
$>1$
[maximal or not]. Let
![]() $u\in P^{-1}$
. Then
$u\in P^{-1}$
. Then
![]() $P\subseteq (\mathcal {E}:u)$
. If
$P\subseteq (\mathcal {E}:u)$
. If
![]() $(\mathcal {E}:u)$
is not all of
$(\mathcal {E}:u)$
is not all of
![]() $\mathcal {E}$
, then it is a proper principal ideal [since
$\mathcal {E}$
, then it is a proper principal ideal [since
![]() $\mathcal {E}$
is Bezout] and must be contained in some principal prime. But this puts P inside a principal prime, a contradiction.) The reader may note the reference and the simple fact. As far as I am concerned, given any prime ideal P of
$\mathcal {E}$
is Bezout] and must be contained in some principal prime. But this puts P inside a principal prime, a contradiction.) The reader may note the reference and the simple fact. As far as I am concerned, given any prime ideal P of
![]() $\mathcal {E}$
of height greater than one, we must have
$\mathcal {E}$
of height greater than one, we must have
![]() $P_{v}$
principal, as noted on page 292 of [Reference Zafrullah25]. Now, if
$P_{v}$
principal, as noted on page 292 of [Reference Zafrullah25]. Now, if
![]() $P_{v}=d\mathcal {E} \neq \mathcal {E}$
, P must be contained in a principal prime. But, in
$P_{v}=d\mathcal {E} \neq \mathcal {E}$
, P must be contained in a principal prime. But, in
![]() $ \mathcal {E}$
, every principal prime is of height one. Thus, whatever the reference and whatever the reason, the following result stands.
$ \mathcal {E}$
, every principal prime is of height one. Thus, whatever the reference and whatever the reason, the following result stands.
Proposition 5 Let P be a nonzero prime of
![]() $\mathcal {E}$
of height greater than one. Then
$\mathcal {E}$
of height greater than one. Then
![]() $P_{v}=\mathcal {E}$
.
$P_{v}=\mathcal {E}$
.
Proof Note that P is contained in no height one prime of
![]() $\mathcal {E}$
. But then, principal primes are maximal (t-) ideals in
$\mathcal {E}$
. But then, principal primes are maximal (t-) ideals in
![]() $\mathcal {E}$
and so by Lemma 4.2,
$\mathcal {E}$
and so by Lemma 4.2,
![]() $S=\mathcal {E}$
, forcing
$S=\mathcal {E}$
, forcing
![]() $P^{-1}=\mathcal {E}_{P}\cap \mathcal {E=E}$
. Indeed, then
$P^{-1}=\mathcal {E}_{P}\cap \mathcal {E=E}$
. Indeed, then
![]() $P_{v}=\mathcal {E}$
. Finally, if M is a maximal ideal of
$P_{v}=\mathcal {E}$
. Finally, if M is a maximal ideal of
![]() $\mathcal {E}$
of height greater than one, then M must contain a nonzero nonmaximal prime
$\mathcal {E}$
of height greater than one, then M must contain a nonzero nonmaximal prime
![]() $\wp $
of height greater than one. But then,
$\wp $
of height greater than one. But then,
![]() $ \mathcal {E=(\wp )}_{v}\subseteq M_{v}$
.▪
$ \mathcal {E=(\wp )}_{v}\subseteq M_{v}$
.▪
Corollary 7 In
![]() $\mathcal {E}$
it is possible to have a multiplicative set S and an ideal A such that
$\mathcal {E}$
it is possible to have a multiplicative set S and an ideal A such that
![]() $(A\mathcal {E} _{S})_{v^{\prime }}\subsetneq (A_{v_{\mathcal {E}}}\mathcal {E} _{S})_{v^{\prime }}$
where
$(A\mathcal {E} _{S})_{v^{\prime }}\subsetneq (A_{v_{\mathcal {E}}}\mathcal {E} _{S})_{v^{\prime }}$
where
![]() $v^{\prime }$
and
$v^{\prime }$
and
![]() $v_{\mathcal {E}}$
are the v -operations in
$v_{\mathcal {E}}$
are the v -operations in
![]() $\mathcal {E}_{S}$
and
$\mathcal {E}_{S}$
and
![]() $\mathcal {E}$
, respectively.
$\mathcal {E}$
, respectively.
Proof Let P be a nonzero nonmaximal prime ideal of
![]() $\mathcal {E}$
of height greater than one contained in a maximal ideal M of
$\mathcal {E}$
of height greater than one contained in a maximal ideal M of
![]() $\mathcal {E}$
. Then, by Proposition 5,
$\mathcal {E}$
. Then, by Proposition 5,
![]() $P_{v_{\mathcal {E}}}=\mathcal {E}$
, where
$P_{v_{\mathcal {E}}}=\mathcal {E}$
, where
![]() $ v_{\mathcal {E}}$
denotes the v-operation on
$ v_{\mathcal {E}}$
denotes the v-operation on
![]() $\mathcal {E}$
. On the other hand, in
$\mathcal {E}$
. On the other hand, in
![]() $\mathcal {E}_{M}$
, which is a valuation ring, we have
$\mathcal {E}_{M}$
, which is a valuation ring, we have
![]() $P\mathcal {E} _{M} $
a nonmaximal prime of
$P\mathcal {E} _{M} $
a nonmaximal prime of
![]() $\mathcal {E}_{M}$
and so must be divisorial being the intersection of all the principal ideals containing
$\mathcal {E}_{M}$
and so must be divisorial being the intersection of all the principal ideals containing
![]() $P\mathcal {E} _{M}$
. Thus, if
$P\mathcal {E} _{M}$
. Thus, if
![]() $v^{\prime }$
is the v-operation on
$v^{\prime }$
is the v-operation on
![]() $\mathcal {E}_{M},$
then
$\mathcal {E}_{M},$
then
![]() $(P\mathcal {E}_{M})_{v^{\prime }}=P\mathcal {E}_{M}\subsetneq \mathcal {E} _{M}=(P_{v_{\mathcal {E}}}E_{M})_{v^{\prime }}$
.▪
$(P\mathcal {E}_{M})_{v^{\prime }}=P\mathcal {E}_{M}\subsetneq \mathcal {E} _{M}=(P_{v_{\mathcal {E}}}E_{M})_{v^{\prime }}$
.▪
We now establish that it is the DC property going missing in localization that is responsible for Corollary 7. For this, note that a DCD that is also a
![]() $\ast $
-domain is a v-G-Dedekind domain. Now, the
$\ast $
-domain is a v-G-Dedekind domain. Now, the
![]() $\ast $
-property, as indicated in [Reference Zafrullah26], fares nicely under localization, and so if the v-G-Dedekind property goes missing in localizing, it is the DC property that goes missing in localizing. However, the situation can be brought under control, once we introduce some restriction.
$\ast $
-property, as indicated in [Reference Zafrullah26], fares nicely under localization, and so if the v-G-Dedekind property goes missing in localizing, it is the DC property that goes missing in localizing. However, the situation can be brought under control, once we introduce some restriction.
Proposition 6 If R is DC such that for each ideal
![]() $A\in F(R)$
there is a finitely generated
$A\in F(R)$
there is a finitely generated
![]() $B\subseteq A$
with
$B\subseteq A$
with
![]() $A_{v}=B_{v}$
, then for each multiplicative set S, the ring
$A_{v}=B_{v}$
, then for each multiplicative set S, the ring
![]() $R_{S}$
is DC.
$R_{S}$
is DC.
Proof Note that a domain R with the given property is DC because for every ideal
![]() $A\in F(R)$
we have a finitely generated ideal
$A\in F(R)$
we have a finitely generated ideal
![]() $B\subseteq A$
with
$B\subseteq A$
with
![]() $ A_{v}=B_{v}$
, thus making
$ A_{v}=B_{v}$
, thus making
![]() $A_{v}$
of finite type, for each
$A_{v}$
of finite type, for each
![]() $A\in F(R)$
. On the other hand, a domain with the given property is known to be a Mori domain ([Reference Zafrullah27] and the references therein) and a Mori domain stays a Mori domain under localization. We include the proof below.
$A\in F(R)$
. On the other hand, a domain with the given property is known to be a Mori domain ([Reference Zafrullah27] and the references therein) and a Mori domain stays a Mori domain under localization. We include the proof below.
Let S be a multiplicative set in R such that for each
![]() $A\in F(R)$
there is a finitely generated
$A\in F(R)$
there is a finitely generated
![]() $B\subseteq A$
with
$B\subseteq A$
with
![]() $A_{v}=B_{v}$
, and let
$A_{v}=B_{v}$
, and let
![]() $\mathcal { \alpha }$
be an ideal of
$\mathcal { \alpha }$
be an ideal of
![]() $R_{S}.$
Then
$R_{S}.$
Then
![]() $\alpha =AR_{S}$
where
$\alpha =AR_{S}$
where
![]() $A=\alpha \cap R.$
Let
$A=\alpha \cap R.$
Let
![]() $B\subseteq A$
where B is finitely generated such that
$B\subseteq A$
where B is finitely generated such that
![]() $ A_{v}=B_{v} $
. Note that
$ A_{v}=B_{v} $
. Note that
![]() $A\subseteq B_{v}$
and so
$A\subseteq B_{v}$
and so
![]() $\alpha =AR_{S}\subseteq B_{v}R_{S}$
. Thus,
$\alpha =AR_{S}\subseteq B_{v}R_{S}$
. Thus,
![]() $\alpha _{v}=(AR_{S})_{v}\subseteq (B_{v}R_{S})_{v}=(BR_{S})_{v}$
, since B is finitely generated [Reference Zafrullah24]. Since
$\alpha _{v}=(AR_{S})_{v}\subseteq (B_{v}R_{S})_{v}=(BR_{S})_{v}$
, since B is finitely generated [Reference Zafrullah24]. Since
![]() $B\subseteq A$
, we have
$B\subseteq A$
, we have
![]() $BR_{S}\subseteq AR_{S}$
and so
$BR_{S}\subseteq AR_{S}$
and so
![]() $ (BR_{S})_{v}\subseteq (AR_{S})_{v}$
. Thus,
$ (BR_{S})_{v}\subseteq (AR_{S})_{v}$
. Thus,
![]() $(BR_{S})_{v}=(AR_{S})_{v}$
with
$(BR_{S})_{v}=(AR_{S})_{v}$
with
![]() $ BR_{S}$
finitely generated and contained in
$ BR_{S}$
finitely generated and contained in
![]() $AR_{S}$
.▪
$AR_{S}$
.▪
Because, in a DCD R,
![]() $A_{v}$
is a finite intersection of principal fractional ideals, we conclude that
$A_{v}$
is a finite intersection of principal fractional ideals, we conclude that
![]() $A_{v}R_{S}$
is divisorial. Thus,
$A_{v}R_{S}$
is divisorial. Thus,
![]() $ (AR_{S})_{v}\subseteq A_{v}R_{S}.$
However, as Corollary 7 indicates, the inclusion may be strict on occasion. Now, generally, if
$ (AR_{S})_{v}\subseteq A_{v}R_{S}.$
However, as Corollary 7 indicates, the inclusion may be strict on occasion. Now, generally, if
![]() $A\in F(R)$
is finitely generated and S is a multiplicative set of R, then
$A\in F(R)$
is finitely generated and S is a multiplicative set of R, then
![]() $ (AR_{S})_{v^{\prime }}=(A_{v_{R}}R_{S})_{v^{\prime }}$
, where
$ (AR_{S})_{v^{\prime }}=(A_{v_{R}}R_{S})_{v^{\prime }}$
, where
![]() $v^{\prime }$
and
$v^{\prime }$
and
![]() $v_{R}$
are v-operations on
$v_{R}$
are v-operations on
![]() $R_{S}$
and R, respectively [Reference Zafrullah24]. This is a general formula, and so it works for DCDs too, but in a slightly modified form.
$R_{S}$
and R, respectively [Reference Zafrullah24]. This is a general formula, and so it works for DCDs too, but in a slightly modified form.
Proposition 7 If
![]() $A\in F(R)$
is nonzero finitely generated, S is a multiplicative set of R, and R is DC, then
$A\in F(R)$
is nonzero finitely generated, S is a multiplicative set of R, and R is DC, then
![]() $(AR_{S})_{v^{\prime }}=A_{v_{R}}R_{S},$
where
$(AR_{S})_{v^{\prime }}=A_{v_{R}}R_{S},$
where
![]() $v^{\prime }$
and
$v^{\prime }$
and
![]() $v_{R}$
are v-operations on
$v_{R}$
are v-operations on
![]() $ R_{S}$
and R, respectively.
$ R_{S}$
and R, respectively.
Proof The proof follows from the fact that
![]() $A_{v_{R}}$
is a finite intersection of principal fractional ideals, and so
$A_{v_{R}}$
is a finite intersection of principal fractional ideals, and so
![]() $A_{v_{R}}R_{S}$
is a divisorial ideal of
$A_{v_{R}}R_{S}$
is a divisorial ideal of
![]() $R_{S}$
and that makes
$R_{S}$
and that makes
![]() $A_{v_{R}}R_{S}=(A_{v_{R}}R_{S})_{v^{\prime }}$
.▪
$A_{v_{R}}R_{S}=(A_{v_{R}}R_{S})_{v^{\prime }}$
.▪
A prime example of a DCD is a Mori domain, and Roitman [Reference Roitman22] has produced an example of a Mori domain R such that
![]() $R[X]$
is not Mori. On the other hand, for integrally closed integral domains, Querre [Reference Querre21] proved the following result.
$R[X]$
is not Mori. On the other hand, for integrally closed integral domains, Querre [Reference Querre21] proved the following result.
Theorem 4.3 An integral domain R is integrally closed if and only if, for every integral ideal B of
![]() $R[X]$
with
$R[X]$
with
![]() $B\cap R\neq 0$
, we have
$B\cap R\neq 0$
, we have
![]() $ B_{v}=(A_{B}[X])_{v}=(A_{B})_{v}[X]$
where
$ B_{v}=(A_{B}[X])_{v}=(A_{B})_{v}[X]$
where
![]() $A_{B}$
is the ideal of R generated by the coefficients of elements of B.
$A_{B}$
is the ideal of R generated by the coefficients of elements of B.
For a clearer treatment of Theorem 4.3, see Section 3 of [Reference Anderson, Kwak and Zafrullah7]. For now, we use this theorem to prove the following result.
Theorem 4.4 Let R be an integrally closed integral domain. The polynomial ring
![]() $R[X]$
is DC if and only if R is.
$R[X]$
is DC if and only if R is.
Proof Suppose that R is DC. Then, for every ideal
![]() $I\in F(R)$
of R, we have
$I\in F(R)$
of R, we have
![]() $ I_{v}=J_{v}$
where J is finitely generated. Now, let
$ I_{v}=J_{v}$
where J is finitely generated. Now, let
![]() $H\in F(R[X])$
. Because R is integrally closed, according to Theorem 2.1 of [Reference Anderson, Kwak and Zafrullah7],
$H\in F(R[X])$
. Because R is integrally closed, according to Theorem 2.1 of [Reference Anderson, Kwak and Zafrullah7],
 $H= \frac {f(X)}{g(X)}B$
where
$H= \frac {f(X)}{g(X)}B$
where
![]() $f(X),g(X)\in R[X]$
and B is an ideal of
$f(X),g(X)\in R[X]$
and B is an ideal of
![]() $R[X]$
with
$R[X]$
with
![]() $B\cap R\neq (0)$
. But then,
$B\cap R\neq (0)$
. But then,
 $H_{v}=\frac {f(X)}{g(X)}B_{v}=\frac {f(X)}{ g(X)}((A_{B})_{v}[X])$
is a v-ideal of finite type because, as
$H_{v}=\frac {f(X)}{g(X)}B_{v}=\frac {f(X)}{ g(X)}((A_{B})_{v}[X])$
is a v-ideal of finite type because, as
![]() $A_{B}$
is an integral ideal of R,
$A_{B}$
is an integral ideal of R,
![]() $(A_{B})_{v}$
is a v-ideal of finite type. Conversely, let I be a nonzero integral ideal of R, and suppose that
$(A_{B})_{v}$
is a v-ideal of finite type. Conversely, let I be a nonzero integral ideal of R, and suppose that
![]() $R[X]$
is DC. Then
$R[X]$
is DC. Then
![]() $(I[X])_{v}=I_{v}[X]$
is a v-ideal of finite type, and this forces
$(I[X])_{v}=I_{v}[X]$
is a v-ideal of finite type, and this forces
![]() $I_{v}$
to be a v-ideal of finite type.▪
$I_{v}$
to be a v-ideal of finite type.▪
Acknowledgment
There were a lot of typos and ambiguities in the original version, as usual. I am indebted to Professor Anthony Bevelacqua for pointing them out to me. Hopefully, the paper is a better read now. I am grateful to Professor Evan Houston for looking into the paper and for his advice on precedence. I also thank the Referee for some valuable advice on presentation. I am thankful to my friends who made me rise from the dead.