We consider a variant of the game of Cops and Robbers, called Lazy Cops and Robbers, where at most one cop can move in any round. We investigate the analogue of the cop number for this game, which we call the lazy cop number. Lazy Cops and Robbers was recently introduced by Offner and Ojakian, who provided asymptotic upper and lower bounds on the lazy cop number of the hypercube. By coupling the probabilistic method with a potential function argument, we improve on the existing lower bounds for the lazy cop number of hypercubes.

Estimating numerically the spectral radius of a random walk on a non-amenable graph is complicated, since the cardinality of balls grows exponentially fast with the radius. We propose an algorithm to get a bound from below for this spectral radius in Cayley graphs with finitely many cone types (including for instance hyperbolic groups). In the genus 2 surface group, it improves by an order of magnitude the previous best bound, due to Bartholdi.

Over 50 years ago, Erdős and Gallai conjectured that the edges of every graph on n vertices can be decomposed into O(n) cycles and edges. Among other results, Conlon, Fox and Sudakov recently proved that this holds for the random graph G(n, p) with probability approaching 1 as n → ∞. In this paper we show that for most edge probabilities G(n, p) can be decomposed into a union of n/4 + np/2 + o(n) cycles and edges w.h.p. This result is asymptotically tight.

We say that a (di)graph G has a perfect H-packing if there exists a set of vertex-disjoint copies of H which cover all the vertices in G. The seminal Hajnal–Szemerédi theorem characterizes the minimum degree that ensures a graph G contains a perfect Kr-packing. In this paper we prove the following analogue for directed graphs: Suppose that T is a tournament on r vertices and G is a digraph of sufficiently large order n where r divides n. If G has minimum in- and outdegree at least (1−1/r)n then G contains a perfect T-packing.

In the case when T is a cyclic triangle, this result verifies a recent conjecture of Czygrinow, Kierstead and Molla [4] (for large digraphs). Furthermore, in the case when T is transitive we conjecture that it suffices for every vertex in G to have sufficiently large indegree or outdegree. We prove this conjecture for transitive triangles and asymptotically for all r ⩾ 3. Our approach makes use of a result of Keevash and Mycroft [10] concerning almost perfect matchings in hypergraphs as well as the Directed Graph Removal Lemma [1, 6].

In this paper we provide two results. The first one consists of an infinitary version of the Furstenberg–Weiss theorem. More precisely we show that every subset A of a homogeneous tree T such that

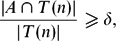$\frac{|A\cap T(n)|}{|T(n)|}\geqslant\delta,$

The second result is the following. For every sequence (mq)q∈ℕ of positive integers and for every real 0 < δ ⩽ 1, there exists a sequence (nq)q∈ℕ of positive integers such that for every D ⊆ ∪k ∏q=0k-1[nq] satisfying

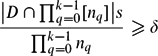$\frac{\big|D\cap \prod_{q=0}^{k-1} [n_q]\big|s}{\prod_{q=0}^{k-1}n_q}\geqslant\delta$