Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-23T00:49:28.306Z Has data issue: false hasContentIssue false
  • English
  • Français

Gravitational-Like Lens Based on Graphene Ripple

Published online by Cambridge University Press:  26 August 2015

Daqing Liu ,
Shuyue Chen ,
Ning Ma ,
Xiang Zhao  and
Zhuo Xu
Daqing Liu*
Affiliation:
School of Mathematics and Physics, Changzhou University, Changzhou 213164, China School of Science, Xi’an Jiaotong University, Xi’an 710049, China
Shuyue Chen
Affiliation:
School of Mathematics and Physics, Changzhou University, Changzhou 213164, China
Ning Ma
Affiliation:
Department of Physics, Taiyuan University of Technology, Taiyuan 030024, China School of Science, Xi’an Jiaotong University, Xi’an 710049, China
Xiang Zhao
Affiliation:
School of Science, Xi’an Jiaotong University, Xi’an 710049, China
Zhuo Xu
Affiliation:
Electronic Materials Research Laboratory, Xi’an Jiaotong University, Xi’an 710049, China
*
*Corresponding author.[email protected]
Get access

Abstract

We conducted a semiclassical study on carrier movement in curved graphene. A previous attempt was made to show that curved graphene is a readily available and cheap laboratory material used to study general relativity effects, especially if the electron energies satisfy 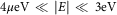${\rm 4}\:\mu {\rm eV}\,\ll\,\left| E \right|\,\ll\,{\rm 3}\:{\rm eV}$ . Furthermore, a gravitational-like lens can be constructed based on a special graphene ripple; this lens has neither chromatic nor cometic aberration. One can design an ideal electron lens using a graphene ripple.

Keywords

electron lensgravitational-like lenscometic aberrationgrapheneripple
Type
Equipment and Software Development
Information
Microscopy and Microanalysis , Volume 21 , Issue 5 , October 2015 , pp. 1207 - 1213
Copyright
© Microscopy Society of America 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahmedov, H. & Aliev, A.N. (2001). Exact solutions in 3D new massive gravity. Phys Rev Lett 106, 021301.Google Scholar
Banados, M., Teitelboim, C. & Zanelli, J. (1992). The black hole in three dimensional space time. Phys Rev Lett 69, 1849–1851.Google Scholar
Bao, W., Miao, F., Chen, Z., Zhang, H., Jang, W., Dames, C. & Lau, C.N. (2009). Controlled ripple texturing of suspended graphene and ultrathin graphite membranes. Nat Nanotechnol 4, 562–566.Google Scholar
Berry, M. (1976). Waves and Thom’s theorem. Adv Phy 25, 1–26.Google Scholar
Calmet, X. & Landsberg, G. (2012). Lower dimensional quantum black holes. In Black Holes: Evolution, Theory and Thermodynamics, Bauer, A.J. & Eiffel, D.G. (Eds.), pp. 157–202). USA: Nova Pub.Google Scholar
Cheianov, V.V., Fal’ko, V. & Altshuler, B.L. (2007). The focusing of electron flow and a Veselago lens in graphene p-n junctions. Science 315, 1252–1255.Google Scholar
Crassee, I., Levallois, J., Walter, A.L., Ostler, M., Bostwick, A., Rotenberg, E., Seyller, T., Marel, D.V.D. & Kuzmenko, A.B. (2010). Giant Faraday rotation in single- and multilayer graphene. Nat Phys 7, 48–51.Google Scholar
Cserti, J., P’alyi, A. & P’eterfalvi, C. (2007). Caustics due to a negative refractive index in circular graphene p-n junctions. Phys Rev lett 99, 246801.Google Scholar
Darabi, F., Atazadeh, K. & Rezaei-Aghdam, A. (2013). Generalized (2+1) dimensional black hole by Noether symmetry. Eur Phys J C 73, 2657–2663.Google Scholar
Deser, S., Jackiw, R. & ‘t Hooft, G. (1984). Three-dimensional Einstein gravity: Dynamics of flat space. Ann Phys 152, 220–235.Google Scholar
Emtsev, K.V., Bostwick, A., Horn, K., Jobst, J., Kellogg, G.L. & Ley, L. (2009). Towards wafer-size graphene layers by atmospheric pressure graphitization of silicon carbide. Nat Mater 8, 203–207.Google Scholar
Gonz’alez, J., Guinea, F. & Herrero, J. (2009). Propagating, evanescent, and localized states in carbon nanotube-graphene junctions. Phys Rev B 79, 165434.Google Scholar
Iorio, A. (2011). Weyl-gauge symmetry of graphene. Ann Phys 326, 1334–1353.Google Scholar
Iorio, A. (2012). Using Weyl symmetry to make graphene a real lab for fundamental physics. Eur Phys J Plus 127, 156–166.Google Scholar
Iorio, A. (2013). Graphene: QFT in curved spacetimes close to experiments. J Phys Conf Ser 442, 012056.Google Scholar
Iorio, A. & Lambiase, G. (2012). The Hawking-Unruh phenomenon on graphene. Phys Lett B 716, 334–337.Google Scholar
Iorio, A. & Lambiase, G. (2014). Quantum field theory in curved graphene spacetimes, Lobachevsky geometry, Weyl symmetry, Hawking effect, and all that. Phys Rev D 90, 025006.Google Scholar
Juan, F.D., Cortijo, A. & Vozmediano, M.A.H. (2007). Charge inhomogeneities due to smooth ripples in graphene sheets. Phys Rev B 76, 165409.Google Scholar
Juan, F.D., Sturla, M. & Vozmediano, M.A.H. (2012). Space dependent Fermi velocity in strained graphene. Phys Rev Lett 108, 227205.Google Scholar
Lee, Y., Bae, S., Jang, H., Jang, S., Zhu, S., Sim, S.H., Song, Y. II., Hong, B.H. & Ahn, J.H. (2010). Wafer-scale synthesis and transfer of graphene films. Nano Lett 10, 490–493.Google Scholar
Lemos, J.P.S. (1995). Cylindrical black hole in general relativity. Phys Lett B 353, 46–51.Google Scholar
Liu, D., Xu, Z., Ma, N. & Zhang, S. (2012 a). Graphene modulated by external fields: A nonresonant left-handed metamaterial. Appl Phys A 106, 949–954.Google Scholar
Liu, D., Zhang, S., Ma, N. & Li, X. (2012 b). Faraday rotation effect in periodic graphene structure. J Appl Phys 112, 023115.Google Scholar
Liu, D., Zhang, S., Zhang, E., Ma, N. & Chen, H. (2010). Anomalous valley magnetic moment of graphene. Europhys Lett 89, 37002.Google Scholar
Neto, A.H.C., Guinea, F., Peres, N.M.R., Novoselov, K.S. & Geim, A.K. (2009). The electronic properties of graphene. Rev Mod Phys 81, 109–162.Google Scholar
Novoselov, K.S., Geim, A.K., Morozov, S.V., Jiang, D., Zhang, Y., Dubonos, S.V., Grigorieva, I.V. & Firsov, A.A. (2004). Electric field effect in atomically thin carbon films. Science 306, 666–669.Google Scholar
Vozmediano, M.A.H., Katsnelson, M.I. & Guinea, F. (2010). Gauge fields in graphene. Phys Rep 496, 109–148.Google Scholar
Wallace, P. (1947). The band structure of graphite. Phys Rev 71, 622–639.Google Scholar
Weinberg, S. (1972). Gravitation and Cosmology: Principle and Applications of the General Theory of Relativity. USA: John Wiley and Sons, Inc. Pub.Google Scholar
Witten, E. (1988). 2+1 gravity as an exactly soluble system. Nucl Phys B 311, 46–78.Google Scholar
Zwierzycki, M. (2014). Transport properties of rippled graphene. J Phys Cond Mater 26, 135303.Google Scholar